A theoretically-modified method for calculating the volumetric stresses in passive confined pressure SHPB tests of granular materials
-
摘要: SHPB被动围压试验为探究散体介质在爆炸和冲击荷载作用下的力学行为提供了一个行之有效的方法。针对相关试验设计和计算中存在的弊端和不足,借助经典板壳理论将SHPB被动围压试验中用于约束散体介质的刚性套筒简化为受均匀带状内压作用的圆柱形壳体。理论计算了套筒径向位移、环向应变与均匀带状内压及套筒几何、力学参数的关系,得到了套筒径向位移、环向应变沿其轴向的分布规律;分析了套筒长度、厚度、内外径以及均匀带状内压宽度之间等无量纲几何参数对计算结果的影响;将理论计算结果与试验和数值模拟结果进行对比,验证了理论计算结果的正确性。本文中提出的理论修正方法可为指导散体介质SHPB被动围压试验提供参考。Abstract: The passive confined pressure SHPB (split Hopkinson pressure bar) test provides an efficient way to study the mechanical behaviors of granular materials at high strain rates (102 − 104 s−1) under the action of explosion and shock wave. In this study, aiming to overcome the disadvantages and demerits of design and calculations in the original passive confined pressure SHPB tests provided in previous works, which has been widely used to investigate the dynamical behaviors of granular materials, a rigid steel sleeve used to restrict the granular specimen is simplified as a circular cylindrical shell, instead of a thick-walled cylinder, subjected to band inner pressure. By using classical shell theory, the theoretical expressions are formulated for radial displacement and hoop strain of the steel sleeve, which contain the mechanical and geometrical parameters of inner pressure and rigid steel sleeve. The distributions of radial displacement and hoop strain along with the steel sleeve are also obtained, while the effects of dimensionless parameters (such as length, thickness, radius of steel sleeve and the width of inner pressure) on the calculation results are studied. Compared with the thick-walled cylinder theory, a modified coefficient k is proposed for estimating the stress states in granular specimens more accurately. To check the feasibility and validate the proposed modified theoretical method, the results derived from shell theory and hollow cylinder theory are compared with experimental and numerical results. It is noted that the shell theory gives better approximations than hollow cylinder theory, especially when d/L is much smaller than one; when the value of d/L approaches to one, the accuracy of two theories is both high, which could be accepted in engineering practices. Therefore, it is concluded that the modified theoretical method proposed in this study for estimating the stress state of specimen can serve as a reference in the passive confined pressure SHPB tests for granular materials.
-
Key words:
- granular materials /
- SHPB tests /
- circular cylindrical shell /
- correction factor
-
散体介质作为消波耗能材料广泛地应用于土木工程以及军事工程等领域,其在高应变率下的动态物理力学性质对于研究爆炸冲击荷载作用下结构的防灾减灾问题具有重要意义。然而,关于中高应变率(102~104 s−1)下散体材料是否具有应变率效应,现有研究尚存在严重分歧。Yamamuro等[1]发现干砂的应力-应变曲线在不同应变率(10−2~103 s−1)下存在明显差别,具有显著的应变率效应。Luo等[2-4]的试验结果却显示,不同应变率下散体材料的应力-应变曲线基本相同,未发现明显的应变率效应。造成上述差异的原因可能在于,约束条件选取的不同,具体原因尚有待进一步研究。而且,随着我国南部海域岛礁建设的蓬勃发展,散体介质中-高应变率下的动态力学性质研究的应用前景越来越广阔[5-6]。
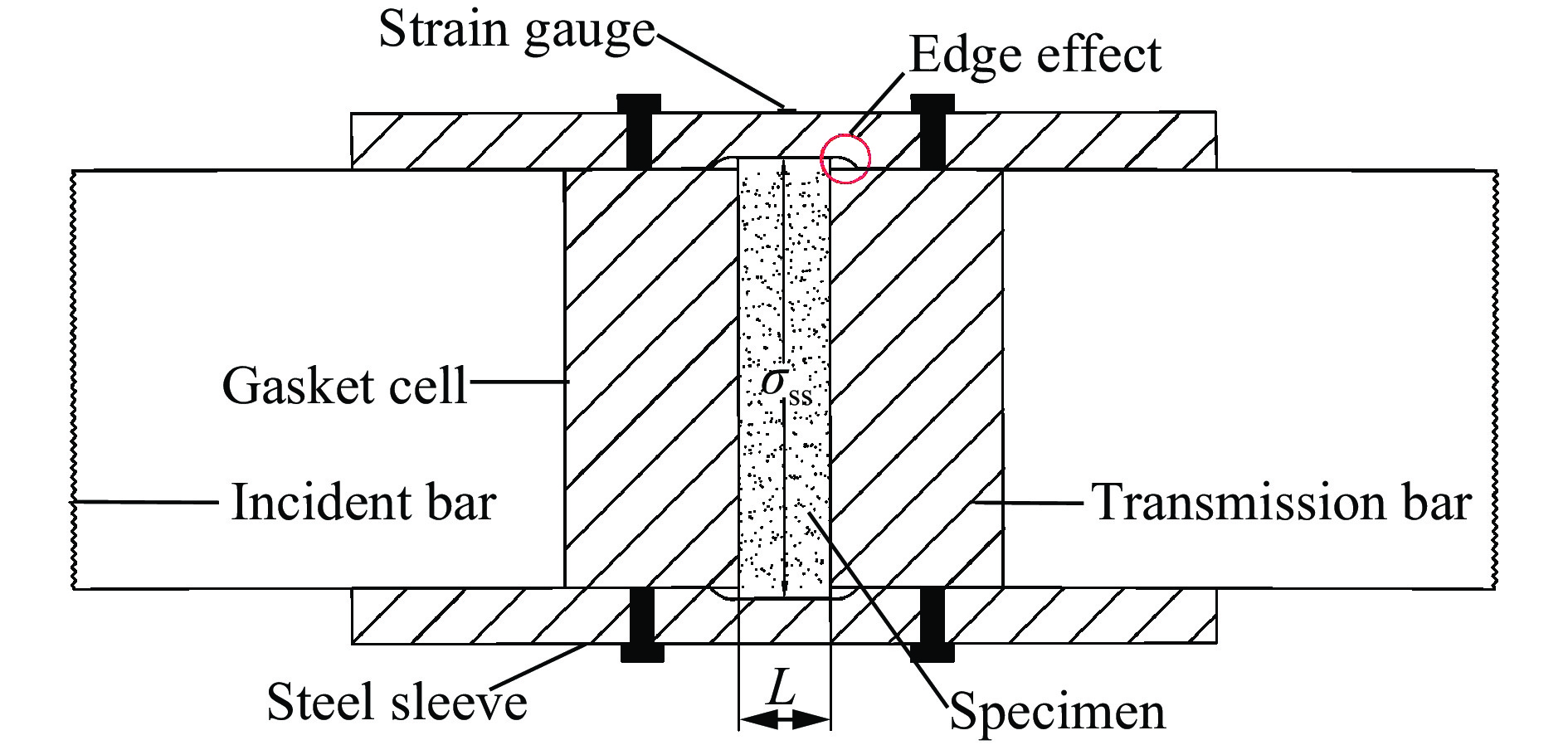
分离式霍普金森压杆(split Hopkinson pressure bar, SHPB)是研究材料中-高应变率下动态力学性质的重要试验手段[7-8]。相较于固体材料,散体介质SHPB试验过程中需要使用刚性套筒固定散体材料试样。通过测量套筒外壁的环向应变,根据厚壁圆筒理论(平面应力状态)即可反推出散体介质试样中的应力与变形场[2-3, 9]。需要指出的是,散体材料的波阻抗较低,为了保证整个试验过程中试样内部的应力平衡,试样的长度通常较短(一般不超过10 mm)。由于套筒的约束使得散体介质试样边缘处产生了显著的端部效应(如图1所示),此时套筒不能满足平面应力假设,因此文献[2-3, 9]中得到的结果并不准确,需要进行修正。魏久淇等[4]指出上述文献中计算存在的缺陷,尝试通过数值模拟手段进行修正并与试验结果对比,验证了数值修正方法的正确性,但受限于数值模拟方法自身的缺陷,他们未能给出修正系数的无量纲形式,导致数值修正方法的适用范围有限。
受魏久淇等[4]研究的启发,本文中借助经典板壳理论,将刚性套筒简化为受均匀带状内压作用的圆柱形壳体,推导套筒径向位移、环向应变与带状内压及套筒几何、力学参数的关系,得到套筒径向位移、环向应变沿其轴向的分布;分析套筒长度、厚度、内外径以及带状内压宽度之间等无量纲几何参数对计算结果的影响;将理论计算结果与试验结果和数值模拟结果进行对比,验证理论计算的正确性。
1. 厚壁圆筒理论计算试样中的应力场
为了探究聚合物材料在大变形条件下的非弹性力学行为,Ma等[10]采用厚壁圆筒理论对试样中的应力场进行了估算,后续的散体介质SHPB被动围压试验研究大多采用了相同的计算方法[2-4,9,11]。
假设散体试样与套筒之间的径向应力满足连续性条件,在极坐标下,试样内部的应力和变形分别为:
σr=σθ=0.5(α2−1)Ecεc (1) εr=εθ=0.5εc[(1−νc)+(1+νc)α2] (2) 式中:
σr 、σθ 分别为试样的径向和环向应力,α为套筒外、内径之比,Ec为套筒弹性模量,εc 为套筒外壁的环向应变,εr 、εθ 分别为试样的径向和环向应变,νc 为套筒泊松比。由式(1)~(2)可知,试样的静水压力
σm 和体积应变εV 分别为:σm=13(σr+σθ+σz)=13(σz + 2σr) (3) εV=εr+εθ+εz=εz+2εr (4) 考虑到试样轴向应变
εz 远大于径向εr 和环向应变εθ ,即εz≫εr=εθ ,于是试样的体积应变可化简为:εV=εr+εθ+εz≈εz (5) 通过测量套筒外壁环向应变
εc ,并结合式(1)~(5),即可得到散体介质试样的应力与变形状态。需要注意的是,上述理论推导过程中假定套筒处于平面应力状态,但由于端部效应的存在,套筒受力并不能简化为平面应力问题,因此计算需要修正。2. 圆柱壳体理论计算试样中的应力场
为了便于环向应变
εc 的量测以及试件装样,在进行散体介质SHPB试验时通常选用壁厚较薄的刚性套筒。试验过程中试样内部始终处于应力平衡状态,因此可将试样与套筒间的相互作用力按静力处理。此时,套筒可简化为受均匀带状内压作用的圆柱形壳体,如图2所示。图2中,套筒长度为2L,均匀内压强度q(x) 作用长度为2d,取极坐标(r, φ, x)。由经典弹性板壳理论可知[12-13],忽略轴向变形,均匀内压作用下套筒的平衡方程为:
D∇2(∇2W(x))+12h2Da2W(x)=q(x) (6) 式中:
D=Ech312(1−ν2c) 为壳体抗弯刚度,∇2=∂2∂x2 为拉普拉斯算符,h为套筒厚度,a为套筒内半径,W(x) 为套筒径向位移,q(x) 为套筒内压。对均匀内压
q(x) 进行Fourier级数展开,可得:q(x)=q(dL+2π ∞∑n=11nsinnπ dLcosnπ xL) (7) 将式(6)对坐标x进行Fourier变换并化简,可得:
s4¯W(x)+12h21a2¯W(x)=¯q(x)D (8) 式中:s为Fourier变换参数,
¯W(x) 为位移函数W(x) 的Fourier变换,¯q(x) 为荷载函数q(x) 的Fourier变换。对式(8)进行Fourier逆变化,可得套筒径向位移函数
W(x) :W(x)=q(1−ν2c)Eca2h[dL+2π ∞∑n=11nsin(nπ dL)cos(nπ xL)1+n4π 412(hL)2(aL)2] (9) 此时,套筒环向应变函数
εθ(x) :εθ(x)=W(x)a=q(1−ν2)Ecah[dL+2π ∞∑n=11nsin(nπ dL)cos(nπ xL)1+n4π 412(hL)2(aL)2] (10) 在x=0处,套筒环向应变函数
ε(0)θ 为:ε(0)θ=q(1−ν2c)Ecah[dL+2π ∞∑n=11nsin(nπ dL)1+n4π 412(hL)2(aL)2]=2kq(1−α2)Ec (11) 式中:k为修正系数。k的表达式为:
k=b+a2a(1−ν2c)[dL+2π ∞∑n=11nsin(nπ dL)1+n4π 412(hL)2(aL)2] (12) 为了与厚壁圆筒理论计算结果进行对比,对式(1)进行如下变换:
εc=2σr(1−α2)Ec=2q(1−α2)Ec (13) 比较式(10)和式(13)可以发现,厚壁圆筒理论与圆柱壳体理论计算结果的主要区别在于修正系数k的取值,且
k=ε(0)θ/ε(0)θεcεc 。当带状内压宽度2d等于套筒长度2L时,修正系数k=1,此时圆柱壳体理论的计算结果退化为厚壁圆筒理论结果。根据修正系数k的计算结果可以发现,系数k为无量纲参数b/a、d/L、a/L 的函数。分析式(12)可知:(1)随着b/baa 的增大,系数k增大;(2)随着d/dLL 的增大,k先增大后减小;(3)随着a/aLL 的增大,k减小。3. 数值模拟与理论对比
为了验证本文计算模型的正确性,采用文献[4]中试验参数并与其数值模拟结果进行对比,数值模拟过程中参数选取为:套筒长度2L=94 mm,套筒厚度h=4.95 mm,套筒内半径a=18.55 mm,套筒外半径b=23.5 mm,套筒弹性模量Ec=210 GPa,泊松比νc=0.3;带状内压宽度2d=7, 8, 9, 10, 12, 15, 20, 30, 40, 50, 60, 70, 80, 90, 94 mm。
将上述计算参数代入式(10),可求得环向应变沿套筒长度方向的分布曲线,如图3所示。由图3发现,随着内压宽度d的增大,环向应变的分布范围扩大、幅值先增大后减小。当带状内压宽度2d=10 mm(散体介质试样的长度极限)时,环向应变的分布范围约为套筒长度的1/3,此时柱壳理论计算结果约为厚壁圆筒理论结果的0.5倍,表明厚壁圆筒理论计算结果与实际变形相差较大;当2d≈40 mm时,柱壳理论计算结果达到最大值,约为厚壁圆筒理论结果的1.06倍;当2d=2L时,柱壳退化为厚壁圆筒。
将文献[4]中数值计算得到的修正系数k与圆柱壳体理论计算结果进行对比,如图4所示。由图4可知,当d/L≤0.25时,壳体理论计算结果与数值计算结果基本重合,而与厚壁圆筒理论计算结果则相差较远;当0.25<d/L≤0.625时,壳体理论计算结果略高于数值和厚壁圆筒理论计算结果,最大误差为6%;当d/L>0.625时,理论计算结果和数值计算结果基本相同,此时2种理论精度都可满足试验要求。
为了进一步验证壳体理论计算结果的准确性,并分析套筒长度、内外径以及均匀带状内压宽度等几何参数对计算结果的影响,本文中在文献[4]中数值计算的基础上,利用Abaqus/Explicit有限元模拟软件进行补充计算,模型网格采用六面体线性缩减积分单元,边界条件为自由边界,不同计算模型网格划分示意图如图5所示。
算例1:套筒长度2L=94 mm,套筒厚度h=3 mm,套筒内半径a=19 mm,套筒外半径b=22 mm,套筒弹性模量Ec=210 GPa,泊松比νc=0.3;带状内压宽度2d=7, 8, 9, 10, 12, 15, 20, 30, 40, 50, 60, 70, 80, 90, 94 mm。此时,理论计算与数值计算对比结果如图6所示。
算例2:套筒长度2L=50 mm,套筒厚度h=4.95 mm,套筒内半径a=18.55 mm,套筒外半径b=23.5 mm,套筒弹性模量Ec=210 GPa,泊松比νc=0.3;带状内压宽度2d=7, 8, 9, 10, 12, 15, 20, 30, 40, 50 mm。此时,理论计算与数值计算对比结果如图7所示。
对比图6~7中理论与数值计算结果可以发现,随着无量纲参数
b/a、d/L、a/L 的改变,壳体理论的计算结果与数值计算结果非常接近,且精度远高于厚壁圆筒理论。4. 试验与理论对比
为了进一步验证壳体理论的准确性,将理论计算与试验结果进行对比,试验结果同样采用文献[4]中的数据,具体如图8所示。根据试验数据计算可知,含水质量分数ω为25%和30%的钙质砂试样SHPB试验对应的饱和点(饱和度Sr = 1)分别为εz=0.221 2和εz=0.157 4。当轴向应变εz超越饱和点之后,水压力将占据主导地位,此时散体试样处于静水压力状态,即σz =σr =σθ。
观察图8可以发现,当轴向应变在饱和点之前,理论计算的径向应力σr明显小于轴向应力σz试验值,这是由散体介质的侧向压力系数λ通常小于1引起的。根据试验和理论数据进行估算,可知λ≈0.548;当轴向应变超过饱和点之后,壳体理论(式(10))计算得到的径向应力σr与轴向应力σz的试验值基本重合,验证了壳体理论的准确性。而厚壁圆筒理论(式(1))的计算结果则明显低于试验值,表明厚壁圆筒理论的计算误差很大,此时并不适用。
5. 结 束 语
SHPB被动围压试验为探究散体介质在爆炸和冲击荷载作用下的力学行为提供了一个行之有效的方法。本文中针对相关试验设计和计算中存在的弊端和不足,借助经典板壳理论对现有计算方法进行修正,总结如下。
(1)将SHPB被动围压试验中用于约束散体介质的刚性套筒简化为受均匀带状内压作用的圆柱形壳体。推导了套筒径向位移、环向应变与均匀带状内压及套筒几何、力学参数的定量关系,得到了套筒径向位移、环向应变沿其轴向的分布。
(2)分析了套筒长度、厚度、内外径以及均匀带状内压宽度之间等无量纲几何参数对计算结果的影响,并与数值计算结果进行对比,验证了修正系数k的准确性。
(3)将理论结果与试验和数值模拟结果进行对比分析,验证了理论计算的正确性。
-
-
[1] YAMAMURO J A, ABRANTES A E, LADE P V. Effect of strain rate on the stress-strain behavior of sand [J]. Journal of Geotechnical and Geoenvironmental Engineering, 2011, 137(12): 1169–1178. DOI: 10.1061/(ASCE)GT.1943-5606.0000542. [2] LUO H, LU H, COOPER W L, et al. Effect of mass density on the compressive behavior of dry sand under confinement at high strain rates [J]. Experimental Mechanics, 2011, 51(9): 1499–1510. DOI: 10.1007/s11340-011-9475-2. [3] LUO H Y, COOPER W L, LU H B. Effects of particle size and moisture on the compressive behavior of dense Eglin sand under confinement at high strain rates [J]. International Journal of Impact Engineering, 2014, 65: 40–55. DOI: 10.1016/j.ijimpeng.2013.11.001. [4] 魏久淇, 张春晓, 曹少华, 等. 一种散体材料SHPB被动围压试验体应力修正方法 [J]. 爆炸与冲击, 2020, 40(12): 124201. DOI: 10.11883/bzycj-2019-0411.WEI J Q, ZHANG C X, CAO S H, et al. A volume stress correction method for SHPB passive confined pressure of granular materials [J]. Explosion and Shock Waves, 2020, 40(12): 124201. DOI: 10.11883/bzycj-2019-0411. [5] 赵章泳, 邱艳宇, 紫民, 等. 含水率对非饱和钙质砂动力特性影响的试验研究 [J]. 爆炸与冲击, 2020, 40(2): 023102. DOI: 10.11883/bzycj-2019-0066.ZHAO Z Y, QIU Y Y, ZI M, et al. Experimental study on dynamic compression of unsaturated calcareous sand [J]. Explosion and Shock Waves, 2020, 40(2): 023102. DOI: 10.11883/bzycj-2019-0066. [6] 文祝, 邱艳宇, 紫民, 等. 钙质砂的准一维应变压缩试验研究 [J]. 爆炸与冲击, 2019, 39(3): 033101. DOI: 10.11883/bzycj-2018-0015.WEN Z, QIU Y Y, ZI M, et al. Experimental study on quasi-one-dimensional strain compression of calcareous sand [J]. Explosion and Shock Waves, 2019, 39(3): 033101. DOI: 10.11883/bzycj-2018-0015. [7] HOPKINSON B. A method of measuring the pressure produced in the detonation of high explosives or by the impact of bullets [J]. Proceedings of the Royal Society of London: Series A, 1914, 89(612): 411–413. DOI: 10.1098/rspa.1914.0008. [8] KOLSKY H. An investigation of the mechanical properties of materials at very high rates of loading [J]. Proceedings of the Physical Society: Section B, 1949, 62(11): 676–700. DOI: 10.1088/0370-1301/62/11/302. [9] BRAGOV A M, LOMUNOV A K, SERGEICHEV I V, et al. Determination of physicomechanical properties of soft soils from medium to high strain rates [J]. International Journal of Impact Engineering, 2008, 35(9): 967–976. DOI: 10.1016/j.ijimpeng.2007.07.004. [10] MA Z, RAVI-CHANDAR K. Confined compression: a stable homogeneous deformation for constitutive characterization [J]. Experimental Mechanics, 2000, 40(1): 38–45. DOI: 10.1007/BF02327546. [11] SONG B, CHEN W N, LUK V. Impact compressive response of dry sand [J]. Mechanics of Materials, 2009, 41(6): 777–785. DOI: 10.1016/j.mechmat.2009.01.003. [12] 曹志远. 板壳振动理论 [M]. 北京: 中国铁道出版社, 1989: 304–305. [13] 王敏中, 王炜, 武际可. 弹性力学教程 [M]. 北京: 北京大学出版社, 2011: 264–266. -








 下载:
下载:

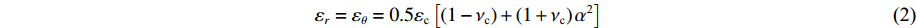

























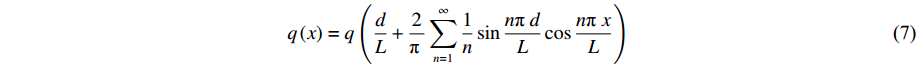










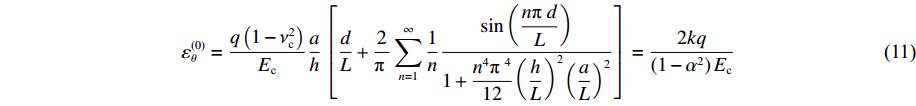















 下载:
下载: