A study of incomplete similar models for tyre fragment impact on fuselage structures
-
摘要: 为降低机身结构抗冲击性能的实验成本,利用相似理论建立机身的非等比例缩放模型,开展模型实验是行之有效的方法。基于量纲分析的方法,建立Johnson-Cook线性应变率函数的修正关系;鉴于生产制造技术的限制,考虑扭曲厚度的非等比例机身模型对相似性行为的影响,采用指数函数法建立了非等比例模型的相似修正关系。通过对比实验中破片冲击过程的变形形态、靶板的应变时间历程曲线和最终变形轮廓,验证了数值模型的有效性。此外,分析了破片偏航姿态、机身材料、厚度和质量等因素对机身结构抗冲击性能的影响。结果表明:(1) 150 m/s的冲击速度下,破片冲击角度90º和着靶角度180º是最严苛的冲击条件。综合多种因素,分析认为3.5 mm厚的钛合金为机身结构的最佳选择,并以此作为全尺寸原型验证相似模型;另外,提出了一种可以快速获取缩比模型的设计方法。(2)应变率效应对轮胎破片冲击机身结构的影响并不显著,等比例缩放模型与原型结果吻合较好。(3)厚度扭曲的非等比例模型能够有效地预测原型结构的变形行为;虽然,在时间尺度上,模型与原型存在一定的偏差;但是,在空间尺度上,非等比例相似模型能够有效地修正扭曲厚度造成中心最大挠度的预测误差,修正后的最大误差不超过5.1%,这表明该方法能够有效地指导机身结构的相似模型设计。Abstract: In order to reduce the cost for the impact test of the full-size airframe structures, an incomplete similar model was established by the similarity theory. Based on dimensional analysis, the correction relation for the Johnson-Cook linear strain-rate function was formulated. Due to the limitation of manufacturing technology, the effect of the incomplete similar model with distorted thickness on similarity behaviors should be taken into account, so an exponential function was adopted to establish the correction formula for the distorted thickness model. The validity of the simulation model was then verified by comparisons relevant to the deformation on the fuselage, the strain-time curves of target plates and the final deformation profile. In addition, the influences of fragment angle, material property, distortion thickness and light weight on the deformation behavior of the fuselage structure were analyzed. The following main results were obtained. (1) Under the impact velocity of 150 m/s, the most severe impact conditions appear at the impact angle of 90° and the fragment attitude of 180º; by considering various factors, the 3.5-mm-thickness titanium alloy plate is regarded as the best choice for fuselage structures, and it is used as a full-size prototype to verify the similar method. Besides, it’s worth noting that an unconventional phenomenon takes place at the impact angle of 30º, while a reasonable explanation is given. (2) The effect of strain rate on the impact of tire fragments on the fuselage structure is not notable, so the incomplete similar model is in good agreement with the prototype results. (3) The incomplete scaled-down model corrected by this method can effectively predict the deformation behavior of prototype fuselage subjected to the impact of tyre fragments. Although there is a certain deviation between the model and the prototype on the time scale, on the spatial dimensions, the incomplete scaled-down model can effectively correct the prediction error for the maximum center deformation caused by the distortion thickness, and the corrected maximum error is less than 5.1%, indicating that the method can effectively guide the design for airframe structures.
-
Key words:
- fuselage structure /
- similarity methods /
- incomplete similar model /
- structure impact
-
由于在化工、轻工和能源等行业广泛使用粉体为原材料或者中间体进行加工生产,粉尘爆炸风险大大增加,而粉尘爆炸一旦发生势必造成大量的人员伤亡和巨大的财产损失。为有效预防粉尘爆炸的发生,降低爆炸灾害后果,国内外学者在粉尘爆炸特性(最大爆炸压力、最大爆炸压力上升速率、最小点火能、爆炸下限等)和粉尘火焰传播特性等方面开展了大量实验研究[1-3],其中火焰传播特性的揭示可为制定粉尘爆炸防控技术提供理论基础。Dobashi等[4]和Chen等[5]通过实验研究揭示十八醇粉尘云火焰形态:中心为形状不规则的黄色发光区域,黄色区域外围的分布大量离散的蓝色发光点火焰。Gao等[6-10]、高伟等[11]、曹卫国等[12]采用带通滤波高速摄影技术系统的研究了半封闭管道及开敞空间有机粉尘粒子热特性及粒径分布特性对粉尘爆炸火焰传播特性的影响。Wingerden等[13]研究发现管道内玉米淀粉、石松子和煤粉粉尘云火焰前锋都呈抛物线形状。孙金华[14]通过实验揭示了PMMA粉尘云火焰结构和燃烧反应区特征,认为火焰结构可分为未燃区、主反应区和黄色发光区。Zhang等[15]通过实验研究发现微米和纳米PMMA粉尘云火焰传播有明显区别。Zhang等[16]还研究了不同粒径分布PMMA粉尘云火焰传播行为,结果发现火焰传播速度越快,则火焰温度越高。
不难发现,前人的研究主要集中在火焰形态、结构、传播行为及影响因素方面,很少涉及火焰温度分布规律。火焰温度对有效评估粉尘爆炸灾害后果具有重要的作用。因此,本文中采用自制R型热电偶和高速比色测温法对开敞空间内不同粒径PMMA粉尘云火焰温度进行测量,旨在揭示粉尘云火焰传播过程中的温度分布特性,为评估粉尘爆炸危害提供实验依据。
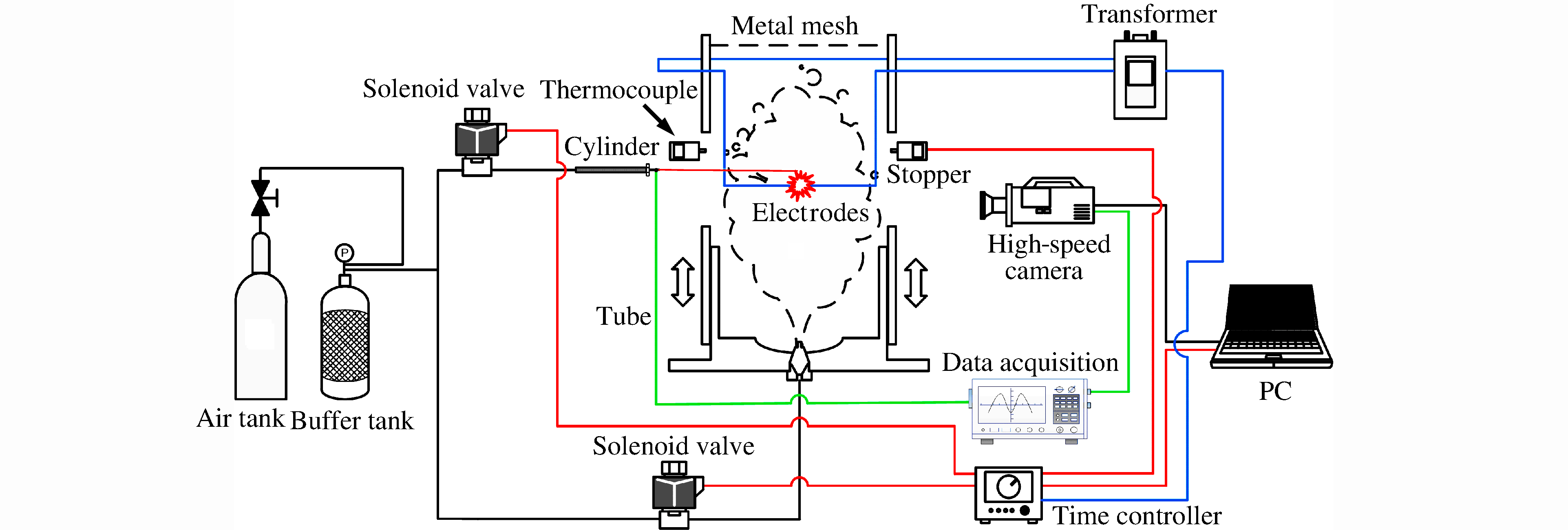
1. 实验系统
图 1所示为开放式粉尘爆炸火焰传播实验系统。该系统主要由圆柱形燃烧管道、配气及喷粉系统、高压点火系统、热电偶测温系统、高速比色测温系统、数据采集仪和时间控制器等组成。燃烧管道分为上、中、下3部分,其中中部管段内径为90 mm,高为125 mm,当限位器通电动作时,中部管道向下滑落形成开敞的燃烧空间。时间控制系统可以对喷粉、中部管道滑落、高速摄像机摄录,热电偶气缸的动作精准控制,时间控制如图 2所示。实验中将一定量的粉尘均匀铺放于实验底座粉尘仓内,然后由0.5 MPa的空气经过分散帽后携带进入燃烧管道形成粉尘云,气流持续时间0.5 s,此时高速摄像机开始摄录,数据采集仪由高速摄像机触发同时开始采集数据。在0.7 s时限位器动作,中部管道滑落形成开仓空间,1.0 s时由直径为1 mm的钨丝放电产生电火花引燃粉尘,点火电极之间间距约为4 mm,点火电压15 kV,电极放电点火时间10 ms。热电偶测温方法中采用FASTCAM SA4型高速摄像机记录火焰传播过程,高速比色测温系统使用型号为Thermera-HS高速摄像机拍摄火焰形态,进行后续处理可得到火焰温度分布。
1.1 热电偶测温系统
采用直径25 μm的铂/铑合金丝制作R型微细热电偶作为温度测量元件,如图 3所示。为消除点火时高压电流诱导产生的电磁场对热电偶测温的影响,实验中将2根热电偶丝分别穿过内径为1 mm的陶瓷管,同时将热电偶焊点处伸出陶瓷管2~3 mm。由于热电偶属于接触式测温元件,其本身具有一定的热惯性,为了获得更加准确的火焰温度值,需要对热电偶测量值进行温度修正。因为在热电偶焊点处对流换热远大于辐射换热,故焊点处的热平衡关系可以表示为[17]:
ρcpVdTmdt=hS(T−Tm) (1) 式中:h为对流换热系数,T为接点处气体的温度,ρ、cp、V、S和Tm分别为热电偶接点密度、质量定压热容、体积、表面积和温度。
假设热电偶焊点为圆球形状,则:
V= π 6d3 (2) S= π d2 (3) 整理以上3式可以得到:
ρcpπd36hπd2dTmdt=T−Tm (4) 式中左边第一项即为时间常数τ:
τ=ρcpπd36πd2=ρcpd26hd=ρcpd26(hdλf)λf=ρcpd26Nuλf (5) 式中:Nu为努塞尔数,根据实验条件取为2;d为热电偶接点直径,取d=5×10-5 m;λf为热电偶周围气体的导热系数,取λf=0.081 W/(m·K);ρ=2.14×104 kg/m3,cp=159 J/(kg·K)。计算得到τ=8.75 ms。因此,采用下式对热电偶测温值进行修正:
T=Tm+8.75×10−3dTmdt (6) 采用自制R型微细热电偶测量100 nm及30 μm PMMA粉尘云火焰传播过程中的温度分布。实验中,将热电偶固定在气动笔形气缸上,开始喷粉后0.85 s时刻,0.5 MPa的高压气流推动笔形气缸将热电偶推入粉尘云流场,保持热电偶的接点位于点火电极正上方2 cm处。
1.2 比色测温系统
热电偶测温属于定点接触式测温,只能测得热电偶焊点所在位置处的火焰温度值,为了获得整个燃烧区域火焰的温度分布,采用高速摄像机拍摄火焰图像,图像经过量化处理后送入计算机,计算机根据比色测温原理处理后获得爆炸火焰的温度分布。
由普朗克定律以及维恩位移定律可知:温度愈高,同一波长下的光谱辐射力愈大;并且在一定的温度下,黑体的光谱辐射力在某一波长下具有最大值。因此通过测量黑体辐射出的光谱分布特性曲线可知黑体温度。然而测量整个光谱辐射曲线十分困难,因此在许多实际问题中,通常由两种相邻波长之间的辐射能量比来测量温度。原理为当黑体温度发生变化时,这两种波长的光谱辐射力之比也会随温度发生变化,因此测量这两种波长光谱辐射力的比值可知黑体温度。虽然粉尘火焰不是黑体,但是可以近似的把火焰当成灰体处理,因为火焰的主要辐射成分是辐射光谱连续的固体颗粒[18]。假定这两种相邻波长的发射率ε相等[19],根据图 4则有:
Eλ1(g)Eλ2(g)=εEλ1(b)εEλ2(b)=Eλ1(b)Eλ2(b) (7) 式中:ε为发射率,Eλ1(g)和Eλ1(b)为波长λ1时灰体和黑体的光谱辐射力,Eλ2(g)和Eλ2(b)为波长λ2时灰体和黑体的光谱辐射力。由此可知,粉尘火焰在这两种波长下的光谱辐射力之比与黑体在对应波长下的光谱辐射力之比相等,因此利用黑体的辐射特性处理火焰图片后能够获得火焰温度。
2. 结果与讨论
2.1 热电偶测温结果
图 5所示为开敞空间内粉尘质量浓度为364 g/m3的100 nm及30 μm的PMMA粉尘云火焰温度。从图中可以看出,100 nm PMMA粉尘云火焰的最高温度可达到1 551 ℃,30 μm PMMA粉尘云火焰最高温度仅达到1 108 ℃。纳米粉尘云的热解/挥发速率较快,燃烧反应充分,因而其火焰的温度高于微米粉尘云。由于纳米粉尘云火焰传播速度大于微米粉尘云火焰[11],因而纳米粉尘云火焰前锋及预热区能更早的传播到热电偶测温接点处。由图可知,纳米粉尘云火焰温度开始上升时刻早于微米粉尘云火焰。对于纳米粉尘云火焰,火焰传播约10 ms后火焰温度开始快速上升,在约70 ms时刻,火焰温度达到一个极大值,之后火焰温度进入波动增长阶段,在约270 ms时刻,火焰温度达到最大值。这一温度波动增长的现象,极有可能是由团聚/结块粒子的热解/挥发与燃烧放热所导致。而对于微米粉尘云火焰,火焰传播约35 ms后火焰温度开始快速持续上升,在约133 ms时刻,火焰温度达到最大值,之后由于热量损失温度值略有下降,而后又升高到最高火焰温度,这是由于30 μm的PMMA粉尘云火焰局部预混燃烧的燃烧机理所导致[11]。
2.2 比色测温法结果
图 6~9显示了质量粉尘浓度均为290 g/m3粒径分别为3、10、20和30 μm的PMMA粉尘云在不同时刻火焰形态及火焰温度分布。
由图 6中可知,3 μm的粉尘云火焰温度分布比较均匀,火焰面内温度梯度较小,粉尘燃烧可以近似为均相燃烧,粉尘火焰与气体预混火焰类似,说明3 μm的PMMA粉尘粒子热解/挥发速率快,在预热区内大部分粒子已经热解/挥发完全。在爆炸火焰未传播到上、下两部分燃烧管壁面期间,火焰中心温度较火焰边缘温度略高。当火焰触碰到上下燃烧壁后,温度较高区域逐渐向上移动,这是因为在点火初期,悬浮的粉尘粒子由于重力作用沉降,当火焰充分发展后沉降的粒子在燃烧产物热膨胀的推动作用下向上运动[20]。
当粒径增加到10 μm时,火焰温度整体较平均,但是在燃烧中后期出现局部间断高温火焰。当粒径继续增大为20 μm时,高温火焰区域随着燃烧的进行逐渐增大并且连成一片,但是高温区域附近同时会形成一片低温火焰区,该温度低于3和10 μm粉尘云的最低火焰温度,并且低温火焰分布区位于高温区上部,这是由于上部区域内粉尘粒子燃尽导致。当粒径达到30 μm时,火焰温度分布并未出现类似20 μm粉尘云时的连续高温火焰,只是在点火电极附近形成局部离散的高温火焰区,其余空间内多处为低温火焰区,说明由于粉尘粒径较大,热解/挥发速率较慢,且需要吸收大量的热量,因此导致火焰温度较低。随着粉尘粒子粒径的增大,火焰温度分布越不均匀,火焰最高温度及高温火焰区面积均增大,在粉尘粒径为20 μm时达到最大值。随着粉尘粒径的继续增大,火焰最高温度不再增加,高温火焰区面积急剧缩小,同时低温火焰区扩大。
比较不同粒径分布火焰形态和火焰温度分布可知,随着粉尘粒径的增大,火焰传播速度逐渐减小,当粒径增大到20 μm后,燃烧初期火焰离散,此时粉尘燃烧表现为异相燃烧。这是由于在大粒径粉尘中也会存在少量的小粒径粒子,小粒径粉尘粒子的热解/挥发速率快,在大粒径粒子受热还未开始热解/挥发之前就已经热解/挥发完毕形成局部预混火焰。同时局部预混火焰也会向大粒径粒子传热加速其热解/挥发过程,因此在燃烧后期火焰传播速度明显增大,并出现高温火焰区。
2.3 两种测温结果分析
由高速比色测温结果可知,随着粉尘粒径增大,粉尘燃烧由均相燃烧逐渐转变为异相燃烧,最高火焰温度和高温火焰区面积先增大后减小,在粉尘粒径20 μm时达到最大。由图 10所示的PMMA粉尘粒子扫描电镜图可知,3 μm粉尘粒子由于团聚/结块效应导致部分粉尘粒子粒径略微增大,导致团聚/结块的粒子热解/挥发速率变慢,其燃烧稍微滞后于未团聚/结块粒子,燃烧空间内主要由未结块粉尘粒子热解/挥发出的可燃气体维持,故3 μm粉尘云火焰温度分布均匀,但高温火焰呈离散点分布。粉尘粒径为20 μm时,由于粉尘粒子分散性好,粒子裂解气化特征时间尺度与燃烧反应特征时间尺度较接近,因而燃烧反应更加剧烈,形成高温火焰区。粉尘粒子粒径增大为30 μm后,由于粉尘粒子粒径增大导致热解/挥发速率变慢,因而燃烧空间内可燃气体燃烧时强度低于20 μm粉尘云,火焰温度和高温火焰区面积均减小。热电偶测温结果表明,100 nm粉尘云最高火焰温度远大于30 μm粉尘云。这是因为即使纳米PMMA粉尘粒子存在严重的团聚/结块效应,但100 nm粉尘粒子的索特直径为10.49 μm,而30 μm粉尘颗粒的索特直径为24.08 μm。由于100 nm粉尘粒子索特粒径较小,热容量与表面积比率较小,粉尘粒子能快速热解/挥发出可燃气体参与反应,燃烧反应更充分,因而其火焰温度更高。
通常情况下,火焰温度越高,说明粉尘燃烧的放热量越大;火焰温度上升速率越快则说明粉尘燃烧速率越大,对应的火焰传播速度越大。热电偶测温方法的优点在于其可以准确测量火焰最高温度和火焰温度上升速率,因此利用热电偶测温数据可以反应粉尘爆炸的危险程度。然而热电偶只能测得固定点的温度变化情况,并且在测量时必须处在火焰中才能测得火焰温度。比色测温法可以在较远的位置获得整个粉尘火焰温度的演变过程及分布,能够更全面的分析火焰传播行为。但是比色测温法的局限在于只能应用于可视化粉尘爆炸装置,并且无法获得准确的火焰温度值。
3. 结论
本文中通过实验研究了不同粒径PMMA粉尘云温度分布特性,利用自制R型微细热电偶测量了100 nm和30 μm粉尘云火焰温度并修正,同时利用高速比色测温系统得到3、10、20和30 μm粉尘云的火焰形态及对应的火焰温度分布,结论如下:
(1) 热电偶测温结果表明,虽然100 nm PMMA粉尘粒子严重的团聚/结块效应导致粒子粒径增大,但是其热容量与表面积比率较小,粉尘粒子热解/挥发速率较大,燃烧充分,因而100 nm PMMA粉尘云火焰的最高温度可达到1 551 ℃,而30 μm PMMA粉尘云火焰最高温度仅为1 108 ℃,因此纳米粉尘爆炸的危险程度远大于微米粉尘。
(2) 比色测温结果表明,在微米尺度,随着PMMA粉尘粒子粒径增大,火焰温度分布越不均匀,粉尘燃烧由均相燃烧向异相燃烧转变,火焰最高温度和高温火焰区面积先增大后减小。由于20 μm粉尘粒子分散性好,裂解气化特征时间尺度与燃烧反应特征时间尺度较接近,粉尘粒子燃烧充分,因而在粉尘粒子粒径为20 μm时,火焰最大温度和高温火焰区面积达到最大值。
(3) 热电偶测温方法和比色测温方法有各自的优缺点,热电偶测温方法精准但局限于单点位置,比色测温方法测温区域广但测温精度差。通过对比分析后发现,在热电偶测温实验系统中的另一个方位加上比色测温系统,则有可能将两种测温方法结合起来全面分析粉尘爆炸火焰温度特性及火焰传播过程。将两种测温方法结合测量粉尘爆炸温度需要开展更多的实验研究。
-
图 1 高速摄影[2]和数值计算分别得到的橡胶轮胎破片以30º冲击角度和135 m/s的冲击速度撞击铝合金靶板后不同时刻的变形形貌
Figure 1. Deformation morphologies of the rubber tire fragment with the initial impact velocity of 135 m/s at the impact angle of 30º after its impacting on an aluminum alloy target at different times obtained by high-speed photography[2] and simulation
材料 ρ/(g·cm−3) E/GPa μ A/MPa B/MPa N C m Al 2024 2.71 72 0.33 225 406 0.34 0.015 1.0 Ti-6Al-4V 4.45 110 0.41 862 331 0.34 0.012 0.8 表 2 靶板中心点最终挠度的实验结果[2]与模拟结果的对比
Table 2. Comparison of the residual deformations at the centers of the target plates between experiment[2] and simulation
破片尺寸 靶板尺寸 β/(º) v/(m·s−1) 靶板中心点的最终挠度 实验值/mm[2] 模拟值/mm 相对偏差/% 30 mm×15 mm×60 mm 260 mm×260 mm×1.6 mm 30 135 2.9 2.4 18.6 30 mm×15 mm×60 mm 260 mm×260 mm×1.6 mm 30 136 8.6 10.1 17.4 表 3 不同冲击条件下方形铝靶板中心的响应参数
Table 3. Response parameters at the centers of square aluminum plates under different impact conditions
β/(º) θ/(º) v/(m·s−1) 瞬时最大挠度/mm 最终挠度/mm 脉宽/ms 30 30 150 7.82 2.04 1.028 120 7.28 3.16 1.300 60 60 12.1 7.80 1.178 150 14.6 10.4 1.420 90 90 15.0 10.2 1.260 180 17.2 13.6 1.450 表 4 机身的几何结构及响应参数
Table 4. Geometric structure and response parameters of the fuselage
材料 厚度/mm 质量/kg 瞬时最大挠度/cm Al01铝合金 4.0 92.9 9.35 5.0 116.2 7.91 6.0 139.4 6.77 钛合金 3.0 113.2 9.08 3.5 132.0 7.28 4.0 150.9 6.24 表 5 对比模型与原型的瞬时最大挠度
Table 5. Comparison of the maximum displacement between the model and the prototype
对比源 比例因子 靶板厚度/mm 原型冲击速度/(m·s−1) 靶板挠度/cm 挠度相对偏差/% 模型修正速度/(m•s−1) 原型 1 3.500 150 7.28 模型 1/10 0.350 7.13 2.06 151.9 1/8 0.438 7.25 0.41 151.7 1/5 0.700 7.26 0.27 151.4 1/2 1.750 7.17 1.51 150.6 表 6 不同指数对应的冲击速度和挠度
Table 6. Impact velocities and central deflections in relation to different exponents
n vm/(m·s−1) αx1=2.0 αx2=3.5
|δx1−δx2|/δx1/%vx1/(m·s−1) δx1/cm vx2/(m·s−1) δx2/cm 1.00 150 279.9 8.66 463.2 7.74 12 1.05 300.0 8.88 525.0 8.20 8 1.12 310.6 9.10 558.9 8.50 7 1.15 321.5 9.25 595.1 8.96 3 表 7 对比原型与模型的靶板瞬时最大挠度
Table 7. Comparison of the maximum deflection between the prototype and models
模型 缩放因子 扭曲因子 机身板厚/mm 冲击速度/(m·s−1) 靶板挠度/cm 与原型挠度的相对偏差 /% 原型 1 1.00 3.5 150.0 7.28 扭曲非等
比例模型1/8 2.29 1.0 150.0 2.12 71.9 2.74 1.2 150.0 1.61 78.9 3.43 1.5 150.0 1.09 85.0 修正后的扭曲
厚度模型1/8 2.29 1.0 329.6 7.58 4.1 2.74 1.2 484.2 7.65 5.1 3.43 1.5 625.9 7.13 2.1 -
[1] 张建敏. 飞机轮胎爆破模式浅析 [J]. 力学季刊, 2014, 35(1): 139–148. DOI: 10.15959/j.cnki.0254-0053.2014.01.019.ZHANG J M. A brief study on damaging effects of Aeroplane tire and wheel failures [J]. Chinese Quarterly of Mechanics, 2014, 35(1): 139–148. DOI: 10.15959/j.cnki.0254-0053.2014.01.019. [2] MINES R A W, MCKOWN S, BIRCH R S. Impact of aircraft rubber tyre fragments on aluminium alloy plates: Ⅰ: experimental [J]. International Journal of Impact Engineering, 2007, 34(4): 627–646. DOI: 10.1016/j.ijimpeng.2006.02.005. [3] KARAGIOZOVA D, MINES R A W. Impact of aircraft rubber tyre fragments on aluminium alloy plates: Ⅱ: numerical simulation using LS-DYNA [J]. International Journal of Impact Engineering, 2007, 34(4): 647–667. DOI: 10.1016/j.ijimpeng.2006.02.004. [4] JIA S Q, WANG F S, YU L J, et al. Numerical study on the impact response of aircraft fuselage structures subjected to large-size tire fragment [J]. Science Progress, 2020, 103(1): 36850419877744. DOI: 10.1177/0036850419877744. [5] 杨娜娜, 赵天佑, 陈志鹏, 等. 破片冲击作用下舰船复合材料结构损伤的近场动力学模拟 [J]. 爆炸与冲击, 2020, 40(2): 023302. DOI: 10.11883/bzycj-2019-0019.YANG N N, ZHAO T Y, CHEN Z P, et al. Peridynamic simulation of damage of ship composite structure under fragments impact [J]. Explosion and Shock Waves, 2020, 40(2): 023302. DOI: 10.11883/bzycj-2019-0019. [6] 陈映秋, 杨永谦. 相似理论在分节驳结构模型试验中的应用 [J]. 中国造船, 1985(4): 56–64.CHEN Y Q, YANG Y Q. The application of similitude theory in integrated barge model test [J]. Shipbuilding of China, 1985(4): 56–64. [7] 秦健, 张振华. 原型和模型不同材料时加筋板冲击动态响应的相似预报方法 [J]. 爆炸与冲击, 2010, 30(5): 511–516. DOI: 10.11883/1001-1455(2010)05-0511-06.QIN J, ZHANG Z H. A scaling method for predicting dynamic responses of stiffened plates made of materials different from experimental models [J]. Explosion and Shock Waves, 2010, 30(5): 511–516. DOI: 10.11883/1001-1455(2010)05-0511-06. [8] 沈雁鸣, 陈坚强. 超高速碰撞相似律的数值模拟验证 [J]. 爆炸与冲击, 2011, 31(4): 343–348. DOI: 10.11883/1001-1455(2011)04-0343-06.SHEN Y M, CHEN J Q. Numerically simulating verification of the comparability rule on hypervelocity impact [J]. Explosion and Shock Waves, 2011, 31(4): 343–348. DOI: 10.11883/1001-1455(2011)04-0343-06. [9] 杨亚东, 李向东, 王晓鸣, 等. 钢筋混凝土结构内爆炸相似模型试验研究 [J]. 南京理工大学学报(自然科学版), 2016, 40(2): 135–141. DOI: 10.14177/j.cnki.32-1397n.2016.40.02.002.YANG Y D, LI X D, WANG X M, et al. Experimental study on similarity model of reinforced concrete structure under internal explosion [J]. Journal of Nanjing University of Science and Technology, 2016, 40(2): 135–141. DOI: 10.14177/j.cnki.32-1397n.2016.40.02.002. [10] OSHIRO R E, ALVES M. Predicting the behaviour of structures under impact loads using geometrically distorted scaled models [J]. Journal of the Mechanics and Physics of Solids, 2012, 60(7): 1330–1349. DOI: 10.1016/j.jmps.2012.03.005. [11] KONG X S, LI X, ZHENG C, et al. Similarity considerations for scale-down model versus prototype on impact response of plates under blast loads [J]. International Journal of Impact Engineering, 2017, 101: 32–41. DOI: 10.1016/j.ijimpeng.2016.11.006. [12] KANG H, GUO X H, ZHANG Q M, et al. Predicting the behavior of armored plates under shallow-buried landmine explosion using incomplete scaling models [J]. International Journal of Impact Engineering, 2021, 156: 103970. DOI: 10.1016/j.ijimpeng.2021.103970. [13] MAZZARIOL L M, OSHIRO R E, ALVES M. A method to represent impacted structures using scaled models made of different materials [J]. International Journal of Impact Engineering, 2016, 90: 81–94. DOI: 10.1016/j.ijimpeng.2015.11.018. [14] 徐坤. 典型结构响应相似律的应变率效应研究 [D]. 北京: 北京理工大学, 2016: 78. DOI: 10.26948/d.cnki.gbjlu.2016.000280.XU K. Research on scaling law of typical structure on strain rate effect [D]. Beijing, China: Beijing Institute of Technology, 2016: 78. DOI: 10.26948/d.cnki.gbjlu.2016.000280. [15] 苏子星, 何继业. 基于Cowper-Symonds方程的相似理论修正方法 [J]. 爆炸与冲击, 2018, 38(3): 654–658. DOI: 10.11883/bzycj-2016-0308.SU Z X, HE J Y. Modified method for scaling law based on Cowper-Symonds equation [J]. Explosion and Shock Waves, 2018, 38(3): 654–658. DOI: 10.11883/bzycj-2016-0308. [16] 刘源, 皮爱国, 杨荷, 等. 非等比例缩比侵彻/贯穿相似规律研究 [J]. 爆炸与冲击, 2020, 40(3): 033302. DOI: 10.11883/bzycj-2019-0086.LIU Y, PI A G, YANG H, et al. Study on similarity law of non-proportionally scaled penetration/perforation test [J]. Explosion and Shock Waves, 2020, 40(3): 033302. DOI: 10.11883/bzycj-2019-0086. [17] 陈材, 石全, 尤志锋, 等. 圆柱形弹药空气中爆炸相似性规律 [J]. 爆炸与冲击, 2019, 39(9): 092202. DOI: 10.11883/bzycj-2018-0255.CHEN C, SHI Q, YOU Z F, et al. Similarity law of cylindrical ammunition explosions in air [J]. Explosion and Shock Waves, 2019, 39(9): 092202. DOI: 10.11883/bzycj-2018-0255. [18] Livermore Software Technology Corporation. LS-DYNA keyword user’s manual: Volume Ⅱ: material model [M]. Livermore, California, USA: Livermore Software Technology Corporation, 2018. [19] NEUBERGER A, PELES S, RITTEL D. Springback of circular clamped armor steel plates subjected to spherical air-blast loading [J]. International Journal of Impact Engineering, 2009, 36(1): 53–60. DOI: 10.1016/j.ijimpeng.2008.04.008. 期刊类型引用(10)
1. 赵宇峰,段继,冯顺山. 反应破片对带壳装药的冲击效应. 爆炸与冲击. 2024(11): 130-140 .  本站查看
本站查看2. 张琨,刘永旭,赵长啸,唐蓉,杨善平,张昌旺. MEFP协同冲击平面带壳装药数值模拟研究. 兵器装备工程学报. 2022(08): 13-19 .  百度学术
百度学术3. 顾阳晨,王金相,陈兴旺,刘志刚,郝春杰,李渊博. 高速动能破片和包覆活性材料对屏蔽装药的串联毁伤效应. 含能材料. 2021(07): 607-616 .  百度学术
百度学术4. 张琨,张少光,张波,唐蓉,杨善平,张昌旺. EFP斜冲击高速运动柱壳装药数值模拟研究. 兵器材料科学与工程. 2021(06): 111-117 .  百度学术
百度学术5. 张涛,刘雨生,高志鹏,杨佳,刘艺,谷岩. 垫层对破片冲击起爆带壳炸药影响的数值模拟. 爆炸与冲击. 2018(06): 1241-1246 .  本站查看
本站查看6. 林辉,夏广东,余万千,刘吉安. 惯性激发式含能毁伤元起爆特性研究. 弹箭与制导学报. 2018(03): 139-142 .  百度学术
百度学术7. 章猛华,阮文俊,宁惠君,董凯,余永刚. 复合型含能破片冲击波起爆特性研究. 弹道学报. 2016(01): 64-69 .  百度学术
百度学术8. 彭加斌,肖勇,段嘉庆,张明哲,张龙,朱伟龙. 一种油气井用双向传爆管极限传爆距离. 含能材料. 2016(08): 804-809 .  百度学术
百度学术9. 李兵,陈曦,杜忠华,王琪,徐立志. LEFP对带壳装药冲击起爆过程的数值模拟与试验. 含能材料. 2016(11): 1034-1040 .  百度学术
百度学术10. 王利侠,谷鸿平,丁刚,刘丰旺,孙兴昀. 聚能射流对带壳浇注PBX装药的撞击响应. 含能材料. 2015(11): 1067-1072 .  百度学术
百度学术其他类型引用(4)
-








 下载:
下载:










 下载:
下载:

















 百度学术
百度学术