Oblique penetration effect of a tungsten ball on high hardness steel
-
摘要: 为研究高硬度钢板抗不同着角钨球的侵彻性能及破坏模式,通过弹道枪进行了
∅ 8 mm、∅ 11 mm钨合金球形破片以0°、20°、40°着角撞击厚度为6 mm、8 mm的高硬度钢板试验,得到了极限贯穿速度v50;分析了钨球轴向径向变形及靶板失效模式与撞击速度的关系,发现高硬度钢板失效模式主要为压缩开坑破坏和沿厚度方向剪切破坏。采用有限元方法对试验进行了模拟,验证了数值模型及参数的合理性,并运用数值模拟方法研究了撞击着角对靶板吸能模式影响,结合试验数据,修正已有极限贯穿速度计算公式。结果表明:随侵彻着角增大,极限贯穿速度提高,且着角越大,极限贯穿速度增长越快;随着角增大,靶板吸能模式逐渐由压缩开坑向剪切冲塞过渡,且着角大于50°时,剪切冲塞耗能将超过压缩开坑耗能;修正后极限贯穿速度计算公式适用范围更广、精度更高,具有较好工程应用价值。Abstract: In order to study the penetration performance and failure modes of high-hardness steel plates against tungsten balls with different angles, a ballistic gun was used to carry out∅ 8 mm,∅ 11 mm tungsten alloy spherical fragments at 0°, 20°, 40° angles to impact the thickness of 6 mm, 8 mm. In the hardness steel plate test, the limit penetration velocity (v50) of the fragments impacting the steel plate was obtained; the relationships between the axial and radial deformation of the tungsten ball after the impact and the failure mode of the target plate and the impact velocity were analyzed. It is found that the failure mode of the high hardness steel plate is mainly the compression opening. For the pit failure and shear failure along the thickness direction, the shear fracture increases as the angle increases. The experiment was simulated by the method of finite element simulation. The simulation results were compared with the test results. The damage morphology of the target plate and the limit penetration velocity were in good agreement. The validity of the numerical simulation model and parameters was verified, and the numerical simulation method was used. The influence of the impact angle on the energy absorption mode of the target plate was studied, and the existing calculation formula of the limit penetration velocity was revised based on the experimental data. The results show that as the penetration angle increases, the limit penetration velocity increases, and the larger the penetration angle, the faster the limit penetration velocity increases; the revised limit penetration velocity calculation formula has a wider application range and higher accuracy, and has better engineering applications. As the angle increases, the energy absorption mode of the target plate gradually changes from compression opening to shearing plugging, and when the angle exceeds 50°, the energy consumption of shearing plugging will exceed the energy consumption of compression opening. -
钻爆法作为一种经济、高效的施工方法,常用于隧道开挖工程。但由于爆破过程释放瞬时高能引发爆破振动,导致岩土体与结构产生负面响应,严重时将发生失稳破坏。因此,明确爆破振动的产生机制及传播特征具有重要的工程意义。
通常采用质点峰值振速(peak particle velocity, PPV)描述爆破振动效应,GB 6722-2014《爆破安全规程》[1]中规定用Sadovskyi公式描述爆破振动的传播。唐海等[2]和蒋楠[3]考虑了高程效应对Sadovskyi公式进行了改进,进一步提升了描述爆破振动传播规律的精确度。随着计算机技术的发展,离散元[4]、有限元[5]、FDEM[6]等数值模拟方法常用于模拟爆破振动。尽管采用数值模拟方法可以得到爆破振动场,但仍无法明确爆破振动的产生机制以及更本质的传播特征。
针对爆破振动,学者们提出了不同的理论模型并得到了振动场解答。Sharpe[7]首先提出了球状药包在无限弹性介质中爆炸动力响应问题的解析表达式。Blake[8]和Ricker[9]针对不同爆炸压力函数以及介质进行了拓展研究。但工程爆破中一般采用柱状药包,球状药包理论模型只适用于远场分析。Heelan[10]采用双重Fourier变换解析得到了柱状药包爆炸振动场,明确提出了柱状药包爆炸会激发P波和S波。Jordan[11]和Abo-Zena[12]通过不同数学方法得到的结论与Heelan[10]的结论基本一致。半无限空间相比无限空间存在自由边界条件,这将导致自由面的振动响应与空间内部的不同。Lamb[13]最早提出了二维半无限空间瞬态动力响应问题,明确了表面源和埋地源激发应力波的产生机制,指出在自由面上存在P波、S波和R波,这些波从震中向远处传播并衰减。此外,学者们对点源[14]、线源[15]、三维半无限空间[15-17]、不同加载形式[14]等Lamb问题进行了延伸研究。尽管此类问题已有较多解答,但大部分为半解析解,不适用于复杂地质情况。在隧道爆破振动方面,缺少相关理论,学者们通常针对爆破振动信号开展研究[18-19],但仍将爆破地震波作为一个整体进行分析,少有将爆破地震波进行解耦研究。由于P波、S波和R波作用于隧道结构会产生不同的动力效应,而这3类波衰减特性不同导致隧道振动有明显的分区特性。高启栋等[20-21]结合P波、S波和R波的传播特性对水平孔和垂直孔诱发的爆破振动进行分区。陈士海等[22]以比例距离
ˉr 为指标,认为ˉr <12 m/kg1/3为爆破近区,12 m/kg1/3<ˉr <22 m/kg1/3为爆破中区,ˉr >22 m/kg1/3为爆破远区。杨年华[23]建议用比例距离ˉr =10 m/kg1/3来界定爆破近区和远区。上述研究主要针对自由面、无限空间做爆破振动解析,未对隧道这种内部自由面做具体分析,且大部分仍集中于对振动数据的处理,未明确隧道表面爆破地震波的产生机制以及各类型波的作用范围。因此,本文中,提出一种二维隧道表面爆破振动理论模型,得到隧道表面爆破振动场的积分表达,明确隧道表面爆破地震波的产生机制;结合现场测试建立数值模型,对理论解答进行验证;采用高精度波场分离方法,综合理论解析和数值模拟结果得到隧道表面爆破地震波的传播特征,确定各类地震波作用分区,以期为分析隧道爆破振动问题提供理论依据。
1. 隧道表面爆破地震波的产生机制
1.1 基本方程
炸药起爆产生的瞬时爆压作用于炮孔壁,从而引发岩体爆破振动,爆破振动以地震波的形式向围岩扩散。按照波传播路径的不同可分为体波和面波。体波在岩体内部传播,包括纵波(P波)和横波(S波)2种类型。一般而言,面波是由不均匀P波和不均匀S波耦合形成的,只存在于介质表面附近,按照形成条件的不同可分为Rayleigh波(R波)和Love波(L波),由于L波只有在介质上覆薄低速层的情况下才出现,所以在讨论一般工程问题时不考虑[21]。利用Helmholtz分解,波动方程通常以位移势函数形式表达:
∇2φ−1c2p∂2φ∂t2=0,∇2ψ−1c2s∂2ψ∂t2=0 (1) 式中:cp为纵波波速,cs为横波波速,
φ 为位移标量势,ψ为位移矢量势。考虑隧道表面对地震波的影响,关键在于R波的产生机制及作用效应。已有隧道爆破振动效应相关研究[16]揭示R波的存在会在爆心距较远处对隧道结构带来一定的破坏。在理论解析方面,结合三维柱面自由边界条件求解式(1)难以获得R波闭合解,因此需要对三维问题进行简化。Biot[24]在分析圆柱形空孔表面Rayleigh波的稳态特征后发现,当爆心距大于圆柱半径时,圆柱形空孔表面Rayleigh方程与平面情况一致,同时Rayleigh波往往在远处才起主导作用。因此,基于这一前提,对隧道表面爆破地震波的产生机制问题进行简化,即沿隧道轴向对称轴取一平面,该问题即转变为半无限空间自由面一端作用有限长荷载问题,同时符合平面应变条件,理论模型如图1所示。图1中已开挖隧道面为自由端,在爆破开挖位置作用长度为l的爆破冲击荷载,模型其余边界均为无限边界。需要注意的是,由于炸药后冲荷载对已开挖区振动影响较小,在此模型中忽略了掌子面处的正向荷载。由于本文的研究对象为已开挖部分,未开挖部分不会对地震波的传播产生影响,因此为了简化计算,考虑对称性可进一步简化理论模型,如图1所示。
上述理论模型中,炸药爆炸荷载等效作用于隧道轮廓线上,则问题的初值条件和定解条件分别为:
˙φ|t=0=∂φ∂t=0,˙ψ|t=0=∂ψ∂t=0 (2) σzz(x,0,t)=P0g(t)[H(x+l/2)−H(x−l/2)],τzx(x,0,t)=0 (3) limz→∞(φ,ψ,˙φ,˙ψ)=0x≥0, t>0 (4) 式中:σzz为法向应力,τzx为切向应力,l为荷载长度,P0为荷载峰值,g(t)为荷载时间函数,H(x+l/2)和H(x−l/2)为Heaviside单位阶跃函数。
1.2 问题解析
求解二维波动问题通常采用数学变换减少未知数。对式(1)中的时间t进行Laplace变换,对坐标x进行Fourier变换:
ˆΛ(x,z,s)=L{Λ(x,z,t)},˜Λ(ω,z,t)=F{Λ(x,z,t)}Λ=φ,ψ (5) 式中:s为Laplace算符,ω为圆频率。
将式(1)代入式(5),有:
∂2ˆ˜φ∂z2−k2pˆ˜φ=0,∂2ˆ˜ψ∂z2−k2sˆ˜ψ=0 (6) 此时有通解:
ˆˉφ=A(ω,s)e−kpz,ˆˉψ=B(ω,s)e−ksz (7) 式中:
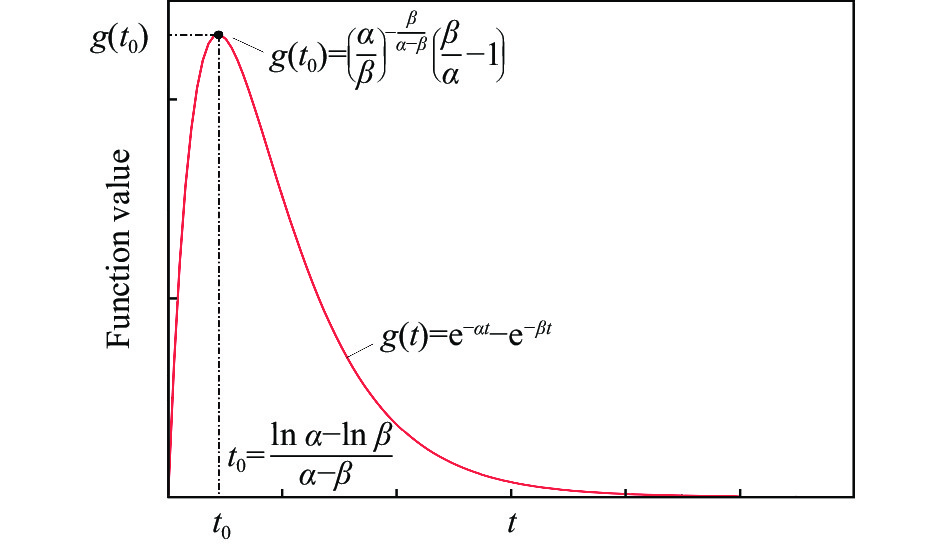
kp=√s2c2p+ω2 ,ks=√s2c2s+ω2 ;A和B为待定系数,通过初值条件和边界条件求解。爆破荷载为瞬时冲击荷载,常见形式有三角型、指数型和双指数型等,其中双指数型荷载与炸药实际爆压时程变化相近[25],令
g(t)=e−αt−e−βt ,其中α和β为衰减系数,爆破荷载时间衰减函数如图2所示,在t=t0处达到峰值,荷载峰值控制爆破荷载峰值大小。结合爆破荷载形式以及初值条件,将边界条件式(3)进行Laplace和Fourier双重变换,代入弹性介质几何方程与本构方程得到:
ˆ˜σzz|z=0=λc2ps2ˆ˜φ+2G(∂2ˆ˜φ∂z2−iω∂2ˆ˜ψ∂z)=P0ˆg(s)˜H(l−x) (8) ˆ˜τzx|z=0=Gs2c2sˆ˜ψ−2G(iω∂ˆ˜φ∂z−∂2ˆ˜ψ∂z2)=0 (9) 式中:G为岩体剪切模量,λ为岩体拉梅常数。
将式(7)代入式(8)~(9),联立解得:
A(ω,s)=P0ˆg(s)˜H(ω)Gkr(k2s+ω2),B(ω,s)=P0ˆg(s)˜H(ω)Gkr(2iωkp) (10) 式中:
kr=(k2s+ω2)2−4ω2kpks ,ˆg(s) 为荷载时间函数的Laplace变换式,˜H(ω) 为荷载分布函数的Fourier变换式。将系数A和B代入式(7),结合位移与位移势的关系得到水平位移ux和垂向位移uz分别为:
ˆ˜ux(ω,z,s)=iωP[(k2s+ω2)e−kpz−2kpkse−ksz],ˆ˜uz(ω,z,s)=−kpP[(k2s+ω2)e−kpz−2ω2e−ksz] (11) 式中:
P=P0ˆg(s)˜H(ω)/(Gkr) 。对式(11)进行双重积分反变换,有:
˜ux(ω,z,t)=12πi∫γ+i∞γ−i∞ˆ˜ux(ω,z,s)estds,ux(x,z,t)=12π∫+∞0˜ux(ω,z,t)eiωxdω (12) ˜uz(ω,z,t)=12πi∫γ+i∞γ−i∞ˆ˜uz(ω,z,s)estds,uz(x,z,t)=12π∫+∞0˜uz(ω,z,t)eiωxdω (13) 式中:γ为实变数。针对频域可采用留数定理选择合适的积分路径进行Laplace逆变换。令z=0,s=csωη,代入到式(12)~(13),得到:
˜ux(ω,0,t)=12πiP0cs˜H(ω)G×∫γ+i∞γ−i∞i(b2+1)−2ab(b2+1)2−4abˆg(s)ecsωηtdη (14) ˜uz(ω,0,t)=−12πiP0cs˜H(ω)G×∫γ+i∞γ−i∞a[(b2+1)−2](b2+1)2−4abˆg(s)ecsωηtdη (15) 式中:
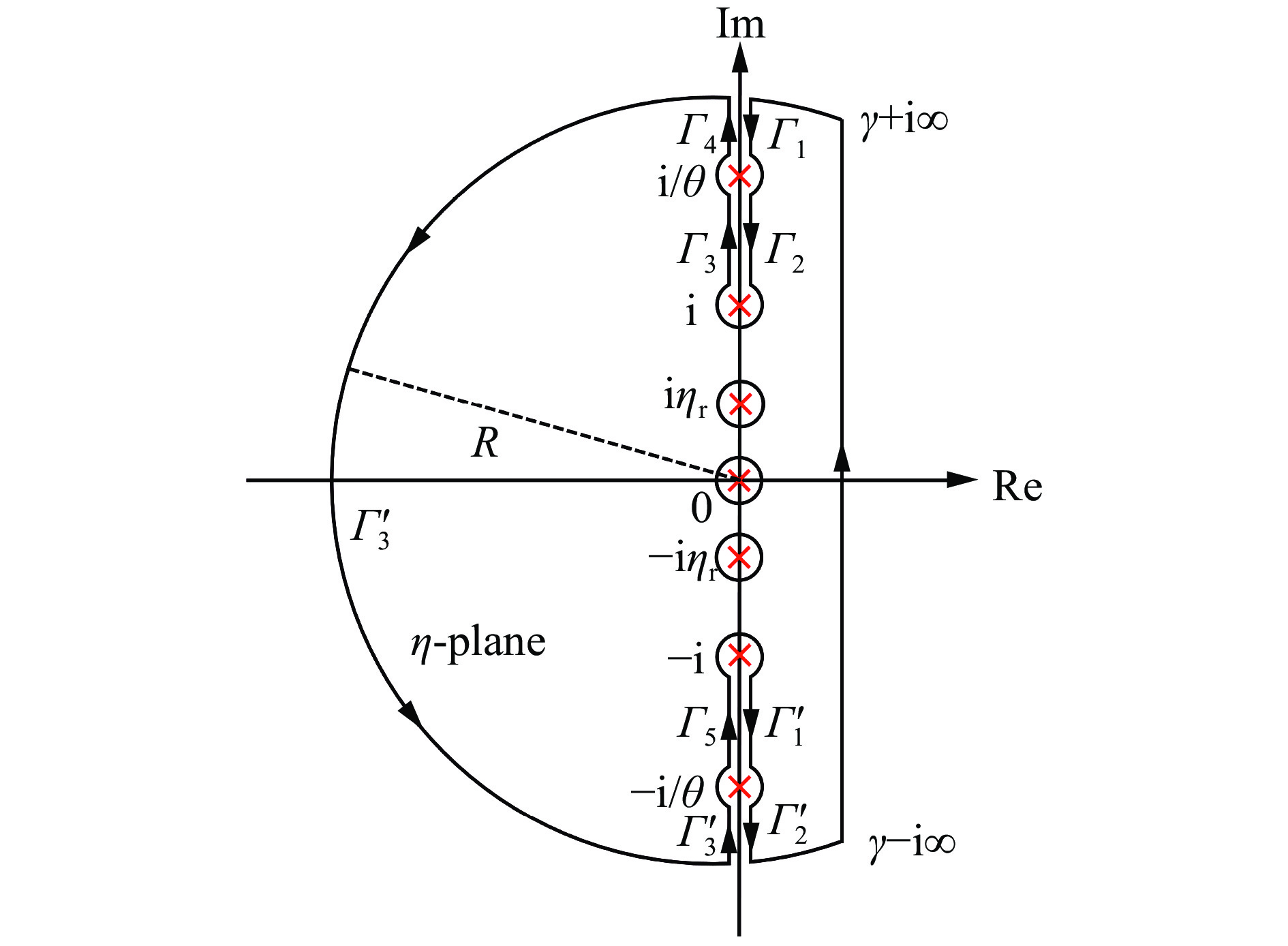
a=√1+η2θ2 ,b=√1+η2 ,θ=cs/cp 。根据式(14)~(15)可知,在η平面内存在极点η=±iηr, 0,与支点η=±i/θ, ±i,构成如图3所示的积分路径
Γn 和Γ′n (n = 1, 2, …, 5),其中R为积分路径半径,这与Eason[14]采用的积分路径一致。根据留数定理得到:
(∫Γ+i∞Γ−i∞+∫Γ1+∫Γ2+∫Γ3+∫Γ4+∫Γ5+∫Γ′1+∫Γ′2+∫Γ′3+∫Γ′4){ˆ˜uxest,ˆ˜uzest}=2πi Res{ˆ˜uxest,ˆ˜uzest} (16) 对于积分路径Γ5,由于R→∞,根据Jordan引理积分为0[14]。将式(14)~(15)代入式(16),引入变量ε=1/η对积分形式进行简化,得到水平位移和垂向位移的时域表达式分别为:
˜ux(ω,0,t)=P0cs˜H(ω)G[2iχr1+2χr2χr3˙kr(εr)Tr(t) + i4π∫1θχ1ˉχ2χ3(χ1−2ε2)χ41+16ε4ˉχ22χ23T(t)dε] (17) ˜uz(ω,0,t)=P0cs˜H(ω)G[sgn(ω)2ˉχr2(2ε2r−χr1)|εr|˙kr(εr)Tr(t)+2sgn(ω)π ∫θ0χ2(χ1−2ε2)|ε|(χ21+4ε2χ2χ3)T(t)dε+ 8sgn(ω)π ∫1θ|ε|ˉχ22χ3(χ1−2ε2)χ41+16ε4ˉχ22χ23T(t)dε] (18) 式中:T(t)为时间相关项。T(t)的具体表达式为:
T(t)=T0(α)−T0(β),T0(α)=ε{csω[e−αt−cos(csωt/ε)]+αεsin(csωt/ε)}(csω)2+α2ε2 (19) 式中:
χ1=2ε2−1 ,χ2=√θ2−ε2 ,ˉχ2=√ε2−θ2 ,χ3=√1−ε2 ,c=χ2/|ε| ˉc=ˉχ2/|ε| ,d=χ3/|ε| ,ˉd=ˉχ3/|ε| ,kr(ε)=(2ε2−1)2−4ε2√ε2−θ2√ε2−1 ,˙kr 为kr 关于ε的偏导,cr 、dr 、χrj (j=1, 2, 3)和Tr 表示ε=εr 时系数的取值,εr 为方程kr(ε)=0 解的虚部。将式(17)~(18)代入式(12)~(13),得到水平和垂直位移的积分表达式分别为:
ux(x,0,t)=P0cs2πG∫+∞−∞[2iχr1+2χr2χr3˙kr(εr)Tr(t)+i4π∫1θχ1ˉχ2χ3(χ1−2ε2)χ41+16ε4ˉχ22χ23T(t)dε]˜H(ω)eiωxdω (20) uz(x,0,t)=P0cs2πG∫+∞−∞[2ˉχr2(χr1−2ε2r)|εr|˙kr(εr)Tr(t)+2π∫θ0χ2(χ1−2ε2)|ε|(χ21+4ε2χ2χ3)T(t)dε+8π∫1θ|ε|ˉχ22χ3(χ1−2ε2)χ41+16ε4ˉχ22χ3T(t)dε]sgn(ω)˜H(ω)eiωxdω (21) 尽管式(20)~(21)为双重积分解,但可通过交换积分次序,优先计算Fourier变换部分可简化为一重积分形式,这一过程中要将Fourier变换基函数展开:
eiωx=cos(ωx)+i sin(ωx) (22) 对式(3)进行Fourier变换,可得:
˜H(ω)=[2sin(ωl/ωl22)]/[2sin(ωl/ωl22)]ωω (23) 将式(22)~(23)代入式(20)~(21),利用函数奇偶性对Fourier反变换积分部分进行化简,通过观察可知,积分公式中存在以下6个一次积分:
I1(α,x,ε)=∫+∞02cos(ωl/2+ωx)(csω)2+α2ε2dω=e−αε|l+2x|/αε|l+2x|(2cs)(2cs)πcsαε (24) I2(α,x,t,ε)=∫+∞0cos(ωl/2+ωx+csωt/ε)(csω)2+α2ε2dω=e−α|2cst+(l+2x)ε|/α|2cst+(l+2x)ε|(2cs)(2cs)π2csαε (25) I3(α,x,t,ε)=∫+∞0sin(ωl/2+ωx+csωt/ε)ω[(csω)2+α2ε2]dω=e−α|2cst+(l+2x)ε|/α|2cst+(l+2x)ε|(4cs)(4cs)πα2ε2sinh(14|2αt+(l+2x)αεcs|) (26) I4(α,x,ε)=∫+∞02sin(ωl/2+ωx)(csω)2+α2ε2dω=√π(l+2x)csαε|l+2x|G2,11,3((l+2x)2α2ε216c2s|1212,12,0) (27) I5(α,x,t,ε)=∫+∞02sin(ωl/2+ωx)(csω)2+α2ε2dω=√π(2cst+lε+2xε)2csαε|2cst+lε+2xε|G2,11,3((2cst+lε+2xε)2α2ε216c2s|1212,12,0) (28) I6(α,x,t,ε)=∫+∞02sin(ωl/2+ωx)sin(csωt/ε)(csω)2+α2ε2dω=12α2ε2[2ln(|2cst+lε+2xε||−2cst+lε+2xε|)−√πG2,11,3((−2cst+lε+2xε)2α216c2s|00,0,12)+√πG2,11,3((2cst+lε+2xε)2α216c2s|00,0,12)] (29) 上述一次积分可组成式(20)~(21)中的Fourier反变换积分部分:
K1(α,ε)=ε{cse−αt[I1(α,x,ε)−I1(α,−x,ε)]−cs[I2(α,−x,−t,ε)−I2(α,x,−t,ε)+I2(α,−x,t,ε)−I2(α,x,t,ε)]+αε[−I3(α,−x,−t,ε)+I3(α,x,−t,ε)+I3(α,−x,t,ε)−I3(α,x,t,ε)]} (30) K2(α,ε)=ε{cse−αt[I4(α,x,ε)+I4(α,−x,ε)]−cs[I5(α,−x,−t,ε)+I5(α,x,−t,ε)+I5(α,−x,t,ε)+I5(α,x,t,ε)]+αε[−I6(α,−x,−t,ε)+I6(α,x,t,ε)]} (31) 将上述K1和K2代入式(20)~(21),得到积分简化形式:
ux(x,0,t)=P0cs2πμ{−2χr1+2χr2χr3˙kr(εr)[K1(α,εr)−K1(β,εr)]−4π∫1θχ1ˉχ2χ3(χ1−2ε2)χ41+16ε4ˉχ22χ23[K1(α,ε)−K1(β,ε)]dε} (32) uz(x,0,t)=P0cs2πμ{2ˉχr2(χr1−2ε2r)|εr|˙kr(εr)[K2(α,εr)−K2(β,εr)]+2π∫θ0χ2(χ1−2ε2)|ε|(χ21+4ε2χ2χ3)[K2(α,ε)−K2(β,ε)]dε+8π∫1θ|ε|ˉχ22χ3(χ1−2ε2)χ41+16ε4ˉχ22χ3[K2(α,ε)−K2(β,ε)]dε} (33) 采用自适应积分方法[26]对式(32)~(33)进行数值积分,得到爆破冲击荷载下隧道爆破位移场分布,进一步通过数值差分可得到隧道爆破速度场分布。
2. 隧道爆破现场试验与数值模拟
2.1 工程概况及现场测试
为了验证理论解析可靠性,依托龙南隧道工程,进行爆破现场试验。龙南隧道位于中国江西省赣州市龙南县,是赣深高铁线路上的主要隧道之一。爆破振动监测现场试验段距离前方掌子面163~213 m,围岩为泥盆系老虎坳组石英砂岩,属Ⅳ级围岩。该段采用三台阶爆破开挖法进行爆破施工,上、中、下台阶分别高5.0、3.5和2.5 m,上、中台阶的纵距分别为8和30 m,二衬中止里程距掌子面80 m。爆破中采用2#岩石乳化炸药和非电毫秒微差雷管分段起爆,炮孔直径为40 mm,循环进尺控制在2.5 m左右。炮孔布置见图4,上台阶采用直眼掏槽方式,炮眼深度为2.5 m,掏槽眼超深0.2 m,辅助眼间距为0.6 m,周边眼间距为0.5~0.6 m,中、下台阶设置周边眼与多排辅助眼,爆破采用一次点火、三台阶同时起爆的方式,最大段别为15段,最大单段药量为19.8 kg。
为了得到隧道表面围岩振速衰减规律,采用TC-4850爆破测振仪对爆破施工进行了监测。该型测振仪由传感器和测振仪构成,能多次触发并存储数据。根据现场实际工况,在考虑安全前提下将测点布置于隧道拱脚处,共布置6个监测点(1#~6#),如图5所示,其中测点均位于已施加二衬段,每个监测点相距10 m,监测点1#距离掌子面163 m。
2.2 数值模拟
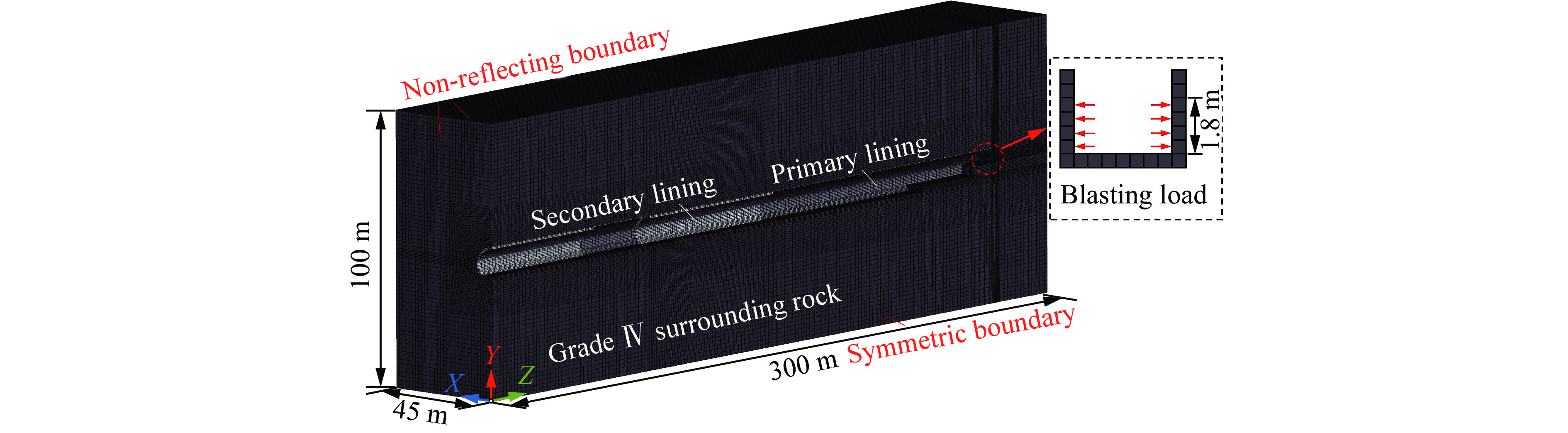
采用ANSYS/LS-DYNA建立现场爆破动力数值模型。由于掏槽爆破引起的爆破振动最大[27],因此在数值模型中只考虑掏槽爆破作用。根据隧道结构的对称性,将模型建为1/2模型,数值模型整体尺寸为300 m×75 m×75 m。由于埋深较小,在计算时忽略地应力的影响。模型单元采用八节点SOLID164实体单元,采用Lagrange网格划分。为了准确反映应力波的传递,单元网格尺寸需控制在应力波最小波长的1/6~1/12,一般R波的波长最小。根据波速与波长和频率的关系,经过计算在掏槽孔弹性边界处网格尺寸应不大于35 cm,其余部位依次增大网格尺寸来缩短计算时间。由于监测点距离隧道掌子面达163 m,模型需要较大范围才能包括所有监测点,这就导致运算时间会较长,因此,采取等效爆破荷载施加于弹性边界的方法代替炸药模型,可大大缩减计算时间。最终模型以及施加荷载位置如图6所示。
爆破荷载形式取图2所示时间函数,爆破荷载峰值可通过计算得到。不耦合装药时,炮孔处初始平均压力Pb[28]为:
Pb=ρeD22(γe+1)(dcdb)2γe (34) 式中:ρe为炸药密度,D为爆速,γe为炸药等熵指数,dc为装药直径,db为炮孔直径。炸药等熵指数γe取3[28]。考虑群孔起爆、冲击波与应力波衰减,等效施加于弹性边界上的爆破荷载Pe[28]为:
Pe=kPb(r0r1)2+ν1−ν(r1r2)2−ν1−ν (35) 式中:k为掏槽孔群孔起爆时的影响系数,其值与掏槽孔的数量和分布有关,本文中取2;r0为炮孔半径;r1为粉碎区半径,为装药半径的3~5倍;r2为破裂区半径,为装药半径的10~15倍;ν为岩石的泊松比。
隧道结构包含初衬、二衬,忽略围岩中节理裂隙等复杂构造,将三者看作弹塑性均匀等效介质,采用双向随动硬化本构模型(*MAT_PLASTIC_KINEMATIC),相关参数见表1。
表 1 数值模型材料参数Table 1. Material parameters of the numerical model结构 密度/(kg·m−3) 弹性模量/GPa 泊松比 屈服强度/MPa 切线模量/MPa Ⅳ级围岩 2300 4.00 0.31 5.0 2.2 初衬 2200 23.00 0.25 4.2 2.5 二衬 2500 32.00 0.20 6.0 2.4 采用2#岩石乳化炸药进行隧道爆破,炸药密度ρe为1.24 g/cm3,爆速D为3200 m/s,代入式(34)~(35)计算得到等效到弹性边界上的Pe=33.28 MPa。一般炸药爆炸压力作用时间在10 ms左右,设炸药爆炸压力上升段时间为1 ms,得到爆炸荷载函数表达式为:
P(t)=142.78(e−814.73t−e−1552.61t) (36) 式中:P的单位为MPa,t的单位为s。
2.3 数值模拟与理论结果验证
参照现场试验中的测试点位在数值模型中选取相同点位进行对比,结果如图7所示。依图中数据可知,模型中各监测点三向峰值振速均与现场实测较吻合,整体趋势一致。数值模拟波形与现场测试波形较接近,尤其是振速峰值,在振动频率有所差异,原因在于监测点较远,数值模型将岩土体看作均一介质导致波阻抗处处相等,在远场相对于实际场地来说应力波会更难到达,因此在结果上存在一定误差。
去除数值模型中的隧道结构,计算得到隧道围岩爆破动力响应结果。理论计算参数通过表1数据以及现场实际工况选取,P波、S波、R波波速由方程:
cp=√λ+2Gρ,cs=√Gρ,(2−c2rc2s)2−4√1−c2rc2p√1−c2rc2s=0 (37) 计算,分别为1552.61、814.73和756.86 cm/s,式中ρ为岩体密度,cr为R波波速。为计算简便,将变量无量纲化:x*=2x/l,t*=2cst/l,u*=πGu/(P0cs),v*=πGv/(P0cs),其中v为振速。由于在理论模型中荷载直接作用在隧道面上,因此爆破荷载需进一步衰减,得到理论计算荷载函数:
P(t)=38.48(e−814.73t−e−1552.61t) (38) 式中:P的单位为MPa,t的单位为s。
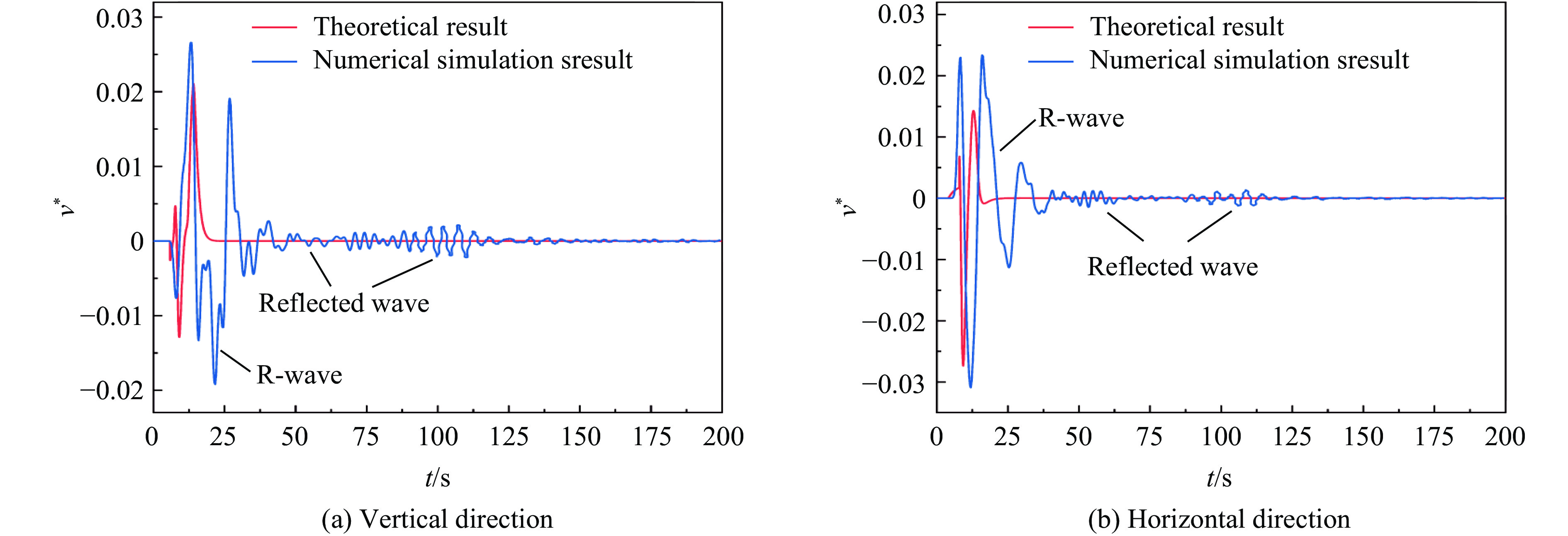
由于在2.2节中理论解析是考虑平面应变条件下的积分解,无法体现R波在传播过程中的衰减特征[14],因此在使用理论解时去掉R波项,仅计算包含P波与S波项。为了保证波形R波成分少,选取对称面距掌子面10 m处的质点振速波形进行对比,如图8所示。从图8可以看到,解析结果与数值模拟波形、振速峰值相近,由于S波和R波波速接近,S波到达后理论与数值模拟波形出现较大差异,此外,数值模拟波形更复杂,包含有其他自由面的反射波,但对于分析P波、S波振速而言,并未有影响,该理论解析适用于计算隧道表面爆破地震波。
3. 隧道爆破地震波传播特征
3.1 爆破地震波波场分离方法
在实际工程中,隧道爆破振动波形往往是耦合、复杂的,需要将爆破地震波进行分离。由于P波、S波与R波在同一介质中波速大小有差异,波场分离一般基于时域进行,在时间上进行波场分离主要是采用地震波偏振分析结合运动学特征进行的[20]。Radon变换法是一种经典函数投影变换方法,常用于地震波信噪分离、波形分解与重构,根据积分路径不同可分为直线型(τ-p变换)、抛物线型(τ-q变换)和双曲线型等形式[29],本文中将采用τ-p变换进行波场分离。τ-p变换法是基于时间正交分解特性将地震记录信号由x-t空间转变为τ-p空间,并沿某一射线路径进行叠加,其实质为一种倾斜叠加。
对于地震波信号u(t, x)进行τ-p正逆变换的关系式为:
ˉu(τ,p)=∫xu(t,x)dx,u(x,t)=∫pˉu(τ,p)dx (39) 式中:t=τ+px,x为偏移距,t为时间,u(t, x)为输入地震波信号,
ˉu(τ,p) 为τ-p正变换信号,τ为截距时间,p为慢度,p=dt/dx。在实际工程中,振动传感器接收的爆破地震波信号是离散的,振动波形的完整性取决于采样频率。对于离散信号u(ti, xi)有τ-p正逆变换:
ˉu(τ,pk)=Nx∑i=1u(ti=τ+pxi, xi), u(t,xi)=Np∑k=1ˉu(τk=t−pkx, pk) (40) 式中:Nx和Np分别为离散信号道数和τ-p变换后p的点数;i=1, 2, …, Nx;k=1, 2, …, Np。
根据式(40)可知,在x-t域内为一倾斜射线时,在τ-p域内为一点;反之,在τ-p域内为一倾斜射线时,在x-t域内即为一点,如图9所示。
为了保证地震波信号反变换分辨率,一般考虑使用频率域τ-p变换,即对式(40)作Fourier变换:
ˉU(ω,pk)=Nx∑i=1U(ti,xi)eiωpkxi,U(ω,xi)=Np∑k=1ˉU(ω,pk)e−iωpkxi (41) 将上述形式表达为矩阵形式:
ˉU=LU,U=LHˉU (42) 式中:
L=|eiωpkxi| ,LH=|e−iωpkxi| 。需要注意的是,采样点数不合适将造成L和LH算子的不适定性。一般线性反变换分辨率低且不具备保幅性,需要精度高的反演方法。Trad[30]提出基于稀疏反演的高分辨率Radon变换(HRT),使用Gauss-Cauchy模型建立反演误差目标函数:J=1σ2n‖ (43) 式中:σn为方差,
\bar \alpha 和\bar \beta 为分布系数。为了保证反变换分辨率及保幅性,误差函数J应取最小值,经过迭代加权后最终得到反演信号:{\boldsymbol{U}} = {\left[ {{{\boldsymbol{L}}^{\text{H}}}{\boldsymbol{L}} + {\boldsymbol{Q}}\left( {\boldsymbol{U}} \right)} \right]^{ - 1}}{{\boldsymbol{L}}^{\text{H}}}{\boldsymbol{\bar U}} (44) 式中:Q(U)为阻尼相关误差项。由此经过线性HRT变换后分离出的波形可保证分离波形的真实幅值,可用于爆破地震波波场分离。
隧道爆破地震波在传播路径上会遇到不连续面、不均匀介质等,发生反射与折射,使得在隧道内部接收到的波形变得复杂,是由P波、S波、PP波、PS波、R波等组成的耦合波。一般而言,直达P波、S波与R波引发的质点振动最大,在波场分离时需过滤反射波和折射波。
假定第1个监测点偏移距为x1,每个监测点之间的间距为∆h,则第i个监测点偏移距为xi=x1+(i−1)∆h,直达波到达时间t=xi/cw,其中cw(w = P, S, R)为视波速。基于不同直达波具有不同慢度,可以在τ-p空间中选取合适的p滤波框来提取各类波,再采用τ-p逆变换得到x-t空间的波形。由于S波与R波波速较接近,通过HRT难以分离S波与R波,因此在此方法中只分离P波和R波,具体波场分离过程如图10所示。
3.2 波场分离结果及可靠性验证
在有限元数值模型中选取拱顶对称面处节点位移波形进行波场分离,如图10所示。数值计算中采用的视窗为0.4 s,采样间隔为0.4 ms,以偏移距为10 m、道距为1 m提取隧道表面质点位移作为输入位移,得到垂直方向与水平方向位移波列如图11(a)和12(a)所示。根据偏振关系,从图11(a)和12(a)可以看到,直达P波和S波呈同相轴走向,反射波则走向相反,由于爆破地震波数据来源于数值模拟,与实际相比,反射波和折射波成分较少且幅值较小,直达波是造成质点振动的主要来源。可见,只分离直达波是可行的。按照图10中的波场分离流程,最终得到垂直位移Radon谱以及分离波形见图11,水平位移波场分离见图12。分离波形清晰且单一,多次波压制效果较好。将输入位移做HRT正反变换,选取输入位移与反演位移进行对比,如图13所示。反演位移峰值Upp与输入位移峰值upp空间分布趋势一致,输入位移峰值稍大于反演位移峰值,两者的比值为1.05~1.18,爆心距较大时水平位移反演误差波动大,而爆心距较小时垂直位移反演波动大。对比10 m处的质点位移波形,可以看到反演波形U与输入波形u基本重合,表明该波场分离方法具有较高精度,适用于隧道爆破地震波波场分离。
3.3 隧道表面爆破地震波的传播规律
理论解析仅能得到P波和S波波场,而HRT波场分离方法仅能得到P波和R波波场,所以在分析隧道表面爆破地震波的传播规律时需要先通过波场分离得到P波和R波波场,再结合理论解析P波和S波的关系得到S波波场,最后综合三波场提出隧道爆破分区。
3.3.1 隧道表面P波与R波传播特征
根据地震波理论可知在几何扩散的影响下,地震波大小与传播距离呈负指数关系,体波在介质中衰减速率明显快于面波,因此考虑爆心距r的影响,采用以下公式描述隧道爆破地震波振速衰减规律:
{v_{{\text{pp}},w}} = k{r^{ - {\alpha _w}}} (45) 式中:vpp,w为质点峰值振速,w表示不同波型,w = P, S, R;r为爆心距,对于隧道表面即为坐标x;k为爆破荷载及场地相关系数;αw为衰减系数。
根据波场分离结果,结合式(45)对隧道壁面围岩质点振速进行分析,得到隧道表面爆破地震波传播规律,如图14所示。可以看到,随着爆心距增大,垂直方向和水平方向P波与R波振幅均呈衰减趋势,衰减系数表现为αP>αR,符合在自由面P波衰减快于面波的特征。
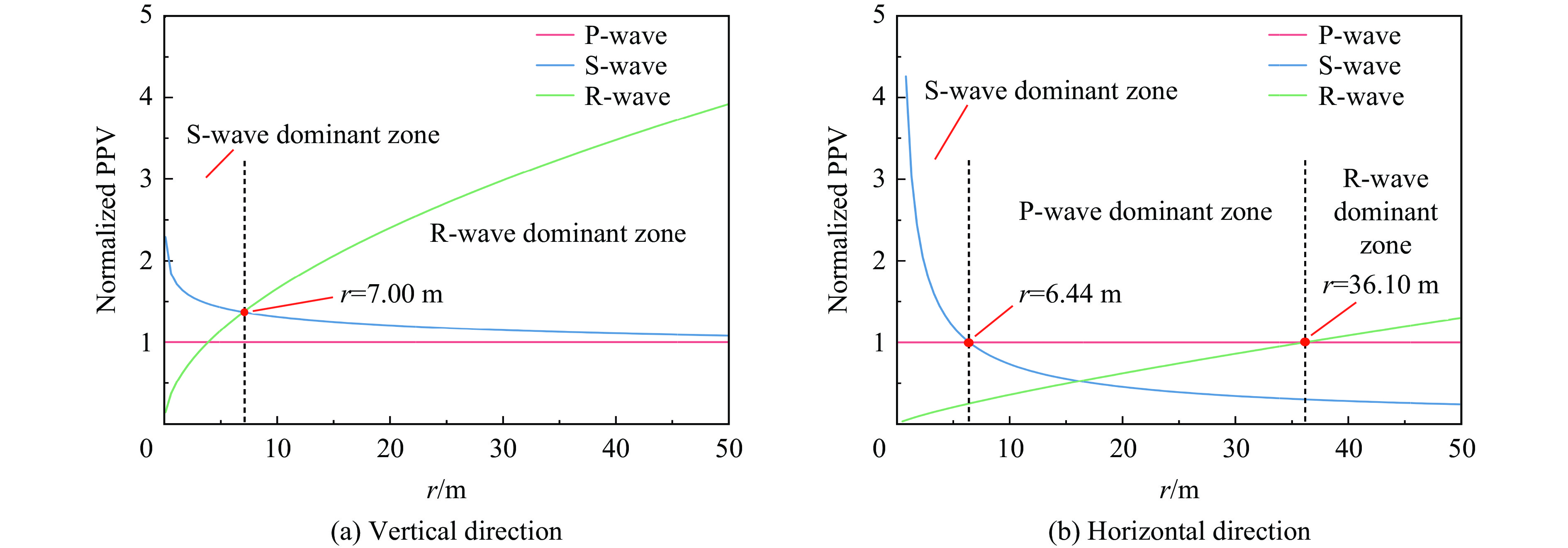
在垂直方向,P波衰减系数和场地系数均大于R波的。P波振速衰减曲线与R波振速衰减曲线相交于r=3.79 m处,表明在r<3.79 m处P波和R波在掌子面附近迅速发育,随着爆心距增大,P波大幅衰减,与R波振速的差值增大,在隧道爆破影响范围内基本由R波控制围岩表面质点垂向振动峰值。
在水平方向,P波的衰减系数和场地系数均大于R波的。P波振速衰减曲线与R波振速衰减曲线相交于r=36.10 m处,表明在r<36.10 m范围内水平向P波主导质点振动,在r>36.10 m范围内水平向R波主导质点振动。
对比P波与R波的传播规律,定义同一方向P波与R波的峰值振速之比为IPR,得到垂向、水平向峰值振速比IPR分布规律如图15所示。图中峰值振速比IPR与爆心距呈负指数衰减,随着爆心距增大,峰值振速比接近于零,表明在较远处P波作用可忽略不计。另外,等值线与两振速比分布曲线的交点与图14中的交点相对应。对比垂直方向与水平方向峰值振速比IPR,在0~100 m范围内水平向振速比始终大于垂直向振速比,但衰减系数相反,采用图中公式可得到两峰值振速比分布曲线的交点。
对比P波与R波垂直方向峰值振速v⊥与水平方向峰值振速v∥,得到峰值振速比的分布如图16所示。随着爆心距增大,P波垂直-水平峰值振速比呈增大趋势,在掌子面附近最小,接近零,说明隧道表面P波以水平振动为主,在较远处逐渐转变成垂直振动,这也就导致隧道表面主要受P波轴向拉伸作用,形成拉裂缝。R波随着爆心距增大呈波动下降趋势,在掌子面附近峰值振速比大于1.60,在较远处接近于1,说明隧道表面R波以垂向振动为主,随着爆心距增大逐渐转向水平振动。
3.3.2 隧道表面S波传播特征
基于隧道表面爆破地震波产生机制的理论解,取荷载作用长度l=1.8 m,将表1中的岩体参数以及爆破压力函数式(38)代入式(32)~(33),计算得到隧道表面质点位移时程曲线。对质点位移进行求导,得到振速曲线,并提取S波的振速幅值,采用式(45)得到S波的衰减曲线,如图17所示,其中计算参数均已无量纲化,用“*”上标表示。图中S波振速呈指数衰减趋势,垂向S波衰减明显慢于水平向。当无量纲爆心距r*<4.74时,垂向S波小于水平向,在靠近掌子面处S波以水平振动为主;当无量纲爆心距r*>4.74时,垂向S波大于水平向,S波主振方向转变为垂向,且随着爆心距增大,S波水平向振速占比减小。
P波与S波具有相似的衰减特征,提取理论解中P波峰值振速,得到P波与S波峰值振速比IPS分布规律,如图18所示。随着爆心距增大,水平向IPS表现出增大的趋势,靠近掌子面时小于1,远离掌子面处大于1,表明隧道爆破激发水平向P波成分低于S波,在远处P波成分高于S波,这是S波远离爆源后衰减迅速导致的必然结果。垂向IPS呈现先增大后减小的趋势,分界点位于r*=55.56,但整体比值接近于1,表明垂向P波、S波成分接近,垂向P波成分始终低于S波。
3.4 隧道表面爆破地震波分区特征
根据隧道表面爆破地震波传播特征分析(见图14、17),隧道表面爆破P波、S波与R波主导不同区域的爆破振动响应。由于3类波的动力作用特征不同,沿隧道轴向呈现出不同的结构振动形式与破坏特征。因此,以3类波主导作用区域为依据划分爆破分区具有工程意义。
为统一描述隧道表面爆破地震波的传播规律,以P波振速为基准对S波、R波爆破振速进行归一化处理,其中无量纲爆心距转变为实际爆心距。联立图15和18,分别得到归一化水平与垂直方向爆破地震波作用范围,如图19所示。
根据图19所示波的作用范围,可以看出垂直方向由S波和R波主导,水平方向由S波、P波和R波主导。随着爆心距的增大,S波迅速衰减,R波逐渐发育,P波在水平和垂直方向均占主要成分。
同时考虑水平和垂直方向振动大小,以垂直P波峰值振速为基准,得到归一化后隧道表面爆破地震波作用分区如图20所示,得到在当前工况下P波、S波和R波的作用分区为:(1) 0<r<6.44 m为S波主导区,属于爆破近区,主导波型为水平S波;(2) 6.44 m<r<21.23 m为P波主导区,属于爆破中区,主导波型为水平P波;(3) r>21.23 m为R波主导区,属于爆破远区,主导波型为垂直R波。
在实际隧道爆破工程中,爆破药量是影响爆破振动大小的重要因素,同时也决定爆破压力的大小,因此在描述隧道表面爆破地震波作用分区时,需考虑单段最大药量Q,采用比例距离
\bar r 进行划定[1]:\bar r = {r \mathord{\left/ {\vphantom {r {\sqrt[3]{Q}}}} \right. } {\sqrt[3]{Q}}} (46) 采用上述波场分离及理论解析方法,选取l=2.4, 3.0 m工况进行分析。根据工程概况可知,当l=1.8 m时,Q=19.8 kg,在相同爆破开挖方案前提下,装药长度l与炸药量Q成正比。因此,当l=2.4, 3.0 m时,相对应的炸药量Q=26.4, 33.0 kg。3种工况下,三波作用分界点与Q的关系如图21所示。图中波作用分界点与单段最大药量呈线性相关,其中P波、S波分界
{\bar r_{{\text{PS}}}} 和P波、R波分界{\bar r_{{\text{PR}}}} 均与Q呈负相关,通过图21中的公式可得到任意单段药量下隧道表面爆破地震波的分区范围,用于指导施工。4. 结 论
通过理论解析和数值模拟,结合HRT波场分离方法,研究了隧道表面爆破地震波的产生机制及传播特征,得到了以下结论。
(1)提出了隧道表面爆破振动平面应变理论模型,采用Laplace-Fourier双重积分变换得到简化的隧道表面爆破振动场积分表达式,该解适用于揭示隧道表面爆破地震波的产生机制。
(2)隧道爆破激发P波与S波,在自由面影响下,R波迅速发育。随着爆心距增大,3类波均呈现指数衰减特征,其中P波、S波衰减明显快于R波,S波衰减最快。
(3)不同方向隧道表面爆破地震波主要成分不同。随着爆心距增大,垂直方向主要成分由S波转变为R波,水平方向主要成分由S波转变为P波,P波转变为R波。
(4)提出以爆破振动主导波型进行隧道爆破分区。在本文工况下隧道爆破应力波作用分区为:0<r<6.44 m为爆破近区,主导波型为水平S波;6.44 m<r<21.23 m为爆破中区,主导波型为水平P波;r>21.23 m为爆破远区,主导波型为垂直R波。
(5)爆破分区分界点比例距离与单段最大药量呈线性关系,可通过爆破药量得到隧道爆破分区位置,用于隧道安全稳定性分析。
-
表 1 试验用93W4Ni3Fe合金破片材料基本力学性能
Table 1. Basic mechanical properties of missile target materials for test
材料 ρ/(g·cm−3) E/GPa σy/MPa σs/MPa δ/% HRC 93W4Ni3Fe 17.7 365 731 955 19~22 29 表 2 试验用22SiMn2TiB靶板材料基本力学性能
Table 2. Basic mechanical properties of 22SiMn2TiB target material for test
材料 h/mm 试验编号 ρ/( g·cm−3) E/GPa σy/MPa σs/MPa δ/% HRC 22SiMn2TiB 6 Test 1 7.781 197.203 1083.0 1516.5 12.60 46.8 Test 2 197.342 1068.5 1516.9 12.64 46.4 Test 3 196.983 1081.6 1514.3 12.16 46.5 Test 4 186.685 1080.4 1508.8 12.92 46.4 8 Test 1 7.785 186.746 1110.2 1569.9 14.40 46.2 Test 2 188.351 1080.5 1563.1 14.52 46.2 Test 3 192.603 1113.7 1574.1 15.92 46.0 Test 4 190.662 1072.4 1563.3 14.84 46.8 平均 7.783 192.070 1086.3 1540.9 13.75 46.4 表 3 钨球撞击22SiMn2TiB钢板的极限贯穿速度
Table 3. Expermental results of tungsten balls impacting 22SiMn2TiB steel plates
h/mm d/mm θ/(°) v/(m·s−1) 是否穿透 v50/( m·s−1) h/mm d/mm θ/(°) v/(m·s-1) 是否穿透 v50/( m·s-1) 6.23 8.00 0 434.15 否 480.80 8.27 0 460.83 否 615.47 566.04 是 561.80 否 518.13 是 569.26 否 466.56 否 608.52 否 495.05 是 622.41 是 20 574.71 是 485.85 607.45 是 483.09 否 8 20 728.16 是 624.52 488.60 是 702.58 是 40 468.02 否 619.68 692.84 是 636.94 是 614.75 否 602.41 否 560.75 否 6.23 11.06 0 361.01 否 376.91 563.27 否 371.75 否 634.29 是 369.46 否 40 649.35 否 798.02 406.50 是 755.67 否 20 427.96 是 389.13 821.92 是 355.03 否 808.63 是 404.31 是 740.74 否 363.2 否 787.40 否 390.63 是 8.27 11.06 0 459.49 是 444.00 40 574.71 是 481.74 428.57 否 460.12 否 473.19 是 503.36 是 20 549.45 是 475.87 452.49 否 461.54 否 510.20 是 490.20 是 40 606.06 是 575.6 561.80 否 589.39 是 表 4 弹靶材料Johnson-Cook模型参数
Table 4. Johnson-Cook model parameters of 22SiMn2TiB steel and 93W4Ni3Fe
材料 ρ/(g·cm−3) G/GPa A/GPa B/GPa C M n 22SiMn2TiB 7.78 81.8 1.086 0.51 0.014 1.03 0.26 93W4Ni3Fe 17.7 160 0.731 1.67 0.03 0.82 0.91 材料 c/(m·s−1) S1 S2 S3 γ0 A 22SiMn2TiB 4600 1.730 0 0 1.67 0.46 93W4Ni3Fe 4046 1.268 0 0 1.58 0.46 表 6 撞击靶板贯穿极限速度的数值模拟与试验值对比
Table 6. Comparison of ultimate penetration velocity between simulation and test
θ/(°) 钨球初速/(m·s−1) 是否穿透 v50/(m·s−1) 数值模拟 试验 相对误差/% 0 480 是 475 480.8 1.14 470 否 20 490 是 485 485.8 0.16 480 否 40 625 是 619.5 619.6 0.02 614 否 表 7 钨球撞击靶板过程能量变化
Table 7. Energy change during tungsten ball impacting target plate
θ/(°) E0/kJ E1/kJ E2/kJ (ΔE1-0/E0)/% (ΔE2-1/E0)/% 0 2.72 1.03 0.74 62.13 10.51 10 2.78 1.13 0.72 59.35 14.86 20 2.84 1.20 0.58 57.75 21.83 30 3.54 1.54 1.05 56.50 13.84 40 4.62 2.32 1.03 49.78 27.92 50 6.39 4.26 2.05 33.33 34.59 表 8 撞击靶板贯穿极限速度的试验与理论值对比
Table 8. Comparison of ultimate penetration velocity between experimental and theoretical value
钨球直径/mm 靶板厚度/mm 着角/(°) v50/(m·s−1) 误差/% 试验 理论 8.00 6.23 20 485.85 474.03 −3.69 11.06 8.27 40 575.60 592.01 2.85 6.84 6.75 0 610.00 604.94 −0.83 8.12 6.75 0 520.30 513.08 −1.39 表 9 撞击靶板贯穿极限速度的计算值与理论值对比
Table 9. Comparison of ultimate penetration velocity between numberical and theoretical value
钨球直径/mm 靶板厚度/mm 着角/(°) v50/(m·s−1) 误差/% 计算 理论 8.00 4.00 0 309.50 309.89 0.12 8.00 3.00 0 195.00 235.11 20.57 8.00 2.00 0 193.00 159.30 17.46 8.00 6.00 10 480.00 464.26 −3.28 8.00 6.00 30 542.00 526.51 −2.86 8.00 6.00 50 730.00 704.89 −3.44 -
[1] AWERBUCH J, BODNER S R . Analysis of the mechanics of perforation of projectiles in metallic plates [J]. International Journal of Solids & Structures, 1974, 10(6): 671–684. [2] 陈志斌, 刘志刚. 球形弹垂直碰撞金属靶板的实验研究 [J]. 弹道学报, 1991, 7(1): 66–70.CHEN Z B, LIU Z G. Experimental investigation on the mental target by normal impact of spherical shell [J]. Journal of Ballistics, 1991, 7(1): 66–70. [3] 任杰, 徐豫新, 王树山. 超高强度平头圆柱形弹体对低碳合金钢板的高速撞击实验 [J]. 爆炸与冲击, 2017, 37(4): 629–636. DOI: 10.11883/1001-1455(2017)04-0629-08.REN J, XU Y X, WANG S S. High-speed impact of low-carbon alloy steel plates by ultra-high strength blunt projectiles [J]. Explosion and Shock Waves, 2017, 37(4): 629–636. DOI: 10.11883/1001-1455(2017)04-0629-08. [4] 陈材, 石全, 尤志锋, 等. 预制破片侵彻靶板临界跳飞角变化规律 [J]. 火力与指挥控制, 2021, 46(5): 29–34. DOI: 10.3969/j.issn.1002-0640.2021.05.006.CHEN C, SHI Q, YOU Z F, et al. Change law of critical ricochet angle of prefabricated fragment penetrating target plate [J]. Fire Control & Command Control, 2021, 46(5): 29–34. DOI: 10.3969/j.issn.1002-0640.2021.05.006. [5] 姚熊亮, 王治, 叶墡君, 等. 球头弹体侵彻舰船板架加强筋时的攻角变化简化理论模型 [J]. 爆炸与冲击, 2021, 41(3): 033301. DOI: 10.11883/bzycj-2020-0092.YAO X L, WANG Z, YE S J, et al. A simplified theoretical model for attack angle change of a hemispherically-nosed projectile while penetrating the stiffener of a ship plate frame [J]. Explosion and Shock Waves, 2021, 41(3): 033301. DOI: 10.11883/bzycj-2020-0092. [6] 午新民. 钨合金球体对有限厚靶板侵彻的理论与试验研究[D]. 北京: 北京理工大学, 1999. [7] 胡邓平, 赵利平, 伍先明, 等. 616装甲防弹钢动态冲击下的性能研究 [J]. 兵器材料科学与工程, 2017, 40(2): 96–99. DOI: 10.19822/j.cnki.1671-6329.20210032.HU D P, ZHAO L P, WU X M, et al. Study on the performance of 616 armored ballistic steel under dynamic impact [J]. Ordnance Material Science and Engineering, 2017, 40(2): 96–99. DOI: 10.19822/j.cnki.1671-6329.20210032. [8] XU Y X, HAN X G, ZHAO X X, et al. Experimentation research on failure behavior of tungsten alloy penetrating low carbon steel plate at high velocity [J]. Rare Metal Materials and Engineering, 2016, 45(1): 122–126. [9] 赵荣贵, 陈林恒, 左秀荣. 超高强度装甲钢抗弹失效机理研究 [J]. 宽厚板, 2020, 26(5): 11–14. DOI: 10.3969/j.issn.1009-7864.2020.05.003.ZHAO R G, CHEN L H, ZUO X R. Research on the ballistic failure mechanism of ultra-high strength armored steel [J]. Wide and Heavy Plate, 2020, 26(5): 11–14. DOI: 10.3969/j.issn.1009-7864.2020.05.003. [10] 程瑶, 赵太勇, 陈智刚, 等. 低中速钨球变形与速度关系计算模型 [J]. 爆破器材, 2019, 48(5): 52–56. DOI: 10.3969/j.issn.1001-8352.2019.05.010.CHENG Y, ZHAO T Y, CHEN Z G, et al. Calculation model of the relationship between deformation and velocity of low and medium speed tungsten ball [J]. Explosive Materials, 2019, 48(5): 52–56. DOI: 10.3969/j.issn.1001-8352.2019.05.010. [11] 曹柏桢, 凌玉崑, 蒋浩征, 等. 飞航导弹战斗部与引信[M]. 北京: 中国宇航出版社, 1995: 140-141. [12] JOHNSON G R, COOK W H. A constitutive model and data for metals subjected to large strains, high strain-rates and high temperatures [C]// Proceeding of the Seventh International Symposium on Ballistics. Hague, Netherlands, 1983: 541−547. [13] 刘铁, 史洪刚, 程新, 等. 钨合金帽型试样的绝热剪切带数值模拟研究 [J]. 兵器材料科学与工程, 2008(2): 75–79. DOI: 10.3969/j.issn.1004-244X.2008.02.020.LIU T, SHI H G, CHENG X, et al. Numerical simulations for adiabatic shear bands of WHA in hat specimen [J]. Ordnance Material Science and Engineering, 2008(2): 75–79. DOI: 10.3969/j.issn.1004-244X.2008.02.020. 期刊类型引用(4)
1. 徐平,侯伟琦,乔世范,董辉,罗晓光,赵炜. 近岸水下钻孔爆破能量波传播规律及对水工构筑物毁伤效应研究. 振动与冲击. 2025(06): 144-156+183 .  百度学术
百度学术2. 李时林,何如,徐昊,孙仁祥,刘喜. 不同因素下隧道初期支护爆破动力响应特性. 森林工程. 2025(03): 636-645 .  百度学术
百度学术3. 刘厚全. 采石场爆破开采对云南某三车道特长公路隧道安全性的影响. 工程技术研究. 2025(07): 32-34 .  百度学术
百度学术4. 罗亮明,韦凡秋,李颂章,伍福寿,张学民,张聪,周峰. 引水隧洞爆破地表振动传播特性研究. 中国农村水利水电. 2024(09): 109-114 .  百度学术
百度学术其他类型引用(1)
-

















 下载:
下载:

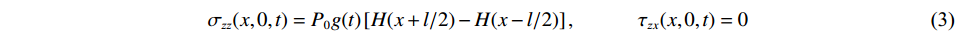

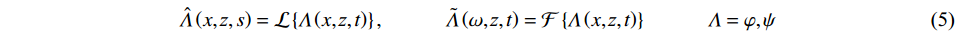



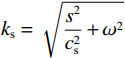


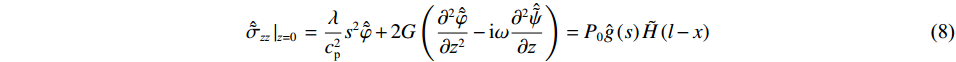















































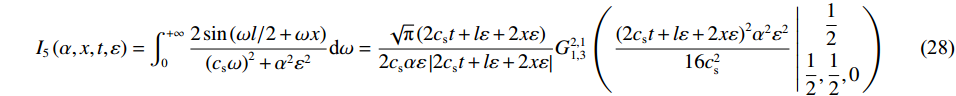








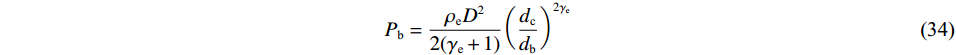




































 下载:
下载:













 百度学术
百度学术