Rate correlation of the ABAQUS damage parameter in the concrete damage plasticity model and its realization method
-
摘要: ABAQUS程序中最常用的混凝土损伤塑性(concrete damage plasticity, CDP)模型无法实现损伤因子与应变率相关。为了准确描述混凝土材料在高应变率下的损伤特性,基于CDP模型定义了新的应变率场变量,编制了VUSDFLD用户子程序,开发了能够考虑损伤因子率相关性的改进的CDP(modified CDP,MCDP)模型。MCDP模型采用能量法求解混凝土拉压损伤因子,主求解程序能够随着应变率场变量的变化而自动更新不同应变率对应的损伤参数,计算得到的混凝土单轴静态加载结果与CDP模型吻合较好。MCDP模型对高应变率下动态压缩性能的模拟结果表明:混凝土材料在不同应变率下的拉压损伤对其动态力学性能有显著影响,编制的VUSDFLD子程序和MCDP模型能够有效地解决损伤应变率相关的模拟难题,可以准确地模拟爆炸荷载作用下钢筋混凝土梁的动态响应,为预测爆炸冲击等强动载作用下混凝土结构的响应和破坏提供了更可靠的技术途径。Abstract: The concrete damage plasticity (CDP) model, as commonly adopted in ABAQUS routine, fails to correlate damage parameters with strain rate. To accurately describe the damage of concrete under high strain rate, a modified CDP (MCDP) model considering the rate correlation of damage parameters was developed by defining a new strain rate field variable and compiling VUSDFLD subroutine. In the MCDP model, the tensile and compressive damage parameters can be obtained by the energy method, and the main solver can automatically update the damage parameters under different strain rates with the change of strain rate field variables. Under static load, the results calculated by the MCDP model are in good agreement with those by the CDP model. The MCDP model was then used to calculate the dynamic compression performance of concrete under high strain rate, indicating that the tensile and compressive damage parameters of concrete under different strain rates have a significant influence on its dynamic mechanical properties. The compiled VUSDFLD subroutine and the MCDP model can solve the problem of the correlation between damage and strain rate, investigate the dynamic response of reinforced concrete beams accurately, and provide a more reliable technical way in predicting the response and destruction of the concrete structures under severe dynamic loading such as explosion and impact.
-
Key words:
- rate-dependent effect /
- concrete /
- damage factor /
- ABAQUS routine /
- damage plasticity model
-
据统计,现代战争中有70%以上的士兵伤亡是由破片和枪弹所致[1]。对第二次世界大战后的历次现代战争的战伤统计数据表明,80%以上的战伤是由手榴弹、迫击弹和其他爆炸物的破片所致[2]。大面积的杀伤破片已成为威胁士兵安全的主要因素。而自防弹衣问世以后,士兵伤亡率大幅降低。这对单兵武器的毁伤元提出了更高的要求。如何设计合适的破片使其贯穿防弹衣后对人体目标造成有效或致命伤害成为单兵破片战斗部设计的重点。
单兵破片战斗部要求体积小,威力大,机动性强,而钨合金材料密度大、强度高,球形小破片体积小、数量多、存速高,可有效提高杀伤威力。因此,在单兵战斗部上采用小质量钨合金球形破片是未来发展的方向之一,既能提高单位面积的破片数量又能保持良好的机动性能。目前,国内外关于防弹衣加人体等效靶的侵彻研究大多以标准枪弹为主,如Lidén等[3]研究了9 mm子弹侵彻带有软防护活体麻醉猪的非贯穿性损伤,结果表明非贯穿损伤能对软防护后的胸膛造成严重伤害;Roberts等[4-5]模拟计算了9 mm手枪弹侵彻带有软质防弹衣的拟人体上躯干,并与假人靶标试验结果进行了对比,分析了非贯穿性弹道冲击对人体躯干内部器官的影响;Merkle等[6]采用试验与数值模拟方法,研究了9 mm手枪弹侵彻带有软质防弹衣的假人体躯干,分析了人体内部器官在非贯穿损伤下的压力分布情况;Gilson等[7]采用试验和数值模拟方法,研究了9、10.9 mm枪弹非贯穿侵彻带软防护的明胶靶的弹道冲击响应;董萍等[8]利用CT扫描和MIMICS软件重建了人体躯干三维模型,并对9 mm手枪弹侵彻带防弹衣的拟人体躯干靶进行了数值模拟,研究了拟人体躯干主要脏器在弹头非贯穿冲击下的瞬态响应特性;韩瑞国等[9]通过试验和数值模拟研究了某步枪弹对带软、硬复合防护明胶靶标的侵彻机制;刘坤等[10]研究了9 mm全铜弹和5.8 mm手枪弹对带软防护明胶靶标的侵彻机理;除此之外,针对92a铅芯弹[11]、7.62 mm[12]和9 mm[13]枪弹分别侵彻带软防护明胶靶的动态力学特性也作了相应的研究。而关于小尺寸破片对带有防弹衣的人体等效靶的侵彻研究却鲜有报道。
本文采用国际惯用标准25 mm厚红松木靶作为人体等效靶,采用实验与数值模拟方法研究小钨球对三级软体防弹衣加25 mm红松靶的侵彻过程及破坏机理,探讨钨球质量变化对弹道极限及靶板能量吸收的影响。在此基础上,利用量纲分析的方法研究钨球的穿靶能量与初速及直径的关系,并建立钨球的穿靶能量公式及弹道极限公式,以期为单兵破片战斗部的设计提供参考。
1. 实验研究
1.1 实验布置
实验采用12.7 mm弹道枪发射置于尼龙弹托中的小钨球,通过调整药筒中装药量来控制钨球的速度。钨球质量为(0.210+0.003) g,直径为(2.80+0.02) mm,密度为18.1 g/cm3,其化学成分及力学性能如表1所示。靶板为FDY3R-01型三级软体防弹衣加25 mm厚红松木,两者紧密贴合组成防弹衣加红松木复合靶。防弹衣由衣套和防弹层构成,衣套为涤纶,防弹层为凯夫拉材料,共45层,总厚度9 mm。复合靶用专用夹具固定在钢靶架上。为测量着靶前钨球速度及穿透靶后的剩余速度,靶前及靶后分别设置梳状通断靶,测速装置采用南京理工大学机械工程学院设计的NLG202-Z型六路测速仪,精度为0.1 μs。图1为实验所用的钨球、弹托及药筒,图2为弹道实验示意图。本次实验的侵彻均为垂直侵彻。
表 1 钨球成分及其力学性能Table 1. Chemical composition and mechanical properties of tungsten sphereρ/(g·cm−3) 质量分数/% 变形量/% HRC硬度 Ra/μm W Ni Fe Co 18.1 95.30 3.15 1.35 0.20 ≤40 ≥26 ≤1.6 注:ρ为密度,Ra为表面粗糙度,变形量为7 kN下的变形量。 1.2 钨球侵彻防弹衣加松木靶的实验结果及分析
1.2.1 实验结果
0.21 g、直径2.8 mm的钨球侵彻防弹衣加红松木复合靶的实验结果如表2所示。图3为实验后的防弹衣加红松木复合靶,其中图3(a)为单独的防弹衣,图3(b)为防弹衣加红松木复合靶。
表 2 钨球侵彻防弹衣加红松木复合靶的实验结果Table 2. Experimental results of tungsten spheres penetrating into pine target covered with body armor序号 着靶速度/(m·s−1) 剩余速度/(m·s−1) 结果 1 681.2 − 穿透防弹衣,嵌入松木靶 2 700.4 74.8 穿透防弹衣和松木靶 3 711.7 111.6 穿透防弹衣和松木靶 4 725.5 160.4 穿透防弹衣和松木靶 5 744.5 194.2 穿透防弹衣和松木靶 6 748.0 204.9 穿透防弹衣和松木靶 7 753.4 220.9 穿透防弹衣和松木靶 8 775.7 250.6 穿透防弹衣和松木靶 1.2.2 实验结果处理与分析
本文利用Recht等[14]提出的R-I公式以获得钨球侵彻防弹衣加红松木复合靶的弹道极限,R-I公式为:
vr=a(vpi−vpbl)(1/p) (1) 式中:vi为破片着靶速度,m/s;vr为破片剩余速度,m/s;vbl为弹道极限,m/s;a、p为模型参数。
依据文献[15],p值设定为2。a和vbl可根据实验数据通过最小二乘法拟合得到。图4给出了钨球侵彻防弹衣加红松木复合靶的剩余速度与着靶速度关系曲线。由图4可看出,通过式(1)拟合出的关系曲线拟合效果较好。表3给出了通过式(1)计算所得的弹道极限及模型参数。
表 3 弹道极限及模型参数Table 3. Ballistic limit and model parameters钨球规格 a p vbl/(m·s−1) 质量0.21 g/直径2.8 mm 0.73 2 692.9 2. 数值模拟研究
2.1 数值模型及其参数
利用TrueGrid软件建模和划分网格,模型均选用8节点6面体单元。为节约计算时间,考虑到模型的对称性,模型简化为1/4模型。钨球尺寸及靶板厚度与实验状态一致,靶板采用圆形靶,其中防弹衣采用分层建模,每层0.2 mm厚,共45层。靶板半径设为30 mm(大于钨球直径10倍),以减小边界效应对侵彻过程的影响。为兼顾计算的时长与精度,网格采用渐进式,弹着点中心8倍半径区域加密,向外逐渐稀疏。密集区网格尺寸控制在0.10~0.15 mm。稀疏区网格尺寸控制在0.15~1.27 mm,钨球最小网格尺寸为0.01 mm,有限元模型如图5所示。
数值模拟选用LSDYNA-3D软件,单位制设为cm-g-μs,算法采用Lagrange算法。根据模型结构的对称性,在模型对称面添加对称边界条件,在靶板边缘添加无反射边界条件。弹靶之间的接触定义为面面侵蚀接触,防弹衣纤维层与层之间的接触定义为固连失效接触,防弹衣与松木靶之间的接触定义为自动面面接触。
钨球选用考虑应变率效应的弹塑性材料模型(MAT_PLASTIC_KINEMATIC),其材料模型参数[16]见表4,其中ρ为密度,E为弹性模量,μ为泊松比,σy为屈服强度,Et为切线模量,β为硬化参数,R1与R2为应变率参数,εf为失效应变。
表 4 钨球材料模型参数Table 4. Material model parameters of tungsten sphereρ/(g·cm−3) E/GPa μ σy/MPa Et/MPa β R1 R2 εf 18.1 367 0.303 1506 792 1 3.9 6 1.2 防弹衣属于层合结构,选用基于经典层合理论和Chang-Chang失效准则[17-18]的复合材料损伤模型(MAT_COMPOSITE_DAMAGE),具体材料模型参数[19]见表5,其中E1、E2、E3为各向弹性模量,μ21、μ32、μ31为各向泊松比,G12、G23、G31为各向剪切模量,κf为损坏材料体积模量,Gs为面内剪切强度,Tx、Ty分别为纵向和横向拉伸强度,Cy为横向压缩强度,α为非线性剪切应力修正系数,Tn为法向拉伸强度,Gyz和Gzx为横向剪切强度。
表 5 凯夫拉材料模型参数Table 5. Material model parameters of kevlarρ/(g·cm−3) E1/GPa E2/GPa E3/GPa μ21 μ31 μ32 1.35 21 21 4.6 0.31 0.14 0.14 G12/GPa G23/GPa G31/GPa κf/GPa Gs/GPa Tx/GPa Ty/GPa 1.2 1.2 1.2 2 0.35 1.0 1.0 Cy/GPa α Tn/GPa Gyz/GPa Gzx/GPa 0.8 0.5 0.55 0.55 0.55 文献[20]指出,红松木在比例极限下可近似看成弹性,当应力超过比例极限后,红松木发生变形和破坏,故红松木可选用MAT_ELASTIC材料模型并添加单元控制失效算法(ADD_EROSION)予以描述,其材料模型参数[21]见表6,其中σf为失效应力。
表 6 红松木材料模型参数Table 6. Material model parameters of pineρ/(g·cm−3) E/GPa μ σf/MPa 0.46 11.68 0.31 294 2.2 数值模型的验证
利用实验数据对仿真模型进行验证,结果见表7和图6。由表7可以看出,剩余速度的仿真值与实验值最大相对误差不超过10%,满足工程误差要求。同时,根据式(1)对计算得到的剩余速度与着靶速度的拟合可得:钨球侵彻防弹衣加红松木复合靶的弹道极限为690.5 m/s,与实验所得的弹道极限相对误差仅为−0.25%。可见该数值模型及其参数可信。
表 7 模拟值与实验值的对比Table 7. Comparison between simulated results and experimental resultsvi/(m·s−1) vr/(m·s−1) 相对误差/% 实验值 计算值 681.2 0 0 0 700.4 74.8 80.9 8.16 711.7 111.6 119.2 6.81 725.5 160.4 168.3 4.93 744.5 194.2 202.8 4.43 748.0 204.9 209.2 2.10 753.4 220.9 218.4 −1.13 775.7 250.6 253.9 1.32 2.3 侵彻过程及破坏机理分析
图7给出了不同着靶速度下钨球的速度v(图7(a))和加速度a变化曲线(图7(b))。从图7可看出,不同侵彻速度下钨球的速度和加速度变化趋势相近。以vi=748.0 m/s为例分析侵彻过程,其速度和加速度随时间的变化分别如图8(a)和图8(b)所示。在图8(a)和图8(b)中分别提取5个特征点并用相同字母不同的角标表示:其中A0和A1点表示钨球与防弹衣初始接触时刻;B0和B1点表示钨球侵彻防弹衣阻力达到最大时刻;C0和C1点表示钨球刚穿透防弹衣并开始侵彻松木靶时刻;D0和D1点表示钨球开始稳定侵彻松木靶时刻;E0和E1点表示钨球在松木靶内部稳定侵彻结束时刻;F0和F1点表示钨球完全穿透松木靶时刻。根据钨球速度的衰减过程,可大致将钨球侵彻过程分为侵彻防弹衣(A0C0段)和侵彻松木靶(C0F0段)两阶段。
(1)侵彻防弹衣阶段。
A0B0段,在侵彻防弹衣初期,钨球速度大,动能高,当钨球与防弹衣初始接触时,由于防弹衣的阻碍作用,钨球速度急剧下降,并对防弹衣产生压力作用,此时纤维受到压缩和剪切作用;当剪应力超过极限时,纤维发生剪切断裂破坏。同时,钨球冲击防弹衣产生的应力波沿纤维轴向和靶体纵向两个方向传播;在纤维轴向上,应力波通过基体连接传递至周围其他纤维,二维面积上产生基体开裂、纤维拉伸变形和断裂等现象;在靶体纵向上生成压缩波,压缩波沿靶体纵向传递至基体与纤维的分界面时发生反复透射和反射并形成拉伸波,当拉伸波强度超过基体与纤维间或纤维层间结合强度时,基体与纤维脱粘,纤维层产生分层破坏[22]。侵彻10 μs左右,钨球阻力达到最大,此时穿透17层纤维。B0C0段,随着侵彻的进行,由于断裂破坏的纤维不断增多,且未断裂的纤维已受到一定的拉伸作用使其强度降低,钨球侵彻阻力逐渐减小;虽然钨球速度持续下降,但降低幅度有所减缓,直至贯穿防弹衣到达C0点。
(2)侵彻红松靶阶段。
C0D0段,穿透防弹衣后,钨球主要受松木靶阻力作用;由于松木材质较软,屈服强度较低,因此,钨球侵彻松木靶的阻力较防弹衣的弱,表现在速度衰减较A0C0段缓慢,其侵彻阻力继续减小直至侵彻1.1 mm厚到达D0点。D0E0段,钨球侵入松木靶并进入稳定侵彻阶段,此时松木靶在钨球压力作用下发生剪切破坏。由于木靶材质较均匀,钨球速度几乎呈线性降低,即速度衰减率基本不变,在85 μs左右,稳定侵彻到达E0点,此时钨球侵彻松木靶23.3 mm厚,还剩1.7 mm厚未穿透,松木靶背面产生微小裂纹并发生部分剥落现象。E0F0段:钨球进入穿透松木靶阶段,其头部已经露出松木靶;受靶板背面自由边界的影响,钨球侵彻阻力迅速减小,直到完全穿透松木靶达到F0点,松木靶在钨球的作用下,主要发生剪切和冲塞剥落破坏。
结合图4和图7(a)可看出,随着钨球着靶速度的提高,剩余速度增加但增加斜率逐渐减小。这主要与复合靶的吸能有关。在弹道极限附近的速度范围内,随着着靶速度的提高,钨球侵彻能力增强,弹靶作用时间相对缩短,这样拉伸波不能及时沿靶体纵向传播,导致纤维的分层损伤降低,纤维分层吸收钨球动能的能力下降,故纤维拉伸分层吸能占总吸能比例逐渐降低。图9给出了不同着靶速度下松木靶背面的损伤形貌。从图9可知,随着速度的提高,松木靶背面剥落程度降低,冲塞剥落吸能占总吸能比例降低,复合靶能量吸收不稳定,因而剩余速度曲线随着靶速度的提高变得陡峭。当着靶速度进一步提高时,基体开裂、纤维剪切断裂和松木靶剪切破坏成为复合靶主要吸能方式,复合靶吸收能量比较稳定,剩余速度曲线更加平缓。从图7(b)上看,由于松木靶破坏模式的转变,在钨球穿透松木靶阶段,随着着靶速度的提高,钨球负加速度下降更快。
图10给出了防弹衣正面与背面的典型von Mises应力变化过程。
由图10可知,破片冲击产生的应力波在纤维层面内通过基体连接传向周围其他纤维,纤维轴向上的波阵面形状类似双纽线[10],着靶处纤维在应力波作用下产生类似“十”字型的损伤,宏观表现为基体开裂,图11的实验结果也验证了这一点。
2.4 质量变化对侵彻的影响
2.4.1 质量变化对弹道极限的影响
表8给出了不同质量(m)钨球侵彻防弹衣加红松木复合靶弹道极限(vbl)的计算结果。图12给出了弹道极限随钨球质量变化的关系曲线。
表 8 不同质量钨球侵彻防弹衣加红松木复合靶弹道极限的模拟结果Table 8. Simulated results of ballistic limit of tungsten spheres with different mass penetrating into pine target covered with body armorm/g vbl/(m·s−1) 0.21 690.5 0.26 647.0 0.31 619.0 0.36 595.0 0.41 575.0 0.46 556.0 从图12可看出,随着钨球质量的增加,钨球侵彻防弹衣加红松木复合靶的弹道极限呈幂函数递减趋势。从减轻破片战斗部质量提高射程或增加破片数量提高杀伤威力等角度考虑,应存在合适的破片质量范围,既能增加破片数量又能提高破片对人体的杀伤。
2.4.2 质量变化对靶板能量吸收的影响
在侵彻靶板过程中,破片部分动能被靶板吸收,靶板的能量吸收可用能量吸收率来表征[23],定义靶板的能量吸收率η为:
η=EabsEk=0.5m(v2i−v2r)0.5mv2i=1−v2rv2i (2) 式中:Eabs为靶板吸收的能量,即破片损失的动能;Ek为破片初始动能。
表9给出了不同质量钨球侵彻防弹衣加红松木复合靶的仿真结果。图13给出了靶板能量吸收率η在不同着靶速度下的变化曲线。由图13可知,当钨球嵌入靶板时,靶板的能量吸收率为1。若钨球穿透靶板,在同一着靶速度下,靶板的能量吸收率随钨球质量增加而降低。并且随着着靶速度的增加,靶板能量吸收率急剧下降。
表 9 不同质量钨球侵彻防弹衣加红松木复合靶的仿真结果Table 9. Simulated results of tungsten spheres with different mass penetrating into pine target covered with body armor着靶速度/(m·s−1) m=0.21 g m=0.26 g m=0.31 g 剩余速度/(m·s−1) 靶板能量吸收率 剩余速度/(m·s−1) 靶板能量吸收率 剩余速度/(m·s−1) 靶板能量吸收率 550 0 1 0 1 0 1 600 0 1 0 1 0 1 650 0 1 26.2 0.998 156.7 0.942 700 79.2 0.987 188.3 0.928 254.0 0.868 750 212.6 0.920 281.6 0.859 322.5 0.815 800 294.3 0.865 350.2 0.808 390.3 0.762 着靶速度/(m·s−1) m=0.36 g m=0.41 g m=0.46 g 剩余速度/(m·s−1) 靶板能量吸收率 剩余速度/(m·s−1) 靶板能量吸收率 剩余速度/(m·s−1) 靶板能量吸收率 550 0 1 0 1 0 1 600 65.1 0.988 121.0 0.959 172.8 0.917 650 197.6 0.908 232.4 0.872 267.1 0.831 700 287.4 0.831 315.0 0.798 345.0 0.757 750 354.9 0.776 376.7 0.748 400.3 0.715 800 416.8 0.729 437.1 0.701 457.0 0.674 3. 量纲分析
穿靶能量Ec,即消耗破片的动能,是反映破片侵彻靶板特性的一个重要参数。为进一步定量研究钨球穿靶能量与着靶速度以及直径之间的关系,本文依据量纲分析建立小钨球侵彻防弹衣加红松木复合靶的穿靶能量计算公式。
通过理论分析,影响钨球侵彻防弹衣加红松木复合靶穿靶能量的主要物理量如表10所示。
表 10 影响穿靶能量的主要的物理量Table 10. Main physical quantities affecting the energy of penetrating into target材料名称 物理量 量符号 量纲式 钨球 着靶速度 vi LT−1 密度 ρp ML−3 直径 Dp L 弹性模量 Ep L−1MT−2 屈服强度 σsp L−1MT−2 特征应变 εp 1 声速 cp LT−1 防弹衣 密度 ρf ML−3 厚度 hf L 弹性模量 Ef L−1MT−2 抗压强度 σsf L−1MT−2 抗剪强度 στf L−1MT−2 抗拉强度 σff L−1MT−2 特征应变 εf 1 声速 cf LT−1 红松木 密度 ρs ML−3 厚度 hs L 弹性模量 Es L−1MT−2 失效应力 σss L−1MT−2 特征应变 εs 1 声速 cs LT−1 可见,穿靶能量Ec是关于上述物理量的函数:
Ec=f(vi,ρp,Dp,Ep,σsp,εp,cp,ρf,hf,Ef,σsf,στf,σff,εf,cf,ρs,hs,Es,σss,εs,cs) (3) 选取ρp、Dp和σsp为量纲独立变量,根据量纲齐次原则,其他导出量可写成以下无量纲形式:
Π=EcD3pσsp,Π1=vi√σsp/ρp,Π2=Epσsp,Π3=εp,Π4=cp√σsp/ρp,Π5=ρfρp, Π6=hfDp,Π7=Efσsp,Π8=σsfσsp,Π9=στfσsp,Π10=σffσsp,Π11=εf,Π12=cf√σsp/ρp, Π13=ρsρp,Π14=hsDp,Π15=Esσsp,Π16=σssσsp,Π17=εs,Π18=cs√σsp/ρp 根据Π定理,式(3)可写为:
Π=EcD3pσsp=f(Π1,Π2,Π3,⋯,Π18) (4) 在弹靶材料不变的情况下,除了Π、Π1、Π6和Π14外,其余导出量均为常数,式(4)可简化为:
Π=EcD3pσsp=f(vi√σsp/ρp,hfDp,hsDp)=C0(vi√σsp/ρp)α(hfDp)β(hsDp)γ (5) 式中:C0、α、β、γ均为待定常数。
将式(5)展开:
Ec=C0σsp1−0.5αρp0.5αhfβhsγviα(1Dp)β+γ−3 (6) 由于本实验中的防弹衣和红松木材料及厚度不变,钨球材料不变,令:
δ=β+γ−3 C1=C0σsp1−0.5αρp0.5αhfβhsγ 则式(6)可写为:
Ec=C1viα(1Dp)δ (7) 式中:C1、α、δ为待定常数。
由式(7)可知,在防弹衣和红松木材料及其厚度不变以及钨球材料不变的情况下,穿靶能量Ec只与着靶速度vi和钨球直径Dp有关。
为方便求解,将式(7)两端同时取对数:
lnEc=αlnvi+δln1Dp+lnC1 (8) 令
y=lnEc ,x1=lnvi ,x2=ln1Dp ,k=lnC1 ,则式(8)为:y=αx1+δx2+k (9) 利用表9中的数据对式(9)进行二元一次线性回归拟合,可得:
α=0.94,δ=−2.04,k=9.77 将α、δ和k回代至式(7),有:
Ec=e9.77v0.94iDp2.04 (10) 为验证式(10)的有效性,对0.17 g、直径2.6 mm和0.44 g、直径3.6 mm两种小钨球侵彻防弹衣加红松木复合靶进行了侵彻实验。表11对比了通过实验和式(10)计算得到的穿靶能量。由表11可知,利用式(10)计算的穿靶能量与实验所得的穿靶能量最大相对误差不超过10%,满足工程误差要求。无需剩余速度,式(10)仅用着靶速度和破片直径就能预测不同质量钨球在不同着靶速度下的穿靶能量(适用范围为m<0.46 g)。
表 11 不同方法计算的穿靶能量的对比Table 11. Comparison of energy of penetrating into target calculated by different methods钨球规格 vi/(m·s−1) vr/(m·s−1) Ec/J 相对误差/% 实验结果 量纲分析结果 质量0.17 g
直径2.6 mm753.6 124.6 46.95 47.22 0.58 771.3 187.7 47.57 48.26 1.45 786.0 230.1 48.01 49.12 2.31 809.1 296.8 48.16 50.48 4.82 834.9 357.9 48.36 51.99 7.51 869.6 410.8 49.93 54.02 8.19 质量0.44 g
直径3.6 mm578.4 78.2 72.25 71.52 −1.01 597.7 141.8 74.17 73.76 −0.55 610.9 187.2 74.39 75.29 1.21 639.5 248.0 76.44 78.60 2.83 660.3 260.9 80.94 81.00 0.07 698.3 335.1 82.57 85.37 3.39 值得注意的是,当着靶速度
vi 为弹道极限vbl 时,穿靶能量为极限穿靶能量:Ec=0.5mv2bl (11) 将式(11)代入式(7)可推导出计算弹道极限的另一个公式:
vbl=[2C1m(1Dp)δ]12−α=[2e9.77mDp2.04]11.06 (12) 根据试验数据,利用式(12)计算弹道极限,并与利用式(1) R-I公式计算得到的弹道极限进行对比,结果如表12所示。由表12可知,与式(1)公式相比,式(12)仅利用破片的直径和质量就能计算弹道极限,且相对误差不超过5%,满足工程应用要求。因此,式(12)可作为计算不同质量钨球侵彻防弹衣加红松木复合靶弹道极限的一个经验公式(适用范围为m<0.46 g)。
表 12 式(1)和式(12)计算的弹道极限的对比Table 12. Comparison of ballistic limit calculated by formula (1) and formula (12)钨球规格 vbl/(m·s−1) 相对误差/% 式(1) 式(12) 质量0.17 g/直径2.6 mm 742.3 738.1 −0.57 质量0.21 g/直径2.8 mm 692.9 697.4 0.65 质量0.44 g/直径3.6 mm 570.1 562.9 −1.26 4. 结 论
采用实验和数值模拟方法研究了小钨球对三级软体防弹衣加25 mm红松靶的侵彻过程及破坏机理;探讨了钨球质量变化对弹道极限及靶板能量吸收的影响;利用量纲分析研究了钨球穿靶能量与其初速及直径的关系,建立了钨球的穿靶能量公式及弹道极限公式。得出研究结论如下:
(1) 0.17 g,直径2.6 mm,0.21 g、直径2.8 mm以及0.44 g、直径3.6 mm的小钨球侵彻防弹衣加红松木复合靶的弹道极限分别为742.3、692.9和570.1 m/s;
(2) 在钨球侵彻防弹衣加红松木复合靶的过程中,防弹衣的破坏形式主要表现为基体开裂、纤维断裂和拉伸分层,纤维层面内出现类似“十”字型的损伤,而松木靶则以剪切和冲塞剥落破坏为主;
(3) 随着钨球质量的增加,钨球侵彻防弹衣加红松木复合靶的弹道极限呈幂函数减小趋势,靶板的能量吸收率逐渐降低;
(4) 利用量纲分析法建立了钨球侵彻防弹衣加红松木复合靶的穿靶能量计算公式及弹道极限计算公式,可分别用于计算不同侵彻速度下的穿靶能量和不同质量钨球的弹道极限。
-
表 1 MCDP模型参数
Table 1. Parameters of the MCDP model
膨胀角/
(°)流动势
偏移量双轴与单轴抗压
强度之比不变量
应力比黏性系数 30 0.1 1.16 0.6667 0.0005 表 2 不同应变率下的C30混凝土动态强度
Table 2. Dynamic strengths of C30 concrete under different strain rates
应变率/s−1 抗压动态增长因子 动态抗压强度/MPa 抗拉动态增长因子 动态抗拉强度/MPa 10−5 1.023 20.56 1.138 2.29 10−4 1.055 21.20 1.175 2.36 10−3 1.169 23.49 1.307 2.63 10−2 1.295 26.02 1.453 2.92 10−1 1.434 28.82 1.616 3.25 1 1.588 31.93 1.796 3.61 10 1.760 35.37 1.997 4.01 100 2.775 55.78 3.154 6.34 表 3 变应变率加载工况参数
Table 3. Parameters for variational strain-rate cases
工况 模型 时段1/ms 应变率1/s−1 时段2/ms 应变率2/s−1 时段3/ms 应变率3/s−1 A MCDP 0~1.5 1 >1.5 100 — — CDP 0~1.5 1 >1.5 100 — — B MCDP 0~0.15 10 >0.15 100 — — CDP 0~0.15 10 >0.15 100 — — C MCDP 0~0.13 10 0.13~0.15 100 >0.15 10 CDP 0~0.13 10 0.13~0.15 100 >0.15 10 D MCDP 0~0.005 100 0.005~0.055 10 >0.055 100 CDP 0~0.005 100 0.005~0.055 10 >0.055 100 -
[1] CHEN L, FANG Q, JIANG X Q, et al. Combined effects of high temperature and high strain rate on normal weight concrete [J]. International Journal of Impact Engineering, 2015, 86: 40–56. DOI: 10.1016/j.ijimpeng.2015.07.002. [2] 方秦, 还毅, 陈力, 等. 应变速率型RC梁柱显式分析单元及其在ABAQUS软件中的实现 [J]. 工程力学, 2013, 30(5): 49–55. DOI: 10.6052/j.issn.1000-4750.2011.08.0545.FANG Q, HUAN Y, CHEN L, et al. The realization of explicit analytical model of rate-dependent fiber beam-column element in ABAQUS [J]. Engineering Mechanics, 2013, 30(5): 49–55. DOI: 10.6052/j.issn.1000-4750.2011.08.0545. [3] BISCHOFF B H, PERRY S H. Compressive behavior of concrete at high strain rate [J]. Materials and Structures, 1991, 24(6): 425–450. DOI: 10.1007/BF02472016. [4] 方秦, 还毅, 张亚栋, 等. ABAQUS混凝土损伤塑性模型的静力性能分析 [J]. 解放军理工大学(自然科学版), 2007, 8(3): 254–260. DOI: 10.7666/j.issn.1009-3443.20070311.FANG Q, HUAN Y, ZHANG Y D, et al. Investigation into static properties of damaged plasticity model for concrete in ABAQUS [J]. Journal of PLA University of Science and Technology, 2007, 8(3): 254–260. DOI: 10.7666/j.issn.1009-3443.20070311. [5] LUBLINER J, OLIVER J, OLLER S, et al. A plastic-damage model for concrete [J]. International Journal of Solids and Structures, 1989, 25(3): 299–326. DOI: 10.1016/0020-7683(89)90050-4. [6] LEE J, FENVES G L. Plastic-damage model for cyclic loading of concrete structures [J]. Journal of Engineering Mechanics, 1998, 124(8): 892–900. DOI: 10.1061/(ASCE)0733-9399(1998)124:8(892). [7] 杨宏明, 杨德思, 吴正昊. 基于二维凸骨料细观模型的混凝土动态力学性能分析 [J]. 混凝土, 2021(6): 88–92. DOI: 10.3969/j.issn.1002-3550.2021.06.020.YANG H M, YANG D S, WU Z H. Analysis of dynamic mechanical properties of concrete based on two-dimensional convex aggregate mesoscopic model [J]. Concrete, 2021(6): 88–92. DOI: 10.3969/j.issn.1002-3550.2021.06.020. [8] 周禹辛, 周晶. 冻融循环下混凝土的动态数值模拟分析 [J]. 水利与建筑工程学报, 2018, 16(5): 181–184, 189. DOI: 10.3969/j.issn.1672-1144.2018.05.035.ZHOU Y X, ZHOU J. Dynamic numerical simulation analysis of concrete under freeze-thaw cycles [J]. Journal of Water Resources and Architectural Engineering, 2018, 16(5): 181–184, 189. DOI: 10.3969/j.issn.1672-1144.2018.05.035. [9] 李敏, 李宏男. ABAQUS混凝土损伤塑性模型的动力性能分析 [J]. 防灾减灾工程学报, 2011(3): 299–303. DOI: 10.3969/j.issn.1672-2132.2011.03.011.LI M, LI H N. Investigation into dynamic properties of damaged plasticity model for concrete in ABAQUS [J]. Journal of Disaster Prevention and Mitigation Engineering, 2011(3): 299–303. DOI: 10.3969/j.issn.1672-2132.2011.03.011. [10] 阎石, 张亮, 王丹. 钢筋混凝土板在爆炸荷载作用下的破坏模式分析 [J]. 沈阳建筑大学学报(自然科学版), 2005, 21(3): 177–180. DOI: 10.3321/j.issn:1671-2021.2005.03.001.YAN S, ZHANG L, WANG D. Failure mode analysis for RC slab under explosive loads [J]. Journal of Shenyang Jianzhu University (Natural Science), 2005, 21(3): 177–180. DOI: 10.3321/j.issn:1671-2021.2005.03.001. [11] 秦浩, 赵宪忠. ABAQUS混凝土损伤因子取值方法研究 [J]. 结构工程师, 2013, 29(6): 27–32. DOI: 10.3969/j.issn.1005-0159.2013.06.005.QIN H, ZHAO X Z. Study on the ABAQUS damage parameter in the concrete damage plasticity model [J]. Structural Engineers, 2013, 29(6): 27–32. DOI: 10.3969/j.issn.1005-0159.2013.06.005. [12] CEB. Concrete structures under impact and impulsive loading: No. 187 [R]. Lausanne, Switzerland: Comité Euro-International du Beton, 1988. [13] 中华人民共和国住房和城乡建设部. 建筑抗震设计规范: GB 50011—2010 [S]. 北京: 中国建筑工业出版社, 2010. [14] KRAJCINOVIC D, FONSEKA G U. The continuous damage theory of brittle materials, part Ⅰ: general theory [J]. Journal of Applied Mechanics, 1981, 48(4): 809–815. DOI: 10.1115/1.3157739. [15] ABAQUS user subroutines reference manual v6.14 [Z]. ABAQUS Inc, 2014. [16] 刘巍, 徐明, 陈忠范. ABAQUS混凝土损伤塑性模型参数标定及验证 [J]. 工业建筑, 2014(Suppl 1): 167–171. DOI: 13204/j.gyjz2014.sl.227.LIU W, XU M, CHEN Z F. Parameters calibration and verification of concrete damage plasticity model of ABAQUS [J]. Industrial Construction, 2014(Suppl 1): 167–171. DOI: 13204/j.gyjz2014.sl.227. [17] 杨飞, 董新勇, 周沈华, 等. ABAQUS混凝土塑性损伤因子计算方法及应用研究 [J]. 四川建筑, 2017, 37(6): 173–177. DOI: 10.3969/j.issn.1007-8983.2017.06.062.YANG F, DONG X Y, ZHOU S H, et al. Study on calculation method and application of the ABAQUS damage parameter in the concrete damage plasticity model [J]. Sichuan Architecture, 2017, 37(6): 173–177. DOI: 10.3969/j.issn.1007-8983.2017.06.062. [18] BIN R, LI C, QIN F, et al. Dynamic responses of RC beams under double-end-initiated close-in explosion [J]. Defence Technology, 2018, 14(5): 527–539. DOI: 10.1016/j.dt.2018.07.024. 期刊类型引用(4)
1. 付世杰, 邵伟平, 郝永平. 基于自注意与图神经网络的弹体侵彻靶板材质检测方法研究. 弹箭与制导学报. 2025(03)  百度学术
百度学术2. 张磊,石少卿,王昊康,田镇华,王文康,廖瑜. 玻璃纤维掩蔽部顶盖构件抗爆炸性能分析. 合成纤维. 2024(07): 57-61+78 .  百度学术
百度学术3. 唱忠良,张志俊,吴晨晨,邹广平,吴松阳,闫安石,李寅辰. 平纹机织可展收层合壳弹道冲击特性研究. 机械强度. 2024(04): 853-858 .  百度学术
百度学术4. 董方栋,杨耿,王舒,王志军,冯志威. 刚性破片侵彻层合板运动模型. 兵工学报. 2022(09): 2350-2359 .  百度学术
百度学术其他类型引用(0)
-







 下载:
下载:



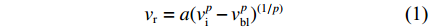
















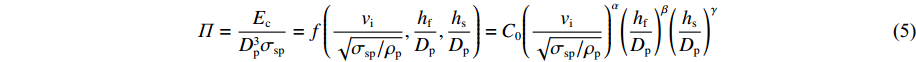

















 下载:
下载:














 百度学术
百度学术











