Impact dynamic mechanical properties of frozen soil with freeze-thaw cycles
-
摘要: 以典型冻土为研究对象,通过不同冻融循环次数的冻融循环实验、不同温度的冻结实验以及不同应变率的冲击动态实验,综合研究了冻融循环冻土的冲击动态力学性能。结果表明,冻土存在冻融循环效应,随着冻融循环次数的增加,冻土的峰值应力有一定程度的降低,但在达到临界冻融循环次数后,峰值应力将维持稳定;同时,冻土表现出明显的应变率效应和温度效应,其峰值应力随应变率的增加或温度的降低而增加。通过定义冻融损伤因子,推导满足Weibull分布的冲击损伤,提出了一个基于Z-W-T方程的损伤黏弹性本构模型。该模型可较好地描述冻融循环后冻土的冲击动态力学行为,为研究季节性冻土区冻土的冲击动态破坏提供参考。Abstract: During engineering construction and service in seasonally frozen soil regions, frozen soil is often subjected to the combined action of freeze-thaw (F-T) cycles and impact loading, which changes its physical state and mechanical properties. In order to explore the effect of F-T cycles on the impact dynamic mechanical properties of frozen soil, in this paper, the typical frozen soil was taken as the research object, and the effect of F-T cycles on the impact dynamic mechanical properties of frozen soil was comprehensively studied with the help of high and low temperature F-T cycles experimental equipment and a split Hopkinson pressure bar device, through F-T cycles experiments with different F-T cycles numbers, freezing experiments at different temperatures, and impact dynamic experiments with different strain rates. The results shows that there is an F-T cycles effect in frozen soil. With the increase of the number of F-T cycles, the peak stress of frozen soil decreases to a certain extent, but after reaching the critical number of F-T cycles, the peak stress remains stable. According to the hydrostatic pressure theory, it is believed that the F-T cycles mainly changes the mechanical properties of frozen soil by changing its microstructural characteristics. Meanwhile, the frozen soil also exhibits obvious strain rate effect and temperature effect, and its peak stress increases with the increase of strain rate or the decrease of temperature. The F-T damage factor was defined by the peak stress, and the impact damage was deduced by a statistical method that it assumes the microstructure strength of frozen soil satisfies the Weibull distribution, a damage viscoelastic constitutive model based on the Z-W-T equation was proposed. The model can better describe the impact dynamic mechanical behavior of frozen soil after F-T cycles and provide reference for the impact dynamic damage of frozen soil in seasonally frozen soil regions.
-
Key words:
- frozen soil /
- freeze-thaw cycle /
- impact /
- damage /
- constitutive model
-
钻孔爆破作为一种经济高效的破岩手段,被广泛应用于水利、矿山和市政等领域的岩体开挖工程[1-3]。根据起爆药卷在炮孔中的位置,起爆方式可分为孔底起爆、孔口起爆、中点起爆等。起爆方式影响钻孔爆破的应力波传播和爆炸能量传输规律,从而影响钻孔爆破的破岩效果[4-9]。因此,研究不同起爆方式下爆炸应力波的相互作用和破岩效果对于工程爆破中炸药能量的高效利用具有重要意义。
Chouhan等[10]研究了装药结构对岩石破碎效果的影响,发现了V型装药结构更有利于岩石的破碎。Miao等[11]通过对称双线性起爆系统进行了收敛爆轰波的碰撞研究,结果表明,采用对称双线性起爆产生的马赫压力最大可达Chapman-Jouguet压力的3.22倍,可显著改善破岩效果。Onederra等[12]建立了复合应力爆破模型,并进行了大型混凝土块控制爆破实验,实验结果表明,孔底起爆时孔底的破坏范围小于孔口。Liu等[13]采用拉伸-压缩损伤模型对不同起爆点的台阶爆破进行了数值模拟,结果表明,中部起爆是减小大块率的最佳起爆方式。胡少斌等[14]运用有限元软件ANSYS/LS-DYNA对不同起爆位置下柱状药包在煤岩体中产生的应力场及其传播规律进行了数值模拟,孔口起爆有利于炮孔底部岩石的破碎,孔底起爆有利于应力集中区煤岩体的破碎。张世豪等[15]分别从爆炸应力波和爆炸能量的角度研究了多点爆炸的能量聚焦效应,多点爆炸应力波在装药对称位置发生相互作用,提高了装药的能量利用率。李洪伟等[16]利用有限元分析软件ANSYS/LS-DYNA对不同起爆方式下岩石的损伤特征进行了数值模拟,发现相较于孔口起爆,孔底起爆时岩石的破碎效果更好。高启栋等[17]对隧道掏槽爆破进行了现场试验和数值模拟,分析了孔口起爆、孔底起爆和中点起爆等不同起爆方式下爆破损伤的演化规律,结果表明,孔底起爆时孔口岩体的破裂隙最为明显,中点起爆次之,孔口起爆最小。杨仁树等[18]采用模型试验研究了不同起爆方式对空气间隔装药结构下炮孔附近岩体损伤分布的影响,发现当起爆点在远离空气段一侧时,爆炸后空气段中心两侧产生损伤,采用其他起爆方式时,空气段未产生损伤。
现有研究主要集中在孔内起爆点位置对爆炸应力波传播和能量传输的影响,鲜有孔间交错起爆方式对破岩效果影响的报道。受爆炸过程的复杂性和实验条件的限制,尚不清楚孔间交错起爆方式下应力波的相互作用机制。因此,本文中,将建立双孔交错起爆模型,并进行交错起爆与孔底起爆对比实验,研究交错起爆方式下孔间爆炸应力波的相互作用,揭示孔间交错起爆方式对破岩效果的影响。
1. 双孔交错起爆下应力波的碰撞机制
不同于传统单一起爆方式,交错起爆是指相邻炮孔分别从孔底、孔口同时起爆的爆破方式,产生的应力波会在两孔间相互碰撞,从而影响爆破破岩效果。图1为双孔交错起爆下孔间应力波的碰撞示意图,两应力波在A点正碰撞后,沿着碰撞面向两侧传播并在B区发生斜碰撞,当应力波与碰撞面的角度达到一定值时,在C区形成马赫反射。
根据应力波碰撞理论,当炮孔内起爆药卷被引爆后,应力波以球面波的形式向四周传播,某时刻相邻炮孔产生的应力波同时到达A点(图1),并发生正碰撞。为研究方便,将碰撞面视为刚性壁面,正碰撞过程可视为单一应力波与刚性壁面正碰撞并产生反射波的过程(图2)。
根据正碰撞理论,假设炸药在初始状态下压力为
p0 ,密度为ρ0 ,粒子速度为u0f ;爆轰后应力波稳定传播时炸药压力为p1 ,密度为ρ1 ,粒子速度为u1f ;当应力波抵达刚性壁面发生反射后,压力为p2 ,密度为ρ2 ,粒子速度为u2f 。对于初始未反应炸药,u0f=0 ,根据质量守恒、动量守恒和能量守恒定律有:ρ0d=ρ1(d−u1f) (1) ρ0d2−ρ1(d−u1f)2=p1−p0 (2) e′1−e′0=p1u1fρ0d−12u21f+QV (3) 式中:
d 为应力波传播速度,e′0 和e′1 分别为炸药在初始状态和爆轰状态下的内能,QV 为炸药爆炸时产生的热量。应力波稳定传播时的压力比初始压力大得多,因此
p0 可忽略不计。对于图2中的反射区,应力波以速度u1f 与刚性壁面碰撞并产生反射波,反射波以速度u2f 向左传播,且反射波阵面后爆炸产物的质点速度为零,根据动量守恒方程和Chaoman-Jouguet理论可得[19]:p2p1=5k+1+√17k2+2k+14k (4) 式中:
k 为绝热指数。可以看出,应力波正反射后和稳定传播时的压力比与绝热指数k 相关,k 越大,压力比越小。根据斜碰撞理论,应力波稳定传播时炸药压力为
p′1 ,密度为ρ′1 ;当应力波抵达刚性壁面发生反射后,炸药压力为p′2 ,密度为ρ′2 。入射波IO以角度φ0 与刚性壁面发生一定角度的斜碰撞后产生反射波OR,入射角φ0 和反射角φ2 一般不相等,如图3所示。入射波沿着刚性壁面传播的速度为μ0 ,为了研究方便,假设应力波固定不动,未爆产物以速度μ0 流入波阵面IO,随即产生角度为θ 的偏转,将速度分解为平行于波阵面的切向速度和垂直于波阵面的法向速度,其中垂直于波阵面的法向速度可视为应力波的传播速度d 。将入射和反射应力波均视为平面波,将碰撞点O视为坐标原点。对于爆轰产物Ⅱ区,设
μh 为应力波稳定传播时粒子的流动速度,Ⅰ区沿波阵面的法向速度μ1n=d−μh=kd/kd(k+1)(k+1) ,切向速度μ1τ=d/dtanφ0tanφ0 ,根据Chapman-Jouguet理论可以得出Ⅰ区爆轰产物的流动速度μ1 为:μ1=√μ21n+μ21τ=√(kdk+1)2+dtan2φ0 (5) 根据图3中的几何关系
tan(φ0−θ)=μ1n/μ1τ ,可以得到偏转角θ 和应力波入射角φ0 的关系式:tanθ=tanφ0ktan2φ0+k+1 (6) 对于反射区(Ⅲ区),同Ⅱ区,由质量守恒、动量守恒和能量守恒定律可得:
ρ′1μ1n=ρ′2μ2n (7) p′2−p′1=μ21nρ′1−μ22nρ′2 (8) e″2−e″1=12(p′1+p′2)(v1−v2) (9) 式中:
v1=1/ρ′1 ,v2=1/ρ′2 ,冲击波在Ⅱ区和Ⅲ区的内能分别为e″1=p′1v1/k−1 和e″2=p′2v2/k−1 。整理式(9)可得应力波斜碰撞后和稳定传播时的压力比:p′2p′1=(k+1)tan(φ2+θ)−(k−1)tanφ2(k+1)tanφ2−(k−1)tan(φ2+θ) (10) 根据式(10)和图3中的几何关系,可得应力波入射角
φ0 和反射角φ2 的关系:tanφ2tanY(φ0)=k−1k+1+2k2k+11[k2+(k+1)2cot2φ0]sinY(φ0) (11) Y(φ0)=φ0+arctan(tanφ0ktan2φ0+k+1) (12) 根据已有文献,当应力波入射角
φ0 增大到一定值时,流动产物通过反射冲击波后再次偏转,不再以平行于刚性壁面的速度流出,从而造成了物质的堆积。当入射角φ0 超过该值时,不再发生斜碰撞,反射波开始从刚性壁面上脱离形成马赫波,如图1中C区所示。根据马赫反射原理[20],在碰撞点处压力比与入射角的关系为:p3p′1=1sinφ0(1+√1−ηsin2φ0) (13) 式中:p3为马赫波产生的压力;
η 为过度压缩系数,即马赫波释放与正常应力波释放的化学能比,根据已有实验数据,η 的取值在1.0~1.2之间[21]。若已知绝热指数
k ,可以求得应力波在不同入射角下的压力比,绝热指数k 可以表示为[22]:k=(1.01+1.313ρe)21.558ρe−1 (14) 式中:炸药密度
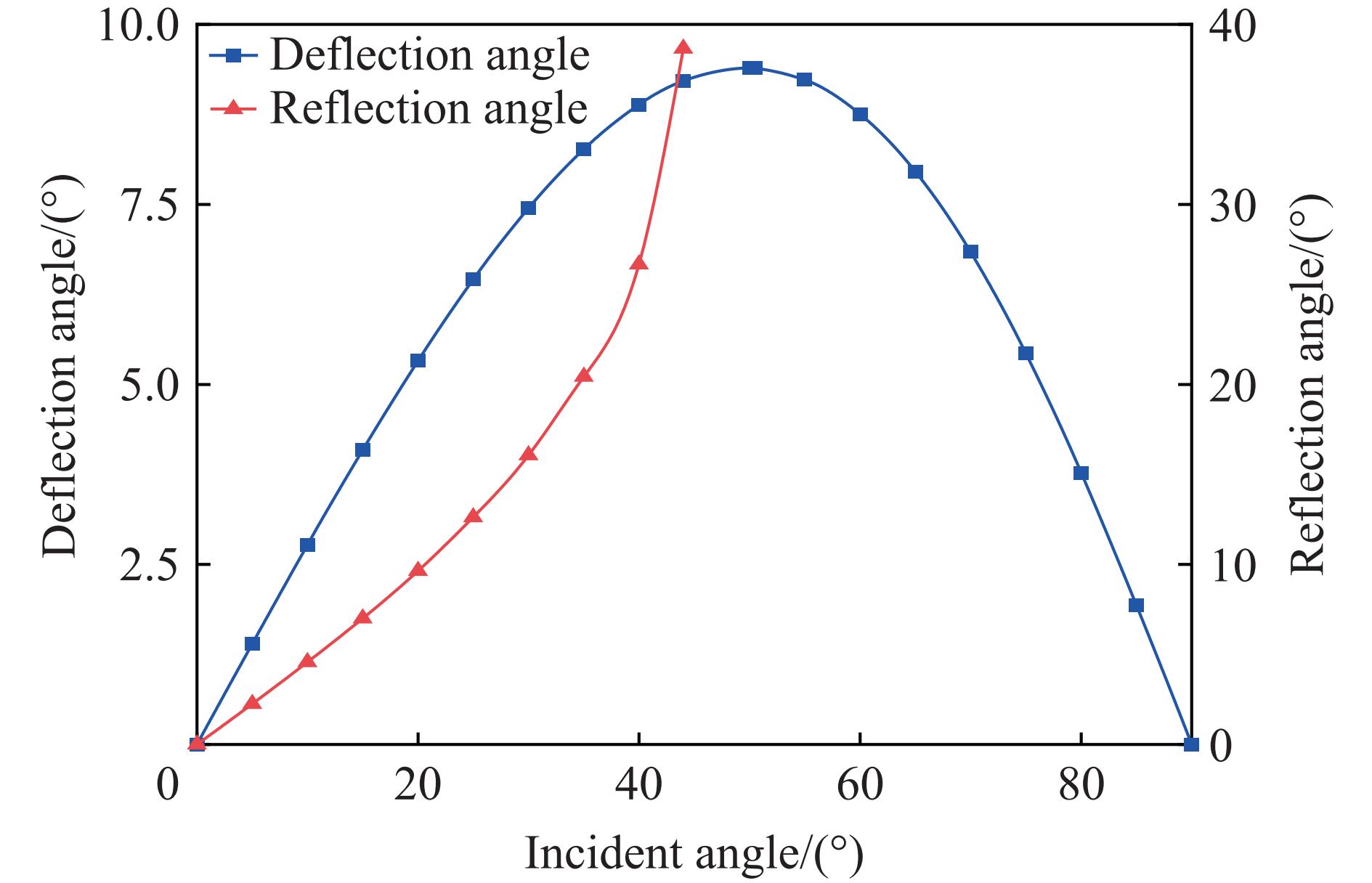
ρe =1.18 g/cm3。由式(14)可得绝热指数k=2.56,通过式(6)和式(11)可以计算出应力波入射角φ0 与偏转角θ 及反射角φ2 的关系,如图4所示。可以看出:随着入射角φ0 的增大,偏转角θ 先增大后减小,当入射角φ0 为50°时,偏转角θ 达到最大值9.3°;反射角φ2 随着入射角的增大呈指数型增大,当入射角φ0 为44°时,通过式(11)求解的反射角出现负数解,即当应力波入射角达44°后不再产生反射波。本文中,过度压缩系数
η 取1,根据式(4)、式(10)和式(13),计算不同碰撞条件下碰撞后(碰撞后压力为p)与应力波稳定传播时的压力(正、斜碰撞后应力波稳定传播时的压力为ph)比,如图5所示。可以看出,当两应力波从正碰撞演变为斜碰撞的过程中,随着入射角φ0 的增大,压力比呈现明显上升趋势,最大压力比为4.1;斜碰撞时,压力比逐渐减小但不小于1.0。当φ0 ≥44°时,发生马赫反射,压力比再次增大到3.5;当φ0 =90°时,压力比降为1.0。2. 数值模拟
2.1 模型与参数
为了探明交错、孔底和孔口起爆条件下孔间应力波的作用机制和破岩效果,建立如图6所示的有限元模型,模型长12 m,宽6 m,高12 m,炮孔间距5 m,孔径0.1 m,孔深8 m,堵塞段长度为2 m,装药采用耦合装药。炮孔中部为椭球砾石,长半径和短半径分别为1.6和1.2 m。利用HyperMesh软件进行网格划分,六面体网格数量为8.85×105。通过关键字*INITIAL_DETONATION控制每个炮孔的起爆位置和起爆时间。交错起爆时,左侧和右侧炮孔起爆点分别设在药柱顶部和底部,并设置两起爆点在零时刻同时起爆;孔口起爆条件下,左侧炮孔在零时刻起爆;孔底起爆条件下,右侧炮孔在t=3 ms时起爆。为模拟真实的爆破效果,模型四周和底部采用无反射边界,顶部采用自由边界。
岩体采用Riedel等[23]提出的RHT材料模型。该模型充分考虑了岩石在破坏过程中的应变硬化、应变率敏感性和压缩损伤软化等特性,是针对岩石等脆性材料的先进拉压损伤模型,被广泛应用于岩石的爆破损伤模拟。
RHT模型引入了最大失效面、弹性极限面和残余失效面等控制破坏面,其失效方程为[24]:
σ∗eq(pf,γ,˙ε)=Y∗TXC(pf)R3(γ)Frate(˙ε) (15) 式中:
pf 、γ 和˙ε 分别为压力、Lode角和应变率,R3(γ) 为偏平面上的角偶函数,Frate(˙ε) 为应变率强化因子,Y∗TXC(pf) 为压缩子午线上的等效应力强度。Y∗TXC(pf) 的表达式为:Y∗TXC(pf)=A[p∗−p∗spallFrate(˙ε)]N (16) 式中:
p∗spall =pspall/ƒc为归一化强度,其中pspall为层裂强度,ƒc为单轴抗压强度;p∗ 为标准化静水压力;A、N为材料常数。RHT模型通过增加塑性应变率来考虑材料的应变率效应,其抗压和抗拉动力增大因子可表示为:
Frate(˙ε)={(˙ε˙ε0)αpf≥fc/3p−ft/3fc/3−ft/3(˙ε˙ε0)α+p−fc/3ft/3−fc/3(˙ε˙ε0)βft/3<p<fc/3(˙ε˙ε0)βpf≤ft/3 (17) 式中:
α 为压缩应变率因子,β 为拉伸应变率因子,˙ε0 为失效应变率,ƒt为单轴抗拉强度。描述残余破坏面时引入损伤变量
D ,假定损伤是非弹性偏应变的累积[25]:D=∑(Δεp/εfailurep) (18) εfailurep=D1(p∗−p∗spall)D2≥εmin (19) 式中:
Δεp 为塑性应变增量,εmin 为最小失效应变,D1为损伤参数,D2为损伤指数。RHT模型采用典型的
pf -α状态方程描述压力与材料密度以及内能之间的关系:pf=A1δ+A2δ2+A3δ3+(B0+B1δ)ρpepδ>0 (20) pf=T1δ+T2δ2+B0ρpepδ<0 (21) 式中:
δ 为体积变化相关系数,δ>0 时表示体积压缩,δ<0 时表示体积膨胀;ρp 为体积材料的初始参考密度;ep 为比内能;A1 、A2 、A3 、B0 、B1 、T1 、T2 为材料参数[26]。岩石和砾石的RHT材料模型参数列于表1,其中α0为初始孔隙率,αP为孔隙率指数,f∗t 为拉压强度比,f∗s 为剪压强度比,Gel为剪切模量,pcr为破碎压力,pco为压实压力,˙ε0C 为参考拉伸应变率,˙ε0T 为参考压缩应变率,˙εC 为失效拉伸应变率,˙εT 为失效压缩应变率,εero为侵蚀体积应变参数,Q0为拉压子午比参数,B为罗德角相关系数,Gc*为压缩屈服面参数,Gt*为拉伸屈服面参数,x为剪切模量缩减系数,Af为残余面参数,Nf为残余面指数。表 1 岩石和砾石的RHT主要参数Table 1. Main RHT parameters of rock and gravel材料 fc/MPa ρp/(kg·m−3) α0 αP f∗t f∗s Gel/GPa pcr/MPa pco/GPa ˙ε0C/s−1 ˙ε0T/s−1 ˙εC/s−1 岩石 64 2500 1.2 3 0.06 0.25 12 60 9 3×10−5 3×10−6 3×1025 砾石 116 2800 1.05 0.04 材料 ˙εT/s−1 εero A1/GPa A2/GPa A3/GPa B0 B1 T1/GPa T2/GPa A N Q0 岩石 3×1025 2 40 57.6 23.6 1.22 1.22 40 0 1.6 0.61 0.68 砾石 材料 B α β Gc* Gt* x D1 D2 εmin Af Nf 岩石 0.0105 0.026 0.031 0.53 0.7 0.5 0.02 1 0.010 1.6 0.61 砾石 0.008 采用LS-DYNA中的关键字*MAT_HIGH_EXPLOSIVE_BURN模拟高能炸药,通过JWL状态方程实现炸药爆轰过程的模拟[27],JWL状态方程如下:
pJ=AJ(1−ωR1V)e−R1V+BJ(1−ωR2V)e−R2V+ωE0V (22) 式中:
pJ 为爆轰产物压力,AJ、BJ、R1、R2和ω 为JWL状态方程的独立常数,V 为相对体积,E0 为初始比内能。炸药的模型参数列于表2,其中ρJ为炸药密度,vJ为爆速,pCJ为Chapman-Jouguet压力。表 2 炸药模型参数Table 2. Explosive model parametersρJ/(kg·m−3) vJ/(m·s−1) pCJ/GPa AJ/GPa BJ/GPa R1 R2 ω E0/(kJ·m−3) 0.931 4160 5.15 49.46 1.891 3.907 1.118 0.333 3.87 2.2 孔间岩体的损伤特征
选取图6中垂直于z轴的炮孔中心截面观察交错起爆、孔底起爆和孔口起爆方式下岩体的爆破损伤结果,如图7所示。可以看出,不同起爆方式下岩体的损伤特征存在明显差异。交错起爆时,孔口附近的损伤范围较小,而炮孔底部的损伤范围较大;孔底起爆时,孔口附近的损伤范围明显大于孔底,且在临空面处出现损伤;孔口起爆时,在临空面处形成一条明显的损伤带,主要是因为传至孔口的应力波遇到临空面后反射形成拉应力波,岩石被拉裂。
为了进一步探明不同起爆方式的爆破破岩效果,观察椭球砾石部分的损伤分布,如图8所示。可以看出,3种起爆方式下砾石的顶端和底部均产生较大面积的损伤。交错起爆时,砾石的中部产生两条具有一定倾角的损伤带,孔底和孔口起爆时这一特征不明显。相较于孔底和孔口起爆,交错起爆时砾石的1/2剖面损伤更加严重。这表明交错起爆有利于含椭球砾石岩体的爆破破碎。
图9显示了3种起爆方式下椭球砾石中部相同单元的爆压时程曲线。可以看出,交错起爆时,压力峰值接近13 MPa。孔口和孔底起爆时的压力时程曲线较为相近,压力峰值均约为7 MPa。交错起爆时的爆压明显高于孔底和孔口起爆时的爆压,应力波碰撞的增强效应促进了椭球砾石的破碎。
利用WipFrag图像块度分析系统[28]对不同起爆方式下砾石中部的剖面损伤进行裂纹识别,数据处理后,获得砾石的块度分布,如图10所示。可以看出,交错起爆时, r<500 mm(r为岩块的粒径)的石块比例为50.0%,r750 mm的石块比例为15.7%;孔底和孔口起爆时,r<500 mm的石块比例分别为34.8%和21.3%,r750mm的石块比例分别为32.0%和39.8%。交错起爆时,较小(较大)粒径的石块比例大于(小于)孔底和孔口起爆。这表明交错起爆可以改善岩石的破碎效果。
3. 工程应用
西昌太和铁矿是攀西四大钒钛磁铁矿之一,铁矿石储量达到9亿吨,是国内特大型矿山之一,其岩体主要为下伏辉长岩,砾石含量为5%~56%,在岩体中随机分布,如图11所示。砾石的强度极高,采用孔底和孔口等常规爆破方式时,岩体中的砾石难以被破碎,大块较多,二次破碎成本极高。为了解决这一问题,采用交错起爆技术爆破岩体。
为了研究交错起爆的爆破破岩效果,采用电子雷管进行现场爆破试验,并在地质条件相似的区域进行传统起爆试验作为对比。炮孔直径为165 mm,相邻炮孔的间距为6.5 m,孔深为12 m,堵塞段长度为5 m。采用耦合装药结构的乳化炸药进行爆破试验。数码电子雷管分别置于相邻炮孔的上部和下部,并设置相同的起爆时间。对比组采用孔底起爆方式,电子雷管置于炮孔底部,两电子雷管的起爆时间间隔为9 ms。在两炮孔后方5 m处布置一个测试孔,测试孔顶部不设填塞,测试孔孔口采用护孔编织袋保护,防止碎石落入测试孔内导致卡孔。采用TP-sxzyc型压力传感器获取爆炸时的信号,由采集仪转为压力数据。为了防止后拉破坏导致测试孔坍塌进而损坏传感器,采用PVC套管保护传感器以上的炮孔。将绑有压力传感器的竹片放入测试孔中的预设位置,并保持悬空状态,压力传感器距离竹片底部一定距离。图12显示了现场仪器布置。
图13显示了现场爆破后爆堆中的大块砾石形态。可以看出:孔底起爆时,砾石基本没有破碎痕迹,仍然是一个整体;交错起爆时,岩体中夹杂的砾石被几条裂纹贯穿,砾石破碎成4块。这表明,相较于传统起爆方式,交错起爆可以有效促进含椭球砾石的破碎,满足铲装设备直接挖装的要求。
图14显示了测试孔附近的爆压时程曲线。可以看出,现场试验交错起爆时的爆压峰值可达17 MPa,大于孔底起爆时的爆压峰值(10 MPa)。爆压持续时间约10 ms,与Raina等[29-30]的测试结果(8~15 ms)接近。交错起爆方式下的应力波碰撞有效地增强了爆破压力,促进了椭球砾石(图13)的破碎。
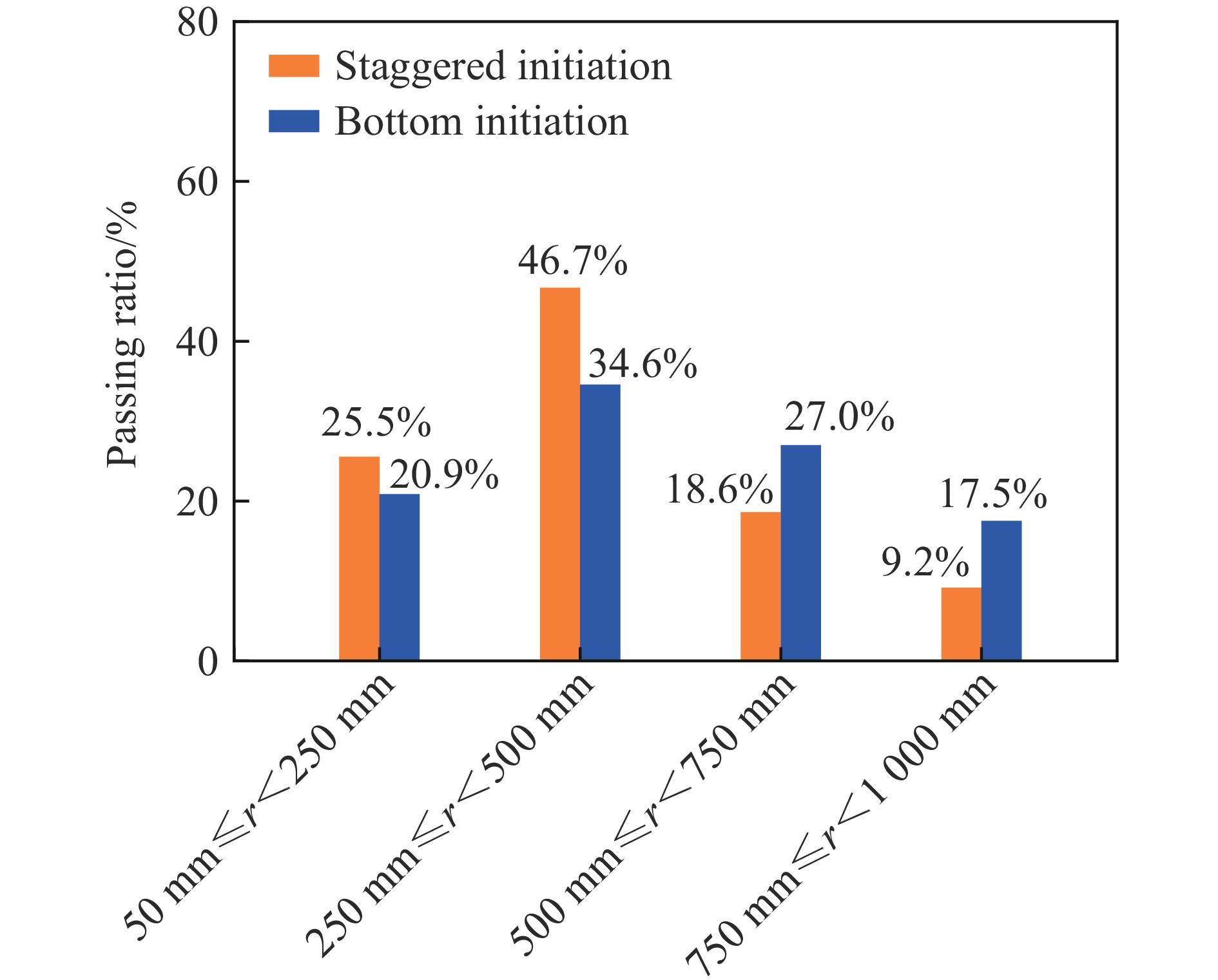
图15显示了不同起爆方式下爆破块度分布。可以看出:交错起爆时, r<250 mm和 r<500 mm的石块比例为25.5%和72.2%,r750 mm的石块比例为9.2%;孔底起爆时,r<250 mm和r<500 mm的石块比例为20.9%和55.5%,r750 mm的石块比例为17.5%。交错起爆时,较小粒径的岩块通过率高于孔底起爆,且较大粒径岩块比例比孔底起爆低。从图16可以看出,交错起爆时的岩块平均尺寸明显小于孔底起爆。相较于孔底起爆,交错起爆有更好的破岩效果。
4. 结 论
通过理论分析、数值模拟和现场试验,对比分析了交错起爆、孔底起爆和孔口起爆方式下的爆破破岩效果,得到以下主要结论。
(1) 双孔交错起爆时,两应力波首先在孔间正碰撞,碰撞后与应力波稳定传播时的压力比为2.1;当入射角在0°~44°区间时,应力波斜碰撞,压力比突增到4.1,然后降低至2.3;当入射角在44°~90°区间时,压力比由3.5降至1.0。双孔交错起爆体现出明显的应力波碰撞增强效应。
(2) 交错起爆和孔底起爆方式下爆破块度尺寸小于250 mm的比例分别为25.5%和20.9%,爆破块度尺寸大于750 mm的比例分别为9.2%和17.5%。交错起爆可以有效地降低大粒径占比,有利于改善爆破破岩效果。
本文中,仅研究了单个椭球砾石处于孔间中心位置时,不同起爆方式对含砾石岩体爆破破碎的影响,当岩体中砾石的形状、数量改变时,相关研究有待进一步完善。
-
表 1 实验方案
Table 1. Experimental scheme
冻融循环次数 T/℃ ˙ε/s−1 0 −20 550,450,350 −15 550 −10 550 1 −20 550,450,350 −15 550 −10 550 3 −20 550,450,350 −15 550 −10 550 5 −20 550,450,350 −15 550 −10 550 表 2 冻融循环冻土冲击实验结果
Table 2. Experimental results of frozen soil with freeze-thaw cycles under impact loading
冻融循环次数 T/℃ ˙ε/s−1 实验1 实验2 实验3 σp/MPa εp/\% σp/MPa εp/\% σp/MPa εp/\% 0 −10 550 6.74 4.07 7.16 4.13 6.96 4.11 −15 550 8.71 4.21 8.42 4.14 8.53 3.91 −20 350 8.55 2.34 8.29 2.54 8.19 2.45 450 9.67 3.36 9.78 3.25 10.11 3.68 550 11.13 4.34 11.06 4.18 10.69 4.29 1 −10 550 6.22 4.16 5.91 4.31 6.42 4.20 −15 550 7.75 3.96 7.55 3.91 7.82 4.12 −20 350 7.48 2.39 7.64 2.58 7.51 2.44 450 8.37 3.43 8.64 3.15 8.74 3.25 550 9.61 4.10 9.51 4.13 9.81 4.07 3 −10 550 5.96 4.13 6.40 4.15 6.41 4.26 −15 550 7.41 4.19 7.95 4.24 7.11 4.11 −20 350 6.72 2.74 7.03 2.51 7.11 2.82 450 8.97 3.41 8.54 3.38 8.62 3.45 550 9.31 4.23 9.54 4.11 9.31 4.08 5 −10 550 6.15 4.32 6.32 4.23 5.92 4.22 −15 550 7.42 4.28 7.12 4.18 7.71 4.13 −20 350 7.11 2.28 7.02 2.21 7.21 2.34 450 8.54 3.08 8.61 3.03 8.54 2.94 550 9.62 4.13 9.36 4.11 9.51 3.92 表 3 本构模型参数 (
T=−20∘C )Table 3. Constitutive model parameters (
T=−20∘C )冻融循环次数 ˙ε/s−1 E0/GPa E2/GPa θ2/μs εf m f 0 550 1.636 11.23 0.705 0.0131 1.16 1.000 450 1.667 7.36 0.971 0.0116 1.23 1.000 350 1.606 4.19 2.863 0.0088 1.23 1.000 1 550 1.655 9.16 0.671 0.0129 1.33 0.871 450 1.560 8.63 0.919 0.0114 1.32 0.871 350 1.624 4.45 2.721 0.0086 1.33 0.871 3 550 1.732 10.23 0.513 0.0139 1.13 0.847 450 1.630 13.21 0.541 0.0122 1.21 0.847 350 1.652 13.52 0.779 0.0091 1.11 0.847 5 550 1.648 14.51 0.542 0.0137 1.14 0.852 450 1.625 11.01 0.467 0.0119 1.07 0.852 350 1.626 14.06 0.467 0.0091 1.17 0.852 表 4 本构模型参数 (
˙ε=550s−1 )Table 4. Constitutive model parameters (
˙ε=550s−1 )冻融循环次数 T/℃ E0/GPa E2/GPa θ2/μs εf m f 0 −20 1.636 11.23 0.705 0.0131 1.16 1.000 −15 1.522 7.25 0.577 0.0134 1.03 1.000 −10 1.340 13.22 0.127 0.0131 1.02 1.000 1 −20 1.655 9.16 0.671 0.0129 1.34 0.871 −15 1.531 16.12 0.209 0.0129 1.12 0.888 −10 1.335 9.01 0.151 0.0131 1.05 0.939 3 −20 1.732 10.23 0.512 0.0139 1.13 0.847 −15 1.541 10.39 0.257 0.0134 1.14 0.881 −10 1.381 4.07 0.397 0.0134 1.02 0.893 5 −20 1.648 14.50 0.542 0.0137 1.14 0.852 −15 1.455 8.86 0.623 0.0134 1.01 0.875 −10 1.153 10.83 0.411 0.0131 1.06 0.878 -
[1] FRENCH H M. The periglacial environment [M]. 4th ed. Hoboken: John Wiley & Sons, 2017. [2] RAN Y H, LI X, CHENG G D, et al. Distribution of permafrost in China: an overview of existing permafrost maps [J]. Permafrost and Periglacial Processes, 2012, 23(4): 322–333. DOI: 10.1002/ppp.1756. [3] 马巍, 徐学祖, 张立新. 冻融循环对石灰粉土剪切强度特性的影响 [J]. 岩土工程学报, 1999, 21(2): 158–160. DOI: 10.3321/j.issn:1000-4548.1999.02.005.MA W, XU X Z, ZHANG L X. Influence of frost and thaw cycles on shear strength of lime silt [J]. Chinese Journal of Geotechnical Engineering, 1999, 21(2): 158–160. DOI: 10.3321/j.issn:1000-4548.1999.02.005. [4] LEE W, BOHRA N C, ALTSCHAEFFL A G, et al. Resilient modulus of cohesive soils and the effect of freeze-thaw [J]. Canadian Geotechnical Journal, 1995, 32(4): 559–568. DOI: 10.1139/t95-059. [5] 王大雁, 马巍, 常小晓, 等. 冻融循环作用对青藏粘土物理力学性质的影响 [J]. 岩石力学与工程学报, 2005, 24(23): 4313–4319. DOI: 10.3321/j.issn:1000-6915.2005.23.018.WANG D Y, MA W, CHANG X X, et al. Physico-mechanical properties changes of Qinghai-Tibet clay due to cyclic freezing and thawing [J]. Chinese Journal of Rock Mechanics and Engineering, 2005, 24(23): 4313–4319. DOI: 10.3321/j.issn:1000-6915.2005.23.018. [6] HOTINEANU A, BOUASKER M, ALDAOOD A, et al. Effect of freeze-thaw cycling on the mechanical properties of lime-stabilized expansive clays [J]. Cold Regions Science and Technology, 2015, 119: 151–157. DOI: 10.1016/j.coldregions.2015.08.008. [7] 苏谦, 唐第甲, 刘深. 青藏斜坡黏土冻融循环物理力学性质试验 [J]. 岩石力学与工程学报, 2008, 27(S1): 2990–2994.SU Q, TANG D J, LIU S. Test on physico-mechanical properties of Qinghai-Tibet slope clay under freezing-thawing cycles [J]. Chinese Journal of Rock Mechanics and Engineering, 2008, 27(S1): 2990–2994. [8] 穆彦虎, 陈涛, 陈国良, 等. 冻融循环对黏质粗粒土抗剪强度影响的试验研究 [J]. 防灾减灾工程学报, 2019, 39(3): 375–386. DOI: 10.13409/j.cnki.jdpme.2019.03.002.MU Y H, CHEN T, CHEN G L, et al. Experimental study on effect of cyclic freeze-thaw on shear behaviors of clayey coarse-grained soil [J]. Journal of Disaster Prevention and Mitigation Engineering, 2019, 39(3): 375–386. DOI: 10.13409/j.cnki.jdpme.2019.03.002. [9] 齐吉琳, 程国栋, VERMEER P A. 冻融作用对土工程性质影响的研究现状 [J]. 地球科学进展, 2005, 20(8): 887–894. DOI: 10.3321/j.issn:1001-8166.2005.08.010.QI J L, CHENG G D, VERMEER P A. State-of-the-art of influence of freeze-thaw on engineering properties of soils [J]. Advances in Earth Science, 2005, 20(8): 887–894. DOI: 10.3321/j.issn:1001-8166.2005.08.010. [10] ZHOU Z W, MA W, ZHANG S J, et al. Effect of freeze-thaw cycles in mechanical behaviors of frozen loess [J]. Cold Regions Science and Technology, 2018, 146: 9–18. DOI: 10.1016/j.coldregions.2017.11.011. [11] XU X T, ZHANG W D, FAN C X, et al. Effect of freeze-thaw cycles on the accumulative deformation of frozen clay under cyclic loading conditions: experimental evidence and theoretical model [J]. Road Materials and Pavement Design, 2021, 22(4): 925–941. DOI: 10.1080/14680629.2019.1696221. [12] FAN C X, ZHANG W D, LAI Y, et al. Mechanical behaviors of frozen clay under dynamic cyclic loadings with freeze-thaw cycles [J]. Cold Regions Science and Technology, 2021, 181: 103184. DOI: 10.1016/j.coldregions.2020.103184. [13] LEE M Y, FOSSUM A F, COSTIN L S, et al. Frozen soil material testing and constitutive modeling [R]. Albuquerque: Sandia National Laboratory, 2002. DOI: 10.2172/793403. [14] ZHANG F L, ZHU Z W, FU T T, et al. Damage mechanism and dynamic constitutive model of frozen soil under uniaxial impact loading [J]. Mechanics of Materials, 2020, 140: 103217. DOI: 10.1016/j.mechmat.2019.103217. [15] MA D D, XIANG H S, MA Q Y, et al. Dynamic damage constitutive model of frozen silty soil with prefabricated crack under uniaxial load [J]. Journal of Engineering Mechanics, 2021, 147(6): 04021033. DOI: 10.1061/(Asce)Em.1943-7889.0001933. [16] SHANGGUAN Z H, ZHU Z W, TANG W R. Dynamic impact experiment and numerical simulation of frozen soil with prefabricated holes [J]. Journal of Engineering Mechanics, 2020, 146(8): 04020085. DOI: 10.1061/(Asce)Em.1943-7889.0001821. [17] TANG W R, ZHU Z W, FU T T, et al. Dynamic experiment and numerical simulation of frozen soil under confining pressure [J]. Acta Mechanica Sinica, 2020, 36(6): 1302–1318. DOI: 10.1007/s10409-020-00999-4. [18] WANG D Y, MA W, NIU Y H, et al. Effects of cyclic freezing and thawing on mechanical properties of Qinghai-Tibet clay [J]. Cold Regions Science and Technology, 2007, 48(1): 34–43. DOI: 10.1016/j.coldregions.2006.09.008. [19] XU J, LI Y F, LAN W, et al. Shear strength and damage mechanism of saline intact loess after freeze-thaw cycling [J]. Cold Regions Science and Technology, 2019, 164: 102779. DOI: 10.1016/j.coldregions.2019.05.005. [20] JI Y K, ZHOU G Q, HALL M R. Frost heave and frost heaving-induced pressure under various restraints and thermal gradients during the coupled thermal-hydro processes in freezing soil [J]. Bulletin of Engineering Geology and the Environment, 2019, 78(5): 3671–3683. DOI: 10.1007/s10064-018-1345-z. [21] XIA K W, YAO W. Dynamic rock tests using split Hopkinson (Kolsky) bar system–a review [J]. Journal of Rock Mechanics and Geotechnical Engineering, 2015, 7(1): 27–59. DOI: 10.1016/j.jrmge.2014.07.008. [22] ZHANG F L, ZHU Z W, MA W, et al. A unified viscoplastic model and strain rate-temperature equivalence of frozen soil under impact loading [J]. Journal of the Mechanics and Physics of Solids, 2021, 152: 104413. DOI: 10.1016/j.jmps.2021.104413. [23] LEE S, KIM K M, PARK J, et al. Pure rate effect on the concrete compressive strength in the split Hopkinson pressure bar test [J]. International Journal of Impact Engineering, 2018, 113: 191–202. DOI: 10.1016/j.ijimpeng.2017.11.015. [24] 董凯, 任辉启, 阮文俊, 等. 珊瑚砂应变率效应研究 [J]. 爆炸与冲击, 2020, 40(9): 093102. DOI: 10.11883/bzycj-2019-0432.DONG K, REN H Q, RUAN W J, et al. Study on strain rate effect of coral sand [J]. Explosion and Shock Waves, 2020, 40(9): 093102. DOI: 10.11883/bzycj-2019-0432. [25] 巫绪涛, 胡时胜, 陈德兴, 等. 钢纤维高强混凝土冲击压缩的试验研究 [J]. 爆炸与冲击, 2005, 25(2): 125–131. DOI: 10.11883/1001-1455(2005)02-0125-07.WU X T, HU S S, CHEN D X, et al. Impact compression experiment of steel fiber reinforced high strength concrete [J]. Explosion and Shock Waves, 2005, 25(2): 125–131. DOI: 10.11883/1001-1455(2005)02-0125-07. [26] ZHU Z W, KANG G Z, MA Y, et al. Temperature damage and constitutive model of frozen soil under dynamic loading [J]. Mechanics of Materials, 2016, 102: 108–116. DOI: 10.1016/j.mechmat.2016.08.009. [27] 陈柏生, 胡时胜, 马芹永, 等. 冻土动态力学性能的实验研究 [J]. 力学学报, 2005, 37(6): 724–728. DOI: 10.6052/0459-1879-2005-6-2004-450.CHEN B S, HU S S, MA Q Y, et al. Experimental research of dynamic mechanical behaviors of frozen soil [J]. Chinese Journal of Theoretical and Applied Mechanics, 2005, 37(6): 724–728. DOI: 10.6052/0459-1879-2005-6-2004-450. [28] LI B, ZHU Z W, NING J G, et al. Viscoelastic-plastic constitutive model with damage of frozen soil under impact loading and freeze-thaw loading [J]. International Journal of Mechanical Sciences, 2022, 214: 106890. DOI: 10.1016/j.ijmecsci.2021.106890. [29] 姜亚成, 周磊, 朱哲明, 等. 冻融循环对含纯Ⅰ型裂隙围岩的动态起裂特性影响规律 [J]. 爆炸与冲击, 2021, 41(4): 043104. DOI: 10.11883/bzycj-2020-0330.JIANG Y C, ZHOU L, ZHU Z M, et al. Effects of freeze-thaw cycles on dynamic fracture initiation characteristics of surrounding rock with pure Ⅰ type fracture under impact loads [J]. Explosion and Shock Waves, 2021, 41(4): 043104. DOI: 10.11883/bzycj-2020-0330. [30] JIN S S, ZHENG G P, YU J. A micro freeze-thaw damage model of concrete with fractal dimension [J]. Construction and Building Materials, 2020, 257: 119434. DOI: 10.1016/j.conbuildmat.2020.119434. [31] ZHANG Z Y, LIU Q, WU Q, et al. Damage evolution of asphalt mixture under freeze-thaw cyclic loading from a mechanical perspective [J]. International Journal of Fatigue, 2021, 142: 105923. DOI: 10.1016/j.ijfatigue.2020.105923. [32] ZENG W, DING Y N, ZHANG Y L, et al. Effect of steel fiber on the crack permeability evolution and crack surface topography of concrete subjected to freeze-thaw damage [J]. Cement and Concrete Research, 2020, 138: 106230. DOI: 10.1016/j.cemconres.2020.106230. [33] GONG F Y, JACOBSEN S. Modeling of water transport in highly saturated concrete with wet surface during freeze/thaw [J]. Cement and Concrete Research, 2019, 115: 294–307. DOI: 10.1016/j.cemconres.2018.08.013. [34] SUN M, ZOU C Y, XIN D B. Pore structure evolution mechanism of cement mortar containing diatomite subjected to freeze-thaw cycles by multifractal analysis [J]. Cement and Concrete Composites, 2020, 114: 103731. DOI: 10.1016/j.cemconcomp.2020.103731. [35] LÖVQVIST L, BALIEU R, KRINGOS N. A thermodynamics-based model for freeze-thaw damage in asphalt mixtures [J]. International Journal of Solids and Structures, 2020, 203: 264–275. DOI: 10.1016/j.ijsolstr.2020.07.021. [36] 徐光苗, 刘泉声. 岩石冻融破坏机理分析及冻融力学试验研究 [J]. 岩石力学与工程学报, 2005, 24(17): 3076–3082. DOI: 10.3321/j.issn:1000-6915.2005.17.012.XU G M, LIU Q S. Analysis of mechanism of rock failure due to freeze-thaw cycling and mechanical testing study on frozen-thawed rocks [J]. Chinese Journal of Rock Mechanics and Engineering, 2005, 24(17): 3076–3082. DOI: 10.3321/j.issn:1000-6915.2005.17.012. [37] FU T T, ZHU Z W, CAO C X. Constitutive model of frozen-soil dynamic characteristics under impact loading [J]. Acta Mechanica, 2019, 230(5): 1869–1889. DOI: 10.1007/s00707-019-2369-6. [38] CHOI K S, PAN J. A generalized anisotropic hardening rule based on the Mroz multi-yield-surface model for pressure insensitive and sensitive materials [J]. International Journal of Plasticity, 2009, 25(7): 1325–1358. DOI: 10.1016/j.ijplas.2008.09.005. [39] WANG L L. Stress wave propagation for nonlinear viscoelastic polymeric materials at high strain rates [J]. Chinese Journal of Mechanics-Series A, 2003, 19(1): 177–183. DOI: 10.1017/s1727719100004184. [40] ZHU Z W, FU T T, ZHOU Z W, et al. Research on Ottosen constitutive model of frozen soil under impact load [J]. International Journal of Rock Mechanics and Mining Sciences, 2021, 137: 104544. DOI: 10.1016/j.ijrmms.2020.104544. [41] 王礼立. 爆炸/冲击动力学学习研究中的若干疑惑 [J]. 爆炸与冲击, 2021, 41(1): 011401. DOI: 10.11883/bzycj-2020-0415.WANG L L. Some doubts in studying explosion/impact dynamics [J]. Explosion and Shock Waves, 2021, 41(1): 011401. DOI: 10.11883/bzycj-2020-0415. 期刊类型引用(10)
1. 杨赛群,葛立芳,李洪伟,夏治园. 地应力影响下的五孔掏槽爆破岩石损伤效应研究. 火工品. 2025(01): 89-96 .  百度学术
百度学术2. 汤智力. 隧道楔形掏槽爆破延期时间优化与现场试验研究. 施工技术(中英文). 2024(24): 25-30 .  百度学术
百度学术3. 冷振东,范勇,涂书芳,周桂松,郭一鸣. 电子雷管起爆技术研究进展与发展建议. 中国工程科学. 2023(01): 142-154 .  百度学术
百度学术4. 吴健春,高宇璠,全明威,聂启强,陈春,傅洪贤. 隧道电子雷管爆破近区振动测试研究. 云南水力发电. 2023(08): 34-39 .  百度学术
百度学术5. 李成孝,杨仁树,王雁冰,徐斌,左进京,谢平. 基于数值模拟的岩石巷道深孔分段装药掏槽爆破研究. 煤炭科学技术. 2023(09): 100-111 .  百度学术
百度学术6. 刘树国. 数码雷管对隧道爆破工程造价的影响研究. 铁道建筑技术. 2023(10): 180-183 .  百度学术
百度学术7. 刘翔宇,龚敏,杨仁树,吴昊骏,王思杰. 基于蒙特卡洛的电子雷管延期误差对隧道爆破振动影响研究. 振动与冲击. 2023(23): 192-198 .  百度学术
百度学术8. 项荣军,刘传鹏,李胜林,凌天龙. 隧道内部爆破振动传播规律与降振技术研究. 爆破. 2023(04): 82-88+200 .  百度学术
百度学术9. 孟彪,贾世杰,赵国强. 精确延时破岩机理综述. 科技创新与应用. 2022(10): 97-101 .  百度学术
百度学术10. 陈之兼,王铭锋,张阳阳. 电子雷管在某井下铁矿应用中存在的问题及对策. 煤矿爆破. 2022(03): 35-38 .  百度学术
百度学术其他类型引用(3)
-








 下载:
下载:







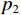























































































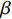



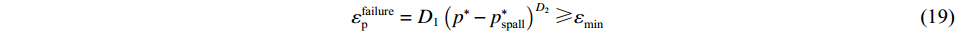




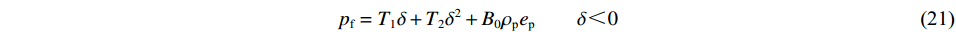

















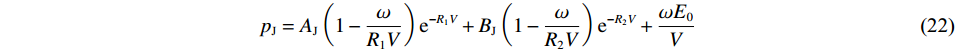















 下载:
下载:



















 百度学术
百度学术