Maximum stiffness topology optimization and dynamic response of a lightweight sandwich arch under impact load
-
摘要: 基于双向渐进结构优化方法(bi-directional evolutionary structural optimization,BESO)框架,将传统动态载荷优化法中的内外层迭代引入到ABAQUS-MATLAB平台集成优化中,改进动态载荷拓扑优化流程。对初速度为100 m/s的子弹冲击下的夹芯拱结构进行拓扑优化设计和动力学响应分析。优化后夹芯拱芯层的变形模式可分为3个对称的部分,跨中区域的中部和上部主要发生压缩变形,呈现类三角点阵桁架结构,边界区域上部发生拉伸变形,下部发生压缩变形,呈现C形型结构,跨中和边界之间的过渡区域以拉弯联合变形为主,呈现Y形结构。通过与两种等质量的拱结构对比,分析了3种结构在不同初速度的子弹冲击下结构的挠度以及芯层的能量吸收情况。结果表明:在相同的冲击速度下,优化后的结构挠度最小,芯层比吸能最高;当冲击速度较低时,优化后的结构的抗冲击性能优势并不明显;在所研究的冲击速度范围内,冲击速度越高,优化后结构的抗冲击性能越好。对比对称载荷与非对称载荷(冲击点偏移量为100%)下2种优化结构在不同载荷工况下的动态响应,结果表明:载荷工况不同,得到的最终优化结果也略有所不同,但在相同载荷下结构的响应相差较小,每种工况下得到的优化结果在相应工况下所展现的力学性能略优,但均明显优于传统结构。因此,在对称冲击载荷下优化所得的结构具有一定的普遍性。Abstract: Based on the bi-directional evolutionary structural optimization method (BESO), the nested loop structure of the traditional dynamic load optimization method was introduced into the ABAQUS-MATLAB platform integrated optimization to improve the dynamic load topology optimization process. Topological optimization design and dynamic response analysis of sandwich arch structure under the impact of projectile with initial velocity of 100 m/s were carried out. After optimization, the deformation mode of the core for sandwich arch can be divided into three symmetrical part: the compression dominated deformation occurs in the middle and the upper part of the mid-span region, which like the triangular lattice truss structure; the tensile and compression dominated deformation occurs in the upper and the lower part of the boundary region respectively, which presents the C-shaped structure; and the transition region, which presents the Y-shaped structure, between the mid-span and the boundary is dominated by the combination of tension and bending deformation. The dynamic response of the optimization results under the impact load was analyzed. The deflections of top and bottom sheets and energy absorption of core of two comparison models with equal mass (Voronoi aluminum foam sandwich arch and solid arch) and optimization arch structure under the impact load with the initial velocity of 100 m/s were compared. The deflection and specific energy absorption of the cores of the three models under the impact of the projectiles with the initial velocities of 100, 80, 50 and 20 m/s were compared. The results show that: under the same impact velocity, the optimization structure has the minimum deflection and the maximum specific energy absorption capability; while with the low impact velocity, the impact-resistance advantage of the optimization structure is not obvious. Furthermore, in the range of the impact velocity which has been studied, the optimization structure shows the better impact-resistance performance with the higher velocity. The dynamic responses of the two optimization structures with symmetric load and asymmetric load (the offset of impact point is 100%) under different load conditions were compared. The deflection of top and bottom sheets and the specific energy absorption of core of four models (symmetric optimization result, asymmetric optimization result, Voronoi aluminum foam sandwich arch and solid arch) were compared. The results show that: under different load conditions, the final optimization results are slightly different, and the different of structural responses under the same load is relatively small. The optimization results obtained under each working condition show slightly better mechanical properties under the corresponding condition, but optimization structures are significantly better than the traditional structures. Therefore, the structure optimized by symmetrical impact load has a certain universality.
-
Key words:
- topology optimization /
- impact load /
- sandwich arch /
- dynamic response /
- energy absorption
-
多孔夹芯结构作为一种具有高比刚度/比强度、轻质、抗冲击、吸能等功能特性的多功能一体化结构,在航空航天、交通运输、工程结构等领域都有着很好的应用前景。高孔隙率的多孔金属(泡沫[1-2]、蜂窝[3]、波纹板[4-5]、 点阵结构[6]等)由于其具有良好的声、热、电磁屏蔽等功能,优异的比强度/比刚度,良好的吸能缓冲特性,丰富多变的胞孔细观构型,作为夹芯结构芯层,可满足各种服役环境下的材料和结构一体化多功能协同设计需求。有关这类结构在冲击载荷作用下动力响应的研究[7-12]大部分集中在性能探索、应用开发和参数优化设计方面[13-14],对于设计空间更大的结构拓扑设计,还缺乏深入的研究。究其原因:首先,材料细观拓扑构型对宏观结构的影响机理复杂,缺乏针对性、专门性的设计目标;其次,优化过程中需要综合考虑载荷历程和结构弹塑性响应对结构设计变量的影响,涉及载荷的时空非线性和材料/结构的惯性效应、应变率效应,导致目标函数复杂,敏度分析困难;最后,制备具有专门设计且拓扑构型复杂的多孔材料难度较大。随着结构拓扑优化技术和3D打印技术的日渐成熟,冲击载荷下结构的拓扑优化设计与性能测试、评估受到越来越多学者的关注。
拓扑优化的主要思想是在给定的约束条件下,通过改变结构和材料的构型和分布,来实现结构和材料性能的提高。常用到的拓扑优化方法有变密度法[15-16]、均匀化法[17-18]和渐进结构优化法(evolutionary structural optimization, ESO)[19]。Querin等[20-21]对渐进结构法进行了改进与延伸,提出了双向渐进结构优化法(bi-directional evolutionary structural optimization,BESO),在优化过程中可以增添和删除单元。之后,Huang等[22-23]针对结构刚度优化,提出了一种基于灵敏度分析的改进BESO法,该方法解决很多拓扑优化中常见的问题,如棋盘格、优化收敛性等。
目前,拓扑优化大多集中在静态载荷优化,而工程应用中常见的是动态载荷。针对动载优化中出现的收敛困难、计算时间长等问题,Choi等[24]和Park等[25-26]提出了等效静态载荷法(equivalent static loads optimization, ESLO),建立双层拓扑优化流程,在外层中对动态载荷工况下的结构响应进行分析,并将动态载荷等效为具有相同位移响应的静态载荷,在内层中对等效静态载荷下的结构进行拓扑优化。蓝萌等[27]针对等效静态载荷法计算效率低、优化流程复杂的问题,将双向渐进结构优化法作为优化方法,对动态载荷优化流程进行了改进,提高了优化效率。王宪杰等[28]引入基于惩罚指数的材料插值方案,对传统BESO法进行了数值计算方面的改进,并分别对宏观结构、微观结构、材料/结构一体化等问题进行了多尺度、多相复合材料布局以及拓扑优化。闫琨[29]和Yan等[30]结合各类算法的优点,在线弹性范围内,提出了一种考虑初始载荷激励和时间效应对结构设计变量影响的灵敏度分析计算方法,利用该方法研究了残余振动最小化结构优化设计问题和结构耐撞性拓扑优化设计问题。
本文中,基于BESO法,将传统动态载荷优化法中的内外层迭代引入到ABAQUS-MATLAB平台集成优化中,改进动态载荷拓扑优化流程,对子弹冲击下的夹芯拱结构进行拓扑优化设计和动力学响应分析,并与传统泡沫铝夹芯拱和实体拱进行对比。
1. ABAQUS-MATLAB平台集成优化
利用BESO方法进行ABAQUS-MATLAB平台集成优化流程如图1所示,包括以下5个步骤。
(1)在ABAQUS中进行初始建模和网格划分,导出inp文件作为优化目标文件。
(2)在MATLAB中设置模型的优化参数:结构的体积分数、体积进化率和过滤半径等。
(3)算法中调用ABAQUS进行有限元分析,读取结果fil文件,并根据结果计算单元应变能灵敏度。
(4)将更新后的结构信息写入inp文件中,完成inp文件的更新,用于下一次的有限元分析。
(5)重复上述步骤(3)~(4),直至优化结果同时满足目标体积分数和收敛条件。
以最小柔度为目标函数、结构体积分数为约束条件,将设计域内的连续体结构离散为有限个单元。将每个单元的相对密度xi(xmin或1)设为优化设计变量,低效单元相对密度为xmin,其他单元相对密度为1。利用有限元分析,计算得出低效的单元,并将其移除。通过设置删除率,并一步步删除设计域中的低效单元,来实现结构优化。其数学模型如下:
C=FTU,V=n∑i=1xiVixi∈{xmin,1} (1) 式中:C为目标函数,即最小柔度值;F和U分别为结构的力和位移,F=KU,K为结构的刚度矩阵;V为当前结构总体积;
Vi 为第i个单元的体积;n为结构单元数;xi 为设计变量,是每个单元的相对密度;xmin为相对密度的极小值;当xi=xmin时,第i个单元为低效待删单元;当xi=1时,第i个单元为实体单元。2. 等效静态载荷法
2.1 原理
在动态载荷的位移u响应-时间图中,把时间分割成有限个时间点,将每个时间点与动态载荷产生相同位移的一组载荷集定义为等效静态载荷,如图2所示[12-14]。
从图2可以看出,动载荷下结构的位移响应u-t可以与等效静载荷
feq 在每个时间段上的位移响应完全一致。动态载荷响应分析方程为:M¨u(t)+c˙u(t)+Ku(t)=f(t) (2) 式中:M为结构的质量矩阵,c为结构的阻尼矩阵,K为结构的刚度矩阵,这3个矩阵都是关于设计变量x的函数;
¨u(t) 、˙u(t) 和u(t) 分别为结构在t时刻的加速度、速度和位移;f(t) 为结构在t时刻受到的外部动载荷。对式(2)进行变换,有:
Ku(t)=f(t)−M¨u(t)−c˙u(t) (3) 再对式(3)进行变换,将式(3)中的连续时间变量t等效为等效静态载荷中m个工况,可得:
fs,eq=Ku(s) (4) 式中:
fs,eq和u(s) 分别为第s个时间点的等效静态载荷和位移。式(4)将非线性动力分析等效变换为m个时间节点的线性静载优化。2.2 基于BESO法的等效静态载荷法
将建立的拓扑优化数学模型与等效静态载荷法结合,式(1)可改写为:
C=12m∑s=1fTs,equs,V=n∑i=1xiVixi∈{xmin,1} (5) 将上述优化模型应用于ABAQUS-MATLAB平台集成,图3为等效静载的BESO方法优化流程图。
(1)先给定优化设计变量以及优化参数:目标体积分数、进化率和惩罚指数等。
(2)建立2个相同的有限元初始模型:模型1为动态载荷工况,用于在ABAQUS中进行非线性分析;模型2为等效静载荷工况,用于MATLAB中进行双向渐进结构拓扑优化。采用双有限元模型虽然增加了平台之间的信息交互流程,但可以充分利用商业有限元软件强大的计算和前后处理能力,提高了计算精度,对几何结构较复杂的模型有更好的适用性。
(3)对模型1在冲击载荷工况下进行非线性响应分析,并提取不同时间节点上的结构响应(接触力、加速度、速度),构造与冲击载荷有相同位移场的等效静载荷
fs,eq,s=1,2,⋯,m ;由于结构受子弹冲击时,其外部动载荷f(t)未知,所以在获得等效静载荷之前,需要将子弹冲击载荷进行转化,如将其转化为接触力、子弹质量与其加速度乘积或者接触面初速度等,在MATLAB与ABAQUS信息交互中提取接触力数据转化为外部动载。(4)将得到的等效静载荷作为模型2的外载荷,在MATLAB中利用双向渐进结构优化法进行拓扑优化,并在MATLAB中进行内层优化迭代,直至完成每个时间节点的静态载荷工况。
(5)更新设计变量,将优化结果写入模型1,检测是否满足优化目标以及收敛条件。若满足,则结束;若不满足,则返回至步骤(3)。收敛条件为:
|N∑i=1(Ck−i+1−Ck−N−i+1)|N∑i=1Ck−i+1≤ε (6) 式中:
ε 为相对误差值,这里取0.01;k为当前迭代次数;N为自然数,通常N取5。则式(6)表示过去10步迭代中柔度的改变量应不超过0.01。3. 冲击载荷下夹芯拱结构的优化设计和响应分析
3.1 优化设计
冲击载荷是工程实际中较常见的外载荷。图4为两端固支的拱形二维夹芯梁结构,在上面板中心处受到初速度v0=100 m/s的子弹冲击,子弹宽度l0=5 mm。设计域是L×dc=120 mm×20 mm的中间拱芯层,拱半径R=185 mm;上、下面板为非设计域,面板厚度d=1 mm;将中间设计域划分为122×20个四边形单元,单元边长为1 mm。BESO方法中通过敏度过滤技术来对过滤半径内其他单元的敏度值进行加权平均,以修正中心单元的灵敏度,减少网格依赖性,可以有效避免因网格尺寸不同导致优化结果的差异[31]。
夹芯拱结构中间芯层和上、下面板材料都选择铝,其主要的力学性能参数[32]:密度为2.7 g/cm3,弹性模量为70 GPa,泊松比为0.3,屈服强度为80 MPa,剪切模量为700 MPa;子弹为密度为7.9 g/cm3的刚体。整个优化过程中不考虑材料的失效和破坏。
主要优化参数:目标体积分数为0.5;删除率为0.03;过滤半径为3。
采用双向渐进结构拓扑优化方法中的软杀法进行结构优化,为了避免刚度矩阵奇异化,用较小的相对密度来表示灵敏度数值低的待删除单元的相对密度,如取
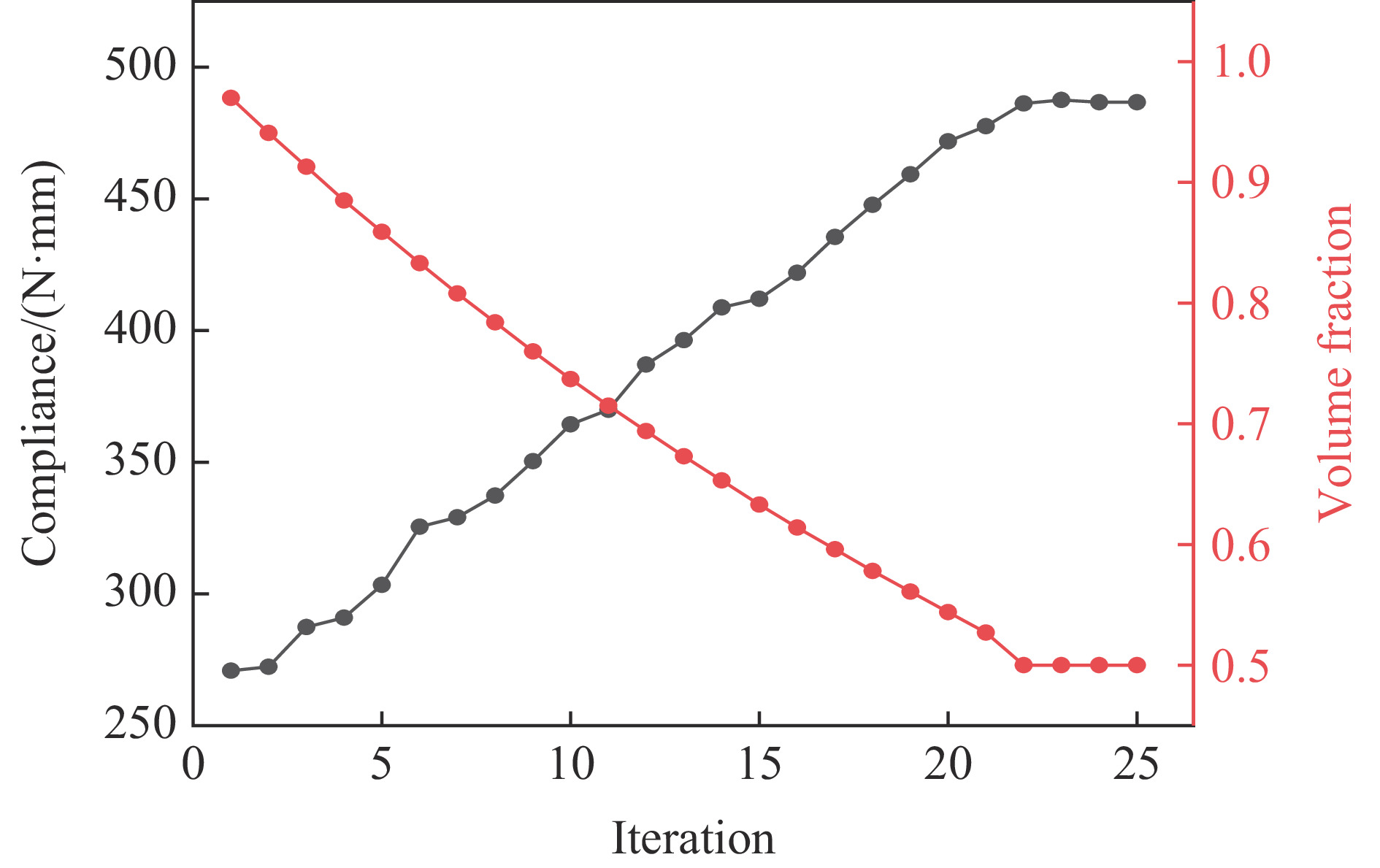
xmin 作为待删除单元的相对密度,同时在优化过程中赋予这些单元较小的弹性模量。在后续的有限元分析中,为避免这些待删单元出现畸变,优化结束后在HyperMesh中删去相对密度较小的单元。最终的优化结果如图5所示。芯层结构主要可分为3个部分:中间区域A,主要呈现出类似三角点阵夹芯结构的构型;过渡区域B,主要呈现出Y形构型;边界区域C,主要呈现C形构型。优化后的夹芯拱结构体积为其初始结构体积的50%,降低了用料成本,减轻了自重,而且优化后的结构对称,在主要承受载荷的位置材料分布较密集,受力合理,也较美观。将优化结果称为模型O。
图6为夹芯拱结构优化历程,夹芯拱结构经历25步外层迭代后收敛完成,即进行了25次非线性动力学分析,每次非线性冲击载荷被等效为25次线性静载,整体优化过程较稳定。
3.2 最终的优化结果在冲击载荷下的动态响应
如图7所示为冲击载荷下,夹芯结构根据上、下面板的速度特征[33],将芯层分为了2种类型。图7(a)所示为硬芯层夹芯结构,其主要特征为:上面板速度vt随时间不断减小,下面板速度vb随时间不断增大,芯层在这一阶段持续压缩,当t=teq时上、下面板速度达到一致,芯层不再压缩,上、下面板速度以相同频率减小。图7(b)所示为软芯层夹芯结构,其主要特征为:面板速度vt随时间不断减小,下面板速度vb随时间先增大,当t=tbd时下面板速度开始减小,当t=teq时上、下面板速度达到一致,芯层不再压缩,上、下面板速度以相同频率减小。
 图 7 不同类型芯层夹芯结构上、下面板速度时程曲线Figure 7. Velocity versus time histories of the mid-span of the top and bottom panels for two types of sandwich response[34]
图 7 不同类型芯层夹芯结构上、下面板速度时程曲线Figure 7. Velocity versus time histories of the mid-span of the top and bottom panels for two types of sandwich response[34]图8为初速度v0=100 m/s、宽度l0=5 mm的子弹冲击时,经过优化后的结构上、下面板速度时程曲线。从图8可以看出,经过优化后的夹芯结构上、下面板的速度特征与图7(a)的芯层特征较接近,说明经过优化后的芯层就有较高的刚度。但同时可以看出,在上、下面板以共同的速度运动之前,速度曲线出现多次相交,这是由于芯层材料分布不均匀,由图5可知,区域A及与区域B连接处材料分布较多,质量较大,受到惯性作用与弹性能释放的影响,下面板受到芯层的冲击作用较大。
优化结构的变形响应过程如图9所示。当t=0 ms时,子弹开始作用于上面板;当t=0.06 ms时,子弹作用区域处面板出现明显的凹陷变形,结合图9以及图5可以看出,芯层中间区域A承受较大压缩变形(图9中蓝色区域),由于芯层的特殊结构,芯层的变形主要以区域A上部凹陷,下部支撑受压向两侧扩展为主,此时下面板并未发生明显的变形。当t=0.10 ms时,压缩变形从A区域下部支撑向结构边界两侧传播,A区域下部横梁受拉开始变形,导致芯层区域B发生以拉弯联合作用的为主的旋转变形模式,B区域上面板向中部挤压,下面板向边界处挤压,均呈现出向上隆起的变形。当t=0.12 ms时,下面板的速度与上面板的相等。当t=0.14 ms时,下面板的速度达到最大值,此时下面板的速度高于前面板的。当t=teq≈0.25 ms时,区域B处上、下面板隆起变形进一步增加,芯层C区域上端出现拉伸变形,下端出现压缩屈曲变形模式,之后上、下面板以相同的速度运动;当t=0.58 ms时,上、下面板速度减小为0 m/s,上、下面板出现轻微的弹性回弹,结构整体在平衡位置(残余挠度)振动。
3.3 相同工况下优化结构与对照结构的响应分析
为了验证最终优化结果的抗冲击性能的优异性,对照模型A选择Voronoi泡沫铝夹芯拱[35]模型,对照模型B选择等质量的实体拱模型,如图10所示。模型A芯层泡沫铝的厚度为0.6 mm,泡沫铝体积为芯层总体积的50%,芯层与上、下面板之间定义为Tie接触;为了研究对比芯层的吸能占比情况,在模型B的实体拱中也分别对应设计了上、下面板,面板与实体芯层之间也采用Tie接触的方式连接。2种对照模型均选用与前述优化模型相同的材料属性,均不考虑材料的破坏与失效。
图10所示的2种对照模型,拱半径R=185 mm,跨度L=120 mm,上、下面板厚度d=1 mm,子弹宽度l0=5 mm,初速度v0=100 m/s。模型A泡沫铝芯层厚度dc=20 mm;模型B实体芯层厚度dc=10 mm。2种对照模型均采用四边形单元,单元边长为1 mm。
2个对照模型上、下面板速度时程曲线如图11所示,模型A在t=teq=0.17 ms时,上、下面板中心处的速度达到一致,面板与芯层开始以相同的速度向下运动,当t=0.58 ms时,上、下面板的速度降低为0 m/s,上、下面板出现轻微的弹性回弹;而对于实体拱模型B,当t=teq=0.04 ms时,上、下面板中心处的速度便达到一致,芯层几乎无压缩,在t=0.60 ms时上、下面板速度降低为0 m/s,上、下面板开始轻微地弹性回弹。2个对照模型的响应完全符合图7(a)的芯层特征。
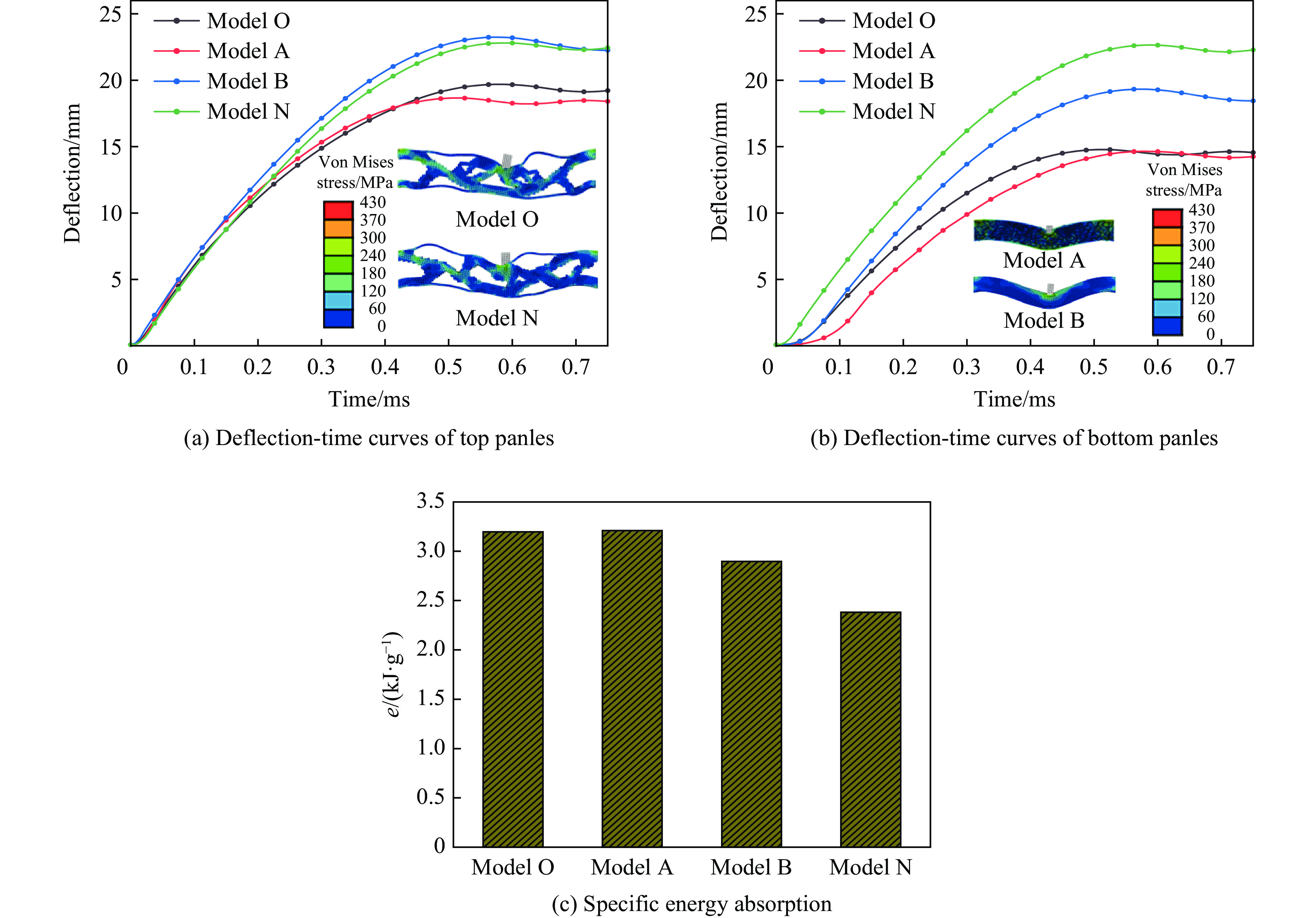
由于人体或者被保护的主体结构一般位于夹芯结构下(后)方,夹芯结构作为防护附层结构,一般可通过考察夹芯结构下面板的挠度和芯层的吸能来判断夹芯结构抗冲击性能优劣[36-39],图12所示为最终优化结果与2种对照模型在相同冲击载荷下上面板中心处的挠度随时间的变化曲线,从图中可以看出,经过优化后的结构上面板挠度最小,2种对照模型上面板挠度几乎相同,上面板挠度达到峰值以后,会发生小幅的弹性回弹,从图11变形模式可以看出,对照模型B整体变形较大,而对照模型A由于子弹压入芯层,造成上面板中心处位移较大。图13为3种结构下面板挠度-时程曲线。由图13(a)可知,经过优化后的结构下面板挠度最小,而对照模型B下面板位移最大。图13(b)为0~20 μs内,下面板中心处的挠度-时间曲线,由图可知,模型B由于实体芯层,芯层无压缩,上、下面板中心处几乎同时开始产生位移,模型A下面板中心处开始产生位移的时间为t=11 μs,而经过优化后的结构下面板开始发生变形的时间最晚,为t=15 μs。芯层压缩变形时间的增加,更有利于减小后面板受到的冲击作用,提高结构的能量吸收能力。
由前面的分析可知,当初始冲击速度为100 m/s时,经过优化后的夹芯拱具有较好的抗冲击性能,但在实际工程中的冲击载荷可能是多样的,针对单一工况的优化是否能满足不同工况的需求,必须经过进一步的验证。为此,图14对比了3种模型在初速度v0=20,50,80,100 m/s的子弹冲击下的下面板的挠度和芯层的能量吸收。图14(a)为3种模型下面板中心处的挠度对比,从图中可以看出:经过优化后的结构在不同初速度的子弹冲击下,下面板挠度均为最小。14(b)为在不同初速度的子弹冲击下,芯层的比吸能(e=Ec/mc,Ec为芯层塑性耗散能,mc为芯层质量)情况,可以看到经过优化后的结构芯层比吸能均高于模型A和模型B的芯层比吸能。由于子弹刚性较大、冲击速度较高,可以忽略弹性段能量,芯层能量耗散直接采用其塑性耗散能量。
由图14(c)可知:不同初速度的子弹冲击下,经过优化后的结构芯层吸能占比(芯层塑性能量耗散与总塑性能量耗散之比,即Ec/Ew)分别为84.6%、85.3%、84.5%和83.9%,模型A的芯层吸能占比分别为74.9%、74.6%、73.6%和74.9%,模型B的芯层吸能占比分别为66.6%、67.9%、69.8%和70.1%。对比以上各组数据可以发现:经过优化后的结构抗冲击性能最优,而对照模型A(Voronoi泡沫铝夹芯拱模型)抗冲击性能次之,对照模型B(等质量实体拱模型)抗冲击性能最差。在相同的体积约束和载荷条件下,删除低效的单元而添加或保留高效的单元,有效地提高了材料的利用率,使得芯层每个部位都充分发挥作用;而对照组的结构中材料均匀分布,必然会导致某些部分的材料不能被充分利用,未起到抵抗变形的作用,材料合理分配使结构具有较好的抗冲击性能。在低速冲击下,几种结构的变形较小,结构的响应均未超过变形阈值,芯层结构受拉压弯扭转作用较小,一些部位未能充分发挥作用,所以优化后的结构抗冲性能优势并不明显;高速冲击下,优化后的结构能够充分发挥材料的性能,在本文研究的冲击载荷范围内,冲击速度越高,这种优势越明显。
较低的峰值冲击力和较高的平台力,代表了结构具有较好的吸能缓冲特性,也是评价结构抗冲击性能的一类指标。图14(d)为3种模型在初速度v0=100 m/s的子弹冲击下的力-位移曲线,从图中可以看出:当结构受到冲击时,在较短的行程内,冲击接触力迅速升高至峰值,随后下降至平台阶段,当子弹反弹后冲击接触力降为零。与随机泡沫铝夹芯拱(模型 A)相比,经过优化后,结构的冲击接触力峰值和平台阶段都较接近;与实体拱(模型 B)相比,经过优化后,结构的冲击接触力峰值下降约17.96%,同时平台阶段冲击接触力水平较高。
3.4 非对称载荷下优化结构响应分析
图15为在初速度v0=100 m/s的非对称载荷下的夹芯拱模型,子弹的偏移量w=5 mm(与对称载荷相比,冲击点位置偏差
\delta = w{\text{/}}{l_0} = 1 ),其他模型尺寸与图4模型相同。图16所示为在非对称载荷下夹芯拱模型的优化结果,与图5所示的优化结果相比,最大的区别在于结构的不对称,由于载荷作用部位偏向右侧,芯层左侧所受的拉压弯扭作用较小,低效单元较多,必然导致在芯层右侧单元分布较为密集;中心孔洞向左侧偏移,但结构仍然保持前者的一些基本特征,即过渡区域主要呈现C形构型,左侧边界处也相应增加了杆件,以提高结构承载能力。
将非对称载荷下的优化模型称为模型N,将原对称载荷下的优化模型称为模型O,分析对比2种优化结构与2种等质量对照模型(模型A、模型B)在相同工况下的动态响应。图17为在初速度v0=100 m/s的子弹无偏移的冲击载荷下,4种模型的动态响应对比。图17(a)为4种模型上面板跨中处挠度时间曲线,从图中可以看出非对称载荷下的优化模型N在子弹无偏差冲击下,上面板挠度大于优化模型O而小于2种对照模型,且有小幅弹性回弹;图17(b)所示为下面板跨中处挠度随时间变化曲线,可以看出2种优化结构下面板挠度几乎相同,模型O下面板最终挠度为14.19 mm,模型N下面板最终挠度为14.28 mm,分析可知:在子弹冲击点位置偏差δ=1时,在相同的对称冲击载荷下,优化模型N的下面板挠度比模型O下面板挠度仅增大0.6%;图17(c)所示4种模型芯层比吸能对比,分析数据可知:在子弹无偏移冲击时,模型N的比吸能仅比模型O减小0.7%。
图18(a)与(b)所示为在子弹初速度v0=100 m/s,偏移量w=5 mm时,4种模型上、下面板跨中处的挠度随时间变化曲线以及最终的响应结果,从图中可以看出:随着时间的变化,2个模型上、下面板挠度不断增大,挠度达到峰值后都有轻微的弹性回弹,且模型O的上、下面板跨中处挠度比模型N面板跨中处挠度仅分别增大了4.4%和2.2%。图18(c)所示为4种模型在子弹初速度v0=100 m/s,偏移量w=5 mm时芯层的比吸能情况对比,由图中数据分析可知:优化模型N的芯层吸能占比略优于优化模型O,且优于2种对照模型。由以上各组数据可得:在子弹偏移量w=0 mm时,优化模型O的抗冲击性能、芯层吸能等略优于优化模型N,且2种优化模型均优于对照模型;在子弹偏移量w=5 mm时,优化模型N的以上性能略优于优化模型O,且均优于对照模型。从而表明:不同载荷工况下,最终的优化结果虽然稍有不同,每种工况下得到的优化结果在相应工况下所展现的力学性能也优于其他优化结构,并且对称优化结果在非对称载荷作用下,力学性能也优于等质量对照模型。
以上分析充分说明,虽然结构在非对称冲击下,优化结果有差异,但在相同冲击载荷下,结构的响应特性与抗冲击性能相差较小,通过对称载荷对结构进行优化,具有一定的普适性,其结果在工程中具有较高的应用价值。
4. 结 论
将等效静态载荷优化思想引入双向渐进结构拓扑优化方法中,基于双向渐进结构优化方法,改进了动态载荷拓扑优化流程,针对未知外部动载荷,在优化过程中对载荷进行了变换处理,增加了MATLAB与ABAQUS的信息交互过程,充分利用了商业有限元软件强大的计算和前后处理能力,提高了计算精度,对几何结构较为复杂的模型有更好的适用性。以夹芯拱芯层刚度最大化为目标,获得了冲击载荷下夹芯拱的最优拓扑构型,并与相同质量的Voronoi泡沫铝夹芯拱、实体拱的动态响应进行了对比。
经过优化后的夹芯拱芯层可分为3个对称的部分:跨中类三角点阵桁架结构区域、边界C形结构区域以及跨中和边界之间的Y形结构过渡区域。与等质量的Voronoi泡沫铝夹芯拱、实体拱相比,在相同的冲击速度下,经过优化后的结构挠度最小,芯层比吸能最高;当冲击速度较低时,结构的响应均未超过变形阈值,芯层结构受拉压弯扭转作用较小,一些部位未能充分发挥作,经过优化后的结构的抗冲击性能优势并不明显;在所研究的冲击速度范围内,冲击速度越高,经优化后的结构的抗冲击性能越好。
对初速度v0=100m/s、子弹偏移量δ=1子弹冲击下的夹芯拱结构进行优化设计,并对比了对称冲击与非对称冲击下2种优化模型的动态响应。当结构受到对称冲击载荷时,在对称冲击载荷下获得优化结构的抗冲击性能、芯层吸能等略优于在非对称载荷下获得的优化结构。当结构受到非对称冲击载荷时,在非对称载荷下获得优化结构的性能略优于在对称冲击载荷下获得优化结构的。但在2种冲击载荷下,经过优化后所得结构的性能均优于对照模型。从而表明:不同载荷工况下,最终的优化结果虽有所不同,每种工况下得到的优化结果在相应工况下所展现的力学性能也优于其他结构,但在相同冲击载荷下,2种优化所得结构的响应特性和抗冲击性能相差较小;通过对称载荷对结构进行优化,具有一定的普适性。
-
图 7 不同类型芯层夹芯结构上、下面板速度时程曲线
Figure 7. Velocity versus time histories of the mid-span of the top and bottom panels for two types of sandwich response[34]
-
[1] RIZOV V, SHIPSHA A, ZENKERT D. Indentation study of foam core sandwich composite panels [J]. Composite Structures, 2005, 69(1): 95–102. DOI: 10.1016/j.compstruct.2004.05.013. [2] HOU W H, ZHU F, LU G X, et al. Ballistic impact experiments of metallic sandwich panels with aluminium foam core [J]. International Journal of Impact Engineering, 2010, 37(10): 1045–1055. DOI: 10.1016/j.ijimpeng.2010.03.006. [3] ZHU F, WANG Z H, LU G X, et al. Some theoretical considerations on the dynamic response of sandwich structures under impulsive loading [J]. International Journal of Impact Engineering, 2010, 37(6): 625–637. DOI: 10.1016/j.ijimpeng.2009.11.003. [4] CHANG W S, VENTSEL E, KRAUTHAMMER T, et al. Bending behavior of corrugated-core sandwich plates [J]. Composite Structures, 2005, 70(1): 81–89. DOI: 10.1016/j.compstruct.2004.08.014. [5] SHU C F, ZHAO S Y, HOU S J. Crashworthiness analysis of two-layered corrugated sandwich panels under crushing loading [J]. Thin-Walled Structures, 2018, 133: 42–51. DOI: 10.1016/j.tws.2018.09.008. [6] ZHU F, ZHAO L M, LU G X, et al. A numerical simulation of the blast impact of square metallic sandwich panels [J]. International Journal of Impact Engineering, 2009, 36(5): 687–699. DOI: 10.1016/j.ijimpeng.2008.12.004. [7] 余同希, 朱凌, 许骏. 结构冲击动力学进展(2010—2020) [J]. 爆炸与冲击, 2021, 41(12): 121401. DOI: 10.11883/bzycj-2021-0113.YU T X, ZHU L, XU J. Progress in structural impact dynamics during 2010—2020 [J]. Explosion and Shock Waves, 2021, 41(12): 121401. DOI: 10.11883/bzycj-2021-0113. [8] 王海任, 李世强, 刘志芳, 等. 爆炸载荷下双向梯度仿生夹芯圆板的力学行为 [J]. 爆炸与冲击, 2021, 41(4): 043201. DOI: 10.11883/bzycj-2020-0132.WANG H R, LI S Q, LIU Z F, et al. Mechanical behaviors of bi-directional gradient bio-inspired circular sandwich plates under blast loading [J]. Explosion and Shock Waves, 2021, 41(4): 043201. DOI: 10.11883/bzycj-2020-0132. [9] 彭克锋, 崔世堂, 潘昊, 等. 冲击载荷作用下柱壳链中的弹性波传播简化模型及其解析解 [J]. 爆炸与冲击, 2021, 41(1): 011403. DOI: 10.11883/bzycj-2020-0246.PENG K F, CUI S T, PAN H, et al. Simplified model of elastic wave propagation in cylindrical shell chain under impact load and its analytical solution [J]. Explosion and Shock Waves, 2021, 41(1): 011403. DOI: 10.11883/bzycj-2020-0246. [10] 曾祥, 刘彦, 许泽建, 等. 爆炸载荷作用下玻璃钢/硬质聚氨酯泡沫夹层结构抗冲击性能实验研究 [J]. 北京理工大学学报, 2021, 41(11): 1145–1153. DOI: 10.15918/j.tbit1001-0645.2021.036.ZENG X, LIU Y, XU Z J, et al. Experimental study on impact resistance of glass fiber reinforced plastic/rigid polyurethane foam sandwich structures under air blast loading [J]. Transactions of Beijing Institute of Technology, 2021, 41(11): 1145–1153. DOI: 10.15918/j.tbit1001-0645.2021.036. [11] 邢运, 杨嘉陵. 动物进化的抗冲击策略及其仿生机理研究 [J]. 力学进展, 2021, 51(2): 295–341. DOI: 10.6052/1000-0992-20-027.XING Y, YANG J L. Research progress of impact-resistance strategies and biomi-metic mechanism in animal evolution [J]. Advances in Mechanics, 2021, 51(2): 295–341. DOI: 10.6052/1000-0992-20-027. [12] 李肖成, 徐绯, 杨磊峰, 等. 薄板在冲击载荷下线弹性理想塑性响应的相似性研究 [J]. 爆炸与冲击, 2021, 41(11): 113103. DOI: 10.11883/bzycj-2020-0374.LI X C, XU F, YANG L F, et al. Study on the similarity of elasticity and ideal plasticity response of thin plate under impact loading [J]. Explosion and Shock Waves, 2021, 41(11): 113103. DOI: 10.11883/bzycj-2020-0374. [13] NIKBAKT S, KAMARIAN S, SHAKERI M. A review on optimization of composite structures Part I: laminated composites [J]. Composite Structures, 2018, 195: 158–185. DOI: 10.1016/j.compstruct.2018.03.063. [14] NIKBAKHT S, KAMARIAN S, SHAKERI M. A review on optimization of composite structures Part II: functionally graded materials [J]. Composite Structures, 2019, 214: 83–102. DOI: 10.1016/j.compstruct.2019.01.105. [15] ROZVANY G I N, BENDSOE M P, KIRSCH U. Layout optimization of structures [J]. Applied Mechanics Reviews, 1995, 48(2): 41–119. DOI: 10.1115/1.3005097. [16] RIETZ A. Sufficiency of a finite exponent in SIMP (power law) methods [J]. Structural and Multidisciplinary Optimization, 2001, 21(2): 159–163. DOI: 10.1007/s001580050180. [17] BENDSØE M P, KIKUCHI N. Generating optimal topologies in structural design using a homogenization method [J]. Computer Methods in Applied Mechanics and Engineering, 1988, 71(2): 197–224. DOI: 10.1016/0045-7825(88)90086-2. [18] SUZUKI K, KIKUCHI N. A homogenization method for shape and topology optimization [J]. Computer Methods in Applied Mechanics and Engineering, 1991, 93(3): 291–318. DOI: 10.1016/0045-7825(91)90245-2. [19] XIE Y M, STEVEN G P. A simple evolutionary procedure for structural optimization [J]. Computers & Structures, 1993, 49(5): 885–896. DOI: 10.1016/0045-7949(93)90035-C. [20] QUERIN O M, STEVEN G P, XIE Y M. Evolutionary structural optimisation (ESO) using a bidirectional algorithm [J]. Engineering Computations, 1998, 15(8): 1031–1048. DOI: 10.1108/02644409810244129. [21] YOUNG V, QUERIN O M, STEVEN G P, et al. 3D and multiple load case bi-directional evolutionary structural optimization (BESO) [J]. Structural Optimization, 1999, 18(2): 183–192. DOI: 10.1007/BF01195993. [22] HUANG X, XIE Y M. Bi-directional evolutionary topology optimization of continuum structures with one or multiple materials [J]. Computational Mechanics, 2009, 43(3): 393–401. DOI: 10.1007/s00466-008-0312-0. [23] HUANG X, XIE Y M. Evolutionary topology optimization of continuum structures with an additional displacement constraint [J]. Structural and Multidisciplinary Optimization, 2010, 40(1): 409–416. DOI: 10.1007/s00158-009-0382-4. [24] CHOI W S, PARK G J. Structural optimization using equivalent static loads at all time intervals [J]. Computer Methods in Applied Mechanics and Engineering, 2002, 191(19/20): 2105–2122. DOI: 10.1016/S0045-7825(01)00373-5. [25] PARK G J, KANG B S. Validation of a structural optimization algorithm transforming dynamic loads into equivalent static loads [J]. Journal of Optimization Theory and Applications, 2003, 118(1): 191–200. DOI: 10.1023/A:1024799727258. [26] PARK K J, LEE J N, PARK G J. Structural shape optimization using equivalent static loads transformed from dynamic loads [J]. International Journal for Numerical Methods in Engineering, 2005, 63(4): 589–602. DOI: 10.1002/nme.1295. [27] 蓝萌. 双向渐进结构优化法原理及其动力学优化研究 [J]. 机电技术, 2017(1): 17–22. DOI: 10.19508/j.cnki.1672-4801.2017.01.006.LAN M. Research of bi-directional evolutionary structural optimization method and dynamic response [J]. Mechanical & Electrical Technology, 2017(1): 17–22. DOI: 10.19508/j.cnki.1672-4801.2017.01.006. [28] 王宪杰. 基于改进BESO算法的多尺度多相材料并行优化设计 [D]. 西安: 西北工业大学, 2015. DOI: 10.7666/d.D689590.WANG X J. Multi-scale concurrent optimization of compositestructure and its periodic multiphase composite materialbased on improved BESO algorithm [D]. Xi'an, Shaanxi, China: Northwestern Polytechnical University, 2015. DOI: 10.7666/d.D689590. [29] 阎琨. 冲击荷载下结构优化设计研究 [D]. 大连: 大连理工大学, 2016.YAN K. Structural optimization method of structure subject to impact loads [D]. Dalian, Liaoning, China: Dalian University of Technology. [30] YAN K, CHENG G D, WANG B P. Adjoint methods of sensitivity analysis for Lyapunov equation [J]. Structural and Multidisciplinary Optimization, 2016, 53(2): 225–237. DOI: 10.1007/s00158-015-1323-z. [31] HUANG X, XIE Y M. Evolutionary topology optimization of continuum structures: methods and applications [M]. Chichester, UK: Wiley, 2010: 17–50. [32] ZHANG Y, JIN T, LI S Q, et al. Sample size effect on the mechanical behavior of aluminum foam [J]. International Journal of Mechanical Sciences, 2019, 151: 622–638. DOI: 10.1016/j.ijmecsci.2018.12.019. [33] TILBROOK M T, DESHPANDE V S, FLECK N A. The impulsive response of sandwich beams: Analytical and numerical investigation of regimes of behaviour [J]. Journal of the Mechanics and Physics of Solids, 2006, 54(11): 2242–2280. DOI: 10.1016/j.jmps.2006.07.001. [34] FLECK N A, DESHPANDE V S. The resistance of clamped sandwich beams to shock loading [J]. Journal of Applied Mechanics, 2004, 71(3): 386–401. DOI: 10.1115/1.1629109. [35] XIE Q H, JING L, WANG Z H, et al. Deformation and failure of clamped shallow sandwich arches with foam core subjected to projectile impact [J]. Composites Part B:Engineering, 2013, 44(1): 330–338. DOI: 10.1016/j.compositesb.2012.04.070. [36] 张鹏飞, 刘志芳, 李世强. 内爆炸载荷下梯度泡沫铝夹芯管的动态响应 [J]. 爆炸与冲击, 2020, 40(7): 071402. DOI: 10.11883/bzycj-2019-0418.ZHANG P F, LIU Z F, LI S Q. Dynamic response of sandwich tubes with graded foam aluminum cores under internal blast loading [J]. Explosion and Shock Waves, 2020, 40(7): 071402. DOI: 10.11883/bzycj-2019-0418. [37] 苏兴亚, 敬霖, 赵隆茂. 爆炸载荷下分层梯度泡沫铝夹芯板的失效模式与抗冲击性能 [J]. 爆炸与冲击, 2019, 39(6): 063103. DOI: 10.11883/bzycj-2018-0198.SU X Y, JING L, ZHAO L M. Failure modes and shock resistance of sandwich panels with layered-gradient aluminum foam cores under air-blast loading [J]. Explosion and Shock Waves, 2019, 39(6): 063103. DOI: 10.11883/bzycj-2018-0198. [38] 叶楠, 张伟, 黄威, 等. PVC夹芯板在冲击载荷下的动态响应与失效模式 [J]. 爆炸与冲击, 2017, 37(1): 37–45. DOI: 10.11883/1001-1455(2017)01-0037-09.YE N, ZHANG W, HUANG W, et al. Dynamic response and failure mode of PVC sandwich plates subjected to impact loading [J]. Explosion and Shock Waves, 2017, 37(1): 37–45. DOI: 10.11883/1001-1455(2017)01-0037-09. [39] 邹广平, 孙杭其, 唱忠良, 等. 聚氨酯/钢夹芯结构爆炸载荷下动力学响应的数值模拟 [J]. 爆炸与冲击, 2015, 35(6): 907–912. DOI: 10.11883/1001-1455(2015)06-0907-06.ZOU G P, SUN H Q, CHANG Z L, et al. Numerical simulation on dynamic response of polyurethane/steel sandwich structure under blast loading [J]. Explosion and Shock Waves, 2015, 35(6): 907–912. DOI: 10.11883/1001-1455(2015)06-0907-06. 期刊类型引用(3)
1. 武凯洁,田昊东,程灵钰,张宇,李臣政,陆秋阳,赵凯. 飞行器回转支撑机构的优化设计及增材制造. 上海航天(中英文). 2025(01): 134-140 .  百度学术
百度学术2. 肖珍,吴晓. 夹芯梁在重物低速冲击下的动力计算分析. 工程与试验. 2023(03): 1-3+58 .  百度学术
百度学术3. 智友海,史向平. 超弹性NiTi合金夹芯体的冲击性能分析. 兵器材料科学与工程. 2023(06): 99-106 .  百度学术
百度学术其他类型引用(1)
-








 下载:
下载:

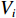
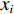








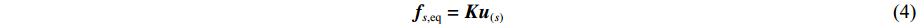























 下载:
下载:

















 百度学术
百度学术