Synchronous and decoupling calibration of tri-axial impact force transducers based on a Hopkinson bar and an artificial neural network
-
摘要: 三分量冲击力载荷的同步激励与输入输出间的精准建模是三轴冲击力传感器标定所面临的主要挑战。为了实现对三轴冲击力传感器的有效标定,使其能够准确测量空间中的三维冲击力载荷。首先,基于Hopkinson杆与矢量分解原理建立了一种高幅值(104 N量级)、窄脉宽(10−4 s量级)可计量三分量冲击力载荷的同步激励方法,实现了对三轴冲击力传感器的同步加载。然后,基于最小二乘原理与矩阵微分构建了三轴冲击力传感器的线性标定模型,并通过改变子弹结构与冲击气压揭示了线性解耦标定模型中传感器主灵敏度系数与轴间耦合灵敏度系数并非固定常数而均与冲击力载荷脉冲构型(幅值、脉宽)相关的冲击特性。最后,将能够反映载荷构型信息的传感器各轴输出电压脉冲的幅值与脉宽作为影响因素,并以神经元的形式添加到人工神经网络(artificial neural network, ANN)的输入层,建立了基于ANN的三轴冲击力传感器输出电压与输入载荷间的代理模型,实现了数据驱动的三轴冲击力传感器非线性解耦标定。结果表明,相对最小二乘模型,ANN标定精度更高,采用ANN进行三轴冲击力传感器标定具有可行性和有效性。
-
关键词:
- 冲击力传感器 /
- 同步标定 /
- 神经网络 /
- 机器学习 /
- Hopkinson杆
Abstract: The impact force transducers are widely used in aerospace, national defense engineering, auto industry and other important fields involving national security and people livelihood. Those transducers usually need to be calibrated before being put into practical use. Realizing synchronous loading and developing an accurate mathematical model to describe the input-output relationship are the major challenges in the calibration of triaxial impact force transducers at this stage. In this paper, a method for the synchronous excitation of three-dimensional impact force loads was established based on a modified Hopkinson bar technique and the principle of vector decomposition. The triaxial impact force transducer being calibrated was mounted at an angle to the axis of the Hopkinson bar, the one-dimensional force excited in the Hopkinson bar was then decomposed onto each sensitive axis of the transducer, thus realizing its synchronous loading. The coupling effect between the sensitive axes of the transducer was assumed to be linear. A linear decoupling calibration model of the triaxial impact force transducer was built based on a sensitivity matrix containing three main sensitivity coefficients and six transverse sensitivity coefficients. The sensitivity matrix was solved using the least squares method. The amplitude and pulse width of the impact force pulses excited in the Hopkinson bar were adjusted by varying the structure and the impact velocity of the bullet. Reference impact force pulses with varied amplitudes and pulse widths were then used to calibrate the triaxial impact force transducer. Characteristics were revealed that both the main sensitivity coefficients and the transverse sensitivity coefficients of the transducer are related to the amplitude and the pulse width of the reference impact force. The amplitude and pulse width information of the input force pulses that the transducer was subjected to can be reflected by the output voltage pulses of the transducer. Therefore, the amplitude and pulse width of the output voltages of the sensitive axes of the transducer were taken as influencing factors and added to the input layer of the artificial neural network (ANN) in form of artificial neurons. A nonlinear decoupling calibration model for the tri-axial impact force transducer was then built based on an ANN model. The calibration results show that the ANN model has higher calibration accuracy compared to the least squares model. It is feasible and valid to use ANNs to calibrate the tri-axial impact force transducers.-
Key words:
- impact force transducers /
- synchronous calibration /
- neural network /
- machine learning /
- Hopkinson bar
-
随着航空航天等科学技术的发展,各型装备在其服役过程中面临的外部载荷环境越来越严酷[1],冲击载荷已成为威胁系统结构安全与运行稳定的重要因素。获悉真实工况或模拟真实工况下的冲击载荷,对承载结构的可靠性设计与强度校核等均有重要意义。实际工程中,通常采用冲击力传感器对冲击载荷进行测量[2-3]。而保证测量结果准确可靠的基本前提之一,是对冲击力传感器进行有效标定。静标动用,即将传感器静态标定的结果用于动态/冲击测量,会造成较大的测量误差,不可行[4]。
目前,发展相对成熟、应用相对广泛的冲击/动态力传感器标定方法按其标定装置激励的载荷类型主要可以分为3类,即振动载荷标定[5-6]、冲击载荷标定[7-8]以及阶跃载荷标定[9-10]。然而,上述标定方法难以适用于三轴冲击力传感器的标定,主要体现在以下2点:首先,载荷激励范围有限,上述标定装置所能激励的载荷幅值通常小于104 N量级,载荷脉宽通常大于毫秒量级;而先进冲击力传感器的测量范围可达104~105 N,频响可达104 Hz以上。其次,上述标定装置无法实现三分量冲击力载荷同步激励,只能对三轴力传感器进行单轴依次标定。另外,复杂的非线性轴间耦合使得建立基于物理的、准确的多轴力/力矩传感器标定模型现实可行性较低[11-13],而采用基于数据驱动的机器学习方法建立的传感器输出与输入间的非线性映射模型被证明是一种切实有效的代理模型[14-19]。但现有机器学习模型在对力传感器进行标定时,通常认为传感器的灵敏度与载荷等外部因素无关,而仅在模型输入侧设置代表传感器输出的变量。因此,当力传感器的灵敏度随载荷等因素发生变化时,目前所采用的机器学习建模方法将无法实现准确的标定。
本文中,基于Hopkinson杆和矢量分解原理,建立一种三分量冲击力载荷同步激励方法,并基于该方法实现对三轴冲击力传感器的同步加载;根据同步加载方式下传感器的输入与输出,揭示三轴冲击力传感器主灵敏度系数和轴间耦合灵敏度系数均与冲击力载荷脉冲构型相关的冲击特性;将能够反映冲击载荷构型信息的三轴冲击力传感器各轴输出电压脉冲的幅值和脉宽作为影响因素,以神经元的形式添加到人工神经网络(artificial neural network, ANN)的输入层,建立基于ANN的三轴冲击力传感器非线性解耦标定模型,并与基于最小二乘的线性解耦标定模型进行对比。
1. 标定原理
1.1 三轴冲击力传感器同步加载
基于Hopkinson杆与矢量分解原理的三轴冲击力传感器同步加载原理如图1所示。子弹自左向右撞击位于标定杆(Hopkinson杆)前端起到保护和整形作用的垫片,在标定杆中激励起右行的加载波。波形可由粘贴在杆中点附近的应变片进行测量。三轴冲击力传感器一端固支于对其施加位移约束的砧座上,一端与承载工装刚性连接。承载工装斜端面与标定杆平端面光滑接触,并使得传感器轴线与标定杆轴线呈
θ 角度,如图1(a)所示。根据一维弹性应力波理论,由标定杆施加给承载工装斜端面的冲击力载荷为[20]:F(t)=AbarE[εi(t)+εr(t)] (1) 式中:
εi(t) 和εr(t) 分别为沿标定杆传播的入射波和反射波,Abar 和E 分别为标定杆的横截面积和弹性模量,F(t) 即为通过标定杆施加给传感器承载工装斜端面的冲击力载荷。由于标定杆轴线与传感器轴线之间呈
θ 角度,则根据矢量分解原理,标定杆施加给传感器的冲击载荷F(t) 可分解为沿传感器法向的分量Fn(t) 和沿传感器切向的分量Ft(t) 。设三轴冲击力传感器的3个敏感轴分别为x 、y 、z 轴,则当Ft(t) 的方向与传感器切向某轴(图1(b)中为x 轴)的方向重合时,即可实现三轴冲击力传感器的双轴同步加载,如图1(b)所示。而当Ft(t) 与传感器切向两轴均匀呈一定角度时,则Ft(t) 又可进一步被分解到传感器切向的两轴上,从而实现冲击力传感器的三轴同步加载,如图1(c)所示。图1(b)~(c)中x 、y 、z 分别为传感器的3个轴;σ(t) 为标定杆施加给传感器的应力脉冲,σ(t)=σi(t)+σr(t) ;σi(t) 和σr(t) 分别为标定杆上的入射应力波和反射应力波,σi(t)=Eεi(t) ,σr(t)=Eεr(t) ;σx(t) 、σy(t) 和σz(t) 分别为σ(t) 按矢量分解原理分解到传感器x 、y 和z轴方向的分量;β 为传感器绕其轴线相对承载工装旋转的角度。基于上述分析,标定杆施加给三轴冲击力传感器x 轴的载荷Fx(t) 、y 轴的载荷Fy(t) 以及z轴的载荷Fz(t) 分别为:Fx(t)=Abarσ(t)sinθsinβ,Fy(t)=Abarσ(t)sinθcosβ,Fz(t)=Abarσ(t)cosθ (2) 1.2 三轴冲击力传感器标定模型
1.2.1 基于最小二乘的线性解耦标定模型
基于最小二乘拟合的线性模型由于数学形式简单、求解高效成为各类力传感广泛采用的标定模型[21-23]。考虑轴间耦合的三轴冲击力传感器线性解耦标定模型可描述为:
[UxUyUz]=[SxxSxySxzSyxSyySyzSzxSzySzz][FxFyFz]+[εxεyεz] (3) 简记为:
U=SF+ε (4) 式中:
U 为三轴冲击力传感器输出电压矩阵;F 为三轴冲击力传感器输入载荷矩阵;ε 为三轴冲击力传感器真实输出与其线性拟合值之间的残差矩阵;S=[Sij]3×3 (i,j=x,y,z )为灵敏度系数矩阵,当i=j 时,Sii 表示i 轴的主灵敏度系数,当i≠j 时,Sij 表示j 轴对i 轴的耦合灵敏度系数。设三轴冲击力传感器的线性拟合输出为
ˆU=SF ,则基于最小二乘原理的灵敏度系数矩阵S∗ 满足:S∗=argminS‖ε‖22=argminStr[(U−ˆU)(U−ˆU)T]=argminStr[(U−SF)(U−SF)T] (5) 对式(5)可采用矩阵微分进行求解,令:
d{tr[(U−SF)(U−SF)T]}dS=2(U−SF)FT=0 (6) 解得:
S∗=UFT(FFT)−1 (7) 1.2.2 基于ANN的非线性解耦标定模型
基于ANN的三轴冲击力传感器非线性解耦标定模型原理如图2所示,模型从结构上分为3层:输入层、隐含层和输出层。输入层用于接收三轴冲击力传感器的电压输出值
Ux 、Uy 、Uz 以及可能影响模型预测输出的影响因素。输出层用于输出与Ux 、Uy 、Uz 对应的三轴冲击力传感器载荷Fx 、Fy 、Fz 。隐含层则用于拟合或学习蕴含在输入与输出数据间的非线性关系。ANN的学习或训练机制可概括为信息正向传递、误差反向传播,其中,信息传递机制为:O(n)j=J(p∑i=1ωijO(n−1)i)j=1,2,⋯,q (8) 式中:
O(n)j 为n层第j 个神经元的输出,O(n−1)i 为(n−1)层(前一层)第i 个神经元的输出,p 、q 分别为(n−1)层和n层中包含的神经元个数;ωij 为(n−1)层第i 个神经元与n层第j 个神经元之间的连接权重;J(x) 为神经元输出的激活函数。采用基于梯度下降的误差逆传播(back propagation, BP)算法对ANN模型进行训练,算法流程如图3所示。训练集中示例
Um=[Uxm,Uym,Uzm]T 为某时刻三轴冲击力传感器的输出,标记Fm=[Fxm,Fym,Fzm]T 为相应时刻三轴冲击力传感器的输入,Nt 为训练集中样本数量。权重初始化按正态分布ωij~N(0,1) 进行。模型损失函数采用均方误差函数,激活函数采用Sigmoid函数:J(x)=11+e−x (9) 训练集中示例和标记按下式进行线性归一化:
Vnorm=V−VminVmax−Vmin (10) 基于Sigmoid激活函数与均方误差损失函数的权重修正因子为:
Δω(n−1,n)ij=αE(n)jO(n)j(1−O(n)j)O(n−1)i (11) 式中:
α 为学习率,Δω(n−1,n)ij 为(n−1)层第i 个神经元与n层第j 个神经元间连接权重的更新因子,E(n)j 为n层第j 个神经元的预测误差。E(n)j 由(n+1)层神经元的预测误差按连接权重逆传播得到:E(n)j=r∑k=1ωjkE(n+1)k (12) 式中:
r 为(n+1)层神经元的个数,ωjk 为n层第j 个神经元与(n+1)层第k 个神经元间连接权重,E(n+1)k 为(n+1)层第k 个神经元的预测误差;误差逆传播的起点输出层神经元的误差为变量预测值与真实值之间的差值。2. 实 验
2.1 传感器
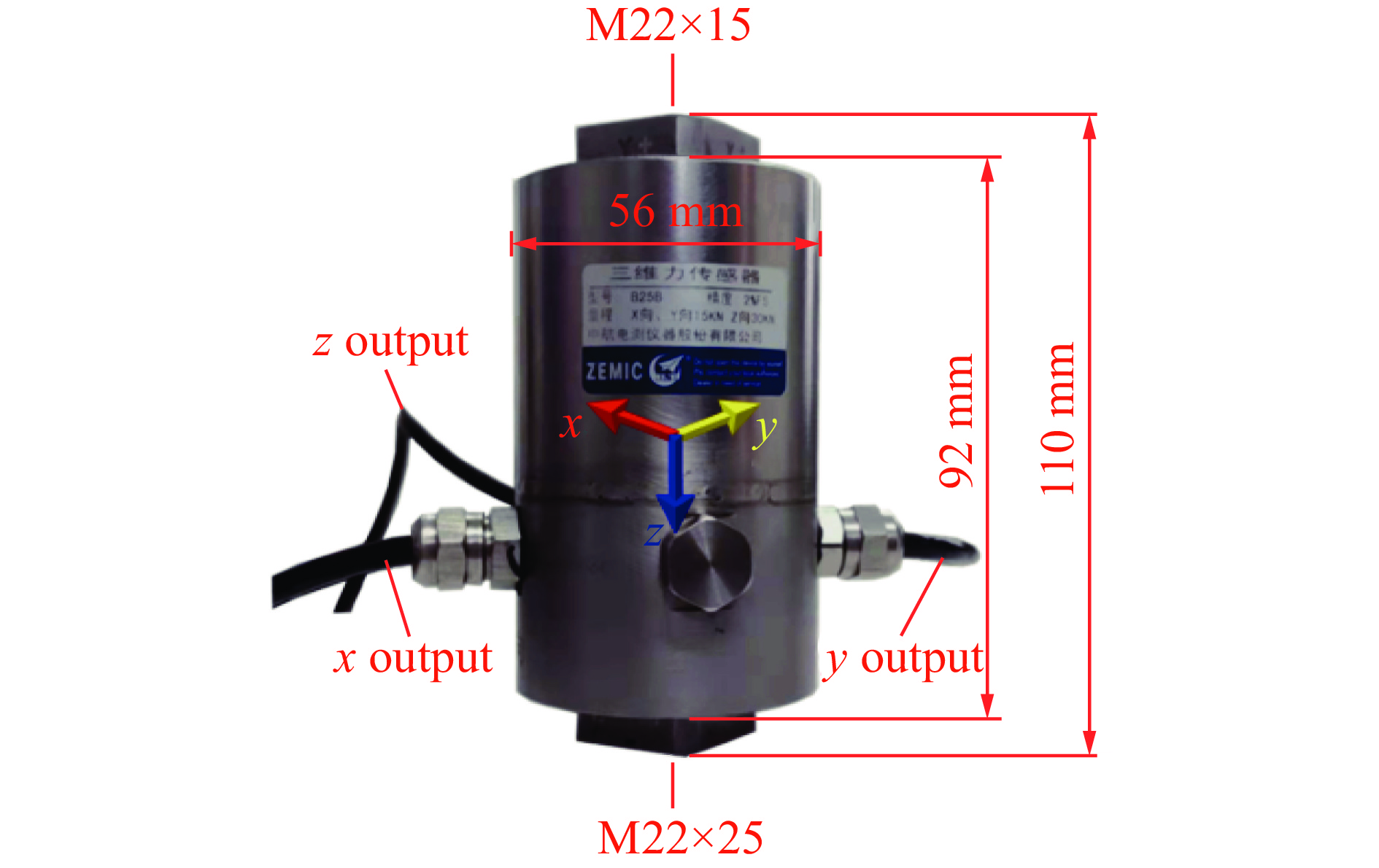
实验中所采用的三轴冲击力传感器为B25B型压阻式传感器,如图4所示。传感器整体结构呈圆柱状,轴线方向为传感器的z轴方向,x轴、y轴与z轴两两垂直,位于与轴线垂直的法平面内;传感器上端设置有公制M22×15内螺纹,用于连接承载工装;下端设置有公制M22×25内螺纹,用于传感器的安装和固定。B25B型三轴冲击力传感器的性能参数如表1所示。
表 1 B25B型三轴冲击力传感器的性能参数Table 1. Parameters of a B25B tri-axial impact force transducer测量轴 量程/
kN激励电压/
V一阶固有频率/
kHz灵敏度系数/
(mV·kN−1)x 15 10 12.275 0.480 y 15 10 12.275 0.480 z 30 10 12.273 0.223 2.2 标定装置
三轴冲击力传感器标定所采用的实验装置如图5所示,子弹由轻气炮驱动,标定杆为截面尺寸为20 mm×20 mm的AA7075铝合金方杆,杆长1 800 mm。由超动态应变仪提供三轴冲击力传感器各轴的激励电压,并由其对传感器及应变片的输出信号进行放大。应变片信号通道激励电压和增益分别设置为2 V、100倍,传感器各轴信号通道的激励电压和增益则均分别设置为10 V、20倍。应变仪放大后的输出信号由高速数采卡采集,采样频率为10 MHz。标定所采用的子弹结构如图5(b)所示,材料为45钢。为激励不同脉宽的冲击力载荷,设置其他结构参数相同、结构参数
d 分别为20、30、40、60、80 mm的5种子弹,分别记为子弹1 ~ 5。整形器为直径15 mm、厚度5 mm的圆柱片,如图5(c)所示,材料为AA2024铝合金。2.3 实验流程
实验主要分4个步骤进行:
(1)
θ=0 °或θ=90 °,采用子弹1 ~ 5以不同速度对传感器各轴进行各自量程范围内的单独加载。(2)
θ=30 °,ω=30 °,采用子弹1以不同速度对传感器各轴进行相同脉宽、不同幅值的同步加载。(3)
θ=30 °,ω=30 °,采用子弹1~5以相同速度对传感器各轴进行相同幅值、不同脉宽的同步加载。(4)将角度
θ= 30°,45°,60°;ω= 30°,45°,60°;两两组合以增加传感器的受载工况,采用子弹1~5在保证各轴均处于量程内的前提下对传感器进行不同幅值、不同脉宽的冲击加载。然后,结合步骤(1)~(3)中获得的实验数据,构建ANN的训练集和验证集。
3. 结果与分析
3.1 杆中载荷一维性分析
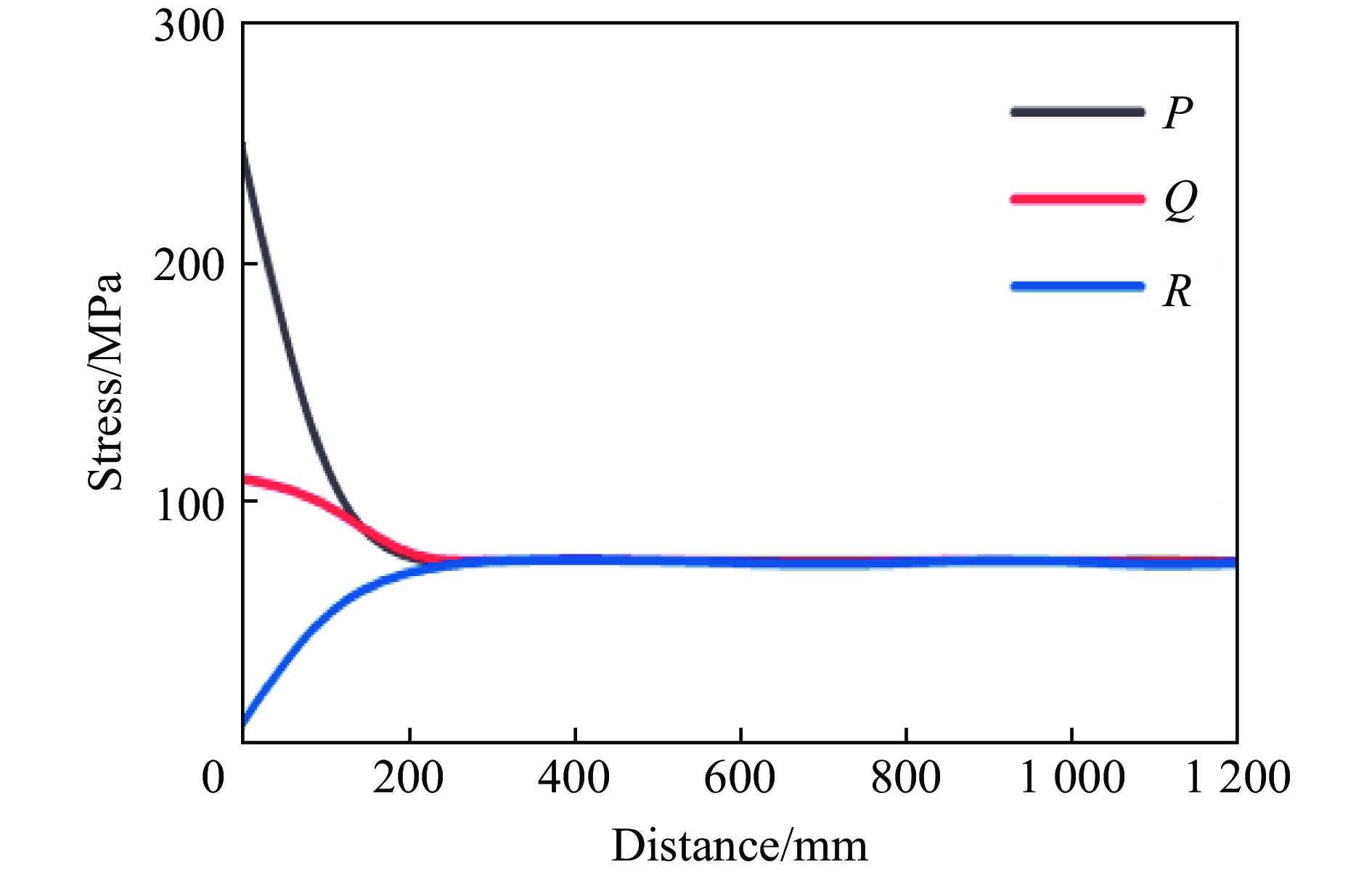
为了对Hopkinson杆中激励的载荷的一维性进行分析,采用有限元软件ABAQUS建立了图6所示的数值模型,P、Q和R为标定杆横截面上的采样点,
l 为采样截面到标定杆前端的距离。当P点、Q点和R点的轴向应力趋于一致或相等时即认为标定杆中的应力达到了均匀的一维应力状态。模拟中采用子弹1进行典型分析,各采样点处轴向应力的幅值随采样截面到标定杆前端面距离l 的变化如图7所示。可以看出,标定杆撞击端面上的轴向应力值有较大分散性,但分散性随截面到杆端距离l 的增大而不断减小。当l 增大到300 mm时,各采样点处的应力状态已基本达到一致。因此,虽然前端带有锥度的子弹会破坏标定杆前端一定距离范围内的轴向应力状态,但对杆后端面的轴向一维应力状态并无影响。而且,数值模拟的结果表明,当标定杆中的应力状态趋于稳定后,应力幅值几乎不再发生变化,因此,对于实验中所采用的截面为20 mm×20 mm的方杆,在根据应变片信号对标定杆激励的冲击力载荷进行解算时波形弥散造成的误差可忽略不计。3.2 载荷频谱分析
为避免引起传感器结构的谐振响应,施加到传感器各轴冲击载荷的上限截止频率应低于该轴的一阶固有频率。而传感器各轴的冲击载荷是由标定杆中激励的冲击载荷经矢量分解(线性变换)得到的。因此,只需使杆中载荷的上限截止频率小于传感器各轴的一阶固有频率即可。子弹1~5在标定杆中激励的典型时域载荷如图8(a)所示。可以看出,不同型号子弹激励的冲击力载荷均为类半正弦载荷,且脉宽随结构参数
d 的增大而增大。采用MATLAB对载荷脉冲进行快速傅里叶分解,得到的载荷的频域曲线如图8(b)所示。可以看出,随着谐波分量频率的增大,冲击力载荷的幅值不断减小。当频率超过12.273 kHz(z轴固有频率)时,冲击力载荷幅值已基本衰减为零。此外,冲击力载荷脉宽越大(d 越大),幅值随频率衰减越快,高频分量相对越小。3.3 感器灵敏度系数冲击特性
单轴(z轴)以及三轴加载方式下传感器的典型输入载荷和输出电压如图9所示。可以看出,当传感器仅z轴受载时,输入载荷和输出电压在脉冲构型上均具有较高的一致性。这表明,标定杆中激励的输入载荷可以被传感器的敏感轴有效探测到,标定装置具有有效性。此外,由图9(a),当传感器只有z轴受载时,x、y轴在没有载荷输入的情况下仍有电压输出,说明传感器各敏感轴之间确实存在耦合关系。将图9(b)中的典型输入输出数据代入式(7),得到的传感器灵敏度系数矩阵为:
S=[SxxSxySxzSyxSyySyzSzxSzySzz]=[0.4140.040−0.0230.0350.422−0.027−0.047−0.0580.246] (13) 由式(13),传感器的主灵敏度系数与标示值之间均存在一定的偏差;其中,
Sxx 、Syy 相对标示值较小,Szz 相对标示值较大。由耦合灵敏度系数,z轴、y轴之间具有正耦合关系,x轴、z主轴以及y轴、z轴之间具有负耦合关系,且各轴间的耦合均具有方向性,即任意两轴间的耦合均为非对称耦合。在
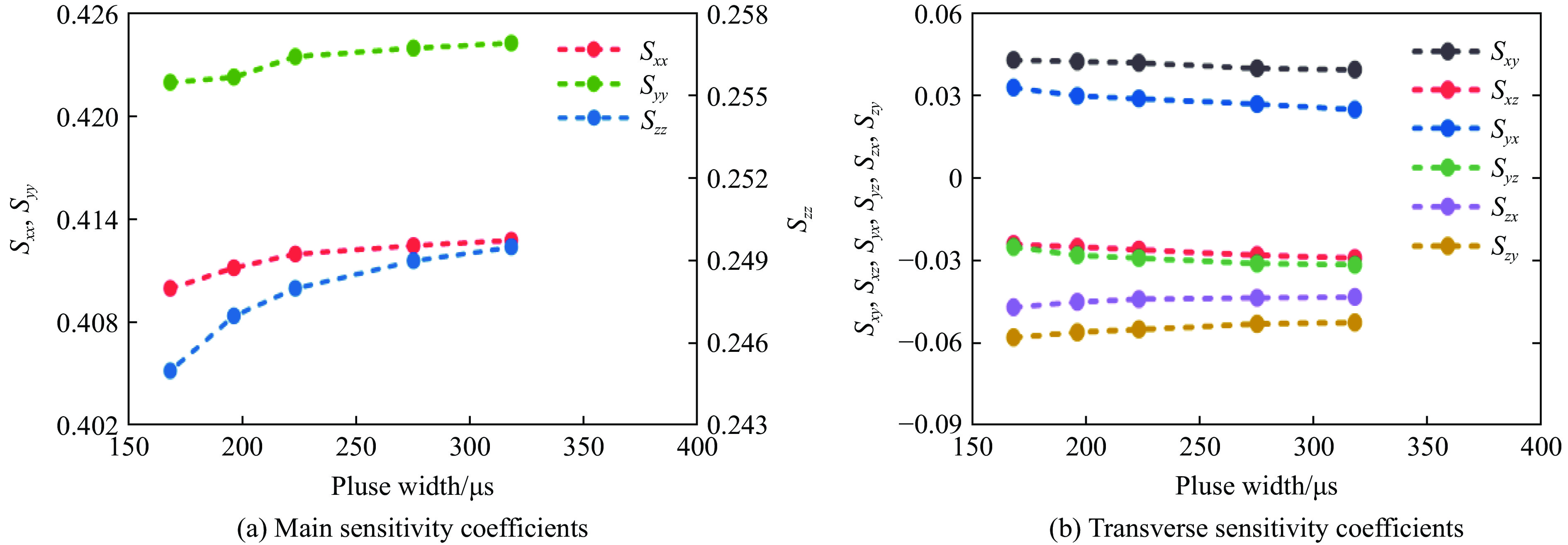
\theta =30°\mathrm{和}\omega =30° 工况下,采用子弹1对传感器主灵敏度系数和轴间耦合灵敏度系数进行相同脉宽168(1±2%) μs、不同幅值的冲击标定,结果如图10所示。采用子弹1 ~ 5进行相同幅值30(1±5%) kN、不同脉宽的冲击标定,结果如图11所示。需要说明的是,此处的脉宽是指脉冲上升沿上力值为脉冲峰值10%的点到脉冲下降沿上力值为峰值脉冲10%的点之间的时间差。此处的冲击力载荷指的是由标定杆上入射波与反射波解算出来的未经矢量分解的冲击力脉冲载荷,受传感器各敏感轴量程的限制,在\theta =30°\mathrm{和}\omega =30° 工况下标定杆施加给传感器承载工装的冲击力载荷不能超过34 kN。由图10~11,三轴冲击力传感器的主灵敏度系数和轴间耦合灵敏度系数均是冲击力载荷脉冲构型的函数。具体表现为主灵敏度系数{S}_{xx} 、{S}_{yy} 和{S}_{{\textit{z}}{\textit{z}}} 与载荷幅值呈负相关,与载荷脉宽呈正相关;轴间耦合灵敏度系数(绝对值){S}_{xy} 和{S}_{x{\textit{z}}} 与载荷幅值呈正相关,{S}_{yx} 、{S}_{y{\textit{z}}} 、{S}_{{\textit{z}}x} 和{S}_{{\textit{z}}y} 与载荷幅值呈负相关;而与载荷脉宽的相关性方面,{S}_{x{\textit{z}}} 和{S}_{y{\textit{z}}} 与载荷脉宽呈正相关,{S}_{yx} 、{S}_{y{\textit{z}}} 、{S}_{{\textit{z}}x} 和{S}_{{\textit{z}}y} 与载荷脉宽呈负相关。3.4 标定模型训练、求解及精度对比
由灵敏度系数的动态特性分析可知,三轴冲击力传感器主灵敏度系数与轴间耦合灵敏度系数均与载荷的脉宽和幅值有关。因此,要实现三轴冲击力传感器的准确标定,在标定模型的输入端须增设能够反映载荷构型信息的变量,以使标定模型具备随载荷构型调整传感器灵敏度关系的能力。而载荷构型信息将最终反映在输出电压的幅值和脉宽上。于是,在ANN模型建模时,将传感器各敏感轴电压脉冲的幅值
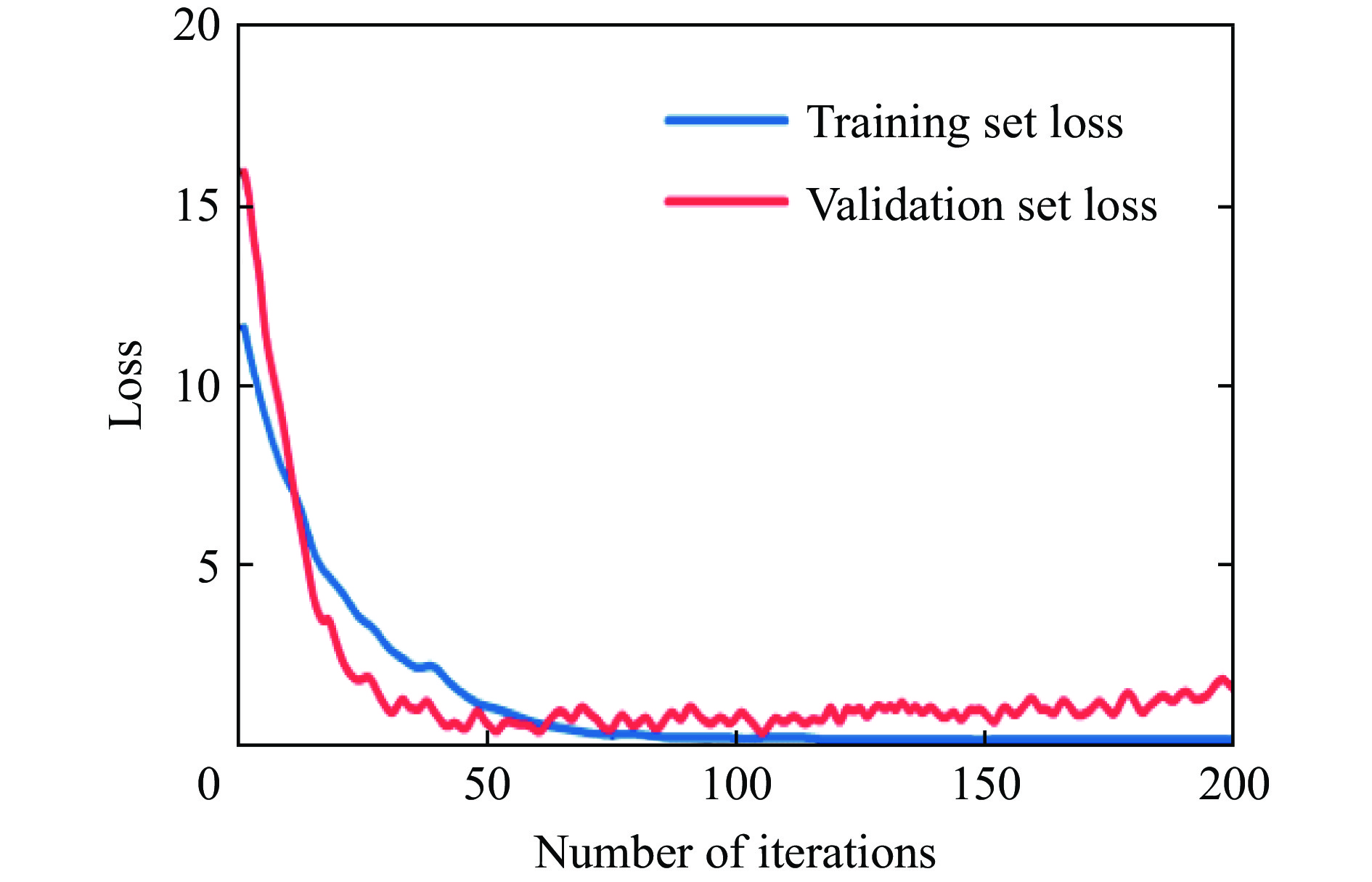
{A}_{x} 、{A}_{y} 、{A}_{{\textit{z}}} 以及脉宽{W}_{\mathrm{u}} 作为影响因素,并以神经元的形式添加到输入层中。因此,在最终建立的ANN模型中,输入层有7个神经元,分别为{U}_{x} 、{U}_{y} 、{U}_{{\textit{z}}} 、{A}_{x} 、{A}_{y} 、{A}_{{\textit{z}}} 和{W}_{\mathrm{u}} ;输出层含有3个神经元,分别为{F}_{x} 、{F}_{y} 和{F}_{{\textit{z}}} ;隐含层神经元的个数的确定目前尚无完备、成熟的理论可以借鉴,采用试错法[24]最终确定为43个。整理实验数据,构建涵盖每一种不同实验工况的样本集。构建的样本集包含36 000个样本,将其以8∶2的比例分解为训练集和验证集,采用训练集对ANN进行训练,BP算法遍历整个训练集一次计为一次迭代。验证集与训练集上的损失(均方误差)随迭代次数的变化如图12所示。可以看出,随迭代次数的增加,训练集上的损失不断减小并逼近于零,说明ANN可进行有效的学习。而验证集上的损失在迭代初期迅速减小至最小值后又呈现出缓慢增大的趋势,造成这一现象的主要原因是当模型在验证集上的损失减小到最小值后,如果继续对模型进行训练会使其陷入过拟合状态,造成模型的预测精度降低,使得验证集上的损失又有所增加。验证集上的损失在第67次迭代后已达到最小值,而训练集上的损失则在第130次迭代后才逐渐收敛于零,两者并不同步。若以训练集上的损失作为学习终止条件,则模型早已处于过拟合状态;此外,以ANN为典型代表的机器学习模型更加关注模型的泛化性能,因此将第67次迭代后的ANN作为训练合格的模型用于三轴冲击力传感器的标定。
将样本集中的示例和标记分别以电压矩阵和力矩阵的形式代入(7)式,即可得到实现三轴冲击力传感器线性解耦标定的最小二乘模型。图13给出了ANN验证集中4种不同加载工况下最小二乘模型与ANN标定结果的对比。其中,图13(a)、(b)为相同脉宽(
{W}_{\mathrm{F}} )、不同幅值({A}_{\mathrm{F}} )冲击力载荷标定结果的对比;图13(c)、(d)为相同幅值、不同脉宽冲击力载荷标定结果的对比。由图13(a)、(b),当冲击力载荷幅值分别为15和32 kN时,最小二乘模型标定结果相对真实值分别偏大和偏小。而由图13(c)、(d),当冲击力载荷脉宽分别为196 和318 μs时,最小二乘模型标定结果相对真实值分别偏小和偏大。造成上述现象的主要原因是影响三轴冲击力传感器灵敏度关系的决定性因素{S}_{xx} 、{S}_{yy} 及{S}_{{\textit{z}}{\textit{z}}} 随冲击力载荷幅值的增大而减小、随冲击力载荷脉宽的增大而增大;而最小二乘模型无法根据冲击力载荷的构型信息对传感器的灵敏度关系做出调整,因为最小二乘法本质上是对所有加载工况下三轴冲击力传感器输出到输入的线性拟合。线性模型无法表征非线性关系,也是最小二乘模型标定结果与真实值之间出现较大偏差的重要原因。不同加载工况下,ANN的标定结果虽存在一定范围的震荡,如图13(a)局部放大所示,但其整体走势与冲击力载荷的真实值基本重合,相对最小二乘模型明显具有更高的标定精度。该结果表明,ANN可实现不同加载工况下三轴冲击力传感器的非线性解耦标定;同时证明,三轴冲击力传感器各轴输出电压脉冲的幅值与脉宽可有效反映载荷的构型信息,将其以神经元的形式添加到输入层是基于ANN实现三轴冲击力传感器准确标定的有效方式。
4. 结 论
基于Hopkinson杆与矢量分解原理建立了一种可计量三分量冲击力载荷同步激励方法,实现了对冲击力传感器的三轴同步加载。基于最小二乘原理与矩阵微分对包含三轴冲击力传感器主灵敏度系数与轴间耦合灵敏度系数的线性解耦标定模型进行了求解。对比不同冲击载荷下的标定结果,揭示了传感器主灵敏度系数与轴间耦合灵敏度系数均与冲击力载荷脉冲构型相关的冲击特性。将能够反映载荷构型信息的传感器各轴输出电压脉冲的幅值与脉宽以神经元的形式添加到ANN输入层,建立了可实现三轴冲击力传感器非线性解耦标定的ANN模型。通过上述工作可以得到以下结论。
(1)三轴冲击力传感器的主轴灵敏度系数与轴间耦合灵敏度系数并非固定常数,而是会随冲击力载荷脉宽与幅值发生变化的变量。
(2)基于最小二乘的线性解耦标定模型无法根据冲击力载荷的构型信息对传感器的灵敏度关系做出相应调整,导致其标定精度较低,不适用于三轴冲击力传感器的标定。
(3)三轴冲击力传感器各轴输出的电压脉冲的幅值和脉宽可有效反映冲击力载荷的构型信息,采用ANN对三轴冲击力传感器进行非线性解耦标定时,将其以神经元的形式添加到模型的输入层是实现准确标定的有效方式。
-
表 1 B25B型三轴冲击力传感器的性能参数
Table 1. Parameters of a B25B tri-axial impact force transducer
测量轴 量程/
kN激励电压/
V一阶固有频率/
kHz灵敏度系数/
(mV·kN−1)x 15 10 12.275 0.480 y 15 10 12.275 0.480 z 30 10 12.273 0.223 -
[1] 郑晓静. 关于极端力学 [J]. 力学学报, 2019, 51(4): 1266–1272. DOI: 10.6052/0459-1879-19-189.ZHENG X J. Extreme mechanics [J]. Chinese Journal of Theoretical and Applied Mechanics, 2019, 51(4): 1266–1272. DOI: 10.6052/0459-1879-19-189. [2] 李波, 杨家斌, 舒亮, 等. 基于Galfenol合金的高灵敏度冲击力传感器 [J]. 机械工程学报, 2019, 55(8): 14–23. DOI: 10.3901/JME.2019.08.014.LI B, YANG J B, SHU L, et al. High sensitivity impact force sensor based on Galfenol alloy [J]. Journal of Mechanical Engineering, 2019, 55(8): 14–23. DOI: 10.3901/JME.2019.08.014. [3] DINOVITZER H A, LARONCHE A, ALBERT J. Fiber Bragg grating high impact force sensors with adjustable sensitivity and dynamic range [J]. IEEE Sensors Journal, 2019, 19(14): 5670–5679. DOI: 10.1109/JSEN.2019.2907867. [4] PARK Y K, KUMME R, KANG D I. Dynamic investigation of a three-component force-moment sensor [J]. Measurement Science and Technology, 2002, 13(5): 654–659. [5] PARK Y K, KUMME R, KANG D I. Dynamic investigation of a binocular six-component force-moment sensor [J]. Measurement Science and Technology, 2002, 13(8): 1311–1318. [6] SCHLEGEL C, KIECKENAP G, GLOCKNER B, et al. Traceable periodic force calibration [J]. Metrologia, 2012, 49(3): 224–235. DOI: 10.1088/0026-1394/49/3/224. [7] FUJII Y. Toward establishing dynamic calibration method for force transducers [J]. IEEE Transactions on Instrumentation and Measurement, 2009, 58(7): 2358–2364. DOI: 10.1109/TIM.2009.2014614. [8] SATRIA E, TAKITA A, NASBEY H, et al. New technique for dynamic calibration of a force transducer using a drop ball tester [J]. Measurement Science and Technology, 2018, 29(12): 125009. DOI: 10.1088/1361-6501/aaeb71. [9] 魏燕定. 标准负阶跃力的实现及其测量研究 [J]. 实验力学, 2001, 16(1): 7–12. DOI: 10.3969/j.issn.1001-4888.2001.01.002.WEI Y D. A study on the generation of standard negative step force and its measurement [J]. Journal of Experimental Mechanics, 2001, 16(1): 7–12. DOI: 10.3969/j.issn.1001-4888.2001.01.002. [10] 孙金锋, 谢殿煌, 张训文. 气动助推负阶跃力校准装置研究 [J]. 仪器仪表学报, 2010, 31(2): 459–463. DOI: 10.19650/j.cnki.cjsi.2010.02.037.SUN J F, XIE D H, ZHANG X W. Study of pneumatic push negative step force calibration device [J]. Chinese Journal of Scientific Instrument, 2010, 31(2): 459–463. DOI: 10.19650/j.cnki.cjsi.2010.02.037. [11] HU G Y, GAO Q, CAO H B, et al. Decoupling analysis of a six-dimensional force sensor bridge fault [J]. IEEE Access, 2017, 6: 7029–7036. DOI: 10.1109/ACCESS.2017.2784485. [12] YOU J J, WANG L K, XI F F, et al. Decoupling algorithm and maximum operation frequency of a novel parallel type six-axis accelerometer [J]. IEEE Sensors Journal, 2020, 20(21): 12637–12651. DOI: 10.1109/JSEN.2020.3001250. [13] TAN Y S, WANG X Y, REN L M. Design and experiment of a cardan-type self-decoupled and self-powered bending moment and torque sensor [J]. IEEE Transactions on Industrial Electronics, 2020, 68(6): 5366–5375. DOI: 10.1109/TIE.2020.2991931. [14] LIANG Q K, LONG J Y, COPPOLA G, et al. Novel decoupling algorithm based on parallel voltage extreme learning machine (PV-ELM) for six-axis F/M sensors [J]. Robotics and Computer-Integrated Manufacturing, 2019, 57: 303–314. DOI: 10.1016/j.rcim.2018.12.014. [15] LI Y J, WANG G C, YANG X, et al. Research on static decoupling algorithm for piezoelectric six axis force/torque sensor based on LSSVR fusion algorithm [J]. Mechanical Systems and Signal Processing, 2018, 110: 509–520. DOI: 10.1016/j.ymssp.2018.03.015. [16] 李映君, 韩彬彬, 王桂从, 等. 基于径向基函数神经网络的压电式六维力传感器解耦算法 [J]. 光学精密工程, 2017, 25(5): 1266–1271. DOI: 10.3788/OPE.20172505.1266.LI Y J, HAN B B, WANG G C, et al. Decoupling algorithms for piezoelectric six-dimensional force sensor based on RBF neural network [J]. Optics and Precision Engineering, 2017, 25(5): 1266–1271. DOI: 10.3788/OPE.20172505.1266. [17] 谢石林, 陈胜来, 张希农, 等. 传感器标定的神经网络杂交建模方法 [J]. 机械工程学报, 2010, 46(22): 6–15. DOI: 10.3901/JME.2010.22.006.XIE S L, CHEN S L, ZHANG X N, et al. Neural network Hybrid modeling method for transducer calibration [J]. Journal of Mechanical Engineering, 2010, 46(22): 6–15. DOI: 10.3901/JME.2010.22.006. [18] LI T L, ZHENG H, PAN A Q, et al. BP Method With Rectified Linear Unit-Based Nonlinear Decoupling for 3-Axis FBG Force Sensor [J]. IEEE Sensors Journal, 2021, 21(3): 2972–2979. DOI: 10.1109/JSEN.2020.3022663. [19] GOODFELLOW I, BENGIO Y, COURVILLE A. 深度学习[M]. 赵申剑, 黎彧君, 符天凡, 等译. 北京: 人民邮电出版社, 2017: 8–15. [20] 郭伟国, 李玉龙, 索涛. 应力波基础简明教程[M]. 西安: 西北工业大学出版社, 2007: 26–42. [21] LIANG Q K, WU W N, COPPOLA G, et al. Calibration and decoupling of multi-axis robotic force/moment sensors [J]. Robotics and Computer-Integrated Manufacturing, 2018, 49: 301–308. DOI: 10.1016/j.rcim.2017.08.008. [22] 姚斌, 张建勋, 代煜, 等. 用于微创外科手术机器人的多维力传感器解耦方法研究 [J]. 仪器仪表学报, 2020, 41(1): 147–153. DOI: 10.19650/j.cnki.cjsi.J1905479.YAO B, ZHANG J X, DAI Y, et al. Research on decoupling method of multi-dimensional force sensor used in minimally invasive surgical robot [J]. Chinese Journal of Scientific Instrument, 2020, 41(1): 147–153. DOI: 10.19650/j.cnki.cjsi.J1905479. [23] 姜力, 刘宏, 蔡鹤皋. 多维力/力矩传感器静态解耦的研究 [J]. 仪器仪表学报, 2004, 25(3): 284–287. DOI: 10.3321/j.issn:0254-3087.2004.03.002.JIANG L, LIU H, CAI H G. Nonlinear static decoupling of multi-axis force/torque sensor [J]. Chinese Journal of Scientific Instrument, 2004, 25(3): 284–287. DOI: 10.3321/j.issn:0254-3087.2004.03.002. [24] 周志华. 机器学习[M]. 北京: 清华大学出版社, 2016: 97−120. 期刊类型引用(1)
1. 宋攀峰. 动态力解耦和动态标定相关技术研究综述. 计量科学与技术. 2023(12): 40-46+66 .  百度学术
百度学术其他类型引用(0)
-









 下载:
下载:
















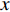


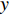





































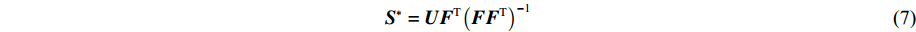


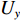

























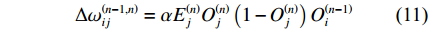






































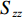




























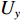












 下载:
下载:












 百度学术
百度学术