Fractal correction of dynamic fracture parameters of black sandstone under impact loads
-
摘要: 基于分形理论研究了偏折裂纹扩展路径对动载荷作用下黑砂岩的动态断裂力学参数的测试误差影响作用，采用传统的分离式霍普金森压杆（split Hopkinson pressure bar, SHPB）实验装置对修正侧开单裂纹半孔板（improved single cleavage semi-circle specimen, ISCSC）试样进行动态冲击实验，随后采用裂纹扩展计进行裂纹起裂时间与裂纹扩展速度等动态断裂力学参数测试，采用分形理论对测试的裂纹扩展速度与动态应力强度因子进行修正，利用实验-数值法对黑砂岩的动态断裂韧度进行计算。研究结果表明，ISCSC构型构件能够有效应用于岩石材料动态裂纹扩展行为的研究，并发生了止裂现象，经分形修正的裂纹扩展速度与动态断裂韧度更接近实际裂纹动态扩展情况，修正前后得到黑砂岩材料的裂纹扩展速度误差为33.51%，动态断裂韧度最大误差为7.68%，说明利用分形理论对动态断裂韧度等动态断裂参数计算更合理。Abstract: When studying the dynamic fracture behavior of cracked rock mass, dynamic fracture toughness is an important mechanical parameter to study the fracture characteristics of cracks, which can accurately reflect the energy required in the crack initiation and propagation stage. However, compared with the static fracture problem, it is difficult to obtain an analytical solution for dynamic fracture toughness. Therefore, many scholars measure the crack propagation speed by using crack propagation gauges, and then calculate the dynamic fracture toughness according to the universal function. In this way, the crack propagation speed plays a leading role in the calculation accuracy, but in the experiment, the crack propagation speed cannot be measured accurately due to the measuring instrument. In this paper, the fractal theory is used to correct this error. According to the fractal theory, the effects of deflected crack propagation trajectories on dynamic fracture properties of black sandstone under impact loads were studied. A traditional modified split Hopkinson pressure bar (SHPB) test device was used to conduct a dynamic impact test by using an improved single cleavage semi-circle (ISCSC) specimen, crack propagation speed and other fracture mechanics parameters were measured using crack propagation gauge (CPG). Subsequently, the fractal theory was applied to correct dynamic crack propagation speed and dynamic stress intensity factor, and the dynamic fracture toughness of black sandstone was also calculated using the experimental-numerical method. The research results indicate that the ISCSC specimen can be effectively applied to study the crack arrest behavior of rock materials. Crack propagation speed and dynamic fracture toughness after fractal correction are closer to the actual dynamic crack propagation characteristics. Comparisons between before and after the correction, the maximum error of the crack propagation speed of black sandstone material is 33.51%, and the maximum error of dynamic fracture toughness is 7.68%, indicating that it is more reasonable to use fractal theory to calculate dynamic fracture parameters such as crack propagation speed and dynamic fracture toughness.
-
聚乙烯作为一种由乙烯聚合而成的高分子材料，被广泛应用于工业、农业及日常生活中[1-4]。在聚乙烯生产过程中（如造粒、干燥、气力输送、卸料等环节）极易形成高浓度聚乙烯粉尘云[5]，存在较高爆炸风险。目前，国内外学者们已对不同粒径、质量浓度以及可燃气体等因素影响下的聚乙烯粉尘爆炸特性进行了研究，发现聚乙烯粉尘爆炸机理复杂，爆炸危险性高[6-10]，一旦发生爆炸事故将造成严重的人员伤亡和财产损失。例如，2002年辽阳石化烯烃厂聚乙烯装置改扩建过程中发生聚乙烯粉尘爆炸事故，导致8人死亡、19人受伤。2003年美国北卡罗来纳州一个生产合成橡胶药物传递原件的制药厂发生聚乙烯粉尘爆炸事故，造成6人死亡、38人受伤。因此开展相应的聚乙烯粉尘爆炸防治技术研究具有重要意义。
爆炸抑制技术因其积极、高效的爆炸防治效果，成为目前最为常用的爆炸防治技术之一。该技术的关键在于抑爆剂性能。粉体抑爆剂由于储运方便、经济高效而被广泛关注[11-13]。目前，研究人员已经开展了大量关于粉体抑爆剂抑制聚乙烯粉尘爆炸方面的研究。Zhang等[14]通过采用哈特曼管实验装置对比研究了化学抑制剂(NH4)2CO3和物理抑制剂SiC对聚乙烯粉尘爆炸的抑制效果，并分析了两者对聚乙烯粉尘爆炸的抑制机理。Wang等[15]实验研究了NaHCO3对聚乙烯粉尘的爆炸特性参数以及火焰传播行为的影响，发现NaHCO3粉末能有效抑制聚乙烯粉尘爆炸。Lin等[16]采用立式透明石英管爆炸装置，研究了Al(OH)3粉末对聚乙烯粉尘爆炸火焰传播的抑制作用及其机理。Addai等[17]通过实验研究发现物理抑制剂如MgO、沙子等可有效降低聚乙烯粉尘活性，MgO具有更好的惰化效果。上述抑爆剂均可在一定程度上抑制聚乙烯粉尘爆炸，但在使用过程中还存在性能有限、释放有毒有害气体[18]、易潮解团聚等问题[19-20]。因此寻求新型、清洁、高效的粉体抑爆剂是目前研究的热点。
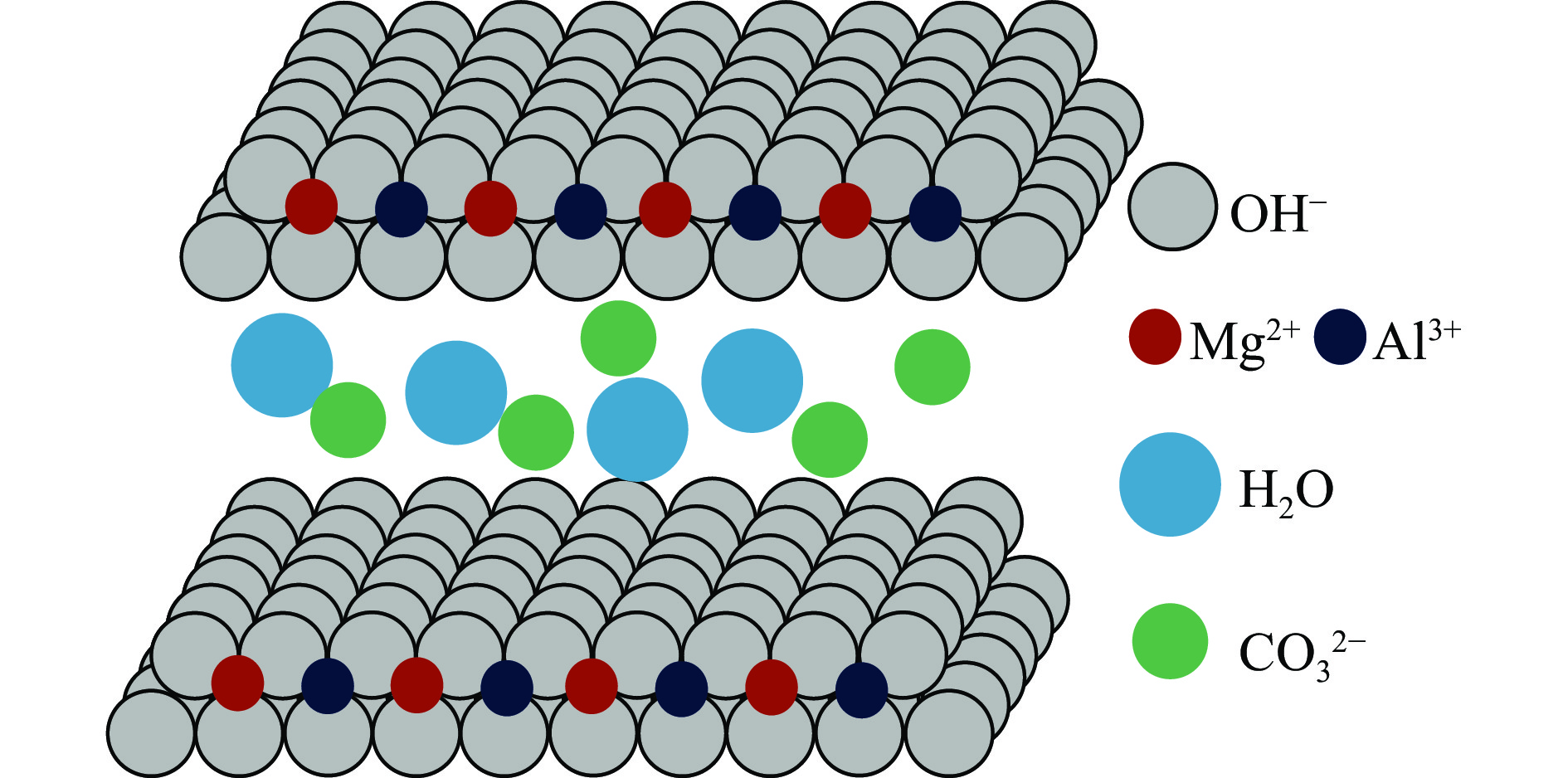
镁铝水滑石是一种具有层间离子的层状双金属氢氧化物，如图1所示。由于其独特的结构和性质[21]，镁铝水滑石在水处理、催化与光催化、生物、农业、传感[22-27]等领域有着广泛应用。此外，高温下镁铝水滑石受热分解生成的金属氧化物能促进基体的催化碳化，形成物理屏障[28]，进而起到隔绝空气的目的，因此它还通常被用作固体材料的阻燃剂。粉尘爆炸的本质是粉末状固体材料的快速燃烧，因此从阻燃的角度分析，镁铝水滑石应具有一定的粉尘爆炸抑制潜力。但是粉尘爆炸比固体材料燃烧更快速、更剧烈，镁铝水滑石抑制粉尘爆炸是否有效还有待验证。目前，已有研究人员开展了和镁铝水滑石相似分子组成的氢氧化镁和氢氧化铝对粉尘的抑爆实验。Huang等[29]研究了不同粒径和质量分数的超细氢氧化镁对木粉爆炸火焰的抑制作用，发现氢氧化镁粉体对木尘爆炸火焰有明显的抑制作用。Zhang等[30]研究了纳米氢氧化铝泡沫的阻燃抑烟性能，阐明了纳米氢氧化铝泡沫的防火灭火机理。Wang等[31]研究了氢氧化铝和氢氧化镁对镁铝合金粉尘爆炸的抑制作用，发现提高氢氧化铝和氢氧化镁的质量分数，可以有效降低爆燃火焰高度、火焰传播速度和爆燃压力。另外，也有学者利用氢氧化镁和氢氧化铝对粉尘爆炸进行了研究[32-34]。上述研究表明氢氧化镁、氢氧化铝具有良好的吸热性能，而且分解产生的氧化物可以附着在粉尘颗粒表面阻碍热传导，进而起到降低火焰温度，削弱爆炸强度的作用，展现了良好的抑爆性能。由于镁铝水滑石的分子组成与氢氧化镁和氢氧化铝相似，且高温下热解也具有吸热性能，并能热解出MgO、Al2O3等氧化物[35]，因此从该角度分析，镁铝水滑石应具有一定的粉尘爆炸抑制效果。但是目前关于镁铝水滑石抑制粉尘爆炸的研究较少，相关性能和机理还不清楚。
基于此，本文中以聚乙烯粉尘爆炸灾害防治为工业背景，开展镁铝水滑石抑制聚乙烯粉尘爆炸特性和机理研究，主要分析镁铝水滑石作用下聚乙烯粉尘爆炸最大爆炸压力、最大爆炸压力上升速率、最低着火温度等参数变化规律，在此基础之上，结合镁铝水滑石的物理化学性质来分析其抑爆机理，同时与相似分子组成的氢氧化镁、氢氧化铝抑制聚乙烯粉尘爆炸特性作对比。研究成果一方面可为聚乙烯粉尘爆炸灾害防治提供参考和依据，另一方面可为新型、清洁、高效抑爆剂的研发提供新思路。
1. 实验装置与材料
1.1 实验装置
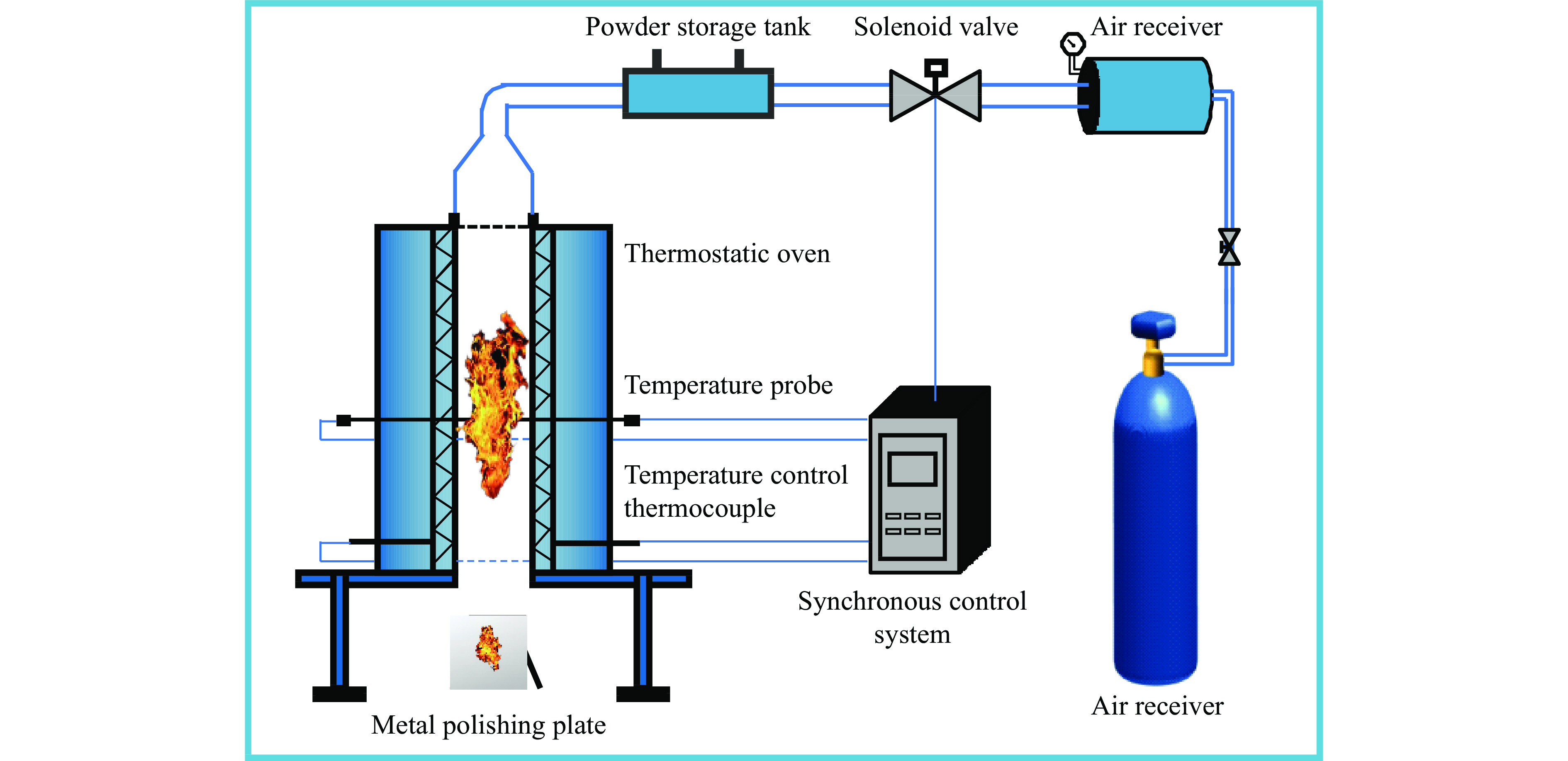
实验采用标准20 L球形爆炸装置测试抑爆粉体作用下聚乙烯粉尘爆炸超压，该装置符合GB/T 16426—1996《粉尘云最大爆炸压力和最大压力上升速率测定方法》标准的要求[36]。如图2所示，标准20 L球形爆炸装置主要包括喷粉系统、点火系统、球形爆炸室、控制系统及数据采集系统。依据国标要求[36]，实验采用10 kJ化学点火能点火，点火延迟时间为60 ms，喷粉压力为2 MPa。为确保实验结果的准确性和科学性，每组实验至少重复3次。
实验采用的粉尘云最低着火温度测试装置符合GB/T 16429—1996《粉尘云最低着火温度测定方法》[37]，如图3所示，主要包括喷粉系统和温控系统。根据国标要求[37]，实验设置喷粉压力为0.05 MPa，温度梯度设置为10 ℃。通过金属抛光板观察容器内部点火的发生与否，发生点火的最低温度被视为最低着火温度。
1.2 实验材料
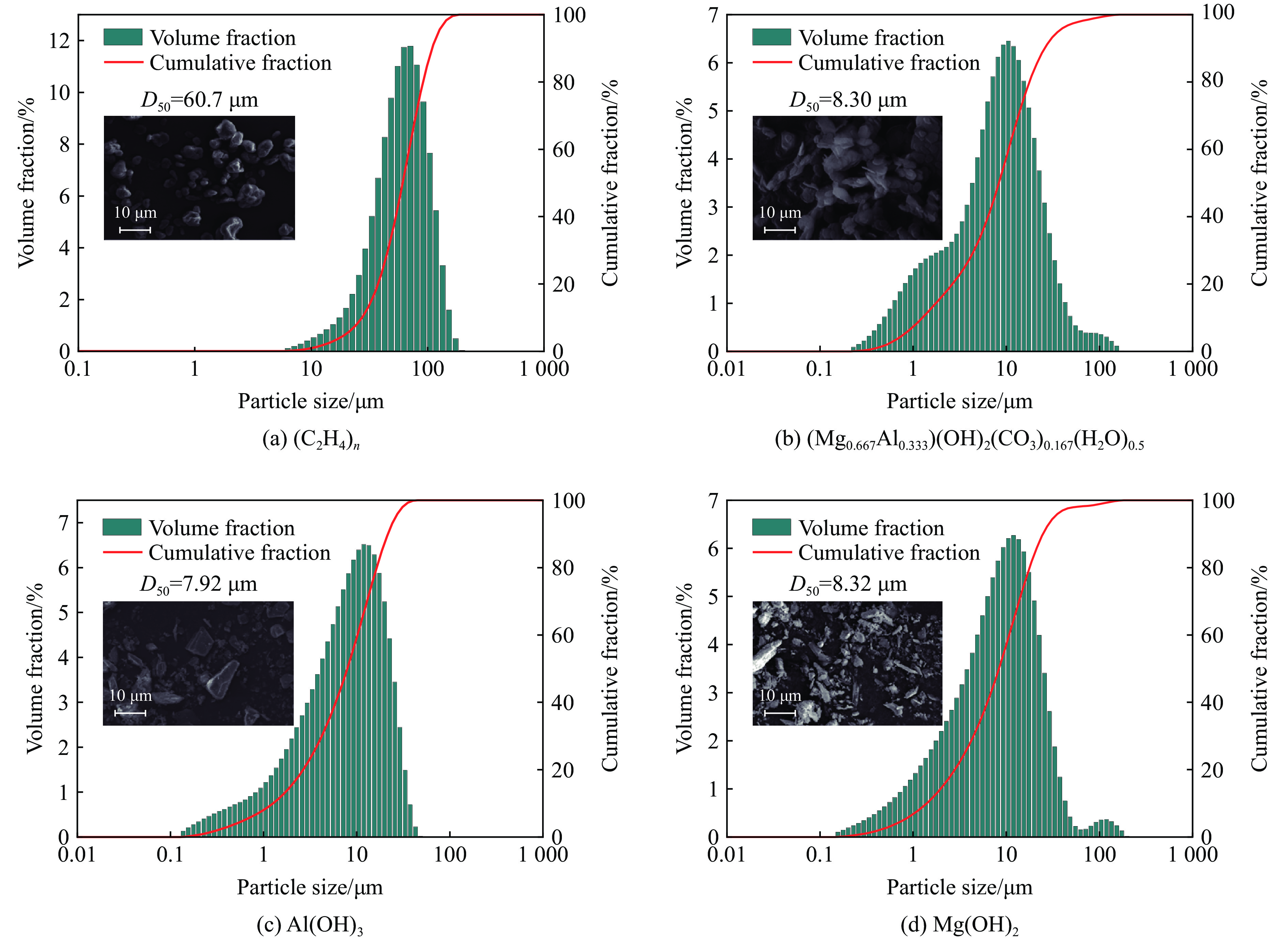
实验中使用的聚乙烯粉尘由江苏豪胜塑料有限公司提供，镁铝水滑石、氢氧化铝及氢氧化镁均由山东优索化工科技有限公司提供。选用的聚乙烯粉尘粒径和工业实际生产过程中形成的聚乙烯粉尘粒径一致。由于抑爆效果和抑爆粉体粒径相关，为了避免粒径差异对抑爆性能的影响，通过研磨调整粒径并检测粒径分布；为进一步观察其表面形态，了解其结构特征，对4种粉体进行扫描电镜，其粒径分布和扫描电镜如图4所示。由图4可知，聚乙烯、镁铝水滑石和氢氧化铝及氢氧化镁的中位粒径D50分别为60.7、8.30、7.92和8.32 μm。镁铝水滑石、氢氧化铝、氢氧化镁这3种抑爆粉体的粒径分布基本一致，进而避免了粒径差异对抑爆性能的影响。聚乙烯粉尘颗粒表面光滑，分散均匀。镁铝水滑石粉体为不规则的六边形片状结构，分散性相对较差。氢氧化铝和氢氧化镁的结构特征相似，都为块状结构，表面粗糙，大小分布不均匀。
图 4 4种粉体的粒径分布及扫描电镜图Figure 4. Particle size distribution and scanning electron microscopy of four powders2. 实验结果与讨论
2.1 抑爆剂对聚乙烯粉尘爆炸超压的影响
作为对照和参考，实验首先对不同质量浓度聚乙烯粉尘爆炸超压进行了测量，结果如图5所示。由图5可知，聚乙烯粉尘的最大爆炸压力pmax和爆炸压力的最大上升速率(dp/dt)max均随粉尘质量浓度的提高呈现先增大后减小的趋势，当聚乙烯粉尘质量浓度为300 g/m3时，爆炸压力和爆炸压力上升速率均达到最大值，分别为0.65 MPa和22.46 MPa/s。因此，抑爆实验中均选用300 g/m3的聚乙烯粉尘作为抑爆对象。
图 5 聚乙烯爆炸超压随粉尘质量浓度的变化规律Figure 5. Variation of polyethylene explosion overpressure with dust mass concentration以300 g/m3聚乙烯粉尘为抑爆对象，对不同抑爆粉体质量浓度下聚乙烯粉尘的爆炸压力和爆炸压力上升速率进行了测量。为了便于对比分析镁铝水滑石、氢氧化铝和氢氧化镁等3种抑爆粉体对聚乙烯粉尘爆炸的抑制性能，特定义无量纲参数抑制比为抑爆粉体与抑爆对象聚乙烯粉体的质量比，用符号λ表示，得到聚乙烯粉尘爆炸超压随抑制比的变化规律，如图6～7所示。
图 6 3种抑爆粉体作用下聚乙烯粉尘最大爆炸压力随抑制比的变化规律Figure 6. Variation of the maximum explosion pressure of polyethylene dust with inhibition ratio under the action of three kinds of explosion suppression powders
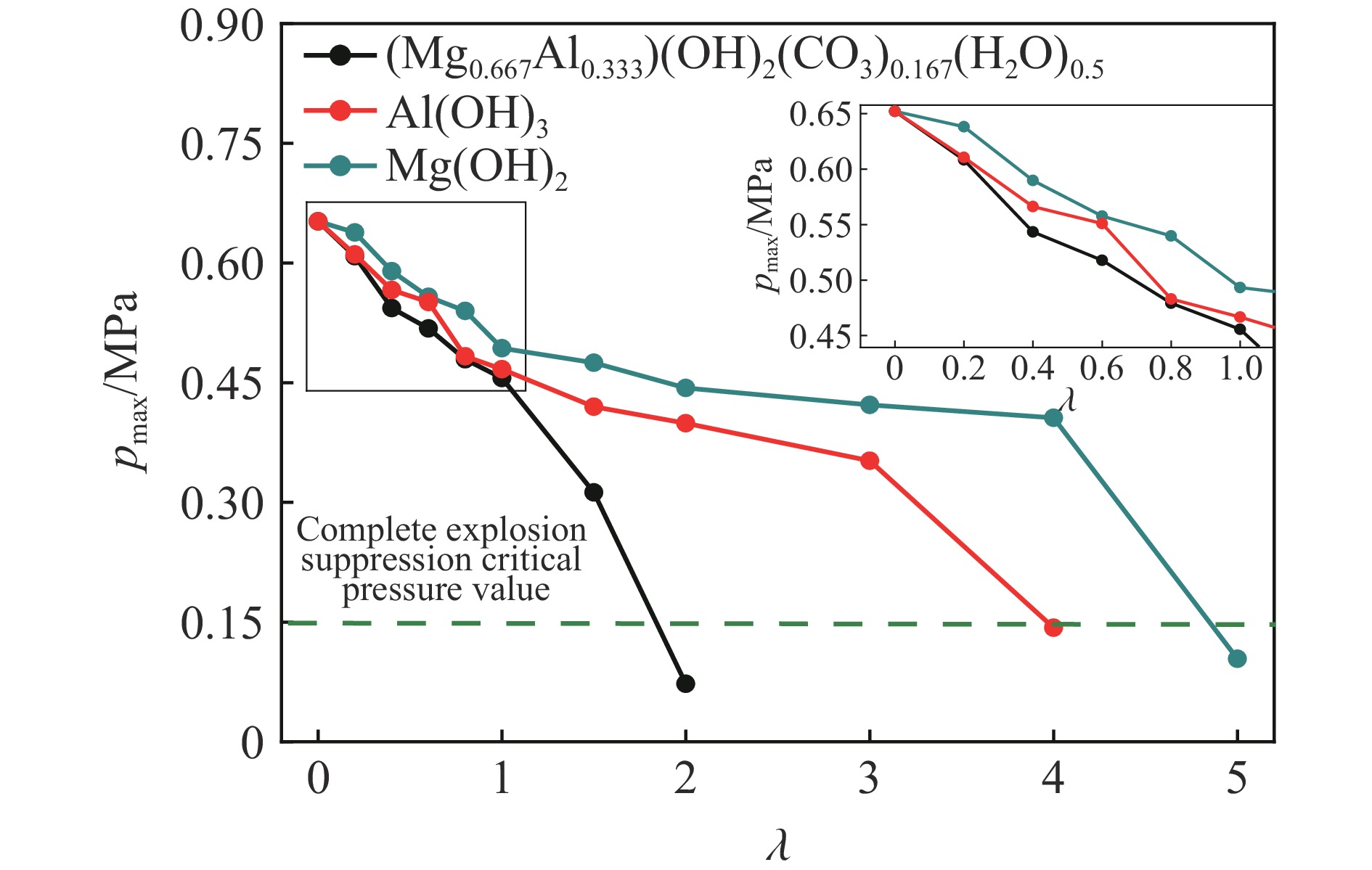
图 7 3种抑爆粉体作用下聚乙烯粉尘最大爆炸压力上升速率随抑制比的变化规律Figure 7. Variation of the maximum explosion pressure rise rate of polyethylene dust with inhibition ratio under the action of three explosion suppression powders图6为3种抑爆粉体作用下聚乙烯粉尘最大爆炸压力pmax随抑制比的变化规律。由图6可以看出，随着抑制比的不断增大，聚乙烯粉尘的最大爆炸压力逐渐减小。当抑制比为1时，在镁铝水滑石、氢氧化铝和氢氧化镁作用下，聚乙烯粉尘的最大爆炸压力分别为0.45、0.46和0.49 MPa，较纯聚乙烯粉尘最大爆炸压力分别下降了30.13%、28.43%、24.62%。此时三者的抑爆性能差距较小，结合3种抑爆粉的扫描电镜可知，这可能是因为镁铝水滑石相对较差的分散性影响了其与聚乙烯粉体的充分混合，阻碍了其抑制性能的发挥。依据EN 14034-3[38]中规定容器中爆炸压力pex≥pig+0.05 MPa时，可认为容器内粉尘发生了爆炸，本实验中pig≈0.10 MPa，因此将0.15 MPa设为临界值，低于0.15 MPa视为完全抑爆。由图6可知，随着抑制比的不断增大，镁铝水滑石的抑爆优势快速凸显，并在抑制比为2时实现完全抑爆，而氢氧化铝和氢氧化镁分别在抑制比为4和5时才能达到完全抑爆。由此可推断，相同抑制比条件下镁铝水滑石对聚乙烯粉尘爆炸压力的抑制性能优于氢氧化铝和氢氧化镁。
图7所示为3种抑爆粉体作用下聚乙烯粉尘最大爆炸压力上升速率(dp/dt)max随抑制比变化规律。从图7可以看出，抑爆粉体作用下聚乙烯粉尘最大爆炸压力上升速率呈现与爆炸压力相似的规律，即聚乙烯粉尘最大爆炸压力上升速率随抑制比的增大逐渐减小。当抑制比为1时，在镁铝水滑石、氢氧化铝、氢氧化镁作用下聚乙烯粉尘的最大爆炸压力的上升速率分别为7.08、9.68和10.37 MPa/s，较纯聚乙烯粉尘最大爆炸压力上升速率分别下降了68.46%、56.92%和53.85%，下降幅度高于最大爆炸压力降幅，即3种抑爆粉体对聚乙烯粉尘爆炸压力上升速率的抑制效果优于爆炸压力的抑制效果。相同抑制比下，镁铝水滑石导致聚乙烯粉尘的最大爆炸压力上升速率降幅最大，即镁铝水滑石对聚乙烯粉尘最大爆炸压力上升速率的抑制性能同样优于氢氧化铝和氢氧化镁的。
2.2 抑爆剂对聚乙烯粉尘最低着火温度的影响
根据GB/T 16429—1996《粉尘云最低着火温度测定方法》[37]要求及相关文献调研结果，以200 g/m3的质量浓度跨度对聚乙烯粉尘最低着火温度ti,min进行测试，结果如图8所示。由图8可知，随着聚乙烯粉尘质量浓度从600 g/m3增大到1400 g/m3，聚乙烯粉尘的最低着火温度先减小后增大。当聚乙烯粉尘质量浓度为1000 g/m3时，聚乙烯粉尘的最低着火温度最小，为460 ℃。因此，选取质量浓度为1000 g/m3的聚乙烯粉尘作为试验介质，对不同抑制比下聚乙烯粉尘的最低着火温度进行了测试，结果如图9所示。由图9可知，3种不同抑爆粉作用下，聚乙烯粉尘的最低着火温度均随着抑制比的增大呈线性增大。当抑制比为1时，在镁铝水滑石、氢氧化铝和氢氧化镁作用下聚乙烯的最低着火温度分别从460 ℃增大至750 、720和710℃，增幅分别为63.04%、56.52%和54.34%，即镁铝水滑石对聚乙烯粉尘的最低着火温度具有最优的抑制性能。但是这3种抑爆粉体对聚乙烯粉尘的最低着火温度的抑制性能差异不大，这可能是由于最低着火温度测试系统为半开放空间，镁铝水滑石热解生成的二氧化碳气体无法积聚，从而使得其抑爆优势不太明显。
图 8 聚乙烯粉尘最低着火温度随粉尘质量浓度变化规律图Figure 8. Variation of minimum ignition temperature of polyethylene dust with dust mass concentration
图 9 3种抑爆粉体作用下聚乙烯粉尘最低着火温度随抑制比变化规律Figure 9. Variation of minimum ignition temperature of polyethylene dust with inhibition ratio under the action of three explosion suppression powders2.3 抑爆机理分析
由上述分析可知，镁铝水滑石对聚乙烯粉尘爆炸具有良好的抑制性能，且优于氢氧化铝和氢氧化镁，其原因和机理可以从物理作用和化学作用两个方面进行分析。
2.3.1 物理作用
图10中给出了氢氧化铝、氢氧化镁和镁铝水滑石粉体的热重TG（thermo gravimetric）测试曲线和DSC（differential scanning calorimetry）曲线。如图10(a)～(b)所示，氢氧化铝、氢氧化镁分别在303 ℃和412 ℃出现1个主要吸热峰，且在该温度区间都有较大的失重。氢氧化铝在225 ℃开始热解，533 ℃结束，失重率为32.35%。氢氧化镁在330 ℃开始热解，702 ℃结束，失重率为29.15%。氢氧化铝、氢氧化镁都热解生成水和高温氧化物：
图 10 氢氧化铝、氢氧化镁和镁铝水滑石粉体的热重曲线和DSC曲线Figure 10. Thermogravimetric and DSC curves of Al(OH)3, Mg(OH)2 and (Mg0.667Al0.333)(OH)2(CO3)0.167(H2O)0.5 powdersMg(OH)2→MgO+H2O (1) Al(OH)3→Al2O3+H2O (2) 图10(c)所示为镁铝水滑石粉体热重分析图，与氢氧化镁粉体和氢氧化铝粉体不同，镁铝水滑石粉体在234 、320和418 ℃呈现3个主要的吸热峰。镁铝水滑石的热解分为2个质量损失阶段，第1个阶段为102～242 ℃，失重率为13.46%，层间结合水和物理吸附水脱除[39]。第2个阶段为242～518 ℃，失重率为27.8%，该阶段镁铝水滑石层间的碳酸根受热分解产生二氧化碳，层板上羟基逐渐脱除，剩余相为氧化铝和氧化镁[40]。具体的分解产物：
(Mg0.667Al0.333)(OH)2(CO3)0.167(H2O)0.5→0.667MgO+0.1665Al2O3+1.5H2O+0.167CO2 (3) 从氢氧化镁、氢氧化铝和镁铝水滑石粉体的热重分析中能够发现，3种粉体的失重率由低到高依次为氢氧化镁粉体、氢氧化铝粉体、镁铝水滑石粉体。
镁铝水滑石一方面可以通过自身分解吸收聚乙烯粉尘爆炸过程中的热量，另一方面热解过程中产生的高温氧化物会吸附在聚乙烯粉尘颗粒表面，隔离热量传递，这和氢氧化铝和氢氧化镁的抑制过程一致。镁铝水滑石、氢氧化铝和氢氧化镁的初始分解温度分别是102、225和330 ℃，即镁铝水滑石具有最低的初始分解温度。这表明镁铝水滑石能够更加快速地分解出惰性物质，及时抑制聚乙烯粉尘爆炸火焰的发展。镁铝水滑石、氢氧化铝和氢氧化镁的总质量损失分别为42.04%、33.18%、29.61%，其中镁铝水滑石的质量损失最多，即镁铝水滑石可以分解出更多的惰性物质阻碍燃烧爆炸反应进行。用Origin软件计算3种粉体的吸热量，得到镁铝水滑石、氢氧化铝和氢氧化镁的吸热量分别为1578、1104和872 J/g，其中镁铝水滑石的吸热量最多，即镁铝水滑石可以更为有效的吸收反应体系的热量，降低反应体系温度，有效抑制反应进行。此外，镁铝水滑石独特的二维层状分子结构可以有效地阻隔热量和可燃气体的传递[41]。热解出的二氧化碳还可以稀释反应体系中的氧气和可燃气体，使得反应更难进行。
为了明确镁铝水滑石粉体对聚乙烯粉尘爆炸过程的影响，分别对抑制比为0（纯聚乙烯）、0.6、1.0、1.5和∞（纯抑爆剂）的混合粉体进行了热重测试，结果如图11所示。从图11中可以看出随着抑制比的增大，混合粉体的热解速率减小，粉体残余率增加。镁铝水滑石的初始热解温度低于聚乙烯粉体的初始热分解温度，即在不同抑制比的混合粉体中，镁铝水滑石优先发生热解，吸收反应热量，降低反应温度，减缓聚乙烯燃烧爆炸发生，从而达到抑爆目的。
2.3.2 化学作用
粉尘爆炸机理复杂，目前学者们对聚乙烯粉尘爆炸机理已进行的研究[42-44]认为，聚乙烯粉尘爆炸反应包括均相反应和非均相反应。均相反应主要是指聚乙烯热解生成的可燃性气体发生的燃烧爆炸反应。聚乙烯热解生成的可燃性气体主要包括乙烯、甲烷、乙烷和气态烷烃等，这些可燃性气体遇氧反应。非均相反应主要是指在聚乙烯粉尘颗粒表面直接发生的燃烧爆炸反应。在高温以及均相反应产生热量的共同作用下，小的聚乙烯粉尘颗粒表面直接发生燃烧爆炸反应。因此，阻断聚乙烯粉尘爆炸均相反应和非均相反应进程即可有效抑制聚乙烯粉尘爆炸。基于链式反应，高温条件下，聚乙烯分子中的碳氢键先发生断裂，形成大分子自由基，链式反应开始。之后大分子自由基产生过氧化自由基和大分子过氧化物，过氧化物分解产生的自由基又与聚合物发生反应，使得整个反应过程不断进行。聚乙烯燃烧爆炸由许多基元反应组成，在这些基元反应中，O•、OH•和H•作为高能自由基，在聚乙烯粉尘爆炸火焰传播中尤为重要[14-15]。因此，阻断这些高能自由基的链式反应是抑制聚乙烯粉尘爆炸的理想途径。
图12为镁铝水滑石吸光度随波数变化规律图。3452 cm−1处的吸收峰对应于层表面/或层间水中的−OH 伸缩振动，而在1562 cm−1处的吸收峰是水中的−OH 弯曲振动[45-46]。1367 cm−1附近出现的强特征吸收峰是由层间
CO2−3 的拉伸振动引起的[47]。400～800 cm−1波段对应的吸收峰归因于镁铝水滑石层板上金属氧晶格振动（Al−O、Mg−O、Mg−O−Al），证明了其为层状结构[48-49]。其中水分子参与了聚乙烯热解产生可燃性气体的链式反应，阻断了聚乙烯均相爆炸反应：
图 12 镁铝水滑石吸光度随波数变化规律图Figure 12. Figure showing the variation of absorbance of Mg-Al hydrotalcite with wavenumberH2O+H•=OH•+H2 (4) H2O+OH•=H•+H2O2 (5) H2O+O•=OH•+OH• (6) 这个抑制效应同氢氧化铝和氢氧化镁热解产生的水的抑制效应相同。不同的是，镁铝水滑石热解产生的CO2能够参与或阻断聚乙烯爆炸链式反应过程。一方面，CO2捕捉H•、OH•自由基后减少了聚乙烯爆炸链式反应中的关键自由基，使链分支反应速率下降；另一方面，当反应系统中CO2的浓度增高时，反应向生成CO的方向移动，这使得聚乙烯的氧化过程不完全，导致燃烧释放出的热量减少，减缓了热量累积速率，一定程度上阻断了聚乙烯粉尘爆炸均相反应和非均相反应的进程，相关反应方程为：
O2+CO=O+CO2 (7) OH•+CO=H•+CO2 (8) HO2•+CO=OH•+CO2 (9) 因此，镁铝水滑石粉体对聚乙烯粉尘爆炸有更好的抑制作用，具体抑爆过程如图13所示。
图 13 镁铝水滑石对聚乙烯粉尘爆炸的抑制机理Figure 13. Mechanism of Mg-Al hydrotalcite suppressing polyethylene dust explosion3. 结 论
本文中基于镁铝水滑石对聚乙烯粉尘爆炸的抑制特性，并与氢氧化镁和氢氧化铝粉体进行对比。结合3种抑爆粉体的理化性质，分析了其抑制聚乙烯粉尘爆炸机理，得到如下结论。
(1)镁铝水滑石对聚乙烯粉尘的爆炸超压和最低着火温度均有显著的抑制效果，当抑制比为1时可使聚乙烯粉尘的最大爆炸压力和最大爆炸压力上升速率分别下降30.13%、68.46%，最低着火温度提高63.04%；当抑制比为2时即可实现对聚乙烯爆炸的完全抑制。
(2)相同抑制比下，镁铝水滑石具有显著优于氢氧化镁和氢氧化铝的抑制聚乙烯爆炸的性能，且抑爆分解产物主要为MgO、Al2O3、H2O、CO2等无毒害产物，是一种性能优异、环保高效的聚乙烯爆炸抑制剂。
(3)镁铝水滑石粉体对聚乙烯粉尘爆炸的抑制机理是物理作用和化学作用耦合效应。物理作用方面，镁铝水滑石较低的初始分解温度、较大的质量损失和较高的吸热量展现了优势，且其独特的二维层状结构有利于阻隔热量和可燃气体的传递；化学作用方面，镁铝水滑石能够热解出水以及二氧化碳气体，这些气体可以参与聚乙烯爆炸链式反应，阻断聚乙烯爆炸反应进程，使得反应不完全，从而达到抑爆目的。
-
图 4 试件裂纹扩展路径
Figure 4. Crack propagation trajectories of black sandstone ISCSC specimens
图 5 试件1中裂纹起裂、扩展、止裂阶段的应力波状态
Figure 5. Stress wave states during crack initiation, propagation and crack arrest in the specimen 1
图 11 裂纹扩展速度分形修正前后的比较
Figure 11. Comparison of crack propagation speeds before and after fractal correction
图 12 有限单元法模型示意图及位移外推法
Figure 12. Finite element method model and displacement extrapolation method
图 14 裂纹动态应力强度因子随裂纹扩展位移的变化
Figure 14. Crack dynamic stress intensity factors varied with displacement of propagation crack
图 15 修正动态扩展韧度随vc/cR的变化
Figure 15. Variation of corrected crack propagation toughness with vc/cR
表 1 分形修正前后的裂纹扩展速度和动态扩展韧度
Table 1. Crack propagation speeds and dynamic crack propagation toughnesses before and after fractal correction
样品 v0/(m·s−1) vc/(m·s−1) ev/% KdI/(MPa·m1/2) KdI,c/(MPa·m1/2) eK/% 1 723.56 781.25 7.97 3.956 3.760 4.94 476.19 518.27 8.84 4.355 4.236 2.72 400.00 502.40 25.60 4.412 4.137 6.22 357.14 388.54 8.79 4.681 4.597 1.81 400.00 423.04 5.76 4.420 4.359 1.38 294.12 310.91 5.71 4.828 4.783 0.91 238.10 296.06 24.35 4.827 4.681 3.03 434.78 504.68 16.08 4.613 4.412 4.37 555.56 638.65 14.96 4.399 4.140 5.89 357.14 394.39 10.43 4.681 4.581 2.15 312.50 376.07 20.34 4.296 4.144 3.54 263.16 317.04 20.47 4.462 4.334 2.87 322.58 335.76 4.09 4.473 4.440 0.73 212.77 246.41 15.81 4.403 4.327 1.71 128.21 158.57 23.68 4.589 4.523 1.45 400.00 429.20 7.30 4.412 4.335 1.75 200.00 229.67 14.83 4.610 4.541 1.50 555.56 594.27 6.97 4.399 4.279 2.72 384.62 486.32 26.44 4.452 4.181 6.09 344.83 434.55 26.02 4.267 4.046 5.17 151.52 164.63 8.66 4.538 4.510 0.63 50.25 53.93 7.33 5.258 5.242 0.30 2 625.00 653.59 4.57 4.230 4.138 2.16 588.24 668.14 13.58 4.298 4.046 5.86 370.37 428.20 15.61 4.794 4.532 5.48 588.24 692.50 17.72 3.907 3.606 7.68 400.00 471.78 17.95 4.132 3.953 4.34 285.71 317.43 11.10 4.521 4.443 1.72 555.56 635.54 14.40 4.399 4.150 5.66 250.00 283.37 13.35 4.607 4.527 1.75 76.92 100.54 30.71 4.917 4.634 5.76 2 370.37 417.47 12.72 4.444 4.322 2.76 123.46 153.54 24.37 4.624 4.558 1.43 370.37 429.51 15.97 4.312 4.162 3.47 625.00 678.86 8.62 4.183 4.012 4.10 714.29 792.18 10.91 4.087 3.816 6.62 232.56 310.49 33.51 4.492 4.309 4.07 625.00 700.12 12.02 3.910 3.685 5.74 588.24 635.83 8.09 3.899 3.764 3.47 256.41 290.27 13.21 4.752 4.667 1.79 555.56 649.17 16.85 4.066 3.795 6.64 322.58 353.43 9.56 4.321 4.246 1.73 263.16 293.77 11.63 4.620 4.545 1.63 217.39 272.11 25.17 4.393 4.269 2.81 333.33 395.52 18.65 4.319 4.167 3.53 156.25 170.84 9.34 4.740 4.706 0.71 312.50 342.41 9.57 4.345 4.273 1.66 86.96 100.09 38.11 4.659 4.574 1.82 65.79 80.47 37.51 5.637 5.584 0.93 8.73 9.60 9.94 5.346 5.344 0.05 4.98 5.40 8.46 4.762 4.761 0.02 
下载: 导出CSV
-
[1] GÓMEZ F J, ELICES M, BERTO F, et al. Local strain energy to assess the static failure of U-notches in plates under mixed mode loading [J]. International Journal of Fracture, 2007, 145(1): 29–45. DOI: 10.1007/s10704-007-9104-3. [2] COOK N G W. Natural joints in rock: mechanical, hydraulic and seismic behaviour and properties under normal stress [J]. International Journal of Rock Mechanics and Mining Sciences & Geomechanics Abstracts, 1992, 29(3): 198–223. DOI: 10.1016/0148-9062(92)93656-5. [3] SOH A K, YANG C H. Numerical modeling of interactions between a macro-crack and a cluster of micro-defects [J]. Engineering Fracture Mechanics, 2004, 71(2): 193–217. DOI: 10.1016/S0013-7944(03)00097-3. [4] MISHURIS G, MOVCHAN A, MOVCHAN N, et al. Interaction of an interfacial crack with linear small defects under out-of-plane shear loading [J]. Computational Materials Science, 2012, 52(1): 226–230. DOI: 10.1016/j.commatsci.2011.01.023. [5] 董玉清, 朱哲明, 王蒙, 等. 中低速冲击载荷作用下SCT岩石试样Ⅰ型裂纹的动态扩展行为 [J]. 中南大学学报(自然科学版), 2018, 49(11): 2821–2830. DOI: 10.11817/j.issn.1672-7207.2018.11.024.DONG Y Q, ZHU Z M, WANG M, et al. Mode Ⅰ crack dynamic propagation behavior of SCT specimens under medium-low speed impact load [J]. Journal of Central South University (Science and Technology), 2018, 49(11): 2821–2830. DOI: 10.11817/j.issn.1672-7207.2018.11.024. [6] 周磊, 朱哲明, 董玉清, 等. 冲击加载下巷道内裂纹的扩展特性及破坏行为 [J]. 爆炸与冲击, 2018, 38(4): 785–794. DOI: 10.11883/bzycj-2016-0383.ZHOU L, ZHU Z M, DONG Y Q, et al. Propagation characteristics and failure behaviors of crack in tunnel under impact loads [J]. Explosion and Shock Waves, 2018, 38(4): 785–794. DOI: 10.11883/bzycj-2016-0383. [7] WANG F, WANG M, NEZHAD M M, et al. Rock dynamic crack propagation under different loading rates using improved single cleavage semi-circle specimen [J]. Applied Sciences, 2019, 9(22): 4944. DOI: 10.3390/APP9224944. [8] THEOCARIS P S, MILIOS J. Crack arrest modes of a transverse crack going through a longitudinal crack or a hole [J]. Journal of Engineering Materials and Technology, 1981, 103(2): 177–182. DOI: 10.1115/1.3224991. [9] MILIOS J, SPATHIS G. Dynamic interaction of a propagating crack with a hole boundary [J]. Acta Mechanica, 1988, 72(3/4): 283–295. DOI: 10.1007/BF01178314. [10] MURDANI A, MAKABE C, SAIMOTO A, et al. A crack-growth arresting technique in aluminum alloy [J]. Engineering Failure Analysis, 2008, 15(4): 302–310. DOI: 10.1016/j.engfailanal.2007.02.005. [11] AYATOLLAHI M R, RAZAVI S M J, YAHYA M Y. Mixed mode fatigue crack initiation and growth in a CT specimen repaired by stop hole technique [J]. Engineering Fracture Mechanics, 2015, 145: 115–127. DOI: 10.1016/j.engfracmech.2015.03.027. [12] CHEN N Z. A stop-hole method for marine and offshore structures [J]. International Journal of Fatigue, 2016, 88: 49–57. DOI: 10.1016/j.ijfatigue.2016.03.010. [13] WANG Y B, YANG R S, ZHAO G F. Influence of empty hole on crack running in PMMA plate under dynamic loading [J]. Polymer Testing, 2017, 58: 70–85. DOI: 10.1016/j.polymertesting.2016.11.020. [14] WANG M, WANG F, ZHU Z M, et al. Modelling of crack propagation in rocks under SHPB impacts using a damage method [J]. Fatigue and Fracture of Engineering Materials and Structures, 2019, 42(8): 1699–1710. DOI: 10.1111/ffe.13012. [15] 王飞, 王蒙, 朱哲明, 等. 冲击荷载下岩石裂纹动态扩展全过程演化规律研究 [J]. 岩石力学与工程学报, 2019, 38(6): 1139–1148. DOI: 10.13722/j.cnki.jrme.2018.1172.WANG F, WANG M, ZHU Z M, et al. Study on evolution law of rock crack dynamic propagation in complete process under impact loading [J]. Chinese Journal of Rock Mechanics and Engineering, 2019, 38(6): 1139–1148. DOI: 10.13722/j.cnki.jrme.2018.1172. [16] FUNATSU T, KURUPPU M, MATSUI K. Effects of temperature and confining pressure on mixed-mode (Ⅰ-Ⅱ) and mode Ⅱ fracture toughness of Kimachi sandstone [J]. International Journal of Rock Mechanics and Mining Sciences, 2014, 67: 1–8. DOI: 10.1016/j.ijrmms.2013.12.009. [17] 周磊, 朱哲明, 董玉清, 等. 动态加载率对巷道内裂纹扩展速度及动态起裂韧度的影响 [J]. 振动与冲击, 2019, 38(4): 129–136. DOI: 10.13465/j.cnki.jvs.2019.04.021.ZHOU L, ZHU Z M, DONG Y Q, et al. Effect of dynamic loading rate on crack propagation velocity and dynamic fracture toughness in tunnels [J]. Journal of Vibration and Shock, 2019, 38(4): 129–136. DOI: 10.13465/j.cnki.jvs.2019.04.021. [18] ZHANG Q B, ZHAO J. Determination of mechanical properties and full-field strain measurements of rock material under dynamic loads [J]. International Journal of Rock Mechanics and Mining Sciences, 2013, 60: 423–439. DOI: 10.1016/j.ijrmms.2013.01.005. [19] JIANG F C, VECCHIO K S. Hopkinson bar loaded fracture experimental technique: a critical review of dynamic fracture toughness tests [J]. Applied Mechanics Reviews, 2009, 62(6): 060802. DOI: 10.1115/1.3124647. [20] WANG Q Z, FENG F, NI M, et al. Measurement of mode Ⅰ and mode Ⅱ rock dynamic fracture toughness with cracked straight through flattened Brazilian disc impacted by split Hopkinson pressure bar [J]. Engineering Fracture Mechanics, 2011, 78(12): 2455–2469. DOI: 10.1016/j.engfracmech.2011.06.004. [21] AVACHAT S, ZHOU M. High-speed digital imaging and computational modeling of dynamic failure in composite structures subjected to underwater impulsive loads [J]. International Journal of Impact Engineering, 2015, 77: 147–165. DOI: 10.1016/j.ijimpeng.2014.11.008. [22] LEE D, TIPPUR H, BOGERT P. Dynamic fracture of graphite/epoxy composites stiffened by buffer strips: an experimental study [J]. Composite Structures, 2012, 94(12): 3538–3545. DOI: 10.1016/j.compstruct.2012.05.032. [23] MANDELBROT B B. The fractal geometry of nature [M]. New York, USA: W. H. Freeman and Company, 1982. [24] THEOCARIS P S, SAKELLARIOU M. A correction model for the incompatible deformations of the shear internal crack [J]. Engineering Fracture Mechanics, 1991, 38(4/5): 231–240. DOI: 10.1016/0013-7944(91)90001-H. [25] NAGAHAMA H. Fractal scalings of rock fragmentation [J]. Earth Science Frontiers, 2000, 7(1): 169–177. DOI: 10.3321/j.issn:1005-2321.2000.01.016. [26] 谢和平. 动态裂纹扩展中的分形效应 [J]. 力学学报, 1995, 27(1): 18–27. DOI: 10.6052/0459-1879-1995-1-1995-401.XIE H P. Fractal effects of dynamic crack propagation [J]. Acta Mechanica Sinica, 1995, 27(1): 18–27. DOI: 10.6052/0459-1879-1995-1-1995-401. [27] 谢和平. 脆性材料裂纹扩展的分形运动学 [J]. 力学学报, 1994, 26(6): 757–762. DOI: 10.6052/0459-1879-1994-6-1995-606.XIE H P. Fractal kinematics of crack propagation in brittle materials [J]. Acta Mechanica Sinica, 1994, 26(6): 757–762. DOI: 10.6052/0459-1879-1994-6-1995-606. [28] 谢和平. 分形-岩石力学导论 [M]. 北京: 科学出版社, 1996: 168−261. [29] 程靳, 赵树山. 断裂力学 [M]. 北京: 科学出版社, 2006: 9−55. [30] FREUND L B. Dynamic fracture mechanics [M]. Cambridge, UK: Cambridge University Press, 1990. [31] ROSE L R F. Recent theoretical and experimental results on fast brittle fracture [J]. International Journal of Fracture, 1976, 12(6): 799–813. DOI: 10.1007/BF00034620. [32] RAVI-CHANDAR K, KNAUSS W G. An experimental investigation into dynamic fracture: I. crack initiation and arrest [J]. International Journal of Fracture, 1984, 25: 247–262.10. DOI: 10.1007/BF00963460. 期刊类型引用(6)
1. 高建村，王乐，胡守涛，孙谞，周尚勇，王涛. 不同磁性金属丝对丙烷爆炸反应抑制机理研究. 中国安全生产科学技术. 2020(07): 125-130 . 
百度学术2. 王连聪，梁运涛，罗海珠. 我国矿井热动力灾害理论研究进展与展望. 煤炭科学技术. 2018(07): 1-9 . 百度学术3. 路长，刘洋，于子凯，潘荣锟，刘磊，滕飞. 四氟乙烷对甲烷/空气爆炸特性的影响. 化工进展. 2017(10): 3596-3603 . 百度学术4. 徐永亮，王兰云，余明高，万少杰，梁栋林. 感应式荷电细水雾对瓦斯爆炸传播速度的影响. 中南大学学报(自然科学版). 2016(08): 2884-2890 . 百度学术5. 余明高，梁栋林，徐永亮，郑凯，纪文涛. 荷电细水雾抑制瓦斯爆炸实验研究. 煤炭学报. 2014(11): 2232-2238 . 百度学术6. 丁小勇，谭迎新，李媛，秦涧，许航. 管道倾斜角对瓦斯爆炸影响的实验研究. 消防科学与技术. 2012(12): 1269-1271 . 百度学术其他类型引用(14)
-
下载:
百度学术
计量
- 文章访问数: 600
- HTML全文浏览量: 194
- PDF下载量: 101
- 被引次数: 20