Simulation analysis on impact resistance of aluminum foam sandwich structures using peridynamics
-
摘要: 针对某光学舱所采用的泡沫铝夹层防护结构在破片冲击下的抗冲击性能问题,采用Monte-Carlo方法创建了泡沫铝结构的二维细观模型,在常规态型近场动力学理论中引入了Mises屈服准则和线性各向同性强化模型,建立了近场动力学塑性本构的数值计算框架。基于近场动力学计算程序模拟了低速冲击作用下泡沫铝夹层结构的塑性变形以及有机玻璃背板的裂纹扩展形态,分析了泡沫铝芯材孔隙率对该夹层结构抗冲击性能和损伤模式的影响规律。结果表明:泡沫铝夹层结构良好的塑性变形能力是其发挥缓冲与防护作用的主要因素,并且在一定范围内,泡沫铝芯材孔隙率越高,则夹层结构具有更好的抗冲击性能;当泡沫铝孔隙率从0.4提升到0.7时,泡沫铝对冲击物的动能吸收率从90%提高到99%;模拟结果与实验结果具有较好的一致性,验证了模拟结果的准确性和分析结论的有效性。通过数值模拟,预测了有机玻璃背板的裂纹扩展形态,发现提高泡沫铝的孔隙率能获得更好的防护效果。Abstract: Under impact, aluminum foam undergoes significant plastic deformation, and the kinetic energy of the impactor is dissipated in the process, thereby protecting the structure from damage. The failure modes of aluminum foam sandwich structures under impact are complex, involving plastic deformation, panel failure, and cracking of the bonding interface. Traditional numerical simulation methods are difficult to solve these discontinuous problems. Peridynamics is a non-local numerical method that describes the mechanical behavior of materials by solving spatial integral equations. It has unique advantages in solving crack propagation, material failure, progressive damage of composite materials, and multi-scale problems. Although the basic bond-based peridynamic theory cannot describe plasticity, the ordinary state-based peridynamic method decouples distortion and dilation and can easily simulate the plastic deformation of materials. Therefore, based on ordinary state-based peridynamics, the Mises yield criterion and the linear isotropic hardening model were introduced to study the factors affecting the impact resistance of aluminum foam sandwich structures. Two-dimensional mesoscopic models of aluminum foam sandwich structure were established by the Monte-Carlo method and impact was simulated using the peridynamic method. The influence of the porosity of aluminum foam on the impact resistance and damage mode of the sandwich structure was analyzed. The results show that the good plastic deformation ability of aluminum foam sandwich structure is the main factor for its buffering and protection, and within a certain range, the higher the porosity of aluminum foam core, the better impact resistance of the sandwich structure. When the porosity of aluminum foam increases from 0.4 to 0.7, the kinetic energy absorption rate of aluminum foam to the impactor increases from 90% to 99%. The simulation results are in good agreement with the experimental results, which verifies the accuracy of the simulation results and the effectiveness of the analysis conclusions. The numerical simulation predicts the crack propagation morphology of the plexiglass backplate, and the results show that improving the porosity of aluminum foam can obtain a better protection effect.
-
纤维增强复合材料具有比强度和比模量高、材料性能可设计性、密度低、热电绝缘性良好、耐腐蚀性能好等优点,被广泛应用于航空航天、国防军工、汽车工业、建筑与基础设施、管道与储罐、体育等领域,尤其是在防护领域应用更广泛,因此,研究复合材料的抗冲击性能具有重要意义[1-4]。
研究人员已通过实验和数值模拟对纤维增强复合材料的弹道冲击与侵彻进行了研究。在弹道极限领域,Jenq等[5-6]利用能量守恒方法提出了平面编织复合材料E-glass/Epoxy层合板在球头弹撞击下的弹道极限;López-Puente等[7]研究了 CFRP(carbon fiber reinforced plastic) 层合板在球形弹体冲击作用下的弹道极限和损伤面积,结果表明,复合材料层合板损伤面积在弹道极限附近最大;杜忠华等[8]在动量和能量守恒的基础上,根据本构关系和失效准则给出了预测复合材料层合板的弹道极限速度的经验公式。在损伤区域的划分方面,王元博[9]根据平头弹体高速冲击的特点,将破坏区域沿靶板厚度方向划分为压入破坏和弯曲破坏。关于能量吸收,江琦[10]研究认为,子弹垂直冲击复合材料板时,能量的吸收机理主要有弹头的摩擦和变形、纤维断裂和拔出、基底开裂或屈服破坏以及纤维和树脂发生界面脱粘。
在数值模拟方面,谭焕成等[11]建立了三维四向编织复合材料的宏观有限元模型,发现钢珠剩余速度的模拟结果与实验结果的误差在5%以内,宏观冲击损伤形态与实验结果吻合较好。王云聪等[12]赋予复合材料正交各向异性材料模型和侵蚀接触属性,模拟出了弹靶作用的三个阶段:初期,上层纤维和基底发生剪切充塞破坏;中期,因应力波在各层之间不断发生反射和透射,导致背板分层;末期,背板分层拉断且弯曲变形。Jagtap 等[13]建立了碳纤维/环氧树脂复合材料层合板的三维有限元模型,以最大应力失效准则判断复合材料的基底开裂、分层等损伤形态,研究了不同网格尺寸对层合板冲击损伤时的接触力和变形的影响。综上所述,复合材料的侵彻防护性能会受到组分、纤维结构、制造工艺、加载条件等的影响。然而,对于以连续纤维增强高孔隙复合材料为代表的轻质防热复合材料的弹道侵彻领域的研究鲜有报道。
连续纤维增强高孔隙复合材料是一种在酚醛树脂中添加酚醛、玻璃、陶瓷粉体为基底,石英等功能纤维为增强相[14]的复合材料,在航空航天和武器装备中的高温和冲击下的防护领域被广泛应用,使得这类材料在实际应用中会面临高速碰撞和侵彻的情况。对这类防热复合材料的研究多基于其隔热与耐烧蚀性能,对力学性能特别是动态力学性能与冲击防护性能的研究较少。因此,从实验、理论和数值模拟等方面开展其侵彻和防护性能研究,获取相关性能参数和特性规律,可为这类材料在侵彻防护领域的应用提供更好的支撑。
本文中,运用二级轻气炮发射不同初速度的球形弹丸,对厚20 mm的连续纤维增强高孔隙复合材料靶板进行正侵彻,获取不同初速度下球形弹丸侵彻靶板后的剩余速度,得到靶板的弹道极限,计算比吸能并与其他防护材料作对比,观察不同初速度下靶板的损伤形态。在此基础上,对球形弹丸侵彻靶板进行数值仿真,选取合适的本构模型进行二次开发,对侵彻过程中的应力分布、损伤分布、弹丸接触力、能量吸收与耗散情况等进行分析,并探究不同侵彻速度下靶板的损伤破坏机理,为连续纤维增强高孔隙复合材料在防护领域的应用提供依据。
1. 弹道侵彻实验
1.1 实验概况
实验所用的连续纤维增强高孔隙复合材料是一种二维编织复合材料。每一发弹道侵彻实验所用的靶板边长均为10.0 cm,厚度均为2.0 cm,如图1所示。侵彻弹体采用Q235钢制作的球形弹丸,直径均为4.5 mm,质量约0.37 g。
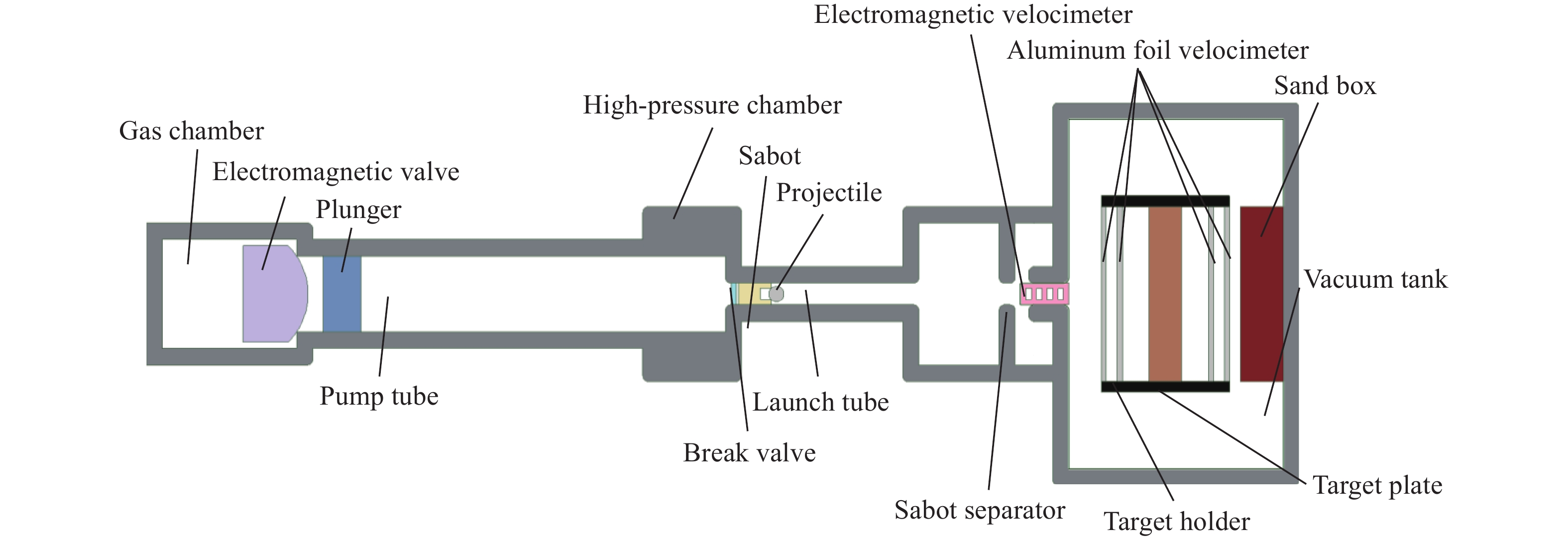
实验在二级轻气炮上进行,二级轻气炮的构造示意图如图2所示。
二级轻气炮采用高压氮气与高压氢气驱动。发射时首先在气室内充入高压氮气,并在活塞右侧的泵管中充入氢气,然后打开电磁阀,让高压氮气流入泵管,推动活塞向右运动压缩泵管的氢气。当氢气压力超过一定水平后,发射管尾部的膜片被冲破;高压氢气推动弹托与弹丸在炮管中前进。在弹托前进过程中活塞继续向前运动,使高压氢气的压强不发生明显的下降,保证弹丸高速飞出炮口,并通过调整弹丸与炮口的距离来改变弹体离开发射管速度。子弹与弹托在弹托分离器处分离,弹托被阻挡在测速装置之前,以免干扰测速与侵彻。为了避免靶板四周由于弯曲应力集中导致的边界失效,采用胶带缠绕的方法将靶板固定在靶架上,以约束靶板的刚体转动和平动自由度。
1.2 实验结果分析
采用Q235钢制球形弹丸正侵彻靶板,进行了多发实验,结果如表1所示,可以看出,初速度为583.7 m/s的弹丸未穿透靶板,其余初速度下的弹丸都穿透了靶板。弹丸动能随初速度的增加而增加,二者基本呈线性关系。
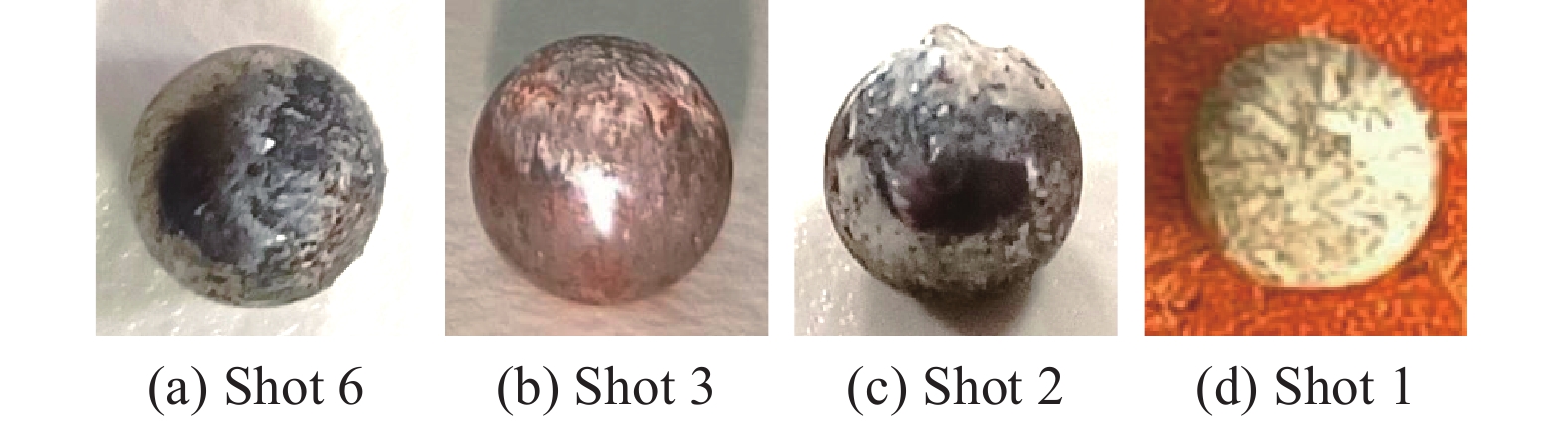
表 1 侵彻实验结果Table 1. Experimental results of penetration实验 初速度/(m∙s−1) 末速度/(m∙s−1) 弹丸动能/J 损伤类型 1 1640.0 1227.5 218.83 切孔型 2 1450.9 1040.1 189.31 切孔型 3 1082.0 715.5 121.87 背面炸裂型 4 1046.2 651.0 124.09 背面炸裂型 5 583.7 未穿透 63.03 未穿透 6 775.0 263.5 98.27 背面裂缝型 随着初速度由低到高增大,侵彻过程给弹丸带来的变形会越来越明显。第6发实验的回收弹丸如图3(a)所示,由于初速度较低,弹丸几乎不发生塑性变形,只是表面变得粗糙,弹丸与靶板的接触面覆盖着少量靶板材料;随着初速度的升高,如图3(b)所示,第3发实验的弹丸发生轻微的塑性变形,表现为轻微的失圆,弹丸与靶板的接触面被较多的靶板材料覆盖;初速度更高时,第1~2发实验的弹丸发生较明显的塑性变形,表现为有一个明显的尖端与尖端周围材料的退缩,弹丸与靶板的接触面覆盖着更多的靶板材料,如图3(c)~(d)所示。上述结果说明侵彻过程中不能将弹丸视为刚体,应考虑弹丸的弹性与塑性变形。
1.2.1 损伤形态分析
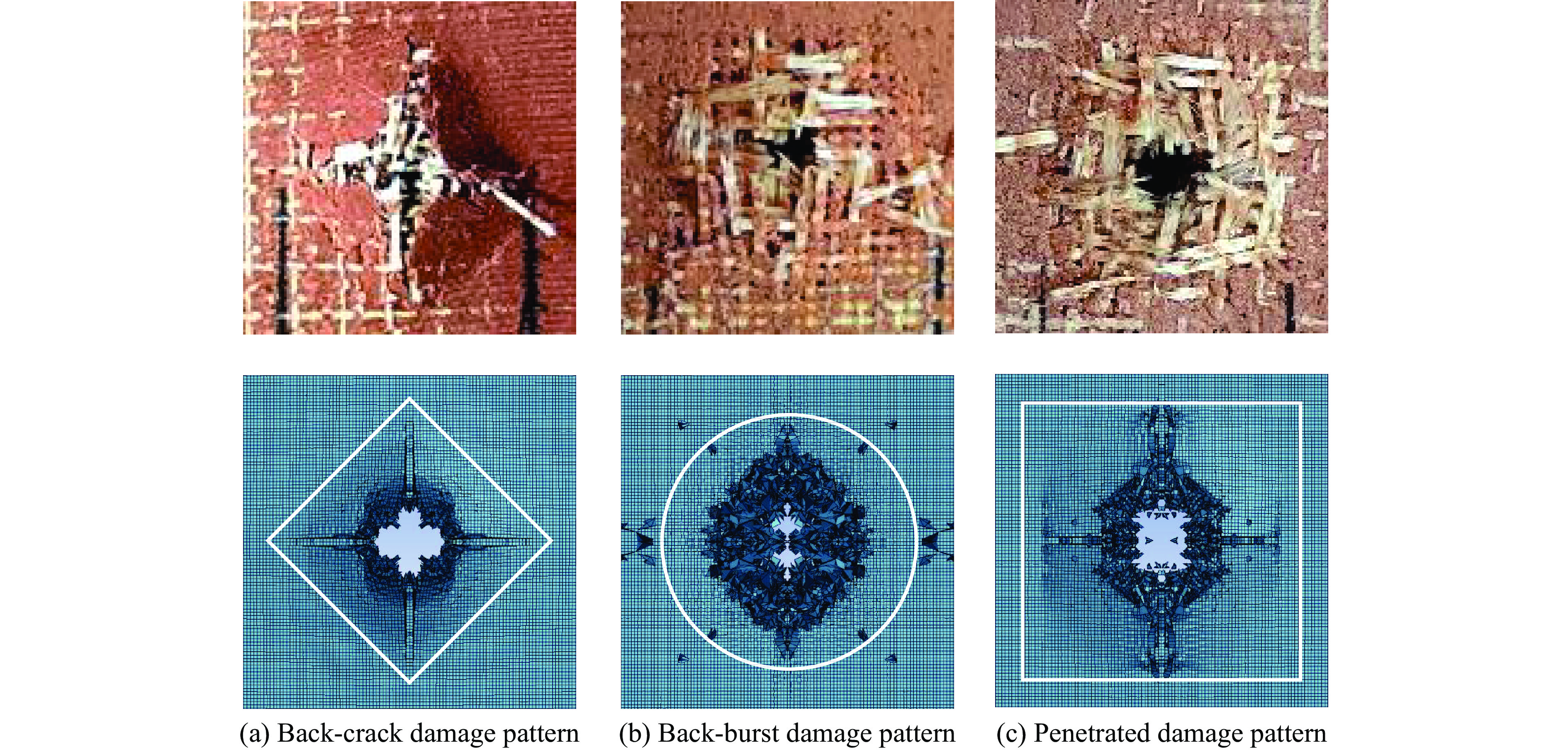
损伤形态是复合材料侵彻冲击下破坏模式和损伤机理的外在表现,也是有限元模型中损伤分析的基础。不同的损伤形态体现了不同的失效与破坏机理和吸能特性,所以根据冲击造成的损伤形态特征划分冲击损伤类型。如表2和图4所示,由于在不同的初速度下,靶板迎弹面的损伤形态差别不明显,体现为纤维断裂、纤维基底脱粘和基底在弹丸侵彻开孔瞬间高压下的粉碎破坏与侵彻弹孔的形成,迎弹面损伤范围直径与初速度无明显关系,通过观察弹丸射出孔周围的损伤形态并参考文献[16],将侵彻损伤的类型分为背面裂缝型、背面炸裂型和切孔型三种。
表 2 靶板迎弹面、背弹面损伤范围Table 2. Damage range of the impact surface and back surface of the target plate实验 初速度/(m∙s−1) 迎弹面损伤范围
直径/mm背弹面损伤范围
直径/mm1 1640.0 6.17 14.80 2 1450.9 8.63 14.78 3 1082.0 6.19 18.83 4 1046.2 5.19 16.07 5 583.7 5.23 未穿透 6 775.0 5.52 17.37 背面裂缝型损伤。损伤形态如图5所示,可以看到,纤维拔出、纤维断裂和纤维基底脱粘主要集中于以弹孔为中心的十字形范围。靶板的背弹面沿着弹丸的运动方向凸出,产生较大的残留变形,而且损伤区域内部有大范围分层。
背面炸裂型损伤。损伤形态如图6所示,在弹孔附近呈现纤维的弯折与靶板材料的折断飞出,背弹面上的凸起程度减小。较高的弹丸速度促进了基底的碎裂,并导致纤维与基底之间出现明显的脱粘,弹孔周边出现了较大范围的分层。背弹面的损伤范围明显大于迎弹面的损伤范围,介于其他两种损伤类型之间。
切孔型损伤。损伤形态如图7所示,当弹丸的速度更高时,侵彻过程中靶板局部损伤的特征更加突出,背弹面的凸起程度比其他两种损伤类型更低。更高的弹丸速度导致基底碎裂得更严重,并加剧了纤维基底的脱粘,使得纤维表面几乎未被基底覆盖。弹丸从靶板中穿透过去后,在靶板上留下一个与弹丸直径几乎相等的弹孔,弹孔未被纤维覆盖,损伤区域小于背部炸裂性损伤与背面裂缝型损伤。
1.2.2 弹道极限
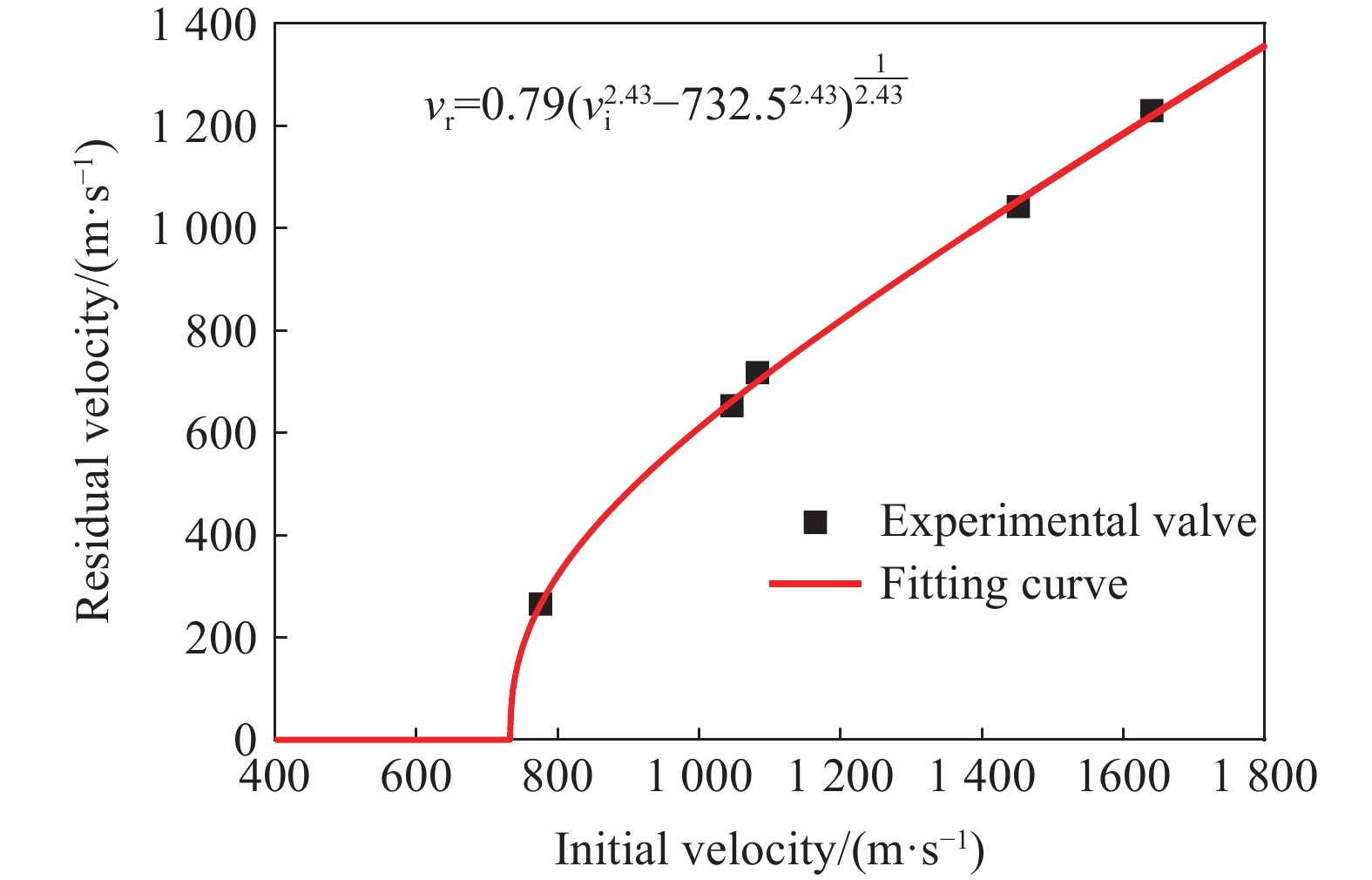
采用Lambert-Jonas弹道极限方程[17]可以较好拟合弹道冲击的剩余速度与初始速度的关系:
vr=a(vpi−vpbl)1p (1) 式中:
vi 为初速度,vr 为末速度,vbl 、a 和p 为拟合参数。式(1)适用于入射速度大于弹道极限的情况。如图8所示,通过拟合曲线,可以得到实验所用的复合材料靶板在正侵彻下的弹道极限约为732.5 m/s。1.2.3 比吸能与侵彻防护能力
弹丸在侵彻过程中的动能损耗(
ΔEk )是通过其初速度(vi )和末速度(vr )来确定的:ΔEk=Ep+Eair=12mp(v2i−v2r) (2) 式中:
ΔEk 包含穿透靶板的能量Ep 和空气阻力耗能Eair 。本文中忽略空气阻力,即Ek=Ep 。按照下式定义比吸能[18-19]:E∗p=Epms=EpρAsh (3) 式中:
ρ 为靶板密度,h 为靶板厚度,As=πr2 为弹丸撞击面的面积。表3给出了不同初速度下靶板的比吸能。表 3 不同初速度下的比吸能Table 3. Specific absorption energy under different initial velocities实验 初速度/
(m∙s−1)末速度/
(m∙s−1)弹丸动能
变化/J比吸能/
(MJ∙kg−1)1 1640.0 1227.5 221.52 0.76 2 1450.9 1040.1 191.64 0.66 3 1082.0 715.5 123.37 0.43 4 1045.8 651.0 125.45 0.43 5 583.7 未穿透 63.81 0.37 6 775.0 263.5 99.48 0.34 将本文中的连续纤维增强高孔隙复合材料与其他金属、非金属、复合材料的比吸能(
E∗p )在图9中进行对比[20-26] ,可以看出,连续纤维增强高孔隙复合材料的比吸能E∗p 与其他材料一样,随初速度的增大而增大,特别是连续纤维增强高孔隙复合材料(类似钢、铝等材料)存在初速度临界点,使得比吸能E∗p 在超过该临界点后急剧增加。结合表3对比发现:在大约1000 m/s的初速度下,连续纤维增强高孔隙复合材料的E∗p 比三维正交机织玻璃纤维复合材料(three-dimensionalorthogonal woven glass fiber composites,3D GFRP)的低2.5%;在大约750 m/s的初速度下,连续纤维增强高孔隙复合材料的E∗p 相当于铝的58%,不锈钢的70%,凯夫拉的76%,平纹玻璃纤维/环氧酚醛层合板(plain weaveglass fabric/epoxy-phenolic laminates,2D GFRP)的81%,碳纤维复合材料(carbon fiber reinforced plastic,CFRP)的65%,比3D GFRP大15%;在大约600 m/s的初速度下,连续纤维增强高孔隙复合材料的E∗p 比聚甲基丙烯酸甲酯(polymethyl methacrylate,PMMA)大68%,比不锈钢大39%,是凯夫拉的98%,比2D GFRP大7%,比3D GFRP大40%,比CFRP小6%。结果表明,连续纤维增强高孔隙复合材料在初速度750 m/s以上的防护能力不如钢、铝等材料,但对初速度600 m/s及以下的低速侵彻的防护性能优于钢、铝、凯夫拉、玻璃纤维复合材料,因而在低密度轻质防护材料领域有较好的应用前景,可以在初速度600 m/s及以下的低速侵彻条件下代替钢、铝等传统金属材料和碳纤维、玻璃纤维、凯夫拉等传统复合材料。2. 弹道侵彻数值模拟
2.1 有限元计算模型
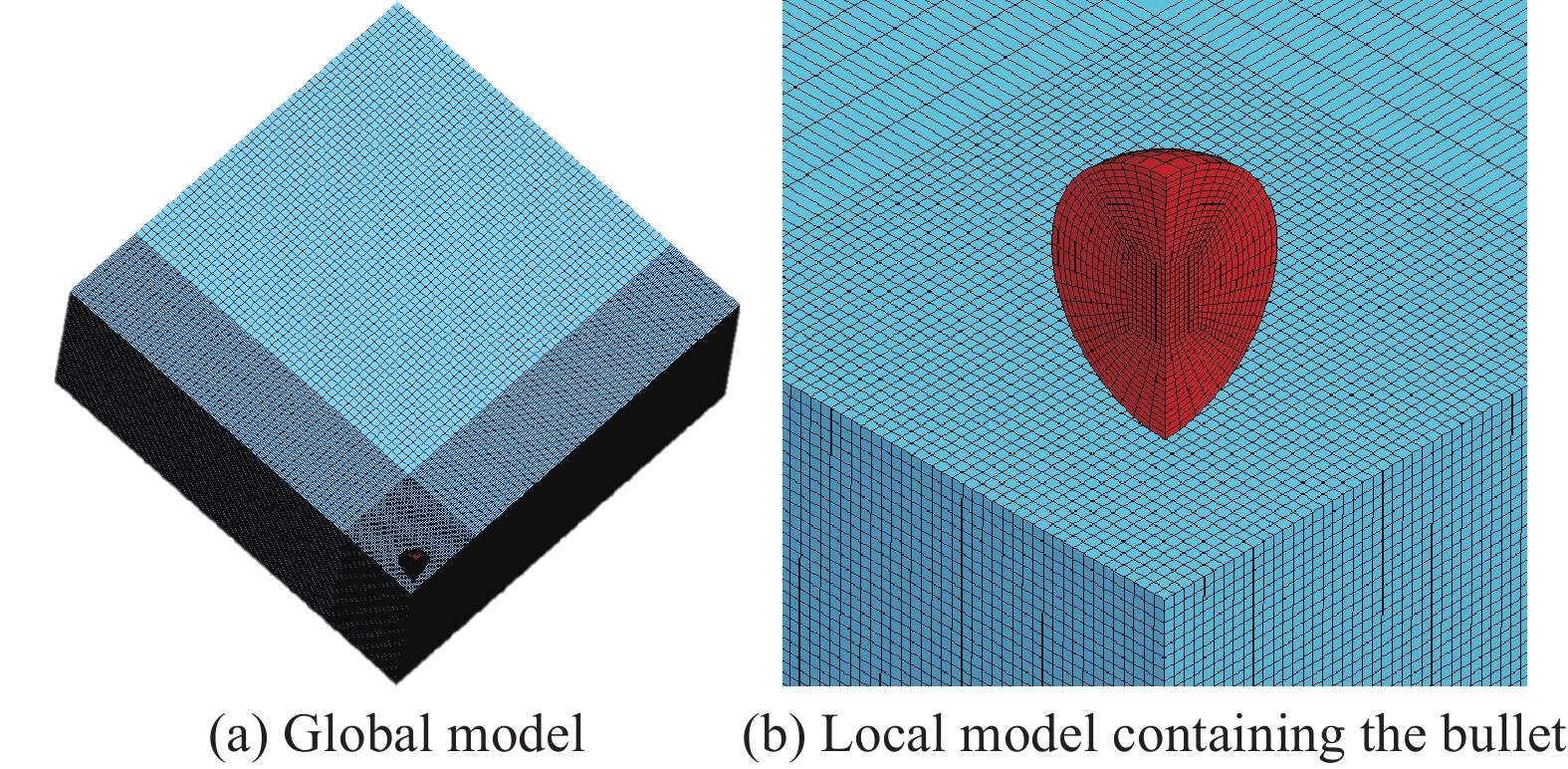
对连续纤维增强高孔隙复合材料在宏观尺度上进行研究,将弹丸和靶板视为连续均匀且无残余应力的材料,侵彻过程中忽略冲击温升、摩擦生热、空气阻力和重力。利用弹丸和靶板本构模型的对称性建立四分之一模型。靶板边缘采用固定约束,对称处采用对称边界约束。建立的有限元计算模型如图10所示,靶板的尺寸为50 mm×50 mm×20 mm。
采用8节点三维实体单元划分网格,模型共划分为
1004802 个单元。又由于侵彻问题的高压和大变形区域主要在弹丸与靶板接触位置附近,为了提高计算速度和保证计算精度,将弹丸和靶板接触区域及周边网格的尺寸设为0.2 mm,其余部分网格的尺寸设为1.0 mm,以保证应力的分布尽量平滑,减轻网格尺度效应对结果的影响。2.2 材料模型及参数
对复合材料靶板,选用正交各向异性线弹性与基于三维 Hashin准则的失效判据作为本构模型[27-29]。由于基于应力的损伤判据可能导致不连续问题[30],所以将应力修改为应变。改进后的失效准则包含以下7种损伤判据。
面内经向纤维拉伸:
F1=F1,t=(E1ε11Xt)2+(G13ε13S13)2ε11≥0 (4) 面内经向纤维压缩:
F2=F1,c=(E1ε11Xc)2ε11<0 (5) 面内纬向纤维拉伸:
F3=F2,t=(E2ε22Yt)2+(G23ε23S23)2ε22≥0 (6) 面内纬向纤维压缩:
F4=F2,c=(E2ε22Yc)2ε22<0 (7) 面内剪切:
F5=F12=(2G12ε12S12)2 (8) 面外压缩:
F6=F3,c=(E3ε33Zc)2ε33<0 (9) 面外拉伸分层:
F7=F3,t=(E3ε33Zt)2+(G13ε13S13)2+(G23ε23S23)2ε33≥0 (10) 式中:
F 为损伤判据因子,E1 为面内经向弹性模量,E2 为面内纬向弹性模量,E3 为面外弹性模量,G12 为面内剪切模量,G13 、G23 为面外剪切模量,Xt 为面内经向拉伸强度,Yt 为面内纬向拉伸强度,Xc 为面内经向压缩强度、Yc 为面内纬向压缩强度,Zt 为面外拉伸强度,Zc 为面外压缩强度,S12 为面内剪切强度,S23 、S13 为面外剪切强度,下角标1、2、3指面内经向、纬向与面外法向。F>1 后,对靶板的拉伸与剪切模量进行如下式所示的指数型损伤退化,退化到一定程度后单元失效删除:φi=1−exp(1−Fimi) (11) 此外,本模型考虑了复合材料的模量和强度的应变率效应:
XrateX0=1+Crateln˙ε˙ε0 (12) 式中:
φi 为退化模量的损伤因子,Fi 为第i 种失效模式对应的损伤函数,mi 为损伤演化特征参数,Xrate 指应变率为˙ε 时的模量、强度等材料参数,X0 指参考应变率˙ε0 下的模量、强度等材料参数,Crate 是为应变率效应系数,˙ε 为当前应变率,˙ε0 为参考应变率。将上述本构模型写成Fortran子程序进行二次开发,生成自定义的求解器求解。材料的模量和强度参数列于表4中,这些数据通过准静态拉伸、压缩与V形缺口梁剪切实验测得,以及通过应力-应变曲线拟合得到。表 4 靶板的材料参数Table 4. Material parameters of the target plateρ/(g·cm−3) E1/GPa E2/GPa E3/GPa ν21 ν31 ν32 G12/GPa G23/GPa G13/GPa Xt/MPa 0.911 4.00 4.00 1.54 0.19 0.25 0.25 3.50 1.60 1.60 30.0 Yt/MPa Xc/MPa Yc/MPa Zt/MPa Zc/MPa S12/MPa S23/MPa S13/MPa mi Crate ˙ε0/s−1 30.0 89.7 89.7 10.0 78.0 20.0 15.0 15.0 1.0~3.0 0.03~0.2 10.0 Q235钢制弹丸采用双线性弹塑性硬化本构,材料参数如表5所示。
2.3 数值模拟结果与分析
2.3.1 有限元模拟结果
对1.2节中的实验进行数值模拟。实验结果和模拟结果的对比如表6所示。
表 6 数值模拟结果与实验结果的对比Table 6. Comparison of numerical simulation results with experimental results实验 实验初速度/(m·s−1) 实验末速度/(m·s−1) 模拟末速度/(m·s−1) 模拟与实验结果的偏差/% 1 1640.0 1227.5 1202.0 2.04 2 1450.9 1040.1 995.0 4.34 3 1082.0 715.5 697.0 2.52 4 1046.0 651.0 624.0 4.59 5 583.7 未穿透,弹孔深约12 mm 未穿透,弹孔深约13 mm 8.30 6 775.0 263.5 280.0 7.22 运用式(1)拟合数值模拟得到的弹道冲击的剩余速度与初始速度的关系,并与实验结果进行了对比,如图11所示,得出数值模拟的弹道极限大约为706.3 m/s,与实验结果732.5 m/s的偏差约为3.51%。上述结果表明,本文选用的本构模型和数值模拟能很好地与实验吻合。
为了对所构建的模拟模型及模拟结果的可靠性进行检验,从损伤的形态、类型以及损伤区域的大小等方面,对相同实验条件下的模拟与实验的损伤形貌和损伤面积进行了比较。实验现象与模拟结果的对比如图12所示。
背面裂缝型的损伤区域呈菱形,对角线方向与面内纤维方向大致平行,靶板沿对角线的纤维拔出、纤维断裂等损伤形态对应数值模拟中的单元失效删除带来的十字形裂缝。实验与数值模拟结果均体现出沿弹丸运动方向的突出现象。数值模拟结果的损伤范围取菱形对角线长度,约为16.40 mm,与实验件的17.37 mm接近。
背面炸裂型的损伤区域呈圆形,弹孔附近纤维断裂与飞出,纤维与基底之间脱粘与拔出,这在数值模拟中体现为弹孔周围单元的变形与飞散。数值模拟结果的损伤范围取圆形,直径约为14.90 mm,与实验件的16.07 mm接近。
切孔型的损伤区域均呈矩形,在更高速度下,靶板背弹面的损伤范围小于前两种损伤,凸起不明显,且形成清晰的侵彻通孔,这在数值模拟中体现为弹孔内变形与飞散的单元减少。数值模拟结果的损伤范围取正方形,其边长约为16.00 mm,与实验件的14.78 mm接近。
实验与数值模拟损伤范围和形态的对比结果说明,本文采用的本构模型与数值模拟方法对连续纤维增强高孔隙复合材料的侵彻损伤进行了较好的还原,体现了损伤的主要特点。
2.3.2 弹丸侵彻过程分析
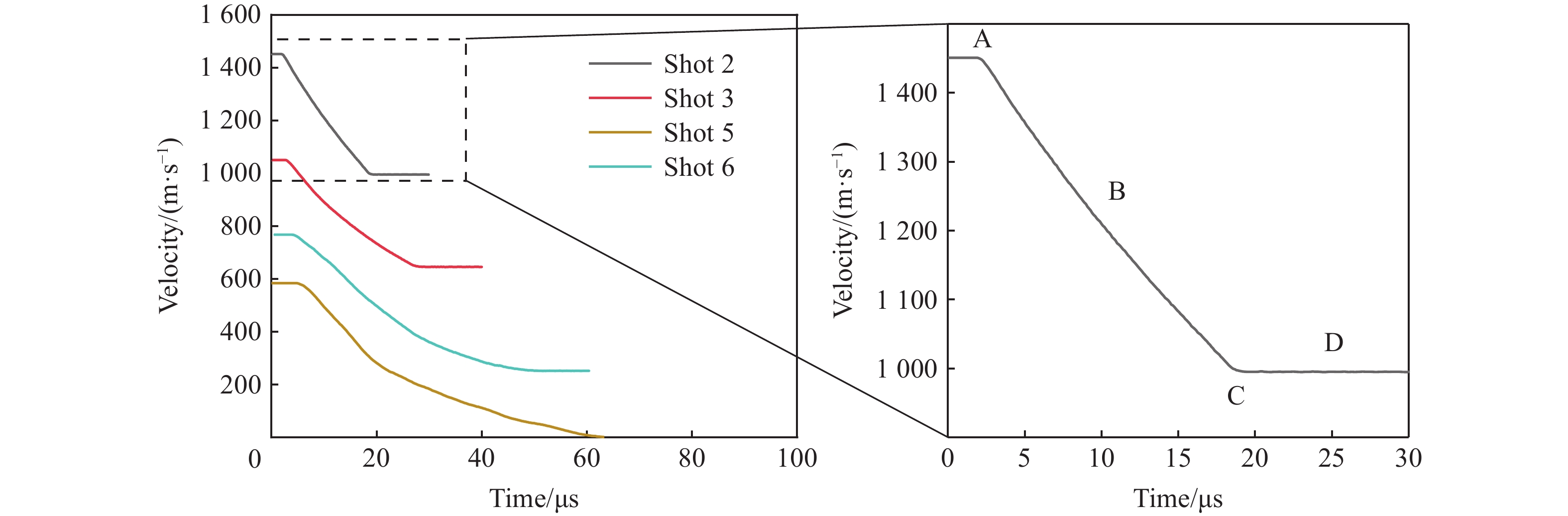
图13为侵彻速度随时间变化的曲线,依据不同的损伤类型,选择第2、3、5、6发实验的模拟结果为代表进行分析。每一条弹丸速度随时间的变化关系曲线都呈现出4个阶段[33]。
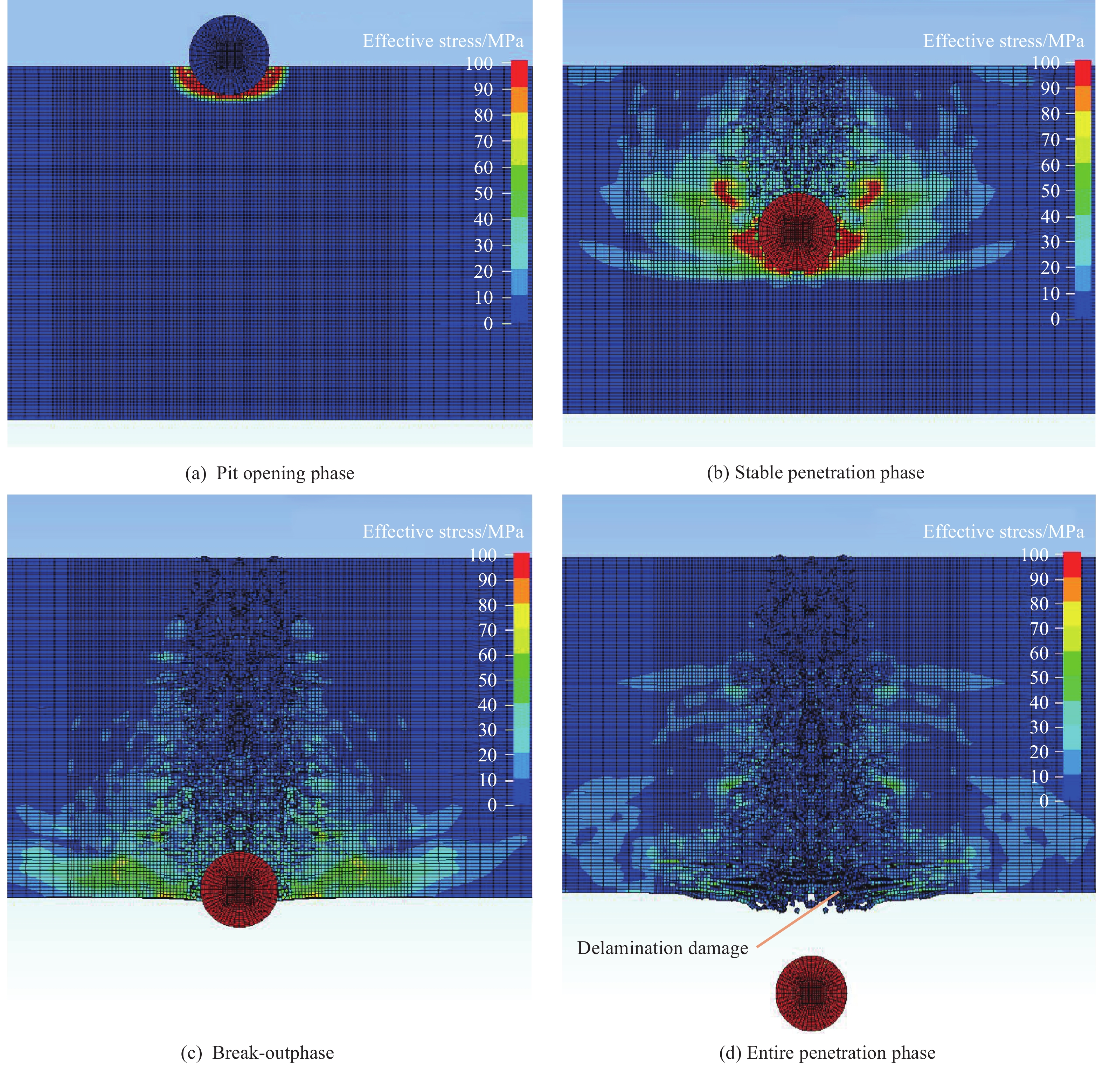
(1)开坑阶段,即阶段A。从弹丸接触靶板开始,由点接触逐渐过渡到面接触。这一阶段的动能与冲击压力最大,压力达到吉帕量级,压缩波向四周传播,如图14(a)所示。在弹丸与靶板接触区域,靶板受到强烈挤压,以至于破碎。此阶段直到弹丸完全没入靶板为止。
(2)稳定侵彻阶段,即阶段B。此阶段弹丸完全没入靶板,侵彻过程中弹丸与靶板接触的区域会形成压缩波,并向四周扩展,如图14(b)所示,而且弹孔区域内的单元会因损伤失效而被删除,在实验中体现为靶板的不断碎裂,形成粉末并飞出弹孔。与此同时,靶板对弹丸的的阻力会随侵彻深度的加大和弹丸速度的减小而逐渐下降。
(3)穿出阶段,即阶段C。弹丸头部开始穿出靶板,所受的阻力明显下降,侵彻阻力的减小使得弹丸速度的衰减急剧减缓,直到弹丸头部完全冲出靶板。靶板背面会由于弹丸速度的不同体现出不同的损伤类型,且由于压缩波在自由面处反射形成拉伸波,在弹丸穿出靶板的过程中会使靶板产生明显的分层损伤,如图14(c)所示。
(4)完全贯穿阶段,即阶段D。此阶段弹丸完全贯穿靶板,弹丸的速度近似为一常数。由于应力波的传播和反射,在穿孔处靶板的破坏范围会明显大于弹丸的大小,如图14(d)所示。
由表4可知,靶板的面外压缩波波速
c=√E3/ρ≈1300m/s 。由于剪切与分层破坏的分界点在压缩波与反射拉伸波的相遇位置[16],所以弹丸的初速度越高,在厚度方向剪切作用形成弹孔的长度越大,分层起始点离靶板背弹面越近。数值模拟很好地体现了这一趋势,图15展示了不同初速度下数值模拟的的弹孔形态,随着初速度越来越低(从左往右),红色圆圈所示的分层起始点离背弹面越来越远。弹丸侵彻过程中接触力随时间的变化关系如图16所示。在开坑阶段,复合材料的接触力急速上升。之后,接触力的下降趋势随初速度的变化有所不同,在第2~3发实验中大致分为两段:在稳定侵彻阶段的缓慢稳定的下降与在弹丸穿出靶板的瞬间极快地降为零,这符合图13中第2~3发实验的弹丸穿出靶板的瞬间速度-时间曲线有不光滑点的特征,原因是应力波波速不显著高于弹丸的速度,使弹丸之前的材料受到应力波损害的范围局限于背弹面附近;而第5~6发实验下弹丸的接触力达到峰值后逐渐降为零,与图13中第5~6发实验下速度随时间的曲线下降较为平缓、弹丸穿出靶板的瞬间速度-时间曲线相对光滑的特征相印证,原因是应力波波速明显高于弹丸速度,使弹丸之前的材料在离背弹面相当大的范围内已经受到应力波来回反射造成的损害。除第2发实验的接触力峰值达到4.5 kN外,其他情况下接触力的峰值约为3.0 kN。
2.3.3 侵彻开孔过程应力与损伤分析
针对复合材料的侵彻损伤形态比金属、高分子等均质材料更加复杂的问题,有必要开展对不同的应力和损伤因子及其分布对复合材料侵彻破坏作用的分析。
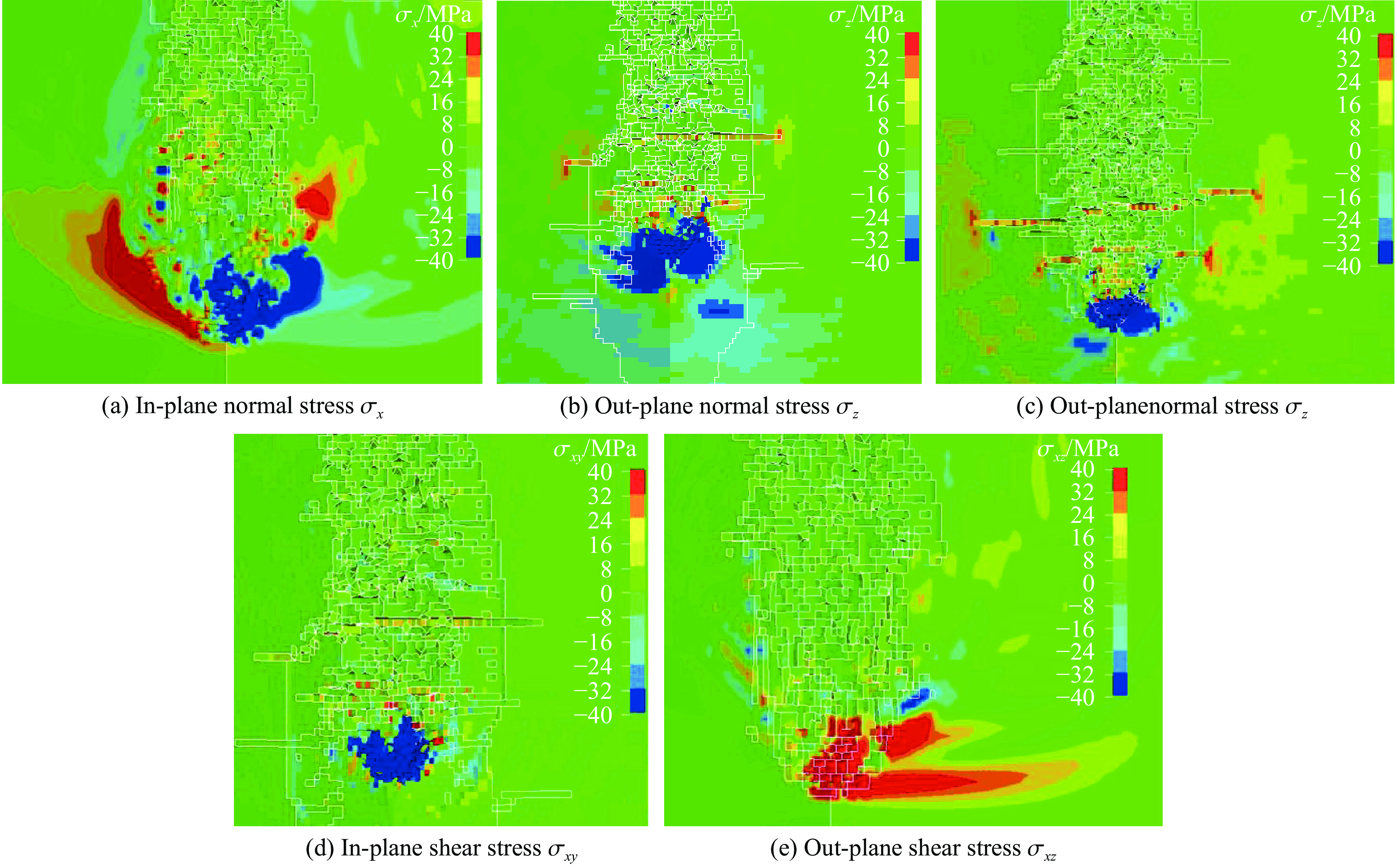
侵彻过程中靶板的应力分布如图17所示。关于面内正应力
σx ,靶板与弹丸的接触区域产生了很大的面内压应力,而面内拉应力主要分布在经过弹孔中心的xOz与yOz平面。关于面外正应力σz ,面外压应力主要分布于靶板与弹丸的接触区域,而拉应力分布于弹孔周围,特别是分层裂纹尖端处,通过前后时刻面外正应力的比较可知,面外压应力形成的压缩波在自由面处反射形成拉伸波,导致靶板层间发生张开型(Ⅰ型)断裂。面内剪应力σxy 主要分布在靶板与弹丸的接触区域,带来一定的基底损伤。侵彻过程中靶板的面外剪应力σxz 不仅数值很大,而且分布在弹丸与靶板接触区域相同厚度的弹孔周围,这表明靶板受撞击部分获得与弹丸相近的速度,与其他相对静止的部分在侵彻方向上出现了速度梯度,形成剪切[34],再加上靶板的抗剪强度较小, 因此很容易产生剪切破坏,导致纤维在剪切梯度作用下被剪断[16],并造成层间滑开型(Ⅱ型)断裂。稳定侵彻阶段的损伤分布如图18所示。弹孔周围纤维的拉伸、拔出等损伤与面内拉伸损伤有密切关系,同时,靶板在面内受到压缩损伤,实际情况下体现为基底材料的碎裂、压溃,失去对纤维的支撑作用。面内剪切损伤主要分布于靶板的面内xOy对角线两侧,对侵彻过程中基底损伤与纤维束的散开有贡献,而由于面内剪切导致的撕开型(Ⅲ型)断裂对分层损伤的贡献很小[35],所以面内剪切损伤对靶板面外分层的贡献可忽略不计。靶板的面外压缩损伤主要分布在弹丸和靶板的接触区,说明球形弹丸侵彻是压缩与剪切共同作用的过程。靶板沿厚度方向的变形和分层与面外拉伸损伤的分布有明显关系,在弹丸下方有面外拉伸损伤严重的区域,这反映了靶板的分层损伤是由压缩波在自由面处反射形成拉伸波所致。
2.3.4 不同损伤因子的影响分析
由于实验过后并未将靶板沿弹孔切开观察内部损伤的形态,所以展示背弹面处实验结果的损伤形态与数值模拟的损伤因子分布,作为推测造成不同损伤类型的影响因素之一,如图19所示。由于三种损伤类型对应的面内压缩损伤较弱,且弹丸穿透靶板后看不到面外压缩损伤,所以仅展示面内拉伸损伤因子
F1,t 、面外拉伸损伤因子F3,t 与面内剪切损伤因子F12 的分布。对比不同的损伤类型及其数值模拟损伤分布,发现面外拉伸分层损伤因子
F3,t 的分布与靶板损伤区域的形状和大小有明显联系,特别是背面裂缝型和背面炸裂型损伤的F3,t 分布范围分别为菱形和圆形,与实验结果基本一致。F3,t 也与靶板背弹面沿弹丸运动方向的凸起有关,例如,对于背面裂缝型损伤,靶板背弹面沿弹丸运动方向的凸起范围与F3,t 的分布范围相当。面内拉伸损伤
F1,t 的分布决定了不管任何损伤类型,弹孔周围纤维断裂之处均保持在以弹孔为中心平行于纤维方向的十字形范围内,弹孔周围纤维的损伤破坏的主导因素是过大的面内拉应力σx 、σy 与面内拉伸损伤F1,t 、F2,t ,与实验现象中各种损伤类型下纤维更倾向于整齐地断裂与弯折,散开的纤维的长度趋于一致而不是随机杂乱地断裂相符。面内剪切损伤
F12 的范围和强弱与背弹面纤维基底脱粘的范围和程度有密切关系,背面裂缝型损伤的纤维基底脱粘范围小、程度微弱与F12 相对严重区域面积小有关,背面炸裂型损伤的F12 相比背面裂缝型损伤显著扩大,程度更剧烈。此外,F12 的分布与靶板损伤区域的形状和大小也有关,切孔型损伤的破坏范围与F12 的分布范围关系更明显,损伤程度极其剧烈,造成更显著的纤维与基底的脱粘。2.3.5 侵彻过程能量转化分析
弹丸冲击复合材料靶板的能量消耗与弹丸特性、靶板材料和结构特性、靶板受到的约束形式以及靶板的损伤模式等相关。为简化分析,认为侵彻前后弹丸的动能主要转化为弹丸的内能、靶板的动能和内能。内能主要体现为变形、断裂等损伤;而由于靶板的边界受到刚性约束,所以靶板的动能大部分是侵彻后飞出的靶板冲击产物的动能。
不同速度下靶板的动能、内能及其占弹丸动能变化量的比重随速度的变化关系如图20所示。随着初速度的增大,大体上靶板的动能增大,内能减小。靶板动能占弹丸动能变化量的比例与速度之间的关系不十分明显,稳定在40%~60%的区间内,而靶板内能占弹丸动能变化量的比例会随速度的升高而降低,在宏观上体现为初速度越高,弹丸的动能转化为靶板碎片的动能的占比越高,而随着初速度的降低,靶板更多通过变形和损伤来吸收弹丸的能量。靶板动能与内能占弹丸动能变化量的比例之和小于100%的原因是,弹丸侵彻靶板的过程中,部分能量转化为弹丸的内能,耗散在弹丸与靶板的界面摩擦中。由于忽略冲击温升和摩擦耗散能量,因此,上述能量主要转化为1.2节所示弹丸的塑性变形产生的耗能,而且弹丸的速度越高,弹丸的动能转化为弹丸的变形等耗能更加显著。
3. 结 论
运用二级轻气炮实验装置和有限元模拟手段,分别通过实验和模拟分析对20 mm 厚的连续纤维增强高孔隙复合材料的高速冲击进行了研究,并基于实验和数值模拟结果分析了连续纤维增强高孔隙复合材料的侵彻损伤特性。得到以下主要结论。
(1) 20 mm 厚的连续纤维增强高孔隙防热复合材料在直径4.5 mm的Q235钢弹冲击下,弹道极限速度约为732.5 m/s,背弹面的损伤形态随初速度的不同分为背面裂缝、背面炸裂、切孔损伤三种。
(2)连续纤维增强高孔隙复合材料在初速度750 m/s以上的防护能力低于钢、铝等材料,在600 m/s以下可以代替钢、铝等传统金属材料和碳纤维、玻璃纤维、凯夫拉等传统复合材料。
(3)数值模拟得到的弹丸末速度、弹道极限、损伤形态与范围的结果与实验结果吻合较好。有限元模拟表明,球形弹丸侵彻过程中,弹孔的形成与纤维基底的脱粘是压缩和剪切共同作用导致的,靶板的分层损伤是压缩波反射形成的拉伸波所致,纤维的断裂属于拉伸损伤。
(4)随弹丸初速度的提高,弹丸的动能更多地转化为靶板碎片的动能和弹丸的内能,而转化为靶板内能及其比例越来越低。
-
表 1 材料参数
Table 1. Material parameters
材料 ρ/(kg∙m−3) E/GPa ν σy/MPa Et/MPa sc 铝 2700 69.5 0.33 127 586 有机玻璃 1190 3.6 0.4 0.047 环氧树脂 3 0.37 0.047 表 2 冲击过程中冲击物的主要运动参数
Table 2. Main motion parameters of impact object in the process of impact
孔隙率 反弹速度/(m∙s−1) 残余动能/mJ 加速度峰值/(km∙s−2) 动能吸收率/% 0.7 0.68 8 47 99 0.6 2.91 15 53 97 0.5 4.72 39 66 94 0.4 6.08 65 85 90 -
[1] ZHANG J X, QIN Q H, WANG T J. Compressive strengths and dynamic response of corrugated metal sandwich plates with unfilled and foam-filled sinusoidal plate cores [J]. Acta Mechanica, 2013, 224(4): 759–775. DOI: 10.1007/s00707-012-0770-5. [2] ZHANG J X, ZHOU R F, WANG M S, et al. Dynamic response of double-layer rectangular sandwich plates with metal foam cores subjected to blast loading [J]. International Journal of Impact Engineering, 2018, 122: 265–275. DOI: 10.1016/j.ijimpeng.2018.08.016. [3] ZHANG J X, YE Y, QIN Q H. On dynamic response of rectangular sandwich plates with fibre-metal laminate face-sheets under blast loading [J]. Thin-Walled Structures, 2019, 144: 106288. DOI: 10.1016/j.tws.2019.106288. [4] 郭亚周, 刘小川, 何思渊, 等. 不同弹形撞击下泡沫铝夹芯结构动力学性能研究 [J]. 兵工学报, 2019, 40(10): 2032–2041. DOI: 10.3969/j.issn.1000-1093.2019.10.008.GUO Y Z, LIU X C, HE S Y, et al. Research on dynamic properties of aluminum foam sandwich structure impacted by projectiles with different shapes [J]. Acta Armamentarii, 2019, 40(10): 2032–2041. DOI: 10.3969/j.issn.1000-1093.2019.10.008. [5] 张博一, 赵威, 王理, 等. 泡沫铝子弹高速撞击下铝基复合泡沫夹层板的动态响应 [J]. 爆炸与冲击, 2017, 37(4): 600–610. DOI: 10.11883/1001-1455(2017)04-0600-11.ZHANG B Y, ZHAO W, WANG L, et al. Dynamic response of aluminum matrix syntactic foams sandwich panel subjected to foamed aluminum projectile impact loading [J]. Explosion and Shock Waves, 2017, 37(4): 600–610. DOI: 10.11883/1001-1455(2017)04-0600-11. [6] 邓旭辉, 李亚斌. 双层泡沫铝夹芯板抗冲击性能数值研究 [J]. 铁道科学与工程学报, 2019, 16(10): 2603–2611. DOI: 10.19713/j.cnki.43-1423/u.2019.10.029.DENG X H, LI Y B. Numerical study on impact resistance of double-layer foam aluminum sandwich panels [J]. Journal of Railway Science and Engineering, 2019, 16(10): 2603–2611. DOI: 10.19713/j.cnki.43-1423/u.2019.10.029. [7] 张永康, 李玉龙, 汤忠斌, 等. 冰雹撞击下泡沫铝夹芯板的动态响应 [J]. 爆炸与冲击, 2018, 38(2): 373–380. DOI: 10.11883/bzycj-2016-0232.ZHANG Y K, LI Y L, TANG Z B, et al. Dynamic response of aluminum-foam-based sandwich panels under hailstone impact [J]. Explosion and Shock Waves, 2018, 38(2): 373–380. DOI: 10.11883/bzycj-2016-0232. [8] 夏志成, 张建亮, 周竞洋, 等. 泡沫铝夹芯板抗冲击性能分析 [J]. 工程力学, 2017, 34(10): 207–216. DOI: 10.6052/j.issn.1000-4750.2016.06.0494.XIA Z C, ZHANG J L, ZHOU J Y, et al. Analysis on impact resistance of aluminum foam sandwich panels [J]. Engineering Mechanics, 2017, 34(10): 207–216. DOI: 10.6052/j.issn.1000-4750.2016.06.0494. [9] CUI T N, ZHANG J H, LI K K, et al. Ballistic limit of sandwich plates with a metal foam core [J]. Journal of Applied Mechanics, 2022, 89(2): 021006. DOI: 10.1115/1.4052835. [10] 苏兴亚, 敬霖, 赵隆茂. 爆炸载荷下分层梯度泡沫铝夹芯板的失效模式与抗冲击性能 [J]. 爆炸与冲击, 2019, 39(6): 063103. DOI: 10.11883/bzycj-2018-0198.SU X Y, JING L, ZHAO L M. Failure modes and shock resistance of sandwich panels with layered-gradient aluminum foam cores under air-blast loading [J]. Explosion and Shock Waves, 2019, 39(6): 063103. DOI: 10.11883/bzycj-2018-0198. [11] 王涛, 余文力, 秦庆华, 等. 爆炸载荷下泡沫铝夹芯板变形与破坏模式的实验研究 [J]. 兵工学报, 2016, 37(8): 1456–1463. DOI: 10.3969/j.issn.1000-1093.2016.08.017.WANG T, YU W L, QIN Q H, et al. Experimental investigation into deformation and damage patterns of sandwich plates with aluminum foam core subjected to blast loading [J]. Acta Armamentarii, 2016, 37(8): 1456–1463. DOI: 10.3969/j.issn.1000-1093.2016.08.017. [12] SILLING S A. Reformulation of elasticity theory for discontinuities and long-range forces [J]. Journal of the Mechanics and Physics of Solids, 2000, 48(1): 175–209. DOI: 10.1016/S0022-5096(99)00029-0. [13] SILLING S A, ASKARI E. A meshfree method based on the peridynamic model of solid mechanics [J]. Computers & Structures, 2005, 83(17/18): 1526–1535. DOI: 10.1016/j.compstruc.2004.11.026. [14] SILLING S A, EPTON M, WECKNER O, et al. Peridynamic states and constitutive modeling [J]. Journal of Elasticity, 2007, 88(2): 151–184. DOI: 10.1007/s10659-007-9125-1. [15] 杨娜娜, 赵天佑, 陈志鹏, 等. 破片冲击作用下舰船复合材料结构损伤的近场动力学模拟 [J]. 爆炸与冲击, 2020, 40(2): 023302. DOI: 10.11883/bzycj-2019-0019.YANG N N, ZHAO T Y, CHEN Z P, et al. Peridynamic simulation of damage of ship composite structure under fragments impact [J]. Explosion and Shock Waves, 2020, 40(2): 023302. DOI: 10.11883/bzycj-2019-0019. [16] 刘宁, 胡梦凡, 周飞. 基于键基近场动力学理论的单裂纹圆孔板冲击破坏研究 [J]. 工程力学, 2020, 37(12): 9–17. DOI: 10.6052/j.issn.1000-4750.2020.02.0076.LIU N, HU M F, ZHOU F. The impacted damage study of a single cleavage drilled compression specimen based on bond-based peridynamics [J]. Engineering Mechanics, 2020, 37(12): 9–17. DOI: 10.6052/j.issn.1000-4750.2020.02.0076. [17] 熊伟鹏, 王超, 傅江妍, 等. 冰球冲击试验的近场动力学方法数值模拟 [J]. 振动与冲击, 2020, 39(7): 148–155. DOI: 10.13465/j.cnki.jvs.2020.07.021.XIONG W P, WANG C, FU J Y, et al. Numerical simulation of ice sphere impact test by peridynamics method [J]. Journal of Vibration and Shock, 2020, 39(7): 148–155. DOI: 10.13465/j.cnki.jvs.2020.07.021. [18] MADENCI E, OTERKUS S. Ordinary state-based peridynamics for plastic deformation according to von Mises yield criteria with isotropic hardening [J]. Journal of the Mechanics and Physics of Solids, 2016, 86: 192–219. DOI: 10.1016/j.jmps.2015.09.016. -








 下载:
下载:








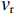





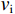





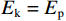

















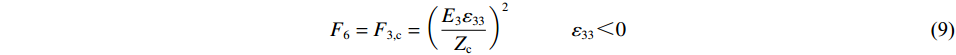
































































 下载:
下载: