Damage effects of underwater explosions on gravity dams and optimal standoff distances
-
摘要: 为研究不同爆距水下爆炸对重力坝的毁伤效应,并探讨是否存在“最优爆距”,基于离心模型试验建立了炸药-库水-空气-重力坝结构的全耦合数值模型,并设计了60组数值计算工况。不同工况水深均为600 mm,炸药量为2.2 g,重力坝模型几何比尺为1/80,包含5组爆深(50~250 mm),每组爆深对应12组爆距,爆距范围为10~200 mm,相应比例爆距范围为0.077~1.54 m/kg1/3。对比分析了不同爆距水下爆炸对重力坝的毁伤程度,并定量比较了重力坝平均损伤、单元删除率、应力、应变等参数。结果表明,对于重力坝整体结构破坏,如重力坝整体弯曲导致的拉伸破坏,水下爆炸对重力坝的毁伤效应存在“最优爆距”,即随着爆距增加重力坝毁伤程度先增加后降低;与之类似,随着爆距的增加,重力坝上游坝面损伤区域的平均损伤、重力坝单元删除率、坝踵最大拉应力平均值和坝踵最大拉应变平均值先增加后降低且在40 mm爆距附近达到最大值。保持水深、炸药量和重力坝几何模型相同,5组不同爆深近水面水下爆炸对重力坝毁伤效应的“最优爆距”均在40 mm附近,表明近水面水下爆炸时爆深对“最优爆距”不存在显著影响。Abstract: To investigate the standoff distance of underwater explosions on the damage to gravity dams and to explore whether there is an “optimal standoff distance”, a numerical model of a fully-coupled explosive-water-air-gravity dam was established. The numerical model was validated by comparing it with centrifuge test results. The results demonstrated that the employed numerical model could predict the dam failures and the effect of bubble pulse well. Then, a numerical scheme including 60 numerical calculations was designed. In these calculations, the water depth is 600 mm, the explosive mass is 2.2 g, and the geometrical scaling factor of the gravity dam model is 1/80. The detonation depth ranges from 50 to 250 mm with five detonation depths. Each detonation depth corresponds to 12 standoff distances ranging from 10 to 200 mm, with the scaled standoff distance ranging from 0.077 to 1.54 m/kg1/3. The damage degrees to the gravity dam under underwater explosions with different standoff distances are compared. Quantitative comparisons of dam average damage, element erosion rate, stress, and strain are also presented. The results show that for the overall structural failure of the gravity dam, such as the structural bending-induced tensile failure, there is an “optimal standoff distance” for the damage effects of underwater explosions on gravity dams, that is, with the increase of standoff distance, the damage degree of gravity dam increases first and then decreases. The quantitative results also indicate that with the increase of standoff distance, the average damage of the damaged area in the dam upstream face, the element erosion rate, the average value of the maximum tensile stress of the dam heel, and the average value of the maximum tensile strain of the dam heel all increase first and then decrease, and reach their maximum values around a standoff distance of 40 mm. With identical water depth, explosive mass, and geometrical model of gravity dam, the “optimal standoff distances” for the damage effects of near-surface underwater explosions at five different detonation depths on the gravity dam are all near 40 mm. It suggests that for near-surface underwater explosions, the detonation depth owns limited influence on the “optimal standoff distance”.
-
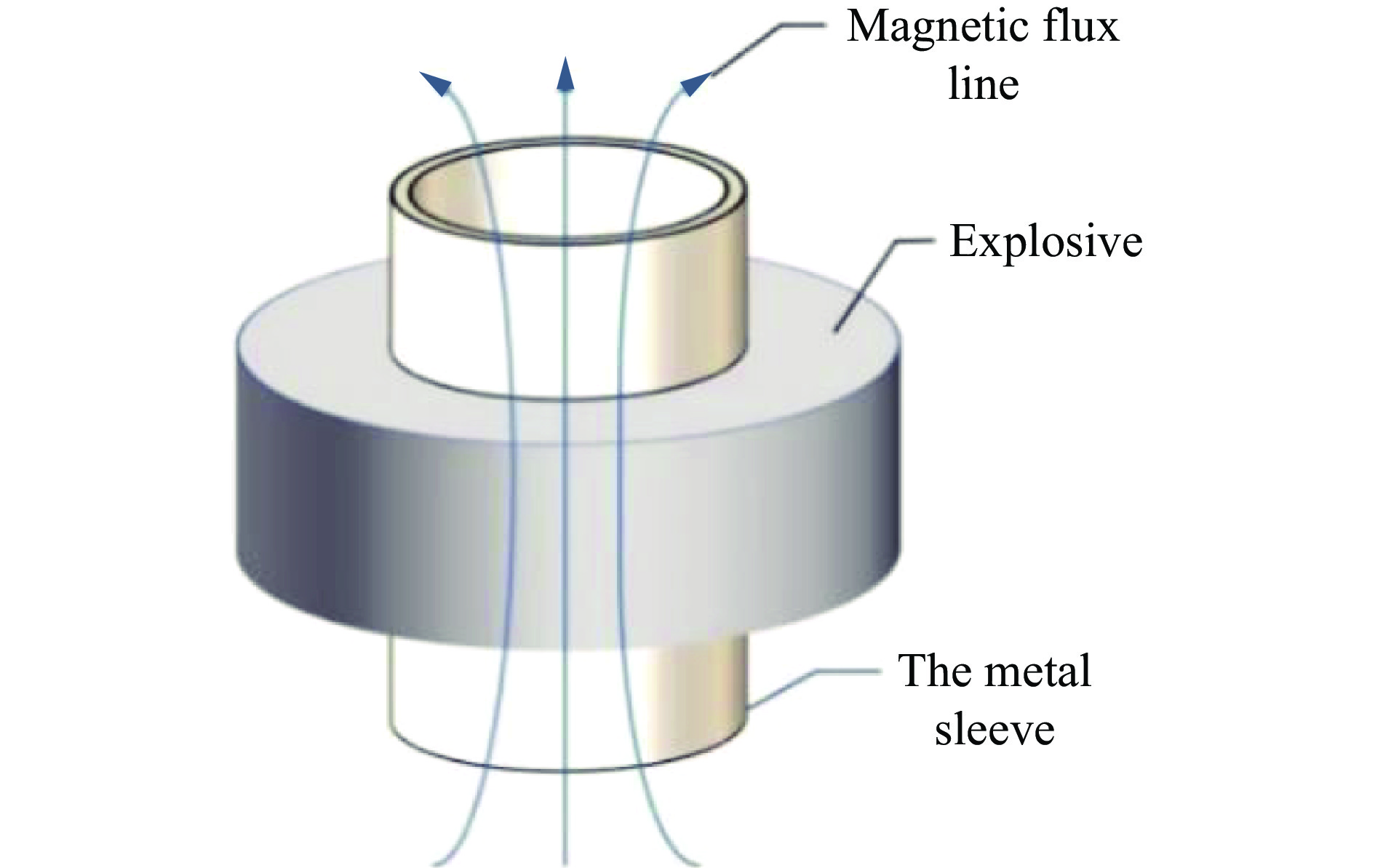
柱面炸药内爆磁通量压缩技术是一种工作原理极为巧妙的极端条件动加载实验技术,它利用炸药驱动金属套筒压缩其内部预先设置磁通量,将炸药化学能转化为磁场能,由于爆炸加载过程短,绝大多数磁通量来不及扩散而被压缩至套筒轴线附近小体积内形成超强磁场,并对其中的材料施加很高的磁压力(洛伦兹力)。20世纪50年代,Sakharov等[1]和Fowler等[2]几乎同时提出了爆磁压缩的物理思想。
内爆磁通量压缩装置基本原理见图1。早期研发的爆磁装置可称为单级装置(只有一级套筒),这类装置在套筒内爆压缩过程中存在明显的失稳现象且难以避免,无法保证实验过程的稳定[3-4]。 20世纪80~90年代,针对单级装置的局限性, Pavlovskii[5]提出了多级MC-1装置的物理思想,其原理示意图如图2所示:炸药爆炸驱动一级套筒压缩其内部磁通量,一级套筒在失稳前会碰撞二级套筒继续压缩磁通量,以此类推,利用套筒接力传递的方式压缩其内部磁通量,这样理论上就可以改善由于套筒失稳对磁通量压缩的影响。
一级套筒是多级装置的核心部件,起到产生初始磁场和作为一级套筒的双重作用。一级套筒采用多层密绕螺线管结构,实验开始时,首先对它通电产生轴向磁场,当磁场达到预定值后炸药起爆,在爆轰波阵面(高温高压状态)的驱动作用下,螺线管变成一个金属套筒约束并开始压缩其内部磁通量,一级套筒在失稳前撞上二级套筒并将二级套筒压缩成一个金属筒,从而约束其内部磁通量并继续压缩其内部磁通量,三级套筒结构与次级类似。
实验表明,采用多级技术路线能够提升内爆磁压缩装置的工作稳定性。Bykov等[6]发展了多种规格的MC-1装置,实现了最高峰值磁场强度达到2800 T。1996~1998年,Clark[7]和Lindemuth等[8]针对超强磁场下凝聚态物质的新物理现象及超高压力压缩(聚能)材料特性进行研究,包括冷冻气体的金属化研究等。谷卓伟等[9]和Zhou等[10]研制了单级内爆磁压缩装置CJ-100,能够稳定实现600~700 T的超强磁场。
本文中将利用磁流体力学编码SSS-MHD开展多级内爆磁压缩技术研究,研制多级内爆磁压缩CJ-150装置,以实现峰值磁场906 T。
1. 实验系统设计方案
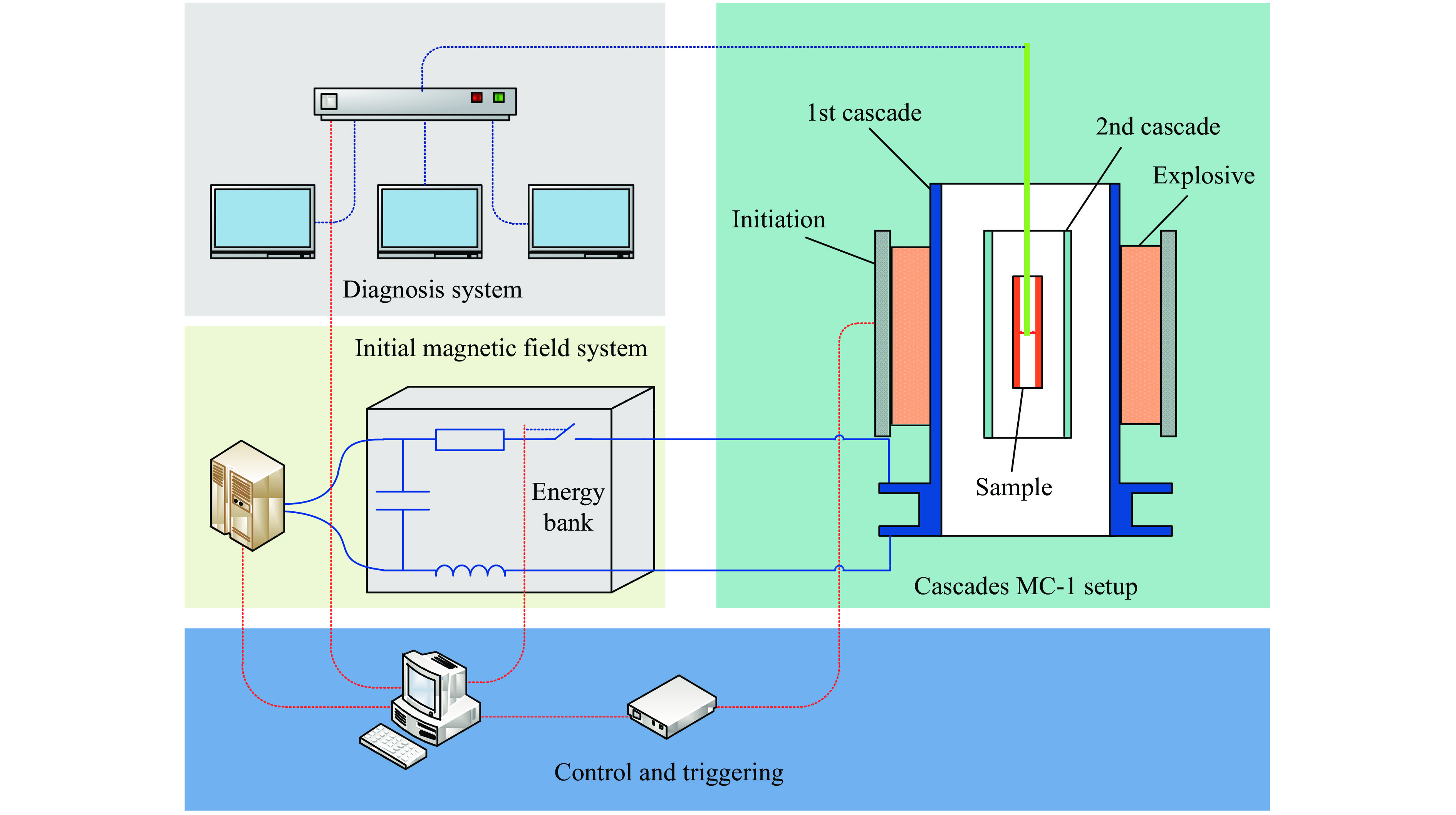
多级内爆磁压缩实验系统主要包括初始磁场能源系统、控制与触发系统、诊断系统及多级实验装置等,其中多级装置包括柱面同步起爆网络、柱状环形炸药、第1级套筒(密绕螺线管)、第2级套筒及样品靶等。多级CJ-150实验系统如图3所示。
1.1 多级装置设计
装置的设计规模取决于物理需求、实验室条件等多种因素。为研制能够在亚立方厘米以上空间内实现千特斯拉量级的超强磁场多级装置,并具备对材料数百吉帕量级准等熵加载能力,将炸药当量设定为20 kg TNT以内,利用一维爆轰磁流体程序SSS-MHD[11]开展了多级磁压缩过程数值模拟。SSS-MHD可对材料动力学、反应流体动力学和磁流体力学进行多物理场、多介质、多组分和多连通区的一体化计算,其拉氏一维方程组的形式如下。
连续性方程:
v=rα∂r∂M (1) u=∂r∂t (2) 动量方程:
∂u∂t=−rα∂∂M(p+q+B22μ0)−αvφr (3) φ=2Sx+Sz (4) 能量方程:
∂E∂t=−∂∂M[(p+q)urα]+λ∂∂M(rα∂T∂r)+ηvJ2 (5) E=I+12u2 (6) 磁扩散方程:
∂∂t(vB)=1μ0∂∂M(ηvr2α∂B∂M) (7) 式中:v和u分别为比容和速度,M为质量坐标,r 为欧拉坐标,p、q、B、μ0、S分别为流体压力、人工黏性压力、磁感应强度、真空磁导率、应力偏量,E、I、λ、T、η分别为总能量、内能、热传导系数、温度和电阻率。指数α取值为0、1时,分别代表平面、柱面的一维几何情况。
金属等材料的状态方程采用列表式数据库;炸药的状态方程采用HOM模型[12]、爆轰反应采用Forest Fire方程[13];材料的电阻率采用Burgess模型[14]描述;材料强度采用SCG修正模型[15]描述。
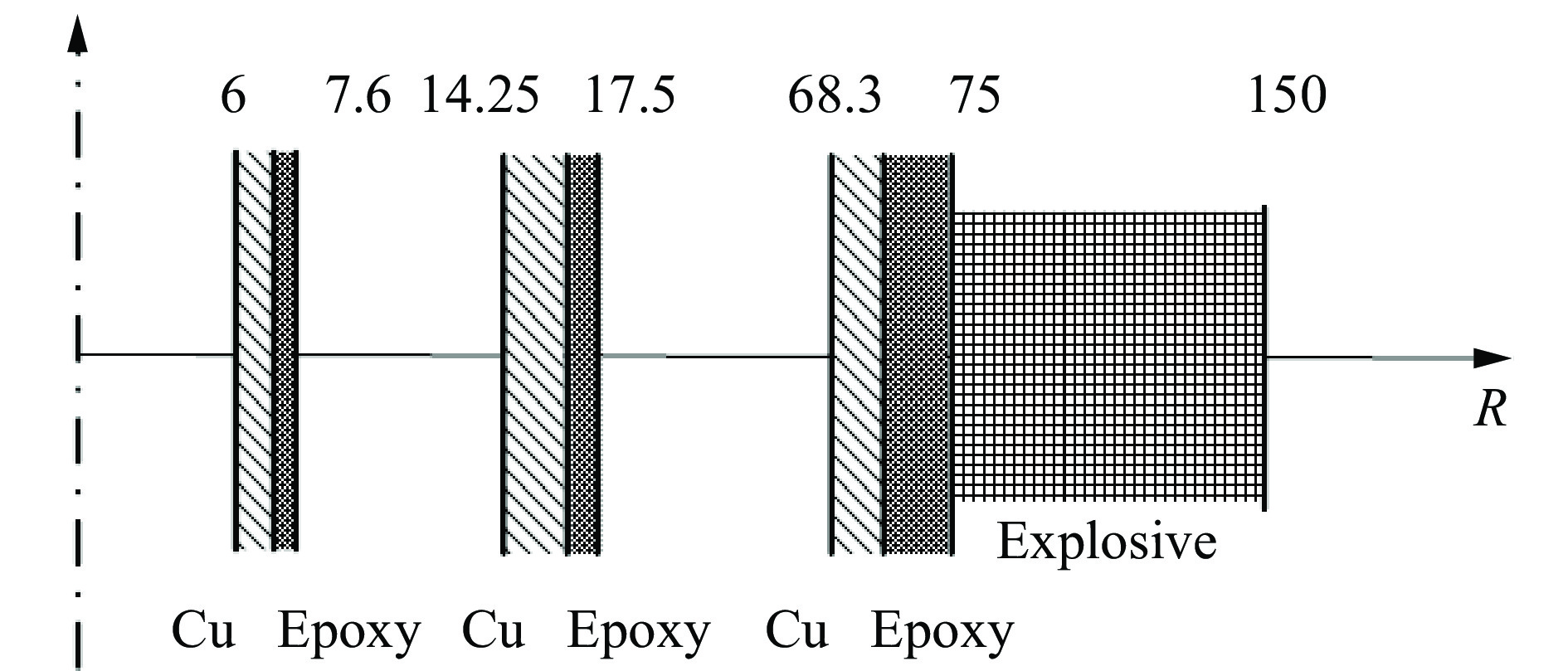
图4为三级装置计算模型,由于各级套筒基本结构为多层密绕螺线管或密排漆包线,外层为起固定绝缘作用的环氧层,在建模上可简化为铜层与环氧层,第3级套筒内空腔直径为12 mm,炸药内径 150 mm,外径 300 mm,高度180 mm,炸药采用RDX/TNT(60/40),TNT当量约20 kg。
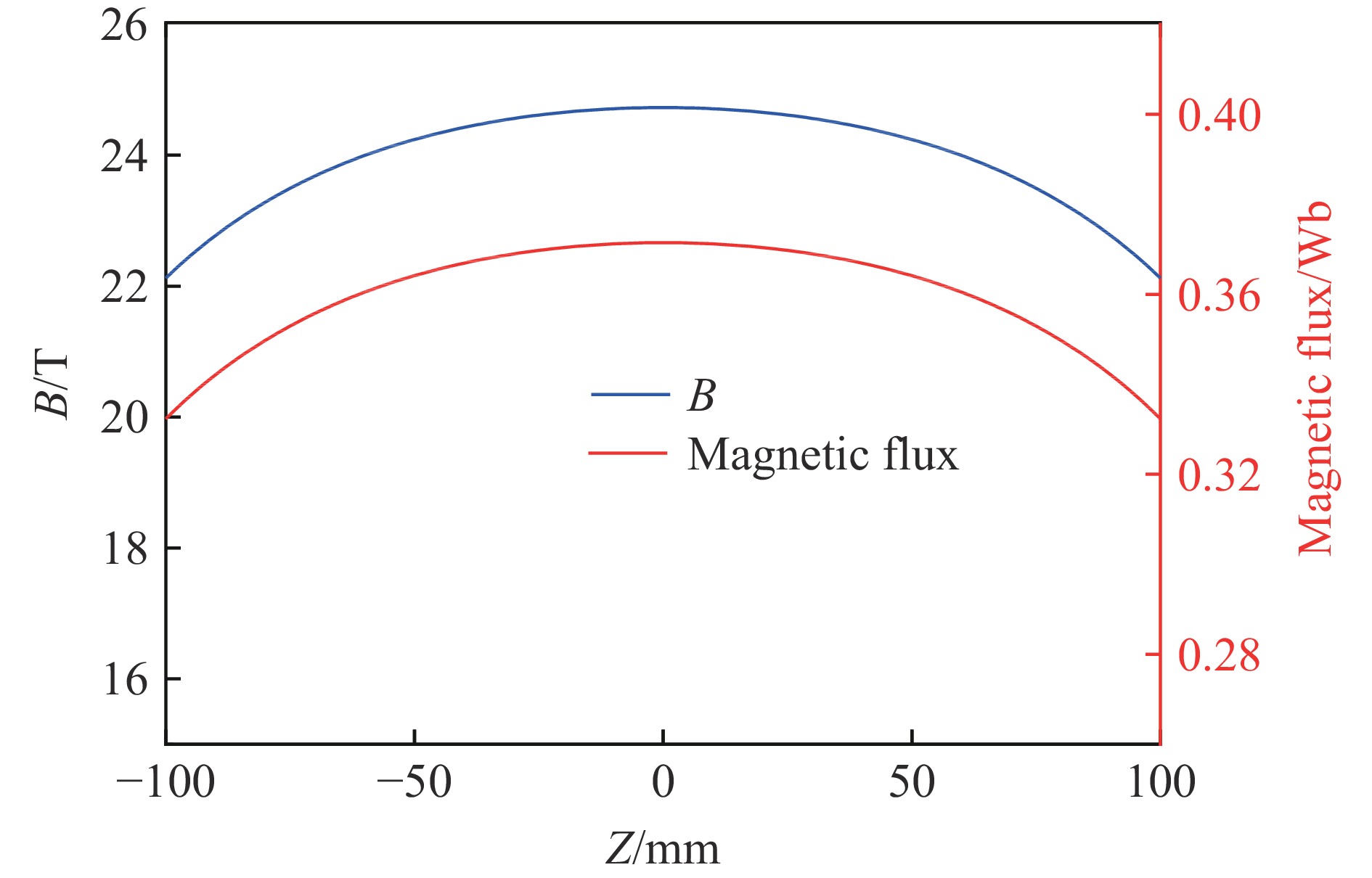
初始磁场设置为9.5 T,装置轴心区域磁通量汇聚历史见图5。计算结果表明,多级磁通量汇聚效果显著,约42%的初始磁通量被最终压缩至轴线周围直径约7 mm的空腔内。轴心空腔区域及相邻的第3级套筒铜线层区域磁场分布见图6。
三级套筒运动轨迹及压缩磁场波形见图7,可以看到第1级套筒碰撞第2级,第2级碰撞第3级,三级套筒反转半径约为3.5 mm,计算峰值磁场超过1460 T。在靠近轴心空腔的第3级套筒铜线层区域,对应的材料峰值压力(计算值)超过500 GPa,见图8。
1.2 线圈参数
多层密绕螺线管(第1级套筒)是多级内爆磁压缩装置的核心部件,其结构主要参数如下。
(1)第1级套筒(密绕螺线管):采用QZY漆包线绕制;线圈匝数为2匝;线圈内径约136.6 mm;线圈外径150 mm;高度约300 mm;螺旋线层采用直径0.25 mm的漆包线缠绕,共8层;绝缘层采用GHG绝缘膜;回流导体层为3层漆包线。螺线管壁剖面设计示意图见图9。
(2) 第2级套筒(线圈):铜线型号为QZY-0.25(外径),分10层轴向紧密排布并绕;套筒内径约28.5 mm、外径约35 mm;铜线区轴向长约200 mm。
(3) 第3级套筒(线圈):铜线型号为QZY-0.25(外径),分6层轴向紧密排布并绕;套筒内径约12 mm、外径约15.2 mm;铜线区轴向长度约150 mm。
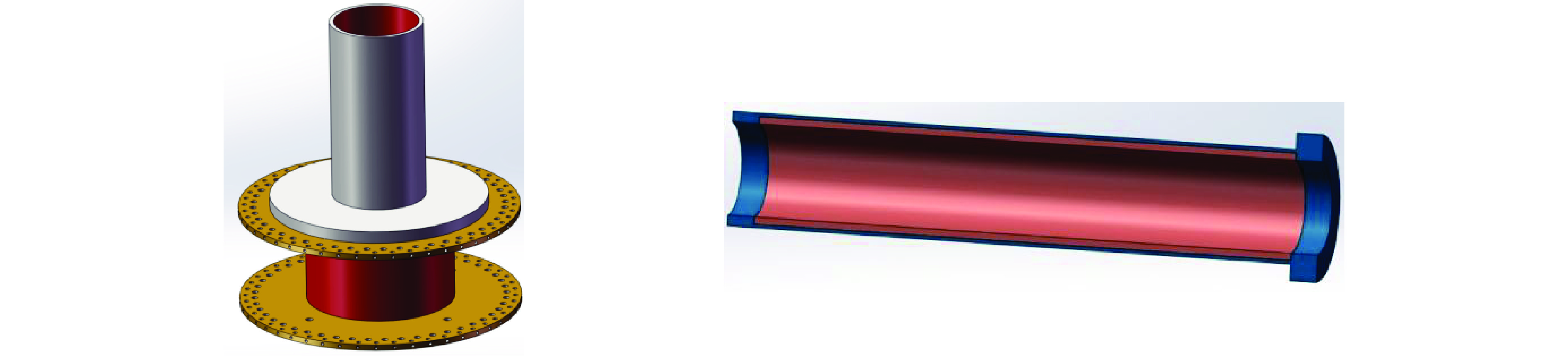
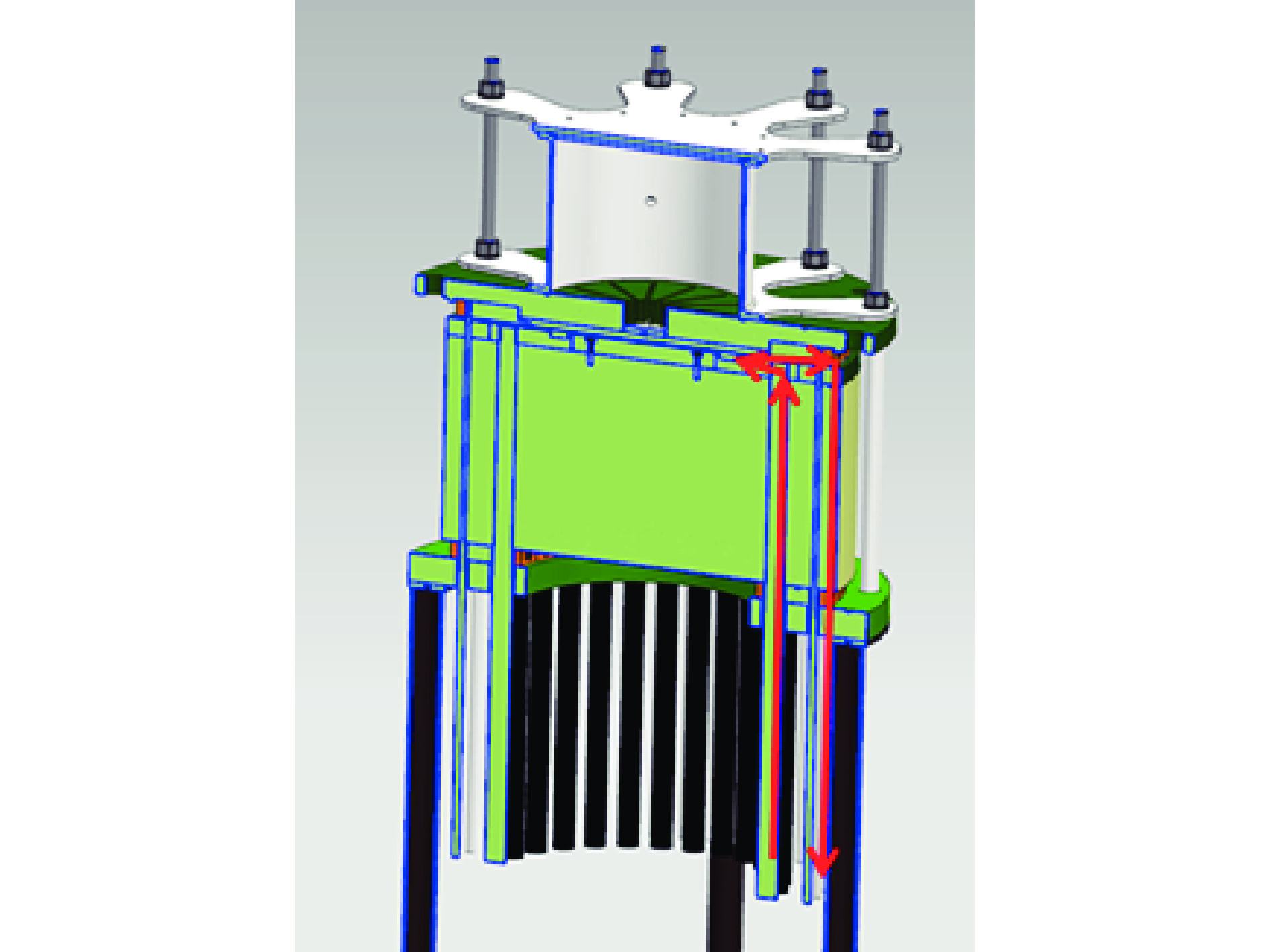
第1级和第2级套筒结构见图10。
1.3 能库电源设计
初始磁场能库设计按照25 T初始磁场的目标来设计。密绕螺线管线圈的磁场分布(归一化)计算结果见图11,线圈内磁通量及平均轴向磁场计算结果见图12。
设计线圈电感最终按照0.3 μH来考虑,多层密绕螺线管线圈电阻按照0.2 mΩ选取。有限元模拟显示,针对设计的线圈负载,输入电流在约3.2 MA时线圈最高磁场约为25 T,螺线管磁场分布见图11,线圈内磁通量和平均轴向磁场分布见图12。
电源系统的总放电电流确定为3.2 MA,由20个160 kA的放电模块构成。160 kA模块由高压电容、电流互感器、电阻分压器、接地开关、泄放限流电阻、高压放电电缆和安装结构等组成。能库电源模块见图13。
放电开关是能库系统的关键部件。由于能库电流输出大,脉冲时间长,电荷量超过100 C,常规开关难以承受,因此采用爆炸开关技术路线。设计的多路爆炸开关结构见图14,利用爆炸网络板同步起爆,14路开关相互独立,能够有效保证能库电源安全。
爆炸网络板采用927-H装药,利用电探针技术开展了网络板同步性测量,14路爆炸开关同步性极差为175 ns,满足装置需要,实验后回收的爆炸网络板见图15。
经过10余发动态实验考核,整个加载系统工作稳定,实验实测的初始磁场分布及放电电流波形见图16,从图中可以看出,在螺线管中磁场分布均匀,脉冲上升沿小于50 μs。
2. 实验结果
装配好的多级内爆磁压缩装置CJ-150见图17,实验场景见图18。
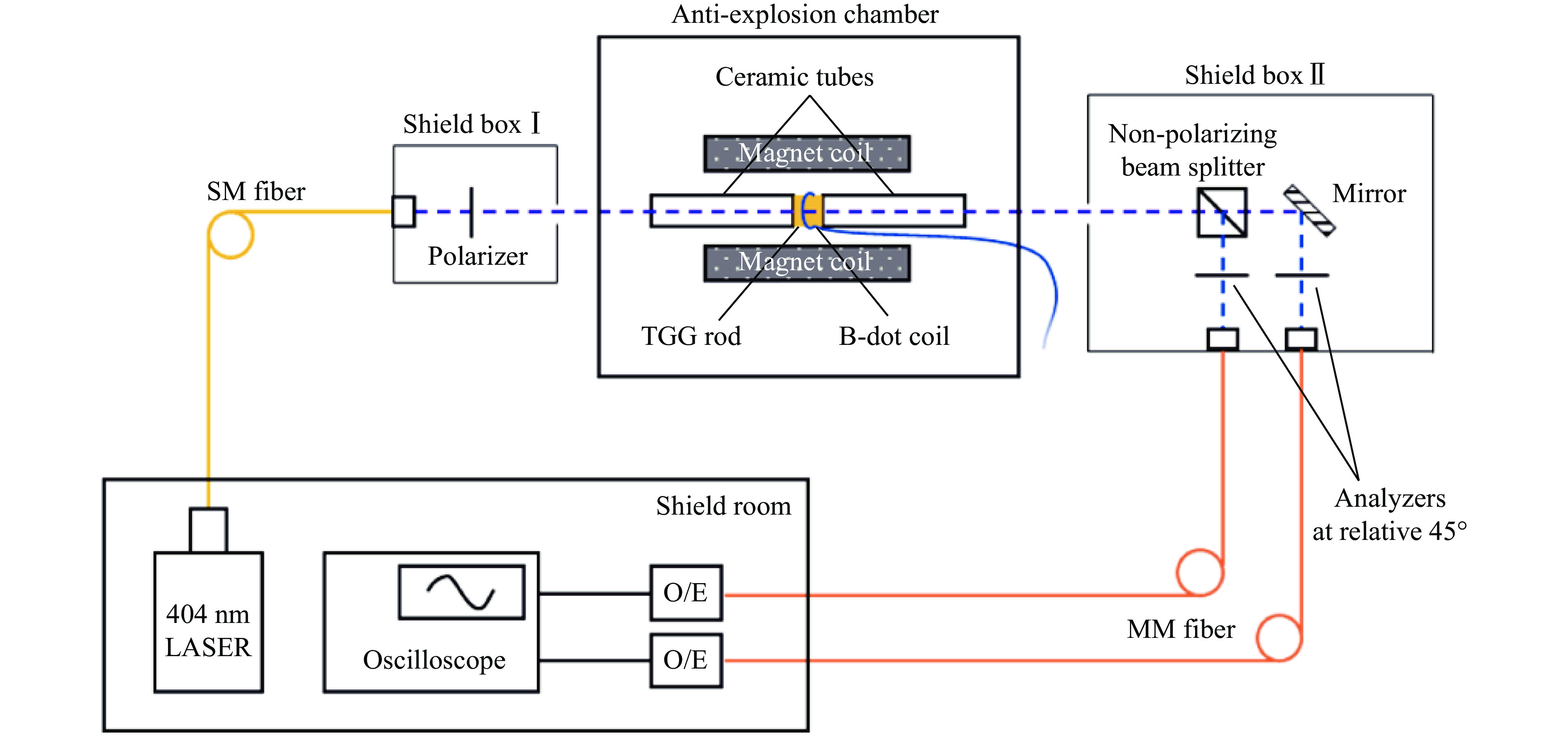
利用磁光测试系统[16]开展了CJ-150峰值磁场测量,磁光探针结构布局见图19。磁光晶体采用熔石英晶体,探针尺寸约
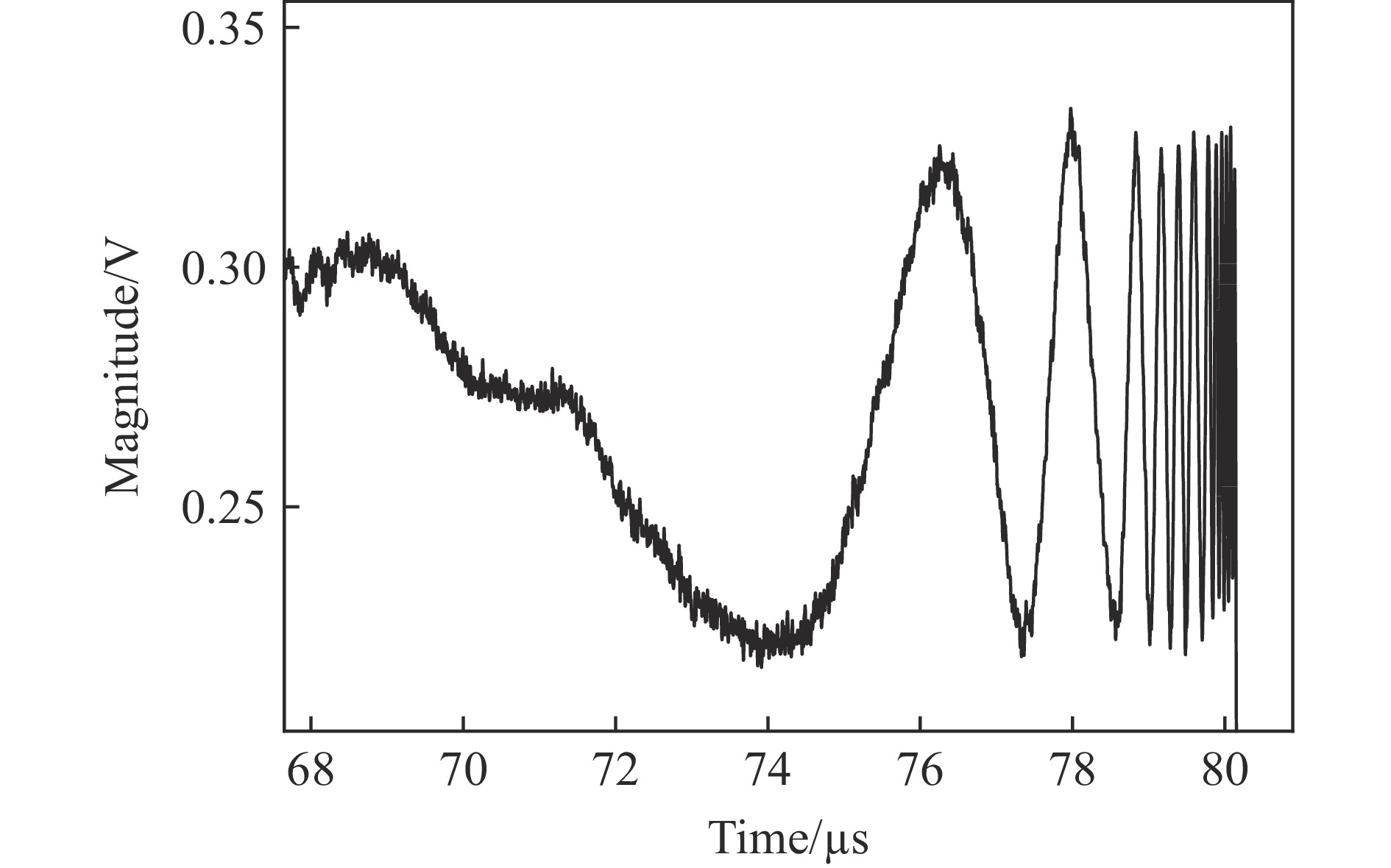
∅ 3 mm×3 mm。磁光探针原始信号如图20所示,信号信噪比非常好。磁场B由下式得到:
θ=∫VBdl=VLB (8) 式中:L 为磁光介质长度,V 为Verdet常数,θ为偏转角。磁场偏量dB由下式得到:
dB=√(1VL)2d2θ+(θLV2)2d2V+(θVL2)2d2L (9) 式中:dV为Verdet常数测量偏量,由磁光介质的波长色散特性决定,检测机构给出;dθ为偏转角测量偏量,由数据处理精度决定; dL为磁光介质长度测量偏量,由磁光晶体测量精度决定。
检测机构给出的Verdet常数测量不确定度dV/V=3.27%;法拉第偏转角测量不确定度
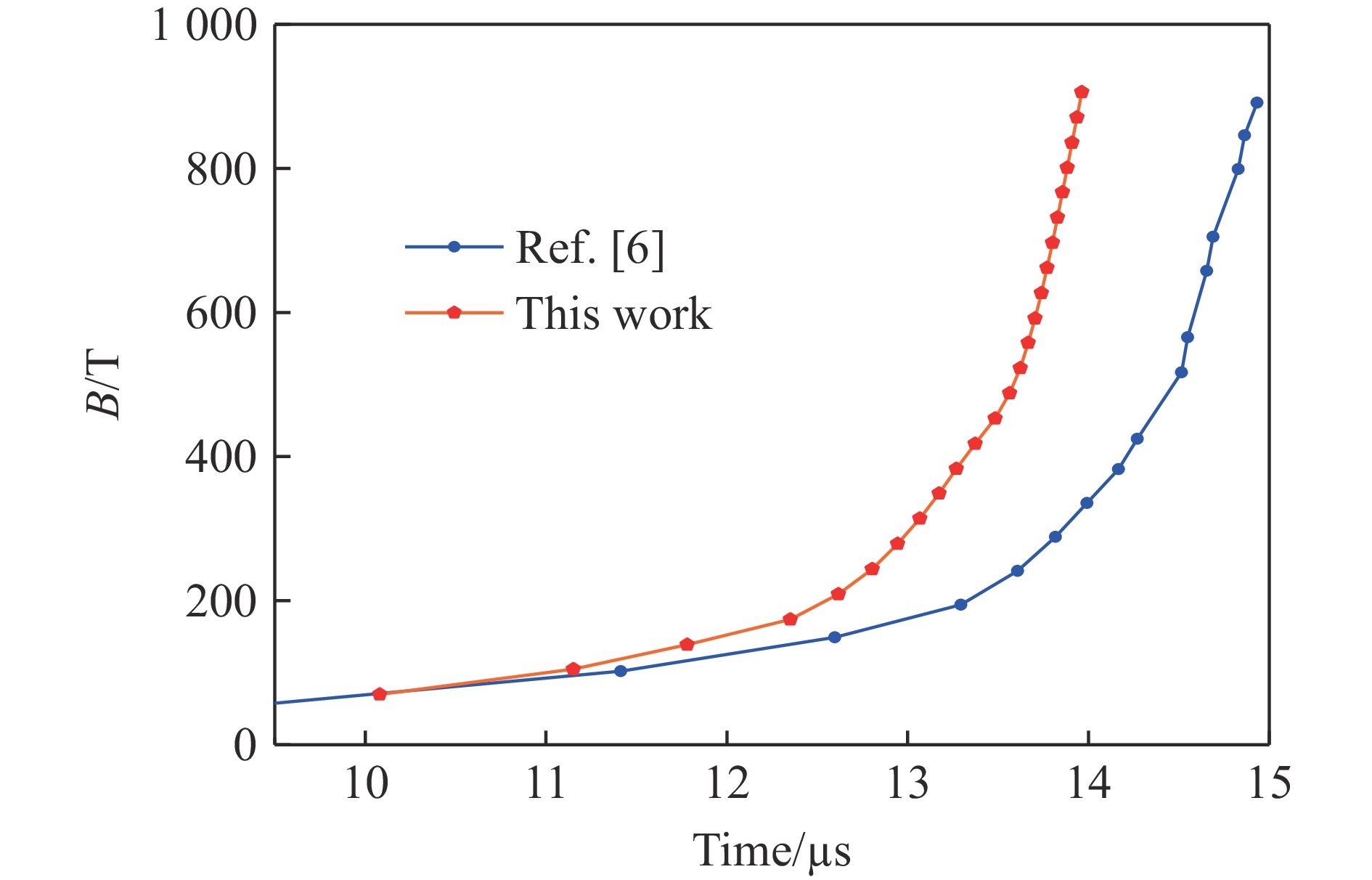
dθ /θ =9,条纹数与磁场值成正比,被测磁场值越高,条纹数越多,其测量不确定度越小;磁光介质长度测量精度可达1 μm。磁场测量不确定度dB/B由Verdet常数的测量不确定度、磁光介质长度精度和法拉第偏转角的精度等3个因素综合得出。最终得到的磁场曲线见图21,CJ-150实现了最高峰值磁场906 T,其数据不确定度为5.35%。文献[17]中同等规模装置的测试结果也见图21,可以看出,CJ-150装置指标与文献[17]的装置水平相当。
利用内置式PDV技术开展了CJ-150加载一维区的实验验证。实验布局见图22,采用二级套筒结构,轴心安置铜管,内径10 mm,外径16.6 mm,壁厚3.3 mm。内置9路PDV探针(分为上、中、下3个平面,间隔20 mm,每个面3路探测,120°均布)。
测量结果见图23。可以看出,9路PDV波形较为吻合,套筒均匀压缩至直径2.5 mm,面积压缩比约16倍。同一时刻3个位置处(间距40 mm)的套筒位移偏差小于5%,套筒加载均匀区不小于40 mm。
3. 结论与分析
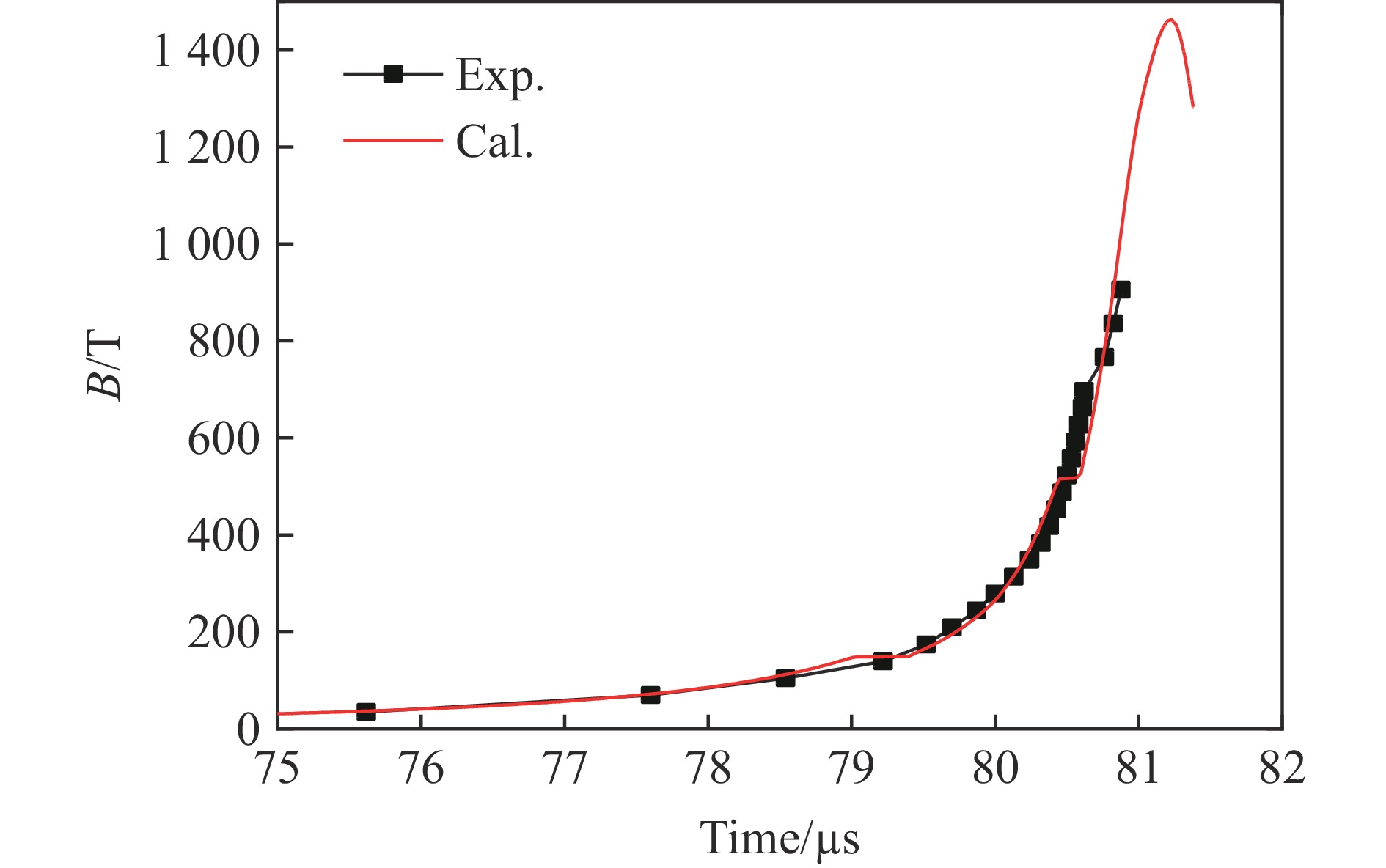
图24是实验数据与SSS-MHD计算结果的对比,可以看出实验数据与计算结果符合良好,但计算峰值与测试峰值差距较大。两者差距较大的原因是由于磁光系统并没有记录到完整的磁压缩过程信号。事实上,在这种极端的爆轰电磁环境中开展磁光测量一直是极为困难的,多级套筒为复合材料,在高速撞击下内表面会发生微喷射现象,这些高速颗粒会严重损伤磁光探针,导致其提前失效。另外,爆轰过程带来的振动等因素也会对磁光测试光路产生影响,最终多方面因素导致磁光测试系统无法记录到完整的磁压缩过程。
实验表明,CJ-150具备千特斯拉量级超强磁场产生能力。利用千特斯拉超强磁场未来在新型聚变点火机制研究、超强磁场下的新物质及新物理现象研究等方面具有广阔的应用前景,对高能密度科学的基础研究具有重要意义。
另外,CJ-150具备对大尺寸样品实现500 GPa以上的准等熵加载能力,可为高压物理研究提供一种全新的极端加载手段。特别要指出的是,这种内爆磁压缩方式特别适合于针对低密度材料如氢氘材料的准等熵压缩。理论研究表明,实现氢氘金属化的压力阈值约为500 GPa,利用CJ-150能够实现对氢氘材料500 GPa以上准等熵压缩,并且具有样品体积大、容易密封等技术优势,这对于开展氢氘金属化相变及超高压物性研究是极为有利的。
-
表 1 混凝土本构模型参数
Table 1. Parameters required in the concrete model
a1 a2/Pa−1 d1 d2 c1 c2 εfrac 0.5876 0.25 × 10–3 0.04 1.5 3 6.93 0.015 Test G/g W/g L/mm R/mm Hw/mm UE-01 80 2.2 100 20 600 UE-02 50 1.1 100 100 600 UE-03 50 1.1 300 300 600 表 3 上游坝面损伤面积占比θ和损伤区域的平均损伤δ
Table 3. The damage area ratio θ and the average damage δ of the dam upstream face
R/mm θ δ L=50 mm L=100 mm L=150 mm L=200 mm L=250 mm L=50 mm L=100 mm L=150 mm L=200 mm L=250 mm 10 0.8159 0.8308 0.8597 0.8569 0.8490 0.2451 0.2519 0.2268 0.2310 0.2172 20 0.8272 0.8332 0.8718 0.8675 0.8653 0.2569 0.2733 0.2512 0.2527 0.2263 30 0.8478 0.8526 0.8664 0.8831 0.8754 0.2608 0.2743 0.2642 0.2602 0.2353 40 0.8195 0.8595 0.8745 0.8817 0.8745 0.2611 0.2786 0.2695 0.2643 0.2395 50 0.8305 0.8793 0.8696 0.8989 0.8718 0.2540 0.2649 0.2698 0.2523 0.2362 60 0.8319 0.8415 0.8675 0.8933 0.8771 0.2616 0.2612 0.2673 0.2453 0.2353 70 0.8416 0.8748 0.8785 0.8936 0.8765 0.2453 0.2510 0.2494 0.2309 0.2296 80 0.8418 0.8588 0.8898 0.8668 0.8831 0.2335 0.2443 0.2363 0.1996 0.2156 90 0.8415 0.8664 0.8628 0.8992 0.8873 0.2238 0.2280 0.2265 0.2166 0.2104 100 0.8390 0.8727 0.8693 0.8914 0.8872 0.2086 0.2210 0.2145 0.2098 0.2002 -
[1] WANG G H, ZHANG S R, KONG Y, et al. Comparative study of the dynamic response of concrete gravity dams subjected to underwater and air explosions [J]. Journal of Performance of Constructed Facilities, 2015, 29(4): 04014092. DOI: 10.1061/(ASCE)CF.1943-5509.0000589. [2] WANG G H, LU W B, YANG G D, et al. A state-of-the-art review on blast resistance and protection of high dams to blast loads [J]. International Journal of Impact Engineering, 2020, 139: 103529. DOI: 10.1016/j.ijimpeng.2020.103529. [3] 陈叶青, 吕林梅, 汪剑辉, 等. 爆炸冲击荷载下的大坝抗爆性能及防护研究进展 [J]. 防护工程, 2021, 43(2): 1–10. DOI: 10.3969/j.issn.1674-1854.2021.02.001.CHEN Y Q, LV L M, WANG J H, et al. Review of blast resistance performance and protection of dams under blast shock load [J]. Protective Engineering, 2021, 43(2): 1–10. DOI: 10.3969/j.issn.1674-1854.2021.02.001. [4] 陈叶青, 吕林梅, 王高辉, 等. 大坝抗爆性能评估研究进展 [J]. 土木工程学报, 2021, 54(10): 9–19. DOI: 10.15951/j.tmgcxb.2021.10.003.CHEN Y Q, LV L M, WANG G H, et al. Review on the blast-resistance performance evaluation of dams [J]. China Civil Engineering Journal, 2021, 54(10): 9–19. DOI: 10.15951/j.tmgcxb.2021.10.003. [5] VANADIT-ELLIS W, DAVIS L K. Physical modeling of concrete gravity dam vulnerability to explosions [C]//2010 International Water Side Security Conference. Carrara: IEEE, 2010: 1-11. DOI: 10.1109/WSSC.2010.5730291. [6] HUANG X P, HU J, ZHANG X D, et al. Bending failure of a concrete gravity dam subjected to underwater explosion [J]. Journal of Zhejiang University-Science A, 2020, 21(12): 976–991. DOI: 10.1631/jzus.A2000194. [7] HUANG X P, KONG X Z, HU J, et al. Failure modes of concrete gravity dam subjected to near-field underwater explosion: centrifuge test and numerical simulation [J]. Engineering Failure Analysis, 2022, 137: 106243. DOI: 10.1016/j.engfailanal.2022.106243. [8] HUANG X P, HU J, ZHANG X D, et al. Effect of bubble pulse on concrete gravity dam subjected to underwater explosion: centrifuge test and numerical simulation [J]. Ocean Engineering, 2022, 243: 110291. DOI: 10.1016/j.oceaneng.2021.110291. [9] ZHANG S R, WANG G H, WANG C, et al. Numerical simulation of failure modes of concrete gravity dams subjected to underwater explosion [J]. Engineering Failure Analysis, 2014, 36: 49–64. [10] 王高辉, 张社荣, 卢文波, 等. 水下爆炸冲击荷载下混凝土重力坝的破坏效应 [J]. 水利学报, 2015, 46(6): 723–731. DOI: 10.13243/j.cnki.slxb.20140908.WANG G H, ZHANG S R, LU W B, et al. Damage effects of concrete gravity dams subjected to underwater explosion [J]. Journal of Hydraulic Engineering, 2015, 46(6): 723–731. DOI: 10.13243/j.cnki.slxb.20140908. [11] LI Q, WANG G H, LU W B, et al. Influence of reservoir water levels on the protective performance of concrete gravity dams subjected to underwater explosions [J]. Journal of Structural Engineering, 2018, 144(9): 04018143. [12] WANG C, WEI P Y, WANG X H, et al. Blast-resistance and damage evaluation of concrete gravity dam exposed to underwater explosion: considering the initial stress field [J]. KSCE Journal of Civil Engineering, 2021, 25(8): 2922–2935. DOI: 10.1007/s12205-021-1650-0. [13] ZHANG Q L, HU Y G, LIU M S, et al. Role of negative pressure in structural responses of gravity dams to underwater explosion loadings: the need to consider local cavitation [J]. Engineering Failure Analysis, 2021, 122: 105270. DOI: 10.1016/j.engfailanal.2021.105270. [14] SHU Y Z, WANG G H, LU W B, et al. Damage characteristics and failure modes of concrete gravity dams subjected to penetration and explosion [J]. Engineering Failure Analysis, 2022, 134: 106030. DOI: 10.1016/j.engfailanal.2022.106030. [15] ZHU J G, CHEN Y Q, LYU L. Failure analysis for concrete gravity dam subjected to underwater explosion: a comparative study [J]. Engineering Failure Analysis, 2022, 134: 106052. DOI: 10.1016/j.engfailanal.2022.106052. [16] REN X D, SHAO Y. Numerical investigation on damage of concrete gravity dam during noncontact underwater explosion [J]. Journal of Performance of Constructed Facilities, 2019, 33(6): 04019066. DOI: 10.1061/(ASCE)CF.1943-5509.0001332. [17] 吕林梅, 陈叶青, 魏晓丽, 等. 基于毁伤面积率指标的混凝土重力坝毁伤评估方法研究 [J]. 防护工程, 2020, 42(2): 28–32. DOI: 10.3969/j.issn.1674-1854.2020.02.005.LV L M, CHEN Y Q, WEI X L, et al. Study of damage assessment method of concrete gravity dam based on damage-area ratio index [J]. Protective Engineering, 2020, 42(2): 28–32. DOI: 10.3969/j.issn.1674-1854.2020.02.005. [18] 李麒, 王高辉, 卢文波, 等. 混凝土重力坝水下爆炸毁伤快速识别方法研究 [J]. 振动与冲击, 2020, 39(24): 46–53,62. DOI: 10.13465/j.cnki.jvs.2020.24.007.LI Q, WANG G H, LU W B, et al. A rapid identification method for underwater explosion damage of a concrete gravity dam [J]. Journal of Vibration and Shock, 2020, 39(24): 46–53,62. DOI: 10.13465/j.cnki.jvs.2020.24.007. [19] WANG X H, ZHANG S R, WANG C, et al. Blast-induced damage and evaluation method of concrete gravity dam subjected to near-field underwater explosion [J]. Engineering Structures, 2020, 209: 109996. DOI: 10.1016/j.engstruct.2019.109996. [20] 张社荣, 王高辉, 王超, 等. 水下爆炸冲击荷载作用下混凝土重力坝的破坏模式 [J]. 爆炸与冲击, 2012, 32(5): 501–507. DOI: 10.11883/1001-1455(2012)05-0501-07.ZHANG S R, WANG G H, WANG C, et al. Failure mode analysis of concrete gravity dam subjected to underwater explosion [J]. Explosion and Shock Waves, 2012, 32(5): 501–507. DOI: 10.11883/1001-1455(2012)05-0501-07. [21] 张启灵, 李端有, 李波. 水下爆炸冲击作用下重力坝的损伤发展及破坏模式 [J]. 爆炸与冲击, 2012, 32(6): 609–615. DOI: 10.11883/1001-1455(2012)06-0609-07.ZHANG Q L, LI D Y, LI B. Damage propagation and failure mode of gravity dam subjected to underwater explosion [J]. Explosion and Shock Waves, 2012, 32(6): 609–615. DOI: 10.11883/1001-1455(2012)06-0609-07. [22] WANG G H, ZHANG S R. Damage prediction of concrete gravity dams subjected to underwater explosion shock loading [J]. Engineering Failure Analysis, 2014, 39: 72–91. DOI: 10.1016/j.engfailanal.2014.01.018. [23] HUANG X P, KONG X Z, HU J, et al. The influence of free water content on ballistic performances of concrete targets [J]. International Journal of Impact Engineering, 2020, 139: 103530. DOI: 10.1016/j.ijimpeng.2020.103530. [24] ROSSI P. A physical phenomenon which can explain the mechanical behaviour of concrete under high strain rates [J]. Materials and Structures, 1991, 24(6): 422–424. DOI: 10.1007/BF02472015. [25] HUANG X P, KONG X Z, CHEN Z Y, et al. Equation of state for saturated concrete: a mesoscopic study [J]. International Journal of Impact Engineering, 2020, 144: 103669. DOI: 10.1016/j.ijimpeng.2020.103669. [26] KONG X Z, FANG Q, CHEN L, et al. A new material model for concrete subjected to intense dynamic loadings [J]. International Journal of Impact Engineering, 2018, 120: 60–78. DOI: 10.1016/j.ijimpeng.2018.05.006. [27] ZHAO F Q, WEN H M. Effect of free water content on the penetration of concrete [J]. International Journal of Impact Engineering, 2018, 121: 180–190. DOI: 10.1016/j.ijimpeng.2018.06.007. [28] XU H, WEN H M. Semi-empirical equations for the dynamic strength enhancement of concrete-like materials [J]. International Journal of Impact Engineering, 2013, 60: 76–81. DOI: 10.1016/j.ijimpeng.2013.04.005. [29] 黄谢平, 孔祥振, 陈祖煜, 等. 近水面、库中、库底水下爆炸荷载作用下混凝土重力坝的破坏模式对比 [J]. 土木工程学报, 2023, 56(3): 116–128. DOI: 10.15951/j.tmgcxb.21121206.HUANG X P, KONG X Z, CHEN Z Y, et al. Comparison of failure modes of concrete gravity dams by underwater explosion loads near the water free surface, the middle, and the bottom of the reservoir [J]. China Civil Engineering Journal, 2023, 56(3): 116–128. DOI: 10.15951/j.tmgcxb.21121206. [30] GEERS T L, HUNTER K S. An integrated wave-effects model for an underwater explosion bubble [J]. The Journal of the Acoustical Society of America, 2002, 111(4): 1584–1601. DOI: 10.1121/1.1458590. 期刊类型引用(1)
1. 柯渊. 基于音频混音技术的影视声音制作方法. 电声技术. 2025(04): 96-98 .  百度学术
百度学术其他类型引用(0)
-








 下载:
下载:



































 下载:
下载:















 百度学术
百度学术