A two-step iterative method for damage calculation of a ship hullsubjected to underwater close-up non-contact explosion
-
摘要: 船体水下近距非接触爆炸产生的破口计算过程复杂,涉及船体板架、武器装药和爆距方位等诸多因素,工程实践中通常应用经验公式求解。基于舰船遭受定向型战斗部攻击、毁伤面近似垂直于毁伤轴和爆炸过程瞬时发生满足近似能量守恒基本条件,根据爆炸冲击波初始动能与爆炸作用区域结构塑性变形能等量传递的假设,给出了计算方法。考虑了附着加强筋的船体壳板等效厚度对抵御冲击波毁伤的影响,运用爆炸冲击波作用下船体壳板产生的极限应变超过板材动态极限应变导致壳板开裂这一基本原理,设计了两步迭代法计算流程,给出了简捷易用的迭代计算表格。针对4种典型装药当量冲击波作用下,5~20 m长度舱段,11 m以内爆炸距离,6 和8 mm这2种典型厚度船体壳板遭受爆炸冲击受损情况进行了768组数据计算。引入平面拟合方程,通过判断截平面相似度分析,给出了计算方法的适用性判据,探讨了计算参数的适用范围,以保证两步迭代法能够客观反映水下近距非接触爆炸的实际破坏效果。结合经验公式计算结果和破损舰船受损实测数据,对该方法进行了检验,实践表明:两步迭代法易于工程实践且具有较好的准确性。Abstract: The calculation of the break of ship hull caused by underwater close-range non-contact explosion is a complex process, involving many factors such as the hull frame, weapon charge, explosion distance and orientation, etc., so empirical formulas are usually used in engineering design. If the ship is attacked by a directional warhead, it is usually assumed that the damage surface is approximately perpendicular to the damage axis, and the explosion process instantaneously meets the basic condition on approximate energy conservation, then the calculation method is proposed according to the assumption that the initial kinetic energy of the explosion shock wave is equally transmitted to the plastic deformation energy of the structure in the explosion action area. Considering the effect of the equivalent thickness of the hull shell-plate attached with stiffeners on the resistance to shock wave damage, and using the fundamental principle that cracking of the shell plate will take place when the ultimate strain of the hull plate under the action of explosion shock wave exceeds the dynamic ultimate strain of the plate, the calculation flow of the two-step iterative method is designed, and a simple and easy-to-use iterative calculation table is given. 768 sets of data are calculated for the damage of hull shell-plates with the typical thicknesses of 6 mm and 8 mm under the action of four typical charge equivalent shock waves, with an explosion distance within 11 m, acting on a compartment with 5-20 m span. By introducing the plane fitting equation, the applicability criterion of the calculation method is given by judging the similarity analysis of the section plane, and the valid range of the calculation parameters is discussed to ensure that the two-step iteration method can objectively reflect the actual damage effect of the underwater short-range non-contact explosion. Combined with the calculation results of empirical formulas and the measured data of damaged ships, the method is verified. The practice shows that the two-step iterative method is easy for engineering practice and has good accuracy.
-
Key words:
- warship survivability /
- hull damage /
- underwater close-up explosion /
- breach calculation
-
炸药在水中爆炸所产生的冲击波和气泡脉动压力是船体结构受损破坏的主要因素,船体产生的破口大小是衡量炸药水中爆炸毁伤效果的重要指标。近距水中爆炸对舰船结构破坏尤其严重,严重威胁舰船生命力,破口尺寸的确定是损管决策过程中损伤预测和预案制定的基础,针对破口计算方法的研究受到国内外学者们的广泛关注[1-4]。近距水下爆炸冲击波破坏作用占主导地位,炸药水中爆炸产生的能量主要以冲击波方式作用于船体,破口计算可通过爆炸冲击波能量与船体壳板结构毁伤变形能近似相等的关系,采取有限步数的迭代计算,设计工程上易于操作的计算方法。
爆炸破口的计算方法主要包括经验公式法、试验归纳法和数值模拟验证法等3类方法。经验公式简捷易用,是最普遍的方法。吉田隆[1]针对二次大战期间日本舰艇遭受爆炸损毁情况分析得出舰船舷板在水下接触爆炸条件下破口半径的经验公式,该方法源于大量战损数据,随着战后炸药性能提升和战损舰艇案例大量减少,运用经验公式给出计算结果准确性偏低。20世纪90年代以后,学者们针对水下爆炸损伤计算问题开展了大量试验研究,试验归纳法得到了快速发展,朱锡等[2]对船板结构模型进行了水下接触爆炸试验,给出了破口形式和引入加强筋影响的破口长度估算公式;在随后的研究中,该团队结合船体板架塑性动力响应及破口研究[3],给出了板架模型等效厚度的计算方法;牟金磊等[4]对近场非接触爆炸损伤破口计算问题开展了数值模拟和试验研究,进一步给出了基于结构塑性变形能等量传递的破口计算理论方法,试验分析结果可信度高,但研究过程也相对复杂,试验组织实施难度较大。近20年来,随着计算能力提升,数值模拟验证法得到了广泛应用,毁伤数值模拟评估软件与经验公式相结合,基于数值模拟结果验证并修正经验公式,进一步运用公式解决实际工程问题成为爆炸破口计算的新思路。陈海龙等[5]针对舰船板壳毁伤效果问题总结了破口计算典型经验公式,结合数值模拟对经验公式的计算结果给出误差比对,运用修正后的经验公式开展了爆炸破口评估。2000年10月,美军伯克级驱逐舰科尔号在也门遭受恐怖袭击,船体中部严重受损,破口长度达12 m,此后美军对舰艇战损评估高度重视,以DDG81为研究对象开展了一系列水下近距爆炸数值模拟和试验研究[6-8],分析了水下爆炸战损评估数学模型,研究了遭受水中爆炸冲击的基本形态,给出了爆炸试验冲击响应试验数据,对破口损伤预测进一步丰富和补充。上述3类计算方法可归纳为基于输入输出模式的破口计算公式法,基本原理是寻求爆炸冲击、外界环境与破口大小的对应关系。
船体水中爆炸损伤破口计算涉及到船体板架、武器装药、爆距方位等诸多参数,且不同的计算公式受适用条件和已知参数限制,实际运用中仍有困难,且部分计算方法须求解超越方程,需要专用软件和高性能计算设备辅助完成,对舰船损管决策来说,计算过程增大了指挥决策者运用难度。因此,本文中,根据爆炸冲击波初始动能与爆炸作用区域结构塑性变形能等量传递的假设,并考虑附着加强筋的船体壳板等效厚度对抵御冲击波毁伤的影响,运用爆炸冲击波作用下船体壳板产生的极限应变超过板材动态极限应变导致壳板开裂这一基本原理,设计两步迭代法计算流程,该方法可快速完成舰船水下爆炸损伤破口计算等现实问题。
1. 水下近距爆炸对船体的损伤机理
1.1 结构变形能等量传递假设
针对船体水下爆炸破坏研究问题,接触爆炸和非接触爆炸在破坏船体结构的原理上有差别:水下接触爆炸爆轰波产生的高温高压气团直接作用于船板,冲塞穿孔使船板撕裂产生破口;而水下非接触爆炸则是爆炸产生的冲击波驱动高压水流产生局部冲塞穿孔效应,并使船板撕裂产生破口。武器攻击条件下,水下非接触爆炸发生概率远大于水下接触爆炸发生概率。对于水下非接触爆炸,牟金磊等[4]和Bogdan等[8]均给出了其破坏效应在产生机理上近似于接触爆炸,结构材料在冲击波近距离作用下发生塑性变形,直至开裂形成破口。牟金磊等[4]认为这个过程非常短暂,爆炸冲击波能转化为结构破坏初始动能。本文中,对近年来经验计算公式进行系统分析,结合文献[4,9-10]等炸药爆炸初始动能计算方法,运用能量守恒原理,进一步探讨冲击波能转化为结构初始动能的合理性假设和适用条件。
1.2 爆炸区域冲击波初始动能与计算作用动能
图1给出了炸点与船舶舱室之间的位置关系。根据炸药爆炸相似率计算方法,非接触爆炸对船体结构的破坏作用与爆炸冲击波峰值压力有关[11],冲击波作用过程为[9,12]:
p(t)=pme−t−t0θ (1) pm=kp(W1/3R)αp (2) θ=kθW1/3(W1/3R)αθ (3) 式中:pm为水中冲击波波阵面峰值压力,kg/cm2;W为TNT装药质量,kg;R为到爆心的距离,m;kp、αp、kθ和αθ为与炸药类别有关的相似常数;t0表示水中冲击波随时间按指数规律衰减的时间常数,可近似表示为R/c0,c0表示静水中声速,约为1500 m/s。
设爆炸发生时初始能量密度为ES,则有[10]:
ES=1ρ0c0∫tt0p(t)2dt (4) 将式(1)代入式(4)得:
ES=1ρ0c0∫tt0(pme−t−t0θ)2dt=p2mθ2ρ0c0e(t−t0θ)2 (5) 近距离非接触爆炸冲击波到达船体结构所需的时间很短暂,即
limt→t0e(t−t0θ)2=1 ,同时,应当注意到式(2)中单位为kg/cm2,计算过程中应与声阻抗ρ0c0的单位保持一致。进一步将式(2)~(3)代入式(5),爆炸初始能量密度ES可表示为:ES=p2mθ2ρ0c0=1×1082ρ0c0k2pW23αpR2αpkθW13Wαθ3Rαθ=1×108k2pkθ2ρ0c0W13(W13R)2αp+αθ (6) 式中:ρ0c0为水中的声阻抗率,kg/(m2·s)。
李金河等[10]给出了部分炸药的相似常数,如表1所示。由式(6)可进一步整理得出文献[4、10]中给出的炸药水中爆炸相似常数计算式:
KE = 1×108k2pkθ2ρ0c0 (7) αE=2αp+αθ (8) ES=KEW13(W13R)αE (9) 式中:KE为爆炸水中相似常数,αE为相似经验常数。
如1.1节所述,忽略作用时间影响,水下近场非接触爆炸作用于船壳板的能量可近似认为与爆炸初始冲击波能相等,式(9)给出了爆炸初始能量密度的计算方法。如图2(a)所示,爆炸冲击波贯穿撕裂壳板后,断裂后的壳板及其附属加强结构与舱室边界强力肋骨构件形成悬臂结构,在爆炸冲击波持续作用下,壳板及其附属加强结构持续弯曲发生塑性变形并开裂,船壳板结构塑性变形能不断抵消爆炸初始动能[13]。由于这个过程瞬时完成,可据此建立爆炸作用区域塑性变形(断裂)能与爆炸初始动能等量关系,基于等量关系,可对壳板的变形角度φ进行计算。如图2所示,假设爆炸初期局部高压冲击破口部位在O点,壳板内凹陷变形φ角度后,壳板结构塑性变形和开裂过程中,与破口点O距离为r0的观测点A0因内卷边变形产生dr位移至A1位置,此时与破口点O距离为r1,E(r)为距离爆心距离为r位置处的爆炸初始能量密度,爆炸冲击波总做功EK可近似表示为:
EK=∫L02πrE(r)dr=∫L02πrKEW13(W13√R2+r2)αEdr (10) 根据炸药水下爆炸相似律,考虑到相似常数与
W13 具有相关性,不妨令W13 等于1个计算当量单位,那么式(10)可求解积分得:EK=2πKEW13∫L0(1√R2+r2)αErdr=2πKEW13(L2+R2)(1−αE2)−R(2−αE)2−αE (11) 由于爆炸过程时间极短,爆炸冲击波初始能量与破坏船壳板过程近似能量守恒,船壳板产生变形开裂所需能量分别是壳板开裂过程中的径向塑性变形能和环向塑性变形能。牟金磊等[4]作者给出船壳板结构件变形开裂塑性变形能计算公式,不妨进一步定义该能量为爆破区计算作用动能EA:
EA=π2σh2Lφ+πσL2h−πσL2hcosφ+π2σh2Ltanφ[1−1−cosφcosφln(1−cosφ)] (12) 式中:σ为钢板材料发生塑性变形的屈服强度,MPa;h为船壳板厚度,m;L为两道水密隔墙之间的半宽距离,m。
1.3 基于动态极限应变相等的破口延展长度计算方法
破口形成过程中,冲击波作用下破坏的船壳板不断内卷开裂。如图3所示,阴影区域为任取环带sr,船壳板环向内卷边弯曲和沿周长方向拉伸产生动态极限应变,冲击波作用下,环带部位在区域A~E均出现应变超出船壳板极限应变的情况,也就是局部变形量超出了材料允许塑性变形量,形成Ⅰ~Ⅴ花瓣开裂,设船壳板材极限应变为εm,对于环带上任意一点产生的动态极限应变ε,当ε>εm时,环带将出现超出屈服强度发生开裂的情况,按照金属材料屈服强度测量时条件参数,εm取值0.2%。值得注意的是,如图2(a)所示。局部应变量值与船壳板厚度h、壳板跨度2L和破损区域内凹陷角度φ有关,假设环带上破损产生的裂口半宽为
r ,文献[4]中给出了环带上的点产生动态极限应变的计算公式:ε=(Lr−1)(1−cosφ)+hsinφ2L−2(L−r)cosφ (13) 对破口尺寸度量而言,船壳板厚度与破损规模相关,对于普通船体板架结构,通常附有肋骨和纵桁,统称加强筋,计算过程中,这些非强力结构部件可采取折算方法等效为船壳板厚度,式(12)~(13)中的船壳板厚度变量h通常取值等效后的船壳板厚度。文献[3]给出了板架结构转换等效厚度船壳板计算方法,设舷侧目标区域板架结构长为2L,高度为H,其上附着有纵向骨架2n根,骨架横截面面积Fi,横向骨架m根,骨架横截面面积Fj,则等效后的船壳板厚度为
ˉh ,即:ˉh=LHh+n∑i=1FiL+m∑j=1FjHLH (14) 通常情况下,式(14)可进一步简化为
ˉh=ˆAh ,其中ˆA 为安全系数,取值6~10。由式(13)~(14)可求得目标区域任意点产生动态极限应变的数值。综上所述,水下爆炸产生的船体破口计算涉及因素很多,也很复杂,确定破坏半径实际上需要求解超越方程,运用直接公式法是无法完成的,近场水下爆炸破口计算核心是求得目标区域裂口延展的最大长度
r ,这需要联合式(11)~(14)求解超越方程,计算过程本身难度很大。从保证计算精度并满足工程应用的实际出发,本文探讨一种迭代试算方法,求得近似解。2. 两步迭代法
2.1 计算步骤与程序表
水中爆炸破口计算可运用前文所述能量守恒和应变相等2个关键假设设计算法,通过迭代试算求得破口尺寸,这种方法可概括为2个基本步骤,具体如下。
步骤(1):运用水中爆炸冲击波初始动能EK与目标爆破区计算作用动能EA相等的能量守恒原理,试算变形凹陷角度φ。
步骤(2):瞄准应变相等这一关键节点条件,计算船壳板受到爆炸冲击产生的应变ε,并将其与开裂条件ε>εm比较,试算破口半宽距离r。
其他参数根据实际设定,两步完成后,试算结果r可初步作为破损区域的半宽长度。为了方便工程运用,设计了计算表格,如表2所示。
表 2 水下爆炸船体破口计算表Table 2. Calculation of hull break caused by underwater explosion序号 项目 物理量 数值 说明 操作方法 1 装药量 W/kg 267 TNT当量 需要输入 2 爆炸产生的破口半径(半宽) R/m 2.71 计算结果,这个半宽数值对应中间完全撕裂破洞,实际破坏要比这个计算数值略小,这个结果越靠近炸点,与实际结果越接近。 输入半宽值试算,结果显示在C区域 3 炸药水中爆炸相似常数KE KE/(KPa∙m) 84.4 经验常数,根据实际查表由式(7)计算/采用TNT装药,代入公式时乘103 不输入 4 炸药水中爆炸相似常数αE αE 2.04 经验常数,根据实际查表由式(8)计算/采用TNT装药 不输入 5 两道水密隔墙之间的半宽距离 L/m 8.00 整个舱段长度为2L(m) 按水密段实际宽度一半输入 6 船壳板原始厚度 H/mm 8.000 直接输入不必转换成单位m 按实际输入 7 船壳板等效厚度(考虑加强筋) ˉh/m 0.064 按实际板厚度8倍计算 不输入 8 爆距 R/m 3.00 炸点距离舷板垂直距离 按实际输入,接触爆炸可按0.01输入 9 爆炸区域冲击波初始动能 EK/(kg∙m2∙s−2) 3349689 A区域:炸药爆炸冲击波初始动能计算值 不输入,用与B区域结果比对 10 钢板强度 σ/MPa 235.00 直接输入不必转换成Pa,对于Q235钢板,此数值是235 MPa,船板一般取值235~440 MPa,代入公式时乘106 按实际输入 11 船壳板变形内凹陷试算角度 Φ/(°) 2.27 从0°~90°取数值试算 输入角度试算,结果显示在B区域 12 内凹陷角度转换弧度制 Φ/rad 0.04 此处是中间转换数值 不输入 13 爆破区计算作用动能 EA/(kg∙m2∙s−2) 3332283 B区域:爆炸冲击波做功近似转化为船壳板塑性变形能 不输入,用与A区域数值比对,直至EK=EA时止 14 动态极限应变 εm/% 0.2 钢板的极限应变是0.2% 不输入 15 计算极限应变 ε/% 0.2 C区域:此处计算得到临界极限应变 不输入 注:*以上计算方法适用于舱室跨度大于7 m,爆距小于9 m。 2.2 破口计算流程与方法
表格使用方法和计算过程简要说明如下。
(1)计算准备。输入基本数据。
序号1:装药量,输入装药重量,例如MK48鱼雷装药TNT当量是267 kg;
序号5:水密段隔墙之间的半宽距离,例如,某船水密段长度16 m,半宽距离8 m;
序号6:舷板厚度,例如一般的船壳板厚度8 mm,或者6 mm等;
序号8:爆距,炸点距离舷板的垂直距离,可以是0.01~9 m以内的任意数值。
(2)计算第1步。通过爆炸区域冲击波初始动能(序号9)和爆破区计算作用动能(序号13)比对,试算船壳板变形内凹陷角度。
试算过程如下:输入已知参数之后,通过不断改变变形内凹陷角度(序号11)的数值(角度值,比如10°、20°、30°,等,0~90°之间任意数值),计算爆破区计算作用动能(序号13),使得B区爆破区计算作用动能数值与A区爆炸区域冲击波初始动能(序号9)接近或者相等。
(3)计算第2步。通过不断改变爆炸产生的破口半径(序号2),对船壳板动态极限应变(序号14)和计算极限应变(序号15)结果进行比对,试算爆炸产生的破口半径,并确定最终结果。
3. 算法的适用性
水下爆炸毁伤舰体主要依靠弹药爆炸时产生的冲击波压力和高速飞散的弹药破片冲击作用摧毁舰体结构。当前水下攻击武器战斗部多以聚能定向型战斗部为主,考虑到弹目交汇状态对计算结果的影响,本计算方法遵循的基本假设条件有:(1)遭受定向型战斗部攻击;(2)爆距满足能量守恒基本原理使用条件;(3)毁伤面近似垂直于毁伤轴。以此为基础设计的两步迭代法计算表格,能够较为方便地用来开展船体水下爆炸破口预测计算。
值得注意的是,该方法适用条件与弹药战斗部爆炸形态、船体结构强力构件位置和船壳板板架结构以及炸点和目标船体壳板之间的距离有关。将通过数据分析给出计算方法适用性判据。
3.1 典型参数船壳板水下非接触爆炸破口计算结果
船体板架在水下爆炸冲击过程中会遭到破坏,但是诸如水密隔墙等强力结构能够在一定程度上限制壳板破损范围进一步扩大。考虑破损计算问题的一般性特点,从确定的炸药爆炸冲击形成破口这一角度分析,水下爆炸对船体的破坏作用与船板当量厚度、水密隔墙宽度和爆距都有紧密关系。在运用两步迭代法计算的基础上,可通过三坐标曲面表现这种量化关系,合理展现某一水下爆炸工况对船体结构的破坏效果。
本文中对4种典型装药TNT当量的爆炸结果进行了计算,计算过程中,在水下爆炸TNT当量确定的情况下,不同船板厚度的船体受损区域所在水密隔段半宽和炸点爆距均发生变化,爆炸形成的舱室破口数据结果可构成一个空间曲面。此处,设定船体受损区域舱段宽度5~20 m,分别选取6和8 mm船板典型厚度,安全系数取8计算当量厚度,对水下近距爆炸设置取值区间为1~11 m,计算结果曲面如图4~5所示。
3.2 数据分析与两步迭代法适用性判据
如3.1节所述,计算基于能量守恒原理,流程方法虽清晰简明,但须探讨计算参数的适用范围,以保证两步迭代法能够客观反映水下近距非接触爆炸的实际破坏效果。
由参考文献[2-6]可知,近距非接触爆炸对船体破坏效应相关理论已是学术界普遍认可的基本理论,两步迭代法计算结果与上述文献算例进行比对,结果令人满意。作为一种适于工程人员快捷使用的计算工具,对768组计算数据结果进行分析,不难发现,运用该方法开展船体受损预测时,方法适用的边界条件值得关注。如图4~5中计算结果所构成的三维空间曲面,对指定TNT装药量和舷板厚度,不妨定义主隔墙半宽为x方向,爆距为y方向,破口半宽为z方向,任取x方向或者y方向上的截平面,基于截平面与曲面交线,可以得到基于交线点集张成的平面型数据拟合方程,记为z=y0+ax+by。令f(z1, z2)=1−[(y02−y01)2+(a2−a1)2+(b2−b1)2]1/2表示任意2个平面型数据拟合方程的相似度。
如图6(a)所示,TNT当量为350 kg,舷板厚度为6 mm,当y=9 m和y=11 m时,平面型数据拟合方程分别可表示为:
z6−350−y9.0=2.9505+0.2422x−0.4037y (15) z6−350−y11.0=2.8756+0.2221x−0.3491y (16) 式中:拟合方程记录符号按照舷板厚度-装药当量-截面位置格式标记。
如图6(b)所示,TNT当量为350 kg,舷板厚度为8 mm,当y=9 m和y=11 m时,平面型数据拟合方程分别可表示为:
z8−350−y9.0=2.8797+0.1787x−0.3884y (17) z8−350−y11.0=2.7417+0.1648x−0.3271y (18) 对于爆距y=9 m,同为TNT装药当量为350 kg,计算船体舷板厚度分别为6和8 mm的拟合曲面相似度f (z6−350−y9.0, z8−350−y9.0)=0.8538;对于爆距y=11 m,同为TNT当量为350 kg,舷板厚度6和8 mm的拟合曲面相似度f (z6−350−y11.0, z8−350−y11.0)=0.8518。以此类推,可以分析获得装药当量相同的情况下,不同舷板厚度的各种典型情况计算结果灵敏性。当f (z1, z2)≥0.8时,可近似认为z1和z2两个平面型数据拟合方程相似,或者说区分度和灵敏度不高。
考虑到计算结果应用于近距爆炸对船体的破坏作用计算,研究爆距对不同TNT装药当量爆炸冲击结果影响可知:典型船板厚度(当量厚度)的船体破口受损计算结果表明,当爆距超过9 m时,计算结果区分度不高,如表3~4所示,取爆距10 m的情况,计算结果数据信息表明爆距超过9 m时差异性很小,验证了平面型数据拟合方程相似度判据分析法确定边界条件的可行性。
表 3 典型TNT装药当量爆炸破坏10 m跨度隔舱6 mm厚度舷板的计算结果Table 3. Calculation results of equivalent explosion damage of typical TNT charge to 10 m span compartment and 6 mm thick sideboard爆距/m 典型TNT当量条件下的破口半宽/m 350 kg 267 kg 240 kg 170 kg 9 0.92 0.87 0.81 0.76 11 0.66 0.61 0.56 0.51 表 4 典型TNT装药当量爆炸破坏10 m跨度隔舱8 mm厚度舷板的计算结果Table 4. Calculation results of equivalent explosion damage of typical TNT charge to 10 m span compartment and 8 mm thick sideboard爆距/m 典型TNT当量条件下的破口半宽/m 350 kg 267 kg 240 kg 170 kg 9 0.68 0.63 0.63 0.53 11 0.48 0.43 0.43 0.39 进一步,对于水密隔舱跨度x=5.0, 6.0, 7.0 m的情形,采用相同的分析方法,求解与之对应的平面型数据拟合方程,分析装药当量相同情况下,不同舷板厚度计算结果的灵敏性。仍然以TNT当量350 kg为例,f (z6−350−x3.5, z8−350−x3.5)=0.7661,f (z6−350−x3.0, z8−350−x3.0)=0.8111,f (z6−350−x2.5, z8−350−x2.5)=0.8518,此处,仍然使用f (z1, z2)≥0.8作为灵敏度判别条件。
通过对不同TNT装药当量爆炸冲击典型船板厚度(当量厚度)的船体破口受损计算结果进一步分析可知,当水密隔舱跨度小于7 m时,计算结果区分度和灵敏度不高,如表5~6所示。取爆距1 m的情况,实际计算结果表明,各种典型装药当量数据在跨度7 m以下差异性很小,相似度判据分析法确定边界条件依然适用。
表 5 7 m以内跨度6 mm厚度舷板隔舱典型TNT装药当量爆距1 m破坏的计算结果Table 5. Calculated damage results of typical TNT charge equivalent explosive distance of 1 m with span of 6 mm and the thickness of the bulkhead compartment within 7 m隔舱跨度/m 典型TNT当量条件下的破口半宽/m 350 kg 267 kg 240 kg 170 kg 7 3.15 3.11 3.08 3.03 6 2.79 2.76 2.75 2.71 5 2.41 2.40 2.39 2.36 表 6 7 m以内跨度8 mm厚度舷板隔舱典型TNT装药当量爆距1 m破坏的计算结果Table 6. Calculated damage results of typical TNT charge equivalent explosive distance of 1 m with span of 8 mm and the thickness of the bulkhead compartment within 7 m隔舱跨度/m 典型TNT当量条件下的破口半宽/m 350 kg 267 kg 240 kg 170 kg 7 3.07 3.01 2.99 2.9 6 2.76 2.72 2.71 2.64 5 2.42 2.39 2.38 2.34 由此,基于3.1节计算结果曲面(图3~5)和本节所进行的计算结果灵敏度分析,给出本文计算方法适用条件判据:爆距不超过9 m,目标区域水密隔舱跨度不小于7 m时,可应用水下近距非接触爆炸损伤计算之两步迭代法实施计算。
4. 案例验证
吉田隆[1]对二次大战期间日本舰艇遭受爆炸损毁情况分析,得到舰船舷板在水下接触爆炸条件下破口半径的经验公式:
Rd=6.4aW0.38/6.4aW0.38hh (19) 式中:Rd为破损半径,m;a为结构特征因数,有加强结构时取0.62;W为装药TNT当量,kg;h为船壳钢板厚度,mm。
该经验公式由统计规律得出,得到了国内外学术界的普遍认可。为检验本文中方法的可行性,将运用该公式和美军舰艇遭受水下爆炸冲击破坏情况实测数据进行验证比对。计算过程中,材料屈服极限假设为235 MPa,材料极限应变取值设定为0.2%。
1988年4月,美军佩里级护卫舰塞缪尔·罗伯茨号在波斯湾触雷,经查明,该水雷型号M-08(苏制),TNT装药当量115 kg,公开资料表明,受损部位燃机舱,长度14 m,爆距1 m,破口宽度约15 ft(5 m)[14]。2000年10月,美军伯克级驱逐舰科尔号在也门遭受恐怖袭击,恐怖分子驾驶小艇于水线处撞击驱逐舰,在0~10 ft(0~3.05 m)处近距离引爆炸药,受损机舱区域在船长方向174~254 ft(53.0~77.4 m)主水密隔墙之间[6],区间长度24.4 m,资料显示装药量约 400~700 lb(181~317 kg),但实际造成的破口长度约40~45 ft(12.19~13.72 m)[7]。针对上述情况,运用经验公式和两步迭代法分别计算,如参考文献[7]附录所述,美舰船板厚度约为8.7 mm,破坏半径的计算结果比较如表7所示。
表 7 运用经验公式和两步迭代法的计算结果比较Table 7. Comparison of calculation results between the empirical formula and the two-step iterative method案例 运用吉田隆经验公式计算破坏半径 运用两步迭代法计算破坏半径 罗伯茨号 2.77 m 2.63 m 科尔号 3.29 m(装药181 kg)/4.07 m(装药317 kg) 5.07 m(装药181 kg)/5.67 m(装药317 kg) 5. 结 论
船体水下遭遇爆炸所产生的破口计算是损管预案制定和损管决策中亟需解决的问题,两步迭代法充分运用了爆炸冲击波初始动能与作用区结构塑性变形能等量传递假设条件,给出了易于计算的工程应用方法,并结合计算数据分析论证了算法适用的边界条件。对过往战损案例验证表明:两步迭代在法用于水下近距非接触爆炸对船体破坏效果预测中,在符合边界条件情况下,可获得相比经验公式计算结果更加贴近实际的结果。
应当指出,水下爆炸破损计算问题本身非常复杂,考虑到爆炸能量耗散和冲击波衰减影响,两步迭代法在一定的水下爆炸冲击范围内能够获得较为满意的计算结果,对于舰船小分舱区域受损和爆距10~20 m等典型情况,笔者将另文研究。
-
表 1 部分典型炸药的相似常数计算参数[10]
Table 1. Calculation parameters of similarity constants of some typical explosives[10]
炸药 kp kθ αp αθ TNT 52.5 0.094 1.13 −0.18 RS211鱼雷装药 59.6 0.101 1.17 −0.23 HLZY-1含铝炸药 49.0 0.132 1.11 −0.22 表 2 水下爆炸船体破口计算表
Table 2. Calculation of hull break caused by underwater explosion
序号 项目 物理量 数值 说明 操作方法 1 装药量 W/kg 267 TNT当量 需要输入 2 爆炸产生的破口半径(半宽) R/m 2.71 计算结果,这个半宽数值对应中间完全撕裂破洞,实际破坏要比这个计算数值略小,这个结果越靠近炸点,与实际结果越接近。 输入半宽值试算,结果显示在C区域 3 炸药水中爆炸相似常数KE KE/(KPa∙m) 84.4 经验常数,根据实际查表由式(7)计算/采用TNT装药,代入公式时乘103 不输入 4 炸药水中爆炸相似常数αE αE 2.04 经验常数,根据实际查表由式(8)计算/采用TNT装药 不输入 5 两道水密隔墙之间的半宽距离 L/m 8.00 整个舱段长度为2L(m) 按水密段实际宽度一半输入 6 船壳板原始厚度 H/mm 8.000 直接输入不必转换成单位m 按实际输入 7 船壳板等效厚度(考虑加强筋) ˉh/m 0.064 按实际板厚度8倍计算 不输入 8 爆距 R/m 3.00 炸点距离舷板垂直距离 按实际输入,接触爆炸可按0.01输入 9 爆炸区域冲击波初始动能 EK/(kg∙m2∙s−2) 3349689 A区域:炸药爆炸冲击波初始动能计算值 不输入,用与B区域结果比对 10 钢板强度 σ/MPa 235.00 直接输入不必转换成Pa,对于Q235钢板,此数值是235 MPa,船板一般取值235~440 MPa,代入公式时乘106 按实际输入 11 船壳板变形内凹陷试算角度 Φ/(°) 2.27 从0°~90°取数值试算 输入角度试算,结果显示在B区域 12 内凹陷角度转换弧度制 Φ/rad 0.04 此处是中间转换数值 不输入 13 爆破区计算作用动能 EA/(kg∙m2∙s−2) 3332283 B区域:爆炸冲击波做功近似转化为船壳板塑性变形能 不输入,用与A区域数值比对,直至EK=EA时止 14 动态极限应变 εm/% 0.2 钢板的极限应变是0.2% 不输入 15 计算极限应变 ε/% 0.2 C区域:此处计算得到临界极限应变 不输入 注:*以上计算方法适用于舱室跨度大于7 m,爆距小于9 m。 表 3 典型TNT装药当量爆炸破坏10 m跨度隔舱6 mm厚度舷板的计算结果
Table 3. Calculation results of equivalent explosion damage of typical TNT charge to 10 m span compartment and 6 mm thick sideboard
爆距/m 典型TNT当量条件下的破口半宽/m 350 kg 267 kg 240 kg 170 kg 9 0.92 0.87 0.81 0.76 11 0.66 0.61 0.56 0.51 表 4 典型TNT装药当量爆炸破坏10 m跨度隔舱8 mm厚度舷板的计算结果
Table 4. Calculation results of equivalent explosion damage of typical TNT charge to 10 m span compartment and 8 mm thick sideboard
爆距/m 典型TNT当量条件下的破口半宽/m 350 kg 267 kg 240 kg 170 kg 9 0.68 0.63 0.63 0.53 11 0.48 0.43 0.43 0.39 表 5 7 m以内跨度6 mm厚度舷板隔舱典型TNT装药当量爆距1 m破坏的计算结果
Table 5. Calculated damage results of typical TNT charge equivalent explosive distance of 1 m with span of 6 mm and the thickness of the bulkhead compartment within 7 m
隔舱跨度/m 典型TNT当量条件下的破口半宽/m 350 kg 267 kg 240 kg 170 kg 7 3.15 3.11 3.08 3.03 6 2.79 2.76 2.75 2.71 5 2.41 2.40 2.39 2.36 表 6 7 m以内跨度8 mm厚度舷板隔舱典型TNT装药当量爆距1 m破坏的计算结果
Table 6. Calculated damage results of typical TNT charge equivalent explosive distance of 1 m with span of 8 mm and the thickness of the bulkhead compartment within 7 m
隔舱跨度/m 典型TNT当量条件下的破口半宽/m 350 kg 267 kg 240 kg 170 kg 7 3.07 3.01 2.99 2.9 6 2.76 2.72 2.71 2.64 5 2.42 2.39 2.38 2.34 表 7 运用经验公式和两步迭代法的计算结果比较
Table 7. Comparison of calculation results between the empirical formula and the two-step iterative method
案例 运用吉田隆经验公式计算破坏半径 运用两步迭代法计算破坏半径 罗伯茨号 2.77 m 2.63 m 科尔号 3.29 m(装药181 kg)/4.07 m(装药317 kg) 5.07 m(装药181 kg)/5.67 m(装药317 kg) -
[1] 吉田隆. 二次世界大战初期日本海军舰船在炸弹攻击下的损伤实例分析 [J]. 舰船科学, 1990(5): 70–81.YOSHIDA. Damage case analysis of Japanese navy ships subjected to bomb attack in the early of the second world war [J]. Ship Science, 1990(5): 70–81. [2] 朱锡, 白雪飞, 黄若波, 等. 船体板架在水下接触爆炸作用下的破口试验 [J]. 中国造船, 2003, 44(1): 46–51. DOI: 10.3969/j.issn.1000-4882.2003.01.007.ZHU X, BAI X F, HUANG R B, et al. Crevasse experiment research of plate membrance in vessels subjected to underwater contact explosion [J]. Shipbuilding of China, 2003, 44(1): 46–51. DOI: 10.3969/j.issn.1000-4882.2003.01.007. [3] 朱锡, 白雪飞, 张振华. 空中接触爆炸作用下船体板架塑性动力响应及破口研究 [J]. 中国造船, 2004, 45(2): 43–50. DOI: 10.3969/j.issn.1000-4882.2004.02.006.ZHU X, BAI X F, ZHANG Z H. Plastic dynamic response and crevasse research of ship panels subjected to air contact explosion [J]. Shipbuilding of China, 2004, 45(2): 43–50. DOI: 10.3969/j.issn.1000-4882.2004.02.006. [4] 牟金磊, 朱锡, 黄晓明, 等. 水下近场非接触爆炸作用下固支方板破口计算 [J]. 振动与冲击, 2011, 30(1): 37–39, 55. DOI: 10.3969/j.issn.1000-3835.2011.01.008.MU J L, ZHU X, HUANG X M, et al. Crevasse computation for a clamped square plate subjected to near-field noncontact underwater explosion [J]. Journal of Vibration and Shock, 2011, 30(1): 37–39, 55. DOI: 10.3969/j.issn.1000-3835.2011.01.008. [5] 陈海龙, 周姝, 孙丰等. 水下接触爆炸对舰船壳板的毁伤试验效果估算方法评估 [J]. 舰船科学技术, 2013, 35(10): 33–37. DOI: 10.3404/j.issn.1672-7649.2013.10.008.CHEN H L, ZHOU S, SUN F, et al. Estimation on estimation method of warship shell experimental damage subjected to underwater contact explosion [J]. Ship Science and Technology, 2013, 35(10): 33–37. DOI: 10.3404/j.issn.1672-7649.2013.10.008. [6] DIDOSZAK J M. Parametric studies of DDG-81 ship shock trail simulation[D]. California: Naval Postgraduate School Monterey, 2004: 140–161. [7] JULIE A K. Feasibility and design of blast mitigation systems for naval applications using water mist fire suppression systems [D]. Cambridge, MA: Massachusetts Institute of Technology. 2004: 9–11. [8] BOGDAN S. The effect of an underwater explosion on a ship [J]. Scientific Journal of Polish Naval Academy (PNA), 2015, 201(2): 57–73. DOI: 10.5604/0860889X.1172074. [9] 李志辉, 刘辉, 李其修,等. 水下非接触爆炸作用下舰船结构损伤评估 [J]. 舰船科学技术, 2012, 34(7): 40–44. DOI: 10.3404/j.issn.1672-7649.2012.07.008.LI Z H, LIU H, LI Q X, et al. Research on damage evaluation of ship structure in underwater non-contact explosion [J]. Ship Science and Technology, 2012, 34(7): 40–44. DOI: 10.3404/j.issn.1672-7649.2012.07.008. [10] 李金河, 赵继波, 谭多望,等. 炸药水中爆炸的冲击波性能 [J]. 爆炸与冲击, 2009, 29(2): 172–176.LI J H, ZHAO J B, TAN D W, et al. Underwater shock wave performances of explosives [J]. Explosion and Shock Waves, 2009, 29(2): 172–176. [11] 宫翔飞, 刘文韬, 张树道,等. 水下爆炸近场峰值压力的数值模拟 [J]. 爆炸与冲击, 2019, 39(4): 041409. DOI: 10.11883/bzycj-2017-0262.GONG X F, LIU W T, ZHANG S D, et al. Numerical simulation of peak pressure in near-field underwater explosion [J]. Explosion and Shock Waves, 2019, 39(4): 041409. DOI: 10.11883/bzycj-2017-0262. [12] HSU C Y, LIANG C C, TENG T L, et al. Numerical study of hovercraft main hull structural response subjected to shockwave from underwater explosion[C]// JAWAID M, AZAD A K, YOUSEFI M. International Conference on Advanced Material Science and Environmental Engineering (AMSEE 2016). Netherlands: Atlantis Press, 2016: 136–139. [13] GEBREHIWOT S Z, REMES H, KARTTUNEN A T. A Stress concentration factor for interacting surface notch and subsurface hole [J]. Rakenteiden Mekaniikka, 2018, 51(4): 20–37.DOI. DOI: 10.23998/rm.70292. [14] BRADLEY P. No higher honor: saving the USS samuel B: Roberts in the persian gulf[M]. Annapolis: Naval Institute Press, 2006: 16–27. 期刊类型引用(1)
1. 梁潇帝,刘寅东. 基于PCA-BOA-KNN模型的水下爆炸舰船结构破损评估. 中国舰船研究. 2024(03): 150-157 .  百度学术
百度学术其他类型引用(0)
-








 下载:
下载:



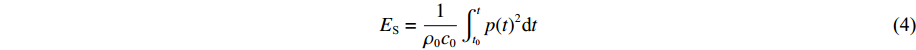

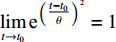
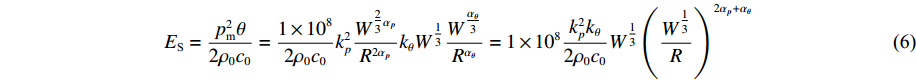


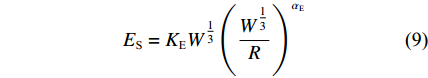




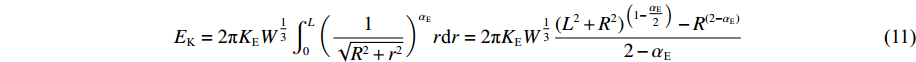
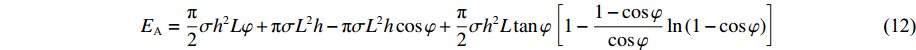







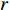









 下载:
下载:





 百度学术
百度学术




