Propagation characteristics of blast wave in diminished ambient temperature and pressure environments
-
摘要: 针对高海拔或高空的低温、低压环境对炸药爆炸冲击波传播的影响，利用量纲分析理论和AUTODYN有限元软件，研究了低温、低压及海拔高度对炸药爆炸冲击波参量（峰值超压、比冲量和波阵面运动轨迹）的影响规律，建立了相应的计算公式，并通过数值模拟和实验数据进行了对比验证。结果表明，该计算公式可以有效预测低温和低压环境下炸药爆炸冲击波参量。环境压力降低，爆炸冲击波峰值超压和爆炸远场（比例距离Z>0.2 m/kg1/3）比冲量减小，冲击波传播速度增大。环境温度降低，冲击波比冲量增大，传播速度降低，峰值超压影响不大。海拔高度在0~9 000 m范围内，每升高1000 m冲击波峰值超压和爆炸远场比冲量分别平均降低约3.9%和3.2%。海拔升高，爆炸近场冲击波传播速度升高，爆炸远场冲击波传播速度则降低。高海拔环境下低压对冲击波峰值超压和比冲量的影响大于低温，爆炸近场冲击波传播速度取决于低压的影响，爆炸远场冲击波传播速度取决于低温的影响。Abstract: The effects of different diminished ambient pressure, temperature and altitude from sea level on blast wave parameters (overpressure, impulse and wave front trajectory) were investigated by employing the dimensional analysis theory and the AUTODYN software. Meanwhile, the relationship equations between the blast wave parameters with the diminished pressure and temperature were established, which were verified by numerical simulations and experimental data. Results indicate that the equations can evaluate the blast wave parameters at diminished temperature and pressure effectively. It is noted that the blast wave overpressure and far-field (scaled distance Z>0.2 m/kg1/3) impulse decrease, but the propagation velocity increases, as the ambient pressure decreases. The blast wave impulse increases, and the propagation velocity decreases, but has little effect on the overpressure, as the ambient temperature decreases. It is shown that when the altitude increases by 1000 m in the range from 0 to 9000 m above sea level, the overpressure and far-field impulse of the blast wave decrease in average by about 3.9% and 3.2%. In addition, the blast wave propagation velocity in the near field increases, but it in the farfield decreases with the altitude increase. The influences of the diminished pressure on the blast wave overpressure and impulse are greater than those of the diminished temperature at high altitudes. The blast wave propagation velocity depends on the diminished pressure in the near field, but on the diminished temperature in the far field.
-
Key words:
- blast wave /
- high altitude /
- diminished temperature /
- diminished pressure /
- propagation characteristics
-
图 2 不同网格尺寸的冲击波超压-时程曲线比较
Figure 2. Comparison of overpressure time history curves for different cell sizes
图 3 标准大气环境下冲击波参量的理论、数值模拟与实验结果对比
Figure 3. Comparison among theoretical, numerical and experimental blast wave parameters in standard atmospheric environment
图 4 低压环境下冲击波参量的理论、数值模拟与实验数据对比
Figure 4. Comparison among theoretical, numerical and experimental shock wave parameters in diminished pressure environments
图 5 低温环境下冲击波参量的理论、数值模拟结果与实验结果的对比
Figure 5. Comparison among theoretical, numerical and experimental shock wave parameters in diminished temperature environments
图 6 高海拔环境下冲击波参量的理论、数值模拟结果与实验结果的对比
Figure 6. Comparison among theoretical, numerical and experimental shock wave parameters in high-altitude environment
图 7 不同低压和低温环境下爆炸冲击波波阵面的运动轨迹
Figure 7. Motion trajectories of blast wave in diminished pressure and temperature environments
图 8 不同低压和低温环境下爆炸冲击波的传播速度
Figure 8. Propagation velocities of blast waves in diminished pressure and temperature environments
图 9 不同海拔高度下爆炸冲击波波阵面运动轨迹及传播速度的理论、数值模拟结果与实验结果的对比
Figure 9. Theoretical, numerical and experimental comparison of shock wave parameters in different high-altitude environments
表 1 爆炸冲击波传播问题中物理量的量纲幂次
Table 1. Dimensional power coefficients of physical quantities in the problem of blast wave propagation
基本量纲 E p ρ r Δpm i t M 1 1 1 0 1 1 0 L 2 −1 −3 1 −1 −1 0 T −2 −2 0 0 −2 −1 1 
下载: 导出CSV
表 2 爆炸冲击波传播问题中物理量的量纲幂次（初等变换）
Table 2. Dimensional power coefficients of physical quantities in the problem of blast wave propagation (elementary transformation)
参考物理量 E p ρ r Δpm i t E 1 0 0 1/3 0 1/3 1/3 p 0 1 0 −1/3 1 1/6 −5/6 ρ 0 0 1 0 0 1/2 1/2
下载: 导出CSV
表 3 不同海拔高度下的大气参数
Table 3. Atmospheric parameters at different altitudes
h/m Th/K ph/kPa ρh/(kg∙m−3) 0 288.15 101.325 1.225 4 500 258.90 57.728 0.777 9 000 229.65 30.742 0.466
下载: 导出CSV
-
[1] 王兆祥, 常颖, 付昭旺, 等. 某型坦克炮常规弹药高原环境下打击效能试验 [J]. 火力与指挥控制, 2017, 42(5): 173–176. DOI: 10.3969/j.issn.1002-0640.2017.05.038.WANG Z X, CHANG Y, FU Z W, et al. Experimental study on operational effectiveness under plateau environment for certain type of tank cannon equipped with conventional ammunition [J]. Fire Control and Command Control, 2017, 42(5): 173–176. DOI: 10.3969/j.issn.1002-0640.2017.05.038. [2] 李科斌, 李晓杰, 闫鸿浩, 等. 不同真空度下空中爆炸近场特性的数值模拟研究 [J]. 振动与冲击, 2018, 37(17): 270–276. DOI: 10.13465/j.cnki.jvs.2018.17.038.LI K B, LI X J, YAN H H, et al. Numerical simulation for near-field characteristics of air explosion under different degrees of vacuum [J]. Journal of Vibration and Shock, 2018, 37(17): 270–276. DOI: 10.13465/j.cnki.jvs.2018.17.038. [3] VELDMAN R L, NANSTEEL M W, CHEN C C T, et al. The effect of ambient pressure on blast reflected impulse and overpressure [J]. Experimental Techniques, 2017, 41(3): 227–236. DOI: 10.1007/s40799-017-0171-8. [4] 汪泉, 陆军伟, 李志敏, 等. 负压条件下柱形爆炸罐内爆炸波传播规律 [J]. 兵工学报, 2021, 42(6): 1250–1256. DOI: 10.3969/j.issn.1000-1093.2021.06.015.WANG Q, LU J W, LI Z M, et al. Propagation law of explosion wave in columnar explosion tank under vacuum conditions [J]. Acta Armamentarii, 2021, 42(6): 1250–1256. DOI: 10.3969/j.issn.1000-1093.2021.06.015. [5] 庞春桥, 陶钢, 周佩杰, 等. 高原环境下爆炸冲击波参数的有效预测方法 [J]. 振动与冲击, 2018, 37(14): 221–226. DOI: 10.13465/j.cnki.jvs.2018.14.031.PANG C Q, TAO G, ZHOU P J, et al. Effective method for predicting the parameters of shock waves in plateau environment [J]. Journal of Vibration and Shock, 2018, 37(14): 221–226. DOI: 10.13465/j.cnki.jvs.2018.14.031. [6] 陈龙明, 李志斌, 陈荣, 等. 高原环境爆炸冲击波传播特性的实验研究 [J]. 爆炸与冲击, 2022, 42(5): 053206. DOI: 10.11883/bzycj-2021-0279.CHEN L M, LI Z B, CHEN R, et al. An experimental study on propagation characteristics of blast waves under plateau environment [J]. Explosion and Shock Waves, 2022, 42(5): 053206. DOI: 10.11883/bzycj-2021-0279. [7] IZADIFARD R A, FOROUTAN M. Blastwave parameters assessment at different altitude using numerical simulation [J]. Turkish Journal of Engineering and Enviromental Sciences, 2010, 34(1): 25–41. DOI: 10.3906/muh-0911-39. [8] BRIDGMAN P W. Dimensional analysis[M]. 2nd ed. New Haven, USA: Yale University Press, 1931: 40–46. [9] LEE E L, HORNIG, H C, KURY, J W. Adiabatic expansion of high explosive detonation products: TID 4500-UCRL 50422 [R]. Livermore, USA: Lawrence Livermore National Laboratory, 1968. [10] COWLER M S, VIRNBAUM N K. AUTODYN user Manual [M]. Oakland, USA: Century Dynamics Inc, 1989: 213–214. [11] BORGNAKKE C, SONNTAG R E. Fundamentals of thermodynamics [M]. 8th ed. Singapore: John Wiley & Sons, 2013: 110–120. [12] HENRYCH J. The dynamics of explosion and its use [M]. Amsterdam, the Netherlands: Elsevier, 1979: 124–132. [13] BRODE H L. Numerical solutions of spherical blast waves [J]. Journal of Applied Physics, 1955, 26(6): 766–775. DOI: 10.1063/1.1722085. [14] HELD M. Blast waves in free air [J]. Propellants, Explosives, Pyrotechnics, 1983, 8(1): 1–7. DOI: 10.1002/prep.19830080102. [15] SADOVSKIY M A. Mechanical action of air shock waves of explosion, based on experimental data [M]. Moscow, Russia: Nauka Press, 1952: 6–10. [16] BAJIĆ Z. Determination of TNT equivalent for various explosives [D]. Belgrade: University of Belgrade, 2007. [17] KINNEY G F, GRAHAM K J. Explosive shocks in air [M]. Berlin: Springer, 1985: 88–105. [18] MILLS C A. The design of concrete structures to resist explosions and weapon effects [C]//1st International Conference on Concrete for Hazard Protection. Edinburgh, UK, 1987: 61–73. [19] KINGERY C N, BULMASH G. Air-blast parameters from TNT spherical air burst and hemispherical surface burst: ARBRLTR-02555 [R]. Maryland, USA: US Army Ballistic Research Laboratory, 1984. [20] USA Department of Defense Explosives Safety Board. Structures to resist the effects of accidental explosions: UFC 3-340-02 [S]. Washington, USA: US Army Corps of Engineers, 2008. [21] DEWEY J M, SPERRAZZA J. The effect of atmospheric pressure and temperature on air shock: BRL report 721 [R]. Maryland, USA: Aberdeen Proving Ground, 1950. [22] 钱翼稷. 空气动力学 [M]. 北京: 北京航空航天大学出版社, 2004: 17–21. [23] 李志斌, 陈龙明, 陈荣, 等. 一种高原爆炸冲击波等效测试系统及测试方法: CN112378563A [P]. 2021-02-19.LI Z B, CHEN L M, CHEN R, et al. Plateau explosive shock wave equivalent test system and test method: CN112378563A [P]. 2021-02-19. [24] DEWEY J M. The properties of a blast wave obtained from an analysis of the particle trajectories [J]. Proceedings of the Royal Society of London. Series A: Mathematical and Physical Sciences, 1971, 324(1558): 275–299. -
计量
- 文章访问数: 859
- HTML全文浏览量: 243
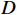
- PDF下载量: 200
- 被引次数: 0