On the evolution mechanism of the shock-accelerated annular SF6 cylinder
-
摘要: 基于可压缩多组分Navier-Stokes控制方程,结合5阶加权本质无振荡格式以及网格自适应加密技术和level-set方法,数值模拟了平面激波(Ma=1.23)与环形SF6气柱(内外半径分别为8和17.5 mm)界面的相互作用过程。相比于之前的实验结果,数值模拟结果揭示了入射激波在界面内4次透射过程中的复杂波系结构,观察到透射激波在内部界面传播时形成自由前导折射结构并向自由前导冯诺依曼折射结构转换的波系演变过程;另外,界面内的复杂激波结构诱导内部下游界面上的涡量发生了3次反向;在界面演化后期,内部界面形成的“射流”结构与下游界面相互作用,诱导界面形成一对主涡、一对次级涡以及一个反向“射流”结构。定量分析了环形界面长度、宽度、位移、环量以及混合率的变化情况,结果表明,内部气柱的存在减弱了前期小涡结构合并形成大涡结构过程中对界面高度与长度的影响,同时提高了重质气体与环境气体的混合率。
-
关键词:
- 激波 /
- 环形气柱 /
- Richtmyer-Meshkov不稳定性 /
- 涡流
Abstract: Based on the compressible multicomponent Navier-Stokes equations, the interaction of a planar shock wave (Ma=1.23) with an annular SF6 cylinder whose inner and outer radii were set as 8 and 17.5 mm respectively was numerically studied. The simulation was conducted based on the finite volume method. For capturing the complex shock and vortex structures as well as the interfaces, the adaptive mesh refinement method, level set method, and fifth-order weighted essentially non-oscillatory scheme were used for the simulation. The adaptive mesh refinement method dynamically refined the uniform Cartesian grids around the multiple moving shocks and accelerated interfaces. The level set method tracked the interface, while the fifth-order weighted essentially non-oscillatory scheme captured discontinuities such as shock waves and contact surfaces. Time advancement was achieved with the third-order strong-stability-preserving Runge-Kutta method. Compared with the previous experimental results, numerical results revealed the complex evolution of shock wave structures generated in the process of four shock transmissions in the annular cylinder. It is found that the transition from free precursor refraction to free precursor von Neumann refraction occurs when the transmitted shock wave passes through the inner cylinder. In addition, the complex shock structures that developed between the inner and outer downstream interfaces cause the pressure gradient direction to reverse several times on the inner downstream interface, which eventually leads to three reversals of vorticity on the inner downstream interface. In the later stage, the “jet” structure formed on the inner cylinder would impact the downstream interfaces, and finally induces the interfaces to generate a pair of primary vortices, a pair of secondary vortices and a reverse “jet”. Quantitative analyses of the variation of the length, width, displacement, the circulation and mixing rate of the annular cylinder were conducted. The results demonstrate that the presence of the inner cylinder attenuates the influence on the height and length of the annular cylinder during the process of small vortexes merging into the large vortexes in the early stage, and increases the mixing rate of the heavy gas and the ambient gas.-
Key words:
- shock wave /
- annular gas cylinder /
- Richtmyer-Meshkov instability /
- vortex
-
激波冲击不同密度的流体界面过程中,诱导界面变形失稳并最终向湍流混合转捩的现象,称为Richtmyer-Meshkov (RM)不稳定性。RM不稳定性广泛存在于兵器发射、燃烧爆炸、航空航天以及惯性约束核聚变(inertial confinement fusion, ICF)[1]等工程领域中。例如在ICF中由于RM不稳定性的作用,降低了靶丸中心温度和压力,导致核聚变反应无法发生;在超燃冲压发动机中,RM不稳定性反而能促进燃料和氧化剂之间的混合,从而提高燃烧效率,增强发动机的推进性能[2-3]。另外,RM不稳定性中耦合了激波结构演化、涡量生成与输运、界面变形、激波与涡相互作用以及湍流混合等复杂物理现象,因此,研究激波诱导不同气体界面RM不稳定性的演化机理,无论是在学术领域还是在工程应用领域均具有重大的研究价值。
近年来,激波冲击不同气体界面构型的RM不稳定性问题得到了广泛的研究[4]。Zou等[5]和黄熙龙等[6]通过实验研究了不同长宽比下无膜椭圆气柱界面的RM不稳定性,发现在大水平方向尺度情况下,界面发展后期会出现二次涡对结构。Zhai等[7]和Luo等[8]通过实验和数值仿真研究了平面激波冲击轻、重质多边形(包括三角形、正方形、菱形以及矩形)气柱的RM不稳定性过程,揭示了激波在多边形界面内的演化情况以及气柱特征的变化规律。沙莎等[9]则基于大涡模拟方法对平面激波与两种SF6梯形重气柱的相互作用过程进行了数值模拟,揭示了两种梯形重气柱的变形过程以及其复杂波系结构。廖深飞等[10]实验研究了不同时刻反射激波二次冲击处于演化中后期SF6气柱界面的演化规律,结果表明,反射距离直接影响反射激波在界面上的折射以及界面上二次涡量的产生和分布,从而影响界面后期涡对结构的生成。王震等[11]通过数值模拟研究了非平面激波诱导非均匀流场的RM不稳定性,发现界面的增长对流场的非均匀性十分敏感,且非平面激波的加载使界面扰动振幅随着流场非均匀性增强而增大。
以往的研究多集中于激波与单层气体界面的相互作用,而在实际应用中广泛存在多层气体界面的情况,比如ICF问题中,靶丸内核为氘和氚的气体混合物,外壳则由外层的腐蚀材料和内层的固相氘氚燃料组成。因此,激波诱导多层界面的RM不稳定性演化问题成为了近年的研究热点。Orlicz等[12]通过实验研究了不同入射激波强度对重气层的RM不稳定性以及混合特性,结果表明,较高的马赫数下,混合层的混合更加均匀,但其诱导的涡流较强,使得涡吸入更多重气体导致涡结构增长,反而限制了混合层吸入更多空气。Shankar等[13]和Zeng等[14]通过数值模拟研究了三维情况下激波与空气-SF6-空气气幕的相互作用及其RM不稳定性,得到了入射激波和反射激波作用后的湍流混合特性。De Frahan等[15]基于二维多流体Euler方程研究了激波冲击连续流体层的界面扰动增长规律,发现第3种气体的声阻抗较大(或较小)时,在第二界面上产生的反射激波(或稀疏波)会使得第一界面上的扰动被抑制(或促进),甚至被冻结,因此,提出利用激波和稀疏波来调控界面的不稳定性增长。Liang等[16]实验研究了激波冲击5种不同波形SF6气体层的RM不稳定性,分析了线性阶段时重气层内波系对界面振幅增长的效应,并对非线性阶段稀疏波对第一界面的影响给出了定量预测结果。Wang等[17]对激波冲击环形SF6/He气柱界面的演化过程进行了数值模拟,提出将通过线性叠加Samtaney & Zabusky (SZ)环量模型[18]和Yang, Kubota & Zukoski (YKZ)环量模型[19]来预测环形气柱的环量变化,得到了不同内圆半径的环形气柱在前期线性阶段的涡量累积和环量分布规律。冯莉莉等[20-21]采用实验与数值模拟方法研究了平面激波诱导下双层SF6气柱的演化规律,并分析了内部气柱半径大小与偏心效应对流场演化的影响,研究表明,内层气柱的存在导致下游界面两侧的压力差减小,使得界面下游没有形成射流结构,而内部气柱偏心和半径变化均会在下游界面诱导生成一对涡对,其中涡对的尺度和内外半径的比值正相关。
虽然前人的工作中得到了环形SF6气柱界面的演化结果,但激波与环形气柱界面相互作用多次透射形成的复杂波系结构,且界面上涡量生成与演化对后期界面演化和气体混合的作用机理尚未完全解释清楚。因此,本文中,基于二维可压缩多组分Navier-Stokes(NS)方程,结合5阶高精度加权本质无振荡(weighted essentially non-oscillatory, WENO)格式以及网格自适应加密(adaptive mesh refinement, AMR)技术和level-set方法,对平面激波与环形SF6重气体界面的作用过程进行数值模拟,着重讨论平面激波与环形界面作用过程中的复杂激波结构、界面演化变形以及涡量分布的详细过程,并探索界面长度、高度、位移、环量以及混合率的变化规律。
1. 数值方法与计算模型
1.1 数值方法
激波与不同气体界面作用过程为无化学反应、多组分的流体运动过程,且激波马赫数小,可忽略气体分子的电离以及重力效应[22]。因此,控制方程采用无反应、多组分的可压缩Navier-Stokes方程组:
∂ρ∂t+∂(ρuj)∂xj=0 (1) ∂(ρui)∂t+∂(ρuiuj+pδij)∂xj−∂σij∂xj=0 (2) ∂E∂t+∂[(E+p)uj]∂xj−∂∂xj(σjiui−Qj−Hj)=0 (3) ∂(ρYα)∂t+∂(ρYαuj)∂xj+∂Jαj∂xj=0 (4) 式中:p为压力;ρ为流体密度;uj为j方向的速度分量;Yα则为组分α的质量分数,
α=1,⋯,N ,N为气体组分种类数;δij为Kronecker delta函数;E为混合气体单位体积的总能;黏性应力张量σij、热通量Qj、内部扩散的焓通量Hj和扩散质量通量Jαj分别为:σij=μ(∂ui∂xj+∂uj∂xi−23δij∂uk∂xk) (5) Qj=−κ∂T∂xj (6) Hj=N∑α=1hαJαj (7) Jαj=−ρ(Dα∂Yα∂xj−YαN∑β=1Dβ∂Yβ∂xj) (8) 式中:μ为黏性系数,T为温度,
κ 为热传导系数,Dα为气体混合物中组分α的质量扩散系数,hα为气体α的焓。补充混合气体的理想气体状态方程使控制方程封闭,则hα和E为:hα=cpαT (9) E=ρcpαT−p+12ρuiui=pγ−1+12ρuiui (10) 混合气体的定压比热容cp、摩尔质量m和比热比γ则分别为:
cp = N∑α = 1Yαcpα (11) 1m=N∑α=1Yαmα (12) γ = cpcp−R/m (13) 式中:cpα和mα分别为组分α的定压比热容和摩尔质量,R为理想气体常数。
基于可压缩多组分Navier-Stokes方程,采用有限体积法进行数值求解。考虑到激波冲击气柱界面过程中包含了激波反射、透射以及激波-涡相互作用等复杂物理现象,因此采用5阶WENO格式对控制方程进行离散,并结合AMR技术和level-set方法以精确捕获流场中的精细波系结构以及界面形态。时间推进则采用3阶Runge-Kutta法。
1.2 算法验证
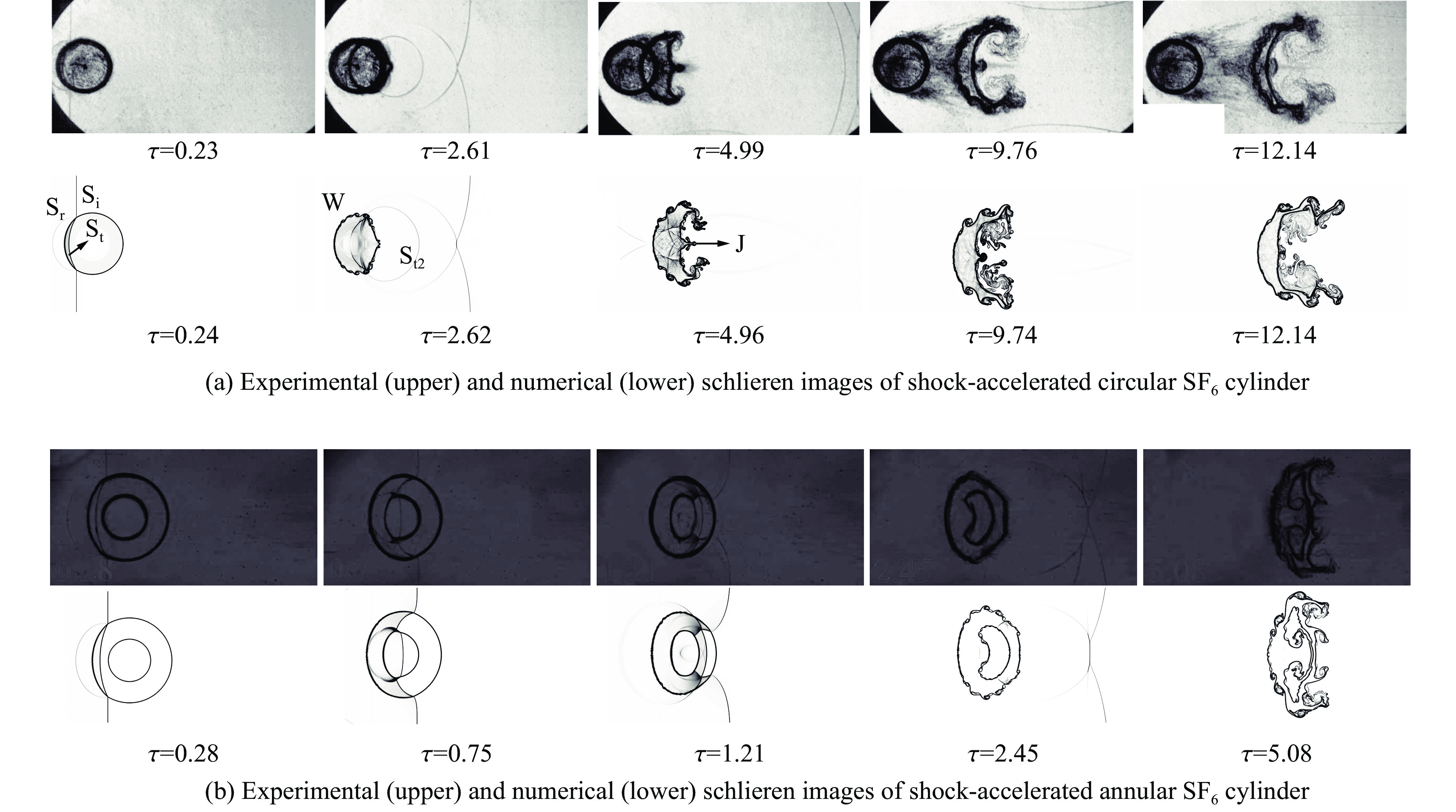
图1为平面激波分别与圆形和环形SF6气柱相互作用过程的实验纹影图(上)与数值模拟结果(下)的对比。图1(a)的数值模拟初始条件、网格数以及加密级数均与文献[23]中完全一致,而图1(b)的初始计算条件参数则与文献[21]中的“case Ⅲ”完全相同。其中的无量纲时间定义为
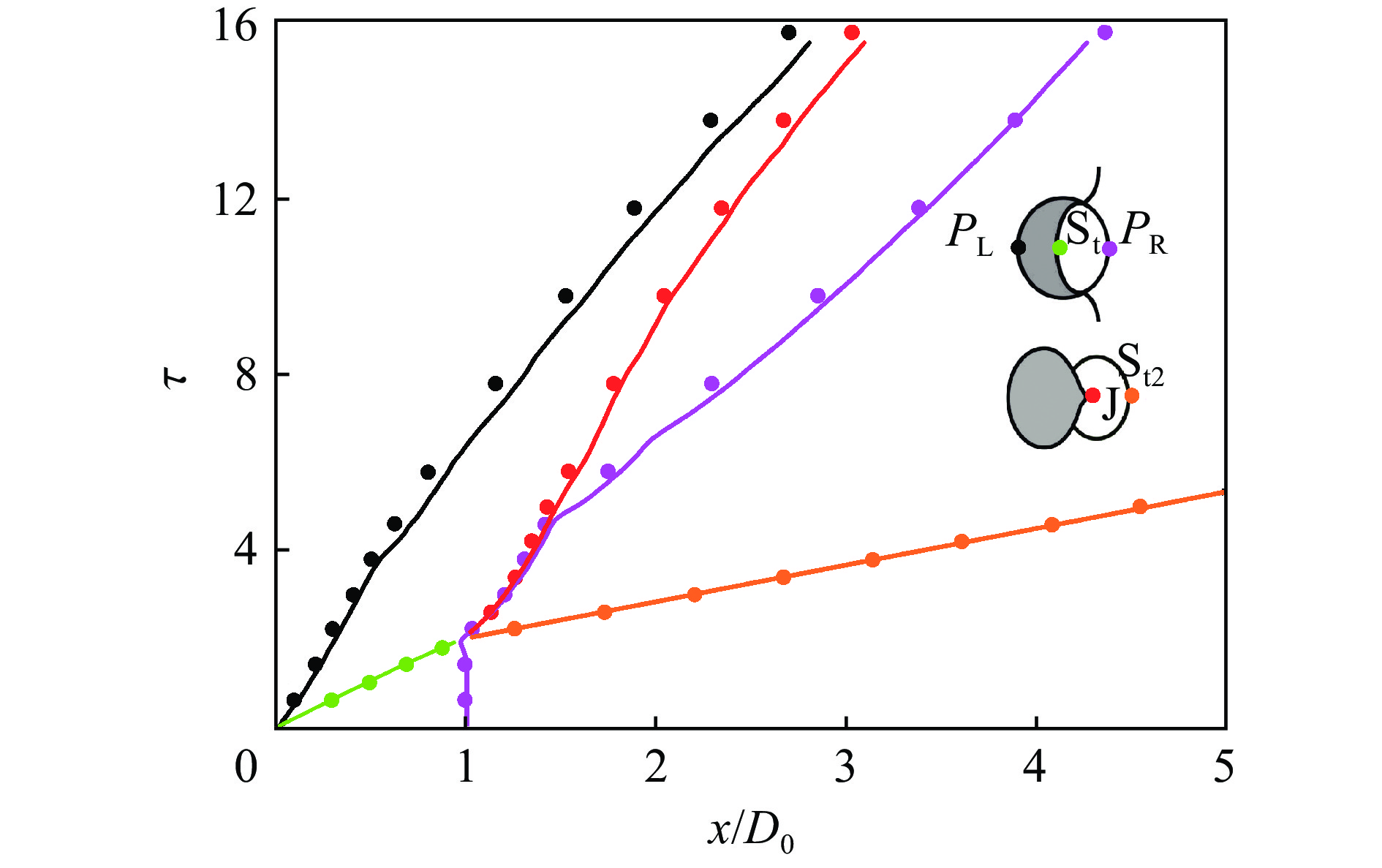
τ=tWs/D0 ,并设定平面激波与气柱界面刚接触瞬时为t=0时刻,Ws为初始激波的速度,D0为初始气柱的直径。由图1可见,无论是圆形气柱还是环形气柱,本文中数值模拟结果描述的激波结构(包括入射激波Si、反射激波Sr、透射激波St、稀疏波W和二次透射激波St2),射流结构J,涡结构以及界面形态与文献[21, 23]中的实验结果完全相符。图2为圆形SF6气柱上的特征点位置随时间的变化规律的定量对比,其中选取的5个特征点分别为气柱左边界点PL、右边界点PR、透射激波St、二次透射激波St2和射流J,并在图2中分别用不同颜色进行了标注。图2中圆点为文献[23]中的实验结果,实线为本文中的数值模拟结果。由图2可知,St和St2的变化规律与文献[23]中的实验结果完全一致,PL、PR以及J的后期位移与实验结果略有差异,但误差在可接受范围之内,说明本文中数值模拟方法准确可信。1.3 计算模型
为了探究内部气柱对界面演化的影响,基于文献[23]中的计算模型,通过设定内部气柱半径,得到平面激波与环形SF6气柱相互作用的计算模型,如图3所示。其中,计算域为160 mm×100 mm的长方形区域,环形气柱的外圆直径为D0 = 35 mm,内圆半径为r = 8 mm。计算域的坐标原点为O,而环形气柱的内、外圆心分别为O1和O2,且内外圆心重合,坐标为(17.5 mm, 0 mm)。初始条件及气体参数与文献[23]保持一致,所采用的气体参数如表1所示。初始时,环形界面内充满SF6重质气体,其余区域为空气,界面内外压力均设为101.49 kPa,温度均为288 K。初始入射平面激波位置为x = 0,入射激波从左向右传播,激波马赫数为Ma=1.23。计算域右侧边界采用无反射边界条件,左边界为入口边界条件,上下边界均为反射边界条件。
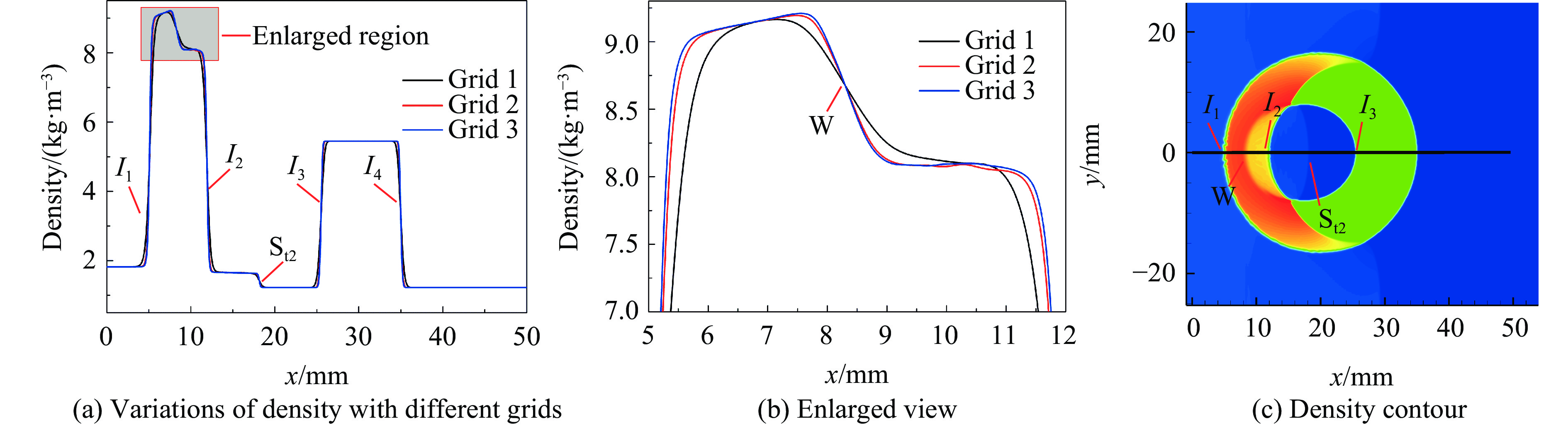
表 1 气体参数Table 1. Gas parameters气体名称 比热比 摩尔质量/(g·mol−1) 密度/(kg·m−3) 空气 1.399 28.967 1.23 SF6 1.103 128.491 5.45 计算域内均采用结构化、无拉伸的笛卡尔网格。为了检验网格无关性,本文中对初始网格数量分别为640×400 (Grid 1)、1280×800 (Grid 2)和1600×1000 (Grid 3)的3种不同网格分辨率的计算结果进行比较,在图4中给出了0.07 ms时环形SF6气柱对称轴(y=0)上气体密度的分布情况。由图4(c)的密度云图可知,此时环形SF6气柱对称轴(y=0)上在外部上游界面I1、反射稀疏波W、内部上游界面I2、二次透射激波St2、内部下游界面I3和外部下游界面I4处存在明显的密度变化。而从图4(a)中线图和图4(b)的局部放大图可知,3种网格分辨率均能准确捕捉到密度变化,其中采用Grid 2网格数计算出的密度分布情况与采用Grid 3网格数的结果基本完全一致,且两种网格分辨率下的计算结果最大相对误差不超过1%,故初始网格数为1280×800 (Grid 2)情况下的计算结果是收敛的。此时初始网格分辨率可达到0.125 mm,采用网格自适应加密方法之后,加密后的局部网格尺度可细化到0.0625 mm。
2. 结果与讨论
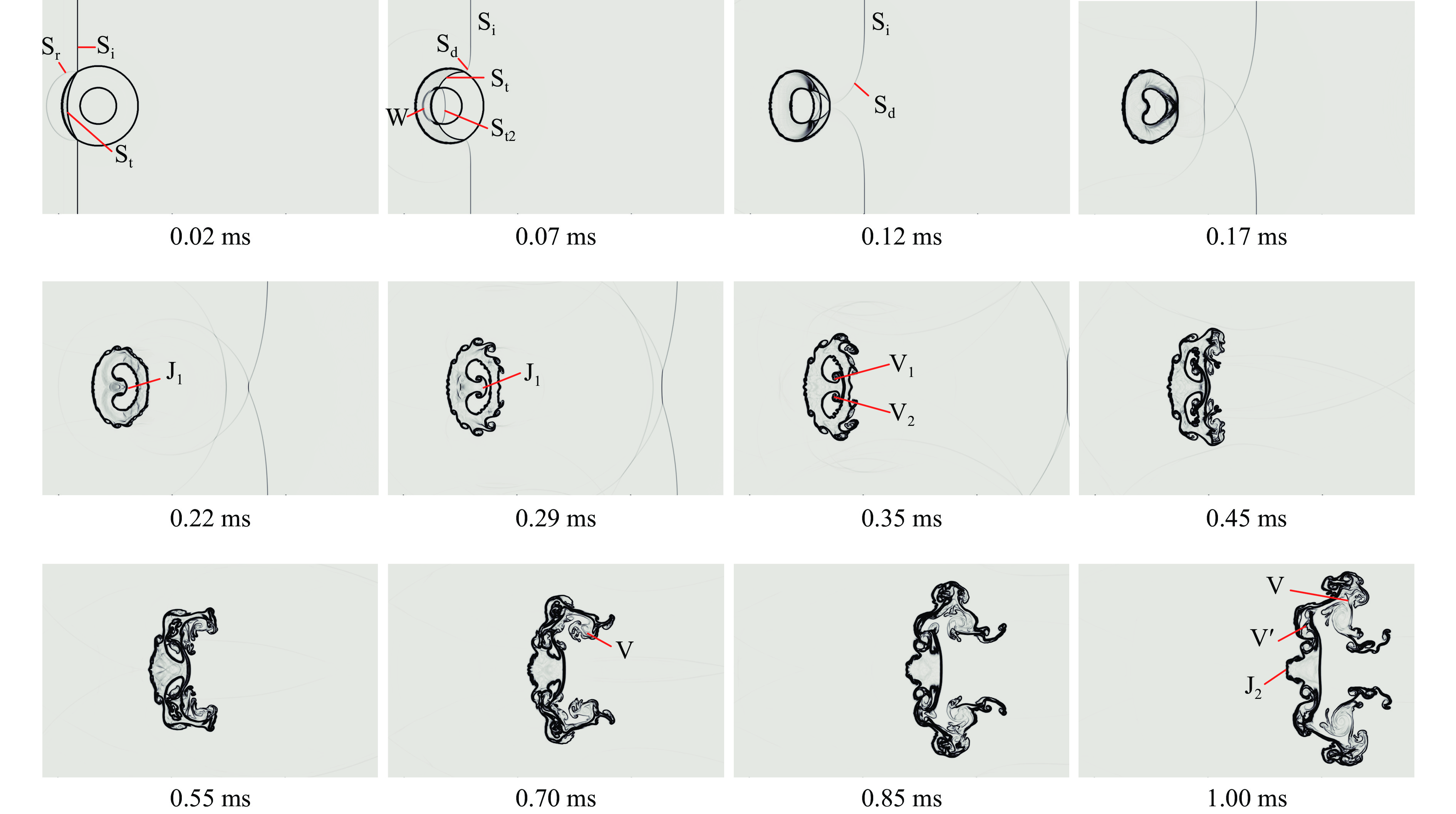
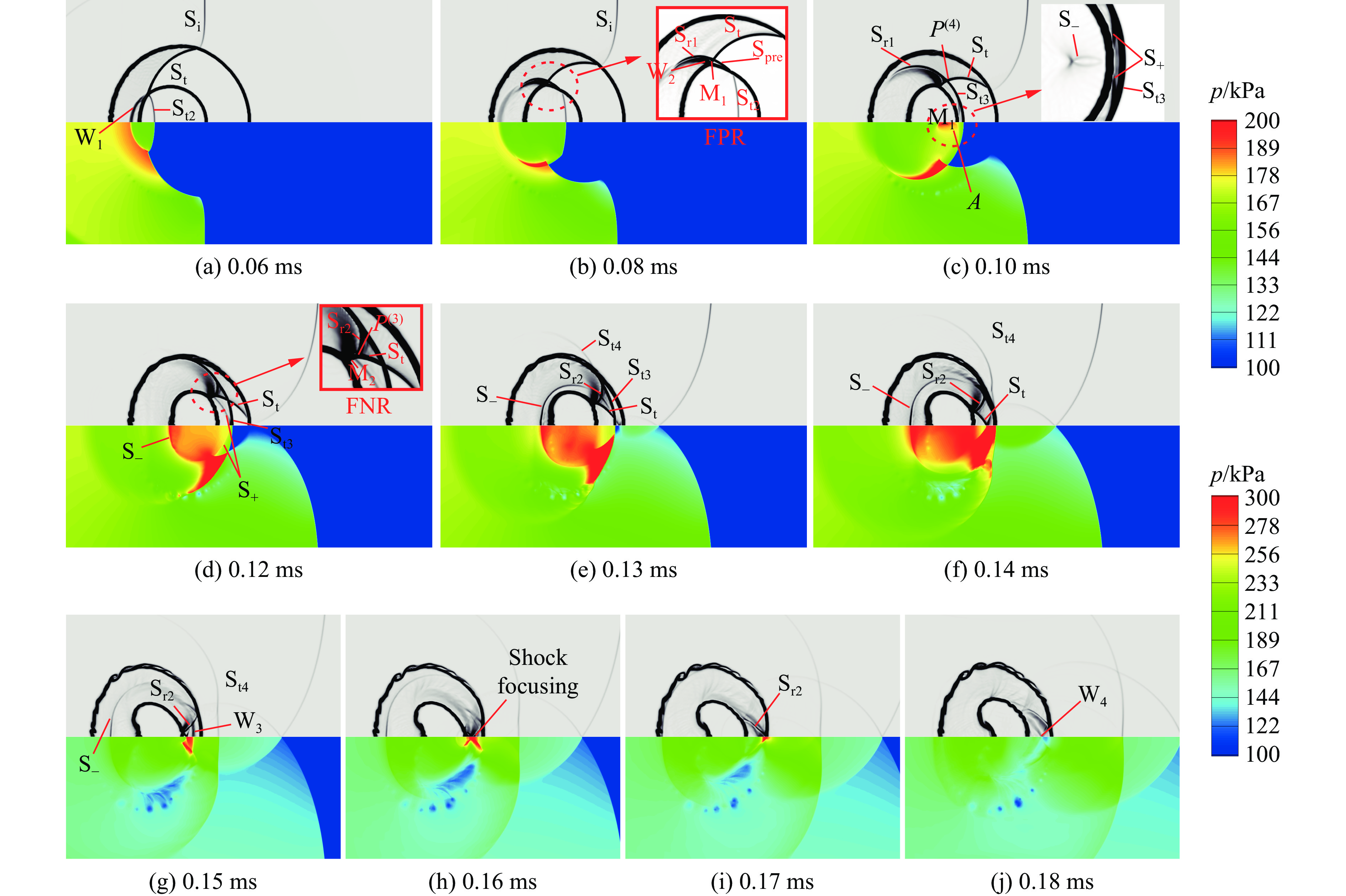
图5描述了平面激波冲击环形SF6气柱过程中的界面演化过程。与圆形SF6气柱界面的演变情况(图1(a))相比可见,环形气柱内形成了更复杂的激波结构和界面形态。通过与文献[21]中的结果对比,发现平面入射激波Si均会在外部上游界面发生反射与透射,形成了弧形的反射激波Sr和透射激波St(图5中0.02 ms),且St在内部上游界面处发生第2次透射,产生二次透射激波St2和反射稀疏波W(图5中0.07 ms)。然而,St在气柱内部界面产生的复杂激波结构却不尽相同。
图6给出了St在环形SF6气柱界面内透射过程中的密度纹影图和对应的压力云图。由图6(a)可知,St在内部上游界面上发生第2次透射,形成了St2和W1;其中St、W1和St2在内部界面上相交于一点。由于St2在轻质气体中传播速度较快,St2和St继续向下游传播过程中,St2在内部界面上产生一道与St相互作用的自由前导激波Spre,并在内部界面上形成自由前导折射(free precursor refraction, FPR)[7]激波结构,生成了反射激波Sr1、马赫杆M1和反射稀疏波W2,如图6(b)所示。
紧接着,St2在内部下游界面发生第3次透射,并与Spre合并形成3次透射激波St3,同时在内部下游界面处产生局部高压区A,并诱导界面前后分别产生前向传导激波S+和后向传导激波S–;其中,S+紧随St3之后,而S–则在内部下游界面左侧呈扁平状。此时,St3、Sr1、St和M1 4道激波相交于四波点P(4),并且St3和Sr1相交形成一道光滑的圆弧激波向外部下游界面运动,如图6(c)中所示。
随着St3继续向下游运动,St3和St在内部下游界面形成了自由前导冯诺依曼折射(free precursor von Neumann refraction, FNR)[7]激波结构,并生成了三波点P(3)、马赫杆M2和一道反射激波Sr2,其中Sr2从P(3)处向上延伸与Sr1相交,并和St、St3在P(3)上方形成一个圆弧三角形(图6 (d))。此时,弧形的S+对称分布在St3的中点之后,且S+一直延伸到M2处,而S–迅速向左侧扩张成圆弧形,并穿过内部上游界面。之后,St3首先在外部下游界面的上部发生第4次透射,形成弧形的4次透射激波St4;同时,绕射激波Sd与St在外部下游界面右侧相交之后,St与Sr2逐渐向内部下游界面的中点汇聚,如图6(e)~(f)所示。
当St3完全透射出外部下游界面后,St4在靠近对称线附近处基本为直线且垂直于对称轴,而在远离对称轴的部分依然保持为弧形。由于第4次透射是从重质气体向轻质气体透射,所以在界面内依然可以发现一道比较明显的反射稀疏波W3,如图6(g)所示。界面内,St与Sr2在内部下游界面右侧发生激波聚焦(shock focusing),形成局部高压区(图6(h))。在激波聚焦之后,Sr2在界面内相交之后继续沿着界面内侧向上下两边运动,同时在界面内侧上产生一道反射稀疏波W4;随着Sr2的分离,W4也在相交之后向内部传播,界面内再无明显的激波结构,如图6(i)~(j)所示。
当界面内部激波完全透射出界面后,在斜压效应的作用下,外部界面失稳并卷起形成珠串状的小涡结构(图5中0.17 ms)。值得注意的是,在图5中0.22 ms时,气柱内部上游界面由于激波冲击作用产生了向下游运动的重质气体“射流”结构J1;并在图5中0.22~0.45 ms之间,J1逐渐向内部下游界面靠近,并最终和内部下游界面合并将环形重质气柱中间的空气区域分成上下两部分。与文献[21]中不同的是,在界面演化后期,外部界面上的珠串状小涡结构逐渐发展变大,并在向下游界面相互合并,最终形成一对主涡V(图5中0.55~0.70 ms),使得气柱界面的高度迅速增加。在界面内部,J1与下游界面合并,其两侧的涡对结构V1、V2逐渐卷起向上下两侧发展,并与外部界面上的涡合并形成为次级涡V′结构(图5中0.55~1.00 ms)。最终,随着V和V′的逐渐发展,气柱界面高度和宽度都逐渐增加,而在界面上游,由于V′的卷吸作用导致下游对称线附近界面向上下两侧拉伸,使得上游界面逐渐形成一个与气柱运动方向相反的“射流”(J2)结构。
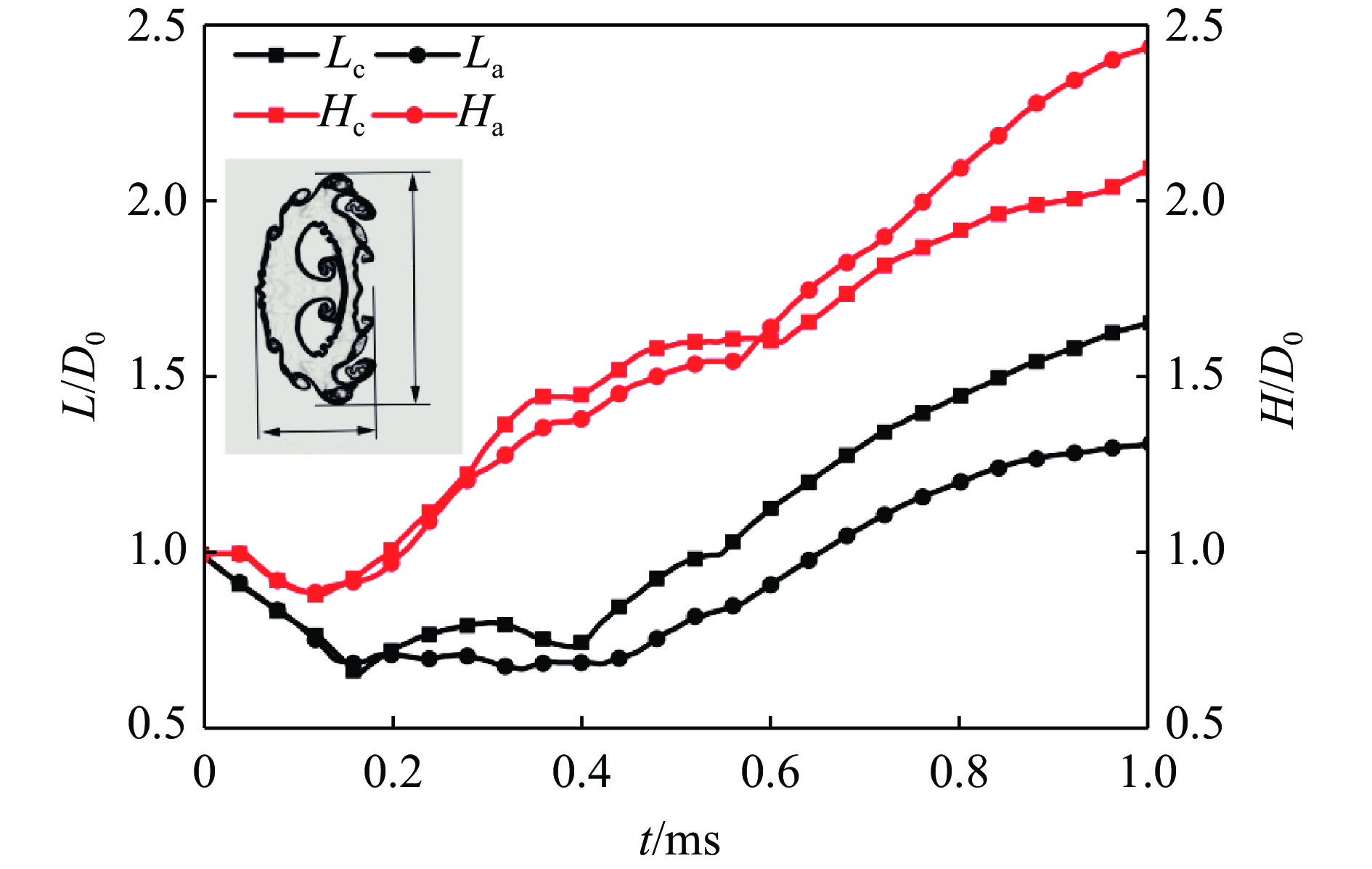
为了定量分析环形和圆形SF6气柱界面演化的区别,图7分别描述了环形气柱界面长度La和高度Ha随时间的变化曲线,以及圆形气柱界面长度Lc和高度Hc随时间的变化曲线,其中,L和H的定义如图7所示。另外,为了描述界面在激波冲击后的位移情况,分别定义界面左边界位移s和平均位移
ˉs 为:s=xL,ˉs=(xL+xR)/2 ,其中xL和xR分别表示界面左、右极点在x方向的坐标。图8描述了环形气柱界面左边界位移sa和平均位移ˉsa 与圆形气柱界面左边界位移sc和平均位移ˉsc 的变化曲线。由图7可见,在激波冲击作用前期,两种气柱的界面长度变化规律完全一致。但在0.2 ms后,圆形界面长度在0.2~0.4 ms阶段有起伏,说明界面上小涡卷起并向后运动形成大涡过程对界面长度影响明显;而环形界面长度在0.2~0.4 ms阶段变化平缓,说明小涡卷起合并过程对界面长度的影响不大。在界面演化后期(t>0.6 ms),大涡结构的发展使得界面长度迅速增加,但环形界面的长度均小于圆形界面的长度,说明环形气柱中心的轻质气体能减缓气柱长度的增长。
对于界面高度而言,环形气柱界面在初始激波作用下受到压缩之后,便一直保持增长趋势,其中在0.12~0.56 ms之间界面高度增长速度较慢,而在0.56 ms之后,环形界面内部“射流”上的涡对结构向上下两侧开始运动,导致界面高度增长速度迅速提升。而圆形气柱界面高度在0.16~0.36 ms之间增长速度较环形界面快,但是在0.36~0.59 ms阶段,由于大涡结构逐渐卷起,导致界面高度出现阶梯性的增长,而在大涡形成后,虽然界面高度的增长速率有所提升,但界面高度以及增长速率仍低于环形气柱界面。
由图8可知,两种界面的位移变化相差不大,在0.1 ms之前,无论是左边界位移还是平均位移均相同。在0.1 ms之后,环形气柱内部的轻质气体更容易被压缩,使得环形气柱界面的左边界位移开始大于圆形气柱界面的左边界位移,而右边界运动具有一定延时,所以在0.1~0.2 ms之间,环形气柱界面的平均位移会略大于圆形界面。随后,环形气柱界面的左边界位移相比圆形界面越来越大,使得环形气柱的平均位移在后期相比圆形气柱界面快。
为进一步分析界面上涡量的演化过程,图9给出了激波作用后不同时刻流场里的涡量ω分布云图,图9中红色代表正涡量(逆时针旋转),蓝色代表负涡量(顺时针旋转)。由于流场结构对称,故只讨论上半部流场的涡量演化情况。当Si和St分别扫过环形气柱内外界面后,由于斜压效应,分别在外部界面和内部界面上生成负涡量和正涡量,如图9中0.14 ms中所示。随后,在内部下游界面上的涡量方向出现了反复反向的现象。
St2在透射过内部下游界面之后,Sr2和St在内部下游界面和外部下游之间传播(图6(c)~(f)),使得内部下游界面右侧的压力升高,导致压力梯度反向而密度梯度方向不变,从而在内部界面上诱导产生负涡量与已有正涡量相互抵消,使得内部下游界面上的正涡量逐渐变小,直到Sr2在内部下游界面的中心处发生激波聚焦(图6(h)),此时内部下游界面上才开始出现负涡量(图9中0.16 ms)。而当Sr2在外部下游界面左侧相交时,内部下游界面已完全转变为负涡量,同时Sr2的相交也使得外部下游界面左侧开始产生正涡量(图9中0.18 ms)。
然而,随着Sr2向上下侧运动,Sr2后的W4使得下游两层界面之间的压力迅速降低(图6(j)),导致此时的压力梯度再一次反向,从而诱导内部下游界面的斜压涡量逐渐转变为正涡量(图9中0.20~0.24 ms)。之后,内部下游界面不断向右运动,同时外部下游界面两侧涡结构的卷起对界面起拉伸作用,使得内部下游界面与外部下游界面不断被压缩,导致两层界面之间的压力逐渐升高,从而诱导内部下游界面再次从正涡量转变为负涡量(图9中0.24~0.28 ms)。至此,内部下游界面上的涡量方向出现了由正→负→正→负的3次反向过程。
随后,气柱外部界面上的负涡量沿着界面向下游输运,诱导界面卷起形成涡串结构并逐渐合并大涡结构,而内部界面的正涡量在界面合并之后分别向上方运动并与外部界面的负涡量融合在一起,导致界面内形成正负涡量复杂交替的无序状态(图9中0.30~0.60 ms)。在涡量演化后期,随着界面上漩涡的发展,正负涡量逐渐分开,其中大量的负涡量在界面下游合并形成主涡结构,而部分正涡量则是在上游合并形成次级涡结构(图9中0.70~1.00 ms)。
激波与界面相互作用生成的涡强度可以通过界面上环量的变化来表征,根据环量
Γ 的定义:Γ=∫Dωds (14) 式中:D为计算区域,ds为微元面积。
图10显示了激波作用后环形气柱与圆形气柱界面上总环量随时间变化的对比。图10中,
Γa 和Γc 分别表示环形和圆形气柱界面上总环量,而|Γa| 和|Γc| 则分别表示环形和圆形气柱界面上的环量绝对值,其中环量绝对值定义为:|Γ|=∫D|ω|ds (15) 由图10可见,由于结构的对称性,无论是环形气柱还是圆形气柱,其界面上的总环量始终为零。但由于内部界面的存在,导致环形气柱界面上的环量绝对值在0.06 ms之后始终高于圆形气柱界面。
为了方便讨论界面上各环量的具体变化情况,分别定义正环量
Γ+ 与负环量Γ− :Γ+=∫Dω+ds (16) Γ−=∫Dω−ds (17) 式中:
ω+ 和ω− 分别为界面上的正涡量和负涡量。考虑到涡量分布的对称性,图11给出了上半部分界面上环量的变化曲线。其中Γ+aup 、Γ−aup 和Γaup 分别表示环形气柱上半部分界面上的正环量、负环量和总环量,而Γ+cup 、Γ−cup 和Γcup 则分别表示圆形气柱上半部分界面上的正环量、负环量和总环量。由图11中气柱上半部分界面的正环量、负环量和总环量的变化情况发现,由于透射激波与环形气柱内部界面相互作用的斜压效应,导致在0.06 ms之后环形气柱上半部分界面的正环量迅速增加,且后期环形气柱上半部分的正环量始终是高于圆形气柱的。而两种气柱的上半部分界面的负环量在0.14 ms之前基本保持一致,但0.14 ms之后,激波完全透射出气柱之后,负环量经历短暂的下降,且由于环形气柱界面内激波聚焦和反射激波作用,使得环形气柱的负环量下降更厉害。紧接着,两种气柱的负环量迅速增加,环形气柱的负环量反超圆形气柱,且一直保持到后期。在0.30 ms之后,两种气柱的上半部分总环量保持在一定的小范围内波动,如图11所示。
图12对比了环形气柱与圆形气柱的气体混合率的变化情况。气体混合率的定义为[24-25]:
ξ=1−∫Df(x,y,t)ρ(x,y,t)ds∫Dρ(x,y,t)ds (18) 式中:ξ为环境气体与界面内SF6的混合程度,f(x,y,t)为流体微元中SF6所占的质量分数,ρ(x,y,t)则为流体微元的密度。区域D包含了所有含有SF6气体的流体微元,即f(x,y,t) > 0。图12中
ξa 和ξc 分别表示环形和圆形气柱的气体混合率。由图12可知,在激波与上游界面相互作用的初始时期(0.01 ms之前),两种气体的混合率迅速上升,且环形界面的上升幅度明显高于圆形界面,而此时激波尚未与气柱界面完全作用,混合率的增加基本靠气体扩散,说明初始时刻重质气体与环境气体之间的接触面积大小决定了气体混合率初期增长的幅度。紧接着激波与气柱的界面相互作用,两种气柱的混合率均开始增长,其中环形气柱的混合率近似线性增长,而圆形气柱的混合率在0.2 ms之前增长速率较低,在0.2~0.6 ms之间增长速率有所增加,最终与环形气柱混合速率基本一致。但整个过程中,环形气柱的混合率始终保持高于圆形气柱的混合率,说明环形气柱的内部界面对气体混合有明显提升作用。3. 结 论
基于可压缩多组分Navier-Stokes控制方程,结合五阶WENO格式以及网格自适应加密技术和level-set方法,通过数值模拟方法研究了马赫数为1.23的入射激波与环形SF6气柱(内外半径分别为8和17.5 mm)界面的相互作用过程。相比于之前的实验结果,本文中数值模拟结果清晰地揭示了环形SF6气柱在激波作用下的界面形态、界面内复杂激波结构以及涡量分布的演化过程,得到以下主要结论。
(1) 透射激波与环形SF6气柱的内界面相互作用,诱导内界面生成“射流”结构,并进一步与下游界面相互作用,导致环形界面后期不仅在下游生成一对主涡结构,而且在界面上游生成一对次级涡和一个与朝向和运动方向相反的新的“射流”结构。
(2) 透射激波在环形SF6气柱内向下游传播过程中,形成了FPR结构向FNR结构转换的波系演变过程;而透射激波在穿过内部下游界面时形成局部高压区,诱导生成了一对前向传导激波和后向传导激波;另外,在内、外下游界面之间形成的复杂激波诱导内部下游界面上的压力梯度方向多次反向,最终导致内部下游界面上的涡量方向出现了由正→负→正→负的3次反向过程。
(3) 环形SF6气柱中的轻质气体能减缓界面长度的增长,同时也使得环形界面受压缩后更易向上下两侧扩展,导致环形界面高度增长迅速;内部气柱的存在减弱了前期小涡结构合并形成大涡结构过程中对界面高度与长度的影响。
(4) 环形SF6气柱早期的混合率由重质气体与环境气体之间的接触面积大小决定,因此环形界面由于内圆的存在,其混合率相比圆形气柱情况较大,但后期两者的混合速率基本一致。
-
表 1 气体参数
Table 1. Gas parameters
气体名称 比热比 摩尔质量/(g·mol−1) 密度/(kg·m−3) 空气 1.399 28.967 1.23 SF6 1.103 128.491 5.45 -
[1] LINDL J D, MCCRORY R L, CAMPBELL E M. Progress toward ignition and burn propagation in inertial confinement fusion [J]. Physics Today, 1992, 45(9): 32–40. DOI: 10.1063/1.881318. [2] YANG J, KUBOTA T, ZUKOSKI E E. Applications of shock-induced mixing to supersonic combustion [J]. AIAA Journal, 1993, 31(5): 854–862. DOI: 10.2514/3.11696. [3] BALAKRISHNAN K, MENON S. Characterization of the mixing layer resulting from the detonation of heterogeneous explosive charges [J]. Flow Turbulence and Combustion, 2011, 87(4): 639–671. DOI: 10.1007/s10494-011-9349-9. [4] 罗喜胜, 翟志刚, 司廷, 等. 激波诱导下的气体界面不稳定性实验研究 [J]. 力学进展, 2014, 44(1): 201407. DOI: 10.6052/1000-0992-14-028.LUO X S, ZHAI Z G, SI T, et al. Experimental study on the interfacial instability induced by shock waves [J]. Advances in Mechanics, 2014, 44(1): 201407. DOI: 10.6052/1000-0992-14-028. [5] ZOU L Y, LIU C L, TAN D W, et al. On interaction of shock wave with elliptic gas cylinder [J]. Journal of Visualization, 2010, 13(4): 347–353. DOI: 10.1007/s12650-010-0053-y. [6] 黄熙龙, 廖深飞, 邹立勇, 等. 激波与椭圆形重气柱相互作用的PLIF实验 [J]. 爆炸与冲击, 2017, 37(5): 829–836. DOI: 10.11883/1001-1455(2017)05-0829-08.HUANG X L, LIAO S F, ZOU L Y, et al. Experiment on interaction of shock and elliptic heavy-gas cylinder by using PLIF [J]. Explosion and Shock Waves, 2017, 37(5): 829–836. DOI: 10.11883/1001-1455(2017)05-0829-08. [7] ZHAI Z G, WANG M H, SI T, et al. On the interaction of a planar shock with a light polygonal interface [J]. Journal of Fluid Mechanics, 2014, 757: 800–816. DOI: 10.1017/jfm.2014.516. [8] LUO X S, WANG M H, SI T, et al. On the interaction of a planar shock with an SF6 polygon [J]. Journal of Fluid Mechanics, 2015, 773: 366–394. DOI: 10.1017/jfm.2015.257. [9] 沙莎, 陈志华, 薛大文, 等. 激波与SF6梯形气柱相互作用的数值模拟 [J]. 物理学报, 2014, 63(8): 085205. DOI: 10.7498/aps.63.085205.SHA S, CHEN Z H, XUE D W, et al. Richtmyer-Meshkov instability induced by the interaction between shock wave and SF6 isosceles trapezoid cylinders [J]. Acta Physica Sinica, 2014, 63(8): 085205. DOI: 10.7498/aps.63.085205. [10] 廖深飞, 邹立勇, 刘金宏, 等. 反射激波作用重气柱的Richtmyer-Meshkov不稳定性的实验研究 [J]. 爆炸与冲击, 2016, 36(1): 87–92. DOI: 10.11883/1001-1455(2016)01-0087-06.LIAO S F, ZOU L Y, LIU J H, et al. Experimental study of Richtmyer-Meshkov instability in a heavy gas cylinder interacting with reflected shock wave [J]. Explosion and Shock Waves, 2016, 36(1): 87–92. DOI: 10.11883/1001-1455(2016)01-0087-06. [11] 王震, 王涛, 柏劲松, 等. 流场非均匀性对非平面激波诱导的Richtmyer-Meshkov不稳定性影响的数值研究 [J]. 爆炸与冲击, 2019, 39(4): 041407. DOI: 10.11883/bzycj-2018-0342.WANG Z, WANG T, BAI J S, et al. Numerical study of non-uniformity effect on Richtmyer-Meshkov instability induced by non-planar shock wave [J]. Explosion and Shock Waves, 2019, 39(4): 041407. DOI: 10.11883/bzycj-2018-0342. [12] ORLICZ G C, BALASUBRAMANIAN S, PRESTRIDGE K P. Incident shock Mach number effects on Richtmyer-Meshkov mixing in a heavy gas layer [J]. Physics of Fluids, 2013, 25(11): 114101. DOI: 10.1063/1.4827435. [13] SHANKAR S K, LELE S K. Numerical investigation of turbulence in reshocked Richtmyer-Meshkov unstable curtain of dense gas [J]. Shock Waves, 2014, 24(1): 79–95. DOI: 10.1007/s00193-013-0478-z. [14] ZENG W G, PAN J H, SUN Y T, et al. Turbulent mixing and energy transfer of reshocked heavy gas curtain [J]. Physics of Fluids, 2018, 30(6): 064106. DOI: 10.1063/1.5032275. [15] DE FRAHAN M T H, MOVAHED P, JOHNSEN E. Numerical simulations of a shock interacting with successive interfaces using the discontinuous Galerkin method: the multilayered Richtmyer-Meshkov and Rayleigh-Taylor instabilities [J]. Shock Waves, 2015, 25(4): 329–345. DOI: 10.1007/s00193-014-0539-y. [16] LIANG Y, LIU L L, ZHAI Z G, et al. Evolution of shock-accelerated heavy gas layer [J]. Journal of Fluid Mechanics, 2020, 886: A7. DOI: 10.1017/jfm.2019.1052. [17] WANG G, WANG Y N, LI D D, et al. Numerical study on shock-accelerated gas rings [J]. Physics of Fluids, 2020, 32(2): 026102. DOI: 10.1063/1.5135762. [18] SAMTANEY R, ZABUSKY N J. Circulation deposition on shock-accelerated planar and curved density-stratified interfaces: models and scaling laws [J]. Journal of Fluid Mechanics, 1994, 269: 45–78. DOI: 10.1017/s0022112094001485. [19] YANG J, KUBOTA T, ZUKOSKI E E. A model for characterization of a vortex pair formed by shock passage over a light-gas inhomogeneity [J]. Journal of Fluid Mechanics, 1994, 258: 217–244. DOI: 10.1017/s0022112094003307. [20] 冯莉莉, 翟志刚, 司廷, 等. 激波诱导双层气柱演化的偏心效应研究 [J]. 气体物理, 2022, 7(2): 13–25. DOI: 10.19527/j.cnki.2096-1642.0959.FENG L L, ZHAI Z G, SI T, et al. Eccentric effect on evolution of shock-accelerated double-layer gas cylinder [J]. Physics of Gases, 2022, 7(2): 13–25. DOI: 10.19527/j.cnki.2096-1642.0959. [21] FENG L L, XU J R, ZHAI Z G, et al. Evolution of shock-accelerated double-layer gas cylinder [J]. Physics of Fluids, 2021, 33(8): 086105. DOI: 10.1063/5.0062459. [22] LOMBARDINI M, HILL D J, PULLIN D I, et al. Atwood ratio dependence of Richtmyer-Meshkov flows under reshock conditions using large-eddy simulations [J]. Journal of Fluid Mechanics, 2011, 670: 439–480. DOI: 10.1017/s0022112010005367. [23] WANG X S, YANG D G, WU J Q, et al. Interaction of a weak shock wave with a discontinuous heavy-gas cylinder [J]. Physics of Fluids, 2015, 27(6): 064104. DOI: 10.1063/1.4922613. [24] NIEDERHAUS J H J, GREENOUGH J A, OAKLEY J G, et al. A computational parameter study for the three-dimensional shock-bubble interaction [J]. Journal of Fluid Mechanics, 2008, 594: 85–124. DOI: 10.1017/s0022112007008749. [25] ZHENG C, ZHANG H H, CHEN Z H, et al. Interaction of cylindrical converging shocks with an equilateral triangular SF6 cylinder [J]. Physics of Fluids, 2019, 31(8): 086104. DOI: 10.1063/1.5094671. -












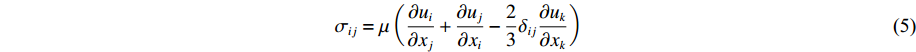





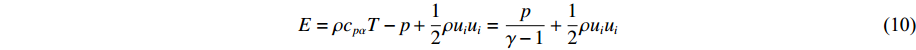





 下载:
下载:








































 下载:
下载: