Failure modes and response characteristics of finite-thickness aluminum targets under normal penetration of elliptical cross-section projectiles
-
摘要: 基于30 mm口径弹道炮平台,开展了3种不同椭圆横截面弹体在200~600 m/s撞击速度范围内正侵彻2A12铝靶的实验,获得了2A12铝靶的破坏形貌及弹体的剩余速度。在此基础上,建立了相应的数值模型,结合实验结果验证了所建模型的有效性,并系统分析了弹体横截面长短轴长度比对靶体的破坏情况及响应特性的影响。研究结果表明:弹体最大横截面面积是影响弹体剩余速度的主要因素,而弹体横截面长短轴长度比对弹体剩余速度的影响较弱;在圆形横截面弹体侵彻下靶体背部形成的花瓣大小和形状一致,空间分布均匀,而在椭圆横截面弹体侵彻下,随着弹体横截面长短轴长度比的增大,靶体背部形成的花瓣数量增加、尺寸变小,且在短轴方向的花瓣数量和靶体表面隆起高度均大于长轴方向的;靶体在圆形横截面弹体侵彻下的径向位移、径向应力和切向应力与其在椭圆横截面弹体侵彻下的显著不同,前者沿周向方向各点的变化规律基本一致,靶体处于简单的压缩状态,切向应力为零,而后者各点的应力状态与弹体横截面长短轴长度比和周向角密切相关,靶体受到压缩和剪切应力的耦合作用。Abstract: By means of a 30-mm-caliber ballistic gun platform, a series of experiments were carried out on 2A12 aluminum targets subjected to normal penetration by three kinds of 30CrMnSi2A steel projectiles with different elliptical cross-section shapes in the striking velocity range from 200 m/s to 600 m/s. The residual velocities of the projectiles and the failure modes of the targets were experimentally obtained. Based on the experimental results, the corresponding numerical models were established and verified. And the influences of the major-to-minor axis length ratios of the projectile cross-sections on the failure modes and response characteristics of the targets were systematically analyzed. The results show as follows. The maximum cross-sectional areas of the projectiles are the main factor affecting the residual velocities of the projectiles, while the major-to-minor axis length ratios of the projectile cross-sections have little effect on the residual velocities. Therefore, in engineering applications, the engineering model for the circular cross-section projectile penetrating a target can be directly used to calculate the residual velocity of the elliptical cross-section projectile with the same maximum cross-sectional area. In addition, under normal penetration of the circular cross-section projectiles, the sizes, shapes and distribution of the petals induced at the back faces of the targets are uniform. However, under normal penetration of the elliptical cross-section projectiles, as the major-to-minor axis length ratios of the projectile cross-sections increase, the numbers of the petals induced at the back faces of the targets increase and the petal sizes decrease, and the petal numbers and the uplifted height in the minor axis direction are greater than those in the major axis direction. The radial displacement, radial stress and tangential stress of the targets under the normal penetration of the elliptical cross-section projectiles are obviously different from those of the targets under the normal penetration of the circular cross-section projectiles. Under normal penetrations of the circular cross-section projectiles, the above response characteristics of the targets change basically the same along the circumferential directions and the targets are under simple compression states with the tangential stress of zero. But, under normal penetrations of the elliptical cross-section projectiles, the stress states of different points of the targets are closely related to the major-to-minor axis length ratios and the circumferential angles of the projectiles, and the targets are subjected to the coupling effects of the compression and shear stresses.
-
长杆弹是现阶段对付装甲目标的一种重要手段,国内外学者对动能弹侵彻威力的研究主要体现在弹体材料和结构两个方面。首先,在动能弹材料研究方面,除常用的钨合金和贫铀合金,近些年出现的以细晶钨合金[1]、钨丝/锆基非晶材料[2-4]作为材料的长杆弹,能够不同程度提高其侵彻能力;而在动能侵彻体结构上,出现了多种不同形式的异型结构侵彻体研究,包括卵形头部刻槽结构侵彻体[5-6]、侵彻体弹体刻槽结构[7-8]、变截面侵彻体[9]、管-杆伸出式异型侵彻体[10]以及异型截面长杆弹等。
异型截面长杆弹是指非圆截面的等截面长杆弹,现在对异型截面长杆弹的研究主要包括其飞行特性和侵彻威力两个方面,研究表明三角形和正方形截面长杆弹在较高飞行速度下可以获得更高的法向力[11]和更好的可控性[12];而对异型截面长杆弹的侵彻威力研究,始于Bless等[13]开展的三叉形与十字形异型截面长杆弹的实验和数值模拟研究,认为三叉形与十字形异型截面长杆弹侵彻威力并没有相同截面积的圆形截面弹好。国内学者也开展了异型截面长杆弹的研究,如大量长径比L/D=15、三种不同截面形状(等截面积的圆形、三角形、正方形)的93钨合金长杆弹垂直侵彻材料为38CrMoAl钢靶板实验研究[14-15],但实验结果与Bless等[13]所得结论不同,实验结果表明:两种异型截面弹在1 700~1 900 m/s的着靶速度范围内相对于圆形截面弹威力提高明显,并且三角形截面弹的威力更优于正方形截面弹,同时,在1700 m/s速度以下,与文献[16]中结果相似,两种异型截面形状长杆弹相对于圆形截面弹并没有明显优势。
在已有的实验基础[15-16]上,开展不同截面形状(圆形、三角形、正方形、十字形)的长径比L/D=8、93钨合金长杆弹垂直侵彻装甲钢靶板以及长径比L/D=15、不同截面形状(圆形、三角形、正方形、两种十字形)的45钢长杆弹侵彻45钢靶板实验,分析不同截面长杆弹侵彻后无量纲侵彻深度差异,并分析93钨合金长杆弹垂直侵彻装甲钢靶板弹坑截面形状和弹体头部裂纹。
1. 长杆弹侵彻半无限厚靶板问题的量纲分析
一般对于圆形等截面长杆弹侵彻半无限厚靶板问题,最终侵彻深度可以表示为:
H=f(σp,σt,Ep,Et,μp,μt,ρp,ρt,D,L,v,Λ) (1) 式中:H为最终侵彻深度,
σp 、σt 分别为长杆弹和靶板材料的动态强度,Ep 、Et 分别为弹/靶材料的杨氏模量,ρp 、ρt 分别为弹/靶材料密度,μp、μt为弹/靶材料的泊松比,v为长杆弹入射速度,L、D分别为长杆弹初始长度和直径,Λ 为长杆弹弹头形状系数。引入长杆弹截面形状系数
Ψ ,研究非圆形等截面长杆弹侵彻半无限板时截面形状对最终侵彻行为的影响,所以式(1)改写为:H=f(σp,σt,Ep,Et,μp,μt,ρp,ρt,D,L,v,Λ,Ψ) (2) 式(2)中自变量主要有13个,研究对象是一个典型的纯力学问题,其基本量纲有3个,取长杆弹材料的密度
ρp 、初始长度L和入射速度v这3个物理量为参考物理量,此14个物理量中弹/靶材料的泊松比、弹体头部形状系数和截面形状系数4个量是无量纲量,其他10个物理量的量纲如表1所示。表 1 各物理量的量纲Table 1. Dimension of each physical quantity物理量 M L T ρp 1 −3 0 L 0 1 0 v 0 1 −1 σp 1 −1 −2 σt 1 −1 −2 Ep 1 −1 −2 Et 1 −1 −2 ρt 1 −3 0 以长杆弹材料的密度
ρp 、初始长度L和入射速度v这3个物理量为参考物理量,对上表进行类似初等变换,可以得到表2。表 2 各物理量量纲(变换后)Table 2. Dimension of each physical quantity (after change)物理量 ρp L V ρp 1 0 0 L 0 1 0 v 0 0 1 σp 1 0 2 σt 1 0 2 Ep 1 0 2 Et 1 0 2 ρt 1 0 0 根据表2各物理量量纲,式(1)形式变换为:
HL=f(σpρpv2,σtσp,Epρpv2,EtEp,ρtρp,LD,μp,μt,Λ,Ψ) (3) 式中:
H/L 为无量纲侵彻深度,是表征长杆弹侵彻效率的重要参数;L/D 为长杆弹长径比。本文针对长杆弹截面形状系数Ψ 对无量纲侵彻深度的影响开展研究。已有研究表明[17],弹、靶材料的泊松比对长杆弹的最终侵彻深度影响可以忽略,从物理意义出发对上式进行简化、变换,上式可表述为:
HL=f(σpρpv2/2,σtσp,√Ep/ρp2v2,√EtEp/ρtρp2,ρtρp,LD,Λ,Ψ) (4) 上式可以进一步变换为:
HL=f(σpρpv2/2,σtσp,vcp,ctcp,ρtρp,LD,Λ,Ψ) (5) 式中:cp与ct分别表示弹、靶材料的弹性声速;
σp/(ρpv2/2) 为长杆弹材料屈服应力对于单位体积入射动能的比值;v/cp 为长杆弹相对材料弹性声速的无量纲入射速度,σt/σp 、ct/cp 、ρt/ρp 分别为弹靶材料屈服强度、弹性声速和密度的比值。2. 实验研究
2.1 实验设置
实验以25 mm口径弹道炮作为发射平台,设计并开展了两组实验:(1) 4种长径比为
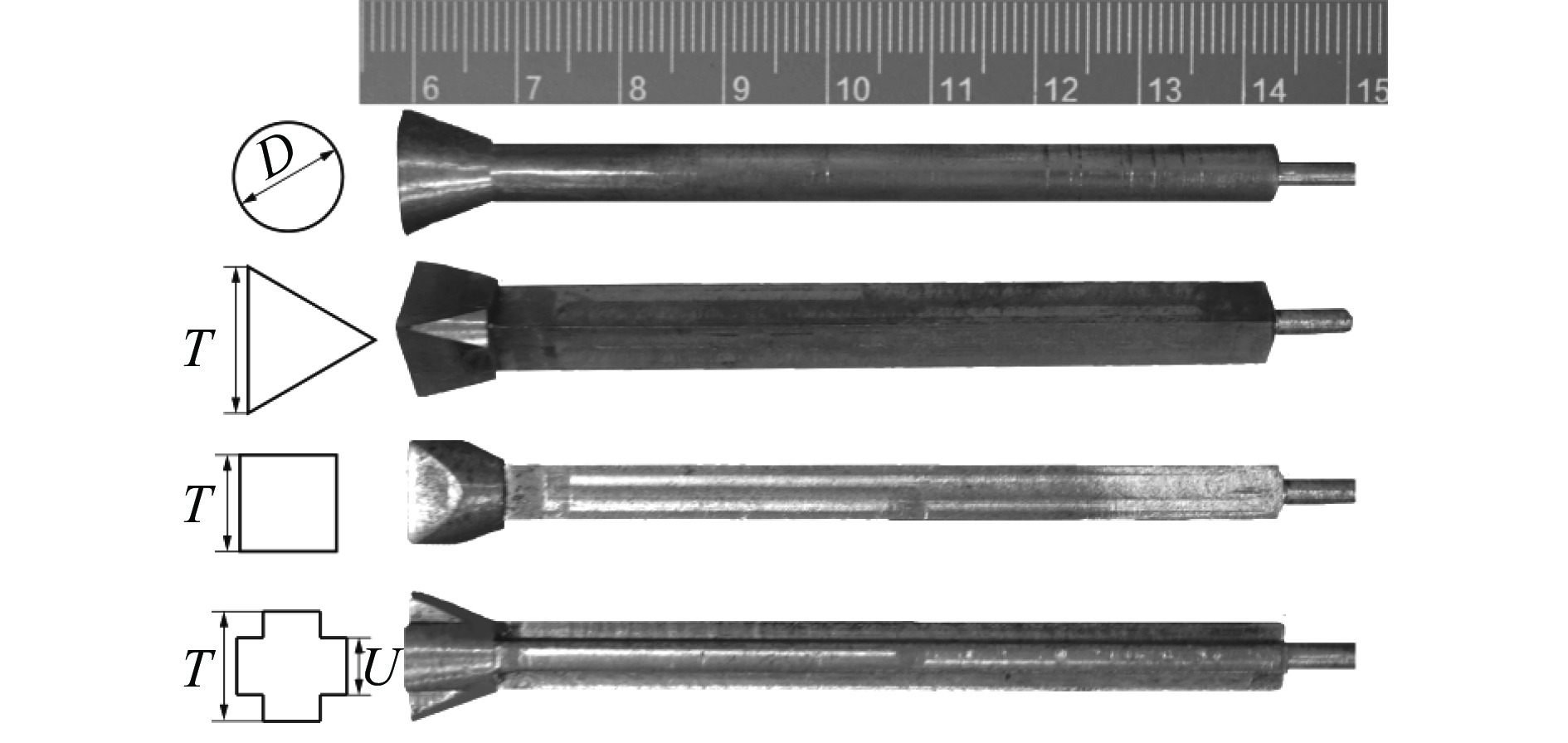
L/D=8 、截面形状不同(圆形、三角形、正方形、十字形)的93钨合金长杆弹垂直侵彻装甲钢(603钢)靶板;(2) 5种长径比L/D=15 、截面形状不同(圆形、三角形、正方形、两种十字形)的45钢长杆弹侵彻45钢靶板实验,具有相同材料的几种长杆弹弹芯之间除去弹芯截面形状不同外,弹芯长度、质量和截面积均相同,图1给出了45钢材料的四种截面形状的弹芯,93钨合金材料弹芯的结构与之相似。表3给出了实验所用弹芯的具体尺寸。表 3 弹芯尺寸Table 3. Projectile dimensions序号 截面形状 材料 L/mm D*/mm T/mm U/mm 1 圆形 93钨合金 64 8 − − 2 三角形 93钨合金 64 8 10.77 − 3 正方形 93钨合金 64 8 7.09 − 4 十字形 93钨合金 64 8 8.08 4.2 5 圆形 45钢 90 6 − − 6 三角形 45钢 90 6 8.08 − 7 正方形 45钢 90 6 5.32 − 8 十字形1 45钢 90 6 3.16 6.06 9 十字形2 45钢 90 6 6.67 4.27 注:D*为等效圆直径,表达式为D∗=√4S/π ;S为弹芯截面积。 目前次口径脱壳穿甲弹多采用环形齿结构,但考虑到环形齿的设置会破坏截面形状,故本文实验用弹丸采用底推结构,如图2所示,弹丸由底推、底推片、弹托、弹芯、风帽五部分构成。靶板厚度设计为110 mm。
图3给出本文实验布置示意图,弹丸由25 mm滑膛炮发射,为避免长杆弹长距离飞行造成的章动,炮口距离与靶板之间距离设置为(8±0.2) m。由于该距离内长杆弹速度衰减可以忽略,所以以飞行过程中平均速度作为着靶速度。
2.2 实验结果
开展两种材料、不同截面长杆弹垂直侵彻半无限靶板实验,通过调整装药量,控制长杆弹着靶速度在1 300~1 700 m/s,实验后,测量并计算每发长杆弹的最终侵彻深度,得到表4所示结果。
表 4 实验结果Table 4. Experimental results序号 弹芯材料 靶板材料 截面形状 着靶速度/(m·s−1) 侵彻深度/mm 序号 弹芯材料 靶板材料 截面形状 着靶速度/(m·s−1) 侵彻深度/mm 1 93W 603钢 圆形 1615 68.0 25 45钢 45钢 圆形 1538 42.5 2 93W 603钢 三角形 1429 53.0 26 45钢 45钢 圆形 1719 55.0 3 93W 603钢 三角形 1462 54.5 27 45钢 45钢 圆形 1747 53.8 4 93W 603钢 三角形 1596 69.3 28 45钢 45钢 三角形 1288 27.5 5 93W 603钢 三角形 1601 64.9 29 45钢 45钢 三角形 1298 25.5 6 93W 603钢 正方形 1371 54.7 30 45钢 45钢 三角形 1371 25.5 7 93W 603钢 正方形 1374 54.7 31 45钢 45钢 三角形 1548 44.0 8 93W 603钢 正方形 1467 54.5 32 45钢 45钢 三角形 1558 41.5 9 93W 603钢 正方形 1489 60.0 33 45钢 45钢 三角形 1695 53.5 10 93W 603钢 正方形 1625 76.0 34 45钢 45钢 正方形 1376 36.0 11 93W 603钢 正方形 1639 72.0 35 45钢 45钢 正方形 1388 35.5 12 93W 603钢 正方形 1642 75.5 36 45钢 45钢 正方形 1401 32.0 13 93W 603钢 十字形 1388 52.0 37 45钢 45钢 正方形 1412 34.0 14 93W 603钢 十字形 1415 54.2 38 45钢 45钢 正方形 1582 46.0 15 93W 603钢 十字形 1452 60.0 39 45钢 45钢 正方形 1589 50.0 16 93W 603钢 十字形 1473 60.7 40 45钢 45钢 正方形 1702 63.0 17 93W 603钢 十字形 1495 63.5 41 45钢 45钢 十字形1 1358 15.5 18 93W 603钢 十字形 1571 67.0 42 45钢 45钢 十字形1 1464 15.5 19 93W 603钢 十字形 1593 75.8 43 45钢 45钢 十字形1 1472 15.0 20 45钢 45钢 圆形 1317 26.5 44 45钢 45钢 十字形1 1502 51.0 21 45钢 45钢 圆形 1335 24.5 45 45钢 45钢 十字形1 1718 66.0 22 45钢 45钢 圆形 1423 32.5 46 45钢 45钢 十字形2 1469 17.0 23 45钢 45钢 圆形 1478 35.0 47 45钢 45钢 十字形2 1445 16.5 24 45钢 45钢 圆形 1516 37.0 48 45钢 45钢 十字形2 1445 15.5 由于不同截面弹中弹托结构尺寸不同造成整弹质量的不同,以及相同装药量下弹丸飞行速度存在章动,实验中不同截面长杆弹的着靶速度无法做到一一对应。为了更直观地分析截面形状对侵彻威力的影响规律,做散点图图4,横坐标为着靶速度、纵坐标为无量纲侵彻深度(实际侵彻深度与长杆弹初始长度的比值,H/L),图4中空心点和实心点分别为93钨合金侵彻装甲钢和45钢长杆弹侵彻45钢靶板实验结果,并对同一截面形状长杆弹侵彻数据做线性拟合。
从图4可以直观的发现:在实验速度范围内,几种不同材料、不同截面长杆弹无量纲侵彻深度与着靶速度之间具有较好的线性关系,几种异型截面长杆弹均比圆形截面杆弹有不同程度的侵彻增益,且两组实验中十字形截面异形弹均具有最优异的侵彻能力,正方形截面异型弹次之。
3. 实验结果分析
3.1 截面形状对无量纲侵彻深度影响分析
上述实验发现三角形截面、正方形截面和十字形截面的长杆弹在相同动能下均比圆形截面长杆弹侵彻深度高,且三种异型截面长杆弹直接的侵彻威力也不相同,为了方便研究截面形状对侵彻行为的影响规律,考虑异型截面与圆形截面长杆弹侵彻效率之间存在如下关系:
HL=F(HL)0 (6) 式中:(H/L)0为等截面积的圆形截面长杆弹无量纲侵彻深度,即当长杆弹截面为圆形时
F=1 。根据式(6),定义长杆弹截面形状对无量纲侵彻深度
H/L 影响能力的无量纲参数F为F=(HL)/(HL)0 (7) 首先,依据图4中45#钢材料的长杆弹侵彻数据的线性拟合结果,分别计算不同速度下的无量纲参数F的值,如图5(a)所示。发现除圆形截面长杆弹外,三角形截面长杆弹的无量纲参数F的值同样为一个常数,约为
F=1.05 ,而正方形和十字形长杆弹,随着靶速度提高,F值均呈现单调增加趋势,且十字形截面长杆弹F值对速度的敏感度高于正方形截面,在1 300~1 850 m/s范围内,正方形和十字形截面长杆弹的F取值分别从1.11、1.13增加到1.16、1.26,增长幅度分别为4.5%、11.5%,随着靶速度的增加,无量纲参数F的增加呈放缓趋势。对于图4中93钨合金侵彻装甲钢的实验结果,由于圆形截面长杆弹侵彻实验数据较少,无法进行线性拟合,所以以三角形截面长杆弹侵彻数据的线性拟合结果为参照,如图5(b)所示,进而分别计算出93钨合金材料的正方形和十字形截面长杆弹在1 300~1 850 m/s范围内不同速度时的
F′ 值,F′=(H/L)/(H/L)▴ ,其中(H/L)▴ 表示三角形截面长杆弹侵彻深度。与图5(a)中结果相似,正方形和十字形长杆弹,随着靶速度提高,F′值均呈现单调增加趋势,且十字形截面长杆弹F′值对着靶速度的敏感度高于正方形截面和三角形截面。为了对比两组实验中具有相同截面的两种长杆弹的侵彻增益,同时考虑到缺少93钨合金材料的圆形长杆弹侵彻数据,以两组实验中三角形截面长杆弹为参照,得到图5(c)中几种长杆弹相对于三角形截面弹的无量纲侵彻增益F′,结果表明:两组实验中具有相同截面形状的长杆弹的侵彻增益F′值并不相同且相差较大,在整个速度区间内,第一组实验(93W长杆弹侵彻装甲钢靶板)与第二组实验(45钢长杆弹侵彻45钢靶板)相比,前者中的正方形和十字形截面的无量纲侵彻增益F′均小于后者,且前者无量纲侵彻增益F′的速度敏感性低于后者。
由于两组实验中所设计长杆弹的长径比以及弹靶材料性能包括强度、密度、弹性模量等部分参数或全部参数不同,本次实验无法确定分析函数
F=F(σp,σt,ρp,ρt,L/D) 的具体形式。综上所述,在一定速度范围内,三种异型截面长杆弹的截面形状对侵彻深度的影响程度不同,十字形截面影响程度最大即无量纲参数F或F′在实验速度范围内取值最大,正方形次之,三角形最小;相同速度下,不同弹靶材料、具有不同长径比的长杆弹截面形状对侵彻深度的影响程度不同;三种异型截面长杆弹的截面形状对侵彻深度的影响程度对速度的敏感程度不同,随着靶速度的变化,45钢长杆弹的三角形截面长杆弹相对于圆形截面长杆弹侵彻深度增益不变,而正方形和十字形截面长杆弹,随着着靶速度的增加,相对于圆形截面长杆弹侵彻增益增加,三种截面形状长杆弹中十字形截面对侵彻深度的影响程度即无量纲参数F的速度敏感性最大,正方形次之。
3.2 弹靶宏观分析
为分析异型截面长杆弹侵彻威力优于圆形截面长杆弹的机理,针对93钨合金侵彻装甲钢后靶板弹坑进行宏观分析,研究不同截面长杆弹侵彻后弹、靶差异。
93钨合金侵彻装甲钢实验后弹坑截面形状如图6所示,从上到下四行分别表示圆形、三角形、正方形、十字形截面长杆弹以不同速度侵彻后的弹坑截面,从图中可以发现圆形截面长杆弹侵彻后弹坑截面为圆形,三角形截面长杆弹侵彻后弹坑截面呈现明显的“圆弧三角形”的形态,而正方形和十字形截面长杆弹侵彻后弹坑截面形状接近圆形,尤其当着靶速度在接近或高于1 600 m/s时,两种截面长杆弹侵彻后弹坑截面形状与圆形截面长杆弹侵彻后的弹坑截面形状基本相同。
几种截面长杆弹侵彻后弹底残渣分布明显不同,如图7所示,虚线上下两图为同一弹坑不同拍摄方向照片,两图拍摄方向分别与弹轴呈90°、45°夹角。一般93钨合金在侵彻过程中,弹头形状为“蘑菇头”状,弹靶材料由于剧烈的塑性变形、破碎所形成的“残渣”,通过长杆弹与弹坑内壁之间的缝隙排出,其中,圆形截面长杆弹头部呈现轴对称的“蘑菇头”形状,残渣均匀地从长杆弹弹体周围向后运动,如图7(a)、图8(a)所示;三角形截面长杆弹头部“蘑菇头”为非轴对称形状,残渣从长杆弹截面三角形周围非均匀排出,从“边”的中部排出最多,最终形成的蘑菇头截面形状为“圆弧三角形”,与弹坑截面形状相同,如图7(b)、图8(b)所示;同样的,正方形和十字形截面长杆弹侵彻头部外轮廓近似为轴对称“蘑菇头”,但由于长杆弹的“非圆”特性,“残渣”从长杆弹截面的周向排出是非均匀的,即从截面“边”的部分排渣多,从“角”的部分排渣少,如图7(c)、7(d)和图8(c)、8(d)所示,同时由于这两种截面形状的特点,所产生的弹坑形状更接近于圆形,如图8所示,随着着靶速度的提高,弹靶材料的塑性流动增强,最终产生的弹坑基本为圆形,如图6中实验结果所示。几种异型截面长杆弹侵彻过程中“残渣”周向分布的非均匀性是异型截面长杆弹侵彻效率提高的一种可能原因。
观察长杆弹侵彻后的残余弹体发现,三种异型截面长杆弹侵彻后弹底侧边出现裂纹,三角形和正方形截面弹体侧边裂纹与弹轴方向之间有一定程度夹角,其中三角形截面弹体裂纹方向有突变,如图9所示,靠近弹底部分裂纹方向平行于弹轴方向,远离弹底部分裂纹与弹轴方向夹角为(27±1)°,正方形截面弹体裂纹方向与弹轴方向夹角为(23.5°截面弹体),同时,而十字形截面弹体裂纹与弹轴方向夹角在(0±5)°之间,弹体裂纹基本与弹轴方向平行。通过上述分析发现,三种异型截面长杆弹在侵彻过程中“蘑菇头”外侧能够形成与弹轴方向夹角小于30°,的裂纹,使得“蘑菇头”外径小于圆形截面弹体“蘑菇头”外径,即形成一种结构上的“自锐性”,最终使得三种截面长杆弹相对于圆形截面长杆弹具有更高的侵彻深度。
4. 结 论
本文开展了截面形状分别为圆形、三角形、正方形、十字形的长径比L/D=8、材料为93钨合金的长杆弹垂直侵彻装甲钢靶板的实验,以及长径比为L/D=15、材料为45钢的长杆弹垂直侵彻45钢靶板的实验,得到实验后长杆弹的无量纲侵彻深度与着靶速度的关系,针对93钨合金材料长杆弹垂直侵彻装甲钢靶板实验,对弹坑和残余弹体宏观形貌进行分析,得到以下结论:
(1)在本文实验速度范围1 350~1 700 m/s内,几种异型截面长杆弹均比圆形截面杆弹有不同程度的侵彻增益,两组实验中十字形截面异形弹均在相同速度下均具有最优异的侵彻能力,正方形截面异型弹次之;
(2)相对于圆形截面长杆弹的无量纲侵彻深度
(H/L)0 ,定义长杆弹截面形状对侵彻威力影响能力的无量纲参数F=(H/L)/(H/L)0 ,其中,对于三角形截面长杆弹在不同着靶速度侵彻时无量纲参数F为定值,对于正方形和十字形截面长杆弹无量纲参数F随着靶速度的增加而增加;(3)几种截面长杆弹侵彻靶板后的弹坑截面不同,其中三角形长杆弹侵彻后的弹坑截面为圆弧三角形,其余近似为圆形;
(4)三种异型截面长杆弹侵彻后“蘑菇头”部分的外侧有裂纹产生,形成结构“自锐性”,是异型截面长杆弹侵彻威力增加的重要原因。
-
表 1 三种弹体主要参数
Table 1. Main parameters of three projectiles
弹体类型 弹体轮廓 2a/mm 2b/mm β L/mm ψ m/g C1 
23.6 23.6 1.00 43.2 3.6 360 T1 
30.0 18.6 1.61 43.2 5.6 360 T2 
30.0 24.0 1.25 43.2 3.5 360 表 2 弹体正侵彻铝靶的实验结果
Table 2. Experimental results for normal penetration of projectiles into aluminum targets
弹体 v0/(m·s−1) vr/(m·s−1) α/(°) γ/(°) C1-1 402.3 336.0 1.08 +0.63 C1-2 310.7 214.5 0.34 +0.19 C1-3 256.2 120.3 0.25 +0.17 C1-4 566.5 522.5 1.11 −0.68 T1-1 402.0 338.0 1.26 −0.62 T1-2 229.4 0 2.03 −1.51 T1-3 570.3 531.6 1.91 −1.49 T2-1 405.3 322.7 0.76 −0.30 T2-2 229.5 0 2.69 −2.39 T2-3 569.3 509.2 1.57 −1.13 表 3 材料参数
Table 3. Material parameters
表 4 弹体剩余速度模拟结果与实验结果的对比
Table 4. Comparison of residual velocities of projectiles between simulation and experiment
弹体类型 v0/(m·s−1) vr/(m·s−1) ɛr/% 实验 数值模拟 C1 256.2 120.3 109.2 −9.2 310.7 214.5 202.7 −5.5 402.3 336.0 323.3 −3.8 566.5 522.5 510.7 −2.3 T1 229.4 0 21.3 402.0 338.0 323.5 −4.3 570.3 531.6 514.5 −3.2 T2 229.5 0 0 0 405.3 322.7 302.5 −6.3 569.3 509.2 497.5 −2.3 表 5 靶体背部塑性应变区域范围对比
Table 5. Comparison of plastic strain ranges on the back of targets
弹体类型 2a/mm 2b/mm 长轴最大坐标/mm 短轴最大坐标/mm 长轴相对增量/% 短轴相对增量/% C1 23.60 23.60 18.55 18.55 57.20 57.20 T3 26.40 21.10 17.30 18.08 31.06 71.37 T1 30.00 18.60 18.13 20.10 20.87 116.13 T4 33.36 16.68 19.13 22.30 14.69 167.39 -
[1] VEDERNIKOV Y A, LEVIN V A, KHUDYAKOV Y S. Evolution and comparative analysis of group armor- and aeroballistics of ancient and modern ruled and poly-wedge arrows [J]. Bulletin of the Novosibirsk Computing Center. Series: Mathematical Modeling in Geophysics, 2005, 10: 93–116. [2] DAI X H, WANG K H, LI M R, et al. Rigid elliptical cross-section ogive-nose projectiles penetration into concrete targets [J]. Defence Technology, 2021, 17(3): 800–811. DOI: 10.1016/j.dt.2020.05.011. [3] MA X H, ZHANG Q M, ZHANG X W. A model for rigid asymmetric ellipsoidal projectiles penetrating into metal plates [J]. International Journal of Impact Engineering, 2022, 163: 104140. DOI: 10.1016/j.ijimpeng.2021.104140. [4] BEN-DOR G, DUBINSKY A, ELPERIN T. Optimal 3D impactors penetrating into layered targets [J]. Theoretical and Applied Fracture Mechanics, 1997, 27(3): 161–166. DOI: 10.1016/S0167-8442(97)00018-9. [5] BEN-DOR G, DUBINSKY A, ELPERIN T. A model for predicting penetration and perforation of FRP laminates by 3-D impactors [J]. Composite Structures, 2002, 56(3): 243–248. DOI: 10.1016/S0263-8223(02)00009-0. [6] YAKUNINA G Y. The construction of optimum three-dimensional shapes within the framework of a model of local interaction [J]. Journal of Applied Mathematics and Mechanics, 2000, 64(2): 289–298. DOI: 10.1016/S0021-8928(00)00051-4. [7] YAKUNINA G Y. The optimum non-conical and asymmetrical three-dimensional configurations [J]. Journal of Applied Mathematics and Mechanics, 2000, 64(4): 583–591. DOI: 10.1016/S0021-8928(00)00084-8. [8] WOO H J. Cavity expansion analysis of non-circular cross-sectional penetration problems [D]. Austin, Texas, USA: The University of Texas at Austin, 1997: 132–154. [9] BLESS S J. Penetration mechanics of non-circular rods [J]. AIP Conference Proceedings, 1996, 370(1): 1119–1122. DOI: 10.1063/1.50861. [10] 杜忠华, 曾国强, 余春祥, 等. 异型侵彻体垂直侵彻半无限靶板试验研究 [J]. 弹道学报, 2008, 20(1): 19–21.DU Z H, ZENG G Q, YU C X, et al. Experimental research of novel penetrator vertically penetrating semi-infinite target [J]. Journal of Ballistics, 2008, 20(1): 19–21. [11] 杜忠华, 朱建生, 王贤治, 等. 异型侵彻体垂直侵彻半无限靶板的分析模型 [J]. 兵工学报, 2009, 30(4): 403–407. DOI: 10.3321/j.issn:1000-1093.2009.04.005.DU Z H, ZHU J S, WANG X Z, et al. Analytical model on non-circular penetrator impacting semi-infinite target perpendicularly [J]. Acta Armamentarii, 2009, 30(4): 403–407. DOI: 10.3321/j.issn:1000-1093.2009.04.005. [12] 高光发, 李永池, 刘卫国, 等. 长杆弹截面形状对垂直侵彻深度的影响 [J]. 兵器材料科学与工程, 2011, 34(3): 5–8. DOI: 10.3969/j.issn.1004-244X.2011.03.002.GAO G F, LI Y C, LIU W G, et al. Influence of the cross-section shapes of long rod projectile on the vertical penetration depth [J]. Ordnance Material Science and Engineering, 2011, 34(3): 5–8. DOI: 10.3969/j.issn.1004-244X.2011.03.002. [13] DONG H, LIU Z H, WU H J, et al. Study on penetration characteristics of high-speed elliptical cross-sectional projectiles into concrete [J]. International Journal of Impact Engineering, 2019, 132: 103311. DOI: 10.1016/j.ijimpeng.2019.05.025. [14] DONG H, WU H J, LIU Z H, et al. Penetration characteristics of pyramidal projectile into concrete target [J]. International Journal of Impact Engineering, 2020, 143: 103583. DOI: 10.1016/j.ijimpeng.2020.103583. [15] GAO X D, LI Q M. Trajectory instability and convergence of the curvilinear motion of a hard projectile in deep penetration [J]. International Journal of Mechanical Sciences, 2017, 121: 123–142. DOI: 10.1016/j.ijmecsci.2016.12.021. [16] 高旭东, 李庆明. 带攻角斜侵彻混凝土的弹道偏转分析 [J]. 兵工学报, 2014, 35(Supp1 2): 33–39.GAO X D, LI Q M. Trajectory analysis of projectile obliquely penetrating into concrete target at attack angle [J]. Acta Armamentarii, 2014, 35(Supp1 2): 33–39. [17] 王浩, 武海军, 闫雷, 等. 椭圆横截面弹体斜贯穿双层间隔薄钢板失效模式 [J]. 兵工学报, 2020, 41(Suppl 2): 1–11. DOI: 10.3969/j.issn.1000-1093.2020.S2.001.WANG H, WU H J, YAN L, et al. Failure mode of oblique perforation of truncated ogive-nosed projectiles with elliptic cross-section into double-layered thin steel plate with gap space [J]. Acta Armamentarii, 2020, 41(Suppl 2): 1–11. DOI: 10.3969/j.issn.1000-1093.2020.S2.001. [18] 王浩, 潘鑫, 武海军, 等. 椭圆截面截卵形刚性弹体正贯穿加筋板能量耗散分析 [J]. 爆炸与冲击, 2019, 39(10): 103203. DOI: 10.11883/bzycj-2018-0350.WANG H, PAN X, WU H J, et al. Energy dissipation analysis of elliptical truncated oval rigid projectile penetrating stiffened plate [J]. Explosion and Shock Waves, 2019, 39(10): 103203. DOI: 10.11883/bzycj-2018-0350. [19] LANDKOF B, GOLDSMITH W. Petalling of thin, metallic plates during penetration by cylindro-conical projectiles [J]. International Journal of Solids and Structures, 1985, 21(3): 245–266. DOI: 10.1016/0020-7683(85)90021-6. [20] 张中国, 黄风雷, 段卓平, 等. 弹体侵彻带加强筋结构靶的实验研究 [J]. 爆炸与冲击, 2004, 24(5): 431–436. DOI: 10.3321/j.issn:1001-1455.2004.05.009.ZHANG Z G, HUANG F L, DUAN Z P, et al. The experimental research for projectile penetrating the structural target with rebar [J]. Explosion and Shock Waves, 2004, 24(5): 431–436. DOI: 10.3321/j.issn:1001-1455.2004.05.009. [21] CHEN Y, WANG Y, TANG P, et al. Impact characteristics of stiffened plates penetrated by sub-ordnance velocity projectiles [J]. Journal of Constructional Steel Research, 2008, 64(6): 634–643. DOI: 10.1016/j.jcsr.2007.12.006. [22] SONG W D, NING J G, WANG J. Normal impact of truncated oval-nosed projectiles on stiffened plates [J]. International Journal of Impact Engineering, 2008, 35(9): 1022–1034. DOI: 10.1016/j.ijimpeng.2007.05.008. [23] 徐双喜, 吴卫国, 李晓彬, 等. 截锥形弹穿甲单加筋板的破坏特性 [J]. 爆炸与冲击, 2011, 31(1): 62–68. DOI: 10.11883/1001-1455(2011)01-0062-07.XU S X, WU W G, LI X B, et al. Falure characteristics of a conical projectile penetrating single stiffened plate [J]. Explosion and Shock Waves, 2011, 31(1): 62–68. DOI: 10.11883/1001-1455(2011)01-0062-07. [24] LIU J W, ZHANG X F, WEI H Y, et al. Study on the penetration of elliptical cross-section projectiles into concrete targets: theory and experiment [J]. Latin American Journal of Solids and Structures, 2022, 19(3): 23. DOI: 10.1590/1679-78256939. [25] 李磊, 张先锋, 吴雪, 等. 不同硬度30CrMnSiNi2A钢的动态本构与损伤参数 [J]. 高压物理学报, 2017, 31(3): 239–248. DOI: 10.11858/gywlxb.2017.03.005.LI L, ZHANG X F, WU X, et al. Dynamic constitutive and damage parameters of 30CrMnSiNi2A steel with different hardnesses [J]. Chinese Journal of High Pressure Physics, 2017, 31(3): 239–248. DOI: 10.11858/gywlxb.2017.03.005. [26] 米双山, 张锡恩, 陶贵明. 钨球侵彻LY-12铝合金靶板的有限元分析 [J]. 爆炸与冲击, 2005, 25(5): 477–480. DOI: 10.11883/1001-1455(2005)05-0477-04.MI S S, ZHANG X E, TAO G M. Finite element analysis of spherical fragments penetrating LY-12 aluminum alloy target [J]. Explosion and Shock Waves, 2005, 25(5): 477–480. DOI: 10.11883/1001-1455(2005)05-0477-04. -


















 下载:
下载:


















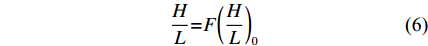
















 下载:
下载: