Failure law of shallow buried reinforced concrete arch structure under secondary explosion of conventional weapons
-
摘要: 为研究常规武器二次爆炸作用下土中浅埋拱结构的破坏规律，对土中浅埋钢筋混凝土直墙拱结构进行爆炸试验和数值模拟。试验对结构模型设置多个缩比工况，同时，利用LS-DYNA对3组工况进行数值模拟。通过对比土中测点压力、结构测点速度和结构挠度等数据，发现模拟结果与试验结果基本一致并拓展了二次爆炸的数值模拟工况。结果表明：比例爆距设置在0.4~0.6 m/kg1/3，以保证结构以整体破坏为主。综合结构毁伤宏观描述和结构最大挠跨比，对整体作用下结构的毁伤等级进行划分。通过讨论结构的初始毁伤及不同爆炸顺序时钢筋混凝土直墙拱结构的破坏规律，结构受爆炸作用发生开裂、弯曲等破坏时，部分混凝土因开裂或进入塑性而退出工作，从而导致结构的刚度发生改变；结构最终毁伤程度受打击顺序影响，初次爆炸对结构最终损伤影响比重较大。Abstract: The failure law of shallow buried reinforced concrete straight wall arch structure in soil under secondary explosion of conventional weapons was studied by explosion test and numerical simulation. Test structure adopts scale model based on similarity principle. Three groups of six shots were set up in the test. LS-DYNA is used to simulate the three groups of working conditions. By comparing the pressure of the measuring point in the soil, the speed of the structural measuring point, the structural deflection and other data, it is found that the simulation results are basically consistent with the experimental results. After comparing the numerical simulation results with the test, the numerical simulation conditions of the secondary explosion are expanded. When the comparison verifies that the numerical simulation is consistent with the experimental results, the secondary explosion conditions under the action of conventional weapons are simulated to study the dynamic response of structures under repeated impacts. Through calculation, it is found that when the proportional distance is set between 0.4-0.6 m/kg1/3, the damage of the structure is mainly caused by the overall damage. Combined with the macroscopic description of structural damage and the maximum deflection span ratio, the damage grade of the structure under the overall effect is divided. By discussing the initial damage of the structure and the failure law of reinforced concrete straight wall arch structure under different explosion sequences, the following conclusions are obtained: when the structure is damaged by explosion, such as cracking and bending, some concrete is out of work due to cracking or entering plasticity, resulting in the change of stiffness of the structure. The final damage degree of the structure is affected by the strike sequence, and the effect of initial explosion on the final damage of structure is greater than that of secondary explosion.
-
Key words:
- shallow buried tunnel /
- reinforced concrete structure /
- secondary explosion /
- failure law
-
爆轰推进是基于爆轰燃烧的新型推进技术，具有热循环效率高的显著优势，在水下推进领域具有重要的应用前景[1-3]。与其他推进系统在水介质中的工作过程相似，高速爆轰燃气射流进入水中时，外部水流场的背压效应会限制燃气的迅速扩散，大量进入水中的燃气形成燃气泡，并形成压力波在水中传播[4-6]。一般认为，在推进系统尾部加装喷管，能够提升推进系统性能[7]，但在水下流场中，燃气泡的演变过程受到推进系统喷管内气体流动的影响，同时燃气泡的存在也会对喷管内流场与外部水流场产生反作用[8]。因此，研究喷管对水下爆轰燃气射流气泡形态和压力特征的影响具有重要意义。
水下爆轰燃气射流是一个复杂的多相流问题，主要可以分为2个部分：一是爆轰波以及气泡脉动产生的复杂压力波系；二是高速燃气射流进入水中时气泡形态的演化。针对水下爆轰燃气射流的研究中，Frolov等[9-10]提出了脉冲爆轰水力冲压发动机（pulsed detonation hydroramjet, PDH）的概念，PDH通过周期性点燃发动机中的混合燃气产生爆轰波，由爆轰波退化的冲击波与导水管中的气液混合物相互作用，使得气液混合物加速流出导水管而产生推力。Frolov等[9-10]分析了发动机单次爆轰形成的燃气射流特性，得到了冲击波传播、衰减以及冲击波形成的复杂波系的相关结果。随后Liu等[11-12]进行了水下单次爆轰实验与模拟研究，通过高速摄影捕捉到水下爆轰燃气射流气泡脉动的演化过程及特征结构，并分析了气泡脉动过程中的水中压力场特征。侯子伟等[13]和Hou等[14]采用时-空守恒元与求解元法，模拟了水下爆轰燃气泡的发展过程及冲击波的传播过程，并分析了气泡内部与管口处冲击波传播、反射及回传等复杂波系的演变。目前，对水下爆轰燃气射流领域的研究较少，现有研究中均未考虑喷管结构对水下爆轰燃气射流特性的影响，针对喷管对爆轰推进性能与燃气射流影响的研究也主要集中在常规大气环境中[15-17]，未见有关水下爆轰的报道。
近年来，针对喷管对水下高速燃气射流的影响，已开展了大量的实验和数值模拟研究，但这些研究主要集中在水下固体火箭发动机领域。水下固体火箭发动机在工作过程中同样会产生高温高压的超音速燃气射流，并与喷管以及水流场相互作用形成含有冲击波、相变、旋涡等复杂的流动现象。这与水下爆轰燃气射流的初期过程是相似的，同时对分析喷管在水下爆轰燃气射流的影响也具有极大的指导意义。针对水下固体火箭发动机领域，汤龙生等[18]研究了水下超声速燃气射流的气泡生长及演变过程，以及气泡压力波在水中的传播特性，并研究了压力波在水介质中的衰减规律。Linck等[19]针对多种结构喷管进行燃气射流实验研究，将射流划分为气泡流态和射流态，并指出燃烧室压力脉动和射流脉动存在耦合现象。唐嘉宁等[20]和Tang等[21]采用计算流体动力学（computational fluid dynamics, CFD）方法对不同喷管扩张比情况下的推力变化进行了数值模拟，发现水下推进总推力不仅与喷管几何形状有关，还与压力分布有关。王利利等[22]基于滤波器的湍流修正模型，对具有不同扩张比喷管的固体火箭发动机水下燃气射流进行数值模拟，揭示了不同喷管下气泡形态、发动机推力、水中压力波传播的变化。曹嘉怡等[23]和Gong等[24]采用Fluent软件，研究了喷管扩张比、水深和有无来流等对固体火箭发动机射流流场特性的影响。虽然对水下爆轰燃气射流的特性和喷管对水下高速燃气射流的影响已开展了大量的实验和数值模拟研究，详细阐述了水下燃气泡、压力波的产生及喷管的作用规律，但关于喷管对水下爆轰燃气射流影响的分析还有所欠缺。
综上所述，在先前研究的基础上，同时为了避免爆轰管多循环工作状态下，上一个循环的燃气射流对下一循环状态产生的不利影响，在实验室水箱中进行爆轰管水下单次爆轰实验，并用高速摄影机对爆轰燃气气泡脉动过程进行拍摄，探索喷管类型对单次爆轰水下燃气射流气泡形态和压力特征的影响。
1. 实验系统
图1为实验室内搭建的单次爆轰水下燃气射流实验装置示意图。实验装置由爆轰管、水箱、喷管、气体填充系统、高速摄影系统、压力监测系统、数据采集系统和点火系统等组成。
实验室水箱长1.2 m，宽1 m，高1 m，水箱正面与两侧预留有观测窗。爆轰管长度为1.7 m，管内壁直径为30 mm。实验过程中，爆轰管竖直插入水箱中，管口距水面300 mm。图2为安装在爆轰管尾部的各型喷管，喷管构型分别为收缩角为20°的收敛喷管、直喷管以及扩张角为60°的扩张喷管，各喷管长度均为30 mm。在距爆轰管管口500和550 mm处装有2个PCB113B24型动态压力传感器，以确认爆轰管内是否形成稳定爆轰；为测量水中压力波，在距离喷管水平位置600 mm处，设置1个PCBW138A01型水下爆炸传感器；3个传感器均以1 MHz的采样率记录2 s。高速摄影机型号为i-Speed 7，实验过程中高速摄影机的帧率为5 kHz，分辨率为512×512，曝光时间为150 μs。
实验过程中，首先用真空泵将爆轰管内的空气抽出，再通过配气盘充入等当量比的氧气/甲烷混合物，总填充压力为0.3 MPa。气体填充过程中，通过爆轰管头部的压力传感器实时检测爆轰管内的填充压力，当填充压力达到预设值时，反馈信号触发控制阀门停止填充。随后使用高能点火器触发点火头引燃爆轰管内的可燃混合气，通过管内扰流片等装置加速燃烧转爆轰过程，使爆轰管内形成稳定的爆轰波传向管口。为便于后续实验数据的分析，实验过程中通过数字信号发生器发射脉冲信号控制高速摄影机、高能点火器与压力传感器同时工作。
2. 射流形态
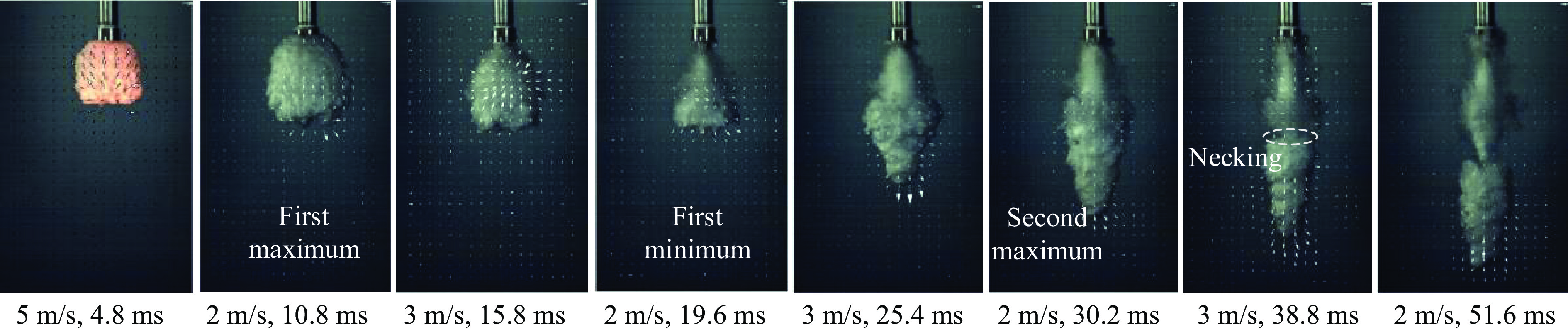
数字粒子图像测速（digital particle image velocimetry, DPIV）技术是一种常用于流动可视化的非接触式测量流场速度的方法。在确定连续2张图片的时间间隔和图像比例尺后，采用DPIV技术可直接对比分析2张相同大小图像的像素点得到图像速度场[25]。采用DPIV技术对气泡脉动图片进行流场分析，得到各喷管不同时间点的气泡速度场，如图3～4所示。图3(a)为直喷管气泡形态发展图，图3(b)为扩张喷管气泡形态发展图，图4为收敛喷管气泡形态发展图。图3～4中的白色箭头方向和大小分别表示气泡运动方向和气泡边界运动速度大小。为了便于对比分析，定义爆轰波到达管口处的时间点为初始时刻。
图 3 直喷管和扩张喷管水下爆轰燃气射流气泡形态及速度场Figure 3. Bubble shapes and velocity fields of underwater detonation gas jets from detonation tubes with straight and divergent nozzles, respectively
图 4 收敛喷管水下爆轰燃气射流气泡形态和速度场Figure 4. Bubble shapes and velocity fields of the underwater detonation gas jet from the detonation tube with a convergent nozzle2.1 直喷管和扩张喷管的射流形态
如图3(a)所示，直喷管工况下的水下爆轰燃气射流气泡脉动过程大致可分为膨胀过程、收缩过程以及随后的再膨胀-收缩循环过程（脉动）。如图3(a)中4.4 ms时刻所示，爆轰燃气射流初期，大量高温高压爆轰燃气冲击进入水中，但因受到外界水的阻碍作用导致气体轴向速度降低，气泡径向增长速度明显大于轴向速度。随着管内燃气全部进入到气泡中，气泡逐渐克服水的阻碍作用并沿轴向迅速向下方扩张，同时气泡内压力逐渐与外部水介质压力平衡，但因惯性作用，气泡继续向周围减速扩张至最大体积，如图3(a)中14 ms时刻所示，此时气泡呈椭圆形。气泡一次收缩阶段，如图3(a)中25.4 ms至29.4 ms时刻所示，气泡内燃气速度差异较大，因为气泡表面张力与速度梯度，气泡尾部气体明显具有更高的收缩速度。尾部气体快速向中心处的收缩形成了颈缩现象，最终使气泡与喷管相互分离，同时气泡头部受到尾部收缩气体的冲击，气泡在收缩的同时保持向下移动。气泡的一次膨胀阶段与收缩阶段构成了气泡的一次脉动过程，气泡一次脉动持续时间为29.4 ms。气泡二次脉动阶段可概述为再膨胀-收缩过程，如图3(a)中37.2 ms至52.4 ms时刻所示，气泡二次脉动过程中，气泡内气体由尾部汇聚于头部，头部具有较高向下运动的速度，同时气泡内部燃气在惯性作用下保持向下运动趋势，气泡形状逐渐由椭圆形拉伸为不规则长条形。气泡二次脉动过程与气泡一次脉动过程相比，气泡膨胀速度与收缩速度均小于一次脉动阶段，这是因为气泡一次脉动过程中消耗了气泡内气体的大部分能量，导致气泡后续脉动强度较低。
如图3(b)中5.2 ms至32 ms时刻所示，扩张喷管工况下的气泡一次脉动阶段，爆轰管内的超声速燃气经扩张喷管进入水中，燃气随后沿喷管内壁迅速向外膨胀，气泡径向扩张速度较大，气泡整体呈圆锥状。超声速燃气经过扩张喷管后燃气速度进一步提高，气泡脉动过程中惯性作用更强，因此气泡膨胀与收缩持续时间也更长，气泡一次脉动持续时间达到32 ms。扩张喷管工况下的气泡二次脉动阶段，如图3(b)中35.4 ms至58.4 ms时刻所示，气泡一次收缩阶段的颈缩使得气泡在后续脉动过程中与喷管完全分离。同时因为扩张喷管内壁的影响，气泡内燃气并未集中在中心轴线处，气泡内燃气向四周分散膨胀，但随着气泡内燃气逐步向头部汇聚，气泡头部更为突出，气泡形态逐渐变为斜三角状。
对比分析扩张喷管和直喷管工况下的气泡脉动形态变化，发现扩张喷管和直喷管工况下的气泡脉动演化过程、典型现象基本一致，但这2种喷管工况下的气泡脉动时间和气泡形状存在些许差异。扩张喷管增强了气泡脉动过程，气泡膨胀体积更大，脉动时间更长。因为扩张喷管内壁的影响，气泡一次脉动阶段，扩张喷管工况下的气泡尾部面积更大，气泡整体呈现圆锥状，气泡二次脉动阶段，扩张喷管工况下的气泡内燃气向四周分散膨胀，并未集中在气泡头部中心轴线处，因此相比于直喷管，扩张喷管工况下的气泡形态未被明显拉伸。
2.2 收敛喷管的射流形态
由于收敛喷管的最小通道面积远小于直喷管和扩张喷管的最小通道面积，收敛喷管的燃气流量也小于直喷管和扩张喷管的燃气流量，而且爆轰波通过收敛喷管时会产生反射冲击波，超声速燃气射流通过收敛喷管时，喷管内也会产生斜激波，喷管与冲击波的组合作用进一步降低了收敛喷管爆轰燃气的喷射速率，因此收敛喷管对爆轰燃气射流气泡形态的影响明显不同于直喷管和扩张喷管。本节中将单独阐述收敛喷管对气泡脉动过程的影响。
收敛喷管工况下气泡一次脉动阶段，如图4中4.8 ms至19.6 ms时刻所示，由于收敛喷管的影响，气泡膨胀和收缩速度均较低，气泡脉动作用较弱，气泡一次脉动持续时间仅为19.60 ms。同时因为燃气射流的持续性，在气泡一次收缩阶段，气泡尾部并未发生明显颈缩现象，气泡与喷管也未发生明显的分离。收敛喷管工况下的气泡二次脉动阶段，如图4中25.4 ms至51.6 ms时刻所示，燃气从气泡的尾部持续射入气泡内，燃气射流主要集中在气泡内中心轴线处，气泡头部始终具有较高的向下移动速度，气泡径向变化速度较低，气泡长径比逐渐变大，气泡被剧烈拉伸，整体呈长条状。在二次收缩阶段，喷管口燃气射流与下方初始气泡的燃气通道会形成一个低压区，水的回流引起颈缩现象，后续气泡与初始气泡被切割开，并最终形成2个气泡。
3. 气泡脉动压力场特征
3.1 爆轰管管内压力特征
通过安装在爆轰管尾部的2个动态压力传感器分析爆轰管管内压力特征，判断爆轰管管内是否形成稳定爆轰。图5为爆轰管尾部两监测点压力随时间的变化。爆轰波传播至爆轰管尾部时，先后经过安装在尾部的2个动态压力传感器，时间间隔约为0.02 ms，2个动态压力传感器相距50 mm，由此计算出爆轰波实际传播速度约为2500 m/s。这与采用NASA CEA[26]程序计算出的爆轰波Chapman-Jouguet (C-J)理论传播速度（2441 m/s）比较接近，说明管内已形成稳定爆轰波。当爆轰波传播至管口进入水气交界面时，因为管内气体与外部介质水的阻抗差异，爆轰波在水气交界面发生反射与透射，爆轰波分为传入水中的透射冲击波和回传至管内的反射冲击波。反射冲击波从水气交界面回传至爆轰管管内引起管内压力升高（如图5中蓝色虚框所示），透射冲击波会透射进入水中传播，随后被水下爆炸传感器捕捉。
图 5 爆轰管尾部监测点压力随时间的变化Figure 5. Pressure-time curves of monitoring points at the tail of the detonation tube3.2 水中压力特征
水下爆轰燃气射流压力场主要可分为传入水中的透射冲击波与气泡脉动引起的压力波。为了区分水中压力波系，同时分析喷管类型对水中压力特征的影响，对水下爆炸传感器采集的压力信号进行小波变换，得到各喷管功率谱密度，如图6所示。
对比分析3种喷管频谱特性，扩张喷管工况下的功率明显更高，这是因为爆轰燃气经扩张喷管后，燃气射流速度加快，气泡内燃气动能更高。在10～200 Hz频率段，各喷管对应的频谱中存在多个峰值，峰值连续且呈现递减趋势。34、36和55 Hz分别为加装扩张喷管、直喷管和收敛喷管的压力变化频谱中第1个峰值频率，这与各喷管工况下水下气泡脉动图像对应的气泡一次脉动周期基本一致。因此，此区域为气泡脉动压力波信号的频谱区域，气泡脉动产生的压力波可确定为低频压力波（频率低于200 Hz）。同样在频率1336 Hz附近，各喷管对应的频谱特征具有一个明显的阶跃峰值，结合水下爆炸传感器测得的水中压力数据与Liu等[11]的研究成果，确定该高频区域为透射冲击波频谱区域。水中压力信号的频谱分析表明，扩张喷管的透射冲击波压力更高，气泡脉动过程能量也更大，收敛喷管对透射冲击波与气泡脉动过程具有明显抑制作用。
3.3 气泡脉动压力特征
根据3.2节的分析结果，透射冲击波为高频压力波（频率高于1000 Hz），气泡脉动产生的压力波为低频压力波。采用1000 Hz的低通滤波器对水下爆炸传感器测得压力数据进行低通滤波处理，得到各喷管水下气泡脉动压力图。
3.3.1 直喷管和扩张喷管气泡脉动压力特征
图7为直喷管与扩张喷管工况下的气泡脉动压力图，图中A点为爆轰燃气射流冲击进入水中时引起的压力扰动，B、C两点为气泡脉动过程中，气泡收缩到最小后开始膨胀时产生的压力波。将图7中各压力极值点与气泡脉动时间汇总，结果见表1。表1中pA、pB和pC分别为图中A、B和C等3点对应的压力极值，t1为气泡一次脉动时长，对应图中A点到B点的时间差，t2为气泡二次脉动时长，对应图中B点到C点的时间差。
图 7 不同喷管气泡脉动压力随时间的变化Figure 7. Bubble pulsation pressure-time curves of different nozzles表 1 直喷管和扩张喷管的气泡脉动压力极值和脉动时间Table 1. Pressure extrema and bubble pulsation time of straight and divergent nozzles喷管 pA/kPa pB/kPa pC/kPa t1/ms t2/ms 直喷管 23.50 12.38 2.54 28.70 20.63 扩张喷管 31.41 13.41 7.52 31.60 21.48 从表1可知，直喷管工况下的气泡一次脉动和气泡二次脉动产生的压力极值分别为12.38 和2.54 kPa，气泡二次脉动的压力极值仅为气泡一次脉动的20.51%，气泡二次脉动强度远低于气泡一次脉动强度。结合2.1节分析认为，直喷管工况下的气泡二次脉动阶段，气泡内燃气集中在头部与中心轴线处，气泡快速向下运动的过程消耗了气泡内大部分能量，导致气泡的后续脉动强度远低于一次脉动阶段。相比于直喷管，超声速爆轰燃气经过扩张喷管后，燃气速度进一步提高，动能增加，因此爆轰燃气进入水中时引起的压力扰动也更大，达到31.41 kPa，这比直喷管工况下的压力扰动大了33.67%。同时因为气泡内燃气速度的提高，扩张喷管工况下的气泡脉动强度也更大，2次气泡脉动产生的压力极值分别达到13.41 和7.52 kPa，扩张喷管工况下二次脉动的压力极值为气泡一次脉动的56.08%，气泡脉动强度衰减明显更低。对比加装不同喷管时气泡脉动时长，扩张喷管工况下的气泡脉动时长更长，其两次气泡脉动时长比直喷管工况下的两次气泡脉动时长分别多10.10%和4.13%。在水下爆轰过程中，扩张喷管工况下的气泡脉动时间与脉动压力都更大，扩张喷管能够提高气泡脉动能力，气泡脉动强度更高，这与高速摄影得出的气泡脉动规律一致。
3.3.2 收敛喷管气泡脉动压力特征
图8为加装收敛喷管时气泡脉动压力图。收敛喷管与管内冲击波的组合作用降低了燃气的喷射速率，水中气泡内的燃气速度也较低，这导致收敛喷管燃气射流冲击进入水中时引起的压力扰动与后续气泡脉动压力极值较小，收敛喷管工况下的初始压力扰动、气泡一次脉动压力极值和二次脉动压力极值仅为9.54、2.68和1.77 kPa。但同样因为收敛喷管燃气射流的持续性，在收敛喷管工况下的气泡二次脉动阶段，爆轰管管内燃气从尾部注入气泡内，减缓了气泡脉动强度的衰减。收敛喷管工况下的气泡二次脉动压力极值相较于一次脉动压力极值，仅减小了34%，并且第二次气泡脉动结束以后，还存在明显的气泡脉动压力变化。收敛喷管工况下的气泡一次脉动时长和二次脉动时长分别为19.12和18.15 ms，虽然2次脉动时间的衰减仅为5.1%，但整体气泡脉动持续时间较短。综合分析，收敛喷管虽然因为射流持续性导致气泡脉动时长和脉动压力都较小，但脉动强度和脉动时长衰减也更小，持续性加强。收敛喷管结构能够降低气泡脉动强度，并抑制气泡脉动衰减。
图 8 收敛喷管气泡脉动压力随时间的变化Figure 8. Bubble pulsating pressure-time curve of the convergent nozzle4. 结 论
搭建了水下爆轰燃气射流实验平台，对比分析了安装直喷管、收敛喷管和扩张喷管时水下爆轰燃气射流气泡形态以及压力特征，得到如下结论。
(1)扩张喷管与直喷管工况下的气泡脉动典型过程基本相似，但扩张喷管工况下的气泡增长速度更快，气泡膨胀体积也更大，气泡脉动时间也更长。扩张喷管提高了气泡脉动强度，在水下爆轰过程中，扩张喷管工况下的气泡脉动时间与脉动压力都大于直喷管。扩张喷管产生的透射冲击波频率与直喷管对应的透射冲击波频率基本相同，但扩张喷管工况下的透射冲击波能量更高。
(2)收敛喷管对气泡形态影响较大，水下爆轰燃气射流持续时间较长。收敛喷管工况下2次气泡脉动时间相差较小，第2次气泡脉动结束以后，还存在明显的气泡脉动压力变化，但是整体气泡脉动的周期时长都较短。收敛喷管降低了爆轰燃气的射流速度，阻碍了气体射流过程，虽然收敛喷管工况下的气泡脉动频率增大，但气泡脉动强度更低。收敛喷管对气泡脉动和透射冲击波均具有明显的抑制作用。
爆轰管多循环工作状态下，上一个循环的燃气泡会对下一个循环的气泡脉动和冲击波传播产生影响。收敛喷管和扩张喷管不仅会影响燃气泡的发展和冲击波的传播，还会对下一个循环的燃料填充过程产生影响，进而降低爆轰管的工作频率。因此，下一阶段将重点探讨喷管对多循环爆轰水下燃气射流的影响。
-
图 1 直墙拱模型尺寸及钢筋布置(单位: mm)
Figure 1. Size of structure and layout of steel bars (unit: mm)
图 8 数值模拟与试验的结构破坏形态对比
Figure 8. Comparison of structural failure modes in numerical simulation and test
图 10 数值模拟与试验的测点压力、速度时程曲线对比
Figure 10. Comparison of pressure and velocity time-history curves at measuring points between simulation and test
图 12 二次打击工况结构的初始损伤特征
Figure 12. Initial damage characteristics of structures under secondary explosion
表 1 试验工况设置
Table 1. Setting of test conditions
工况 爆炸距离/m 装药当量/kg 比例爆距/（m∙kg−1/3） T1-0 1.0 5.0 0.585 T1-1 0.8 5.0 0.468 T2-0 0.8 5.0 0.468 T2-1 0.8 7.5 0.409 T3-0 0.8 7.5 0.409 T3-1 0.8 7.5 0.409 
下载: 导出CSV
密度/
（kg∙m−3）杨氏模量/
GPa剪切模量/
GPa抗压强度/
MPa最小残余
损伤应变2440 32.5 16.7 40 0.01
下载: 导出CSV
表 3 TNT材料关键参数
Table 3. Key parameters of TNT material
密度/( kg∙m−3) 爆速/(m∙s−1) pCJ/GPa E0/GPa R1 R2 ω A/GPa B/GPa 1600 6300 28.5 7 4.15 0.95 0.3 3730 3.75
下载: 导出CSV
表 4 数值模拟初次打击局部震塌计算
Table 4. Numerical simulation of local collapse in initial shock
试验 工况
(距离-当量)震塌系数KZ 毁伤描述 S1-0 1.0 m-5.0 kg 0.371 无明显震塌现象 S2-0 0.8 m-5.0 kg 0.319 小范围内混凝土脱落 S3-0 1.0 m-7.5 kg 0.330 小范围内混凝土脱落 S4-0 0.8 m-7.5 kg 0.284 较大范围的混凝土层裂
下载: 导出CSV
表 5 初次打击数值模拟计算结果
Table 5. Numerical simulation results of initial explosion
试验 工况
(距离-当量)自振周期/ms 刚度比 挠跨比/% 毁伤程度 S1-0 1.0 m-5.0 kg 6.03 0.990 0.395 轻度毁伤 S2-0 0.8 m-5.0 kg 7.00 0.735 0.772 中度毁伤 S3-0 1.0 m-7.5 kg 6.95 0.745 0.797 中度毁伤 S4-0 0.8 m-7.5 kg 7.24 0.687 1.729 中度毁伤
下载: 导出CSV
表 6 二次打击数值模拟计算
Table 6. Numerical simulation of secondary explosion
试验 工况(距离-当量) 拱顶挠度/mm 挠跨比/% 累积挠度/mm 累积挠跨比/% 毁伤等级 S1-1 1.0 m-7.5 kg 7.94 0.827 11.735 1.222 中度毁伤 S1-2 0.8 m-5.0 kg 7.90 0.823 11.695 1.218 中度毁伤 S1-3 1.0 m-5.0 kg 4.70 0.490 8.495 0.885 中度毁伤 S2-1 1.0 m-5.0 kg 5.06 0.527 12.470 1.299 中度毁伤 S2-2 0.8 m-7.5 kg 17.20 1.792 24.610 2.564 重度毁伤 S2-3 0.8 m-5.0 kg 8.53 0.870 15.760 1.642 中度毁伤 S3-1 1.0 m-5.0 kg 4.97 0.518 12.620 1.315 中度毁伤 S3-2 0.8 m-7.5 kg 17.20 1.792 24.850 2.589 重度毁伤 S3-3 1.0 m-7.5 kg 8.09 0.843 15.740 1.640 中度毁伤 S4-1 0.8 m-5.0 kg 22.50 2.344 39.100 4.073 重度毁伤 S4-2 1.0 m-7.5 kg 14.30 1.490 30.900 3.219 重度毁伤 S4-3 0.8 m-7.5 kg — — — — 重度毁伤
下载: 导出CSV
表 7 相同工况下不同初始毁伤结构响应对比
Table 7. Responses of different initial damaged structures under the same conditions
试验 工况
(距离-当量)初始刚度比 初始毁伤程度 挠跨比/% S2-0 0.8 m-5.0 kg 1.000 无毁伤 0.772 S1-2 0.8 m-5.0 kg 0.990 轻度毁伤 0.823 S2-3 0.8 m-5.0 kg 0.735 中度毁伤 0.870 S4-1 0.8 m-5.0 kg 0.687 中度毁伤 2.344
下载: 导出CSV
表 8 不同起爆次序下结构响应对比
Table 8. Structural response under different initiation sequence
试验 工况顺序(距离-当量) 累积挠跨比/% S2-2 先0.8 m-5.0 kg，后0.8 m-7.5 kg 2.564 S4-1 先0.8 m-7.5 kg，后0.8 m-5.0 kg 4.073
下载: 导出CSV
-
[1] 王辉明, 刘飞, 晏麓晖, 等. 接触爆炸荷载对钢筋混凝土梁的局部毁伤效应 [J]. 爆炸与冲击, 2020, 40(12): 121404. DOI: 10.11883/bzycj-2020-0171.WANG H M, LIU F, YAN L H, et al. Local damage effects of reinforced concrete beams under contact explosions [J]. Explosion and Shock Waves, 2020, 40(12): 121404. DOI: 10.11883/bzycj-2020-0171. [2] SHI Y C, HONG H, LI Z X. Numerical derivation of pressure–impulse diagrams for prediction of RC column damage to blast loads [J]. International Journal of Impact Engineering, 2008, 35(11): 1213–1227. DOI: 10.1016/j.ijimpeng.2007.09.001. [3] YAO S J, ZHANG D, CHEN X G, et al. Experimental and numerical study on the dynamic response of RC slabs under blast loading [J]. Engineering Failure Analysis, 2016, 66: 120–129. DOI: 10.1016/j.engfailanal.2016.04.027. [4] 汪维, 张舵, 卢芳云, 等. 钢筋混凝土楼板在爆炸荷载作用下破坏模式和抗爆性能分析 [J]. 兵工学报, 2010, 31(S1): 102–106.WANG W, ZHANG D, LU F Y, et al. Analysis for blast resistance and damage mode of reinforced concrete slab subjected to explosive load [J]. Acta Armamentarii, 2010, 31(S1): 102–106. [5] KIGER S A, DALLRIVA F D, HALL R L. Dynamic skin-friction effects on buried arches [J]. Journal of Structural Engineering, 1989, 115(7): 1768–1781. DOI: 10.1061/(ASCE)0733-9445(1989)115:7(1768). [6] 孙惠香, 许金余, 李庆. 爆炸荷载作用下地下结构破坏模式研究 [J]. 弹箭与制导学报, 2011, 31(5): 89–92, 98.SUN H X, XU J Y, LI Q. The failure mode study of underground structure subjected to blast load [J]. Journal of Projectiles, Rockets, Missiles and Guidance, 2011, 31(5): 89–92, 98. [7] 李秀地, 郑颖人, 徐干成. 爆炸荷载作用下地下结构的震塌破坏模型研究 [J]. 爆破, 2006, 23(1): 6–9. DOI: 10.3963/j.issn.1001-487X.2006.01.002.LI X D, ZHENG Y R, XU G C. Spall model of underground structures under blast loads [J]. Blasting, 2006, 23(1): 6–9. DOI: 10.3963/j.issn.1001-487X.2006.01.002. [8] 李秀地, 郑颖人, 徐干成. 爆炸荷载作用下地下结构的局部层裂分析 [J]. 地下空间与工程学报, 2005, 1(6): 853–855,877. DOI: 10.3969/j.issn.1673-0836.2005.06.010.LI X D, ZHENG Y R, XU G C. Spall response analysis of underground structures under blast loads [J]. Chinese Journal of Underground Space and Engineering, 2005, 1(6): 853–855,877. DOI: 10.3969/j.issn.1673-0836.2005.06.010. [9] 邓春梅, 许金余, 沈刘军. 装药爆炸下地下拱形结构变形及破坏特征分析 [J]. 解放军理工大学学报(自然科学版), 2007(5): 534–537. DOI: 10.7666/j.issn.1009-3443.20070522.DENG C M, XU J Y, SHEN L J. Deformation and damage characteristics analysis of underground arch structure subjected to subsurface blast [J]. Journal of PLA University of Science and Technology (Natural Science Edition), 2007(5): 534–537. DOI: 10.7666/j.issn.1009-3443.20070522. [10] 霍庆, 王逸平, 刘光昆, 等. 地下拱形结构侧顶爆炸的破坏模式及影响因素 [J]. 兵工学报, 2021, 42(S1): 105–116.HUO Q, WANG Y P, LIU G K, et al. Failure mode and influencing factors of underground arched structure subjected to side top blast [J]. Acta Armamentarii, 2021, 42(S1): 105–116. [11] 邓国强. 重复打击下防护结构地冲击初步分析[C]//第26届全国结构工程学术会议论文集(第Ⅲ册). 2017: 38−42. [12] 戎志丹, 孙伟, 张云升, 等. 超高性能钢纤维混凝土抗二次接触爆炸性能研究 [J]. 华北水利水电学院学报, 2012, 33(6): 1–4. DOI: 10.19760/j.ncwu.zk.2012.06.001.RONG Z D, SUN W, ZHANG Y S, et al. Study on the characteristics of ultra-high performance steel fiber reinforced concrete under the second explosion [J]. Journal of North China Institute of Water Conservancy and Hydroelectric Power, 2012, 33(6): 1–4. DOI: 10.19760/j.ncwu.zk.2012.06.001. [13] 马林建, 赵岩, 张晓, 等. 二次爆炸荷载作用下钢筋混凝土梁动力响应分析 [J]. 工业建筑, 2011, 41(S1): 145–148. DOI: 10.13204/j.gyjz2011.s1.179.MA L J, ZHAO Y, ZHANG X, et al. Dynamic response analysis of reinforced concrete beams subjected to secondary impulsive loading [J]. Industrial Construction, 2011, 41(S1): 145–148. DOI: 10.13204/j.gyjz2011.s1.179. [14] 马淑娜, 刘新宇, 马林建, 等. 常规武器在土中二次爆炸后对钢筋混凝土梁的动力响应分析[C]//.第2届全国工程安全与防护学术会议论文集. 2010: 401−405. [15] 杨大兴, 马林建, 马淑娜, 等. 常规武器对钢筋混凝土梁二次爆炸效应分析 [J]. 防护工程, 2012(6): 38–41.YANG D X, MA L J, MA S N, et al. An analysis of the damage effects of a second conventional weapon explosion on reinforced concrete beams [J]. Protective Engineering, 2012(6): 38–41. [16] 唐廷, 周健南. 地震后地下受损拱结构的抗爆炸能力研究 [J]. 兵工学报, 2017, 38(9): 1736–1744. DOI: 10.3969/j.issn.1000-1093.2017.09.010.TANG T, ZHOU J N. Study of anti-blasting ability of damaged underground arch structure after earthquake [J]. Acta Armamentarii, 2017, 38(9): 1736–1744. DOI: 10.3969/j.issn.1000-1093.2017.09.010. [17] WANG J. Simulation of landmine explosion using ls-dyna3d software: benchmark work of simulation of explosion in soil and air [R]. Fishermans Bend, Victoria, Australia: DSTO Aeronautical and Maritime Research Laboratory, 2001. [18] YANG G D, WANG G H, LU W B, et al. A SPH-lagrangian-eulerian approach for the simulation of concrete gravity dams under combined effects of penetration and explosion [J]. KSCE Journal of Civil Engineering, 2018(22): 3085–3101. DOI: 10.1007/s12205-017-0610-1. [19] ZHANG Y D, FANG Q, LIU O, et al. Numerical and experimental investigation into plane charge explosion technique [J]. International Journal of Impact Engineering, 2008, 35(10): 1179–1185. DOI: 10.1016/j.ijimpeng.2008.01.009. [20] 孙善政, 卢浩, 李杰, 等. 侵爆作用下混凝土靶破坏效应试验与数值模拟 [J]. 振动与冲击, 2022, 41(1): 206–212. DOI: 10.13465/j.cnki.jvs.2022.01.026.SUN S Z, LU H, LI J, et al. Test and numerical simulation for damage effect of concrete target under penetration and explosion [J]. Journal of Vibration and Shock, 2022, 41(1): 206–212. DOI: 10.13465/j.cnki.jvs.2022.01.026. [21] 马维. 地下管道结构爆振效应和冲击破坏行为实验 [J]. 解放军理工大学学报(自然科学版), 2008, 9(1): 39–46. DOI: 10.7666/j.issn.1009-3443.20080109.MA W. Experimental investigations on effects of blast vibration and behaviors of impacting failure of underground pipeline structures [J]. Journal of PLA University of Science and Technology (Natural Science Edition), 2008, 9(1): 39–46. DOI: 10.7666/j.issn.1009-3443.20080109. [22] 钱七虎. 防护结构计算原理[M]. 南京: 中国人民解放军工程兵工程学院, 1981: 73−77. 期刊类型引用(1)
1. 郭维东，张潇文，王冰欣. 矩形孔口射流条件下承泄水体紊动规律研究. 中国水利水电科学研究院学报(中英文). 2025(01): 19-30 . 
百度学术其他类型引用(2)
-
下载:
百度学术
计量
- 文章访问数: 591
- HTML全文浏览量: 131
- PDF下载量: 208
- 被引次数: 3