Research on penetration depth of projectiles into ultra-high performance concrete targets
-
摘要: 为了评估超高性能混凝土(UHPC)的抗侵彻性能,对UHPC靶板进行了侵彻试验与数值模拟。首先,利用
∅ 35 mm火炮对抗压强度为160 MPa的UHPC靶板开展了216~345 m/s速度下的弹体侵彻试验,结果表明:随着弹体速度的增加,侵彻深度与开坑直径皆有明显增加。随后在数值模拟过程中,确立了UHPC的RHT材料模型参数,为了验证材料模型的有效性,采用单轴压缩与霍普金森压杆试验结果对三维有限元模型进行了验证,模拟结果与实验结果吻合良好,表明参数选取科学合理。最后,对弹体侵彻UHPC的过程进行数值模拟,参数化分析了UHPC抗压强度、弹体质量、侵彻速度、弹径、弹头形状对UHPC侵彻深度的影响,并据此推导出弹体对UHPC侵彻深度计算公式。Abstract: Aiming to evaluate the penetration resistance of the ultra high performance concrete (UHPC) target, both penetration tests and numerical simulations were carried out on UHPC targets. Firstly, the∅ 35 mm gun was used to carry out a series of penetration tests on the C160 UHPC with striking velocities varying from 216 m/s to 345 m/s. The test results show that with the increase of projectile velocity, the penetration depth and crater diameter increase obviously. Besides, UHPC notably decreased the damage to targets caused by the projectile, efficiently reduced the penetration depth and regarding crater damage and crack propagation, which was superior to ordinary concrete in the performance against penetration. Then, 3D finite element models were established and the corresponding numerical simulations were carried out. In the process of numerical simulation, the key parameters of the RHT model for UHPC was determined. In order to verify the accuracy of the RHT material model, uniaxial compressive and split Hopkinson pressure bar (SHPB) testing results are used to validate 3D finite element material model. The numerical simulated results exhibited fair agreement with the test data, these observations demonstrated the applicability and validity of the calibrated RHT model. Finally, with the validated RHT material model, parametric studies were further conducted to explore the effect of uniaxial compressive strength of UHPC, projectile mass, projectile striking velocity, projectile diameter and projectile caliber-radius-head ratio on the final depth of penetration values of UHPC targets. Moreover, an empirical formula to predict the depth of penetration is derived according to the numerical simulated data, which can provide a reference for the design and evaluation of the UHPC protective structures against projectile penetrations.-
Key words:
- penetration /
- UHPC /
- CRH /
- RHT model
-
提升建筑结构自身抗力的途径除了寻求先进的结构体系外,最重要的就是开发新型防护材料[1]。因此关于新型建筑材料的抗冲击荷载的研究已引起广泛关注。
超高性能混凝土(ultra high performance concrete, UHPC)是一种广受关注的新型建筑材料,为了获得高强度和高韧性,UHPC在制备时需要提高胶凝材料使用量,并辅助以高效减水剂、钢纤维等[2]。与传统混凝土相比,UHPC具有抗压抗拉强度高、断裂韧性大、耐久性能优异等特点[3-4],这些特点使其成为结构抗冲击设计中的理想材料。
对于普通混凝土的抗侵彻性能研究,已有大量的研究成果,但对于UHPC抗侵彻方面的研究开展的相对较少[5-7],张文华等[8]使用质量340 g的高强度钢试验弹对C40普通混凝土和C180 UHPC开展了侵彻试验,结果表明:相对于普通混凝土,UHPC可以显著减小弹体对靶体的损伤,有效降低侵彻深度和弹坑直径。赖建中等[9]制备了抗压强度为200 MPa的UHPC靶板,并进行了侵彻试验,发现钢纤维与玄武岩纤维混杂的混凝土在经过两次高速弹丸侵彻后仍能保持完整,抗侵彻性能优越。Zhai等[10]研究了装甲钢/陶瓷/UHPC复合靶板的抗侵彻性能,并对复合靶板的冲击抗力和弹道效率因子进行了定量评价,发现装甲钢/陶瓷/UHPC复合靶板具有优异的抗侵彻性能,可以显著降低弹丸的侵彻深度。Zhang等[11]通过实验研究了靶板抗压强度、弹性模量、有效硬度指数、密度、劈裂抗拉强度和弯曲韧性等参数对混凝土材料侵彻深度影响,结果表明单靠靶板材料抗压强度并不能准确的表征侵彻深度,材料的有效硬度指数与弹性模量也是影响侵彻深度的重要因素。Wu等[12]对UHPC靶标进行了速度为510~1320 m/s的高速弹丸侵彻实验,研究表明:抗压强度、纤维掺混率以及粗集料均有助于降低弹体的侵彻深度;当强度大于90 MPa时,弹体侵彻深度不再明显降低。
由于进行UHPC原型侵彻试验周期长,经济投入大,实验次数有限,因此通过数值模拟研究侵彻问题越来越受到青睐。Liu等对120和140 MPa的UHPC在550~800 m/s侵彻速度下的冲击响应进行了数值模拟,模拟结果与实验结果非常吻合[13],并采用*MAT CONCRETE DAMAGE REL3混凝土模型研究了90~190 MPa的UHPC圆柱靶板在300~1000 m/s的弹丸侵彻下的冲击响应,并分析了抗压强度、弹丸速度以及弹头形状对侵彻深度的影响[14]。
目前对于UHPC抗弹体冲击侵彻性能的研究依然相对有限,已有的研究大多只针对特定弹型或者特定强度的UHPC,研究结论的适用性有限。本文制备强度约160 MPa(C160)的UHPC,并使用
∅ 35 mm火炮对UHPC靶板进行216~345 m/s不同速度的侵彻试验;同时利用LS-DYNA有限元软件,采用经过验证的RHT材料本构模型,模拟弹体侵彻UHPC靶板过程,在此基础上,研究抗压强度、侵彻速度、弹头形状、弹体质量、弹径对侵彻深度的影响,并据此推导适用于UHPC侵彻深度预测的公式,以期为UHPC在防护工程中设计与应用提供理论支撑。1. UHPC抗侵彻试验
1.1 UHPC制备
本文UHPC材料的配合比如表1所示,主要由普通硅酸盐水泥(P.O.52.5)、石英砂、石英粉、硅灰、粉煤灰、钢纤维及减水剂配制而成,钢纤维的相关参数如表2所示。在搅拌UHPC时,采用先干后湿的搅拌工艺。首先将称重好的石英砂、石英粉以及钢纤维倒入到搅拌机中搅拌5 min以上;待其搅拌均匀后,再将称重好的水泥、粉煤灰及硅灰倒入搅拌机中继续搅拌5 min;最后,将水与减水剂称重后倒入搅拌机内搅拌约15 min,形成流动性良好的浆体。
表 1 UHPC配合比Table 1. Mixture design of UHPCkg 水泥 石英砂 石英粉 硅灰 粉煤灰 钢纤维 水 减水剂 666 1065 160 160 80 157 135 6.7 表 2 钢纤维参数Table 2. Parameters of steel fiber直径/mm 长度/mm 拉伸强度/MPa 弹性模量/GPa 密度/(kg·m−3) 0.2±0.02 13±1.3 2000±300 200 7800 将制备好的UHPC浆体倒入100 mm×100 mm×100 mm的方形模具中,先倒入一半高度的浆体,并将模具放置于振动台上振捣1 min,再将剩下一半浆体倒入,并振捣1 min,最后将试件表面抹平。将浇筑好的试件放入温度20 ℃、湿度95%的养护室进行养护,养护28天后将试件从模具中取出,对试件加载面的两端进行打磨,以保证试件两端面的平整度小于5‰。参照《活性粉末混凝土规范》(GB/T 31387-2015)与《混凝土物理力学性能试验方法标准》(GB/T 50081-2019)使用MTS815实验系统对UHPC试件进行准静态单轴压缩实验,加载速率为1.2 MPa/s,测得UHPC标准单轴抗压强度为160 MPa。
1.2 UHPC试验靶板制作
文献[15]认为,弹丸对混凝土、陶瓷等脆性靶体进行侵彻时,其结果对边界条件通常是非常敏感。混凝土靶体径向直径较小时,压缩波在靶体侧面边界发生反射卸载。在压缩波和反射拉伸波的双重作用下,侵彻阻力会有所下降。同时文献[16]显示,当采用5 mm厚的钢箍对混凝土靶进行约束时,侵彻速度低于800 m/s、靶板直径大于15倍弹径即可忽略边界效应对侵彻结果的影响。
UHPC靶板设置为圆柱形,为节约成本同时最大限度消除侧向边界效应的影响,靶板分两次浇筑,先制作0.9 m×0.9 m×0.6 m的方形UHPC靶板,并将其放入直径1.3 m、高0.6 m、厚0.005 m的钢套筒内,最后在靶板与钢套筒间浇筑普通混凝土,如图1所示。UHPC靶板的配合比及养护条件皆与1.1节中制作UHPC试件时相同。
1.3 试验装置
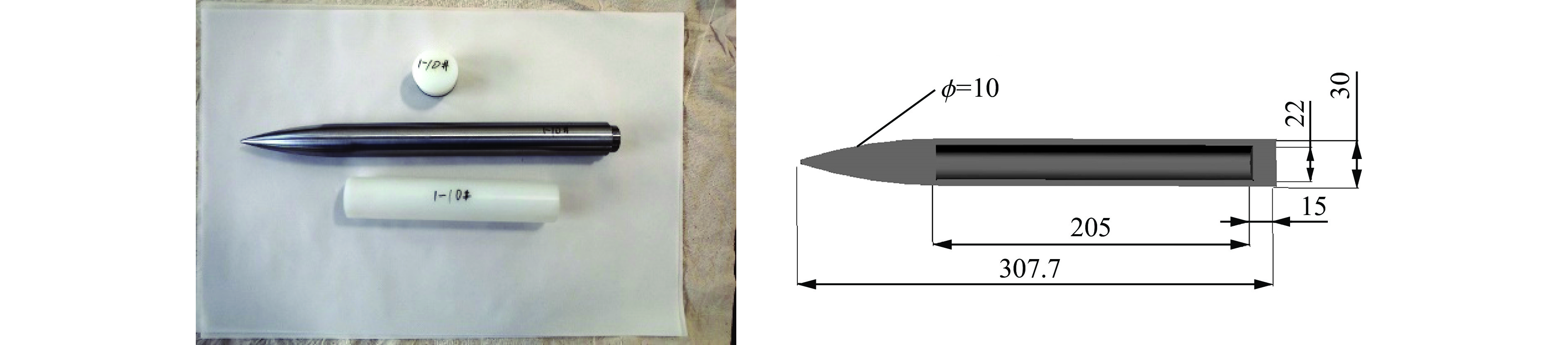
采用
∅ 35mm口径火炮作为试验发射设备,如图2所示。弹体材料为35CrMnSiA,屈服强度为1300 MPa。试验弹长约307.7 mm;质量约为1000 g;弹径30 mm;长径比为10∶1;弹头形状系数ϕ(弹体头部的曲径比)为10;弹体壁厚4 mm(见图3)。因弹体直径小于火炮内径,故采用次口径发射技术,加工尼龙弹托,并在发射前安装在试验弹上。试验靶板安装在炮口的正前方10 m处,底部设置若干沙袋,通过调整沙袋摆放位置确保每次试验靶板迎弹面始终与弹体行进方向垂直,背面固定在混凝土试验台上。试验时,通过调节发射药的药量,控制弹体速度在200~350 m/s之间。在试验靶板的正前方放置两个测速锡箔靶,通过同步时钟记录弹体通过两个测速锡箔靶的时间差,进而得到弹体的着靶速度。同时使用高速相机观察弹体侵彻过程。1.4 试验结果
弹体撞击到靶板后,使靶板表面的混凝土介质破碎。由弹体高速撞击引起靶板介质之间相互挤压以及靶板自由面效应使得靶板表面破碎的混凝土向后飞溅,这一飞溅现象可以清楚地从高速相机记录的照片观察到,如图4所示。共进行8炮次试验,其中速度约220 m/s的有3枚试验弹,速度约300 m/s的有4枚试验弹,速度在345 m/s的有1枚试验弹。侵彻试验数据如表3所示。
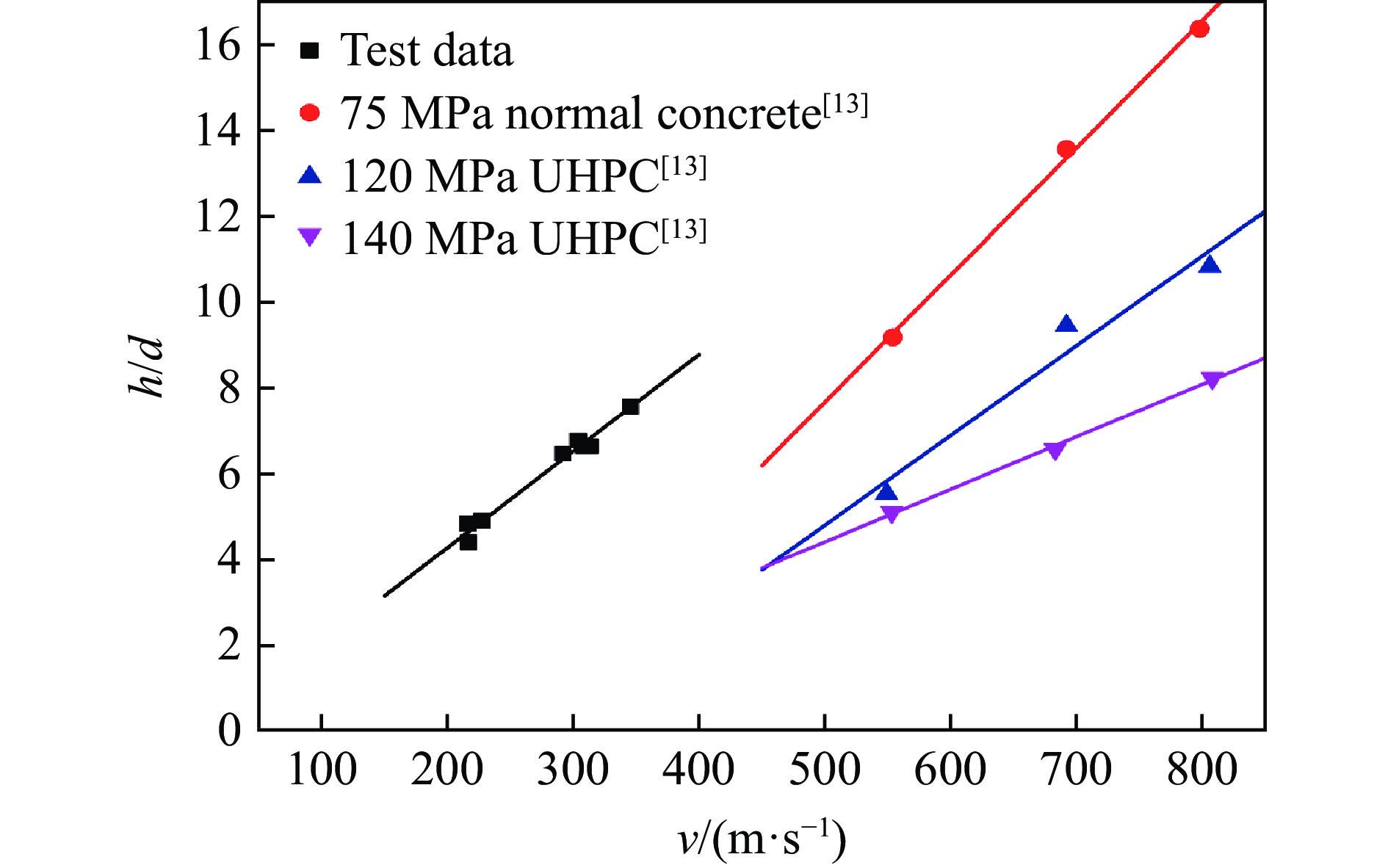
表 3 侵彻试验结果Table 3. Penetration tests data试验 M/kg v/(m·s−1) h/mm h/d dc/mm dc/d 1# 1.001 216.2 145 4.83 200 6.67 2# 1.004 216.7 132 4.40 155 5.17 3# 1.000 226.9 147 4.90 220 7.33 4# 0.999 292.0 194 6.47 250 8.33 5# 1.003 304.1 203 6.77 200 6.67 6# 1.001 308.7 199 6.63 300 10.00 7# 0.999 313.2 199 6.63 300 10.00 8# 1.003 345.4 227 7.57 320 10.67 注:M为弹体质量,v为着靶速度,h为侵彻深度,d为弹体直径,dc为开坑直径。 从试验结果可以看出,侵彻深度随着侵彻速度的增加而增大。钱七虎等[17]在对弹体侵彻岩石介质的研究中发现,当弹体速度在一定范围内(v<0.2c,其中c为靶板材料声速),侵彻深度与弹体速度具有线性关系。图5给出了本文试验及文献[13]得到的无量纲侵彻深度(h/d)随弹体速度的变化曲线,可以看出,对于UHPC靶,在一定速度范围内,侵彻速度与侵彻深度同样具有线性关系。同时,结合文献[13]中3种混凝土的无量纲侵彻深度随速度的变化曲线可以看出,相同侵彻条件下UHPC的侵彻深度远小于普通混凝土。
图6为试验前后弹体变形情况的对比,可以看出,在整个侵彻过程中,弹体未发生明显变形与磨蚀。图7、图8分别给出了本文及文献[13]中试验后靶板破坏情况,可以看出,UHPC靶板中的弹坑形状更接近于规则的圆形,而普通混凝土靶板中的弹坑形状不规则,且裂纹的数量也远多于UHPC。这主要是由于普通混凝土中存在较多的初始孔隙与裂纹,在面对弹体撞击靶板时,靶体材料发生强烈压缩和剪切变形,同时,撞击产生冲击波在靶板表面反射,引起靶板表混凝土沿薄弱面发生破坏并脱离靶体,造成弹坑形状不规则。而UHPC拥有更低的水胶比,且添加了粒径更小的石英粉、硅灰等,可以更好地填充材料内部的孔隙,减小靶体的初始缺陷与损伤,在面对弹体侵彻时,破坏更均匀,弹坑形状也更加规则。
试验后所有的UHPC靶板弹坑周围皆产生一定高度的凸起,部分破坏的UHPC基体附着在弹坑周围(如图7(b)),这是由于UHPC基体在强烈的冲击荷载下发生断裂与破碎,但由于UHPC中钢纤维与基体间仍存在着粘结力,阻止了破碎UHPC基体的飞溅,使大量破碎的UHPC基体附着在弹坑周围。从图8也可以看出,相同侵彻条件下,UHPC的弹坑直径也远小于普通混凝土,说明UHPC能有效地抑制靶体破坏,防止靶体材料的崩飞,维持靶体的完整性。
2. 弹体侵彻UHPC数值模拟
2.1 材料模型
在数值模拟中,弹体材料模型采用LS-DYNA中的*MAT_RIGID模型,UHPC靶板材料模型选用LS-DYNA有限元软件材料库第272号材料*MAT_RHT模型。RHT模型近年来在弹体侵彻混凝土靶板的数值计算中应用越来越广泛,由强度模型与状态方程两部分组成,其中强度模型包括5个部分,分别为失效面、弹性极限面、应变硬化、残余强度面与损伤。模型综合考虑了混凝土材料的应变率效应、孔隙压实、应变硬化、损伤等因素,可以较好地反应UHPC在冲击荷载下的响应[18]。
RHT模型部分参数通过准静态力学试验确定,密度ρ=2450 kg/m3,抗压强度fc=160 MPa,弹性模量E=44 GPa,泊松比为υ=0.19,剪切模量G=18.5 GPa,拉压强度比
f∗t=0.0613 ,剪压强度比f∗s 取值参考了文献[19]中152 MPa UHPC三轴压缩试验的结果,计算得f∗s=0.267 。按照文献[20]关于冲击绝热方程的描述,计算得雨贡纽系数A1=ρc2=44 GPa,A2=ρc2(2s−1)=49.38 GPa,A3=ρc2(3s2−4s+11)=11.28 GPa,其中,c为材料声速,s为材料的经验参数。拉伸状态下的状态方程参数为T1=ρc2=A1=44 GPa,T2=0。根据文献[21]分析的结果,取孔隙压碎时的压力pel=fc/3=53.3 GPa,其他较难获得的参数,在原始文献35 MPa混凝土[22]参数基础上进行微调,并进行大量试算,获取了能表征UHPC材料的RHT本构模型。具体参数取值见表4。表 4 UHPC的RHT模型参数Table 4. RHT model parameters of UHPCρ/(kg·m−3) G/GPa fc/MPa B1 B2 T1/GPa T2 A ˙εc/s−1 ˙εt/s−1 ˙εc0/s−1 ˙εt0/s−1 2450 18.5 160 1.22 1.22 44 0 1.6 3.0×1025 3.0×1025 3.0×10−5 3.0×10−6 pel/MPa g*c g*t ξ D1 εmp Af nf A1/GPa A2/GPa A3/GPa βc 53.3 0.53 0.7 0.67 0.04 0.008 1.75 0.52 44 49.38 11.28 0.0125 βt B N D2 Q0 n f*t f*s pcom/GPa α0 0.0143 0.0105 4.0 1 0.681 0.61 0.0613 0.267 6 1.18 注:B1、B2为状态方程参数,A、n为失效面参数;˙εc为压缩失效应变率,˙εt为拉伸失效应变率,˙εc0为参考压缩应变率,˙εt0为参考拉伸应变率,g*c为压缩屈服面参数,g*t为拉伸屈服面参数,ξ为剪切模量缩减系数;D1、D2为损伤参数;εmp为最小失效应变,Af、nf为残余应力面参数,βc为压缩应变率指数,βt为拉伸应变率指数,B为罗德角相关系数,N为孔隙度指数,Q0为拉压子午比参数,pcom为孔隙完全压实时压力,α0为初始孔隙度。 2.2 材料模型验证
UHPC材料本构参数选择的准确性,直接影响到弹体侵彻UHPC靶板数值模拟的准确性,故需要对RHT本构参数进行校核。本文使用MTS815试验机以及
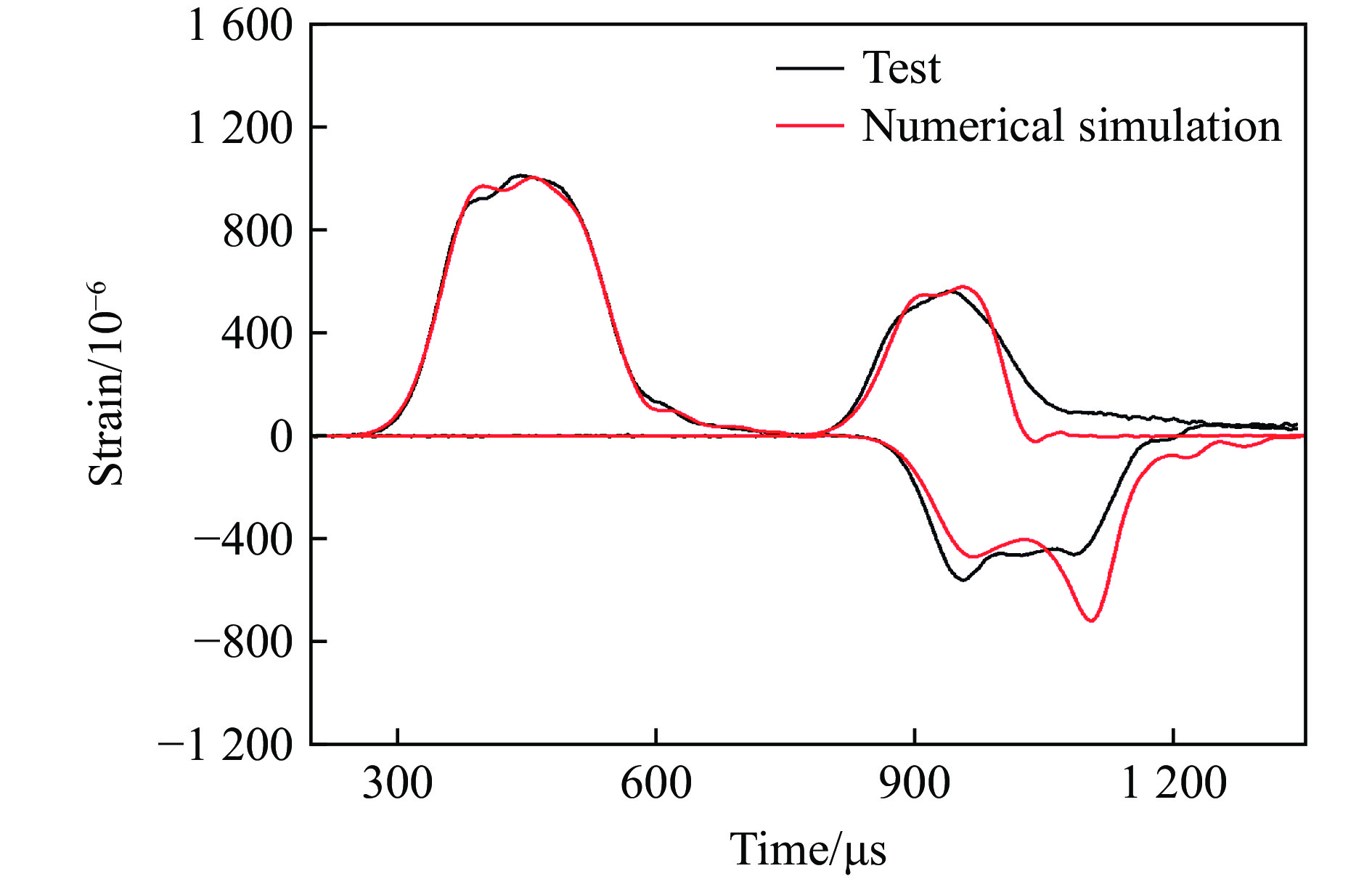
∅ 100 mm霍普金森压杆对UHPC试件进行了2种加载速率下的压缩实验,并与数值模拟进行对比(如图9所示)。其中,SHPB实验子弹冲击速度设置为8 m/s,数值模拟波形与SHPB实验波形对比,如图10所示,可以看出,数值模拟得到的波形图在趋势、峰值、持续时间都与实测波形图基本一致。同时,对数据进行处理,得到了UHPC在5×10−5 s−1和100 s−1加载速率下的应力-应变曲线,如图11所示,可以看出数值模拟得到的应力-应变曲线与实验得到的应力-应变曲线基本吻合,说明选取的材料模型可以准确地表征UHPC在压缩荷载下的响应。2.3 有限元模型
采用LS-DYNA有限元软件对弹体侵彻UHPC过程进行数值模拟,弹体与靶板模型的几何尺寸皆与试验相同。弹体与UHPC靶板均采用SOLID164三维实体单元,网格皆为六面体结构化网格。同时,为了保证计算的精度,在靶板中心0.4 m直径范围内对靶板进行加密,使靶板中心区域网格尺寸与弹体网格尺寸相近,加密区靶板网格尺寸约为2 mm,非加密区网格尺寸为4 mm,弹体网格尺寸为1.5 mm。所建模型如图12所示。
2.4 接触与边界效应
为了更好地模拟真实的试验情况,数值模型采用全尺寸计算模型,靶板与弹体接触采用面面侵蚀接触,在LS-DYNA中采用关键字*CONTACT_EROSION_SURFACE_TO_SURFACE进行定义,设置子弹为主动面,靶板为被动面。由于RHT模型中没有定义失效删除的参数,在计算中,为了避免高加载率下的计算溢出,一般通过添加关键字*MAT_ADD_EROSION来删除变形较大的单元,但值得注意的是,单元的删除会破坏模型的动量守恒和质量守恒,因此,在计算时需谨慎选取。文献[14]设定主应变与剪应变均为0.2作为失效准则,文献[13]选用拉应变与剪应变作为失效准则,并对两种UHPC分别设定为0.15与0.2。本文通过反复试算,并与试验结果进行对比,来选出合理的参数,参数一旦选定,所有计算工况都以选定的参数值进行计算。本文在计算时选用最大主应变作为失效准则,设定值为0.2,模型一旦超过设定值,即判定失效,单元便会自动删除。
2.5 模拟结果及分析
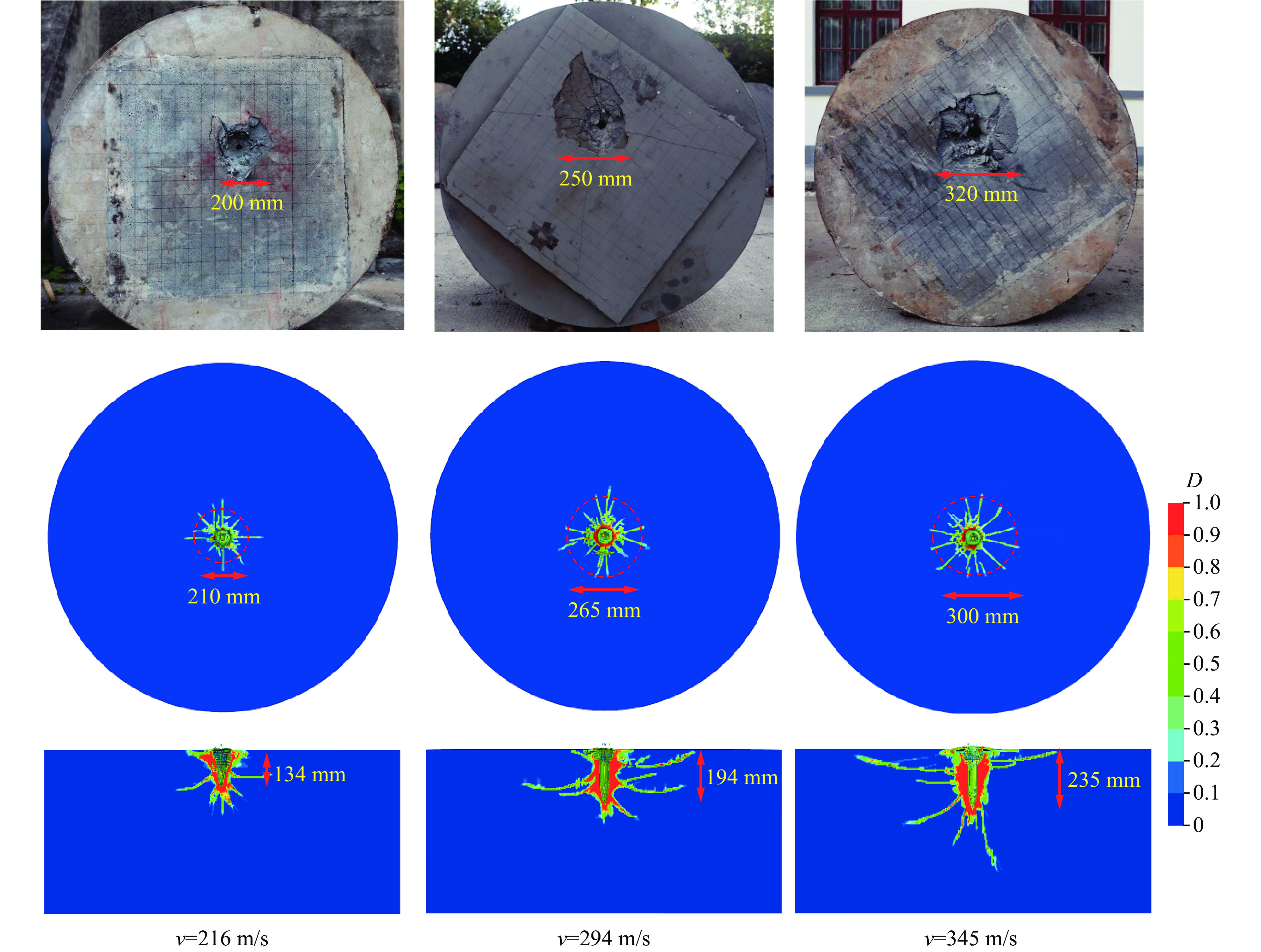
表5给出了3组试验与数值计算结果的对比,计算结果与试验结果吻合较好:在侵彻深度方面,最大误差在7.0%,最小误差约在2.9%;对于开坑直径,最大误差为6.3%,最小误差为5.0%。在数值模拟中,采用RHT模型中的损伤变量D来反映材料受弹体冲击后损伤破坏程度,损伤变量D定义为累积等效塑性应变增量与最终失效等效塑性应变的比值,0≤D≤1,当D=0时,认为材料完好无损伤,当D=1时,认为材料完全破坏。图13给出了UHPC靶板表面及内部的损伤情况,可以看出,数值模拟的结果与试验结果吻合较好。
表 5 数值模拟与试验结果对比Table 5. Comparison of experimental and numerical results试验 弹速/
(m·s−1)侵彻深度/mm 深度
误差/%开坑直径/mm 直径
误差/%试验 计算 试验 计算 1 216.2 145 134 2.9 200 210 5.0 4 294.0 209 194 5.0 250 265 6.0 9 345.4 227 243 7.0 320 300 6.3 3. 侵彻深度影响参数
对于弹体侵彻混凝土的问题,已经有大量的经验公式可供参考,但是这些经验公式都有自身的适用范围,绝大多数经验公式仅适用于普通混凝土,对UHPC侵彻深度的预测较差。
对于刚性弹侵彻混凝土问题,其侵彻深度一般可表示为[23]:
h=f(M,d,v,ϕ,ρ,E,fc,a,r,μ) (1) 式中:M、d、v分别为弹体质量、弹径、初始撞击速度;ϕ为弹头形状系数,定义为弹头曲率半径与弹体直径之比;ρ、E、fc分别为混凝土靶板密度、弹性模量、抗压强度;a为混凝土中骨料的特征长度;r为靶板中钢筋的体积含量;
μ 为弹靶间的滑动摩擦因子。为了获得更高的抗压强度,UHPC材料中通常会去除粗骨料,以改善底层材料的均匀性,所以参数a无需考虑;同时UHPC靶中无配筋,可忽略参数r的影响;根据Forrestal等[24-25]研究,当撞击速度小于800 m/s时,弹靶间的滑动摩擦仅起二阶影响,也可忽略不计;弹性模量E跟混凝土强度相互依赖,不同混凝土的弹性模量变化较小,靶板的弹性模量仅对侵彻深度有二阶影响[26-27];而对于混凝土材料,密度ρ大都集中在2300~2500 kg/m3,变化较小。式(1),表达式可进一步简化为:h=f(M,d,v,ϕ,fc) (2) 本文中共设计了29个计算工况,如表6所示,在控制其他参数不变的条件下,分别对参数M、d、v、fc、ϕ对侵彻深度的影响进行详细的分析。并基于文献[28]经验公式进行修正,使修正后的经验公式适用于UHPC侵彻深度预测。
表 6 数值模拟侵彻深度Table 6. numerical simulated penetration depth工况 fc/MPa ϕ v/(m·s−1) M/kg d/mm h/mm 1 160 10 200 1.0 30 134 2 300 209 3 350 245 4 400 288 5 450 330 6 500 370 7 550 410 8 160 10 300 0.25 30 78 9 0.5 110 10 1.0 209 11 1.5 245 12 2.0 350 13 2.5 386 14 3.0 422 15 160 4 300 1.0 30 142 16 6 161 17 8 175 18 10 209 19 12 215 20 160 10 300 1.0 18 335 21 24 239 22 30 209 23 36 162 24 42 138 25 35 10 300 1.0 30 360 26 60 270 27 90 255 28 140 211 29 160 209 3.1 弹体速度对侵彻深度的影响
图14给出了160 MPa UHPC靶板的侵彻深度随弹体速度的变化趋势,可以看出,弹体速度从200 m/s增加到550 m/s时,侵彻深度从134 mm增加到410 mm,随着速度的增加,侵彻深度显著增加。Zhang等[29]对高强混凝土进行了250~675 m/s的侵彻试验时发现侵彻深度与侵彻速度几乎为线性关系。钱七虎等[17]经过细致的研究认为,当弹体速度在一定范围内(v<0.2c,其中c为靶板材料声速),侵彻深度与弹体速度具有线性关系。本文经过拟合数值模拟数据,得出了类似的结论,认为在弹体速度200~550 m/s时,侵彻深度与弹体速度v具有线性关系。
3.2 弹体质量侵彻深度的影响
图15给出了质量分别为0.25、0.5、1.0、1.5、2.0、2.5和3.0 kg的弹体在300 m/s的侵彻速度下对160 MPa UHPC的侵彻深度结果,弹体质量从0.25 kg增长到3 kg时,侵彻深度从76 mm增加到360 mm,可以看出,随着弹体质量的增加,侵彻深度也有明显的增加。关于弹体质量对侵彻深度的影响,不同学者有不同的观点,Young[30]在大量的混凝土及岩石的侵彻试验基础上,认为对于质量大于182 kg的弹体,侵彻深度与M 0.7具有线性关系,而对于质量小于182 kg的弹体,侵彻深度与M 0.85具有线性关系。NDRC公式[28]则表明,侵彻深度与质量M具有线性关系。本文经过对模拟结果进行拟合,认为侵彻深度与M 0.825线性相关。
3.3 弹头形状对侵彻深度的影响
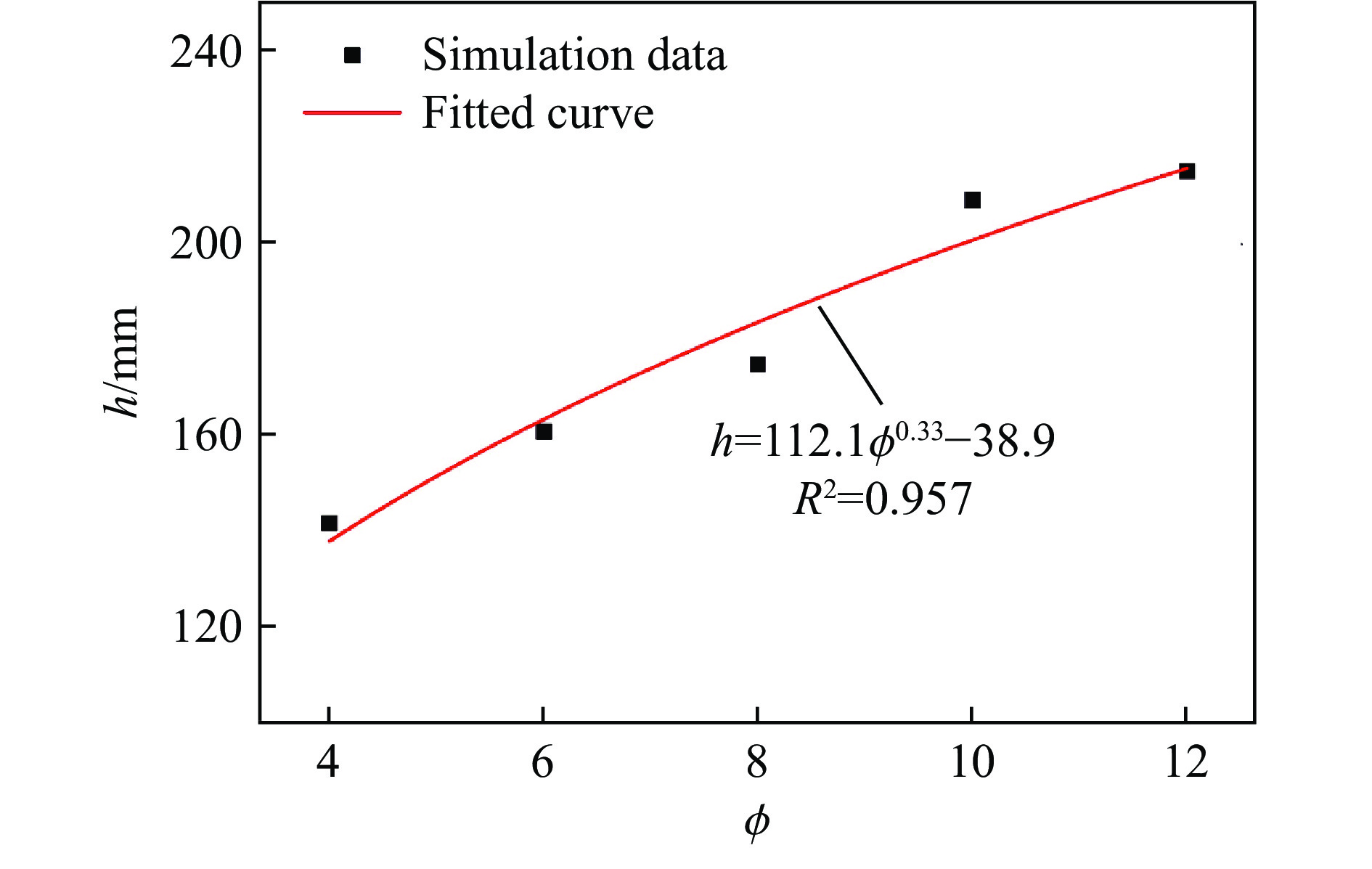
对于弹头形状对侵彻深度影响的相关研究较少,根据Wang等[31]的研究,弹头形状系数ϕ对弹体侵彻UHPC的侵彻深度有重要的影响,ϕ越大,弹头越尖锐,侵彻过程中受到的阻力也就越小,侵彻深度越大。Liu等[14]对ϕ为1、2、3的卵形弹侵彻UHPC开展了数值模拟,模拟结果显示,随着ϕ值的增大,侵彻深度也逐渐增大。本文在不改变弹体的质量与弹径的前提下,研究了ϕ为4、6、8、10、12时弹体对160 MPa UHPC靶板的侵彻性能,如图16所示,在侵彻速度为300 m/s时,ϕ从4增加到12,侵彻深度从142 mm增加到215 mm。这表明随着弹体ϕ的增大,侵彻深度也有显著增大。通过拟合计算结果,认为侵彻深度与ϕ0.33具有线性相关。
3.4 弹体直径对侵彻深度的影响
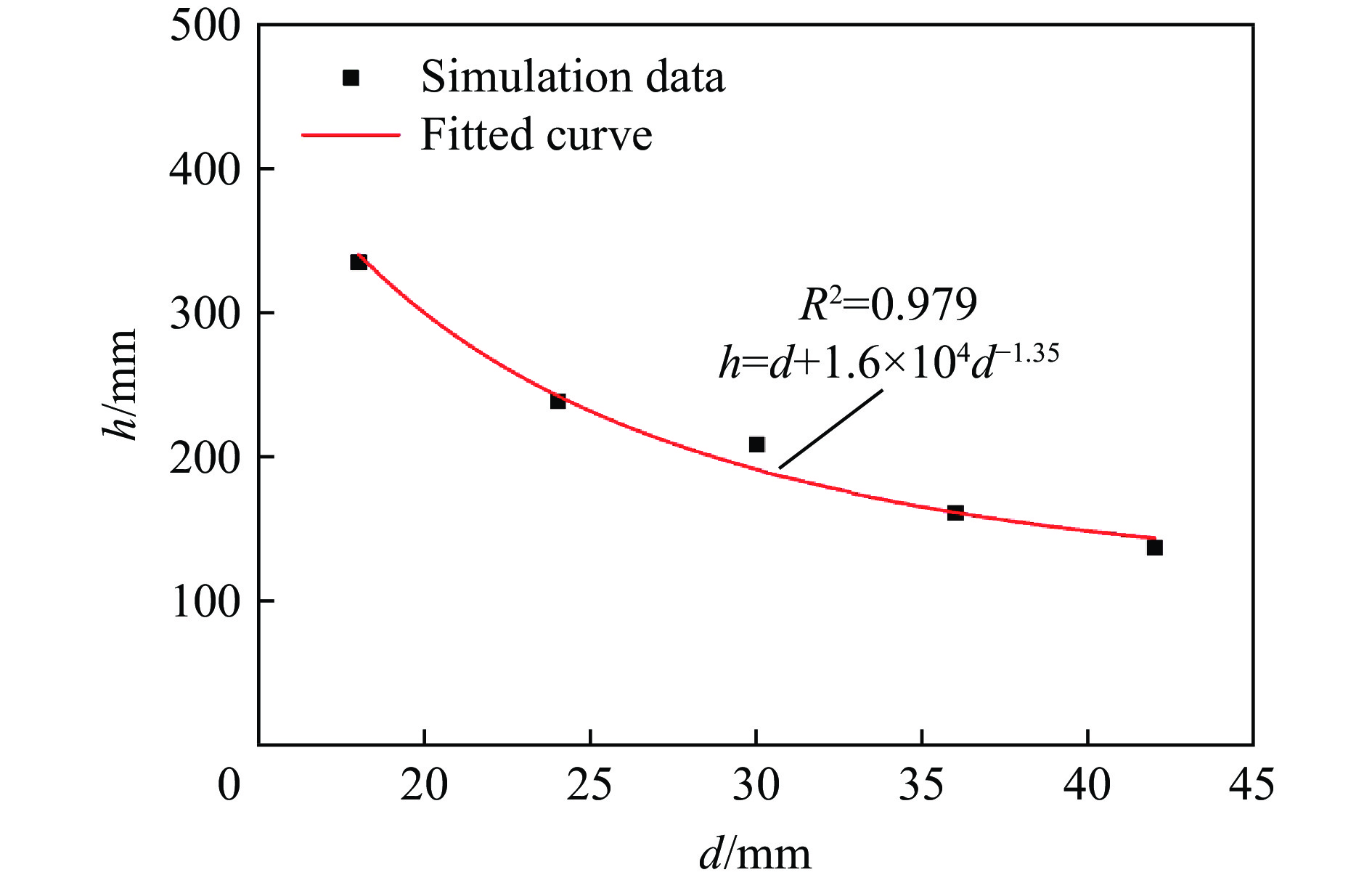
杨华伟[32]研究了不同直径的弹体对普通混凝土的侵彻,发现弹体直径对侵彻深度有显著影响,在控制弹体质量与形状不变的前提下,直径越大的弹体侵彻过程中受到的阻力越大,对应侵彻深度越低。文献[28]认为侵彻深度与弹径d −1.35线性相关,而Young公式[30]则忽略弹径的影响。本文控制弹体质量和弹头形状系数均与试验用弹相同的前提下,仅改变弹体直径,计算了弹体直径为18、24、30、36、42 mm时,弹体以300 m/s的速度侵彻160 MPa UHPC靶的侵彻深度,计算结果如图17所示,弹径从18 mm增加到48 mm,侵彻深度从335 mm降低到118 mm,经过拟合得到侵彻深度与弹径d −1.35具有线性关系。
3.5 抗压强度对侵彻深度的影响
混凝土材料的抗压强度对侵彻深度有着重要的影响,Zhang等[11]在研究中发现,侵彻深度一般随着抗压强度的增加而降低,但当抗压强度增加到一定程度后,侵彻深度的降低将不再明显。图18给出了本文数值模拟得到抗压强度为35、60、90、140和160 MPa的混凝土在速度为300 m/s时的侵彻深度。抗压强度从35 MPa增加到160 MPa时,侵彻深度从360 mm降低到209 mm。可以看出,随着混凝土抗压强度的提高,侵彻深度有明显降低。对数据进行拟合发现,侵彻深度与
f−035c 具有线性关系。3.6 侵彻深度预测模型
本文基于文献[28]的公式,提出了修正的经验公式来预测混凝土的侵彻深度:
h=d+0.0017×ϕ0.33M0.825vd1.35f0.35c200≤v≤500 (3) 式中:d为弹体直径,m;M为弹体质量,kg;v为弹体侵彻初速度,m/s;fc为混凝土的无侧限抗压强度,Pa。
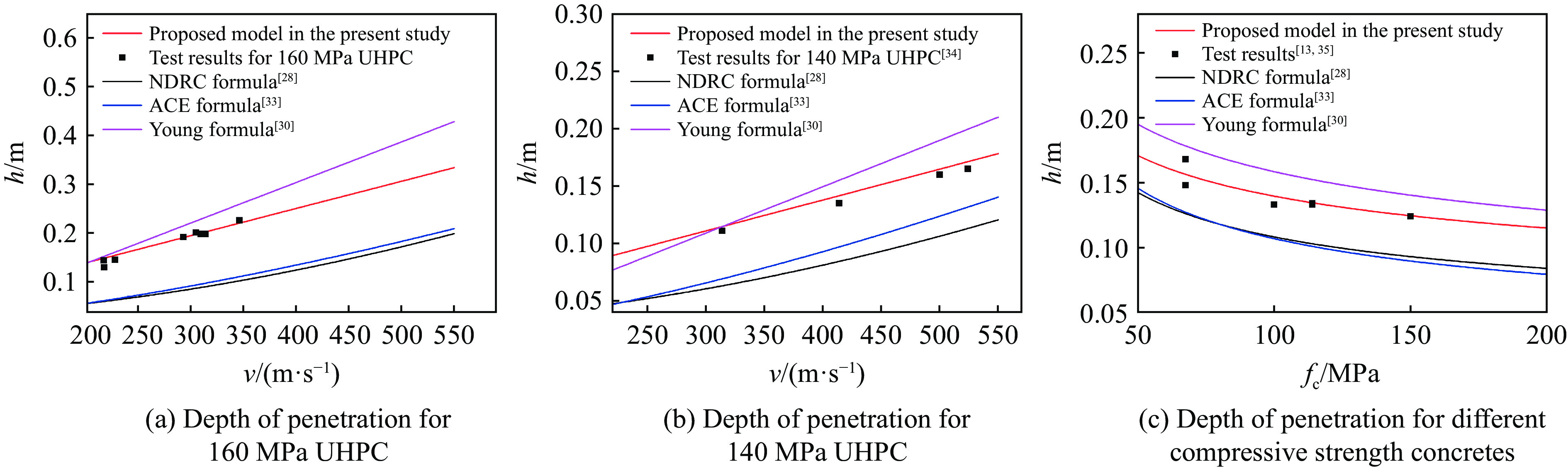
将本文拟合得到的公式与已有的常用侵彻深度计算公式进行对比,如图19所示。可以看出,文献[33]公式与文献[28]公式在预测UHPC侵彻深度时,计算结果低于实际试验值。而Young[30]公式则高估了UHPC的侵彻深度。本文拟合公式对UHPC侵彻深度的计算值与试验结果基本吻合。
4. 结 论
本文中对160 MPa UHPC靶板进行了速度为216~345 m/s的侵彻试验,并分析了侵彻深度随速度的变化规律。采用*MAT_RHT模型作为UHPC靶板材料模型,通过单轴压缩试验与SHPB试验验证了材料模型参数的有效性。在此基础上,采用LS-DYNA有限元软件对弹体侵彻UHPC过程进行了数值模拟。通过研究,得到如下结论:
(1) 对UHPC靶板进行了速度为216~345 m/s的侵彻试验,试验发现随着侵彻速度的提高,侵彻深度有较明显的增加,侵彻深度与速度具有线性关系;
(2) 确定了一套适用于UHPC的RHT材料本构参数,并通过单轴压缩试验与SHPB试验验证了模型的合理性与有效性;
(3) 利用验证过RHT模型模拟弹体对UHPC的侵彻过程,数值模拟结果与试验结果十分接近,在此基础上参数化研究了弹体速度、弹体质量、弹头形状、弹体直径、靶板强度对侵彻深度的影响,并提出了一个预测侵彻深度的经验公式,可为UHPC防护结构设计提供指导。
-
表 1 UHPC配合比
Table 1. Mixture design of UHPC
kg 水泥 石英砂 石英粉 硅灰 粉煤灰 钢纤维 水 减水剂 666 1065 160 160 80 157 135 6.7 表 2 钢纤维参数
Table 2. Parameters of steel fiber
直径/mm 长度/mm 拉伸强度/MPa 弹性模量/GPa 密度/(kg·m−3) 0.2±0.02 13±1.3 2000±300 200 7800 表 3 侵彻试验结果
Table 3. Penetration tests data
试验 M/kg v/(m·s−1) h/mm h/d dc/mm dc/d 1# 1.001 216.2 145 4.83 200 6.67 2# 1.004 216.7 132 4.40 155 5.17 3# 1.000 226.9 147 4.90 220 7.33 4# 0.999 292.0 194 6.47 250 8.33 5# 1.003 304.1 203 6.77 200 6.67 6# 1.001 308.7 199 6.63 300 10.00 7# 0.999 313.2 199 6.63 300 10.00 8# 1.003 345.4 227 7.57 320 10.67 注:M为弹体质量,v为着靶速度,h为侵彻深度,d为弹体直径,dc为开坑直径。 表 4 UHPC的RHT模型参数
Table 4. RHT model parameters of UHPC
ρ/(kg·m−3) G/GPa fc/MPa B1 B2 T1/GPa T2 A ˙εc/s−1 ˙εt/s−1 ˙εc0/s−1 ˙εt0/s−1 2450 18.5 160 1.22 1.22 44 0 1.6 3.0×1025 3.0×1025 3.0×10−5 3.0×10−6 pel/MPa g*c g*t ξ D1 εmp Af nf A1/GPa A2/GPa A3/GPa βc 53.3 0.53 0.7 0.67 0.04 0.008 1.75 0.52 44 49.38 11.28 0.0125 βt B N D2 Q0 n f*t f*s pcom/GPa α0 0.0143 0.0105 4.0 1 0.681 0.61 0.0613 0.267 6 1.18 注:B1、B2为状态方程参数,A、n为失效面参数;˙εc为压缩失效应变率,˙εt为拉伸失效应变率,˙εc0为参考压缩应变率,˙εt0为参考拉伸应变率,g*c为压缩屈服面参数,g*t为拉伸屈服面参数,ξ为剪切模量缩减系数;D1、D2为损伤参数;εmp为最小失效应变,Af、nf为残余应力面参数,βc为压缩应变率指数,βt为拉伸应变率指数,B为罗德角相关系数,N为孔隙度指数,Q0为拉压子午比参数,pcom为孔隙完全压实时压力,α0为初始孔隙度。 表 5 数值模拟与试验结果对比
Table 5. Comparison of experimental and numerical results
试验 弹速/
(m·s−1)侵彻深度/mm 深度
误差/%开坑直径/mm 直径
误差/%试验 计算 试验 计算 1 216.2 145 134 2.9 200 210 5.0 4 294.0 209 194 5.0 250 265 6.0 9 345.4 227 243 7.0 320 300 6.3 表 6 数值模拟侵彻深度
Table 6. numerical simulated penetration depth
工况 fc/MPa ϕ v/(m·s−1) M/kg d/mm h/mm 1 160 10 200 1.0 30 134 2 300 209 3 350 245 4 400 288 5 450 330 6 500 370 7 550 410 8 160 10 300 0.25 30 78 9 0.5 110 10 1.0 209 11 1.5 245 12 2.0 350 13 2.5 386 14 3.0 422 15 160 4 300 1.0 30 142 16 6 161 17 8 175 18 10 209 19 12 215 20 160 10 300 1.0 18 335 21 24 239 22 30 209 23 36 162 24 42 138 25 35 10 300 1.0 30 360 26 60 270 27 90 255 28 140 211 29 160 209 -
[1] 葛涛, 潘越峰, 谭可可, 等. 活性粉末混凝土抗冲击性能研究 [J]. 岩石力学与工程学报, 2007, 26(S1): 3553–3557. DOI: 10.3321/j.issn:1000-6915.2007.z1.148.GE T, FAN Y F, TAN K K, et al. Study on resistance of reactive powder concrete to impact [J]. Chinese Journal of Rock Mechanics and Engineering, 2007, 26(S1): 3553–3557. DOI: 10.3321/j.issn:1000-6915.2007.z1.148. [2] 曹方良. 纳米材料对超高性能混凝土强度的影响研究 [D]. 长沙: 湖南大学, 2012: 1–4.CAO L F. Study on the effects of nano-materials on the strength of ultra high performance concrete [D]. Changsha: Hunan University, 2012: 1–4. [3] 任亮, 何瑜, 王凯, 等. 基于SHPB的UHPC冲击试验径向惯性效应分析 [J]. 爆炸与冲击, 2019, 39(10): 104104. DOI: 10.11883/bzycj-2018-0335.REN L, HE Y, WANG K, et al. Radial inertia effect analysis of UHPC impact test based on SHPB [J]. Explosion and Shock Waves, 2019, 39(10): 104104. DOI: 10.11883/bzycj-2018-0335. [4] 程月华, 吴昊, 谭可可, 等. 装甲钢/UHPC复合靶体抗侵彻性能试验与数值模拟研究 [J]. 爆炸与冲击, 2022, 42(5): 053302. DOI: 10.11883/bzycj-2021-0278.CHENG Y H, WU H, TAN K K, et al. Experimental and numerical studies on penetration resistance of armor steel/UHPC composite targets [J]. Explosion and Shock Waves, 2022, 42(5): 053302. DOI: 10.11883/bzycj-2021-0278. [5] TAI Y S. Flat ended projectile penetrating ultra-high strength concrete plate target [J]. Theoretical and Applied Fracture Mechanics, 2009, 51(2): 117–128. DOI: 10.1016/j.tafmec.2009.04.005. [6] WU H, FANG Q, GONG J, et al. Projectile impact resistance of corundum aggregated UHP-SFRC [J]. International Journal of Impact Engineering, 2015, 84: 38–53. DOI: 10.1016/j.ijimpeng.2015.05.007. [7] SOVJÁK R, VAVŘINÍK T, MÁCA P, et al. Experimental investigation of ultra-high performance fiber reinforced concrete slabs subjected to deformable projectile impact [J]. Procedia Engineering, 2013, 65: 120–125. DOI: 10.1016/j.proeng.2013.09.021. [8] 张文华, 张云升, 陈振宇. 超高性能混凝土抗缩比钻地弹侵彻试验及数值仿真 [J]. 工程力学, 2018, 35(7): 167–175,186. DOI: 10.6052/j.issn.1000-4750.2017.03.0237.ZHANG W H, ZHANG Y S, CHEN Z Y. Penetration test and numerical simulation of ultral-high performance concrete with a scaled earth penetrator [J]. Engineering Mechanics, 2018, 35(7): 167–175,186. DOI: 10.6052/j.issn.1000-4750.2017.03.0237. [9] 赖建中, 过旭佳, 朱耀勇. 超高性能混凝土抗侵彻及抗爆炸性能研究 [J]. 河北工业大学学报, 2014, 43(6): 50–53. DOI: 10.14081/j.cnki.hgdxb.2014.06.013.LAI J Z, GUO X J, ZHU Y Y. Properties of ultra-high performance concrete subjected to penetration and explosion [J]. Journal of Hebei University of Technology, 2014, 43(6): 50–53. DOI: 10.14081/j.cnki.hgdxb.2014.06.013. [10] ZHAI Y X, WU H, FANG Q. Impact resistance of armor steel/ceramic/UHPC layered composite targets against 30CrMnSiNi2A steel projectiles [J]. International Journal of Impact Engineering, 2021, 154: 103888. DOI: 10.1016/j.ijimpeng.2021.103888. [11] ZHANG F L, POH L H, ZHANG M H. Critical parameters for the penetration depth in cement-based materials subjected to small caliber non-deformable projectile impact [J]. International Journal of Impact Engineering, 2020, 137: 103471. DOI: 10.1016/j.ijimpeng.2019.103471. [12] WU H, FANG Q, CHEN X. W, et al. Projectile penetration of ultra-high performance cement based composites at 510–1320 m/s [J]. Construction and Building Materials, 2015, 74: 188–200. DOI: 10.1016/j.conbuildmat.2014.10.041. [13] LIU J, WU C Q, SU Y, et al. Experimental and numerical studies of ultra-high performance concrete targets against high-velocity projectile impacts [J]. Engineering Structures, 2018, 173: 166–179. DOI: 10.1016/j.engstruct.2018.06.098. [14] LIU J, WU C Q, CHEN X W, et al. Numerical study of ultra-high performance concrete under non-deformable projectile penetration [J]. Construction and Building Materials, 2017, 135: 447–458. DOI: 10.1016/j.conbuildmat.2016.12.216. [15] 梁斌. 弹丸对有界混凝土靶的侵彻研究 [D]. 北京: 中国工程物理研究院, 2004: 10−15. [16] 薛建锋. 弹体侵彻与贯穿混凝土靶的效应研究 [D]. 南京: 南京理工大学, 2016: 96−105.XUE J F. Research on the performance of projectile penetration and perforation into concrete target [D]. Nanjing: Nanjing University of Science & Technology, 2016: 96−105. [17] 钱七虎, 王明洋. 岩土中的冲击爆炸效应 [M]. 北京: 国防工业出版社, 2010.QIAN Q H, WANG M Y. Impact and explosion effects in rock and soil [M]. Beijing: National Defense Industry Press, 2010. [18] 刘志林, 王晓鸣, 李文彬, 等. 靶板厚度对卵形弹丸垂直贯穿中等厚度混凝土靶的影响 [J]. 爆炸与冲击, 2018, 38(5): 1083–1090. DOI: 10.11883/bzycj-2017-0078.LIU Z L, WANG X M, LI W B, et al. Numerical and experimental study of an ogival projectile vertical perforating a medium thickness concrete target [J]. Explosion and Shock Waves, 2018, 38(5): 1083–1090. DOI: 10.11883/bzycj-2017-0078. [19] 王晓飞, 周海龙, 王海龙. 超高性能混凝土的抗剪强度 [J]. 硅酸盐学报, 2022, 50(8): 2190–2195. DOI: 10.14062/j.issn.0454-5648.20220127.WANG X F, ZHOU H L, WANG H L. Shear strength of ultra-high performance concrete [J]. Journal of the Chinese Ceramic Society, 2022, 50(8): 2190–2195. DOI: 10.14062/j.issn.0454-5648.20220127. [20] 李洪超. 岩石RHT模型理论及主要参数确定方法研究 [D]. 北京: 中国矿业大学(北京), 2016: 56–57.LI H C. The study of the rock RHT model and to determine the values of main parameters [D]. Beijing: China University of Mining & Technology (Beijing), 2016: 56–57. [21] HOLMQUIST T J, JOHNSON G R, COOK W H. A computational constitutive model for concrete subjected to large strains, high strain rates and high pressures [C]//Proceedings of the 14th International Symposium on Ballistics. Québec City, 1993: 591–600. [22] RIEDEL W, THOMA K, HIERMAIER S. Penetration of reinforced concrete by BETA-B-500 numerical analysis using a new macroscopic concrete model for hydrocodes [C]// Proceedings of the 9th International Symposium Interaction of the Effect of Munitions with Structures. Berlin, 1999. [23] LI Q M, CHEN X W. Dimensionless formulae for penetration depth of concrete target impacted by a non-deformable projectile [J]. International Journal of Impact Engineering, 2003, 28(1): 93–116. DOI: 10.1016/S0734-743X(02)00037-4. [24] FORRESTAL M J. Penetration into dry porous rock [J]. International Journal of Solids and Structures, 1986, 22(12): 1485–1500. DOI: 10.1016/0020-7683(86)90057-0. [25] FORRESTAL M J, ALTMAN B S, CARGILE J D, et al. An empirical equation for penetration depth of ogive-nose projectiles into concrete targets [J]. International Journal of Impact Engineering, 1994, 15(4): 395–405. DOI: 10.1016/0734-743X(94)80024-4. [26] FORRESTAL M J, LUK V K. Dynamic spherical cavity-expansion in a compressible elastic-plastic solid [J]. Journal of Applied Mechanics, 1988, 55(2): 275–279. DOI: 10.1115/1.3173672. [27] LUK V K, FORRESTAL M J, AMOS D E. Dynamic spherical cavity expansion of strain-hardening materials [J]. Journal of Applied Mechanics, 1991, 58(1): 1–6. DOI: 10.1115/1.2897150. [28] National Defense Research Committee. Effects of impact and explosion: summary technical report of division 2, Vol. 1 [R]. Washington DC: National Defense Research Committee, 1946. [29] ZHANG M H, SHIM V P W, LU G, et al. Resistance of high-strength concrete to projectile impact [J]. International Journal of Impact Engineering, 2005, 31(7): 825–841. DOI: 10.1016/j.ijimpeng.2004.04.009. [30] YOUNG C W. Penetration equations: SAND-97-2426 [R]. Albuquerque: Sandia National Laboratories, 1997. [31] WANG Z L, LI Y C, SHEN R F, et al. Numerical study on craters and penetration of concrete slab by ogive-nose steel projectile [J]. Computers and Geotechnics, 2007, 34(1): 1–9. DOI: 10.1016/j.compgeo.2006.09.001. [32] 杨华伟. 尖卵形长杆弹侵彻半无限混凝土靶的动力学行为研究 [D]. 太原: 太原理工大学, 2018: 44–49.YANG H W. Investigation on the dynamic response of long ogive-nosed projectiles penetrating into semi-infinite concrete targets [D]. Taiyuan: Taiyuan University of Technology, 2018: 44–49. [33] CHELAPATI C V, KENNEDY R P, WALL I B. Probabilistic assessment of aircraft hazard for nuclear power plants [J]. Nuclear Engineering and Design, 1972, 19(2): 333–364. DOI: 10.1016/0029-5493(72)90136-7. [34] 任辉启, 穆朝民, 刘瑞超, 等. 精确制导武器侵彻效应与工程防护 [M]. 北京: 科学出版社, 2016: 293–294.REN H Q, MU C M, LIU R C, et al. Penetration effects of precision guided weapons and engineering protection [M]. Beijing: Science Press, 2016: 293–294. [35] RONG Z D, SUN W, ZHANG Y S, et al. Anti-penetration behavior of ultra-high performance steel fiber reinforced concrete and its numerical simulation [J]. Journal of the Chinese Ceramic Society, 2010, 38(9): 1723–1730. DOI: 10.14062/j.issn.0454-5648.2010.09.001. 期刊类型引用(2)
1. 程月华,吴昊,岑国华,张瑜. 侵彻爆炸联合作用下超高性能混凝土遮弹层设计. 爆炸与冲击. 2025(01): 90-103 .  本站查看
本站查看2. 成鑫磊,穆锐,刘晓英. 超高性能混凝土的制备及力学性能研究进展. 硅酸盐通报. 2024(12): 4295-4312 .  百度学术
百度学术其他类型引用(1)
-










 下载:
下载:










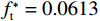
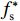
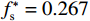








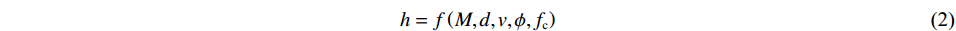









 下载:
下载:



















 百度学术
百度学术