Distribution pattern and simplified model of blast load for building columns under near-field near-ground explosion
-
摘要: 为了快速评估近场近地爆炸荷载下建筑柱的动力响应和破坏模式,通过数值仿真方法,探究了近场近地爆炸工况下冲击波在建筑柱迎爆面的分布规律,并提供了该工况下的爆炸荷载简化模型。为此,首先利用已有实验数据验证数值模型,并建立典型近地近场爆炸工况的数值模型,然后研究比例爆距和比例爆高对建筑柱冲击波特征参数的影响规律,最后拟合出柱迎爆面反射冲量和正相超压持续时间的计算公式,将柱迎爆面各点爆炸荷载转化为等效三角形荷载模型,为工程实践中建筑柱遭受近场近地爆炸作用下的抗爆设计提供荷载输入。研究结果表明:当比例爆高小于0.3 m/kg1/3、比例爆距在0.4~0.6 m/kg1/3范围时,最大反射冲量沿柱高可简化为三折线分布;当比例爆距在0.6~1.4 m/kg1/3范围时,最大反射冲量沿柱高可近似简化为双折线分布;在同一比例爆距和比例爆高工况下,随着炸药当量的增加,柱迎爆面相同比例高度处反射超压峰值保持不变而反射冲量正比于当量的立方根。Abstract: To rapidly assess the dynamic responses and failure modes of the building columns under near-field near-ground explosions, in this paper numerical simulation method is employed to investigate the distribution pattern of the shock waves that are applied on the front face of building columns under near-field near-ground blast scenarios, and a corresponding simplified blast load model is proposed. To this end, firstly, the existing experimental data of overpressure and impulse were selected to validate the numerical model for blast load. Then, a typical numerical model under near-field near-ground blast scenarios was established to study the effects of the scaled distance and the scaled height of spherical charges on the characteristic values of the shock waves acting at the building columns. Finally, formulae for the maximum reflected impulse and the representative value of the positive overpressure duration were derived based on nonlinear regression analysis, and the blast load at each location of the column front face was represented by an equivalent triangular load model. The results indicate that when the scaled height of the charge is less than 0.3 m/kg1/3, the distribution of the maximum reflected impulse along the column length can be represented as a trilinear model and a bilinear model for the scaled distance of 0.4−0.6 m/kg1/3 and 0.6−1.4 m/kg1/3, respectively. In comparison, the distribution of the shock waves in the transverse direction of a column section was approximately uniform. Moreover, under a given scaled distance and a scaled height, the peak reflected overpressure remains constant as the charge weight increases, but the maximum reflected impulse is proportional to the cubic root of the charge weight at the locations with the identical scaled height of the column.
-
Key words:
- building column /
- near-field blast /
- near-ground blast /
- blast load /
- load model
-
结构柱作为建筑关键竖向承力构件,一旦失效可能导致结构倒塌[1-3]。尤其,建筑结构边框架柱在服役期间更有可能遭受各种意外爆炸事故或恐袭爆炸的影响,故对柱进行抗爆设计具有重大意义。抗爆设计的第一要务是确定爆炸冲击波在柱表面形成的荷载分布情况。现有抗爆设计手册[4]将非密闭空间内的爆炸工况分为空中自由爆炸、近地爆炸和地表爆炸,并使用冲击波在目标位置形成的入射(反射)超压峰值、冲量、正相超压持续时间等特征参数来评估爆炸荷载。
对于空中自由爆炸工况,很多学者在爆炸相似定律理论的基础上给出了入射超压与基于无限大刚性反射面的反射超压计算公式或图表,如Brode公式(1955)[4]、Baker公式(1973) [5]、Henrych公式(1979)[6]以及UFC-3-340-02(2008)手册[7]。对于远场爆炸的防护设计,爆炸荷载常取均匀分布,但随着精确制导武器和恐袭爆炸的发展,近年来近场爆炸对建筑结构的破坏受到更多关注。汪维等[8]通过数值模拟分析空中爆炸工况下(比例爆距0.25~2 m/kg1/3)方板表面的反射超压峰值和冲量的空间分布情况,建立了对应的分布函数,提出了计算板面任意点爆炸荷载的简化预测公式。Wu等[9]通过数值方法研究了地表爆炸时入射超压与反射超压的关系,提出了在地表爆炸工况下预测入射超压、反射超压和正相超压持续时间等参数的计算公式,并得出地表爆炸冲击波在刚性墙竖直方向形成的超压分布规律,建立了对应的爆炸荷载模型。Xiao等[10]关注近地爆炸工况下爆炸冲击波的自由传播规律,研究由马赫波、地面反射波和入射波三波交汇形成的三波点高度发展规律,并回归出三波点高度的计算公式。
近年来,学者对建筑柱在近场爆炸[11-13]和接触爆炸[14-15]下的动力响应、破坏机理和评估方法展开了大量的研究,但仅有少量工作关注了近场爆炸条件下柱子表面上的爆炸荷载分布。Hu等[16]研究了空中两端起爆圆柱形炸药(比例爆距0.2~2 m/kg1/3)在建筑柱迎爆面形成的爆炸荷载分布,提出将沿柱高不均匀分布的爆炸荷载简化为分区段面荷载,并通过数值结果拟合了每段面荷载的超压峰值、正相超压持续时间、衰减系数等关键参数,建立了在对应柱中位置的圆柱形炸药两端起爆工况下柱迎爆面的荷载分布模型。由于实验中柱子是平躺放置的,无法考虑地表反射对爆炸荷载的影响。彭玉林等[17]研究了近地爆炸下圆柱形桥墩柱表面的爆炸荷载分布(比例爆距0.5~2.1 m/kg1/3,起爆高度0~0.3倍柱高),结果表明起爆高度在0.1倍柱高范围内反射冲量沿柱高分布可近似为单线性,在0.1~0.3倍高度范围内反射冲量沿柱高可看作双线性分布,并提出了柱表面的净反射冲量计算方法。而值得注意的是,相对于桥梁柱,建筑柱周围的围护结构使冲击波无法第一时间绕射到柱侧面及背面,从而导致建筑柱的爆炸荷载分布有别于桥梁柱。
综上所述,现有研究已经为空中自由爆炸、地表爆炸和近地爆炸工况提供了较多可用于预测结构构件表面爆炸荷载的经验公式,但对于近场近地爆炸下单面受荷的建筑柱表面形成的爆炸荷载研究较少。而且,爆炸入射波与地面反射波的相互追赶、叠加使近场近地爆炸工况更加复杂。因此,本文首先利用已有实验数据验证冲击波数值模型,构建典型近场近地爆炸工况的数值模型,然后研究比例爆距和比例爆高对建筑柱冲击波特征参数的影响,最后拟合柱迎爆面反射冲量和正相超压持续时间的计算公式,获取爆炸荷载等效的简化三角形荷载模型,为工程实践中建筑柱遭受近场近地爆炸作用下的抗爆设计提供荷载输入。
1. 钢筋混凝土柱抗爆的荷载数值模型验证
1.1 参照实验的介绍
分别选取Woodson等[18]与Liu等[19]实验得到的钢筋混凝土(reinforced concrete, RC)柱表面超压、冲量数据并建立对应的数值模型,以验证本文数值模型的合理性。
(1) Woodson实验[18]
Woodson的爆炸实验如图1(a)所示,两层的缩尺框架平面长2跨宽1跨,半球形的炸药放在底层中柱的正前方。根据迎爆面立面形式共有四种工况类型,分别为:两层均无填充墙(Test 1、Test 2)、两层均有全填充墙(Test 3)、两层均有开口填充墙(Test 4)和仅顶层有全填充墙的车库(Test 5)。在实验时由于Test 1炸药放在距离柱1520 mm处未得到预期的柱子损伤结果,故将Test 2~5炸药距离减小为1070 mm。由于Test 4中的填充墙开口尺寸未提供,故本文数值模型验证针对Test 1、2、3、5的底层中柱爆炸荷载进行模拟,并将数值模拟结果与迎爆面测点BC1、BC3以及背爆面测点BC2实验数据进行对比,其中测点BC1、BC2、BC3分别位于柱高305、457、762 mm处。对应的实验工况如表1所示。
(2) Liu实验[19]
Liu等[19]进行了四次不同比例爆距的近场爆炸下拼装桥墩柱表面反射超压测试实验。在每次实验中,在0.21、0.63、1.05、1.47、1.89 m高度处的柱迎爆面、侧爆面、背爆面均布置反射超压测点(pr),并在另一侧一定水平距离处布置入射超压测点(pso1和pso2),如图1(b)所示,对应的实验工况如表2所示。
1.2 有限元数值模型
考虑到上述两组参照实验中炸药起爆后的冲击波在空气域中轴对称传播,因此,为了提高计算效率,基于LS-DYNA软件分别建立二维和三维有限元模型,如图2所示,其中,Woodson实验[18]使用半球形炸药,Liu实验[19]使用方形炸药。在冲击波即将抵达目标面之前采用二维轴对称模型计算,然后通过关键字*ALE_MAPPING将冲击波信息映射到三维模型中,并进行后续考虑冲击波反射过程的计算。二维模型由空气、炸药和刚性地面组成,三维模型由空气、刚性柱和刚性地面组成。炸药和空气域均采用ALE网格描述(*SECTION_ALE);刚性地面与刚性柱表面使用约束节点自由度的方法进行模拟(*SPC_SET);二维对称边界使用默认设置;二维和三维空气边界均设为无反射边界。基于网格敏感性分析,对于Woodson实验[18]和Liu实验[19]对应的数值模型,二维空气网格尺寸取为4 mm,三维空气网格取10 mm。
1.3 材料模型
(1) 空气材料本构及状态方程
假定空气为理想气体,通过不含剪切模量只有动力粘性的流体*MAT_NULL材料模型进行表征,对应的状态方程采用关键字*EOS_LINEAR_POLYNOMIAL描述:
p=C0+C1μ+C2μ2+C3μ3+(C4+C5μ+C6μ2)E (1) 式中:p为空气压力;E为空气的初始单位体积内能,本文取E=0.25 J/mm3;μ=ρ/ρ0−1,ρ和ρ0分别为空气当前和初始密度,其中ρ0=1.29×10−6 g/mm3;C0~C6为状态方程的参数,当用于空气时,除了C4=C5=0.4,其余均取0。
(2) TNT炸药材料本构及状态方程
对TNT炸药材料的模拟采用材料模型*MAT_HIGH_EXPLOSIVE_BURN,其中炸药密度取ρ0=1630 kg/m3,爆速为D=6930 m/s。炸药采用JWL方程描述,其形式为:
p=A(1−ωR1V)e−R1V+B(1−ωR2V)e−R2V+ωE0V (2) 式中:p表示炸药的轰爆压力;E0表示单位体积炸药的初始内能,本文中取E0=7×109 J/mm3;V为爆炸前后的相对体积;A、B、ω、R1、R2均为材料计算常数,本文取值A=21 GPa, B=3.23 GPa, ω=0.3, R1=4.15, R2=0.9。上述空气和炸药材料参数选取与学者广泛使用的取值保持一致[17]。
1.4 数值模型验证
针对以上两组参照实验,数值结果与实验结果的对比分别如表3和表4所示。可见,本文模拟方法对超压的预测误差平均值在20%以内,对冲量的预测误差平均值在6%以内。由于近场作用下冲击波传播的复杂性、数值模型刚性地面假设与实验测量质量高低均会导致误差,故上述误差在可接受范围内,因此上述模型可用来研究近场近地爆炸荷载在建筑柱上的分布规律。
表 3 Woodson实验[18]爆炸荷载的模拟结果与实测值对比Table 3. Comparisons of numerical values and Woodson’s experimental[18] results工况 测点 pr/MPa Ir/(kPa∙s) 误差/% 工况 测点 pr/MPa Ir/(kPa∙s) 误差/% 实测[18] 模拟 实测[18] 模拟 pr Ir 实测[18] 模拟 实测[18] 模拟 pr Ir 实验1 BC1 8.32 12.17 1.04 1.10 46.27 5.77 实验3 BC1 15.05 16.61 3.02 3.43 10.37 13.58 BC2 0.40 0.56 0.24 0.19 40.00 −20.83 BC2 BC3 9.36 6.23 1.22 1.02 −33.44 −16.39 BC3 9.57 15.67 1.71 2.28 63.74 33.33 实验2 BC1 11.52 12.53 2.77 2.38 8.77 −14.08 实验5 BC1 11.52 12.53 2.33 2.38 8.77 2.15 BC2 0.66 0.47 −28.79 BC2 0.66 0.47 −28.79 BC3 10.41 15.23 1.23 1.67 46.30 35.77 BC3 10.41 15.23 1.48 1.67 46.30 12.84 注:pr为超压,Ir为冲量;所有超压和冲量模拟误差的算术平均值为16.32%和5.79%。 表 4 Liu实验[19]超压模拟结果与实测值对比Table 4. Comparison of numerical predictions and Liu’s experimental[19] results测点 Case 1超压/MPa 误差/% 测点 Case 2超压/MPa 误差/% 测点 Case 3超压/MPa 误差/% 实测 模拟 实测 模拟 实测 模拟 so 0.21 0.12 −42.86 ry2 0.31 0.23 −25.81 ry2 0.52 0.57 9.62 ry3 0.14 0.15 7.14 ry3 0.43 0.21 −51.16 ry3 0.77 0.84 9.09 rb3 0.06 0.03 −50.00 ry4 0.39 0.23 −41.03 ry4 0.50 0.57 14.00 rb3 0.08 0.04 −50.00 so 0.74 0.82 10.81 平均 −28.57 −42.00 10.88 注:三个工况超压模拟误差的算术平均值为−19.90% 2. 典型近场近地球形炸药爆炸作用下的荷载数值模型
2.1 数值模型的建立
在上述验证的数值模型基础上,为了研究近场近地爆炸下建筑柱迎爆面爆炸荷载的分布规律,选取典型原型柱尺寸为500 mm×500 mm×3 000 mm,并在柱周围建立刚性填充墙(墙厚250 mm)以避免冲击波在迎爆面的荷载作用时间内发生绕射,数值模型如图3所示。为了消除无反射边界的影响,结构到空气边界距离预留500 mm。数值模型中空气网格尺寸严重影响计算精度与计算效率[20],且柱边填充墙的宽度如果过大,会导致数值模型尺寸增大,进而增加空气单元数量,降低计算效率。因此,在计算之前先对数值模型的二维、三维空气网格尺寸和柱边填充墙宽度(Bw)进行参数敏感性研究。参数分析时均取炸药起爆爆心高度Hc=1 000 mm,取二维模型中比例爆距Z=0.5 m/kg1/3、高度h=1 000 mm位置处入射超压时程曲线展示二维网格尺寸对计算精度的影响;取三维模型中比例爆距Z=1.0 m/kg1/3、柱高h=500 mm位置处得反射超压时程曲线展示三维网格和柱边墙宽对计算精度的影响,分析结果如图4所示。
通过图4的结果对比,最终确定数值模型二维和三维网格分别为5和20 mm,避免冲击波发生绕射的墙宽为500 mm。预选球形TNT炸药当量W=8 kg,通过改变爆心到柱表面和地面的距离(R和Hc)从而改变比例爆距(Z)和比例爆高(Hc/W1/3),研究不同近地近场爆炸工况下柱迎爆面爆炸荷载的分布规律。
2.2 数值模拟结果
以比例爆距0.6 m/kg1/3、比例爆高0.1 m/kg1/3的工况为例,对应的冲击波传播过程如图5~7所示。可见,近地球形炸药起爆后,形成入射波、地面反射波和地表马赫波三波交汇向前传播,三波交汇点(三波点T)以下冲击波传播过程更加复杂[10];在冲击波到达柱迎爆面之后,由于填充墙的存在,冲击波无法在柱表面绕射,此时刚性柱迎爆面将充当类似地面的作用,入射波、柱表面反射波和柱表面马赫波在柱迎爆面也形成了三波点,三波在柱表面交汇后继续沿柱高向上传播。
已有研究[20]发现,数值模拟计算时冲量受模型网格尺寸的影响往往比超压的小,故冲量的数值结果更加准确,也有人提出了基于最大冲量的超压修正方法,所以近场下对最大冲量的拟合更加有效。通过改变比例爆距和比例爆高,并沿柱高度0~3000 mm内每隔20 mm布置测点,获得多组近场近地爆炸工况下柱迎爆面最大反射冲量(Ir)的分布规律,如图8所示。可见,在给定比例爆高条件下,随比例爆距增大,柱迎爆面最大反射冲量Ir减小,且当Z>0.6 m/kg1/3时,Ir沿柱高变化趋于平缓。Ir的分布特征有以下几种形式:(1) 底部“针状峰值”分布,由于刚性地表反射增强柱底位置的Ir,如图8所示;(2) “1/x函数形分布”,即柱底大,柱顶小的分布,见图8(a)和图8(b);(3) 与爆心等高处的“局部峰值”分布,由于冲击波的直接入射、反射占主导地位,见图8(d)和图8(e)。基于模拟结果,Ir可分为四个区间的分布形态,如表5所示。
表 5 反射冲量沿柱高分布特征及简化模型Table 5. Distribution characteristics and simplified models of reflected impulse along column length爆心高度(Hc/W1/3) 分布特征及简化模型 小比例爆距(0.4≤Z/(m∙kg−1/3)≤0.6) 大比例爆距(0.6<Z/(m∙kg−1/3)≤1.4) 小比例爆高(≤0.3 m/kg1/3) “1/x函数形分布”,可简化为三折线模型 柱底“针状峰值”分布,可简化为双折线模型 大比例爆高(>0.3 m/kg1/3) “局部峰值”归为局部突变分布,建议通过数值方法确定荷载 线性分布,可简化为双折线模型 3. 近场近地爆炸荷载简化模型
为了能够使用公式预测近地近场下最大反射冲量的分布规律,将表5最大反射冲量可简化为3种分布模型:(1) 双折线模型,适用范围,Z>0.6 m/kg1/3;(2) 三折线模型,适用范围,Z≤0.6 m/kg1/3且Hc/W1/3≤0.3 m/kg1/3;(3) 局部突变模型,适用范围为,Z≤0.6 m/kg1/3且Hc/W1/3>0.3 m/kg1/3。其中,对于局部突变模型工况,爆炸产生的最大反射冲量分布规律应根据具体实验或数值分析获得。由于在近场爆炸作用下,冲量相对于超压对结构的动力响应和破坏模式往往更具主导作用,所以本文针对冲量分布可简化的工况首先提出冲量分布函数,再将爆炸冲击波在柱迎爆面某点形成的反射超压(pr)时程曲线根据最大反射冲量值相等的原则等效为三角形荷载。最后,给出柱迎爆面各点最大反射冲量(Ir)和正相超压持续时间(t0)的计算公式。
3.1 冲量沿高度分布
最大反射冲量双折线和三折线模型的简化如图9所示。为了确定对应的分布函数,定义如下参数:Ir-t、Ir-b、Ir-m分别为柱顶、柱底和柱中的最大反射冲量;Ir-a为三折线模型转折点处最大反射冲量。
在近场近地爆炸工况下,柱迎爆面距离地面高度为h处反射冲量Ir-h是炸药当量W、爆炸距离R、起爆中心距离地面高度Hc的函数,采用中间变量比例爆距(Z)和比例爆高(Hc/W1/3)对Ir-t、Ir-b和Ir-m进行拟合,且Ir-b,Ir-m/Ir-b和Ir-t/Ir-b均取相同的拟合方程形式:
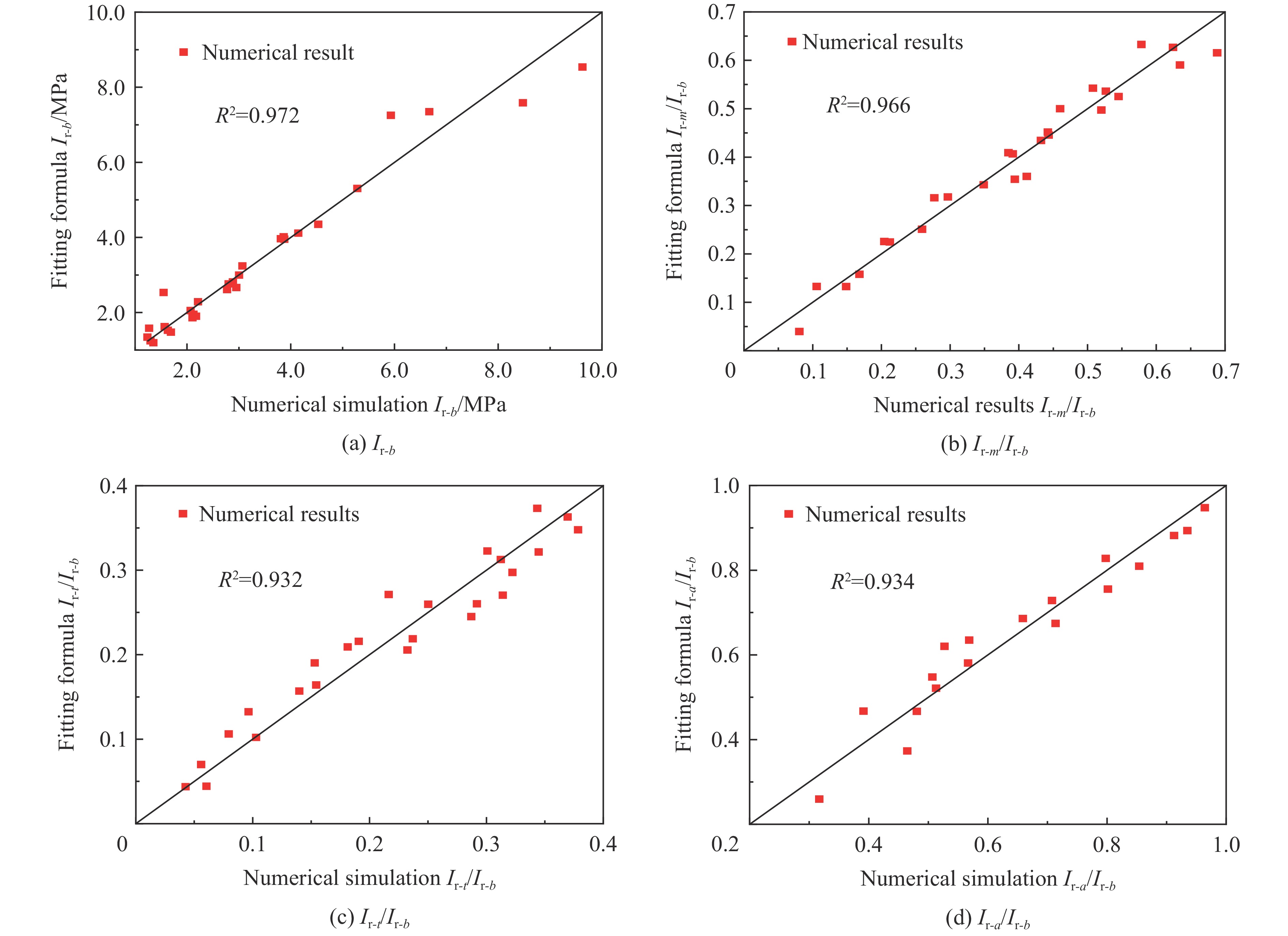
i=a(R3√W)b+c(Hc3√W)d (3) 式中:i代表Ir-b、Ir-m/Ir-b或Ir-t/Ir-b。式(3)的使用范围为:0.4 m/kg1/3≤Z≤1.4 m/kg1/3, 0.1 m/kg1/3≤Hc/ W1/3≤0.5 m/kg1/3;参数a、b、c和d的取值见表6,式(3)的拟合精度如图10(a)~(c)所示,相关系数平方(R2)均在0.93以上。
表 6 反射冲量拟合计算公式的参数取值Table 6. Coefficients of regressed formula for reflected impulse calculationi a b c d Ir-b 1.9833 −1.4980 0.0062 −2.2080 Ir-m/Ir-b 0.4690 0.9709 −0.0068 −1.3546 Ir-t/Ir-b 0.3440 0.7766 −0.0491 −0.5805 Ir-a/Ir-b 0.8792 0.4335 −0.0126 −1.4213 三折线模型在双折线模型的基础上增加转折点最大反射冲量Ir-a,考虑由地面反射冲击波导致的“针状峰值”分布。Ir-a的计算公式为:
Ir-a/Ir-b=a(R3√W)b+c(Hc3√W)d (4) 式(4)的使用范围为:0.4 m/kg1/3≤Z≤0.6 m/kg1/3, 0.1 m/kg1/3≤Hc/W1/3≤0.3 m/kg1/3;参数a、b、c和d的取值见表6,拟合精度如图10(d)所示,相关系数平方值为0.934。
在建立冲量分布的双折线和三折线模型后,任意高度位置的冲量值可根据线性插值获得。
3.2 冲量横向分布
由于柱子具有一定的截面宽度,还需要对沿宽度方向的荷载横向分布进行确认。以Z=0.4 m/kg1/3、Hc/W1/3=0.1 m/kg1/3工况为例,沿柱宽方向每隔20 mm布置测点,在不同柱高(h)处冲量沿柱横向分布如表7和图11所示。可见,在同一高度,距离柱中轴线不同距离的冲量变化小于7%,故近似均布分布。因此,为了便于工程设计,将冲量横向简化为均匀分布,如图11所示。
表 7 不同高度处最大反射冲量横向分布(Z=0.4m/kg1/3,Hc/W1/3=0.1m/kg1/3 )Table 7. Transverse distribution of the maximum impulse at different column heights (Z=0.4m/kg1/3,Hc/W1/3=0.1m/kg1/3 )h/mm Imid/(kPa∙s) Iedg/(kPa∙s) Iedg/Imid 200 6.54 6.06 0.93 700 2.25 2.11 0.94 1200 0.63 0.62 0.98 1700 0.35 0.35 0.99 2200 0.12 0.11 0.94 注:Imid为中轴线最大反射冲量,Iedg为柱边缘最大反射冲量。 3.3 正相超压持续时间
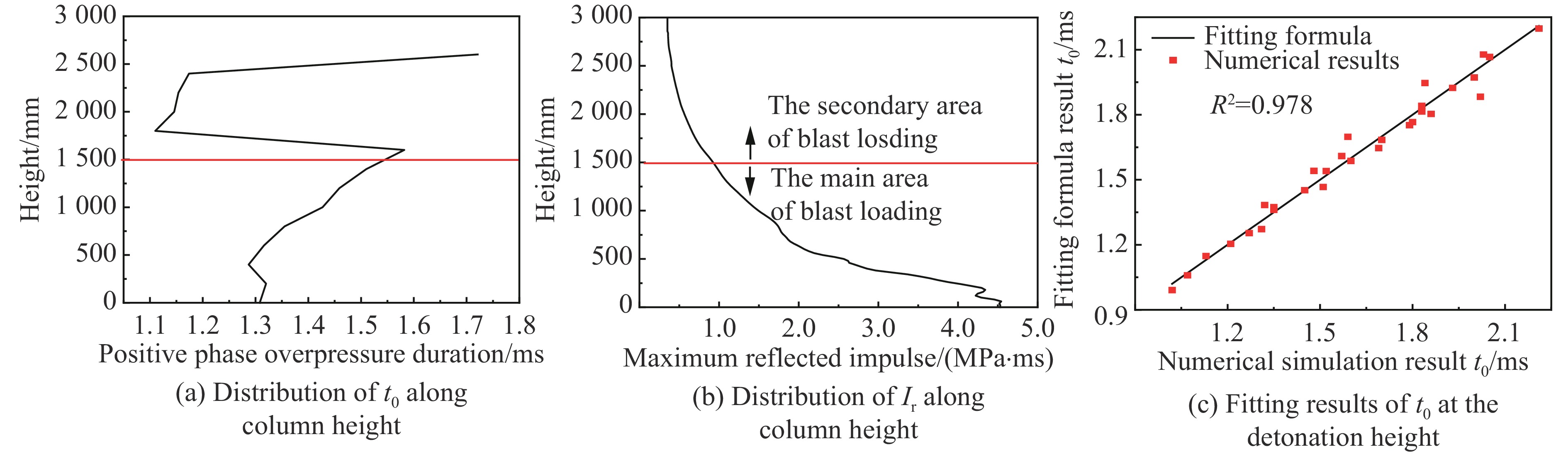
为确定三角形爆炸,除了冲量尚需超压或者正相超压持续时间t0,本文选择回归法确定t0。以Z=0.4 m/kg1/3, Hc/W1/3=0.1 m/kg1/3工况为例,研究t0沿柱高的分布特点。如图12(a)所示,t0随柱高度变化集中于1.1到1.7 ms之间,且在该区间内不具有规律性,这是因为地表反射波到达柱迎爆面的时间在不同柱高时存在差别。因此,难以精确拟合出各柱高位置的t0。同时由图12(b)可以看出,爆炸冲击波形成的冲量主要分布在柱下半段(h≤1500 mm),该部分冲量之和占总冲量的80%以上,故柱下半段为爆炸荷载分布的关键部分;而柱的上半段(h>1500 mm)可视为爆炸荷载的次要分布区域。故为了便于工程应用,忽略t0沿柱子高度的变化,取与爆心等高处的t0近似代表不同柱高位置的爆炸荷载作用时间。根据数值结果对t0进行拟合:
t0=μ[a(R3√W)b+c(Hc3√W)d] (5) 式中:μ为修正系数。式(5)的适用范围为:0.4 m/kg1/3≤Z≤1.4 m/kg1/3, Hc/W1/3≤0.5 m/kg1/3。a、b、c、d为拟合参数,取值分别为1.5047、0.4968、1.1968、1.5119。拟合结果如图12(c)所示,可见拟合公式计算值精度较高。
图13为Z=0.4 m/kg1/3, Hc/W1/3=0.1 m/kg1/3工况下不同柱高处的超压时程曲线,可见:在柱高较小处,爆炸入射波与地表正反射波同时到达,超压时程曲线为单峰值;随着柱高的增加,地面反射波到达时刻逐渐滞后,使超压时程曲线出现双峰值;在柱顶高度附近,地面反射波甚至在入射波进入负压区后才到达。当地面反射波到达时,原本已经衰减的超压再次上升,从而使t0数值偏大,但此时作用在柱表面的反射超压(考虑入射波和地面反射波的叠加)已十分微弱,对结构的作用较小。为了使三角形荷载结果与实际超压时程曲线更加接近,基于多组模拟结果,式(5)修正系数μ的双折线和三折线模型取值分别为0.4和0.3。
3.4 炸药相似比
适用于自由场爆炸工况的Hopkinson-Cranz比例定律(又称立方根比例定律)可以在给定比例爆距的条件下得到爆炸荷载特征参数,但在近场近地爆炸工况下的适用性尚待验证。且基于比例爆距和比例爆高得到的柱迎爆面最大反射冲量沿柱高(h)的分布模型在炸药当量变化时将会失效。为此,本节定义炸药相似比(
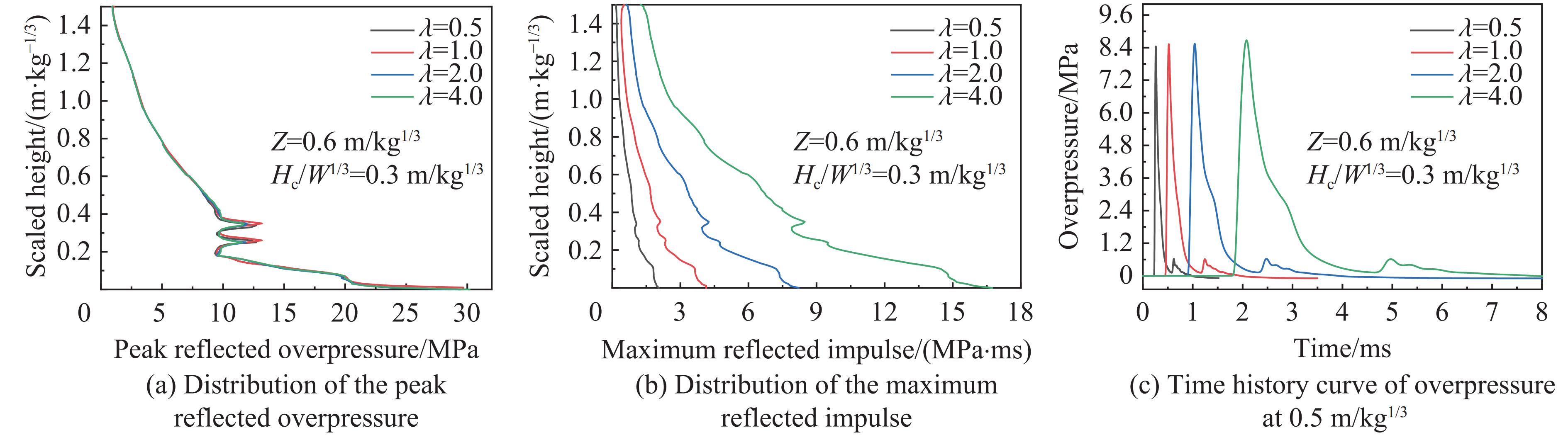
λ=W1/31/W1/32 )为球形TNT炸药当量立方根的比值,研究炸药当量对双折线和三折线冲量分布模型的影响。选取两组近场近地爆炸工况为例,保持比例爆距和比例爆高不变,以8 kg球形炸药近场近地爆炸工况为基准参照,对λ=0.5、1、2、4(对应TNT当量为1、8、64、512 kg)进行数值模拟计算,其中不同数值模型网格尺寸、测点间距、柱高等均按相似比进行等比例放缩。为了考虑炸药当量对爆炸荷载沿柱高分布的影响,引入比例高度(h/W1/3),数值模拟结果如图14和图15所示。由图14(a)和图15(a)可见,在相同比例高度处,不同炸药相似比条件下的反射超压峰值保持不变。由图14(b)和图15(b)可见,不同炸药相似比下的最大反射冲量随比例高度的分布规律保持一致,且在相同比例高度处最大反射冲量值是参照值的λ倍。对比图14(c)和图15(c)不同相似比但在同一比例高度处的超压时程曲线可以看出,超压到达时间和正相超压持续时间都与λ成正比,这些模拟结果表明了立方根比例定律在近场近地爆炸工况下的适用性。针对近场近地爆炸下简化的三角形爆炸荷载模型,为了考虑炸药相似比对最大反射冲量沿柱高分布的影响,对相同比例高度处正相超压持续时间采用t1:
t1=λt0 (6) 式(6)适用范围为近场近地爆炸工况且t1、t0为同一比例高度处的正相超压持续时间,即
h1/W1/31=h/W1/3 (W1、h1、t1分别为任意工况的炸药当量、柱迎爆面高度和正相超压作用时间;W=8 kg;h与Ir分别为式(3)和式(4)的柱迎爆面高度与冲量计算结果。3.5 等效三角荷载模型计算流程
任意近场近地工况下柱迎爆面等效的三角荷载模型简化步骤:
(1) 根据工况具体参数确定炸药当量W、爆炸距离R、起爆高度Hc,并求出中间参量比例爆距Z和比例爆高Hc/W1/3,确定该近场近地爆炸工况是否在本文所考虑的范围之内;
(2) 使用式(3)和(4)计算Ir的分布,其中任意高度下最大反射冲量由插值法得出;使用式(5)计算正相超压持续时间t0,然后采用式(6)考虑炸药相似比λ的影响求出t1。由pr=2Ir/ t1求出反射超压pr,得到等效三角形爆炸荷载。
3.6 荷载模型结果验证
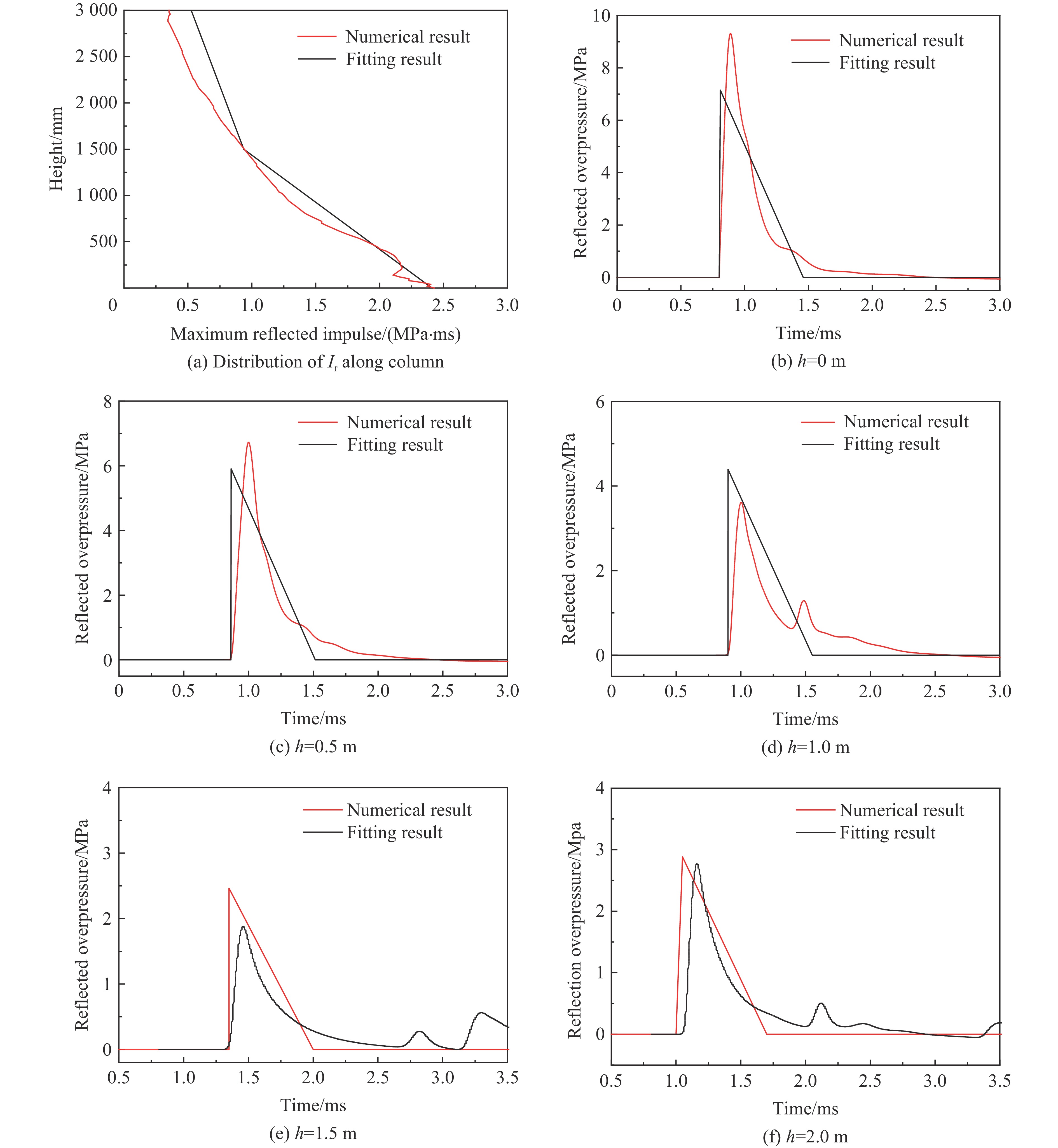
为了验证本文三角形荷载模型对爆炸荷载简化的合理性,现任取双折线模型适用范围内的工况W=8.0 kg, R=1.8 m, Hc=0.6 m(Z=0.9 m/kg1/3, Hc/W1/3=0.3 m/kg1/3)和三折线模型适用范围内的Woodson Test 3[18]工况W=9.585 kg, R=1.07 m, Hc=0.229 m(Z=0.504 m/kg1/3, Hc/W1/3=0.108 m/kg1/3),比较双折线模型和三折线模型简化得到的爆炸荷载结果与ALE模拟结果,如图16~17所示。由图16(a)、图17(a)可见本文提出的荷载模型得到的最大反射冲量沿柱高分布形态与数值结果以及Woodson实验数据接近,沿柱高各点荷载模型与模拟得到的最大冲量值误差在30%以内,如表8所示,且由图16和图17(b)~17(e)可以看出,任意高度下简化的三角荷载超压时程曲线与模拟吻合良好。因此可认为本文荷载模型可以应用于近场近地爆炸下柱的工程抗爆设计。
表 8 双折线与三折线模型冲量误差比较Table 8. Comparison of fitting results and numerical predictionsh/m 冲量/(kPa·s) 误差/% 双折线 三折线 模拟值 公式计算值 模拟值 公式计算值 双折线 三折线 0 2.52 2.41 7.09 6.37 −4.37 −10.16 0.5 1.90 1.91 3.16 2.27 0.52 −28.17 1.0 1.25 1.43 1.60 1.46 14.40 −8.75 1.5 0.94 0.94 0.88 0.66 0.00 −25.00 2.0 0.68 0.8 0.54 0.49 17.65 −9.26 平均误差 5.64 −16.27 4. 结 论
针对近场近地爆炸工况下建筑柱迎爆面爆炸荷载的分布规律展开了系统的数值研究,考虑地面反射的影响,对不同比例爆距(Z)、比例爆高(Hc/W1/3)和炸药当量下的爆炸荷载冲量分布和正相超压持续时间进行了回归分析和讨论,获得了适用于近场近地工况下(0.4 m/kg1/3≤Z≤0.6 m/kg1/3, 0.1 m/kg1/3≤Hc/W1/3≤0.3 m/kg1/3;0.6 m/kg1/3<Z≤1.4 m/kg1/3, 0.1 m/kg1/3≤Hc/W1/3≤0.5 m/kg1/3)柱迎爆面简化的三角形荷载模型。具体结论如下:
(1) 当比例爆高小于0.3 m/kg1/3,比例爆距在0.4~0.6 m/kg1/3范围时,冲量沿柱高可简化为三折线分布;当比例爆距在0.6~1.4 m/kg1/3范围时,冲量沿柱高可近似简化为双折线分布;基于上述规律通过回归分析获得了柱迎爆面的反射冲量分布计算公式;
(2) 由于地面反射波的存在,沿柱高正相超压持续时间分布具有很大的离散性,故使用与爆心等高处的正相超压持续时间作为代表值,拟合出正相超压持续时间的计算公式;
(3) 在同一比例爆距和比例爆高工况下,柱迎爆面相同比例高度处反射超压峰值保持不变,反射冲量最大值和正向超压持续时间正比于炸药当量的立方根;
(4) 基于冲量分布的双折线和三折线模型,提出了近场近地爆炸下建筑柱迎爆面爆炸荷载的简化模型,可用于近场近地爆炸工况下的建筑柱抗爆设计。
-
工况 结构 mC4/kg mTNT/kg R/m Z/(m·kg−3) 实验1 无填充墙 7.1 9.6 1.52 0.72 实验2 无填充墙 7.1 9.6 1.07 0.50 实验3 全填充墙 7.1 9.6 1.07 0.50 实验4 含窗填充墙 7.1 9.6 1.07 0.50 实验5 车库 7.1 9.6 1.07 0.50 注:m为质量,C4是实验所使用炸药,模拟使用TNT炸药,当量转化系数取1.35;Z为比例爆距;R为爆距。 工况 TNT/kg R/m Hc/m Z/(m·kg−3) Rso/m 1 1 3 1.05 3.00 2 2 2 3 1.05 2.38 2 3 4 2 1.05 1.89 2 注:R为炸药中心到柱等高位置处的爆距;Hc为爆心距地表高度;Rso为自由场超压传感器布置距离;Z为比例爆距。 表 3 Woodson实验[18]爆炸荷载的模拟结果与实测值对比
Table 3. Comparisons of numerical values and Woodson’s experimental[18] results
工况 测点 pr/MPa Ir/(kPa∙s) 误差/% 工况 测点 pr/MPa Ir/(kPa∙s) 误差/% 实测[18] 模拟 实测[18] 模拟 pr Ir 实测[18] 模拟 实测[18] 模拟 pr Ir 实验1 BC1 8.32 12.17 1.04 1.10 46.27 5.77 实验3 BC1 15.05 16.61 3.02 3.43 10.37 13.58 BC2 0.40 0.56 0.24 0.19 40.00 −20.83 BC2 BC3 9.36 6.23 1.22 1.02 −33.44 −16.39 BC3 9.57 15.67 1.71 2.28 63.74 33.33 实验2 BC1 11.52 12.53 2.77 2.38 8.77 −14.08 实验5 BC1 11.52 12.53 2.33 2.38 8.77 2.15 BC2 0.66 0.47 −28.79 BC2 0.66 0.47 −28.79 BC3 10.41 15.23 1.23 1.67 46.30 35.77 BC3 10.41 15.23 1.48 1.67 46.30 12.84 注:pr为超压,Ir为冲量;所有超压和冲量模拟误差的算术平均值为16.32%和5.79%。 表 4 Liu实验[19]超压模拟结果与实测值对比
Table 4. Comparison of numerical predictions and Liu’s experimental[19] results
测点 Case 1超压/MPa 误差/% 测点 Case 2超压/MPa 误差/% 测点 Case 3超压/MPa 误差/% 实测 模拟 实测 模拟 实测 模拟 so 0.21 0.12 −42.86 ry2 0.31 0.23 −25.81 ry2 0.52 0.57 9.62 ry3 0.14 0.15 7.14 ry3 0.43 0.21 −51.16 ry3 0.77 0.84 9.09 rb3 0.06 0.03 −50.00 ry4 0.39 0.23 −41.03 ry4 0.50 0.57 14.00 rb3 0.08 0.04 −50.00 so 0.74 0.82 10.81 平均 −28.57 −42.00 10.88 注:三个工况超压模拟误差的算术平均值为−19.90% 表 5 反射冲量沿柱高分布特征及简化模型
Table 5. Distribution characteristics and simplified models of reflected impulse along column length
爆心高度(Hc/W1/3) 分布特征及简化模型 小比例爆距(0.4≤Z/(m∙kg−1/3)≤0.6) 大比例爆距(0.6<Z/(m∙kg−1/3)≤1.4) 小比例爆高(≤0.3 m/kg1/3) “1/x函数形分布”,可简化为三折线模型 柱底“针状峰值”分布,可简化为双折线模型 大比例爆高(>0.3 m/kg1/3) “局部峰值”归为局部突变分布,建议通过数值方法确定荷载 线性分布,可简化为双折线模型 表 6 反射冲量拟合计算公式的参数取值
Table 6. Coefficients of regressed formula for reflected impulse calculation
i a b c d Ir-b 1.9833 −1.4980 0.0062 −2.2080 Ir-m/Ir-b 0.4690 0.9709 −0.0068 −1.3546 Ir-t/Ir-b 0.3440 0.7766 −0.0491 −0.5805 Ir-a/Ir-b 0.8792 0.4335 −0.0126 −1.4213 表 7 不同高度处最大反射冲量横向分布(
Z=0.4m/kg1/3,Hc/W1/3=0.1m/kg1/3 )Table 7. Transverse distribution of the maximum impulse at different column heights (
Z=0.4m/kg1/3,Hc/W1/3=0.1m/kg1/3 )h/mm Imid/(kPa∙s) Iedg/(kPa∙s) Iedg/Imid 200 6.54 6.06 0.93 700 2.25 2.11 0.94 1200 0.63 0.62 0.98 1700 0.35 0.35 0.99 2200 0.12 0.11 0.94 注:Imid为中轴线最大反射冲量,Iedg为柱边缘最大反射冲量。 表 8 双折线与三折线模型冲量误差比较
Table 8. Comparison of fitting results and numerical predictions
h/m 冲量/(kPa·s) 误差/% 双折线 三折线 模拟值 公式计算值 模拟值 公式计算值 双折线 三折线 0 2.52 2.41 7.09 6.37 −4.37 −10.16 0.5 1.90 1.91 3.16 2.27 0.52 −28.17 1.0 1.25 1.43 1.60 1.46 14.40 −8.75 1.5 0.94 0.94 0.88 0.66 0.00 −25.00 2.0 0.68 0.8 0.54 0.49 17.65 −9.26 平均误差 5.64 −16.27 -
[1] YU J, RINDER T, STOLZ A, et al. Dynamic progressive collapse of an RC assemblage induced by contact detonation [J]. Journal of Structural Engineering, 2014, 140(6): 04014014. DOI: 10.1061/(ASCE)ST.1943-541X.0000959. [2] SHI Y C, LI Z X, HAO H. A new method for progressive collapse analysis of RC frames under blast loading [J]. Engineering Structures, 2010, 32(6): 1691–1703. DOI: 10.1016/j.engstruct.2010.02.017. [3] LI J, HAO H. Numerical study of structural progressive collapse using substructure technique [J]. Engineering Structures, 2013, 52: 101–113. DOI: 10.1016/j.engstruct.2013.02.016. [4] BRODE H L. Blast wave from a spherical charge [J]. The Physics of Fluids, 1959, 2(2): 217–229. DOI: 10.1063/1.1705911. [5] BAKER W E. Explosions in air [M]. Austin, Texas: University of Texas Press, 1973: 6–10. [6] HENRYCH J. The dynamics of explosion and its use [M]. Amsterdam: Elsevier Scientific, 1979: 161–164. [7] Department of Defense (DOD). UFC 3-340-02 Structures to resist the effects of accidental explosions [S]. Washington: Department of Defense, 2008. [8] 汪维, 刘光昆, 赵强, 等. 近爆作用下方形板表面爆炸载荷分布函数研究 [J]. 中国科学: 物理学 力学 天文学, 2020, 50(2): 024615. DOI: 10.1360/SSPMA-2019-0188.WANG W, LIU G K, ZHAO Q, et al. Study on load distributing function of square slab surface under close-in blast loading [J]. Scientia Sinica: Physica, Mechanica & Astronomica, 2020, 50(2): 024615. DOI: 10.1360/SSPMA-2019-0188. [9] WU C Q, HAO H. Modeling of simultaneous ground shock and airblast pressure on nearby structures from surface explosions [J]. International Journal of Impact Engineering, 2005, 31(6): 699–717. DOI: 10.1016/j.ijimpeng.2004.03.002. [10] XIAO W, ANDRAE M, GEBBEKEN N. Development of a new empirical formula for prediction of triple point path [J]. Shock Waves, 2020, 30(6): 677–686. DOI: 10.1007/s00193-020-00968-7. [11] CUI J, SHI Y C, LI Z X, et al. Failure analysis and damage assessment of RC columns under close-in explosions [J]. Journal of Performance of Constructed Facilities, 2015, 29(5): B4015003. DOI: 10.1061/(ASCE)CF.1943-5509.0000766. [12] 闫秋实, 杜修力. 典型地铁车站柱在爆炸荷载作用下损伤评估方法研究 [J]. 振动与冲击, 2017, 36(1): 1–7. DOI: 10.13465/j.cnki.jvs.2017.01.001.YAN Q S, DU X L. Damage evaluation for a column of a typical subway station subjectedto internal blast loading [J]. Journal of Vibration and Shock, 2017, 36(1): 1–7. DOI: 10.13465/j.cnki.jvs.2017.01.001. [13] CHEN L, HU Y, REN H Q, et al. Performances of the RC column under close-in explosion induced by the double-end-initiation explosive cylinder [J]. International Journal of Impact Engineering, 2019, 132: 103326. DOI: 10.1016/j.ijimpeng.2019.103326. [14] DUA A, BRAIMAH A, KUMAR M. Experimental and numerical investigation of rectangular reinforced concrete columns under contact explosion effects [J]. Engineering Structures, 2020, 205: 109891. DOI: 10.1016/j.engstruct.2019.109891. [15] YU J, YU X F, TANG J H, et al. Local damage of precast concrete columns with grout sleeve connections under contact detonation [J]. Engineering Structures, 2022, 265: 114499. DOI: 10.1016/j.engstruct.2022.114499. [16] HU Y, CHEN L, FANG Q, et al. Blast loading model of the RC column under close-in explosion induced by the double-end-initiation explosive cylinder [J]. Engineering Structures, 2018, 175: 304–321. DOI: 10.1016/j.engstruct.2018.08.013. [17] 彭玉林, 吴昊, 方秦. 爆炸荷载在圆截面桥梁墩柱上的分布规律 [J]. 爆炸与冲击, 2019, 39(12): 122201. DOI: 10.11883/bzycj-2018-0317.PENG Y L, WU H, FANG Q. Blast loading distributions on the circular sectional bridge columns [J]. Explosion and Shock Waves, 2019, 39(12): 122201. DOI: 10.11883/bzycj-2018-0317. [18] WOODSON S C, BAYLOT J T. Structural collapse: quarter-scale model experiments [R]: Vicksburg: Engineer Research and Development Center, US Army Corps of Engineers, 1999. [19] LIU L, MA Z J, ZONG Z H, et al. Blast response and damage mechanism of prefabricated segmental RC bridge piers [J]. Journal of Bridge Engineering, 2021, 26(4): 04021012. DOI: 10.1061/(ASCE)BE.1943-5592.0001698. [20] 胡志乐, 马亮亮, 吴昊, 等. 远距离近地面爆炸空气冲击波计算的网格尺寸优化与验证 [J]. 爆炸与冲击, 2022, 42(11): 114201. DOI: 10.11883/bzycj-2021-0499.HU Z L, MA L L, WU H, et al. Optimization and verification of mesh size for air shock wave from large distance and near ground explosion [J]. Explosion and Shock Waves, 2022, 42(11): 114201. DOI: 10.11883/bzycj-2021-0499. 期刊类型引用(3)
1. 李浩浩, 翟红波, 唐泓. 爆炸冲击波作用下钢筋混凝土构件的毁伤效应研究进展. 兵器装备工程学报. 2025(06)  百度学术
百度学术2. 徐训, 徐波, 董舒娅, 边兴, 刘元明. 爆炸作用下RC剪力墙毁伤效应及评估研究. 武汉理工大学学报. 2025(05)  百度学术
百度学术3. 朱宏伟,李邦杰,马峰,庞哲楠. 内爆荷载下框架建筑的毁伤效应. 火箭军工程大学学报. 2025(01): 97-105 .  百度学术
百度学术其他类型引用(4)
-








 下载:
下载:


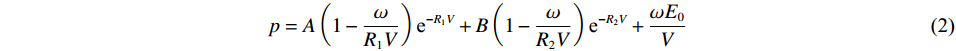







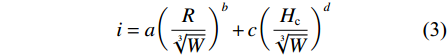


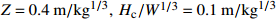



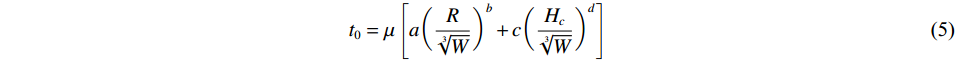




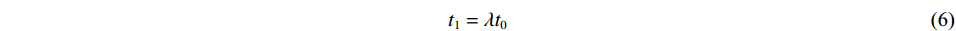
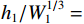



 下载:
下载:


















 百度学术
百度学术