Uncertainty quantification of magnetically driven quasi-isentropic compression experiments based on the Monte Carlo method
-
摘要: 磁驱动准等熵压缩实验是研究材料偏离Hugoniot状态高压物性和动力学行为的重要实验技术之一，开展不确定量化评估具有重要意义和价值。基于Monte Carlo原理，结合磁驱动准等熵压缩实验过程分析、Lagrange分析和特征线正向数据处理方法建立了适用于此类实验的Monte Carlo不确定度量化评估方法，实现利用磁驱动准等熵压缩实验获取材料声速、应力、应变等物理量以及状态方程和本构关系等物理模型的不确定度量化评估。利用建立的不确定度评估方法，对文献中已开展的钽、铜和NiTi合金的磁驱动准等熵压缩实验结果进行不确定度量化评估与分析。结果表明，基于本文中方法的评估结果与国外文献以相同原理得到的评估结果一致。对基于CQ-4装置开展的NiTi合金磁驱动准等熵压缩实验的评估结果表明，设计的磁驱动准等熵压缩实验是一种可靠的精密物理实验。在此基础上，深入讨论了磁驱动准等熵压缩实验的误差相关性和敏感性。结果表明：台阶样品厚度和粒子速度的测量是影响实验精度的主要因素。
-
关键词:
- 磁驱动准等熵压缩 /
- 不确定度 /
- Monte Carlo方法 /
- 量化评估
Abstract: Magnetically driven quasi-isentropic compression is one of the important experimental techniques to study high-pressure physics and dynamic behaviors of materials under off Hugoniot states. It is of great significance to carry out quantitative evaluation of experimental uncertainty. By combining with the process analysis of magnetically driven quasi-isentropic compression experiments and two forward data processing methods, an uncertainty quantitative evaluation method was established for such experiments based on the Monte Carlo method (MCM). The uncertainty quantification evaluations of physical quantities such as sound speed, stress, strain, and the parameters of equations of state and constitutive models were realized. Compared with the conventional method such as guide to the expression of uncertainty in measurement (GUM), the MCM uncertainty evaluation is more applicable to the cases in which the probability distribution of the input quantities is non-symmetric and the measurement model is non-linear. In fact, the uncertainty evaluation results obtained by the MCM is reasonable and not the ones under the maximum estimation condition. Employing the law of large numbers, nested cycle setting of the probability density functions (PDF) and nested loop construction of virtual samples makes the uncertainty evaluation results more accurate. By using the established MCM uncertainty evaluation method, the uncertainty evaluations of the experimental results of tantalum and copper samples under magnetically-driven quasi-isentropic compression were quantitatively analyzed firstly. The results are consistent with the data proposed in the literatures, which proves the correctness and reliability of our method. And then, the quantitative evaluation was conducted on magnetically-driven quasi-isentropic compression experiments of NiTi alloy carried out on a CQ-4 device. The results show that the experiments on the CQ-4 device are reliable and precise for high-pressure physics and material dynamics studies. Finally, the error correlation and sensitivity of magnetically-driven quasi-isentropic compression experiments were discussed in depth, and the results show that the measurements of step sample thickness and particle velocity are the main factors affecting the experimental accuracy but have different influence weights. This work has important guiding significance for studying high-pressure physics and dynamic behaviors of materials by using magnetically-driven quasi-isentropic compression experimental technology. -
旋转爆轰发动机（rotating detonation engine, RDE）是利用环形燃烧室内连续旋转传播的一道或者多道爆轰波扫过推进剂混合物、生成的高温高压爆轰产物从喷管排出从而产生推力的新型推进装置。燃料通过环形面的微型喷嘴喷注到燃烧室内，使用火花塞或其他起爆装置进行起爆，形成燃烧波沿环形燃烧室周向传播，逐渐发展成为爆轰波在燃烧室内连续旋转传播。由于旋转爆轰发动机具有结构简单、推重比大、热循环效率高等优点，近年来已成为研究热点。液态燃料与气态燃料相比具有更高的密度比冲，在相同体积的情况下能产生更大的冲量，因此，随着对旋转爆轰发动机的深入研究以及工程应用的需求，研究采用液态燃料组织燃烧的旋转爆轰成为了关注的重点。
Bykovskii等[1-6]对以煤油/液氧、煤油/富氧空气、煤油/氧气、柴油/富氧空气以及汽油/富氧空气为推进剂的两相连续旋转爆轰做了大量工作。首先，Bykovskii等[1]在外径204 mm、出口直径50 mm的圆盘型燃烧室内开展了煤油/富氧空气、煤油/空气以及柴油/空气的旋转爆轰实验，成功获得了连续旋转传播的爆轰波。Bykovskii等[2]采用丙烷/空气以及液态煤油/空气，在直径306 mm的环形燃烧室内进行了实验，发现这两种推进剂活性较低，以空气作为氧化剂无法起爆，只有在推进剂中加入氧气（氧气与氮气质量比为1）才能获得连续传播的旋转爆轰波。当富氧空气流量为1.75 kg/s、丙烷流量为0.24 kg/s、当量比为1时，获得的爆轰波传播速度为1.6 km/s；在富氧空气流量为6.3 kg/s、煤油流量为0.9 kg/s、当量比为0.96时，爆轰波传播速度为1.5 km/s。随后，Bykovskii等[3]针对煤油/液氧开展了旋转爆轰实验，采用速度补偿的方法通过观察窗对燃烧室内流场进行了拍摄，得到了两相连续旋转爆轰波的结构特征。在外径40～100 mm、宽度10～15 mm的燃烧室内，以氧气为氧化剂，推进剂混合物质量流量为0.1～2.9 kg/s，化学当量比0.8～1.7，实现了多种液体燃料的两相旋转爆轰波的稳定传播，爆轰波传播速度为1.1～2 km/s。在点火方面，Bykovskii等[4]通过实验验证了旋转爆轰波在煤油/空气以及汽油/空气混合物中连续稳定传播的可能性，实验结果表明爆轰燃烧室直径必须大于306 mm，且氧化剂气体中氧气与氮气的质量比大于0.41。此外，Bykovskii等[5-6]采用航空煤油、空气以及氢气或者合成气（CO/H2）进行了旋转爆轰实验，燃烧室外径、宽度和长度分别为503、18和490 mm，随着煤油、空气和氢气流量的改变，爆轰波传播模态从单波模态切换到五波模态，爆轰波速度分布在1.15～1.67 km/s，频率范围为0.73～4.86 kHz，实验证明能否维持煤油燃料的旋转爆轰状态是由混合气中的氢含量决定的。当燃料中氢气的质量分数达到42%，得到的最大比冲值约为4 000 s。Frolov等[7]采用氢气、液态丙烷和空气三组元推进剂在大尺寸环形燃烧室内进行了旋转爆轰实验，其中燃烧室外径为406 mm、宽度为25 mm，氢气、丙烷和空气流量范围分别为0.1～0.5、0.1～0.5和5～12 kg/s，在氢气空气混合物实现自持传播的旋转爆轰后加入液态丙烷，获得了连续旋转传播的两相旋转爆轰。
Kindracki等[8]对煤油和空气喷入环形燃烧室的雾化流场进行了实验研究，测量了空气的速度分布以及煤油液滴的直径。随后，Kindracki等[9]开展了煤油氧气混合物的起爆特性实验，研究了不同管径、点火能量以及氧化剂中不同氧含量条件下爆轰波能否直接起爆。Kindracki等[10]采用煤油、氢气和空气三组元推进剂开展了旋转爆轰实验，燃烧室外径和长度分别为168和120 mm，氢气、煤油和空气流量范围分别为1～10、7.45～16.5和160～550 g/s。他们研究了燃料中氢含量对燃烧室压力以及爆轰波传播速度的影响，在燃料中氢气占比57%时，爆轰波传播速度为1 550 m/s，实验结果表明非均相混合物的旋转爆轰有20%～25%的速度亏损。此外，还将液态硝酸异丙酯加入煤油中，并对其提高煤油空气混合物爆轰敏感性的影响进行了考察。
以上大多采用富氧空气或氧气作为氧化剂或者在液体燃料中加入氢气，以此提高反应物的活性，获得连续传播的两相旋转爆轰波。目前，在我国对于旋转爆轰发动机的实验研究大多采用气态燃料，Liu等[11]、Xie等[12]、Lin等[13]、魏万里等[14]和Deng等[15]对采用氢气、甲烷、乙烯以及乙炔为燃料的气相旋转爆轰开展了大量的实验研究，并对爆轰波起爆、传播模态与控制、速度亏损以及推力性能等开展了深入研究，而对液体燃料组织燃烧的两相旋转爆轰发动机研究较少。
郑权等[16-17]开展了汽油/富氧空气的旋转爆轰实验，成功起爆并实现了旋转爆轰波的自持传播，发现了单波头、双波头和多波头同时存在的混合传播模态，且旋转爆轰波传播速度存在亏损。在一定范围，旋转爆轰波的传播速度和压力随推进剂质量流量的增大而增大。此外，在富氧空气流量为945.3 g/s、汽油流量为84.3 g/s、当量比为0.82时，爆轰波传播模态为双波对撞，平均传播速度为1 051 m/s，有效推力为607.3 N，燃料比冲为735.1 s。实验发现，双波对撞模态下发动机推力波动较大，推力曲线围绕平均推力振荡。
李宝星等[18]开展了燃料/氧化剂分别为煤油/富氧空气的两相旋转爆轰实验研究，常温下成功实现了旋转爆轰的起爆与自持传播。研究发现，混合物的反应活性至关重要，当氧化剂中氧含量偏低时，旋转爆轰波无法起爆，直至氧含量增加到39.2%时，才能形成自持传播的爆轰波；爆轰波成功起爆后均以双波对撞模态传播，平均传播速度偏低；在贫油状态下，爆轰波传播速度随当量比提高而增大；当空气质量流量大于822 g/s时，发动机基本以缓燃形式工作。
王迪等[19]采用富氧空气或氧气为氧化剂对煤油两相旋转爆轰波的起爆和传播过程进行了研究。基于PDA方法对雾化流场进行了测量，发现随着煤油液滴受到气流剪切作用距离的增加，雾化细度和均匀度变好。当氧化剂为富氧空气时，燃烧室在单波模态下工作，爆轰波频率为0.904 kHz。在纯氧状态下，燃烧室在双波模态下工作，爆轰波传播频率为5.882 kHz。在实验范围内，随着氧化剂中含氧量的增加，爆轰波速度逐步增大。
综上所述，研究多以常温富氧空气或氧气作为氧化剂开展实验，对液体燃料与空气的两相旋转爆轰波的传播模态与特性研究较少。这主要是因为气液两相旋转爆轰实验存在几个难点：（1）液态燃料的破碎雾化问题，液态燃料破碎雾化后液滴颗粒直径直接影响到爆轰波的起爆特性以及传播特性；（2）液态燃料与氧化剂的掺混问题，掺混效果对爆轰波的传播特性以及稳定性影响较大；（3）相比于气态燃料，液态燃料组织燃烧的旋转爆轰需要更大的点火能量，才能成功激发连续旋转传播的爆轰波；（4）以常温空气作为氧化剂的油气混合物活性差，难以发展形成连续传播的旋转爆轰波。针对气液两相旋转爆轰的难点，本文中采用高总温空气与汽油液滴对撞喷注，在高总温空气的剪切力作用下，汽油液滴迅速破碎蒸发成气态，以此提高推进剂的掺混效果与活性；开展不同当量比下的两相旋转爆轰实验，分析爆轰波的传播模态、传播特性以及发动机的工作特性。
1. 实验系统
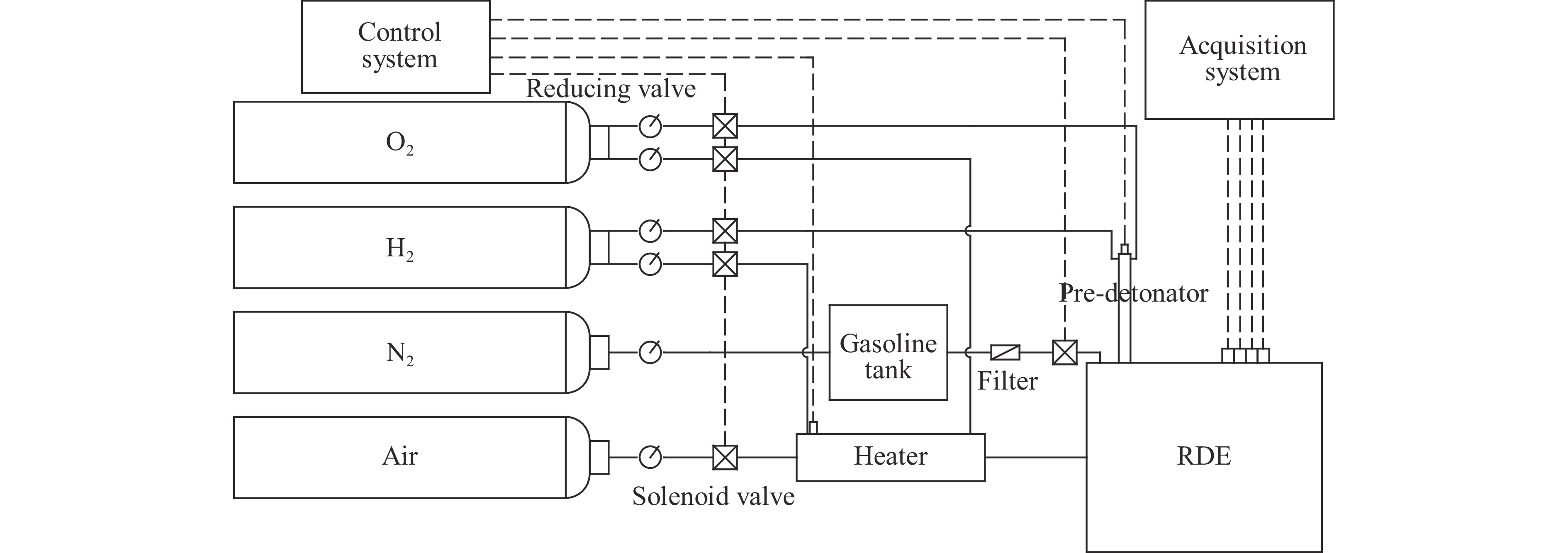
两相旋转爆轰实验系统主要由空气供给系统、加热器系统、燃油供给系统、两相旋转爆轰燃烧室、点火系统以及控制与采集系统组成，如图1所示。
1.1 推进剂供给系统
空气供给系统主要包括高压气源、球阀、过滤器、减压阀、流量计以及电磁阀，通过调节减压阀出口压力控制空气流量，流量设计范围为0.5～2 kg/s。汽油存储在高压油罐中，在高压氮气的挤压作用下，汽油依次通过过滤器、球阀、液体涡轮流量计、单向阀以及电磁阀，最后通过周向均匀分布的高压雾化喷嘴喷注进入燃烧室，以此提高雾化效果，冷流状态下雾化后的最小液滴粒径可达到20 μm。实验中，通过控制高压油罐内的压力达到控制汽油流量的目的，汽油流量最大可达到200 g/s。空气和汽油流量由流量计进行监测，通断时间通过电磁阀进行控制。
1.2 加热器系统
加热器系统由高压氢气罐、高压氧气罐、球阀、减压阀、限流喉道、电磁阀、单向阀以及点火装置组成。加热器的燃料和氧化剂分别为氢气和空气，实验过程中采用火花塞放电对冷流空气和氢气预混气进行点火，加热冷流空气至高总温，通过在加热器出口补充氧气保持高温空气中的氧含量不变。加热器具有稳定可靠、加热速度快等优点，最大可加热质量流量2 kg/s的空气至1 000 K。
1.3 两相旋转爆轰燃烧室
图2是两相旋转爆轰发动机实物，其中爆轰燃烧室采用一端封闭一端开口的等直圆环形结构，燃烧室外径和内径分别为202和166 mm，环形通道宽度为18 mm，轴向长度为155 mm。空气与汽油采用环缝与喷嘴对撞喷注的方式进行混合，其中汽油通过周向均匀分布的36个高压微细雾化喷嘴沿轴向喷注，而空气则通过收缩扩张型环缝进入燃烧室，如图3(a)所示。汽油和空气的流量通过安装在管路中的流量计进行测量，实验中的全局当量比采用管路中的汽油与氧气质量流量进行计算。
图 3 喷注结构以及传感器分布Figure 3. Schematic diagram of injection configuration and sensor instrumentation主要通过测量的压力信号对旋转爆轰波的传播特性进行分析，燃烧室外壳测量孔位置如图3所示。空气管路与汽油集油腔分别安装有扩散硅式压力变送器P-air和P-fuel，测量空气以及集油腔内的平均压力，如图2所示。此压力变送器具有响应时间快、测量精度高的优点。燃烧室外壁面分别安装有4个高频动态压力传感器和1个扩散硅式压力变送器，用来测量燃烧室内高频瞬态压力以及燃烧室的平均压力。高频动态压力传感器和扩散硅式压力变送器均采用平齐安装的方式，以减小对爆轰波面的干扰。高频压力传感器P1、P2和P3在相同周向位置，分别位于空气环缝下游8、24和40 mm处，扩散硅式压力变送器P-c距环缝24 mm，其中P4与P2在相同轴向位置，周向间隔90°，P-c与P4轴对称分布。
1.4 点火系统
利用垂直安装的预爆轰管对汽油空气混合物进行起爆，预爆轰管内径为20 mm，长度为600 mm，内充一定浓度的H2/O2预混气，以达到足够的起爆能量。点火时刻选择为汽油集油腔和空气管路压力处于稳定的区间。为了减少填充阶段燃烧室对预爆轰管内混合气体的影响，预爆轰管出口与燃烧室连接处采用薄膜隔离，点火位置如图3所示。实验环境温度为293 K，燃烧室出口直接与大气相通，环境压力为1个标准大气压。
1.5 控制与采集系统
采用自主开发的时序控制程序实现电磁阀的通断控制和火花塞状态的控制，其中时序控制精度达毫秒级。高频数据采集系统由高频压力传感器及NI公司X系列多功能高频压力采集模块组成，其中NI高频数据采集系统采用NI-STC3定时同步与USB总线技术，数据采集卡型号为USB-4716，共有8通道同步模拟信号输入，单通道采样频率高达2×106 s−1，ADC位数为16位，能够捕捉瞬态的旋转爆轰波传播信号。
图4为两相RDE点火实验工作时序，其中蓝色箭头向上表示打开，红色箭头向下表示关闭。实验过程中，先打开空气与加热器氢氧管路的电磁阀，随后开启采集系统，几十毫秒后使用火花塞对加热器进行点火，高总温空气进入燃烧室作为氧化剂。空气流量稳定后，打开汽油管路的电磁阀，同时向预爆轰管内喷注氢气和氧气，当集油腔压力稳定后点火，预爆轰管内形成的初始爆轰波进入燃烧室引燃油气混合物。Δt为两相旋转爆轰发动机的工作时间，实验结束停止喷注汽油以及加热器的氢气和氧气，通过持续喷注一段时间的空气实现发动机的熄火与冷却。为保证传感器与发动机的使用寿命，实验中发动机工作时间设置为1 s。
2. 结果与讨论
在两相旋转爆轰发动机实验过程中，固定空气质量流量以及总温，通过改变汽油质量流量，在各种工况下进行了热试实验，研究了推进剂当量比对两相RDE内旋转爆轰波传播模态以及特性的影响。
表1为实验工况表，固定空气质量流率为1 110 g/s以及空气总温为713 K，研究两相旋转爆轰发动机的当量比工作范围。在当量比0.79～1.25范围，燃烧室内均得到了连续自持传播的旋转爆轰波，传播模态为双波对撞和单波共存的混合模态以及单波模态，爆轰波传播频率范围为1.6～1.9 kHz；降低当量比至0.61～0.66，爆轰波传播稳定性变差，表现为间断爆轰以及零星爆轰；进一步降低当量比至0.53，爆轰波起爆失败。随着推进剂当量比的提高，爆轰波的传播频率先增大后减小，在当量比1.19附近达到最大。在空气质量流量为1 110.0 g/s、汽油质量流量为85.7 g/s、空气总温为713 K、当量比为1.19的工况下，燃烧室内获得了连续稳定自持传播的旋转爆轰波，爆轰波传播频率为1 900.9 Hz，传播模态为单波爆轰，此工况为最佳工况。
表 1 实验工况Table 1. Experimental conditions工况 当量比 空气总温/K 空气流量/（g·s−1） 汽油流量/（g·s−1） 传播频率/Hz 传播模态 1 0.53 713.0 1 110.0 38.4 — 起爆失败 2 0.61 713.0 1 110.0 44.2 1 452.0 零星爆轰 3 0.66 713.0 1 110.0 47.9 1 521.7 间断爆轰 4 0.79 713.0 1 110.0 57.3 1 613.7 混合模态 5 0.84 713.0 1 110.0 62.4 1 818.2 混合模态 6 0.97 713.0 1 110.0 70.3 1 827.3 混合模态 7 1.06 713.0 1 110.0 76.3 1 849.1 单波 8 1.19 713.0 1 110.0 85.7 1 900.9 单波 9 1.25 713.0 1 110.0 90.6 1 878.4 单波 2.1 发动机工作过程
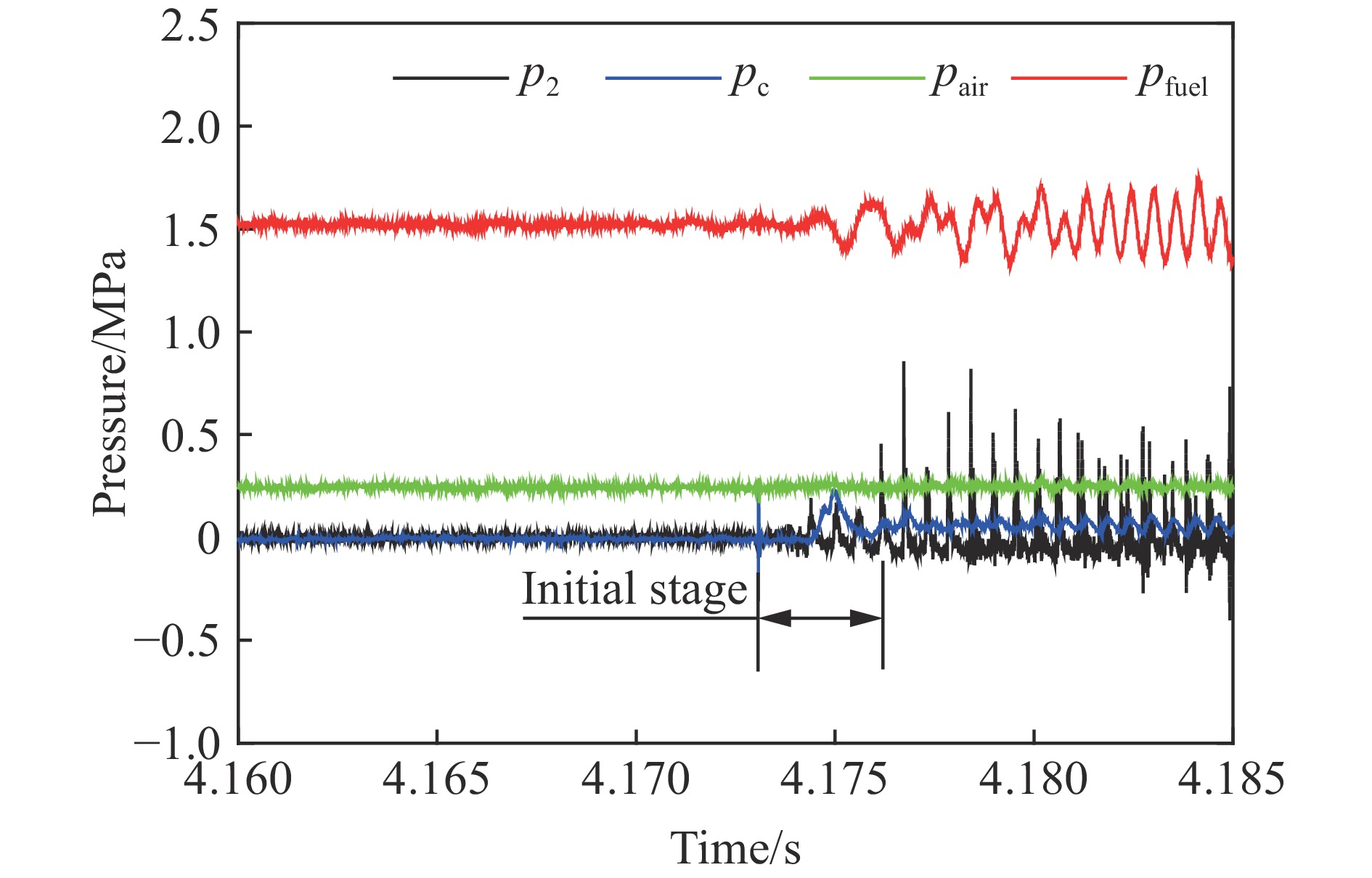
当汽油质量流量为62.4 g/s、空气质量流量为1 110.0 g/s、推进剂当量比为0.84时，加热空气总温至713 K（即工况5），对两相旋转爆轰发动机的工作过程进行研究，该工况下发动机成功起爆。在实验过程中，由于高频压力传感器受到高温爆轰燃烧产物的影响，测得的高频压力原始信号出现温度漂移现象。为便于分析爆轰波的传播过程，对高频原始压力信号进行了高通滤波处理，处理后的高频压力曲线p2如图5所示，图中pc、pair以及pfuel分别是燃烧室、空气喷注以及集油腔内的平均压力。由图可见，P2处的高频周期性压力跃升信号表明燃烧室内成功获得了连续自持传播的旋转爆轰波，在发动机整个工作过程中，高频压力信号较稳定，没有出现大幅度的爆轰波熄灭现象，爆轰波压力峰值存在一定的波动。
图6为点火初期燃烧室内压力信号。冷流空气通过加热器加热后进入环形燃烧室，打开汽油管路电磁阀，集油腔压力逐渐上升，当空气喷注压力以及集油腔压力达到稳定状态后，采用填充一定浓度氢氧预混气的预爆轰管进行点火，预爆轰管内形成的初始爆轰波击穿薄膜进入环形燃烧室引燃汽油空气混合物。燃烧室稳压传感器在4 173.096 ms时采集第1个压力尖峰，压力峰值约为0.22 MPa，为初始爆轰波进入环形燃烧室引起。初始爆轰波进入燃烧室后引燃汽油空气混合物，压力信号出现波动，但并没有直接形成爆轰波，而是经过约3.06 ms后，高频压力传感器采集到了第1个压力跃升信号，压力峰值约为0.46 MPa。这主要是因为实验采用垂直安装的预爆轰管作为点火装置，初始爆轰波进入环形燃烧室后发生衍射衰退为缓燃波，沿燃烧室周向相反方向传播，随后在燃烧室曲率、推进剂喷注以及边界层发展等湍流作用下，火焰燃烧锋面加速与前导激波耦合，最终在4 176.156 ms时形成连续旋转传播的爆轰波。
在5 114.812 ms时，关闭汽油管路电磁阀，集油腔压力逐渐降低，而此时空气管路压力基本不变，致使波前汽油空气混合物当量比下降，爆轰波强度逐渐降低，表现为爆轰波压力峰值的持续衰减，如图7所示。关闭汽油管路电磁阀并未导致爆轰波的瞬时熄灭或解耦，而是逐渐衰减至熄灭。主要原因是，汽油管路以及集油腔内剩余的汽油维持爆轰波继续传播了一段时间，这说明旋转爆轰波具有自适应当量比变化的能力。由图可见，在约5 189.456 ms时，剩余的汽油耗尽，发动机熄火，持续通冷流空气进行爆轰燃烧产物吹除以及发动机冷却。
2.2 爆轰波传播模态
当汽油质量流量为57.3 g/s、空气质量流量为1 110.0 g/s、推进剂当量比为0.79（即工况4）时，旋转爆轰波的传播模态为单波与双波对撞的混合模态。图8为P2、P4处的高频压力信号的局部放大图，爆轰波压力峰值较低，且存在较大波动。由图可见，在4 325.38～4 336.0 ms时，P2、P4处的每个压力尖峰基本重合，对比传感器的位置（见图3），判断环形燃烧室内存在两道传播方向相反的爆轰波，周期性发生对撞，此时爆轰波传播模态为双波对撞，且对撞点在P2和P4之间。进一步发现，在每个双波对撞周期内，第1个压力峰值远低于第2个压力峰值。这主要是因为，第1个压力尖峰是由爆轰波解耦后的透射激波引起的，压力峰值较低。由于燃烧室宽度远大于空气喷注环缝，燃烧室头部存在回流区吸附爆轰燃烧产物，两道相向传播的透射激波在P2和P4间碰撞后，在新鲜油气混合物以及局部热点的支持下，透射激波与火焰锋面耦合迅速发展形成爆轰波，表现为第2个较高的压力峰值。重新形成的两道爆轰波继续向前传播，在另一个相位点发生碰撞后透射，透射激波在没有新鲜油气混合物的支持下，旋转爆轰波发生解耦，周而复始，形成了双波对撞周期内压力峰值强弱交替的现象。
图 8 工况4双波对撞点位于P2和P4之间的局部压力分布Figure 8. Local pressure distributions of two-wave collision point locating between P2 and P4 in case 4在4 503.128～4 510.272 ms时，对高频压力信号进行分析，发现在爆轰波传播过程中双波对撞点存在偏移的现象，如图9所示。这可能是因为，在爆轰波双波对撞过程中，每个对撞周期后燃烧室内的局部压力以及透射激波的强度存在差异，进而影响了汽油空气的喷注状态以及掺混效果，导致新鲜油气混合层高度以及活性存在空间差异，因此爆轰波双波对撞点产生了偏移。在4 511.236 ms时，双波对撞模态衰减为单道爆轰波，这主要是因为上个周期P2处压力过高，抑制了新鲜空气和汽油的喷注以及掺混过程，导致P2处积累的预混反应物层高度较低。当顺时针（定义从燃烧室出口观察）传播的透射激波再次传播到P2处，由于可燃气体层高度以及活性较低，透射激波没有得到增强，难以发展形成新的爆轰波，因此燃烧室内仅存在一道逆时针传播的爆轰波。但单波模态维持时间较短，随着爆轰产物膨胀排出燃烧室，P2处的压力低于空气喷注总压，空气恢复喷注，前导激波在新鲜油气混合物以及局部热点的支持下发展形成新的爆轰波，爆轰波传播模态恢复为双波对撞。
图 9 工况4模态转变过程中的局部压力分布Figure 9. Local pressure distributions during mode transition in case 4进一步提高推进剂当量比至1.19，此时汽油质量流量为85.7 g/s、空气质量流量为1 110.0 g/s、空气总温为713 K（即工况8），对旋转爆轰波的传播模态进行分析。图10为P2、P4处的高频压力信号的局部放大图，压力信号出现周期性陡升骤降趋势，表明燃烧室内存在连续旋转传播的爆轰波。传感器P2和P4相位差为90°，测量的相邻压力尖峰的时间差Δt2恰好为Δt1的3倍，因此推断爆轰波以单波模态在燃烧室内传播。根据传感器的位置分布，爆轰波传播方向为顺时针。此外，爆轰波压力尖峰后存在峰值较低的压力振荡（见图10中区域O），这是由于该工况处于富油状态，汽油中重组分含量较高。在高总温空气的剪切力作用下，汽油液滴中的轻组分以及部分重组分破碎蒸发形成气态汽油，爆轰波优先扫过气态汽油与空气的混合物，波后区域仍剩余部分汽油重组分小液滴以两相湍流燃烧方式与新鲜空气组织燃烧，因此形成了爆轰波后的低峰值压力振荡。
2.3 爆轰波传播特性
为进一步分析旋转爆轰波的传播特性，对工况4下P2处的高频压力信号进行频域与时域分析，分别采用快速傅里叶变换(FFT)和短时傅里叶变换(STFT)进行处理。通过FFT得到的功率谱密度随频率的分布如图11所示，FFT的旋转爆轰波传播频率振荡范围为1 544.0～1 663.7 Hz，功率谱密度最大的主频为1 613.7 Hz。FFT可以得到高频压力信号的振荡主频分布，但无法反映压力信号的时频特性，STFT结果反映了高频压力信号在不同时刻的振荡特性，如图12所示。由图可见，旋转爆轰波的平均传播频率约为1 625.6 Hz，在发动机工作过程中，旋转爆轰波的传播频率围绕平均频率振荡，振荡范围为1 543.7～1 651.4 Hz，与FFT得到的结果基本一致。关闭汽油管路电磁阀后，波前反应物当量比降低，爆轰波强度逐渐降低，导致爆轰波传播速度降低，传播频率降低（见图12～13中的熄火阶段）。
捕捉P2处的高频压力信号的尖峰时刻，对爆轰波每个传播周期内的传播速度进行计算，得到的瞬时传播速度随时间的分布如图13所示。由图可见，旋转爆轰波传播速度范围为897.4～989.6 m/s，平均传播频率为1 614.7 Hz，与FFT以及STFT得到的结果较吻合。
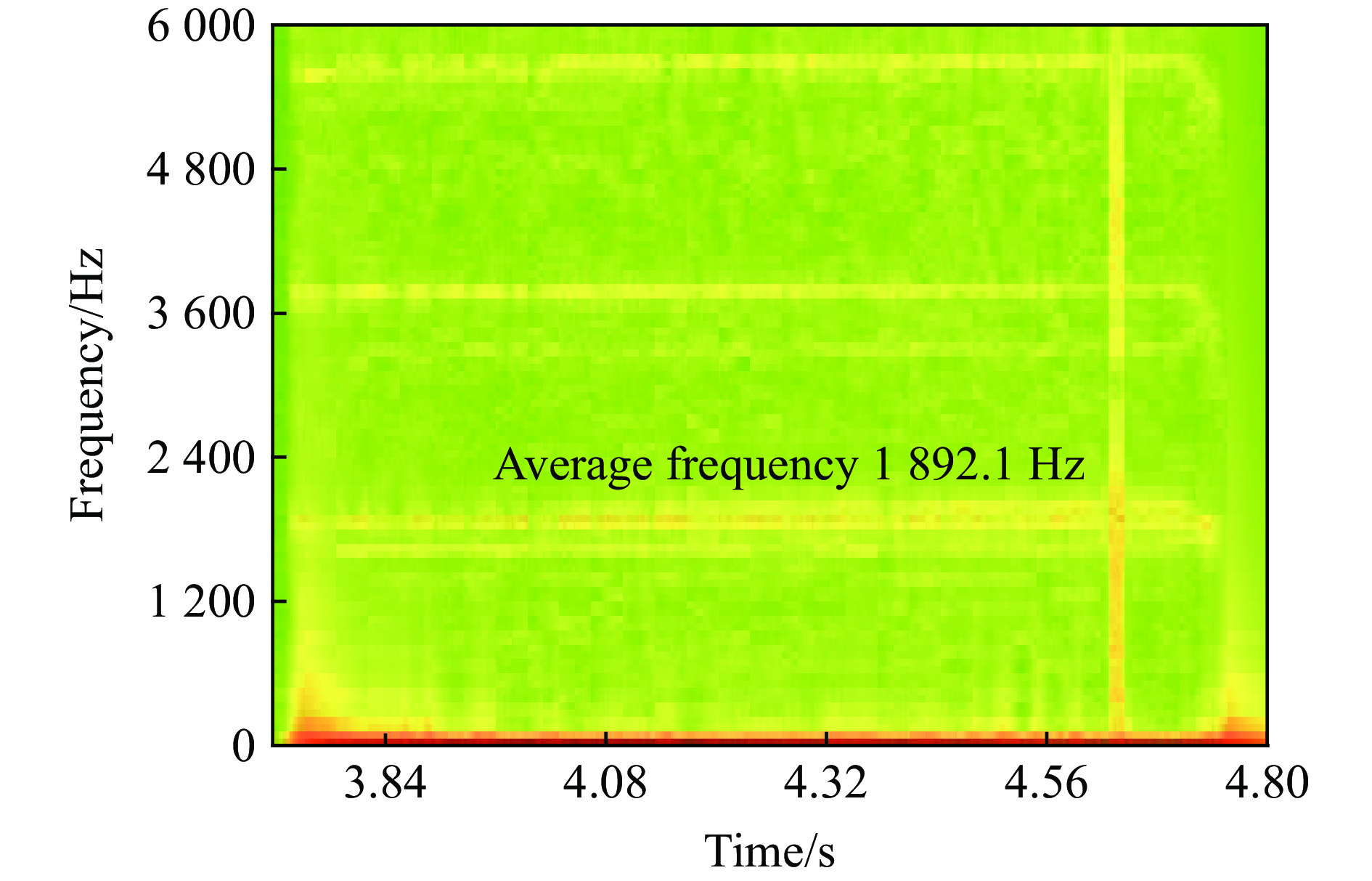
图14～15分别为工况8下P2处的高频压力信号的FFT和STFT结果。FFT结果显示，爆轰波振荡主频的分布范围为1 885.7～1 924.3 Hz，功率谱密度最大的主频为1 900.9 Hz。由此可见，工况8的爆轰波传播频率高于工况4的，且主频分布更加集中，说明工况8的爆轰波传播稳定性高于工况4的。STFT结果显示，在发动机工作前期，爆轰波传播频率较稳定，没有出现明显的频率振荡，爆轰波平均传播频率约为1 892.1 Hz。在发动机工作后期，爆轰波传播频率出现轻微上升的现象。其原因可能为，随着发动机工作时间的增加，燃烧室壁面温度上升，汽油液滴蒸发速率提高，燃料中气态汽油占比增加，推进剂混合物的活性提高，导致旋转爆轰波传播频率增加，但发动机工作时间较短，频率波动量较小。图16为爆轰波瞬时传播速度随时间的分布，旋转爆轰波的传播速度范围为1 054.6～1 155.9 m/s，基于平均传播速度计算得到的平均传播频率为1 891.5 Hz，与FFT以及STFT得到的结果吻合较好。该工况下CJ爆轰速度为1 817.2 m/s，受到燃烧室壁面曲率、燃料的蒸发与掺混、侧向膨胀等影响，爆轰波传播速度存在严重亏损。在发动机工作后期，爆轰波瞬时速度略微上升，与STFT结果中瞬时频率的变化趋势基本一致。
2.4 发动机工作特性
为研究当量比对两相旋转爆轰发动机工作特性的影响，通过固定空气质量流量以及空气总温，改变汽油质量流量，开展了各种工况的热试实验，得到了旋转爆轰波传播模态随当量比的变化（见表1）。当量比较低时，爆轰波传播稳定性较差，传播模态多表现为零星爆轰以及间断爆轰，降低当量比至0.53，发动机起爆失败。这主要是因为，低当量比条件下汽油质量流量低，从而可燃反应物中气态汽油浓度较低，且存在严重的空间分布不均匀性，导致爆轰波传播稳定性较差。当量比处于0.79～0.97时，爆轰波传播模态为双波对撞与单波的混合模态，进一步增加当量比至1.06～1.25的富油状态，爆轰波传播模态转变为单波模态。发生模态转变的原因可能为：双波对撞的关键条件之一是新鲜反应物层的快速建立，高当量比时爆轰波峰值压力较高，导致波后局部区域处于壅塞状态，抑制了新鲜反应物层的形成，因此爆轰波从混合模态转变为单波模态。
图17为燃烧室平均绝对压力以及爆轰波平均传播频率随当量比变化的曲线。从实验数据分布可见，燃烧室压力和爆轰波传播频率均随着当量比的增加呈现先增大后减小的趋势，极大值出现在当量比1.19附近，即为轻微富油时。这主要是因为：一方面，在高总温空气的剪切力作用下，仍剩余部分汽油重组分以小液滴形态存在，没有参与爆轰燃烧；另一方面，由于径向喷注的空气导致轴向喷注的高速雾化汽油颗粒之间碰撞聚合加剧，部分汽油颗粒吸附在燃烧室外壁面上，导致实际反应物当量比低于实验给定值，因此在当量比1.19时，实际参与反应的推进剂当量比接近理想化学当量比，实验结果最佳。
图 17 燃烧室绝对压力和爆轰波传播频率随当量比的分布Figure 17. Distributions of absolute pressure in combustor and propagation frequency of detonation wave with equivalent ratio3. 结 论
为了研究当量比对汽油燃料两相旋转爆轰发动机工作特性的影响，开展了高总温空气与汽油燃料的旋转爆轰实验研究，结论如下。
（1）当量比为0.61～1.25时，发动机成功起爆并实现了旋转爆轰波的自持传播，降低当量比至0.53，爆轰波起爆失败。
（2）随着当量比的增大，爆轰波传播稳定性提高，传播模态发生变化。当量比为0.61～0.66时，爆轰波传播稳定性较差，传播模态为零星爆轰或间断爆轰；提高当量比为0.79～0.97时，爆轰波以双波对撞与单波的混合模态在燃烧室内连续传播；当量比为1.06～1.25的轻微富油时，爆轰波传播模态从混合模态转变为较为稳定的单波模态。
（3）燃烧室平均绝对压力与爆轰波平均传播频率均随着当量比的增加呈现先增大后减小的趋势，极大值出现在当量比1.19附近。在此当量比时，获得了最佳实验结果，旋转爆轰波的平均传播频率为1 900.9 Hz，平均传播速度为1 110.8 m/s，爆轰波传播速度存在严重亏损。
（4）实验采用高温空气与汽油液滴对撞喷注，以此提高推进剂的掺混效果与活性，它对汽油雾化液滴细小程度与反应物混合均匀程度的影响有待进一步研究。
-
图 1 磁驱动准等熵压缩实验原理图
Figure 1. A principle of magnetically-driven quasi-isentropic compression experiment
图 2 基于Monte Carlo原理评价不确定度的主要阶段
Figure 2. The main stages of evaluation uncertainty based on the Monte Carlo method
图 3 磁驱动准等熵压缩实验的Monte Carlo不确定度评价流程
Figure 3. Monte Carlo uncertainty evaluation process for magnetically-driven quasi-isentropic compression experiments
图 9 自由面速度历史曲线的虚拟样本构造结果
Figure 9. Band construction results of virtual samples for free surface velocity profiles
图 12 应力应变的不确定度评价结果及其极大估计
Figure 12. Uncertainty evaluations and their great estimates of stress and strain
图 14 两种数据处理方法的不确定度评价结果比较
Figure 14. Comparison of uncertainty evaluation results by two kinds of data processing methods
图 16 自由面速度历史曲线基于时序误差和速度误差的模拟带
Figure 16. Simulation bands of free surface velocity histories based on time deviation and velocity deviation
图 17 插值前后变量的映射关系
Figure 17. Mapping relationships of variables before and after interpolation
图 20 输出量误差与输入量误差的敏感系数
Figure 20. Sensitivity coefficients of the output deviation and input deviation
表 1 分布形式设置
Table 1. Settings of distribution form
设定形式名称 时间分布形式 自由面速度分布形式 A 正态分布 正态分布 B 正态分布 伽马分布 C 均匀分布 正态分布 D 均匀分布 伽马分布 
下载: 导出CSV
-
[1] SINARS D B, SWEENEY M A, ALEXANDER C S, et al. Review of pulsed power-driven high energy density physics research on Z at Sandia [J]. Physics of Plasmas, 27(7):, 2020, 27(7): 070501. DOI: 10.1063/5.0007476. [2] 王桂吉, 罗斌强, 陈学秒, 等. 磁驱动平面准等熵加载装置, 实验技术及应用研究新进展 [J]. 爆炸与冲击, 2021, 41(12): 62–83. DOI: 10.11883/bzycj-2021-0119.WANG G J, LUO B Q, CHEN X M, et al. Recent progress on the experimental facilities, techniques and applications of magnetically driven quasi-isentropic compression [J]. Explosion and Shock Waves, 2021, 41(12): 62–83. DOI: 10.11883/bzycj-2021-0119. [3] 孙承纬, 赵剑衡, 王桂吉, 等. 磁驱动准等熵平面压缩和超高速飞片发射实验技术原理、装置及应用 [J]. 力学进展, 2012, 42(2): 206–219. DOI: 10.6052/1000-0992-2012-2-20120208.SUN C W, ZHAO J H, WANG G J, et al. Progress in magnetic loading techniques for isentropic compression experiments and ultra-high velocity flyer launching [J]. Advances in Mechanics, 2012, 42(2): 206–219. DOI: 10.6052/1000-0992-2012-2-20120208. [4] DAVIS J P, KNUDSON M D , SHULENBURGER L, et al. Mechanical and optical response of [100] lithium fluoride to multi-megabar dynamic pressures [J]. Journal of Applied Physics, 2016, 120(16): 165901. DOI: 10.1063/1.4965869. [5] DAVIS J P, BROWN J L, KNUDSON M D, et al. Analysis of shockless dynamic compression data on solids to multi-megabar pressures: application to tantalum [J]. Journal of Applied Physics, 2014, 116(20): 2734–2224. DOI: 10.1063/1.4902863. [6] DAVIS J P. Experimental measurement of the principal isentrope for aluminum 6061-T6 to 240 GPa [J]. Journal of Applied Physics, 2006, 99(10): 782. DOI: 10.1063/1.2196110. [7] HALL C A, ASAY J R, KNUDSON M D, et al. Experimental configuration for isentropic compression of solids using pulsed magnetic loading [J]. Review of Scientific Instruments, 2001, 72(9): 3587–3595. DOI: 10.1063/1.1394178. [8] SEAGLE C T, DAVIS J P, MD KNUDSON. Mechanical response of lithium fluoride under off-principal dynamic shock-ramp loading [J]. Journal of Applied Physics, 2016, 120(16): 033515–984. DOI: 10.1063/1.4965990. [9] 罗斌强, 张红平, 种涛, 等. 磁驱动斜波压缩实验结果的不确定度分析 [J]. 高压物理学报, 2017, 31(3): 295–300. DOI: 10.11858/gywlxb.2017.03.011.LUO B Q, ZHANG H P, CHONG T, et al. Experimental uncertainty analysis of magnetically driven ramp wave compression [J]. Chinese Journal of High Pressure Physics, 2017, 31(3): 295–300. DOI: 10.11858/gywlxb.2017.03.011. [10] 张红平, 罗斌强, 王桂吉, 等. 基于特征线反演的斜波加载实验数据处理与分析 [J]. 高压物理学报, 2016, 30(2): 7. DOI: 10.11858/gywlxb.2016.02.006.ZHANG H P, LUO B Q, WANG G J, et al. Inverse characteristic analysis of ramp loading experiments [J]. Chinese Journal of High Pressure Physics, 2016, 30(2): 7. DOI: 10.11858/gywlxb.2016.02.006. [11] BROWN J L, ALEXANDER C S, ASAY J R, et al. Extracting strength from high pressure ramp-release experiments [J]. Journal of Applied Physics, 2013, 114(22): 223518–223516. DOI: 10.1063/1.4847535. [12] WANG G J, LUO B Q, ZHANG X P, et al. A 4 MA, 500 ns pulsed power generator CQ-4 for characterization of material behaviors under ramp wave loading [J]. The Review of Scientific Instruments, 2013, 84(1): 015117. DOI: 10.1063/1.4788935. [13] 经福谦. 动高压原理与技术 [M]. 2版. 北京: 科学出版社, 1999: 222–223. [14] SONG H, WU X, HUANG C, et al. Measurement of fast-changing low velocities by photonic Doppler velocimetry [J]. The Review of Scientific Instruments, 2012, 83(7): 073301. DOI: 10.1063/1.4731014. [15] 马云, 胡绍楼, 汪小松, 等. 样品-窗口界面运动速度的VISAR测试技术 [J]. 高压物理学报, 2003, 17(4): 290–294. DOI: 10.3969/j.issn.1000-5773.2003.04.008.MA Y, HU S L, WANG X S, et al. VISAR measurement on interface velocity between shocked specimen and window [J]. Chinese Journal of High Pressure Physics, 2003, 17(4): 290–294. DOI: 10.3969/j.issn.1000-5773.2003.04.008. [16] 罗斌强, 张红平, 赵剑衡, 等. 斜波压缩实验数据的正向Lagrange处理方法研究 [J]. 爆炸与冲击, 2017, 37(2): 243–248. DOI: 10.11883/1001-1455(2017)02-0243-06.LUO B Q, ZHANG H P, ZHAO J H, et al. Lagrangian forward analysis in data processing of ramp wave compression experiments [J]. Explosion And Shock Waves, 2017, 37(2): 243–248. DOI: 10.11883/1001-1455(2017)02-0243-06. [17] LUO B Q, JIN Y S, LI M, et al. Direct calculation of sound speed of materials under ramp wave compression [J]. AIP Advances, 2018, 8(11). DOI: 10.1063/1.5047479. [18] 张红平, 孙承纬, 李牧, 等. 准等熵实验数据处理的反积分方法研究 [J]. 力学学报, 2011, 43(1): 105–111. DOI: 10.6052/0459-1879-2011-1-lxxb2010-053.ZHANG H P, SUN C W, LI M, et al. Backward integration method in data processing of quasi-isentropic compression experiment [J]. Chinese Journal of Theoretical Applied Mechanics, 2011, 43(1): 105–111. DOI: 10.6052/0459-1879-2011-1-lxxb2010-053. [19] HAYES D B. Backward integration of the equations of motion to correct for free surface perturbations: AC04-94AL85000 [R]. Office of Scientific & Technical Information Technical Reports, 2001. DOI: 10.2172/783087. [20] 许淑艳. 蒙特卡罗方法在实验核物理中的应用 [M]. 2版. 北京: 原子能出版社, 2006: 3. [21] 国家质量监督检验检疫总局. 用蒙特卡洛法评定测量不确定度: JJF 1059.2—2012 [S]. 北京: 中国质检出版社, 2013. [22] 国家市场监督管理总局, 中国国家标准化管理委员会. 测量不确定度评定和表示补充文件1: 基于蒙特卡洛方法的分布传播: GB/T 27419—2018 [S]. 北京: 中国标准出版社, 2018. [23] DENG J X, DENG Y, CHEONG K H. Combining conflicting evidence based on Pearson correlation coefficient and weighted graph [J]. International Journal of Intelligent Systems, 2021, 36(12): 7443–7460. DOI: 10.1002/int.22593. [24] BROWN J L, ALEXANDER C S, ASAY J R, et al. Flow strength of tantalum under ramp compression to 250GPa [J]. Journal of Applied Physics, 2014, 115(4): 10340–1177. DOI: 10.1063/1.4863463. [25] VALE C D, MAURELLI V A. Simulating multivariate nonnormal distributions [J]. Psychometrika, 1983, 48(3): 465–471. DOI: 10.1007/BF02293687. [26] KRAUS R G, DAVIS J P, SEAGLE C T, et al. Dynamic compression of copper to over 450 GPa: a high-pressure standard [J]. Physical Review B, 2016, 93(13): 134105. DOI: 10.1103/physrevb.93.134105. [27] ZHANG X P, WANG G J, LUO B Q, et al. Mechanical response of near-equiatomic NiTi alloy at dynamic high pressure and strain rate [J]. Journal of Alloys, 2017, 731: 569–576. DOI: 10.1016/j.jallcom.2017.10.080. 期刊类型引用(5)
1. 刘国秋，蔡承霖，孙宇航，郑权，翁春生. 基于弯型预爆管的煤油燃料旋转爆轰发动机起爆实验研究. 弹道学报. 2024(01): 26-34+56 . 
百度学术2. 舒晨，顾福涛，陈斌，晏成龙，仝毅恒，林伟. 煤油燃料宽域冲压旋转爆震燃烧特性. 气体物理. 2024(02): 21-32 . 百度学术3. 杨帆，姜春雪，王宇辉，李世全，王健平，张国庆. 煤油液滴直径对两相旋转爆轰发动机流场的影响. 爆炸与冲击. 2023(02): 3-17 . 
本站查看4. 刘思远，汪洋，李真珍，滕宏辉，田诚. 中心锥喷管喉道比参数对旋转爆轰燃烧影响的数值研究. 推进技术. 2023(09): 120-131 . 百度学术5. 吴敏宣，白桥栋，翁春生，孟豪龙，韩家祥，张世健，王研艳. C_2H_4/CH_4/H_2混合气旋转爆轰波传播特性数值模拟研究. 推进技术. 2022(11): 303-314 . 百度学术其他类型引用(3)
-
下载:
百度学术
计量
- 文章访问数: 518
- HTML全文浏览量: 151
- PDF下载量: 95
- 被引次数: 8