Prediction of natural gas explosion overpressure considering external turbulence
-
摘要: 为预先评估外加湍流工况下天然气的爆炸超压峰值，通过揭示外加湍流对天然气爆炸火焰形态、火焰前锋速度和爆炸超压的影响规律，建立了耦合外加湍流的天然气爆炸超压峰值预测模型。结果表明：外加湍流可使火焰加速传播，且随着外加湍流强度的增加，火焰前锋速度逐渐增加；随着外加湍流强度的增加，爆炸超压峰值和最大升压速率逐渐增加；随着压力监测点和点火位置间距的增加，爆炸超压峰值和最大升压速率整体呈减小的变化趋势。外加湍流工况下天然气的爆炸超压预测必须考虑火焰的加速特征，实验测得爆炸超压峰值介于层流火焰模型和湍流火焰模型计算的爆炸超压峰值之间。Abstract: Natural gas is an important energy material for national development, but its hazardous properties lead to frequent explosion accidents. In order to pre-evaluate the maximum overpressure of natural gas explosion under the condition with external turbulence, it is proposed to reveal the mechanism of accelerated propagation of turbulent flame, and to establish a prediction model of natural gas explosion overpressure coupled with external turbulence. To this end, an experimental platform for natural gas explosion under external turbulence was firstly established, and the particle image velocimetry system was used to obtain the intensity of external turbulence. Then, the effects of external turbulence with different turbulent intensities on the flame evolution, flame front velocity and explosion overpressure of natural gas explosion were obtained for the methane-air premixed gas with stoichiometric ratio. Finally, through introducing the folding factors of flame instability-induced folds, flame turbulence-induced folds and external turbulence-induced folds, a theoretical model of predicting maximum explosion overpressure of natural gas explosion by considering external turbulence is established. The results indicated that compared with the condition without external turbulence, the external turbulence can exacerbate the degree of flame surface folds. Without external turbulence, the flame radius increases linearly with time; in the presence of external turbulence, the flame is characterized by self-accelerating propagation. The flame acceleration can be triggered by external turbulence, with the increasing intensity of external turbulence, the flame front velocity increases gradually. Additionally, with the increasing intensity of external turbulence, maximum explosion overpressure and maximum rate of pressure rise continue to increase. With the increasing distance between pressure monitoring point and ignition position, maximum explosion overpressure and maximum rate of pressure rise totally decrease. The flame acceleration must be considered to predict natural gas explosion overpressure under external turbulence. Maximum explosion overpressure measured in the experiments is between the value calculated using laminar flame model and turbulent flame model.
-
天然气具有易燃易爆属性，若泄漏至大气环境，在环境风作用（湍流作用）下形成的可燃气云可能被意外点燃。为确保能源安全，需要加强天然气可燃气云爆炸的基础理论研究。
湍流火焰加速传播是一种瞬态的、高度非线性的、非定常的、耦合了湍流和燃烧反应的流体动力学现象，涉及湍流积分尺度、层流火焰厚度及Kolmogorov尺度等宽广尺度范围。相关研究表明[1-4]，各向同性湍流可显著影响火焰表面积和火焰内部热-质输运速率，增加湍流燃烧速率，进而引发湍流火焰加速传播。Bauwens等[5]研究了弱湍流对大尺度甲烷-空气火焰的影响规律，指出弱湍流对火焰初期传播行为几乎无影响，随着当量比的增加，湍流火焰表面出现褶皱的时间推迟，火焰传播速度伴有震荡加速现象。Lawes等[6]研究了湍流均方根速度和初始压力对湍流火焰传播速度的影响规律，强调随着湍流均方根速度和初始压力的增加，异辛烷-空气湍流火焰传播速度逐渐增大，且湍流火焰传播速度会随火焰半径的增长而增加。Wang等[7]研究了含氢量和初始压力对湍流火焰结构的影响规律,发现对于特定的湍流均方根速度，增加含氢量和初始压力，能够增强质热扩散不稳定和流体动力学不稳定，致使湍流火焰更加褶皱，进而增加湍流燃烧速度。Brequigny等[8]对比了层流燃烧速率相同，刘易斯数和马克斯坦长度不同的三种燃料的湍流火焰结构，结果表明，随着湍流强度的增加，火焰褶皱程度增大，当刘易斯数较高时，质热扩散不稳定和湍流相互竞争，致使火焰褶皱程度降低。Chaudhuri等[9]采用粒子示踪技术，对湍流火焰传播速度进行了定量分析，结果表明，随着湍流均方根速度的增加，小尺度涡团能量增强，促进火焰正向拉伸，进而引发火焰加速传播，且曲率拉伸对火焰作用时间是切向拉伸的两倍。在充分认识湍流火焰加速传播的基础之上，Kobayashi等[10-11]、Chaudhuri等[12]、Liu等[13]和Bradley等[14]建立了系列湍流燃烧速度模型用以研究湍流火焰加速过程。
目前研究重点集中于分析各向同性湍流对湍流火焰加速传播的影响规律，及建立湍流燃烧速率模型。外加湍流工况的火焰加速传播对爆炸超压形成的影响机理几乎未见报道。鉴于此，本文拟获取外加湍流对火焰形态的影响规律，揭示湍流火焰加速传播机理，建立耦合外加湍流的天然气爆炸超压预测模型。
1. 实验装置和实验方法
图1是外加湍流工况天然气爆炸实验平台。该实验平台主要包括立方体框架（0.3 m×0.3 m×0.3 m）、高速摄像机（帧率为10000 s−1）、质量流量计、自由场声压传感器、数据采集仪（每秒记录105个采样点）、湍流生成系统（标定三个风扇转速档位：TP3、TP6和TP9）、高压点火器及时序控制器等。湍流生成系统包含八个风扇、转速控制器、直流电机。风扇分别分布在立方体框架的八个对角，面向装置中心旋转，通过无刷式直流电机驱动，转速控制器可以精确地同步调整八个风扇的旋转速度。声压传感器和点火位置处于同一水平直线上，距离点火位置依次为0.4 m（#1）、0.8 m（#2）和1.2 m（#3）。高压点火器、高速摄像机、数据采集仪均由时序控制器进行控制。实验中天然气由体积分数99.999%的甲烷气体代替，与干燥空气在常温状态下以化学计量比混合。
图 1 外加湍流工况下天然气爆炸实验平台Figure 1. Experimental platform of natural gas explosion under external turbulence实验步骤为：(1) 封膜—采用聚乙烯薄膜密封立方体框架，以模拟开敞空间；(2) 配气—调节质量流量计至所需流量，持续向立方体框架内通入天然气和空气，逐渐置换密封框架内的空气；(3) 开启湍流生成系统，待转速稳定；(4) 点火记录—在立方体框架中心点燃可燃气云，时序控制器触发高压点火器点火，并同步触发高速摄像机和数据采集仪分别记录火焰形态演变图像和动态压力数值；(5) 关闭湍流生成系统。
为定量表征外加湍流，采用粒子图像测速系统获取外加湍流的瞬时速度。湍流均方根速度和湍流积分尺度的计算方法如下[15-16]：
urms=√N∑i=1(ui−um)2 (1) LI=0.07L (2) 式中：urms为湍流均方根速度，ui为第i个时刻的瞬时速度，um为平均速度，LI为湍流积分尺度，L为立方体框架的特征尺度。
经计算可知，随着湍流生成系统的档位由TP3增至TP9，湍流强度逐渐增加，TP3、TP6、TP9对应的湍流均方根速度分别为0.65、0.67和0.73 m/s，湍流积分尺度为21 mm。
2. 结果与讨论
图2给出了外加湍流对天然气爆炸火焰形态演变的影响规律（同列火焰等效半径相近）。未加外加湍流时（TP0），点火后火焰形态整体呈球形状态向外传播；存在外加湍流时（TP3、TP6、TP9），点火后火焰形态难以维持球形结构，火焰表面凹凸不平。对于特定外加湍流强度，随着时间发展，火焰表面褶皱程度逐渐增加；对于特定等效半径的火焰形态，随着外加湍流强度的增加，火焰表面褶皱程度逐渐增加。需要强调的是，凹凸不平的褶皱结构必然增加火焰燃烧表面积和增强燃烧速率，进而使得随着外加湍流强度的增加，相近火焰半径需要时间逐渐减小。
图 2 外加湍流对天然气爆炸火焰形态演变的影响规律Figure 2. Effects of external turbulence on flame evolution of natural gas explosion图3给出了外加湍流对天然气爆炸火焰半径和火焰前锋速度的影响规律，火焰半径由Photron FASTCAM Viewer软件通过LUT处理后测得，火焰前锋速度由火焰半径求导获取。层流火焰传播速度的理论计算方法如下[17-18]：
图 3 外加湍流对天然气爆炸火焰半径和火焰前锋速度的影响规律Figure 3. Effects of external turbulence on flame radius and flame front velocity of natural gas explosionVL=drdt=σSL (3) 式中：VL为层流火焰传播速度，r为火焰半径，t是时间，σ为热膨胀比，SL为层流燃烧速度。
如图3所示，未加外加湍流时（TP0），火焰半径随着时间变化而线性增加；存在外加湍流时（TP3、TP6、TP9），火焰半径与时间的变化关系偏离线性趋势，呈自加速特征。对于特定时间，随着外加湍流强度的增加，火焰半径逐渐增加。另外，未加外加湍流工况实验测量火焰前锋速度和层流火焰传播速度相近，而外加湍流工况实验测量火焰前锋速度明显高于层流火焰传播速度，且随着外加湍流强度的增加，火焰前锋速度逐渐增加。
通过以上分析可知，外加湍流会显著增加火焰传播速度，且随着外加湍流强度的增加，火焰前锋速度逐渐增加。为揭示湍流火焰加速传播机理，外加湍流实验的湍流燃烧分区详见图4，火焰厚度的计算方法如下[19]
δL = 2λρucpSL(TbTu)0.7 (4) 式中：δL为火焰厚度，λ为热导率，ρu为未燃气体密度，cp为定压比热容，Tb为燃烧产物温度，Tu为未燃气体温度。
湍流预混火焰根据湍流Karlovitz数Ka，将湍流火焰分为薄火焰面区、薄反应区和破碎/增厚反应区。如图4所示（Da为湍流Damkohler数），外加湍流实验的湍流燃烧分区属于薄火焰面区（Ka<1），即外加湍流只能使火焰面产生褶皱，未影响火焰面内部的温度和组分传输，火焰保持原有的层流火焰结构。火焰的宏观和微观传播满足质量守恒，湍流扰动使火焰锋面实际表面积远大于宏观表面积，因而观察所得的火焰传播速度明显大于层流燃烧速度，火焰出现加速现象。
图5是外加湍流对天然气爆炸超压峰值和最大升压速率的影响规律。对于特定的压力监测点，随着外加湍流强度的增加，爆炸超压峰值和最大升压速率逐渐增加；对于特定的外加湍流强度，随着压力监测点和点火位置间距的增加，爆炸超压峰值和最大升压速率整体呈减小的变化趋势。
图 5 外加湍流对天然气爆炸超压峰值和最大升压速率的影响规律Figure 5. Effects of external turbulence on the maximum explosion pressure and the maximum rate of pressure rise of natural gas explosion文献[20]表明，修正后的单极子声源理论可用于评估外加湍流工况气云爆炸超压，理论表达式如下：
pmax=2ρaird(σ−1)σS2LΞ2max3√3σV04π (5) 式中：pmax为爆炸超压峰值，ρair为空气密度，d为压力监测点和点火点之间的距离，Ξmax为火焰褶皱因子峰值，V0为立方体框架的体积。
无湍流扰动工况下，火焰受火焰不稳定影响失稳加速；湍流扰动工况下，火焰加速由火焰不稳定和湍流扰动共同作用[20]。对于外加湍流，火焰褶皱因子主要由3方面构成：火焰不稳定诱发火焰褶皱、火焰自身湍流诱发火焰褶皱和外加湍流诱发火焰褶皱，详细如下[20]：
Ξmax=Ξfi,max+Ξtd,max+Ξft,max (6) Ξfi,max=2.4649 (7) Ξtd,max=1+urmsSL (8) Ξft,max=σ−1√3 (9) 式中：Ξfi,max为火焰不稳定诱发火焰褶皱，Ξtd,max为外加湍流诱发火焰褶皱，Ξft,max为火焰自身湍流诱发火焰褶皱。
图6是耦合外加湍流的天然气爆炸超压峰值预测。对于层流火焰模型（计算爆炸超压峰值），火焰褶皱因子峰值Ξmax=1.0；对于湍流火焰模型（计算爆炸超压峰值），火焰褶皱因子峰值采用式(6)～(9)计算，由于引入的褶皱因子表示最大扰动程度，该模型的计算结果为极限值。层流火焰模型与湍流火焰模型的爆炸超压峰值理论计算值随着压力监测点距离增加而降低，这与实验结果的趋势一致。实验测得的爆炸超压峰值介于层流火焰模型与湍流火焰模型理论预测值之间，层流火焰模型忽略了外加湍流对火焰加速传播的影响，实验测量值高于层流火焰模型计算的爆炸超压峰值；湍流火焰模型高估火焰不稳定诱发火焰褶皱和火焰自身湍流诱发火焰褶皱，实验测量值低于湍流火焰模型计算的爆炸超压峰值。
图 6 耦合外加湍流的天然气爆炸超压峰值预测Figure 6. Theoretical prediction of the maximum explosion pressure considering external turbulence3. 结 论
为预先评估外加湍流工况天然气爆炸超压峰值，本文获取了外加湍流对火焰形态、火焰传播速度和爆炸超压的影响规律，建立了耦合外加湍流的天然气爆炸超压预测模型，结论如下：
(1) 与未加湍流工况相比，外加湍流可加剧火焰表面褶皱程度；未加外加湍流时，火焰半径随着时间变化而线性增加；存在外加湍流时，火焰呈自加速传播特征；随着外加湍流强度的增加，火焰前锋速度逐渐增加；
(2) 随着外加湍流强度的增加，爆炸超压峰值和最大升压速率逐渐增加；随着压力监测点和点火位置间距的增加，爆炸超压峰值和最大升压速率整体呈减小的变化趋势；
(3) 外加湍流工况天然气爆炸超压预测必须考虑火焰加速特征，实验测量爆炸超压峰值介于层流火焰模型和湍流火焰模型计算的爆炸超压峰值之间。
-
图 1 外加湍流工况下天然气爆炸实验平台
Figure 1. Experimental platform of natural gas explosion under external turbulence
图 2 外加湍流对天然气爆炸火焰形态演变的影响规律
Figure 2. Effects of external turbulence on flame evolution of natural gas explosion
图 3 外加湍流对天然气爆炸火焰半径和火焰前锋速度的影响规律
Figure 3. Effects of external turbulence on flame radius and flame front velocity of natural gas explosion
图 5 外加湍流对天然气爆炸超压峰值和最大升压速率的影响规律
Figure 5. Effects of external turbulence on the maximum explosion pressure and the maximum rate of pressure rise of natural gas explosion
-
[1] KAMINSKI C F, HULT J, ALDÉN M, et al. Spark ignition of turbulent methane/air mixtures revealed by time-resolved planar laser-induced fluorescence and direct numerical simulations [J]. Proceedings of the Combustion Institute, 2000, 28(1): 399–405. DOI: 10.1016/S0082-0784(00)80236-2. [2] VAN OIJEN J A, GROOT G R A, BASTIAANS R J M, et al. A flamelet analysis of the burning velocity of premixed turbulent expanding flames [J]. Proceedings of the Combustion Institute, 2005, 30(1): 657–664. DOI: 10.1016/j.proci.2004.08.159. [3] CAI X, WANG J H, BIAN Z J, et al. Self-similar propagation and turbulent burning velocity of CH4/H2/air expanding flames: effect of Lewis number [J]. Combustion and Flame, 2020, 212: 1–12. DOI: 10.1016/j.combustflame.2019.10.019. [4] FAIRWEATHER M, ORMSBY M P, SHEPPARD C G W, et al. Turbulent burning rates of methane and methane-hydrogen mixtures [J]. Combustion and Flame, 2009, 156(4): 780–790. DOI: 10.1016/j.combustflame.2009.02.001. [5] BAUWENS C R, BERGTHORSON J M, DOROFEEV S B. On the interaction of the Darrieus-Landau instability with weak initial turbulence [J]. Proceedings of the Combustion Institute, 2017, 36(2): 2815–2822. DOI: 10.1016/j.proci.2016.07.030. [6] LAWES M, ORMSBY M P, SHEPPARD C G W, et al. The turbulent burning velocity of iso-octane/air mixtures [J]. Combustion and Flame, 2012, 159(5): 1949–1959. DOI: 10.1016/j.combustflame.2011.12.023. [7] WANG J H, ZHANG M, XIE Y L, et al. Correlation of turbulent burning velocity for syngas/air mixtures at high pressure up to 1.0 MPa [J]. Experimental Thermal and Fluid Science, 2013, 50: 90–96. DOI: 10.1016/j.expthermflusci.2013.05.008. [8] BREQUIGNY P, HALTER F, MOUNAÏM-ROUSSELLE C. Lewis number and Markstein length effects on turbulent expanding flames in a spherical vessel [J]. Experimental Thermal and Fluid Science, 2016, 73: 33–41. DOI: 10.1016/j.expthermflusci.2015.08.021. [9] CHAUDHURI S, SAHA A, LAW C K. On flame-turbulence interaction in constant-pressure expanding flames [J]. Proceedings of the Combustion Institute, 2015, 35(2): 1331–1339. DOI: 10.1016/j.proci.2014.07.038. [10] KOBAYASHI H, TAMURA T, MARUTA K, et al. Burning velocity of turbulent premixed flames in a high-pressure environment [J]. Symposium (International) on Combustion, 1996, 26(1): 389–396. DOI: 10.1016/s0082-0784(96)80240-2. [11] KOBAYASHI H, SEYAMA K, HAGIWARA H, et al. Burning velocity correlation of methane/air turbulent premixed flames at high pressure and high temperature [J]. Proceedings of the Combustion Institute, 2005, 30(1): 827–834. DOI: 10.1016/j.proci.2004.08.098. [12] CHAUDHURI S, WU F J, ZHU D L, et al. Flame speed and self-similar propagation of expanding turbulent premixed flames [J]. Physical Review Letters, 2012, 108(4): 044503. DOI: 10.1103/PhysRevLett.108.044503. [13] LIU C C, SHY S S, PENG M W, et al. High-pressure burning velocities measurements for centrally-ignited premixed methane/air flames interacting with intense near-isotropic turbulence at constant Reynolds numbers [J]. Combustion and Flame, 2012, 159(8): 2608–2619. DOI: 10.1016/j.combustflame.2012.04.006. [14] BRADLEY D, LAU A K C, LAWES M, et al. Flame stretch rate as a determinant of turbulent burning velocity [J]. Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences, 1992, 338(1650): 359–387. DOI: 10.1098/rsta.1992.0012. [15] GALMICHE B, MAZELLIER N, HALTER F, et al. Turbulence characterization of a high-pressure high-temperature fan-stirred combustion vessel using LDV, PIV and TR-PIV measurements [J]. Experiments in Fluids, 2014, 55(1): 1636. DOI: 10.1007/s00348-013-1636-x. [16] KUMAR V. FLUENT 6.3 user’s guide [S]. New York: Fluent Inc, 2006. [17] KIM W K, MOGI T, DOBASHI R. Flame acceleration in unconfined hydrogen/air deflagrations using infrared photography [J]. Journal of Loss Prevention in the Process Industries, 2013, 26(6): 1501–1505. DOI: 10.1016/j.jlp.2013.09.009. [18] LI Y C, BI M S, ZHOU Y H, et al. Experimental and theoretical evaluation of hydrogen cloud explosion with built-in obstacles [J]. International Journal of Hydrogen Energy, 2020, 45(51): 28007–28018. DOI: 10.1016/j.ijhydene.2020.07.067. [19] XIAO H H, HE X C, DUAN Q L, et al. An investigation of premixed flame propagation in a closed combustion duct with a 90° bend [J]. Applied Energy, 2014, 134: 248–256. DOI: 10.1016/j.apenergy.2014.07.071. [20] JIANG Y T, LI Y C, ZHOU Y H, et al. Investigation on unconfined hydrogen cloud explosion with external turbulence [J]. International Journal of Hydrogen Energy, 2022, 47(13): 8658–8670. DOI: 10.1016/j.ijhydene.2021.12.167. 期刊类型引用(1)
1. 张丽，康青春，杨克，纪虹，方奇明. 爆炸过程中预混气体流动状态对甲烷爆炸影响分析. 消防科学与技术. 2025(01): 35-40 . 
百度学术其他类型引用(1)
-
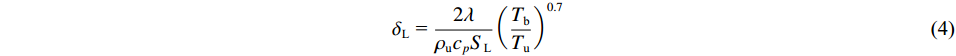
下载:
下载:
百度学术
计量
- 文章访问数: 214
- HTML全文浏览量: 80
- PDF下载量: 51
- 被引次数: 2