Blast-induced damage characteristics and fracture mechanism of rock mass under initial stress
-
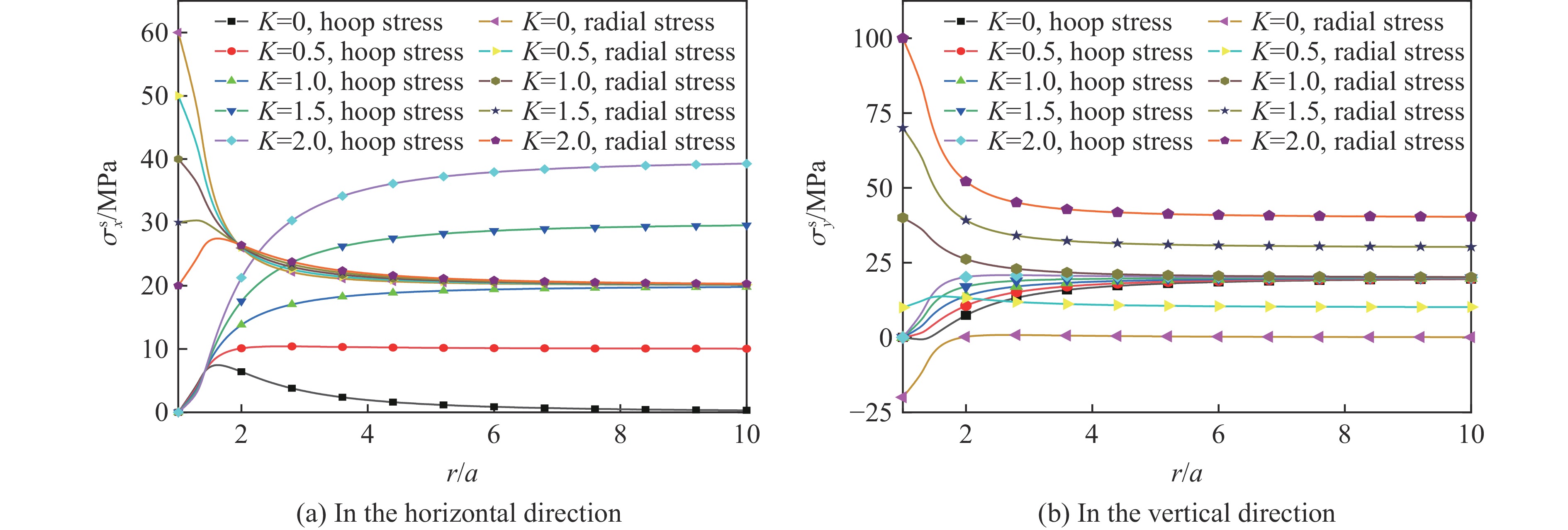
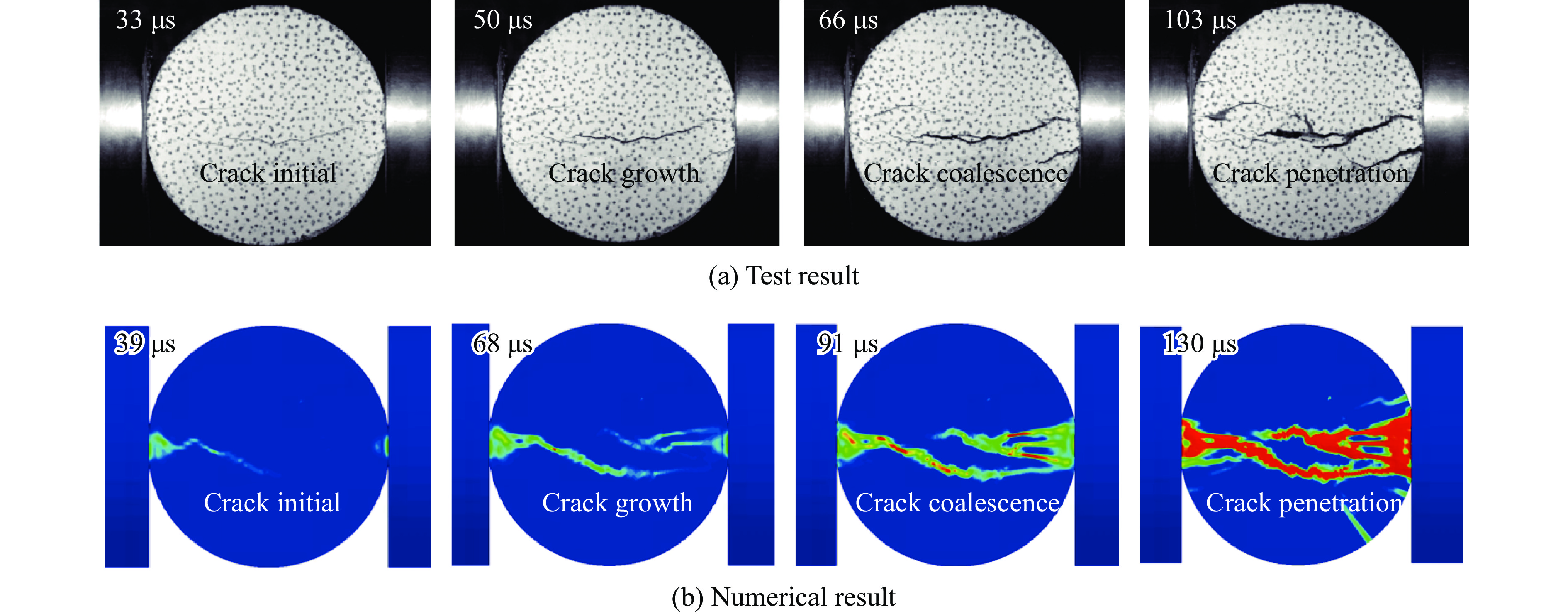
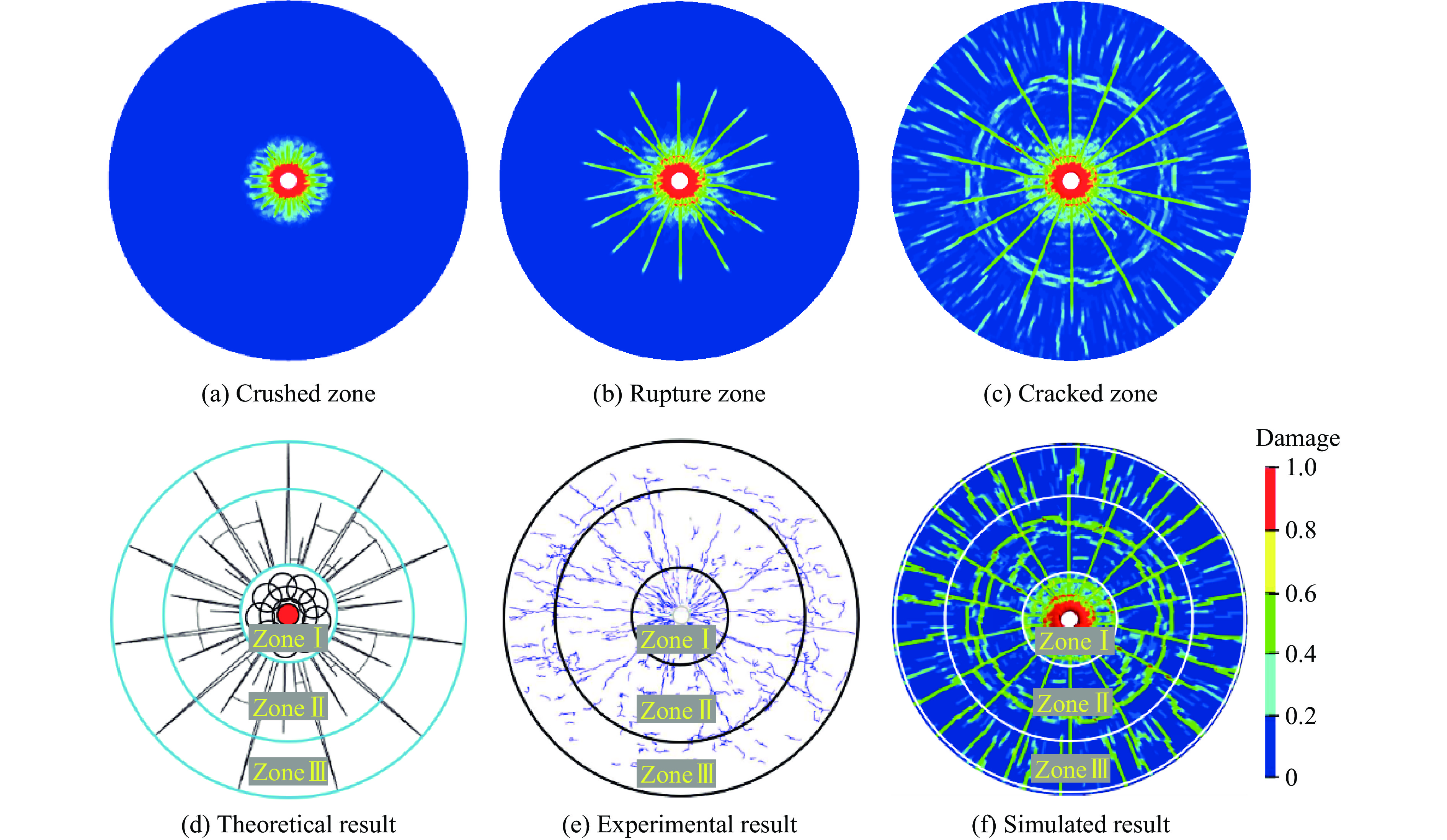
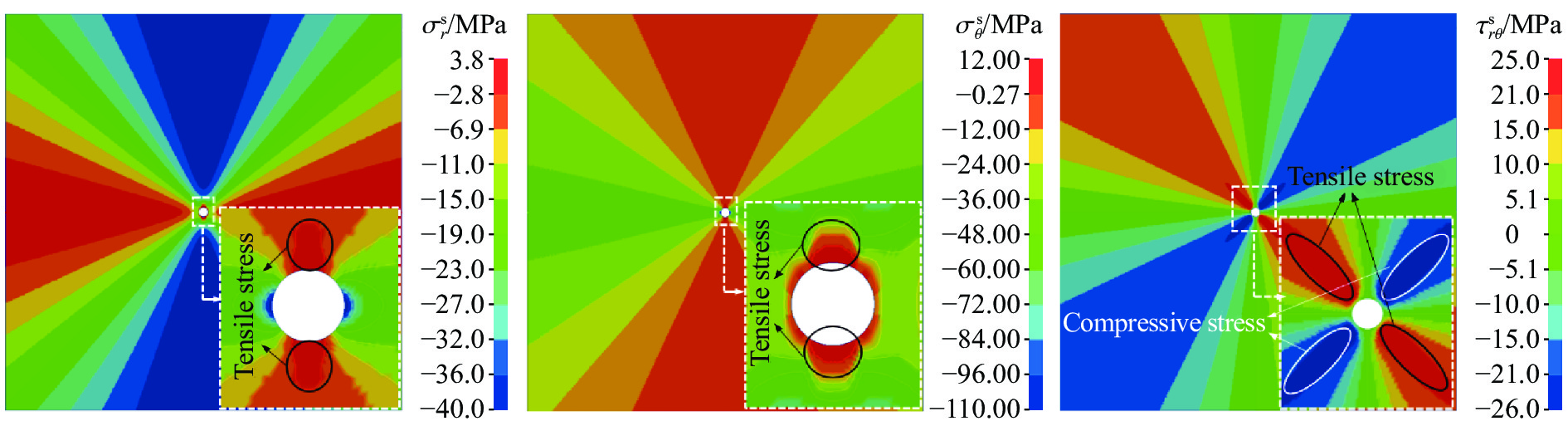
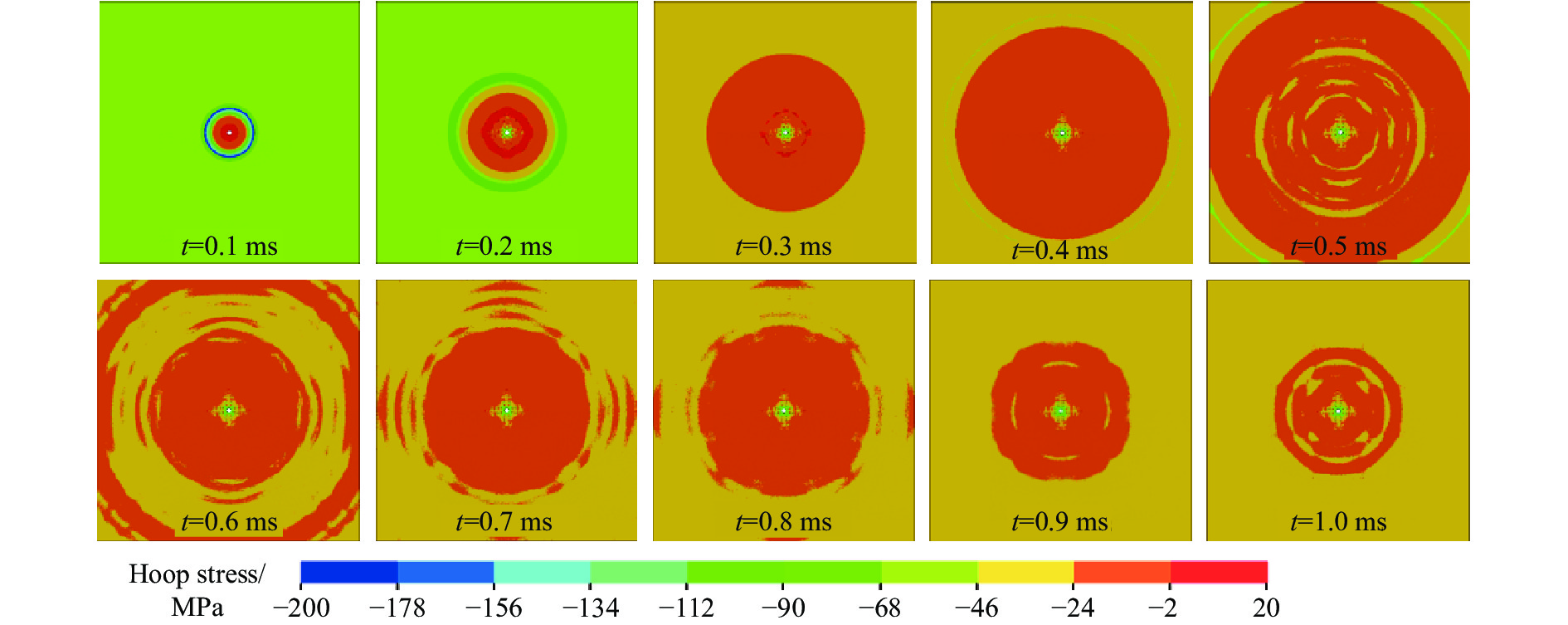
摘要: 钻爆法在岩体开挖工程中应用广泛，其工作效率与岩体结构及地质环境密切相关。初始应力会显著影响岩体爆破裂纹的扩展行为及破坏特征，导致深部岩体超/欠挖等问题。本文采用理论分析和数值模拟相结合的方法研究了不同初始应力下岩体的爆破损伤特性及破裂机理。基于弹性力学建立单孔爆破动静组合理论模型，分析了静态应力分布及动态应力演化特征，揭示了初始应力作用下岩体爆破损伤机制。通过经验公式及动态力学试验对Riedel-Hiermaier-Thoma (RHT) 模型参数进行标定与修正，并结合室内爆破实验及理论结果对数值模型进行验证。此外，在单孔爆破数值模型中分析了爆炸压力演化规律、裂纹扩展行为及分形特征，结果表明数值模拟结果可以较好地论证理论模型的合理性。研究发现：环向拉应力是影响爆破裂纹扩展的重要因素，当初始应力较大时，合理调整环向应力分布可改善岩体爆破碎裂效果，实际工程中可结合预裂技术进行地压调控改变应力分布。Abstract: The technique of drilling and blasting is widely applied in rock excavation, and its working efficiency mainly relies on the rock mass structure and geological conditions. The initial stress plays a crucial role in the blast-induced cracking behavior and failure characteristics, and troubles such as over/underbreak and insufficient fragmentation may be arisen in deep rock masses, leading to other issues related to the waste of mineral resources and production costs. In this paper, the damage features and fracture mechanism of rock blasting under various initial stresses were studied using combined theoretical analysis and numerical simulation. Considering the effect of initial stress on the mechanical response of rock blasting, a theoretical model for single-hole blasting was developed based on elastic mechanics, and then the features of static stress distribution and dynamic pressure evolution were analyzed separately, revealing the fracture mechanism of rock blast-induced damage under initial stress. In addition, the Riedel-Hiermaier-Thoma (RHT) model parameters of rock were determined and adjusted based on a series of empirical formulas as well as dynamic mechanical tests. After calibrating the numerical model against the blasting crack pattern and attenuation of peak pressure by combining test and theoretical results, the single-hole geometric model was created and meshed in the commercial software ANSYS to study the dynamic tangential stress evolution as well as cracking behavior, and the fractal features of rock blasting cracks under different initial stresses were also discussed. The results show that the rationality of the theoretical model can be proved by the numerical simulation: the tangential tensile stress is a critical factor affecting the blasting crack propagation and the rock fragmentation can be improved by adjusting the distribution of hoop stress reasonably when the initial stress is large. In practical engineering, the stress distribution can be changed by controlling the field of geo-stress with presplitting technology.
-
Key words:
- initial stress /
- rock blasting /
- theoretical model /
- numerical simulation /
- crack propagation /
- fractal dimension
-
图 3 不同位置处岩体静态应力的变化
Figure 3. Stress variation of rock mass at different distances under static load
图 4 不同距离处岩体的动态应力波演化
Figure 4. Dynamic stress waves evolution in rock mass at various distances
图 5 RHT模型破坏面的相关参数拟合
Figure 5. Fitting curve of failure surface parameters for RHT model
图 6 数值模拟与试验中岩体冲击破坏过程比较
Figure 6. Comparison of failure process between numerical simulation and test
图 7 数值模拟与试验中岩体应力时程曲线的对比
Figure 7. Comparison of stress with time between numerical simulation and test
图 9 岩体爆破裂纹形态理论、试验与模拟结果比较
Figure 9. Comparison of rock blasting crack patterns among theoretical, experimental and simulated results
图 15 应力初始化模拟结果 (K=0.5)
Figure 15. Numerical results of the stress initialization (K=0.5)
图 16 应力初始化模拟结果 (K=1.0)
Figure 16. Numerical results of the stress initialization (K=1.0)
图 17 应力初始化模拟结果 (K=1.5)
Figure 17. Numerical results of the stress initialization (K=1.5)
图 18 应力初始化模拟结果 (K=2.0)
Figure 18. Numerical results of the stress initialization (K=2.0)
图 19 无初始压力下环向应力的演化过程 (E-1)
Figure 19. Evolution of hoop stress without initial pressure (E-1)
图 20 各向同性压力下环向应力的演化过程 (E-4)
Figure 20. Evolution of hoop stress under equibiaxial pressure (E-4)
图 21 各向异性压力下环向应力的演化过程 (A-4)
Figure 21. Evolution of hoop stress under anisotropic pressure (A-4)
图 22 不同压力条件下环向应力时程曲线
Figure 22. Time histories of hoop stress under various pressure conditions
图 24 各向同性压力下爆破裂纹扩展特征
Figure 24. Characteristics of blasting crack propagation under different equibiaxial pressures
图 25 各向异性压力下爆破裂纹扩展特征
Figure 25. Characteristics of blasting crack propagation under different anisotropic pressures
图 27 各向同性压力下爆破裂纹分形维数拟合线
Figure 27. Fractal dimension of blasting cracks and its fitting lines under equibiaxial pressure
图 28 各向异性压力下爆破裂纹分形维数拟合线
Figure 28. Fractal dimension of blasting cracks and its fitting lines under anisotropic pressure
表 1 不同围压下花岗岩力学参数
Table 1. Mechanical parameters of granite sample under various confining pressure
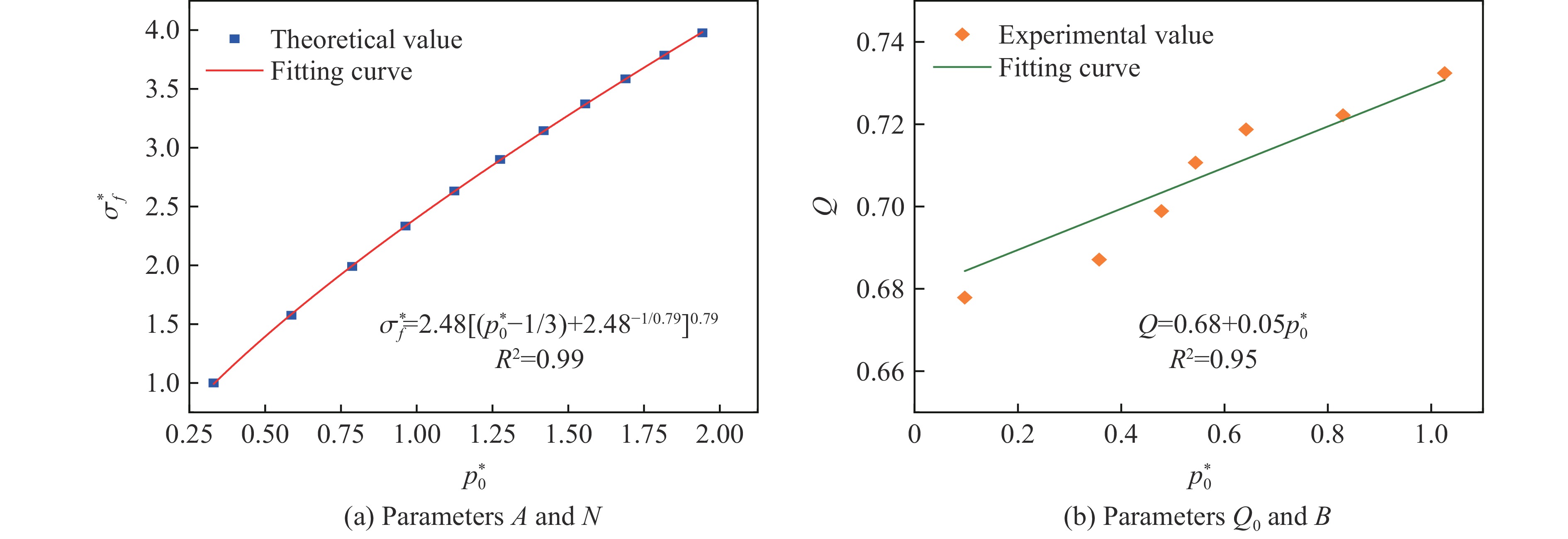
σ2/MPa σ3/MPa σ1/MPa $ {p}_{0}^{*} $ $ {\sigma }_{\mathrm{f}}^{*} $ σ2/MPa σ3/MPa σ1/MPa $ {p}_{0}^{*} $ $ {\sigma }_{\mathrm{f}}^{*} $ 0 0 162 0.33 1.00 60 60 569 1.42 3.14 10 10 265 0.59 1.58 70 70 616 1.56 3.37 20 20 342 0.79 1.99 80 80 660 1.69 3.58 30 30 408 0.96 2.33 90 90 703 1.82 3.79 40 40 466 1.12 2.63 100 100 466 1.94 3.98 50 50 520 1.28 2.90 
下载: 导出CSV
表 2 岩石RHT模型材料参数
Table 2. RHT model parameters for rock mass
参数名称 符号 值 来源 参数名称 符号 值 来源 密度 ρ0 2620 kg/m3 试验测定 损伤因子 D1 0.04 参考文献[14] 初始孔隙度 α 1.00 状态方程参数 B0 1.22 抗压强度 fc 162 MPa 状态方程参数 B1 1.22 参考压缩应变率 ${\dot{\varepsilon }}_{0}^{\rm{c}} $ 3.0×10−5 s−1 模型给定 拉伸体积塑性应变分数 $ {P}_{\mathrm{t}}^{\mathrm{f}} $ 0.001 参考拉伸应变率 $ \dot{\varepsilon }_{0}^{\mathrm{t}} $ 3.0×10−6 s−1 孔隙压实压力 Pcomp 6.00 GPa 理论计算 破坏压缩应变率 $ {\dot{\varepsilon }}_{\mathrm{c}} $ 3.0×1025 s−1 压缩应变率指数 βc 0.008 破坏拉伸应变率 $ {\dot{\varepsilon }}_{\mathrm{t}} $ 3.0×1025 s−1 拉伸应变率指数 βt 0.011 损伤因子 D2 1.00 状态方程参数 T1 33.95 GPa 侵蚀塑性应变 $ {\varepsilon }_{\mathrm{p}}^{\mathrm{f}} $ 2.00 默认取值 状态方程参数 T2 0.00 GPa 孔隙度指数 NP 3.0 Hugoniot多项式系数 A1 33.95 GPa 最小损伤残余应变 $ {\varepsilon }_{\mathrm{p}}^{m} $ 0.012 Hugoniot多项式系数 A2 41.42 GPa 剪切模量减小因子 ξ 0.50 Hugoniot多项式系数 A3 8.71 GPa 相对抗剪强度 $ {F}_{\mathrm{s}}^{*} $ 0.18 试验优化 孔隙坍塌压力 pcrush 108 相对抗拉强度 $ {F}_{\mathrm{t}}^{*} $ 0.06 洛德角相关因子 Q0 0.68 压缩屈服面参数 $ {G}_{\mathrm{c}}^{*} $ 0.50 洛德角相关因子 B 0.05 拉伸屈服面参数 $ {G}_{\mathrm{t}}^{*} $ 0.70 破坏面参数 A 2.48 残余面参数 Af 1.62 破坏面参数 N 0.79 残余面参数 Nf 0.62 弹性剪切模量 G 21.9 GPa
下载: 导出CSV
表 3 炸药模型材料参数
Table 3. Parameters for the explosive material
ρe/(kg·m−3) vd /(m·s−1) pCJ/GPa $ {E}_{0}^{\mathrm{e}} $/(J·m−3) Ae/GPa Be/GPa R1 R2 ω 1320 6690 16 7.38×109 586 21.6 5.81 1.77 0.282
下载: 导出CSV
表 4 空气模型材料参数
Table 4. Mateiral parameters for the air
ρa/(kg·m−3) C0 C1 C2 C3 C4 C5 C6 $ {E}_{0}^{\mathrm{a}} $/(kJ·m−3) V0 1.29 0 0 0 0 0.4 0.4 0 250 1.0
下载: 导出CSV
表 5 初始应力加载条件
Table 5. Initial stress conditions in numerical simulation
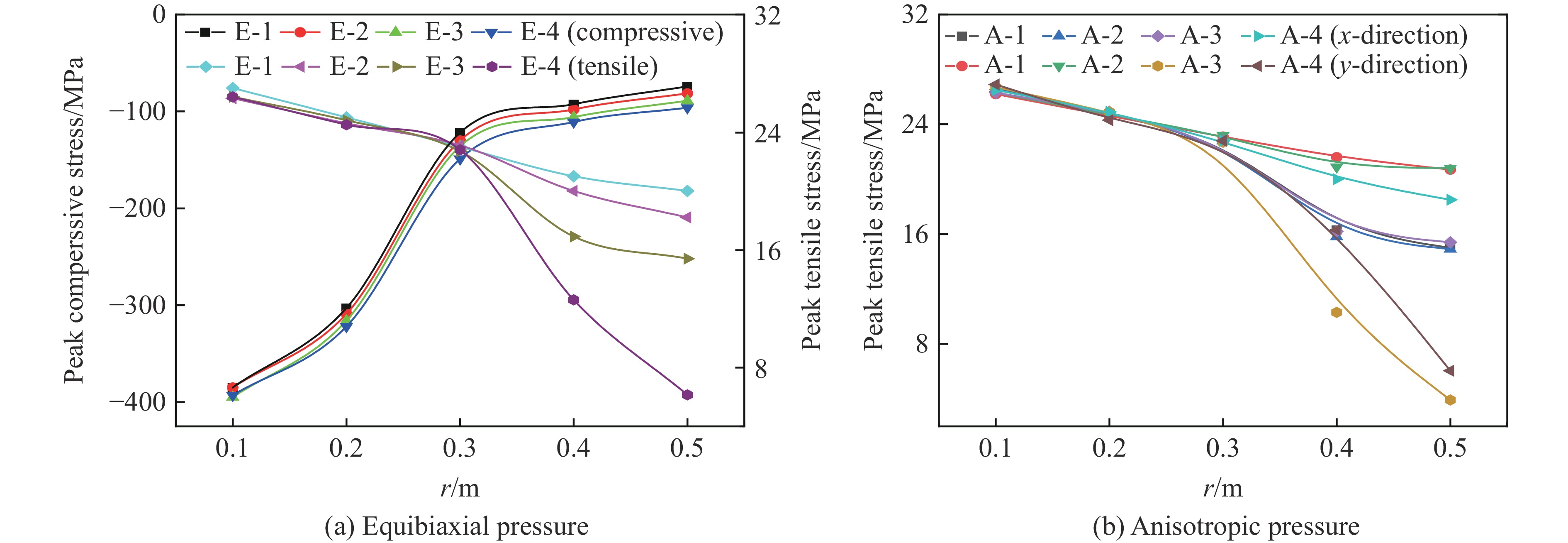
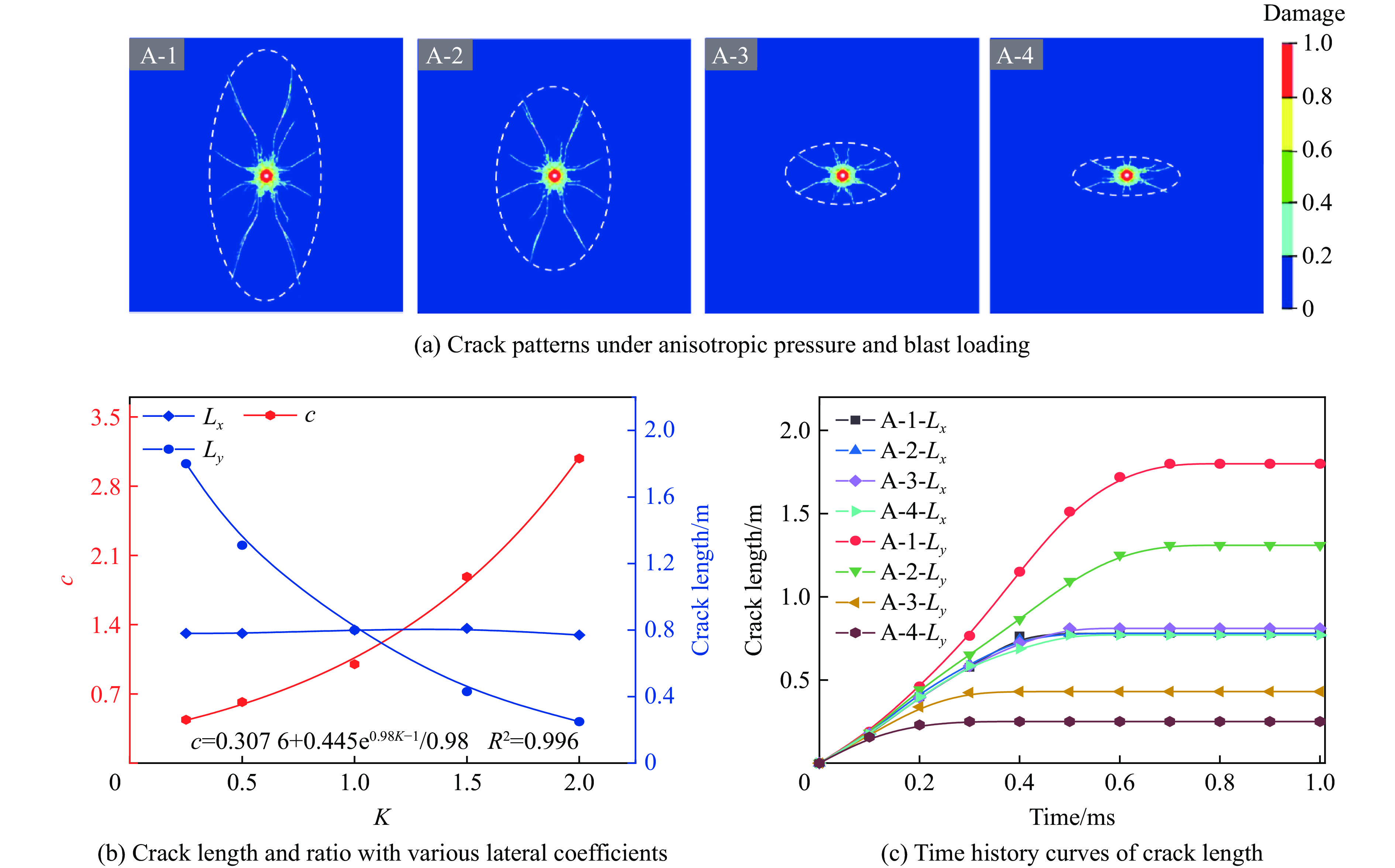
应力状态 工况 埋深/m σx/MPa σy/MPa 应力状态 工况 埋深/m σx/MPa σy/MPa 各向同性初始压力 E-1 0 0 0 各向异性初始压力 A-1 750 5 20 E-2 375 10 10 A-2 750 10 20 E-3 750 20 20 A-3 1125 30 20 E-4 1125 30 30 A-4 1500 40 20
下载: 导出CSV
-
[1] LI X B, GONG F Q, TAO M, et al. Failure mechanism and coupled static-dynamic loading theory in deep hard rock mining: a review [J]. Journal of Rock Mechanics and Geotechnical Engineering, 2017, 9(4): 767–782. DOI: 10.1016/j.jrmge.2017.04.004. [2] 杨建华, 孙文彬, 姚池, 等. 高地应力岩体多孔爆破破岩机制 [J]. 爆炸与冲击, 2020, 40(7): 075202. DOI: 10.11883/bzycj-2019-0427.YANG J H, SUN W B, YAO C, et al. Mechanism of rock fragmentation by multi-hole blasting in highly-stressed rock masses [J]. Explosion and Shock Waves, 2020, 40(7): 075202. DOI:10.11883/bayzj-2019-0427. DOI: 10.11883/bzycj-2019-0427. [3] 朱万成, 唐春安, 左宇军. 深部岩体动态损伤与破裂过程 [M]. 北京: 科学出版社, 2014: 112–121. [4] NICHOLLS H R, DUVALL W I. Presplitting rock in the presence of a static stress field [R]. Washington DC: U. S. Department of the Interior, Bureau of Mines, 1966. [5] KUTTER H K, FAIRHURST C. On the fracture process in blasting [J]. International Journal of Rock Mechanics and Mining Sciences & Geomechanics Abstracts, 1971, 8(3): 181–202. DOI: 10.1016/0148-9062(71)90018-0. [6] 肖正学, 张志呈, 李端明. 初始应力场对爆破效果的影响 [J]. 煤炭学报, 1996, 21(5): 497–501. DOI: 10.13225/j.cnki.jccs.1996.05.011.XIAO Z X, ZHANG Z C, LI D M. The influence of initial stress field on blasting [J]. Journal of China Coal Society, 1996, 21(5): 497–501. DOI: 10.13225/j.cnki.jccs.1996.05.011. [7] 刘殿书, 王万富, 杨吕俊, 等. 初始应力条件下爆破机理的动光弹实验研究 [J]. 煤炭学报, 1999, 24(6): 612–614. DOI: 10.13225/j.cnki.jccs.1999.06.012.LIU D S, WANG W F, YANG L J, et al. Holophotoelasticity study on mechanism of blasting under initiative stress field [J]. Journal of China Coal Society, 1999, 24(6): 612–614. DOI: 10.13225/j.cnki.jccs.1999.06.012. [8] YANG R S, DING C X, LI Y L, et al. Crack propagation behavior in slit charge blasting under high static stress conditions [J]. International Journal of Rock Mechanics and Mining Sciences, 2019, 119: 117–123. DOI: 10.1016/J.ijrmms.2019.05.002. [9] 杨仁树, 肖成龙, 丁晨曦, 等. 空孔与运动裂纹相互作用的动焦散线实验研究 [J]. 爆炸与冲击, 2020, 40(5): 052202. DOI: 10.11883/bzycj-2019-0091.YANG R S, XIAO C L, DING C X, et al. Experimental study on dynamic caustics of interaction between void and running crack [J]. Explosion and Shock Waves, 2020, 40(5): 052202. DOI: 10.11883/bzycj-2019-0091. [10] 岳中文, 田世颖, 张士春, 等. 单向围压作用下切缝药包爆破爆生裂纹扩展规律的研究 [J]. 振动与冲击, 2019, 38(23): 186–195. DOI: 10.13465/j.cnki.jvs.2019.23.027.YUE Z W, TIAN S Y, ZHANG S C, et al. Expanding law of cracks formed by slotted cartridge blast under unidirectional confining pressure [J]. Journal of Vibration and Shock, 2019, 38(23): 186–195. DOI: 10.13465/j.cnki.jvs.2019.23.027. [11] YANG L Y, HUANG C, BAO S J, et al. Model experimental study on controlled blasting of slit charge in deep rock mass [J]. Soil Dynamics and Earthquake Engineering, 2020, 138: 106318. DOI: 10.1016/j.soildyn.2020.106318. [12] MA G W, AN X M. Numerical simulation of blasting-induced rock fractures [J]. International Journal of Rock Mechanics and Mining Sciences, 2008, 45(6): 966–975. DOI: 10.1016/j.ijrmms.2007.12.002. [13] XIE L X, LU W B, ZHANG Q B, et al. Damage evolution mechanisms of rock in deep tunnels induced by cut blasting [J]. Tunnelling and Underground Space Technology, 2016, 58: 257–270. DOI: 10.1016/j.tust.2016.06.004. [14] XIE L X, LU W B, ZHANG Q B, et al. Analysis of damage mechanisms and optimization of cut blasting design under high in-situ stresses [J]. Tunnelling and Underground Space Technology, 2017, 66: 19–33. DOI: 10.1016/j.tust.2017.03.009. [15] LI X D, LIU K W, YANG J C, et al. Numerical study on blast-induced fragmentation in deep rock mass [J]. International Journal of Impact Engineering, 2022, 170: 104367. DOI: 10.1016/j.ijimpeng.2022.104367. [16] LI X D, LIU K W, SHA Y Y, et al. Numerical investigation on rock fragmentation under decoupled charge blasting [J]. Computers and Geotechnics, 2023, 157: 105312. DOI: 10.1016/j.compgeo.2023.105312. [17] KIRSCH G. Die theorie der elastizitat und die bedurfnisse der festigkeitslehre [J]. Zantralblatt Verlin Deutscher Ingenieure, 1898, 42: 797–807. [18] MIKLOWITZ J, KAUL R K. The theory of elastic waves and waveguides [J]. Journal of Applied Mechanics, 1979, 46(4): 969. DOI: 10.1115/1.3424709. [19] YI C P, JOHANSSON D, GREBERG J. Effects of in-situ stresses on the fracturing of rock by blasting [J]. Computers and Geotechnics, 2018, 104: 321–330. DOI: 10.1016/j.compgeo.2017.12.004. [20] TAO J, YANG X G, LI H T, et al. Effects of in-situ stresses on dynamic rock responses under blast loading [J]. Mechanics of Materials, 2020, 145: 103374. DOI: 10.1016/j.mechmat.2020.103374. [21] LI X H, ZHU Z M, WANG M, et al. Numerical study on the behavior of blasting in deep rock masses [J]. Tunnelling and Underground Space Technology, 2021, 113: 103968. DOI: 10.1016/j.tust.2021.103968. [22] WANG Z L, WANG H C, WANG J G, et al. Finite element analyses of constitutive models performance in the simulation of blast-induced rock cracks [J]. Computers and Geotechnics, 2021, 135: 104172. DOI: 10.1016/j.compgeo.2021.104172. [23] 俞茂宏. 双剪理论及其应用 [M]. 北京: 科学出版社, 1998: 849–860. [24] BORRVALL T, SWEDEN L, RIEDEL W. The RHT concrete model in LS-DYNA [C]// Proceedings of the 8th European LS-DYNA Conference. Strasbourg, France, 2011. [25] 李洪超, 陈勇, 刘殿书, 等. 岩石RHT模型主要参数敏感性及确定方法研究 [J]. 北京理工大学学报, 2018, 38(8): 779–785. DOI: 10.15918/j.tbit1001-0645.2018.08.002.LI H C, CHEN Y, LIU D S, et al. Sensitivity analysis determination and optimization of rock RHT parameters [J]. Transactions of Beijing Institute of Technology, 2018, 38(8): 779–785. DOI: 10.15918/j.tbit1001-0645.2018.08.002. [26] MA S Z, LIU K W, GUO T F, et al. Experimental and numerical investigation on the mechanical characteristics and failure mechanism of cracked coal & rock-like combined sample under uniaxial compression [J]. Theoretical and Applied Fracture Mechanics, 2022, 122: 103583. DOI: 10.1016/j.tafmec.2022.103583. [27] BANADAKI M M D, MOHANTY B. Numerical simulation of stress wave induced fractures in rock [J]. International Journal of Impact Engineering, 2012, 40/41: 16–25. DOI: 10.1016/j.ijimpeng.2011.08.010. [28] YANG J C, LIU K W, LI X D, et al. Stress initialization methods for dynamic numerical simulation of rock mass with high in-situ stress [J]. Journal of Central South University, 2020, 27(10): 3149–3162. DOI: 10.1007/s11771-020-4535-3. [29] 谢和平, 高峰, 周宏伟, 等. 岩石断裂和破碎的分形研究 [J]. 防灾减灾工程学报, 2003, 23(4): 1–9. DOI: 10.3969/j.issn.1672-2132.2003.04.001.XIE H P, GAO F, ZHOU H W, et al. Fractal fracture and fragmentation in rocks [J]. Journal of Disaster Prevention and Mitigation Engineering, 2003, 23(4): 1–9. DOI: 10.3969/j.issn.1672-2132.2003.04.001. [30] DING C X, YANG R S, YANG L Y. Experimental results of blast-induced cracking fractal characteristics and propagation behavior in deep rock mass [J]. International Journal of Rock Mechanics and Mining Sciences, 2021, 142: 104772. DOI: 10.1016/J.ijrmms.2021.104772. -
计量
- 文章访问数: 213
- HTML全文浏览量: 37
- PDF下载量: 100
- 被引次数: 0