Theoretical and numerical studies on the scale effects for strong explosion fireball thermal radiation characteristics
-
摘要: 针对火球热辐射双脉冲极值特征问题，基于辐射流体热传导近似模型，理论推导了表征尺度效应的相似参数来界定热辐射极值特征的适用域。选取火球特征尺度和辐射自由程特征尺度差异较大的2类典型问题来验证尺度效应相似参数的有效性，并采用高精度Euler辐射流体计算程序来模拟火球热辐射对尺度效应相似参数的依赖性。理论和数值模拟结果表明：尺度效应相似参数能较好地描述不同条件下的火球热辐射演化规律，并可扩展到实验分析中；仅依靠爆炸高度来表征火球演化中的尺度效应存在一定局限性。Abstract: As a typical characteristic of fireball phenomena, thermal radiation plays an important role in damage assessments. Up to now, many studies of thermal radiation using theoretical, numerical, and experimental methods have been carried out and empirical formulas in forms of yield or density are constructed to feature the extremal characteristic of fireball thermal radiation. However, due to the combined action of radiation free path (RFP) and fireball characteristic length (FCL), it is difficult to identify these formula’s application scope, and further theoretical studies are needed to take the scale effect (SE) into account. By radiation heat conduction approximation model under optical thickness assumption, scale effect similarity parameter (SESP) was theoretically derived and its scope of application is further verified by high-precision numerical method. The numerical code is developed within a framework of Euler method, and adaptive mesh refinement method is employed to improve the precision in the radiation front. The results of theoretical analysis show that SESP is consistent with existed conclusions regarding the thermal radiation of fireball at different altitudes, and it can be applied to the analysis of laboratory scale fireball. Meanwhile, numerical results also show that both scale effects at different altitudes and laboratory scale can be characterized by SESP.
-
图 1 爆炸当量为1 kt、爆炸高度为海平面高度时爆炸火球有效半径和有效温度随时间的变化规律(42群)
Figure 1. Effective temperature and radius of yield 1 kt explosion at sea level (42 group)
图 2 爆炸当量为1 kt、爆炸高度为海平面高度时爆炸火球有效半径和有效温度随时间的变化规律（单群）
Figure 2. Effective temperature and radius of yield 1 kt explosion at sea level (single group)
图 3 爆炸高度不同时尺度效应相似参数与密度的关系
Figure 3. Scale effect similarity parameter vs. density at different altitudes
图 4 不同爆炸高度下火球的热辐射功率和有效半径
Figure 4. Thermal radiant power and effective radius at different altitudes
图 5 爆炸高度为35 km时不同爆炸当量下火球的热辐射功率和有效半径
Figure 5. Thermal radiant power and effective radius when the altitude is 35 km for different yields
图 6 R0取0.2和0.5 mm时火球的热辐射功率和有效半径（hb=0）
Figure 6. Thermal radiant power and effective radius under different initial radius for laboratory scale fireball (hb=0)
图 7 R0取10.0和1.0 mm时火球的热辐射功率和有效半径（hb=20 km）
Figure 7. Thermal radiation power and effective radius under different initial radius for laboratory scale fireball (hb=20 km)
表 1 爆炸当量为1 kt时不同爆炸高度下的尺度效应参数
Table 1. Scale effect similarity parameters of yield 1 kt at different altitudes
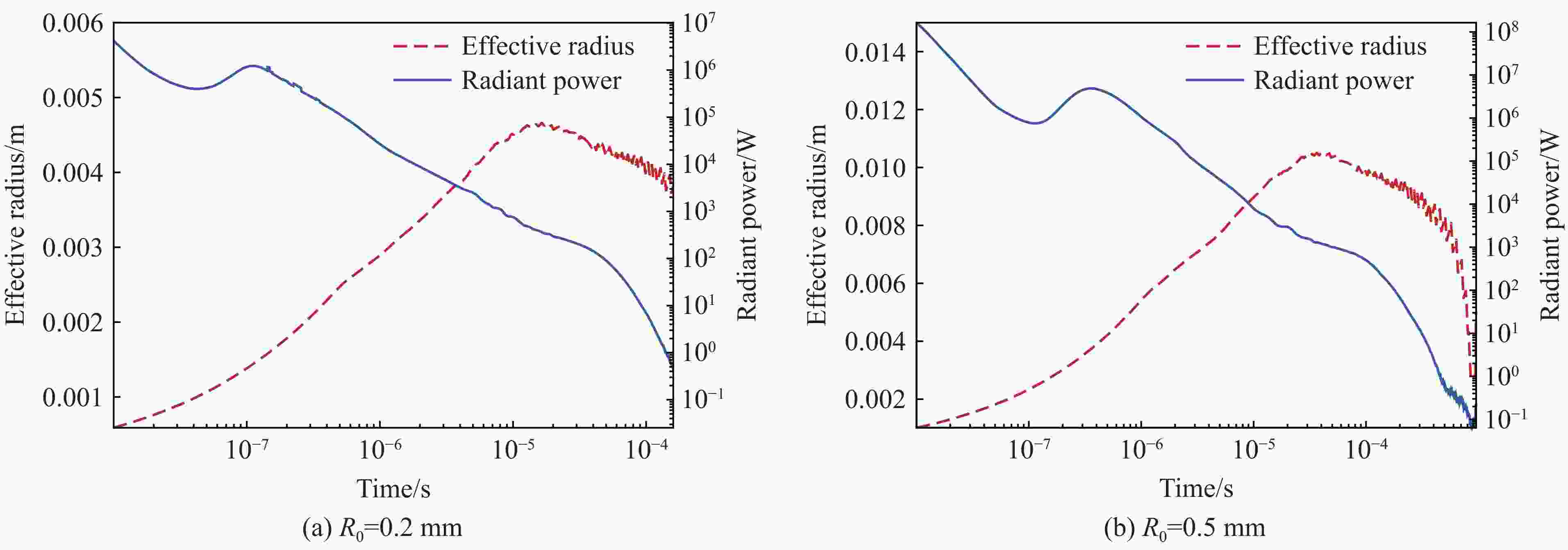
hb/km $ \rho_{h_{\mathrm{b}}} $/(kg·m−3) LMFP/m LFB/m fscale 0 1.22×100 5.98×10−5 32.00 1.87×10−6 10 4.13×10−1 3.87×10−4 40.19 2.85×10−5 20 8.84×10−2 5.70×10−3 55.55 1.42×10−3 30 1.82×10−2 9.32×10−2 77.45 8.10×10−2 35 8.30×10−3 3.70×10−1 91.30 5.97×10−1 40 3.90×10−3 1.44×100 107.01 4.22×100 50 9.92×10−4 1.67×101 146.62 1.44×102 60 2.93×10−4 1.49×102 184.19 3.37×103 70 7.58×10−5 1.71×103 244.72 1.12×105 
下载: 导出CSV
表 2 海平面高度时不同火球尺度所对应的尺度效应参数（
$h_{\mathrm{b}}=0 $ ）Table 2. Scale effect similarity parameter in different laboratory scales (
$h_{\mathrm{b}}=0 $ )R0/m $ \rho_{h_{\mathrm{b}}} $/(kg·m−3) LMFP/m LFB/m fscale 2.81 1.225 5.98×10−5 32.00 1.87×10−6 1.00 1.225 5.98×10−5 9.86 6.07×10−6 1.00×10−1 1.225 5.98×10−5 7.14×10−1 8.37×10−5 1.00×10−2 1.225 5.98×10−5 5.17×10−2 1.16×10−3 1.00×10−3 1.225 5.98×10−5 3.75×10−3 1.60×10−2 5.00×10−4 1.225 5.98×10−5 1.70×10−3 3.52×10−2 2.00×10−4 1.225 5.98×10−5 5.98×10−4 1.00×10−1
下载: 导出CSV
表 3
$h_{\mathrm{b}}=20\;{\mathrm{km}} $ 时不同火球尺度所对应的尺度效应参数Table 3. Scale effect similarity parameter in different laboratory scales (
$h_{\mathrm{b}}=20\;{\mathrm{km}} $ )R0/m $ \rho_{h_{\mathrm{b}}} $/(kg·m−3) LMFP/m LFB/m fscale 2.81 8.84×10−2 5.70×10−3 55.55 1.42×10−3 1.00 8.84×10−2 5.70×10−3 17.11 4.61×10−3 1.00×10−1 8.84×10−2 5.70×10−3 1.24 6.37×10−2 1.00×10−2 8.84×10−2 5.70×10−3 8.98×10−2 8.79×10−1 1.00×10−3 8.84×10−2 5.70×10−3 6.51×10−3 1.21×101 5.00×10−4 8.84×10−2 5.70×10−3 2.95×10−3 2.67×101 2.00×10−4 8.84×10−2 5.70×10−3 1.04×10−3 7.60×101
下载: 导出CSV
-
[1] 张守中. 爆炸与冲击动力学 [M]. 北京: 兵器工业出版社, 1993. [2] DRAKE R P. 高能量密度物理——基础、惯性约束聚变和实验天体物理学 [M]. 孙承纬, 译. 北京: 国防工业出版社, 2013.DRAKE R P. High-energy-density physics: fundamentals, inertial fusion, and experimental astrophysics [M]. Translated by SUN C W. Beijing: National Defense Industry Press, 2013. [3] 乔登江. 核爆炸物理概论 [M]. 北京: 国防工业出版社, 2003. [4] ZHANG J, PEI W B. Similarity transformations of radiation hydrodynamic equations and investigation on laws of radiative conduction [J]. Physics of Fluids B: Plasma Physics, 1992, 4(4): 872–876. DOI: 10.1063/1.860241. [5] FOURNIER K B, BROWN JR C G, MAY M J, et al. A geophysical shock and air blast simulator at the National Ignition Facility [J]. Review of Scientific Instruments, 2014, 85(9): 095119. DOI: 10.1063/1.4896119. [6] BOUQUET S, FALIZE E, MICHAUT C, et al. From lasers to the universe: scaling laws in laboratory astrophysics [J]. High Energy Density Physics, 2010, 6(4): 368–380. DOI: 10.1016/j.hedp.2010.03.001. [7] KOENIG M, VINCI T, BENUZZI-MOUNAIX A, et al. Radiative shocks: an opportunity to study laboratory astrophysics [J]. Physics of Plasmas, 2006, 13(5): 056504. DOI: 10.1063/1.2177637. [8] VINCI T, KOENIG M, BENUZZI-MOUNAIX A, et al. Temperature and electron density measurements on laser driven radiative shocks [J]. Physics of Plasmas, 2006, 13(1): 010702. DOI: 10.1063/1.2162804. [9] 赵多, 李守先, 安建祝, 等. 氙气中辐射激波的发光特性 [J]. 物理学报, 2021, 70(7): 075201. DOI: 10.7498/aps.70.20200944.ZHAO D, LI S X, AN J Z, et al. Radiation properties of radiative shock in xenon [J]. Acta Physica Sinica, 2021, 70(7): 075201. DOI: 10.7498/aps.70.20200944. [10] 孙景文. 高空核爆炸和美苏高空核试验的述评 [J]. 中国核科技报告, 1999(1): 966–985.SUN J W. A brief introduction to high altitude nuclear explosion and a review on high altitude nuclear tests of USA and former USSR [J]. China Nuclear Science and Technology Report, 1999(1): 966–985. [11] BRODE H L. Fireball phenomenology: P-3026 [R]. The RAND Corporation, 1964. [12] BRODE H L. Review of nuclear weapons effects [J]. Annual Review of Nuclear and Particle Science, 1968, 18: 153–202. DOI: 10.1146/annurev.ns.18.120168.001101. [13] SVETTSOV V V. Explosions in the lower and middle atmosphere: the spherically symmetrical stage [J]. Combustion, Explosion and Shock Waves, 1994, 30(5): 696–707. DOI: 10.1007/BF00755841. [14] 田宙, 乔登江, 郭永辉. 不同高度强爆炸早期火球数值研究 [J]. 兵工学报, 2009, 30(8): 1078–1083. DOI: 10.3321/j.issn:1000-1093.2009.08.014.TIAN Z, QIAO D J, GUO Y H. Numerical investigation of early fireball of strong explosion for different altitudes [J]. Acta Armamentarii, 2009, 30(8): 1078–1083. DOI: 10.3321/j.issn:1000-1093.2009.08.014. [15] 田宙, 郭永辉, 乔登江. 高空强爆炸早期火球参量分布的数值研究 [J]. 空气动力学学报, 2010, 28(3): 336–340. DOI: 10.3969/j.issn.0258-1825.2010.03.018.TIAN Z, GUO Y H, QIAO D J. Numerical investigation of early fireball parameters distribution of high-altitude strong explosion [J]. Acta Aerodynamica Sinica, 2010, 28(3): 336–340. DOI: 10.3969/j.issn.0258-1825.2010.03.018. [16] ZINN J. A finite difference scheme for time-dependent spherical radiation hydrodynamics problems [J]. Journal of Computational Physics, 1973, 13(4): 569–590. DOI: 10.1016/0021-9991(73)90034-X. [17] JOHNSEN E, COLONIUS T. Implementation of WENO schemes in compressible multicomponent flow problems [J]. Journal of Computational Physics, 2006, 219(2): 715–732. DOI: 10.1016/j.jcp.2006.04.018. [18] 李康, 李守先, 刘娜. 强爆炸火球辐射流体自适应网格高精度数值模拟 [J]. 计算物理, 2021, 38(2): 146–152. DOI: 10.19596/j.cnki.1001-246x.8230.LI K, LI S X, LIU N. High-precision numerical simulation of strong explosion fireball with adaptive mesh [J]. Chinese Journal of Computational Physics, 2021, 38(2): 146–152. DOI: 10.19596/j.cnki.1001-246x.8230. [19] BRODE H L, ASANO W, PLEMMONS H M, et al. A program for calculating radiation flow and hydrodynamic motion: RM-5187-PR [R]. The RAND Corporation, 1967. [20] SYMBALISTY E M D, ZINN J, WHITAKER R W. RADFLO physics and algorithms [R]. Los Alamos: Los Alamos National Laboratory, 1995. [21] ZINN J, SUTHERLAND C D. Special numerics for a nuclear-fireball model [R]. Los Alamos: Los Alamos National Laboratory, 1982. [22] FALIZE É, MICHAUT C, BOUQUET S. Similarity properties and scaling laws of radiation hydrodynamic flows in laboratory astrophysics [J]. The Astrophysical Journal, 2011, 730(2): 96. DOI: 10.1088/0004-637X/730/2/96. [23] 乔登江. 核爆炸火球物理 [J]. 物理学进展, 1983, 3(2): 236–267. DOI: 10.3321/j.issn:1000-0542.1983.02.004.QIAO D J. The physics of fireballs [J]. Progress in Physics, 1983, 3(2): 236–267. DOI: 10.3321/j.issn:1000-0542.1983.02.004. [24] 王坚, 李路翔. 核武器效应及防护 [M]. 北京: 北京理工大学出版社, 1993. -
计量
- 文章访问数: 144
- HTML全文浏览量: 43
- PDF下载量: 59
- 被引次数: 0