考虑岩体破坏分区的岩石爆破爆炸荷载历程研究

孙鹏昌; 杨广栋; 卢文波; 范勇; 孟海利; 薛里

doi:10.11883/bzycj-2023-0206

考虑岩体破坏分区的岩石爆破爆炸荷载历程研究

doi: 10.11883/bzycj-2023-0206

孙鹏昌^{1, 2,},
杨广栋^2, ,,
卢文波³,
范勇²,
孟海利¹,
薛里¹

1.
中国铁道科学研究院集团有限公司铁道建筑研究所，北京 100081
2.
三峡大学湖北省水电工程施工与管理重点实验室，湖北宜昌 443002
3.
武汉大学水资源工程与调度全国重点实验室，湖北武汉 430072

基金项目: 国家自然科学基金（52209162）；湖北省自然科学基金（2023AFA048）；水电工程施工与管理湖北省重点实验室（三峡大学）开放基金（2023KSD01）；北京市科协青年人才托举工程项目（BYESS2022219）

详细信息

作者简介:
孙鹏昌（1994－　），男，博士，助理研究员，sunpch@whu.edu.cn

通讯作者:
杨广栋（1992－　），男，博士，副教授，ygd@ctgu.edu.cn

中图分类号: O383
计量
- 文章访问数: 173
- HTML全文浏览量: 55
- PDF下载量: 76
- 被引次数: 0
出版历程
- 收稿日期: 2023-06-07
- 修回日期: 2024-01-16
- 网络出版日期: 2024-01-17
- 刊出日期: 2024-03-14

A study on explosive load history of rock blasting considering rock failure zones

SUN Pengchang^{1, 2
,},
YANG Guangdong^{2
, ,},
LU Wenbo³,
FAN Yong²,
MENG Haili¹,
XUE Li¹

1.
Railway Engineering Research Institute, China Academy of Railway Sciences Corporation Limited, Beijing 100081, China
2.
Hubei Key Laboratory of Construction and Management in Hydropower Engineering, China Three Gorges University, Yichang 443002, Hubei, China
3.
State Key Laboratory of Water Resources Engineering and Management, Wuhan University, Wuhan 430072, Hubei, China

摘要

摘要: 针对岩石爆破爆炸荷载历程中未联合考虑岩石爆破动态过程和炮孔周围岩体破坏分区的不足，开展了考虑岩体破坏分区的岩石爆破爆炸荷载历程及其适用性研究。联合岩石爆破动态过程和岩体破坏分区的理论解，推导了考虑岩体破坏分区的岩石爆破爆炸荷载理论公式，比较了考虑岩体破坏分区的岩石爆破爆炸荷载历程与实测炮孔爆炸压力曲线，开展了单孔爆破现场试验和相应条件下3种爆炸荷载工况的数值模拟，并对爆破振动现场实测和数值模拟结果进行了对比。研究结果表明：考虑岩体破坏分区的爆炸荷载历程包括上升段和衰减段Ⅰ、Ⅱ、Ⅲ，上升段持续时间极短，衰减段持续时间较长且主要由填塞情况控制；考虑岩体破坏分区的爆炸荷载历程理论计算结果与实测炮孔爆炸压力曲线的变化趋势一致，验证了考虑岩体破坏分区的岩石爆破爆炸荷载理论公式的可靠性；考虑岩体破坏分区的爆炸荷载工况下，单孔爆破振动波形的数值模拟结果与现场实测结果的主要特征一致，该荷载工况下质点峰值振速计算结果与现场实测值偏差率最小，绝大部分在7%以内，表明了其应用于数值模拟的优越性；考虑岩体破坏分区的爆炸荷载可随岩石爆破系统条件的变化而动态调整，其可靠性好、适应性强、应用效果佳。
- 岩石爆破 /
- 爆炸荷载 /
- 理论公式 /
- 破坏分区 /
- 破岩过程
Abstract: Due to the deficiency that dynamic processes of rock blasting and rock failure zones around a blasthole are not simultaneously considered, the explosion load history of rock blasting considering rock failure zones and its reliability were investigated. Combining theoretical solutions of the dynamic processes of rock blasting and the rock failure zones around a blasthole, a theoretical formula of the explosive load history considering rock failure zones was derived, and a comparison was made between the derived explosive load history and a measured explosion pressure curve inside a blasthole. Both the field test on an ideal site and the numerical simulation including three explosion load conditions of single hole blasting were carried out, and the field and numerical results of blasting vibration were compared. The results show that the explosive load history considering rock failure zones consists of an ascending stage and three attenuation stages Ⅰ, Ⅱ, and Ⅲ, among which the ascending stage lasts for an extremely short time, while the attenuation stages last for a long time and are controlled by the stemming conditions. The change tendency of the calculated explosive load history considering rock failure zones is consistent with that of the measured explosion pressure curve, indicating the reliability of the explosive load history considering rock failure zones. The numerical results of single hole blasting vibration waveforms under the theoretical explosive load condition are consistent with the filed results, and the deviation ratios between the calculated peak particle velocity (PPV) results under the theoretical explosive load condition and the field PPV results are the smallest, most of which are within 7%, indicting the explosive load history considering rock failure zones has strong reliability. The explosive load history considering rock failure zones can be adjusted as the rock blasting system changes, and it has wide adaptability and good application potentials. The research results may help provide a theoretical basis for realizing efficient and accurate calculation about rock blasting.
- rock blasting /
- explosive load /
- theoretical formula /
- failure zone /
- rock breaking process

HTML全文

图 1 岩石爆破动态过程

Figure 1. Dynamic processes of rock blasting

下载: 全尺寸图片幻灯片

图 2 楔形裂纹扩展力学模型

Figure 2. A mechanical model of wedge-shaped crack propagation

下载: 全尺寸图片幻灯片

图 3 爆生气体逸散波场示意图

Figure 3. Stress wave filed of escaped explosion gases

下载: 全尺寸图片幻灯片

图 4 炮孔周围岩体破坏分区

Figure 4. Failure zones in rock mass around a blasthole

下载: 全尺寸图片幻灯片

图 5 爆炸荷载衰减段的计算过程

Figure 5. Calculation flow chart of blasthole pressure combining blasting processes and rock failure zones

下载: 全尺寸图片幻灯片

图 6 考虑岩体破坏分区的爆炸荷载历程

Figure 6. Explosive load history considering rock failure zones

下载: 全尺寸图片幻灯片

图 7 铵油炸药装药结构^[38]

Figure 7. Charge configuration with ANFO^[38]

下载: 全尺寸图片幻灯片

图 8 实测炮孔爆炸压力曲线^[38]

Figure 8. Measured explosive load history^[38]

下载: 全尺寸图片幻灯片

图 9 实测与理论爆炸荷载压力曲线对比

Figure 9. Comparison of measured and calculated explosive loads

下载: 全尺寸图片幻灯片

图 10 单孔爆破试验现场炮孔布置

Figure 10. Blasthole layout for single hole blasting test

下载: 全尺寸图片幻灯片

图 11 单孔爆破试验装药结构示意图

Figure 11. Charge configuration for single hole blasting test

下载: 全尺寸图片幻灯片

图 12 爆破振动监测系统

Figure 12. Blasting vibration monitoring system

下载: 全尺寸图片幻灯片

图 13 爆破振动监测点布置

Figure 13. Layout of blasting vibration monitoring points

下载: 全尺寸图片幻灯片

图 14 监测点M3的爆破振动波形

Figure 14. Blasting vibration waveforms at monitoring point M3

下载: 全尺寸图片幻灯片

图 15 单孔爆破实测PPV随水平爆心距的变化

Figure 15. Variation of measured PPV with horizontal distance from the explosive center

下载: 全尺寸图片幻灯片

图 16 单孔爆破的数值模型

Figure 16. A numerical model for single hole blasting

下载: 全尺寸图片幻灯片

图 17 3种工况下的爆炸荷载历程曲线

Figure 17. Explosive load-time history curves for three working conditions

下载: 全尺寸图片幻灯片

图 18 实测和数值模拟爆破振动波形比较（监测点M4）

Figure 18. Comparison of measured and numerical blasting vibration waveforms at monitoring point M4

下载: 全尺寸图片幻灯片

图 19 PPV实测值和数值模拟结果比较

Figure 19. Comparison of measured and numerical PPVs

下载: 全尺寸图片幻灯片

表 1 常见的指数函数类爆炸荷载

Table 1. Typical explosive loads in exponential function forms

指数函数类爆炸荷载	来源	指数函数类爆炸荷载	来源
$p\left( t \right) = {p_0}\;{ {\text{e} }^{ - \alpha t} }$	文献[10]	$ p\left( t \right) = {p_0}H\left( t \right){t^n}{{\text{e}}^{ - \beta t}} $	文献[16]
$p\left( t \right) = {p_0}( { { {{\text{e} }} ^{ - \alpha t} } - { {{\text{e} }} ^{ - \beta t} } } )$	文献[11]	$ p\left( t \right) = {p_{{\text{VN}}}}{\left( {{{{\text{e}}\beta } / n}} \right)^n}H\left( t \right){t^n}{{\text{e}}^{ - \beta t}} $	文献[17]
$ p\left( t \right) = {p_0}\zeta ( {{{\text{e}}^{ - \alpha t}} - {{\text{e}}^{ - \beta t}}} ) $	文献[12-13]	$ p\left( t \right) = {p_{{\text{JWL}}}}{p_{\text{s}}}\left( t \right) $	文献[18]
$ p\left( t \right) = 4{p_0}\left( {{{\text{e}}^{ - {{\alpha t} / {\sqrt 2 }}}} - {{\text{e}}^{ - \sqrt 2 \alpha t}}} \right) $	文献[14-15]	$ p\left( t \right) = {p_{{\text{VN}}}}{p_{\text{u}}}\left( t \right){p_{\text{d}}}\left( t \right) $	文献[19]

下载: 导出CSV

表 2 爆炸荷载计算用炸药参数^[36]

Table 2. Explosive parameters for explosive load calculation^[36]

药卷直径d_c/mm	ρ_e/(kg·m⁻³)	D/(m·s⁻¹)	爆热Q/(MJ·kg⁻¹)
70	1200	4000	3.991

下载: 导出CSV

表 3 爆炸荷载计算用岩体参数^[36]

Table 3. Rock mass parameters for explosive load calculation^[36]

ρ/(kg∙m⁻³)	E/GPa	μ	σ_c/MPa	σ_t/MPa	ϕ/(°)	ψ
2670	59.5	0.23	129.1	10.3	45	2

下载: 导出CSV

表 4 爆炸荷载计算用填塞材料参数^[36]

Table 4. Stemming parameters for explosive load calculation^[36]

ρ_s/(kg·m⁻³)	弹性模量E_s/GPa	泊松比μ_s	$\varphi_{\mathrm{s}} $/(°)	f_d
1800	0.2	0.30	28	0.055

下载: 导出CSV

表 5 铵油炸药性能参数^[38]

Table 5. Performance parameters for ANFO^[38]

密度/(kg·m⁻³)	爆速/(m·s⁻¹)	爆热/(kJ·g⁻¹)	装药直径/mm
821	3800	4.0	95

下载: 导出CSV

表 6 单孔爆破试验岩体参数

Table 6. Rock mass parameters for single hole blasting test

密度/(kg·m⁻³)	弹性模量/GPa	泊松比	单轴抗压强度/MPa	单轴抗拉强度/MPa
2400	60	0.26	116	10.9

下载: 导出CSV

表 7 单孔爆破试验钻孔装药参数

Table 7. Blasting parameters for single hole blasting test

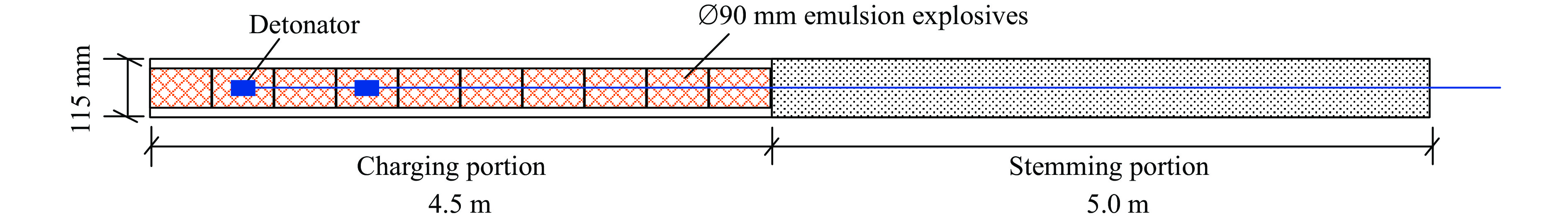
炮孔直径/mm	炮孔长度/m	炮孔倾角/(°)	药卷直径/mm	装药长度/m	单孔药量/kg	填塞长度/m
115	9.5	90	90	4.5	30	5.0

下载: 导出CSV

参考文献(41)

[1]	汪旭光. 中国工程爆破与爆破器材的现状及展望 [J]. 工程爆破, 2007, 13(4): 1–8. DOI: 10.3969/j.issn.1006-7051.2007.04.001. WANG X G. Current status and future prospect of engineering blasting and explosive materials in China [J]. Engineering Blasting, 2007, 13(4): 1–8. DOI: 10.3969/j.issn.1006-7051.2007.04.001.
[2]	WANG M L, LI X, LI Q H, et al. Study on blasting technology for open-pit layering of complex mine adjacent to high and steep slope [J]. Frontiers in Earth Science, 2021, 9: 773872. DOI: 10.3389/feart.2021.773872.
[3]	王志亮, 毕程程, 李鸿儒. 混凝土爆破损伤的SPH-FEM耦合法数值模拟 [J]. 爆炸与冲击, 2018, 38(6): 1419–1428. DOI: 10.11883/bzycj-2017-0209. WANG Z L, BI C C, LI H R. Numerical simulation of blasting damage in concrete using a coupled SPH-FEM algorithm [J]. Explosion and Shock Waves, 2018, 38(6): 1419–1428. DOI: 10.11883/bzycj-2017-0209.
[4]	杨建华, 代金豪, 姚池, 等. 爆破开挖扰动下锚固节理岩质边坡位移突变特征与能量机理 [J]. 爆炸与冲击, 2022, 42(3): 035201. DOI: 10.11883/bzycj-2021-0126. YANG J H, DAI J H, YAO C, et al. Displacement mutation characteristics and energy mechanisms of anchored jointed rock slopes under blasting excavation disturbance [J]. Explosion and Shock Waves, 2022, 42(3): 035201. DOI: 10.11883/bzycj-2021-0126.
[5]	HUSTRULID W. Blasting principles for open pit mining: theoretical foundations [M]. Rotterdam: Balkema, 1999: 992.
[6]	孙鹏昌. 基于模态分析的岩石高边坡爆破振动影响评价 [D]. 武汉: 武汉大学, 2021: 47–60. SUN P C. Impact evaluation of blasting vibration on high rock slope based on modal analysis [D]. Wuhan: Wuhan University, 2021: 47–60.
[7]	CASTEDO R, NATALE M, LÓPEZ L M, et al. Estimation of Jones-Wilkins-Lee parameters of emulsion explosives using cylinder tests and their numerical validation [J]. International Journal of Rock Mechanics and Mining Sciences, 2018, 112: 290–301. DOI: 10.1016/j.ijrmms.2018.10.027.
[8]	KUZMENKO A A, VOROBEV V D, DENISYUK I I, et al. Seismic effects of blasting in rock [M]. Rotterdam: A. A. Balkema, 1993: 35–37.
[9]	王文龙. 钻眼爆破 [M]. 北京: 煤炭工业出版社, 1984: 178–179. WANG W L. Drilling and blasting [M]. Beijing: China Coal Industry Publishing House, 1984: 178–179.
[10]	SHARPE J A. The production of elastic waves by explosion pressures: I. theory and empirical field observations [J]. Geophysics, 1942, 7(2): 144–154. DOI: 10.1190/1.1445002.
[11]	DUVALL W I. Strain-wave shapes in rock near explosions [J]. Geophysics, 1953, 18(2): 310–323. DOI: 10.1190/1.1437875.
[12]	CHO S H, MIYAKE H, KIMURA T, et al. Effect of the waveform of applied pressure on rock fracture process in one free-face [J]. Science and Technology of Energetic Materials, 2003, 64(3): 116–125.
[13]	CHO S H, KANEKO K. Influence of the applied pressure waveform on the dynamic fracture processes in rock [J]. International Journal of Rock Mechanics and Mining Sciences, 2004, 41(5): 771–784. DOI: 10.1016/j.ijrmms.2004.02.006.
[14]	STARFIELD A M, PUGLIESE J M. Compression waves generated in rock by cylindrical explosive charges: a comparison between a computer model and field measurements [J]. International Journal of Rock Mechanics and Mining Sciences & Geomechanics Abstracts, 1968, 5(1): 65–77. DOI: 10.1016/0148-9062(68)90023-5.
[15]	JONG Y, LEE C, JEON S, et al. Numerical modeling of the circular-cut using particle flaw code [C]//Proceedings of the 31st Annual Conference on Explosives and Blasting Technique. Orlando, 2005: 289–298.
[16]	BLAIR D, MINCHINTON A. On the damage zone surrounding a single blasthole [J]. Fragblast, 1997, 1(1): 59–72. DOI: 10.1080/13855149709408390.
[17]	BLAIR D P. A comparison of Heelan and exact solutions for seismic radiation from a short cylindrical charge [J]. Geophysics, 2007, 72(2): E33–E41. DOI: 10.1190/1.2424543.
[18]	CHO S H, KANEKO K. Rock fragmentation control in blasting [J]. Materials Transactions, 2004, 45(5): 1722–1730. DOI: 10.2320/matertrans.45.1722.
[19]	TRIVIÑO L F, MOHANTY B, MUNJIZA A. Seismic radiation patterns from cylindrical explosive charges by analytical and combined finite-discrete element methods [C]//Proceedings of the 9th International Symposium on Rock Fragmentation by Blasting. Boca Raton, FL, USA: CRC Press, 2009: 415–426.
[20]	陶振宇, 董振华, 卢文波. 敞口炮孔压力变化历程的理论分析与计算 [J]. 武汉水利电力大学学报, 1994, 27(4): 394–399. TAO Z Y, DONG Z H, LU W B. A theoretical analysis and calculation of the pressure course of unstemmed borehole [J]. Journal of Wuhan University of Hydraulic and Electric Engineering, 1994, 27(4): 394–399.
[21]	卢文波, 陶振宇. 爆生气体驱动的裂纹扩展速度研究 [J]. 爆炸与冲击, 1994, 14(3): 264–268. LU W B, TAO Z Y. A study of fracture propagation velocity driven by gases of explosion products [J]. Explosion and Shock Waves, 1994, 14(3): 264–268.
[22]	卢文波. 岩石爆破中应力波的传播及其效应研究 [D]. 武汉: 武汉水利电力大学, 1994: 15–24. LU W B. Propagation and effect of stress wave in rock blasting [D] Wuhan: Wuhan University of Hydraulic and Electric Engineering, 1994: 15–24.
[23]	LU W B, YANG J H, CHEN M, et al. An equivalent method for blasting vibration simulation [J]. Simulation Modelling Practice and Theory, 2011, 19(9): 2050–2062. DOI: 10.1016/j.simpat.2011.05.012.
[24]	杨建华, 卢文波, 陈明, 等. 岩石爆破开挖诱发振动的等效模拟方法 [J]. 爆炸与冲击, 2012, 32(2): 157–163. DOI: 10.11883/1001-1455(2012)02-0157-07. YANG J H, LU W B, CHEN M, et al. An equivalent simulation method for blasting vibration of surrounding rock [J]. Explosion and Shock Waves, 2012, 32(2): 157–163. DOI: 10.11883/1001-1455(2012)02-0157-07.
[25]	卢文波, 杨建华, 陈明, 等. 深埋隧洞岩体开挖瞬态卸荷机制及等效数值模拟 [J]. 岩石力学与工程学报, 2011, 30(6): 1089–1096. LU W B, YANG J H, CHEN M, et al. Mechanism and equivalent numerical simulation of transient release of excavation load for deep tunnel [J]. Chinese Journal of Rock Mechanics and Engineering, 2011, 30(6): 1089–1096.
[26]	李庆文, 乔兰, 陈璐. 基于精确爆破载荷分析的安全距离判据 [J]. 工程力学, 2015, 32(10): 123–129. DOI: 10.6052/j.issn.1000-4750.2014.03.0248. LI Q W, QIAO L, CHEN L. Based on the accurate blasting loading to estimate the safety criterion [J]. Engineering Mechanics, 2015, 32(10): 123–129. DOI: 10.6052/j.issn.1000-4750.2014.03.0248.
[27]	钱七虎. 岩石爆炸动力学的若干进展 [J]. 岩石力学与工程学报, 2009, 28(10): 1945–1968. DOI: 10.3321/j.issn:1000-6915.2009.10.001. QIAN Q H. Some advances in rock blasting dynamics [J]. Chinese Journal of Rock Mechanics and Engineering, 2009, 28(10): 1945–1968. DOI: 10.3321/j.issn:1000-6915.2009.10.001.
[28]	陈士海, 王明洋, 赵跃堂, 等. 岩石爆破破坏界面上的应力时程研究 [J]. 岩石力学与工程学报, 2003, 22(11): 1784–1788. DOI: 10.3321/j.issn:1000-6915.2003.11.006. CHEN S H, WANG M Y, ZHAO Y T, et al. Time-stress history on interface between cracked and uncracked zones under rock blasting [J]. Chinese Journal of Rock Mechanics and Engineering, 2003, 22(11): 1784–1788. DOI: 10.3321/j.issn:1000-6915.2003.11.006.
[29]	CHAPMAN D L, OXON A B. VI. On the rate of explosion in gases [J]. The London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science, 1899, 47(284): 90–104. DOI: 10.1080/14786449908621243.
[30]	JOUGUET E. On the propagation of chemical reactions in gases [J]. Journal de Mathématiques Pures et Appliquées, 1905, 1: 347–425.
[31]	HENRYCH J. The dynamics of explosion and its use: developments in civil engineering [M]. Amsterdam: Elsevier, 1979: 59–64.
[32]	Л. Д. 朗道, E. M. 栗弗席兹. 连续介质力学(第二册) [M]. 彭旭麟, 译. 北京: 人民教育出版社, 1960: 500–509.
[33]	WANG L L. Foundations of stress waves [M]. Oxford: Elsevier, 2007: 337–347.
[34]	李世愚, 和泰名, 尹祥础. 岩石断裂力学 [M]. 北京: 科学出版社, 2015: 23–25. LI S Y, HE T M, YIN X C. Rock fracture mechanics [M]. Beijing: Science Press, 2015: 23–25.
[35]	冷振东, 卢文波, 陈明, 等. 岩石钻孔爆破粉碎区计算模型的改进 [J]. 爆炸与冲击, 2015, 35(1): 101–107. DOI: 10.11883/1001-1455(2015)01-0101-07. LENG Z D, LU W B, CHEN M, et al. Improved calculation model for the size of crushed zone around blasthole [J]. Explosion and Shock Waves, 2015, 35(1): 101–107. DOI: 10.11883/1001-1455(2015)01-0101-07.
[36]	LU W B, LENG Z D, CHEN M, et al. A modified model to calculate the size of the crushed zone around a blast-hole [J]. Journal of the Southern African Institute of Mining and Metallurgy, 2016, 116(5): 413–422. DOI: 10.17159/2411-9717/2016/v116n5a7.
[37]	RAKISHEV B, RAKISHEVA Z B. Basic characteristics of the stages of rock massif destruction by explosive crushing [C]//Proceedings of the 3rd Asia-Pacific Symposium on Blasting Techniques. Kunming, 2011: 65–69.
[38]	MENCACCI S, CHAVEZ R. The measurement and analysis of detonation pressure during blasting [C]//Proceedings of 2005 European Federation of Explosives Engineers. Brighton, 2005: 231–237.
[39]	SUN P C, LU W B, ZHOU J R, et al. Comparison of dominant frequency attenuation of blasting vibration for different charge structures [J]. Journal of Rock Mechanics and Geotechnical Engineering, 2022, 14(2): 448–459. DOI: 10.1016/j.jrmge.2021.07.002.
[40]	SINGH P K, ROY M P. Damage to surface structures due to blast vibration [J]. International Journal of Rock Mechanics and Mining Sciences, 2010, 47(6): 949–961. DOI: 10.1016/j.ijrmms.2010.06.010.
[41]	LANGEFORS U, KIHLSTRÖM B. The modern technique of rock blasting [M]. 3rd ed. New York: Wiley, 1979: 28–56.