孔洞增长层裂模型的改进及其在模拟不同加载波形层裂实验结果方面的应用

张凤国; 王裴; 王言金; 胡建波

doi:10.11883/bzycj-2023-0218

孔洞增长层裂模型的改进及其在模拟不同加载波形层裂实验结果方面的应用

doi: 10.11883/bzycj-2023-0218

1.
北京应用物理与计算数学研究所，北京 100094
2.
中国工程物理研究院流体物理研究所冲击波物理与爆轰物理全国重点实验室，四川绵阳 621999

基金项目: 国家自然科学基金（12271054）

详细信息

作者简介:
张凤国（1969－　），男，硕士，研究员，zhang_fengguo@iapcm.ac.cn

中图分类号: O346.1
计量
- 文章访问数: 253
- HTML全文浏览量: 108
- PDF下载量: 101
- 被引次数: 0
出版历程
- 收稿日期: 2023-06-20
- 修回日期: 2023-10-08
- 网络出版日期: 2023-12-20
- 刊出日期: 2024-05-08

Improvement of void growth model and its application in simulating spallation experiments under different impact loading wave forms

1.
Institute of Applied Physics and Computational Mathematics, Beijing 100094, China
2.
National Key Laboratory of Shock Wave and Detonation Physics, Institute of Fluid Physics, China Academy of Engineering Physics, Mianyang 621999, Sichuan, China

摘要

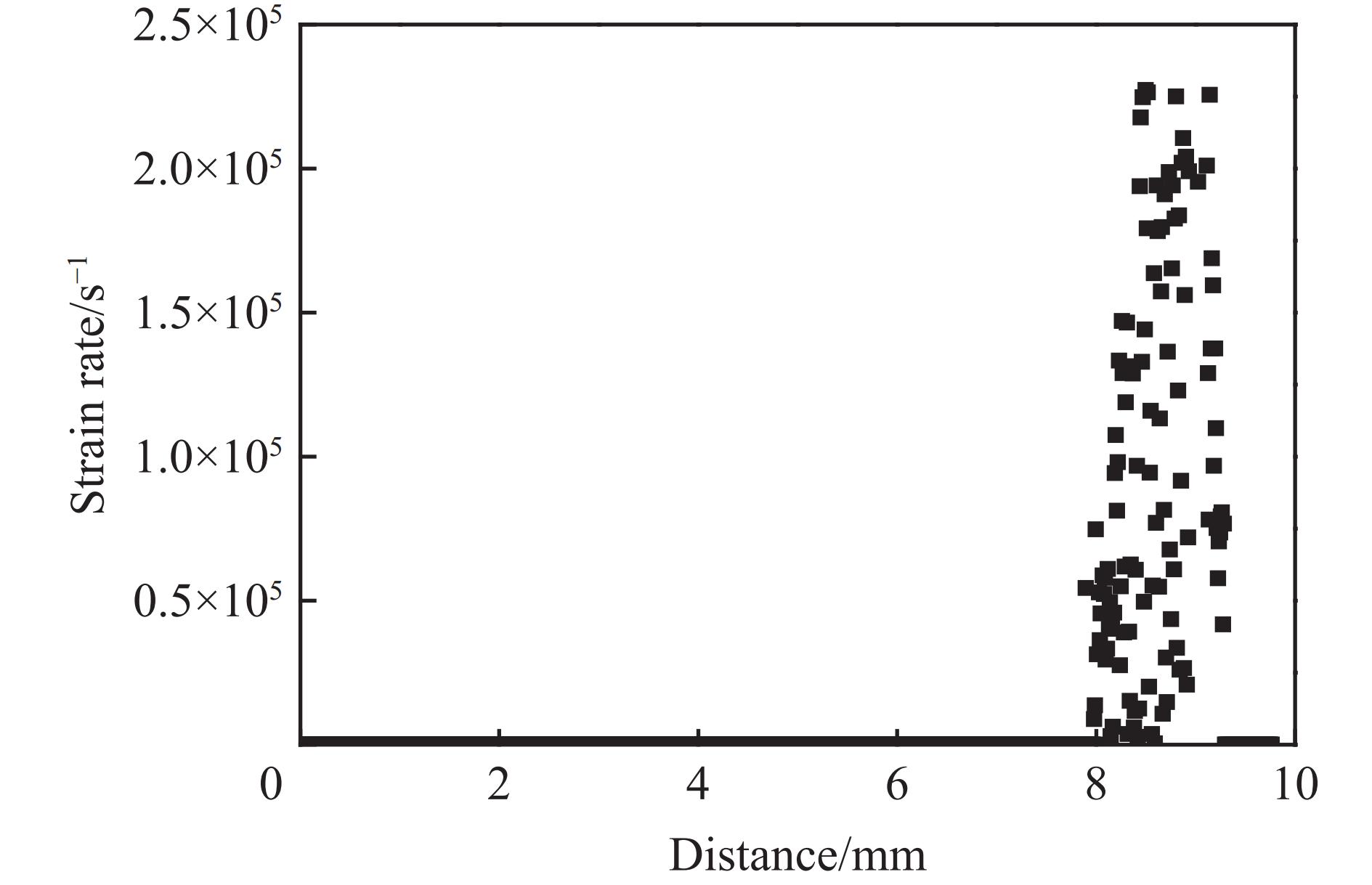
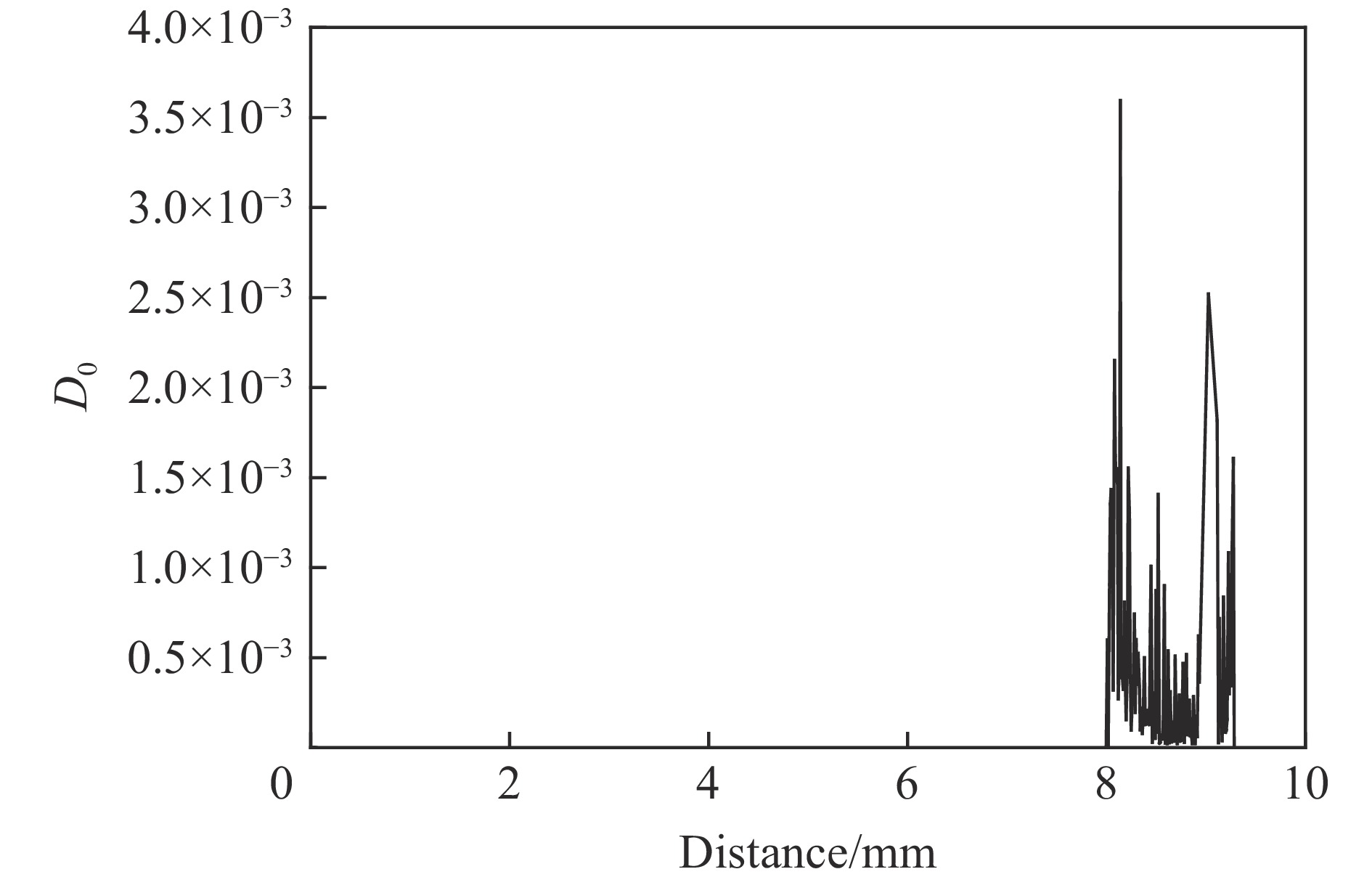
摘要: 冲击波在靶板自由面反射导致靶板材料内部产生动态拉伸层裂损伤是材料的典型损伤破坏形式之一，材料的初始微结构、冲击加载的强度和应变率、温度等因素直接影响材料内部的损伤演化过程。靶板自由面速度曲线变化间接反映材料内部损伤的演化过程，在层裂损伤物理模型研究方面，目前采用适宜的层裂损伤模型较好地模拟不同冲击加载波形下靶板自由面速度曲线的相关文献很少，主要借助实验手段探讨加载波形与自由面速度曲线变化以及层裂损伤演化过程之间的关联。对于孔洞增长层裂损伤模型，通过解析加载应变率与层裂强度以及损伤模型初始损伤参数之间的相互关系，给出了模型初始损伤参数的计算方法，有效地将损伤模型初始损伤参数与加载应变率关联在一起。该计算方法不仅可以较好地模拟方波、三角波以及泰勒波冲击加载铝材料层裂实验的自由面速度曲线，同时，计算得到的层裂强度和层裂片厚度也与实验结果符合。此外，还进一步分析了靶板内部不同位置的初始损伤、层裂强度的分布与应变率之间的关联，以及其对自由面速度曲线的影响。
- 冲击加载波形 /
- 层裂损伤 /
- 自由面速度 /
- 数值分析
Abstract: The dynamic tensile spallation damage caused by shock wave reflection on the free surface of target is one of the typical damage modes of materials. The initial microstructure of materials, the magnitude and strain rate of impact loading, temperature and other factors directly affect the spallation damage evolution process in materials. The change of free surface velocity c at the target indirectly reflects the evolution process of spall damage in materials. Regarding the study of physical model of spallation damage, only limited literatures are available on using suitable spallation damage model to simulate the free surface velocity profile of target under different impact loading waveforms. The relationship between loading waveforms and free surface velocity profile as well as the evolution process of spallation damage were mainly investigated by means of experiments. Considering that both the shear viscosity coefficient and the hardening coefficient are the basic parameters of the material, the calculation of the initial damage parameters of the damage model is now obtained by analyzing the relationship between the spall strength, the loading strain rate and the initial damage parameter of the damage model. The initial damage parameter of the damage model is effectively associated with the loading strain rate, and the programmed automatic calculation of the initial damage parameter under different loading strain rate is realized. On this basis, not only the free surface velocity profiles of spallation tests of aluminum materials loaded by square wave, triangular wave and Taylor wave can be well simulated, the calculated spall strengths and spall plate thicknesses are also consistent with the tests results. In addition, the relationship between the distribution of initial damage, spall strength and loading strain rate at different positions in the target is further anayzed. Consequently, compared with the existing damage model, the new method not only further improves the existing damage model, but also improves the validity of the calculation results. At the same time, it also provides ideas for improving other spall damage models.
- shock-wave profile shape /
- spall damage /
- free surface velocity /
- numerical analysis

HTML全文

图 1 实验1的自由面速度曲线与数值模拟结果

Figure 1. Simulations of free-surface velocity profiles and comparison with experimental dataset 1 on aluminum under square wave loading

下载: 全尺寸图片幻灯片

图 2 实验2的自由面速度曲线及数值模拟结果

Figure 2. Simulations of free-surface velocity profiles and comparison with experimental dataset 2on aluminum under triangular wave loading

下载: 全尺寸图片幻灯片

图 3 实验3的自由面速度曲线及数值模拟结果

Figure 3. Simulations of free-surface velocity profiles and comparison with experimental dataset 3 on aluminum under Taylor wave loading

下载: 全尺寸图片幻灯片

图 4 靶板内部应变率分布情况

Figure 4. Distribution of strain rate in target

下载: 全尺寸图片幻灯片

图 5 靶板内部初始损伤分布情况

Figure 5. Distribution of initial damage in target

下载: 全尺寸图片幻灯片

图 6 靶板内部层裂强度分布情况

Figure 6. Distribution of spall strength in target

下载: 全尺寸图片幻灯片

图 7 靶板内部损伤分布情况

Figure 7. Distribution of damage in target

下载: 全尺寸图片幻灯片

表 1 层裂实验的实验测量结果与数值计算结果

Table 1. Experiment data and calculation results for spall experiments

实验	层裂强度/GPa		层裂片厚度/mm		层裂面处初始孔隙度
实验	实验值	计算值	实验值	计算值	层裂面处初始孔隙度
1	1.07±0.04	1.11	1.23±0.10	1.15	1.000 29
2	1.06±0.04	1.05	0.54±0.05	0.58	1.000 50
3	0.91±0.08	0.89	0.61±0.06	0.66	1.002 52

下载: 导出CSV

参考文献(22)

[1]	SEAMAN L, CURRAN D R, SHOCKEY D A. Computational models for ductile and brittle fracture [J]. Journal of Applied Physics, 1976, 47: 4814–4826. DOI: 10.1063/1.322523.
[2]	JOHNSON J N. Dynamic fracture and spallation in ductile solids [J]. Journal of Applied Physics, 1981, 52(4): 2812–2825. DOI: 10.1063/1.329011.
[3]	IKKURTHI V R, CHATURVEDI S. Use of different damage models for simulating impact-driven spallation in metal plates [J]. International Journal of Impact Engineering, 2004, 30: 275–301. DOI: 10.1016/S0734-743X(03)00070-8.
[4]	JACQUES N, CZAMOTA C, MERCIER S, et al. A micromechanical constitutive model for dynamic damage and fracture of ductile materials [J]. International Journal of Fracture, 2010, 162: 159–175. DOI: 10.1007/s10704-009-9436-2.
[5]	MAYER A E, MAYER P N. Strain rate dependence of spall strength for solid and molten lead and tin [J]. International Journal of Fracture, 2020, 222: 171–195. DOI: 10.1007/s10704-020-00440-8.
[6]	WILKERSON J W. On the micromechanics of void dynamics at extreme rates [J]. International Journal of Plasticity, 2017, 95: 21–42. DOI: 10.1016/j.ijplas.2017.03.008.
[7]	CHEN X, ASAY J R, DWIVEDI S K, et al. Spall behavior of aluminum with varying microstructures [J]. Journal of Applied Physics, 2006, 99(2): 023528. DOI: 10.1063/1.2165409.
[8]	TONKS D L, THISSELLl W R, SCHWARZ D S. Modeling incipient copper damage data from the tensile Hopkinson bar and gas gun [C] // Shock Compression of Condensed Matter-2003. Portland, Oregon, USA: AIP Conference Proceedings, 2003: 507–510. DOI: 10.1063/1.1780288.
[9]	VIDEAU L, COMBIS P, LAFFITE S, et al. Laser-driven spall experiments in ductile materials in order to characterize Johnson fracture model constants [C] // Shock Compression of Condensed Matter-2011. Chicago Illinois, USA: AIP Conference Proceedings, 2011: 1011–1014. DOI: 10.1063/1.3686449.
[10]	翟少栋, 李英华, 彭建祥, 等. 平面碰撞与强激光加载下金属铝的层裂行为 [J]. 爆炸与冲击, 2016, 36(6): 767–773. DOI: 10.11883/1001-1455(2016)06-0767-07. ZHAI S D, LI Y H, PENG J X, et al. Spall behavior of pure aluminum under plate-impact and high energy laser shock loadings [J]. Explosion and Shock Waves, 2016, 36(6): 767–773. DOI: 10.11883/1001-1455(2016)06-0767-07.
[11]	JOHNSON J N, GRAY G T, BOUME N K. Effect of pulse duration and strain rate on incipient spall fracture in copper [J]. Journal of Applied Physics, 1999, 86: 4892–4901. DOI: 10.1063/1.371527.
[12]	KOLLER D D, HIXSON R S, GRAY III G T, et al. Influence of shock-wave profile shape on dynamically induced damage in high-purity copper [J]. Journal of Applied Physics, 2005, 98: 103518. DOI: 10.1063/1.2128493.
[13]	张凤国, 王裴, 王昆, 等. 关于延性金属材料层裂强度概念的解读 [J]. 防护工程, 2020, 42(5): 33–36. ZHANG F G, WANG P, WANG K, et al. Interpretation of the concept of spalling strength of ductile metal materials [J]. Protective Engineering, 2020, 42(5): 33–36.
[14]	张凤国, 刘军, 何安民, 等. 孔洞增长层裂损伤模型初始参数的确定方法及其应用 [J]. 物理学报, 2020, 69(20): 204601. DOI: 10.7498/aps.69.20200527. ZHANG F G, LIU J, HE A M, et al. Determination method of parameters of void growth damage model and its application to simulation of spall test [J]. Acta Physica Sinca, 2020, 69(20): 204601. DOI: 10.7498/aps.69.20200527.
[15]	张凤国, 刘军, 王言金, 等. 含氦泡辐照老化材料层裂损伤计算方法分析 [J]. 爆炸与冲击, 2023, 43(10): 103105. DOI: 10.11883/bzycj-2022-0486. ZHANG F G, LIU J, WANG Y J, et al. Simulation method of spall damage for self-radiation damage aging materials with helium bubbles [J]. Explosion and Shock Waves, 2023, 43(10): 103105. DOI: 10.11883/bzycj-2022-0486.
[16]	张凤国, 赵福祺, 刘军, 等. 延性金属层裂强度对温度、晶粒尺寸和加载应变率的依赖特性及其物理建模 [J]. 物理学报, 2022, 71: 034601. DOI: 10.7498/aps.71.20210702. ZHANG F G, ZHAO F Q, LIU J, et al. Spall strength dependence on temperature, grain size and strain rate in pure ductile metals [J]. Acta Physica Sinca, 2022, 71: 034601. DOI: 10.7498/aps.71.20210702.
[17]	RAZORENOV S V, KANEL G I. Spall strength of metals over a wide range of magnitudes and durations of shock load [R]. Chernogolovka, Russia: Institute of Chemical Physics, 1986: 46–49.
[18]	KANEL G I, RAZORENOV S V, FORTOV V E. Kinetics of spallation rupture in the aluminum alloy AMg6M [J]. Journal of Applied Mechanics and Technical Physics, 1984, 25(5): 707–711. DOI: 10.1007/BF00909372.
[19]	WILKERSON J W, RAMESH K T. Unraveling the anomalous grain size dependence of cavitation [J]. Physics Review Letter, 2016, 117(21): 215503. DOI: 10.1103/PhysRevLett.117.215503.
[20]	NGUYEN T, LUSCHER D J, WILKERSON J W. A physics-based model and simple scaling law to predict the pressure dependence of single crystal spall strength [J]. Journal of the Mechanics and Physics of Solids, 2020, 137: 103875. DOI: 10.1016/j.jmps.2020.103875.
[21]	ROMANCHENKO V I, STEPANOV G V. The dependence of critical stresses upon the time parameters of load at spalling in copper, aluminum and steel [J]. Journal of Applied Mechanics Technical Physics, 1980, 21(4): 141–147. DOI: 10.1007/BF00916495.
[22]	WU X Y, RAMESH K T, WRIGHT T W. The dynamic growth of a single void in a viscoplastic material under transient hydrostatic loading [J]. Journal of the Mechanics and Physics of Solids, 2003, 51: 1–26. DOI: 10.1016/S0022-5096(02)00079-0.