Dynamic responses of metallic hierarchical corrugated sandwich beams under shock loadings
-
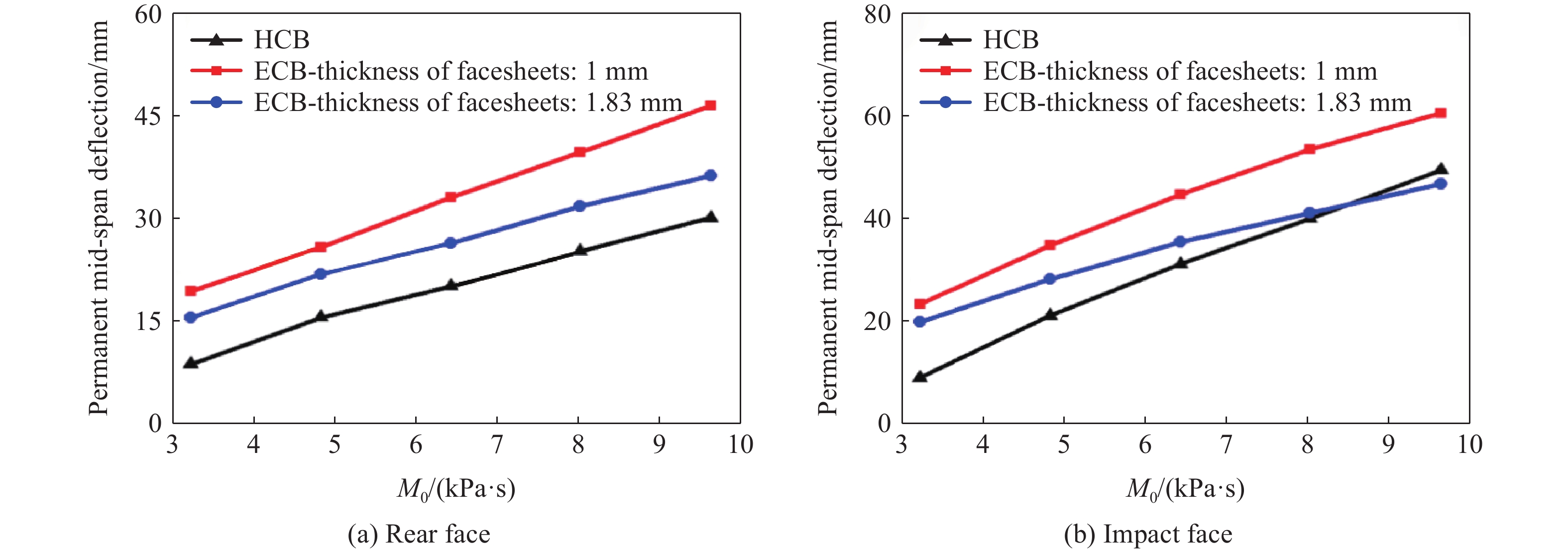
摘要: 为了研究采用多级夹层设计的金属多级波纹芯体夹层梁在泡沫子弹冲击下的动态力学行为，在验证数值方法可靠性的基础上，通过 Abaqus-Explicit 仿真分析了不同子弹动量水平下金属多级波纹芯体夹层梁的动态变形过程、定量挠度结果、变形破坏模式和能量吸收特性。进一步地，设计了3种不同几何参数的单层波纹夹层结构，比较了单层和多级波纹夹层结构在等质量条件下的抗冲击性能差异。结果表明，多级波纹夹层梁冲击侧夹层面板的二级波纹芯体和一级波纹芯体的压溃程度始终大于背侧夹层面板二级波纹芯体的压溃程度。多级波纹夹层梁背侧面板的最终跨中挠度始终小于等质量单级波纹夹层梁的相应挠度，体现出多级夹层梁的抗冲击防护性能优势。这种增强机理主要在于增加的多孔芯体压缩吸能保护了背侧面板，另外，多级夹层梁的塑性轴向拉伸强度几乎保持不变，而塑性弯曲强度因梁结构总厚度增加而增大，从而扩大了夹层结构的塑性屈服面。Abstract: The dynamic mechanical behavior of a metallic hierarchical corrugated sandwich beam subjected to foam projectile impact was systematically studied. After verifying the reliability of the numerical method, the dynamic deformation evolution, quantitative deflection results, deformation failure modes, and energy absorption characteristics of the metallic hierarchical corrugated sandwich beam under different projectile momentum levels were analyzed using Abaqus-Explicit simulations. Subsequently, three metallic single-layer empty corrugated sandwich beams with different geometric parameters were designed, aiming to compare the shock resistance between single-layer and hierarchical corrugated sandwich beams under equal mass conditions. The results showed that the degree of crushing of the secondary corrugated core on the impact side and the first-order corrugated core of the hierarchical sandwich beam was always greater than that of the rear sandwich’s secondary corrugated core. The final mid-span deflection of the rear face of the hierarchical corrugated sandwich beam was always smaller than the corresponding deflection value of the equivalent mass single-level empty corrugated sandwich beam, demonstrating the superior impact protection performance of the hierarchical sandwich beam. This enhancement mechanism is mainly attributed to the increased energy absorption because of the added cellular cores, which protects the rear face sheet. Besides, the plastic longitudinal stretching strength of the hierarchical sandwich beam remains almost unchanged, while the plastic bending strength increases due to the increase in the total beam thickness, thereby enlarging the plastic yield surface of the sandwich structure.
-
图 3 单级波纹夹层梁（左）和多级波纹夹层梁（右）泡沫子弹冲击有限元模型
Figure 3. Finite element model of foam projectile impact on the single-level empty corrugated sandwich beam (left) and the hierarchical corrugated sandwich beam (right)
图 4 单级波纹夹层梁的前后面板跨中挠度时程曲线的结果可靠性验证
Figure 4. Validation of mid-span deflection time histories of impact and rear face sheets for single-level empty corrugated sandwich beam
图 5 单级波纹夹层梁在不同子弹动量作用下前后面板最终跨中挠度值的数值仿真结果与实验结果对比
Figure 5. Comparison of numerical and experimental results for permanent mid-span deflection values of impact and rear face sheets of single-level empty corrugated sandwich beams under different projectile momentum
图 6 单级波纹夹层梁在不同子弹动量作用下最终变形模式的数值仿真结果与实验结果对比
Figure 6. Comparison of numerical and experimental results for final deformation modes of single-level empty corrugated sandwich beams under different projectile momentum
图 7 泡沫子弹冲击夹层结构的冲量传递过程
Figure 7. Impulse transfer process of foam projectile impacting sandwich structures
图 8 模拟计算得到的多级波纹夹层梁在泡沫子弹冲击下的动态变形过程
Figure 8. Numerical simulated structural evolution of hierarchical corrugated sandwich beams under foam projectile impacts
图 9 不同子弹初始动量水平下多级波纹夹层梁背侧面板跨中挠度时程曲线
Figure 9. Mid-span deflection time histories of the rear face of hierarchical corrugated sandwich beams under different projectile initial momentum
图 10 不同子弹初始动量下多级波纹夹层梁（HCB）和两种不同厚度面板的单级波纹夹层梁（ECB）最终跨中挠度
Figure 10. Mid-span deflection of the hierarchical corrugated sandwich beam (HCB) and two kinds of single-level empty corrugated sandwich beams with different face thicknesses (ECB) impacted by projectiles with different momentum
图 11 梁ECB-S1（左）和ECB-S2（右）的几何图例
Figure 11. Geometry of ECB-S1 (left) and ECB-S2 (right)
图 12 不同子弹初始动量下多级波纹夹层梁（HCB）和等质量等梁高单级波纹夹层梁（ECB）的最终跨中挠度
Figure 12. Mid-span deflection of the hierarchical corrugated sandwich beam (HCB) and two kinds of single-level empty corrugated sandwich beams with the mass and beam height same to the HCB (ECB) impacted by projectiles with different momentum
图 13 多级波纹夹层梁在不同动量水平冲击条件下的最终变形破坏模式
Figure 13. Final deformation modes of hierarchical corrugated sandwich beams under different projectile momentum
图 14 多级波纹夹层梁各部件最终塑性应变能及能量比率对比
Figure 14. Final plastic strain energy and energy ratio of each part of hierarchical corrugated sandwich beams
表 1 多级波纹夹层结构几何尺寸
Table 1. Geometric parameters of the hierarchical sandwich beam
L/mm W/mm lc1/mm lp1/mm tc1/mm α1/(°) lc2/mm lp2/mm tc2/mm α2/(°) tf2/mm 300 60 20 5 0.5 60 8 2 0.5 60 0.5 
下载: 导出CSV
表 2 单层波纹夹层梁ECB-S1和ECB-S2的几何参数
Table 2. The geometry parameters of the empty corrugated sandwich beams ECB-S1 and ECB-S2
梁 lp/mm lc/mm tc/mm α/(°) tf/mm ECB-S1 9.12 36.46 2.10 60 0.5 ECB-S2 5.00 34.88 1.31 73.34 0.5
下载: 导出CSV
-
[1] RATHBUN H J, RADFORD D D, XUE Z, et al. Performance of metallic honeycomb-core sandwich beams under shock loading [J]. International Journal of Solids and Structures, 2006, 43(6): 1746–1763. DOI: 10.1016/j.ijsolstr.2005.06.079. [2] YUE Z S, WANG X, HE C, et al. Elevated shock resistance of all-metallic sandwich beams with honeycomb-supported corrugated cores [J]. Composites Part B: Engineering, 2022, 242: 110102. DOI: 10.1016/j.compositesb.2022.110102. [3] WANG X, HE C, YUE Z S, et al. Shock resistance of elastomer-strengthened metallic corrugated core sandwich panels [J]. Composites Part B: Engineering, 2022, 237: 109840. DOI: 10.1016/j.compositesb.2022.109840. [4] REN J W, ZHOU Y L, QIANG L S, et al. Enhancing impact resistance of metallic foam core sandwich constructions through encasing high-strength fibrous composites [J]. Thin-Walled Structures, 2024, 196: 111546. DOI: 10.1016/j.tws.2023.111546. [5] ZHANG D J, ZHAO Z Y, GAO H Y, et al. Dynamic response of sandwich panel attached with a double mass-spring-damping system to shallow-buried explosion: analytical modeling [J]. Science China Technological Sciences, 2024, 67(2): 568–586. DOI: 10.1007/s11431-023-2375-0. [6] 习会峰, 黄丽琴, 余同希, 等. 拓扑互锁结构研究现状及展望 [J]. 应用力学学报, 2023, 40(2): 241–252. DOI: 10.11776/j.issn.1000-4939.2023.02.001.XI H F, HUANG L Q, YU T X, et al. A review on the studies of topological interlocking structures [J]. Chinese Journal of Applied Mechanics, 2023, 40(2): 241–252. DOI: 10.11776/j.issn.1000-4939.2023.02.001. [7] YUE Z S, HAN B, WANG Z Y, et al. Data-driven multi-objective optimization of ultralight hierarchical origami-corrugation meta-sandwich structures [J]. Composite Structures, 2023, 303: 116334. DOI: 10.1016/j.compstruct.2022.116334. [8] 吴文旺, 夏热. 轻质点阵超结构设计及多功能力学性能调控方法 [J]. 力学进展, 2022, 52(3): 673–718. DOI: 10.6052/1000-0992-22-002.WU W W, XIA R. Design of lightweight lattice meta-structures and approaches to manipulate their multi-functional mechanical properties [J]. Advances in Mechanics, 2022, 52(3): 673–718. DOI: 10.6052/1000-0992-22-002. [9] CÔTÉ F, DESHPANDE V S, FLECK N A, et al. The compressive and shear responses of corrugated and diamond lattice materials [J]. International Journal of Solids and Structures, 2006, 43(20): 6220–6242. DOI: 10.1016/j.ijsolstr.2005.07.045. [10] RADFORD D D, FLECK N A, DESHPANDE V S. The response of clamped sandwich beams subjected to shock loading [J]. International Journal of Impact Engineering, 2006, 32(6): 968–987. DOI: 10.1016/j.ijimpeng.2004.08.007. [11] HAN B, YUE Z S, WU H, et al. Superior compressive performance of hierarchical origami-corrugation metallic sandwich structures based on selective laser melting [J]. Composite Structures, 2022, 300: 116181. DOI: 10.1016/j.compstruct.2022.116181. [12] RUBINO V, DESHPANDE V S, FLECK N A. The collapse response of sandwich beams with a Y-frame core subjected to distributed and local loading [J]. International Journal of Mechanical Sciences, 2008, 50(2): 233–246. DOI: 10.1016/j.ijmecsci.2007.07.007. [13] RUBINO V, DESHPANDE V S, FLECK N A. The dynamic response of end-clamped sandwich beams with a Y-frame or corrugated core [J]. International Journal of Impact Engineering, 2008, 35(8): 829–844. DOI: 10.1016/j.ijimpeng.2007.10.006. [14] ZHANG P, CHENG Y S, LIU J, et al. Experimental and numerical investigations on laser-welded corrugated-core sandwich panels subjected to air blast loading [J]. Marine Structures, 2015, 40: 225–246. DOI: 10.1016/j.marstruc.2014.11.007. [15] LIBOVE C, HUBKA R E. Elastic constants for corrugated-core sandwich plates [R]. Washington: National Advisory Committee for Aeronautics, 1951. [16] LOK T S, CHENG Q H, JACOB B P. Equivalent stiffness parameters of truss-core sandwich panel [C]//Proceedings of the Ninth International Offshore and Polar Engineering Conference. Brest, France: International Society of Offshore and Polar Engineers, 1999: 292–298. [17] BUANNIC N, CARTRAUD P, QUESNEL T. Homogenization of corrugated core sandwich panels [J]. Composite Structures, 2003, 59(3): 299–312. DOI: 10.1016/S0263-8223(02)00246-5. [18] CHANG W S, VENTSEL E, KRAUTHAMMER T, et al. Bending behavior of corrugated-core sandwich plates [J]. Composite Structures, 2005, 70(1): 81–89. DOI: 10.1016/j.compstruct.2004.08.014. [19] LU T J, ZHU G. The elastic constants of corrugated board panels [J]. Journal of Composite Materials, 2001, 35(20): 1868–1887. DOI: 10.1177/002199801772661498. [20] PENG L X, LIEW K M, KITIPORNCHAI S. Analysis of stiffened corrugated plates based on the FSDT via the mesh-free method [J]. International Journal of Mechanical Sciences, 2007, 49(3): 364–378. DOI: 10.1016/j.ijmecsci.2006.08.018. [21] SAMANTA A, MUKHOPADHYAY M. Finite element static and dynamic analyses of folded plates [J]. Engineering Structures, 1999, 21(3): 277–287. DOI: 10.1016/S0141-0296(97)90172-3. [22] 彭林欣, 严世涛, 杨绿峰. 波纹夹层板自由振动的移动最小二乘无网格法 [J]. 广西大学学报(自然科学版), 2010, 35(5): 703–710. DOI: 10.13624/j.cnki.issn.1001-7445.2010.05.016.PENG L X, YAN S T, YANG L F. Free vibration analysis of corrugated-core sandwich plate structures by the moving-least square meshfree method [J]. Journal of Guangxi University (Natural Science Edition), 2010, 35(5): 703–710. DOI: 10.13624/j.cnki.issn.1001-7445.2010.05.016. [23] NORDSTRAND T M. Parametric study of the post-buckling strength of structural core sandwich panels [J]. Composite Structures, 1995, 30(4): 441–451. DOI: 10.1016/0263-8223(94)00066-2. [24] LU T J, CHEN C, ZHU G. Compressive behaviour of corrugated board panels [J]. Journal of Composite Materials, 2001, 35(23): 2098–2126. DOI: 10.1177/002199801772661371. [25] VALDEVIT L, WEI Z, MERCER C, et al. Structural performance of near-optimal sandwich panels with corrugated cores [J]. International Journal of Solids and Structures, 2006, 43(16): 4888–4905. DOI: 10.1016/j.ijsolstr.2005.06.073. [26] TILBROOK M T, RADFORD D D, DESHPANDE V S, et al. Dynamic crushing of sandwich panels with prismatic lattice cores [J]. International Journal of Solids and Structures, 2007, 44(18/19): 6101–6123. DOI: 10.1016/j.ijsolstr.2007.02.015. [27] HOU S J, ZHAO S Y, REN L L, et al. Crashworthiness optimization of corrugated sandwich panels [J]. Materials & Design, 2013, 51: 1071–1084. DOI: 10.1016/j.matdes.2013.04.086. [28] ST-PIERRE L, DESHPANDE V S, FLECK N A. The low velocity impact response of sandwich beams with a corrugated core or a Y-frame core [J]. International Journal of Mechanical Sciences, 2015, 91: 71–80. DOI: 10.1016/j.ijmecsci.2014.02.014. [29] ST-PIERRE L, FLECK N A, DESHPANDE V S. The dynamic indentation response of sandwich panels with a corrugated or Y-frame core [J]. International Journal of Mechanical Sciences, 2015, 92: 279–289. DOI: 10.1016/j.ijmecsci.2014.11.021. [30] RUBINO V, DESHPANDE V S, FLECK N A. The dynamic response of clamped rectangular Y-frame and corrugated core sandwich plates [J]. European Journal of Mechanics-A/Solids, 2009, 28(1): 14–24. DOI: 10.1016/j.euromechsol.2008.06.001. [31] ZHANG P, LIU J, CHENG Y S, et al. Dynamic response of metallic trapezoidal corrugated-core sandwich panels subjected to air blast loading—an experimental study [J]. Materials & Design (1980-2015), 2015, 65: 221–230. DOI: 10.1016/j.matdes.2014.08.071. [32] LIU K, KE L, SHA Y Y, et al. Dynamic response of laser-welded corrugated sandwich panels subjected to plane blast wave [J]. International Journal of Impact Engineering, 2022, 164: 104203. DOI: 10.1016/j.ijimpeng.2022.104203. [33] WANG X, YUE Z S, XU X, et al. Ballistic impact response of elastomer-retrofitted corrugated core sandwich panels [J]. International Journal of Impact Engineering, 2023, 175: 104545. DOI: 10.1016/j.ijimpeng.2023.104545. [34] WANG X, YU R P, ZHANG Q C, et al. Dynamic response of clamped sandwich beams with fluid-filled corrugated cores [J]. International Journal of Impact Engineering, 2020, 139: 103533. DOI: 10.1016/j.ijimpeng.2020.103533. [35] YU R P, WANG X, ZHANG Q C, et al. Effects of sand filling on the dynamic response of corrugated core sandwich beams under foam projectile impact [J]. Composites Part B: Engineering, 2020, 197: 108135. DOI: 10.1016/j.compositesb.2020.108135. [36] ZHANG P, CHENG Y S, LIU J, et al. Experimental study on the dynamic response of foam-filled corrugated core sandwich panels subjected to air blast loading [J]. Composites Part B: Engineering, 2016, 105: 67–81. DOI: 10.1016/j.compositesb.2016.08.038. [37] KOOISTRA G W, DESHPANDE V, WADLEY H N G. Hierarchical corrugated core sandwich panel concepts [J]. Journal of Applied Mechanics, 2007, 74(2): 259–268. DOI: 10.1115/1.2198243. [38] FARROKHABADI A, TAGHIZADEH S A, MADADI H, et al. Experimental and numerical analysis of novel multi-layer sandwich panels under three point bending load [J]. Composite Structures, 2020, 250: 112631. DOI: 10.1016/j.compstruct.2020.112631. [39] GRYGOROWICZ M, PACZOS P, WITTENBECK L, et al. Experimental three-point bending of sandwich beam with corrugated core [J]. AIP Conference Proceedings, 2015, 1648(1): 800002. DOI: 10.1063/1.4913003. [40] WITTENBECK L, GRYGOROWICZ M, PACZOS P. Numerical analysis of sandwich beam with corrugated core under three-point bending [J]. AIP Conference Proceedings, 2015, 1648(1): 800007. DOI: 10.1063/1.4913008. [41] LAKES R. Materials with structural hierarchy [J]. Nature, 1993, 361(6412): 511–515. DOI: 10.1038/361511a0. [42] FRATZL P, WEINKAMER R. Nature’s hierarchical materials [J]. Progress in Materials Science, 2007, 52(8): 1263–1334. DOI: 10.1016/j.pmatsci.2007.06.001. [43] GIBSON L J. Biomechanics of cellular solids [J]. Journal of Biomechanics, 2005, 38(3): 377–399. DOI: 10.1016/j.jbiomech.2004.09.027. [44] 搜狗百科. 建筑艺术: 建筑的文化与审美价值 [EB/OL]. [2023-08-17]. https://baike.sogou.com/v64536923.htm?ch=zhihu.topic. [45] RADFORD D D, DESHPANDE V S, FLECK N A. The use of metal foam projectiles to simulate shock loading on a structure [J]. International Journal of Impact Engineering, 2005, 31(9): 1152–1171. DOI: 10.1016/j.ijimpeng.2004.07.012. [46] RADFORD D D, MCSHANE G J, DESHPANDE V S, et al. The response of clamped sandwich plates with metallic foam cores to simulated blast loading [J]. International Journal of Solids and Structures, 2006, 43(7/8): 2243–2259. DOI: 10.1016/j.ijsolstr.2005.07.006. [47] YAHAYA M A, RUAN D, LU G, et al. Response of aluminium honeycomb sandwich panels subjected to foam projectile impact—an experimental study [J]. International Journal of Impact Engineering, 2015, 75: 100–109. DOI: 10.1016/j.ijimpeng.2014.07.019. [48] JING L, WANG Z H, ZHAO L M. The dynamic response of sandwich panels with cellular metal cores to localized impulsive loading [J]. Composites Part B: Engineering, 2016, 94: 52–63. DOI: 10.1016/j.compositesb.2016.03.035. [49] DESHPANDE V S, FLECK N A. Isotropic constitutive models for metallic foams [J]. Journal of the Mechanics and Physics of Solids, 2000, 48(6/7): 1253–1283. DOI: 10.1016/S0022-5096(99)00082-4. [50] FLECK N A, DESHPANDE V S. The resistance of clamped sandwich beams to shock loading [J]. Journal of Applied Mechanics, 2004, 71(3): 386–401. DOI: 10.1115/1.1629109. 期刊类型引用(36)
1. 何理，殷琳，钟冬望，张鑫玥，赵永明，熊海涛，陈莎莎，NJAMBA Bruno. 爆破振动强度、波形与频谱研究综述：预测及主动控制. 爆破. 2024(03): 189-204+262 . 
百度学术2. 王士明，周发明，刘聪. 浅孔控制爆破技术在环境和地质双复杂条件下基坑开挖工程中的应用. 煤矿爆破. 2024(04): 33-37 . 百度学术3. 汤松，李立峰，郑雅风，赵渊，仲鹏，王淼. 基于改进的线性叠加法和岩石破碎效果的最优延期时间分析. 爆破. 2023(03): 12-19 . 百度学术4. 杨璐瑶，莫宏毅，王雪松，全铭，徐振洋. 应力叠加下的爆破减振效应现场试验研究. 金属矿山. 2023(10): 24-30 . 百度学术5. 白晓杰，耿新宇，蔺海洋，董法，春坚超，陈亚军. 非均质复杂岩体深凹露天煤矿爆破参数优选确定. 煤炭技术. 2023(12): 46-49 . 百度学术6. 赵民强. 下穿山岭隧道爆破振动效应的数值模拟分析. 科技与创新. 2023(24): 95-97 . 百度学术7. 项荣军，刘传鹏，李胜林，凌天龙. 隧道内部爆破振动传播规律与降振技术研究. 爆破. 2023(04): 82-88+200 . 百度学术8. 龚敏，曹贞洋，石发才，吴昊骏，吴晓东，周世均. 双临空面条件下隧道爆破近区振动波形构造与应用. 振动与冲击. 2022(01): 52-59+97 . 百度学术9. 谢先启，黄小武，姚颖康，何理，伍岳. 露天深孔台阶精细爆破技术研究进展. 金属矿山. 2022(07): 7-18 . 百度学术10. 何理，杨仁树，钟冬望，解联库，张奎，杨磊. 非电起爆网路修正单响药量计算及PPV预测应用实例. 振动与冲击. 2022(15): 54-62 . 百度学术11. 黄强. 隧道爆破振动场中断层对岩体远场振动特性影响研究. 铁道建筑技术. 2022(09): 55-59+80 . 百度学术12. 赵岩，王小敬，王海龙，王东升. 交叉隧道爆破振速回归分析及对比研究. 工程爆破. 2022(05): 121-127 . 百度学术13. 褚怀保，余梦飞，严少洋，王昌，孙博. 高压气体爆破与炸药爆破振动试验研究. 爆破. 2022(04): 177-185 . 百度学术14. 林飞. 复杂环境下爆破降振的微差时间优选. 金属矿山. 2021(04): 59-63 . 百度学术15. 孙鹏昌，卢文波，雷振，陈明，李瑞泽，李福千. 单薄山体岩质高边坡爆破振动响应分析及安全控制. 岩土工程学报. 2021(05): 877-885 . 百度学术16. 关振长，朱凌枫，俞伯林. 隧道掘进排孔爆破的精细化数值模拟. 振动与冲击. 2021(11): 154-162 . 百度学术17. 李永刚，马修胜，马修利. 露天煤矿剥离台阶降低大块率爆破方法研究. 科学技术创新. 2021(19): 102-103 . 百度学术18. 李祥龙，张其虎，王建国，杨德源，李斌，朱兴彪. 地下爆破精确延时逐孔起爆减振试验研究. 黄金科学技术. 2021(03): 401-410 . 百度学术19. 包松，郭连军，莫宏毅，徐振洋. 考虑高程与能量的爆破振动持时分析. 金属矿山. 2021(08): 67-70 . 百度学术20. 王子明，闫建文，杨振军. 洞室爆破振动信号时频分析及能量分布研究. 西安理工大学学报. 2021(02): 261-268 . 百度学术21. 何理，杨仁树，钟东望，李鹏，吴春平，陈江伟. 毫秒延时爆破等效单响药量计算及振速预测. 爆炸与冲击. 2021(09): 132-144 . 
本站查看22. 何理，谢先启，韩传伟，钟冬望，王洪刚，黄小武，黄炳林. 基于地震波频谱分析与线性叠加的电子雷管延时优选. 金属矿山. 2021(11): 41-48 . 百度学术23. 余良松，周龙杰，胡英国，胡伟. 两河口水电站级配料开采爆破孔间延时优选的试验研究. 爆破. 2021(04): 81-88 . 百度学术24. 杨茂森，陈永祥，郝润华. 露天煤矿超高台阶抛掷爆破振动效应评价. 爆破. 2021(04): 156-162 . 百度学术25. 孙冰，罗志业，曾晟，何旺. 爆破振动影响因素及控制技术研究现状. 矿业安全与环保. 2021(06): 129-134 . 百度学术26. 李猛，范延静，李彬，潘建荣，王湛. 爆破振动对高层框架-剪力墙结构的影响. 工程爆破. 2021(06): 26-31 . 百度学术27. 何山，郭剑锋，韩全吉. 山岭隧道微差爆破技术在周边建筑物保护中的应用. 科技通报. 2019(01): 15-18 . 百度学术28. 陈亚军，常治国，赵斐. 干旱区露天矿爆破作用线理论与实践研究. 采矿与安全工程学报. 2019(02): 357-363 . 百度学术29. 叶海旺，袁尔君，雷涛，龙梅. 基于量纲分析的爆破振动质点峰值速度预测公式. 金属矿山. 2019(05): 56-61 . 百度学术30. 相志斌，杨仕教，蒲成志，朱忠华，郑建礼. 基于爆破振动对孔间微差时间的确定. 中国矿业. 2019(11): 123-127 . 百度学术31. 王伟，李兴华，陈作彬，范磊，孙飞. 基于小波包变换的爆破振动信号能量熵特征分析. 爆破器材. 2019(06): 19-23 . 百度学术32. 李祥龙，骆浩浩，胡辉，张松涛，李克钢. 延期时间对高台阶抛掷爆破效果的影响. 北京理工大学学报. 2018(06): 579-584 . 百度学术33. 张绍银，王瑞鹏，吴顺川，赵革. 露天矿边坡爆破振动高程效应分析. 化工矿物与加工. 2018(01): 35-39 . 百度学术34. 张景华，刘钟阳. 露天采矿爆破对中缅油气管道振动的影响试验. 油气储运. 2018(07): 816-821 . 百度学术35. 陈士海，吴建. 双孔微差及长柱药包爆破振动数值模拟研究. 爆破. 2017(03): 46-52 . 百度学术36. 何理，钟冬望，陈晨，黄雄. 岩质高边坡开挖施工的爆破振动监测与分析. 金属矿山. 2017(01): 6-10 . 百度学术其他类型引用(28)
-
百度学术
计量
- 文章访问数: 332
- HTML全文浏览量: 88
- PDF下载量: 70
- 被引次数: 64