An assessment method for ammunition damage effectiveness based on multiple rectangular cookie cutter function
-
摘要: 为了快速准确地评估弹药对目标的毁伤效能,提出了一种基于多矩形饼切函数的弹药毁伤效能评估方法。该方法采用梯形法则、分区等效的思想,可以较大程度地保留实际毁伤区域中毁伤概率值的分布规律,从而保证计算的准确度。通过算例分析,研究了弹药落角和投放精度对目标平均毁伤概率的影响,并与基于矩形饼切和卡尔顿毁伤函数方法的结果进行了比较。结果表明,在弹药落角范围为30°~75°及弹药投放精度(circular error probable, CEP)范围为5~50 m时,与矩形饼切毁伤函数相比,基于多矩形饼切毁伤函数的计算方法使毁伤效能计算精度最大提高了26.4%;同时,与卡尔顿毁伤函数相比,计算效率提高了518倍。Abstract: When assessing the damage effectiveness of blast-fragmentation ammunitions against ground targets, the traditional approach involves calculating the overall target damage probability based on component damage criteria. Typically, the shooting line tracing method is used to determine the specific location on the target where fragments from the munition hit. However, this computation process is time-consuming. Therefore, to rapidly and accurately evaluate the ammunition damage effectiveness on the target, this study proposes a method called the multiple rectangular cookie cutter damage function. This method adopts the concept of the trapezoidal rule and performs equivalent processing on different regions corresponding to different damage probability intervals based on the gradient of damage probability changes within the actual damage area. This method can effectively retain the distribution pattern of damage probability values in the practical damage area, thus ensuring the accuracy of the computations. When describing ammunition delivery accuracy, a two-dimensional normal distribution is commonly employed to simulate the impact point locations of projectiles. Therefore, when calculating the mean of damage probability of the ammunition on the target, integration operations on the normal distribution function are necessary. However, due to the absence of an analytical solution for integrating the normal distribution function, polynomial equations are introduced as substitutes to enhance computational efficiency. The effects of ammunition drop angle and accuracy on the mean of damage probability of the target were investigated through example analysis, and the results were compared with those of methods based on the rectangular cookie cutter and Carlton damage function. The results show that within the ammunition drop angle range from 30° to 75°, and the circular error probable (CEP) precision range from 5 m to 50 m, compared with the rectangular cookie cutter damage function, the calculation method based on the multiple rectangular cookie cutter damage function improves the accuracy of damage effectiveness calculation by up to 26.4%. At the same time, the computational efficiency is improved by a factor of 518 compared with the Carlton damage function.
-
Key words:
- damage effectiveness /
- damage function /
- trapezoidal rule /
- calculation accuracy
-
弹药毁伤效能评估[1-4]是战斗部设计的重要环节,是弹药使用决策及火力规划的基础,对确定打击方案、提高作战效果和保障作战成功具有重要意义。破片式战斗部主要依靠战斗部爆炸产生的破片毁伤元毁伤目标,其杀伤范围由破片场决定。弹药杀伤范围内,对不同位置处目标的毁伤概率阵列称为毁伤矩阵[5]。毁伤矩阵中概率值及其分布与破片场、目标易损性和弹目交会条件等有关。由于毁伤矩阵中毁伤概率分布不规律、边界不规则,对应的数据应用于毁伤效能评估的计算时计算效率低。为了提高计算效率,常用特定的毁伤函数[6]近似描述弹药对不同位置处目标的毁伤能力。若使用的毁伤函数不能准确表征弹药对目标的毁伤能力,将导致弹药毁伤效能评估精度低,进而影响作战时耗弹量的估算及火力规划。
事实上,许多学者已经提出了大量毁伤函数以表征弹药对不同位置处目标的毁伤概率。Driels[7]通过对毁伤矩阵绘制等概率轮廓线,发现轮廓线围成的区域近似为椭圆形状,且这些轮廓线对应概率值服从高斯分布,由此引入卡尔顿函数表征弹药对目标的毁伤概率分布。Chusilp等[8]引入了饼切毁伤函数表征弹药对不同位置处目标的毁伤概率。Eckler等[9]归纳总结了许多毁伤函数形式,其分析表明卡尔顿毁伤函数与饼切毁伤函数的应用最广泛。
弹药对目标的毁伤效能不仅取决于弹药对目标的毁伤能力,也与弹药投放精度有关。在描述弹药对目标的毁伤能力时,可采用毁伤函数表征;而在描述弹药投放精度时,通常采用炸点分布的概率密度函数。Anderson等[10]和Moon等[11]采用卡尔顿毁伤函数和矩形饼切毁伤函数的方法,对比研究了弹药投放精度对弹药毁伤效能的影响,结果表明随着弹药投放精度的变化,两者的计算结果有较大差异。Klopcic等[12]研究发现,采用基于饼切毁伤函数方法计算得到的弹药对目标的毁伤效能大于采用基于卡尔顿毁伤函数方法的计算结果,并且随着弹药投放精度的提高,两者计算结果的差异逐渐增大。Chusilp等[13]分别采用基于卡尔顿毁伤函数和实际毁伤矩阵的方法计算弹药对目标的毁伤效能,验证了基于卡尔顿毁伤函数方法计算的准确性。基于毁伤函数的方法计算弹药对目标的毁伤效能的数学表达式通常可表示为炸点分布的概率密度函数与毁伤函数乘积的积分[14]。由于卡尔顿毁伤函数的原函数为非初等函数,因此基于该函数求解弹药对目标的毁伤效能时,无法得到解析解。针对此种情况,丁贵鹏等[15]、Wang等[16-17]和Lee等[18]采用蒙特卡罗方法求解,但该方法计算量较大,效率较低。相比之下,采用基于矩形饼切毁伤函数的方法可以得到解析解,然而,由于基于矩形饼切毁伤函数的计算往往高估弹药对矩形区域内目标的毁伤概率,忽略弹药对矩形区域外目标的毁伤概率,导致计算结果偏离真实值,从而降低了计算精度。
综上所述,卡尔顿毁伤函数可以近似表征破片式战斗部对目标的毁伤能力,并被成功应用于许多场景,但采用基于卡尔顿毁伤函数的方法评估弹药对目标的毁伤效能时,计算效率较低。尽管采用基于矩形饼切毁伤函数的方法计算弹药对目标的毁伤效能时,存在解析解,但其计算精度较低。鉴于此,本文中提出一种多矩形饼切毁伤函数,兼具上述2种毁伤函数的优点,在保证计算精度的同时,有效地提升计算效率,以期快速准确地评估弹药对目标的毁伤效能。
1. 毁伤函数
为了计算弹药爆炸对周围目标的毁伤概率,建立了表征弹目关系的坐标系,以弹药炸点A在地面上的投影点O为原点建立地面坐标系,定义弹药速度方向在地面上的投影为x轴,y轴垂直x轴且位于地面上,z轴由右手法则确定,如图1所示,
θ 为弹药速度方向→AP 与地面之间的夹角(弹药落角),T(x,y) 为目标位置。下面介绍常用的卡尔顿、椭圆及矩形饼切毁伤函数以及本文中提出的多矩形饼切毁伤函数。1.1 卡尔顿毁伤函数
记弹药对位于
(x,y) 处目标的毁伤概率为PK(x,y) ,若采用卡尔顿毁伤函数表征弹药对不同位置处目标的毁伤能力,则其表达式为:PK(x,y)=PKC(x,y)=exp[−(x2R2x+y2R2y)] (1) 式中:
Rx 和Ry 为卡尔顿毁伤函数的参数,分别描述了x轴方向和y轴方向上毁伤概率的变化梯度。卡尔顿毁伤函数如图2所示。弹药毁伤效能评估中,常用效能指数[19]表征弹药对目标的总体毁伤能力。效能指数的具体形式与弹药和目标的类型有关。评估破片式战斗部对地面装备的毁伤效能时,使用的效能指数为有效杀伤面积
S [7,12],表达式为:S=∫+∞−∞∫+∞−∞PK(x,y)dxdy (2) 若用卡尔顿毁伤函数表征弹药对目标的毁伤能力,将式(1)代入式(2),则有效杀伤面积为:
S=πRxRy (3) 由式(3)可知,有效杀伤面积等于以
Rx 、Ry 为半长、半短轴椭圆的面积,定义此椭圆长和短轴的比为:a=Rx/Ry (4) a 值的大小与弹药的落角相关,弹药落角越大,a 值越大,当弹药落角为90°时,a=1 。Driels[7]给出了a 值与弹药落角θ 的关系:a=max[(1−0.8cosθ),0.3] (5) 若弹药对目标的有效杀伤面积
S 已知,可求得卡尔顿毁伤函数在x轴和y轴方向上的2个相关参数:Rx=√Sa/Saππ (6) Ry=Rx/Rxaa (7) 1.2 椭圆及矩形饼切毁伤函数
采用卡尔顿毁伤函数可简化弹药对目标毁伤能力的计算,但评估弹药的毁伤效能时,通常需要结合蒙特卡罗方法,其计算效率较低。为了提高计算效率,Driels[7]将卡尔顿毁伤函数等效为椭圆饼切毁伤函数,其函数形式如式(8),几何图形如图3所示,杀伤区域等效为一个椭圆,如图4所示,其面积等于弹药的有效杀伤面积,半长、短轴分别为卡尔顿函数的2个参量
Rx 与Ry 。如果目标位于椭圆区域内,则弹药对其毁伤概率为1;若目标位于椭圆区域外,则弹药对其毁伤概率为0。若用椭圆饼切毁伤函数表征弹药对不同位置处的目标毁伤能力,则弹药对位于
(x,y) 处目标的毁伤概率PKE(x,y) 可表示为:PK(x,y)=PKE(x,y)={1x2Rx+y2Ry≤10x2Rx+y2Ry>1 (8) 评估弹药的毁伤效能时,需要考虑弹药命中精度,即计算目标在弹药杀伤区域(椭圆)内的概率。如果弹药散布服从正态分布,则求解目标在椭圆区域内的概率时较困难。因此,可进一步将杀伤区域等效为矩形,其面积与椭圆面积相等,如图4所示,用矩形饼切毁伤函数替代卡尔顿毁伤函数,矩形长宽比等于椭圆长轴与短轴之比,其几何图形如图5所示。若目标位于矩形区域内则表示目标被毁伤,毁伤概率为1;若目标位于矩形区域外,则表示目标未被毁伤,毁伤概率为0。矩形饼切毁伤函数的表达式为:
PK(x,y)=PKR(x,y)={1|x|≤L/2,|y|≤W/20|x|>L/2,|y|>W/2 (9) 式中:
L 和W 分别为矩形区域在x轴和y轴方向上长度。矩形饼切毁伤函数对应的几何矩形参数
L 和W 分别为:L=√Sa (10) W=L/Laa (11) 式中:
a 的值可通过式(5)计算得到。1.3 多矩形饼切毁伤函数
本文中提出一种新的毁伤函数—多矩形饼切毁伤函数,该函数采用了梯形法则的思想,可快速准确地求解弹药对目标的毁伤效能。如图6所示将毁伤矩阵等效为多矩形饼切毁伤函数,其过程可以分为2个步骤:首先,根据毁伤矩阵中的概率分布绘制一系列等概率线,如图6(a)所示;其次,为便于使用这些等概率线,将每个等概率线所包围的区域等效为一个面积相等的矩形,矩形中心与等概率线中心相同,由外到内第
i 个矩形对应的长宽依次为Li 和Wi (i=1, 2, …, n ),n 为矩形数量,也为概率分区数,如图6(b)所示。多矩形饼切毁伤函数的表达式为:
PK(x,y)=PKMR(x,y)={(Pi+Pi+1)/2Li+1/2≤|x|≤Li/2,Wi+1/2≤|y|≤Wi/20|x|>L1/2,|y|>W1/2 (12) 图6(c)所示为多矩形饼切毁伤函数的图形,由外往内第
i 个概率区间为[Pi,Pi+1]∈(0,1] ,若等概率线对应概率值服从高斯分布,则可得第i 个矩形的面积Ai :Ai=−S lnPi (13) 式中:
Pi 为第i 个概率区间下限。第
i 个矩形对应的长度Li 和宽度Wi 分别为:Li=√Aia (14) Wi=Li/Liaa (15) 2. 弹药对目标的毁伤效能
通常使用弹药对目标平均毁伤概率表征弹药对目标的毁伤效能,平均毁伤概率与弹药对目标的毁伤能力及弹药投放精度有关,其中弹药对目标的毁伤能力可用毁伤函数表示,弹药投放精度可通过弹药落点散布大小衡量,弹药落点散布通常用圆概率误差(circular error probable, CEP)或二维正态分布表征。CEP是指以瞄准点为圆心,包含50%的弹药落点所围成圆的半径。
如果弹药落点散布在x方向和y方向相互独立,服从正态分布,则弹药落点分布的概率密度函数为:
f(x,y)=u1(x)u2(y) (16) 式中:
{u1(x)=1√2πσxexp[−(x−x0)22σ2x]u2(y)=1√2πσyexp[−(y−y0)22σ2y] (17) 式中:
x0 和y0 分别为瞄准点的x坐标和y坐标,σx 和σy 分别为弹药落点在x方向和y方向分布的标准差,其与圆概率误差CEP的量值δCEP 关系为[20]:σx=σy=δCEP/1.1774 (18) 弹药对目标的平均毁伤概率:
ˉPK=∫+∞−∞∫+∞−∞PK(x,y)f(x,y)dxdy (19) 式中:
PK(x,y) 可采用不同的毁伤函数计算。2.1 弹药对目标的毁伤效能计算模型
由式(19)可知,采用基于矩形饼切毁伤函数或多矩形饼切毁伤函数的方法求解弹药对目标的毁伤效能时,需要对正态分布概率密度函数积分,由于正态分布概率密度函数的原函数为非初等函数,其积分表达式无解析解。为了提高计算效率,得到解析解,Abramowitz等[21]在1976年提出了多项式等效算法,其绝对精度可达
7.5×10−8 ,等效形式为:F(z)=∫z−∞1√2πexp(−x22)dx={ϕ(z)z≥01−ϕ(−z)z<0 (20) 式中:
ϕ(z)=1−φ(z)(a1t+a2t2+a3t3+a4t4+a5t5), φ(z)=1√2πexp(−z22), t=11+a0z (21) 式中:
a0 ~a5 为常数,a0 =0.2316419,a1 =0.319381530,a2 =−0.356563782,a3 =1.781477937,a4 =−1.821255978,a5 =1.330274429。一般正态分布
x∼N(x0,σ2x) 可转化为标准正态分布x−x0σx∼N(0,1) ,则有:∫z−∞1√2πσxexp[−(x−x0)22σ2x]dx=F(z−x0σx) (22) 令:
{ξx(t)=F(t−2x02σx)−F(−t+2x02σx)ξy(t)=F(t−2y02σy)−F(−t+2y02σy) (23) 若采用
F(x) 替代标准正态分布概率密度函数积分,则基于矩形饼切毁伤函数计算得到的平均毁伤概率为:ˉPK=∫L/2−L/2∫W/2−W/212πσxσyexp[−(x−x0)22σ2x−(y−y0)22σ2y]dxdy=ξx(L)ξy(W) (24) 同理,则基于多矩形饼切毁伤函数计算得到的平均毁伤概率为:
ˉPK=n∑i=1Pi+Pi+12[ξx(Li)ξy(Wi)−ξx(Li+1)ξy(Wi+1)] (25) 若
PK(x,y) 采用卡尔顿毁伤函数形式表征,则式(19)无法通过解析法求解。因此,常用蒙特卡罗抽样方法求解,求解步骤如图7所示,图中,i为循环变量;(xi,yi) 为第i次蒙特卡罗抽样时的弹药落点位置;PK(xi,yi) 为第i次蒙特卡罗抽样时的弹药对目标的毁伤概率;N为蒙特卡罗抽样次数;Psum 为N次抽样下弹药对目标的毁伤概率之和,其初始值为0;ˉPK 为N次抽样下弹药对目标的平均毁伤概率。基于卡尔顿毁伤函数方法求解弹药对目标的毁伤效能步骤为:首先,根据弹药投放精度得到式(16)所示的弹药落点分布的概率密度函数,基于该函数随机抽样弹药落点位置(xi,yi) ;然后,将抽样的弹药落点位置代入式(1)所示的卡尔顿毁伤函数,得到弹药在该位置爆炸时对目标的毁伤概率PK(xi,yi) ;最后,循环上述步骤,直至循环次数达到蒙特卡罗抽样次数N,结束循环,输出N次抽样条件下弹药对目标的平均毁伤概率ˉPK 。2.2 概率分区数对毁伤效能计算精度的影响
基于多矩形饼切毁伤函数计算得到的毁伤效能的精度受概率分区数n的影响,概率分区数越大,毁伤效能的计算结果越精确,但计算时间越多,因此,计算了在某弹目交会条件(
S 为2000 m2,δCEP 为30 m,弹药落角为30°)下概率分区数对平均毁伤概率和计算时间的影响,结果图8所示。由图8可知,随着概率分区数的增加,计算得到的平均毁伤概率逐渐减小并趋于定值,而计算时间与概率分区数呈线性增加关系。当概率分区数为10时,计算得到的平均毁伤概率相对概率分区数为40的相对偏差为4.1%;当概率分区数为20时,计算得到的平均毁伤概率相对概率分区数为40的相对偏差为1.4%。因此,当概率分区数达到20之后,可近似认为随着概率分区数的增加,平均毁伤概率变化缓慢,因而后续基于多矩形饼切毁伤函数计算平均毁伤概率时,概率分区数n取20。2.3 蒙特卡罗抽样次数的确定
基于卡尔顿毁伤函数计算平均毁伤概率时,通常采用蒙特卡罗抽样的方法,蒙特卡罗抽样次数对计算结果的精度影响较大,抽样次数越多结果越准确,但计算效率越低。为了兼顾计算精度与时间,获取最佳匹配,本文中分析了蒙特卡罗抽样次数对计算平均毁伤概率的影响。图9为平均毁伤概率随蒙特卡罗抽样次数的变化曲线,由图9可知,当蒙特卡罗抽样次数达到104之后,平均毁伤概率随蒙特卡罗抽样次数增加变化缓慢。因此,本文中基于卡尔顿毁伤函数计算平均毁伤概率时,蒙特卡罗抽取次数取为104。
3. 结果分析
3.1 毁伤效能
为了比较基于3种毁伤函数的弹药毁伤效能计算结果,计算了在不同弹目条件(有效杀伤面积S为2000 m2,弹药落角
θ 为30°~75°)及弹药投放精度(δCEP 为5~50 m)下弹药对目标的平均毁伤概率,对比了基于多矩形饼切函数和矩形饼切函数的计算结果相对于卡尔顿毁伤函数计算结果的误差,结果如图10~13所示。由图10~13可知,基于多矩形饼切毁伤函数计算得到的平均毁伤概率与基于卡尔顿毁伤函数的计算结果吻合较好;而基于矩形饼切毁伤函数计算得到的平均毁伤概率高于基于卡尔顿毁伤函数的计算结果,当
δCEP 小于30 m时,前者相较于后者的最大偏差为27.5%(图13,δCEP 为15 m)。这是由于弹药投放精度越高,目标位于矩形杀伤区域内的概率越大,使得高估矩形区域内目标毁伤概率的问题更加突出,导致计算得到的平均毁伤概率高于真实值。记
εMR 为基于多矩形饼切函数的计算结果相对于基于卡尔顿毁伤函数的计算结果的误差,εR 为基于矩形饼切函的计算结果相对于基于卡尔顿毁伤函数的计算结果的误差,θ 为30°时,εR 与εMR 之差最大为16.3%,即相较于矩形饼切毁伤函数,基于多矩形饼切毁伤函数的计算结果精度提升了16.3%。随着θ 的增大,εR 逐渐变大,εMR 变化较小,因而计算精度提升更明显,当弹药落角增大至75°时,εR 与εMR 之差最大为26.4%,计算精度提升了26.4%。3.2 计算时间
为了评估3种毁伤函数的计算效率,编写了基于3种毁伤函数计算平均毁伤概率的程序,在弹目交会条件及软硬件平台(Microsoft Visual Studio 2015,Intel(R) Core(TM)i5-12500H@2.50GHz,16GB RAM)相同的条件下,记录单次平均毁伤概率计算所耗时间,如表1所示。从表1可以得出:与基于卡尔顿毁伤函数的方法相比,采用基于多矩形饼切毁伤函数的方法能够显著提高计算效率,具体提升了518倍。虽然与基于矩形饼切毁伤函数的方法相比,采用基于多矩形饼切毁伤函数的方法计算效率略有降低,但能在保持较高计算效率的同时实现计算精度的大幅度提升。
表 1 基于3种毁伤函数的计算时间Table 1. Computational time consumption based on three damage functions毁伤函数 计算时间/μs 卡尔顿毁伤函数 2174 矩形饼切毁伤函数 0.2 多矩形饼切毁伤函数 4.2 4. 结 论
基于梯形法则的思想,将弹药杀伤范围分区等效为多个矩形,推导得到了多矩形饼切毁伤函数的数学表达式,并将它运用于弹药对目标的毁伤效能评估,计算了弹药在落角范围为30°~75°及投放精度CEP范围为5~50 m时弹药对目标的毁伤效能,并与采用基于卡尔顿和矩形饼切毁伤函数方法的计算结果进行对比,得到如下结论。
(1)基于卡尔顿毁伤函数评估的毁伤效能(平均毁伤概率)与基于矩形饼切函数评估的结果差异较大,且随着落角的增大,差异随之增大,后者相对于前者的最大相对偏差为27.5%,而基于卡尔顿毁伤函数和多矩形饼切毁伤函数的评估结果吻合较好,在本文的计算条件下的后者相对于前者的相对偏差均小于10%。
(2)采用基于多矩形饼切毁伤函数的方法可通过解析求解弹药对目标的毁伤效能,计算效率较高,相较于采用基于卡尔顿毁伤函数的方法,单次毁伤效能计算效率提高了518倍,同时相较于采用基于矩形饼切毁伤函数的方法,毁伤效能计算精度最大可提高26.4%。
(3)本文中提出的多矩形饼切毁伤函数可以用于评估杀爆弹对地面目标的毁伤效能,在已知弹药对目标的毁伤威力(有效杀伤面积)时,能够快速准确地评估弹药毁伤效能,可为战斗部设计及火力规划提供参考。
-
表 1 基于3种毁伤函数的计算时间
Table 1. Computational time consumption based on three damage functions
毁伤函数 计算时间/μs 卡尔顿毁伤函数 2174 矩形饼切毁伤函数 0.2 多矩形饼切毁伤函数 4.2 -
[1] 徐豫新, 蔡子雷, 吴巍, 等. 弹药毁伤效能评估技术研究现状与发展趋势 [J]. 北京理工大学学报, 2021, 41(6): 569–578. DOI: 10.15918/j.tbit1001-0645.2020.194.XU Y X, CAI Z L, WU W, et al. Current research and development of ammunition damage effect assessment technology [J]. Transactions of Beijing Institute of Technology, 2021, 41(6): 569–578. DOI: 10.15918/j.tbit1001-0645.2020.194. [2] 曾松林, 韩玉龙, 陈榕, 等. 一种评估航空炸弹对集群目标毁伤效能的新方法 [J]. 航空兵器, 2023, 30(1): 25–30. DOI: 10.12132/ISSN.1673-5048.2022.0037.ZENG S L, HAN Y L, CHEN R, et al. A new method to evaluate aerial bomb damage effectiveness hitting cluster targets [J]. Aero Weaponry, 2023, 30(1): 25–30. DOI: 10.12132/ISSN.1673-5048.2022.0037. [3] 徐文旭, 李召, 陆凡东. 基于状态空间的反坦克导弹毁伤效能精准评估方法 [J/OL]. 兵工学报[2023-08-23]. DOI: 10.12382/bgxb.2022.0686.XU W X, LI Z, LU F D. Accurate evaluation method of antitank missile damage effectiveness based on state space [J/OL]. Acta Armamentarii[2023-08-23]. DOI: 10.12382/bgxb.2022.0686. [4] 赵晓旭, 韩旭光, 吴浩, 等. 制导杀爆弹毁伤效能评估的应用研究 [J]. 北京理工大学学报, 2019, 39(6): 551–557. DOI: 10.15918/j.tbit1001-0645.2019.06.001.ZHAO X X, HAN X G, WU H, et al. Applied research on damage effectiveness evaluation of guided explosive bomb [J]. Transactions of Beijing Institute of Technology, 2019, 39(6): 551–557. DOI: 10.15918/j.tbit1001-0645.2019.06.001. [5] 田浩成, 卢芳云, 李志斌, 等. 基于改进毁伤矩阵的人员目标毁伤效能评估 [J]. 兵器装备工程学报, 2022, 43(7): 101–108. DOI: 10.11809/bqzbgcxb2022.07.015.TIAN H C, LU F Y, LI Z B, et al. Evaluation of damage effectiveness of personnel target based on improved damage matrix [J]. Journal of Ordnance Equipment Engineering, 2022, 43(7): 101–108. DOI: 10.11809/bqzbgcxb2022.07.015. [6] KATSEV I, EVLOGIEV S. Damage functions evaluation coherent to weapon target interaction [J]. Machines, Technologies, Materials, 2019, 13(3): 124–126. [7] DRIELS M R. Weaponeering: conventional weapon system effectiveness [M]. Reston: American Institute of Aeronautics and Astronautics, 2004. [8] CHUSILP P, CHARUBHUN W, NILUBOL O. Effects of the cookie cutter function shapes on Monte Carlo simulations of weapon effectiveness [C]//2014 Seventh IEEE Symposium on Computational Intelligence for Security and Defense Applications (CISDA). Cau Giay: IEEE, 2014: 1–7. DOI: 10.1109/CISDA.2014.7035622. [9] ECKLER A R, BURR S A. Mathematical models of target coverage and missile allocation: DTIC: AD-A953517 [R]. Alexandria: Military Operations Research Society, 1972. [10] ANDERSON C M. Generalized weapon effectiveness modeling [D]. Monterey: Naval Postgraduate School, 2004. [11] MOON S H. Weapon effectiveness and the shapes of damage functions [J]. Defence Technology, 2021, 17(2): 617–632. DOI: 10.1016/j.dt.2020.04.009. [12] KLOPCIC J T. A comparison of damage functions for use in artillery effectiveness codes: DTIC_ADA222589 [R]. Maryland: Army Ballistic Research Laboratory, Aberdeen Proving Ground, 1990. [13] CHUSILP P, CHARUBHUN W, KOANANTACHAI P. Monte Carlo simulations of weapon effectiveness using Pk matrix and Carleton damage function [J]. International Journal of Applied Physics and Mathematics, 2014, 4(4): 280–285. DOI: 10.7763/IJAPM.2014.V4.299. [14] 李向东, 周兰伟, 纪杨子燚. 目标易损性评估及应用 [M]. 北京: 北京理工大学出版社, 2022: 372-373. [15] 丁贵鹏, 陶钢, 庞春桥, 等. 基于卡尔顿毁伤函数的杀伤榴弹效能评估模拟 [J]. 兵器装备工程学报, 2021, 42(8): 8–13. DOI: 10.11809/bqzbgcxb2021.08.002.DING G P, TAO G, PANG C Q, et al. Evaluation simulation of grenade effectiveness based on Carleton damage function [J]. Journal of Ordnance Equipment Engineering, 2021, 42(8): 8–13. DOI: 10.11809/bqzbgcxb2021.08.002. [16] WANG H Y, LABARIA G, MOTEN C, et al. Average damage caused by multiple weapons against an area target of normally distributed elements [J]. American Journal of Operations Research, 2017, 7(5): 289–306. DOI: 10.4236/ajor.2017.75022. [17] WANG H Y, MOTEN C, DRIELS M, et al. Explicit exact solution of damage probability for multiple weapons against a unitary target [J]. American Journal of Operations Research, 2016, 6(6): 450–467. DOI: 10.4236/ajor.2016.66042. [18] LEE J H, CHOI W J. Assessment of vulnerable area and naval ship’s vulnerability based on the Carleton damage function [J]. Journal of the Society of Naval Architects of Korea, 2018, 55(3): 274–280. DOI: 10.3744/SNAK.2018.55.3.274. [19] GALLAGHER M A, HORTA M C. Cyber joint munitions effectiveness manual (JMEM) [J]. American Intelligence Journal, 2013, 31(1): 73–81. [20] 宋谢恩, 宋卫东, 宋高兴. 弹道修正火箭弹对面目标射击的最佳CEP研究 [J]. 弹道学报, 2014, 26(4): 51–55. DOI: 10.3969/j.issn.1004-499X.2014.04.010.SONG X E SONG W D, SONG G X. Research on the best CEP of ballistic correction rocket firing on surface target [J]. Journal of Ballistics, 2014, 26(4): 51–55. DOI: 10.3969/j.issn.1004-499X.2014.04.010. [21] ABRAMOWITZ M, STEGUN I A. Handbook of mathematical functions with formulas, graphs, and mathematical tables [M]. Washington: Government Printing Office, 1967. 期刊类型引用(0)
其他类型引用(1)
-











 下载:
下载:











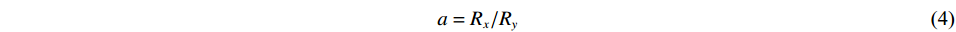






















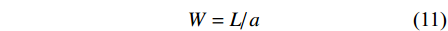







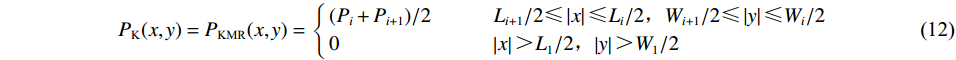








































































 下载:
下载:
























