Meticulous analysis of one-dimensional elastic-plastic wave evolution in sandwich bar system (part Ⅰ): transmitted and reflected waves for typical loading waves
-
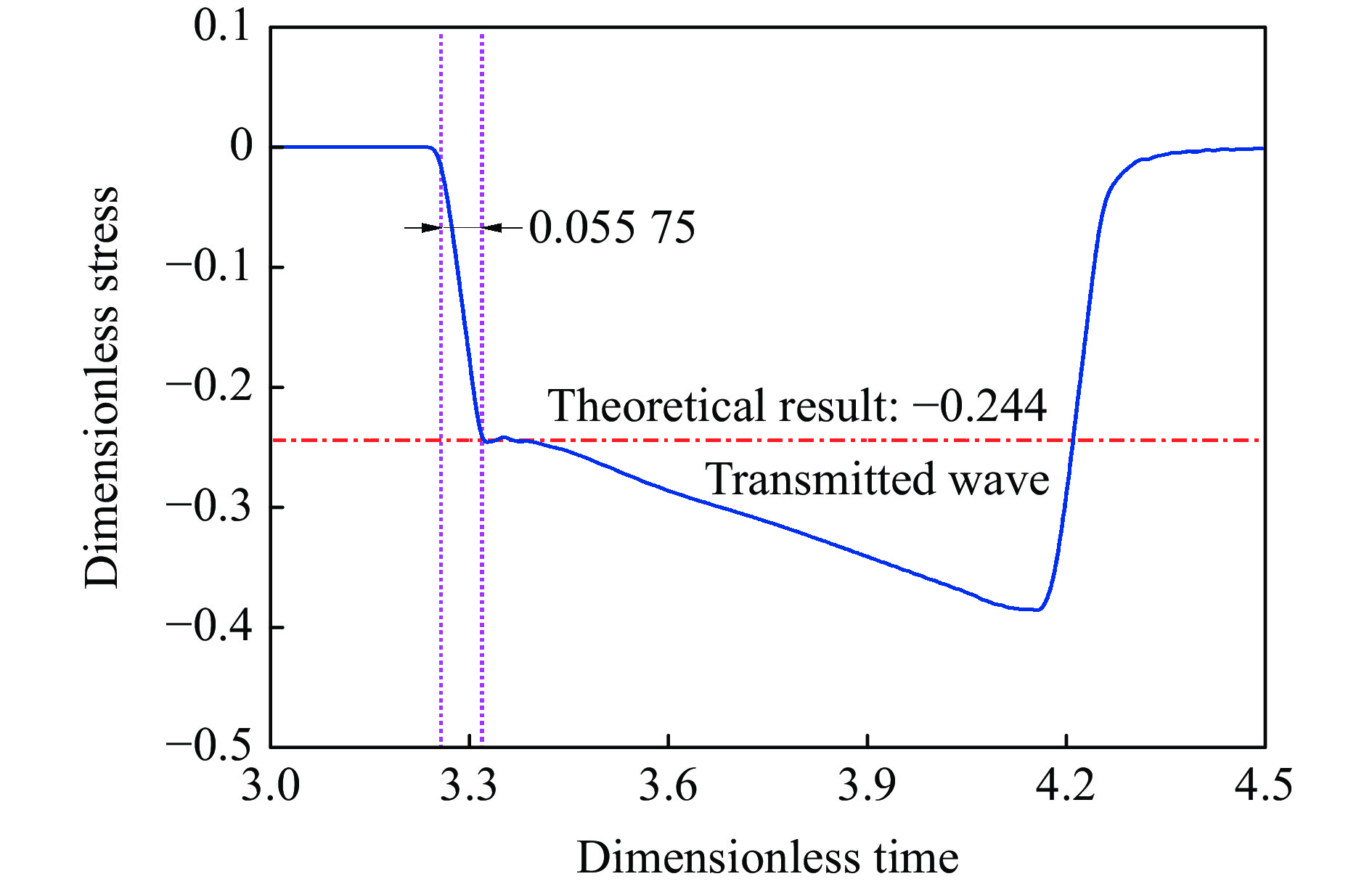
摘要: 准确地剖析反射波与透射波的形成过程与影响机理是SHPB（split Hopkinson pressure bar）精细化试验设计与精准数据处理的核心前提之一。针对夹心杆系统，分析矩形、梯形与半正弦三种典型入射波加载阶段系统中一维弹塑性波的传播与演化，定量研究试件中弹塑性波的传播、两个界面上弹塑性的透反射及其系列透反射波的相互作用影响。结果表明：首先，弹塑性波特别是应力波在弹塑性交界面上的透反射中占主要地位，纯弹性波的透反射与传播反而影响较小；其次，当入射波加载区间有一定的宽度时，杆2中弹性波在两个界面上的多次透反射对反射波造成衰减的同时对透射波进一步强化，这种衰减使得半正弦波对应的反射波峰值并不是在0.5个无量纲时间时，而是有所提前；第三，与传统SHPB分析中弹性波在界面上透反射的初步规律不同，无论是矩形波、梯形波还是半正弦波入射时，试件材料的杨氏模量与密度改变虽然明显影响其弹性波的阻抗比，但对透反射波波形及其峰值强度影响并不明显。研究结果可为SHPB的精细化设计与数据的精准分析提供科学依据。Abstract: The reflected and transmitted waves in split Hopkinson pressure bar (SHPB) tests provide crucial information for obtaining the stress-strain relationship of materials. Accurately analyzing the formation process and influencing mechanisms of the reflected and incident waves is a key prerequisite for precise experimental design and accurate data processing. In this paper, the propagation and evolution of one-dimensional elastic-plastic waves in the loading stages of the SHPB test are presented particularly for a sandwich bar system consisting of the incident wave, specimen, and transmitted bar. Based on the theory of elastic-plastic incremental waves and numerical simulation calculations, the propagation of elastic-plastic waves in the specimen, the transmission and reflection of elastic-plastic waves at the two interfaces, and the interaction of the resulting series of transmitted and reflected waves are quantitatively investigated. The research findings are as follows. Firstly, although the design principle of the SHPB apparatus is based on linear elastic wave theory, the elastic-plastic waves, especially the stress waves, have a major influence on the transmission and reflection at the elastic-plastic interface, while the transmission and propagation of purely elastic waves have a relatively minor effect. Secondly, when the loading interval of the incident wave has a certain width, the multiple transmission and reflection of elastic waves at the two interfaces in bar 2 attenuate the reflected wave while further strengthening the transmitted wave. This attenuation causes the peak of the reflected wave for the half-sine wave to occur earlier than at 0.5 nondimensional time. Thirdly, in contrary to the preliminary laws of elastic wave transmission and reflection at interfaces in traditional SHPB analysis, variations in the Young’s modulus and density of the specimen material have little effect on the waveform and peak intensity of the transmitted wave, regardless of whether the incident wave is rectangular, trapezoidal, or half-sine. This investigationprovides a scientific basis for the refined design of SHPB experiments and precise analysis of data.
-
图 1 准一维夹心杆结构示意图
Figure 1. Schematic diagram of the quasi-one-dimensional sandwich bar structure
图 4 杆2直径为8 mm时矩形入射波的反射波峰值
Figure 4. Peak value of reflected wave for rectangular incident wave in a bar of diameter 8 mm
图 5 杆2直径为8 mm时矩形入射波透射波初始峰值
Figure 5. Initial peak value of transmitted wave for rectangular incident wave in a bar of diameter 8 mm
图 6 梯形波时加载阶段的弹塑性转换点
Figure 6. Elastic-plastic transition point during the loading stage for trapezoidal incident wave
图 7 点C到达界面1之前的透反射物理平面图
Figure 7. Physical plane diagram of transmission before point C reaches interface 1 for trapezoidal incident wave
图 8 梯形波时反射波峰值仿真值与理论值
Figure 8. Simulation calculation and theoretical value of peak value of reflected wave for trapezoidal incident wave
图 9 梯形波时透射波首个峰值仿真值与理论值
Figure 9. Simulation calculation and theoretical value of first peak value of transmitted wave for trapezoidal incident wave
图 11 6个点透射波后方的无量纲应力
Figure 11. Dimensionless stress of transmitted wave at six points after the wave
图 12 杆2左端无量纲平均应力时程曲线
Figure 12. Dimensionless time history curve of average stress at the left end of bar 2
图 13 反射波弹性段的无量纲时间宽度
Figure 13. Dimensionless time width of elastic segment in the reflected wave
图 14 临近点E的应力波传播等效物理平面图
Figure 14. Equivalent physical plane diagram of stress wave propagation near point E
图 15 半正弦时反射波峰值对应的无量纲时间
Figure 15. Dimensionless time corresponding to peak value of reflected wave for half-sine incident wave
图 16 透射波首次峰值时间与应力理论与仿真对比
Figure 16. Comparison of theoretical and simulation results for time of first peak value and stress of transmitted wave for trapezoidal incident wave
图 17 直径8 mm时不同杆2密度时的反射波
Figure 17. Reflected wave for different densities of bar 2 of diameter 8 mm
图 18 无量纲密度为1/6时入射波中的三个特征点
Figure 18. Three characteristic points in incident wave for density of bar 2 equal to 1/6
图 19 三种入射波对应的反射波波形图
Figure 19. Waveform of reflected wave for three kinds of incident waves
图 20 不同无量纲密度时梯形入射波的反射波
Figure 20. Reflected wave for trapezoidal incident wave under different dimensionless densities
图 21 梯形入射波在不同杆2直径时的反射波峰值的理论结果与仿真结果
Figure 21. Theoretical and simulation results of reflected wave peak stress for trapezoidal incident wave at different diameters
表 1 梯形入射波上的特征点参数与反射波峰值应力
Table 1. Parameters of characteristic points on trapezoidal incident wave and peak stress of reflected wave
杆2直径/
mm点C无量
纲应力点C无量
纲时间点D无量
纲应力点D无量
纲时间峰值无量
纲应力4 −0.300 0.060 0.261 0.052 0.934 6 −0.353 0.071 0.316 0.063 0.852 8 −0.441 0.088 0.409 0.082 0.736 10 −0.553 0.111 0.529 0.106 0.599 12 −0.668 0.134 0.654 0.131 0.431 
下载: 导出CSV
-
[1] LIU F, LI Q M. Strain-rate effect of polymers and correction methodology in a SHPB test [J]. International Journal of Impact Engineering, 2022, 161: 104109. DOI: 10.1016/j.ijimpeng.2021.104109. [2] LIU P, HU D A, WU Q K, et al. Sensitivity and uncertainty analysis of interfacial effect in SHPB tests for concrete-like materials [J]. Construction and Building Materials, 2018, 163: 414–427. DOI: 10.1016/j.conbuildmat.2017.12.118. [3] KARIEM M A, BEYNON J H, RUAN D. Misalignment effect in the split Hopkinson pressure bar technique [J]. International Journal of Impact Engineering, 2012, 47: 60–70. DOI: 10.1016/j.ijimpeng.2012.03.006. [4] NIE H L, MA W F, HE X L, et al. Misalignment tolerance in one-side and symmetric loading Hopkinson pressure bar experiments [J]. Acta Mechanica Solida Sinica, 2022, 35(2): 273–281. DOI: 10.1007/s10338-021-00267-3. [5] GUO Y B, GAO G F, JING L, et al. Dynamic properties of mortar in high-strength concrete [J]. International Journal of Impact Engineering, 2022, 165: 104216. DOI: 10.1016/j.ijimpeng.2022.104216. [6] PANOWICZ R, JANISZEWSKI J, KOCHANOWSKI K. Effects of sample geometry imperfections on the results of split Hopkinson pressure bar experiments [J]. Experimental Techniques, 2019, 43(4): 397–403. DOI: 10.1007/s40799-018-0293-7. [7] BRIZARD D, JACQUELIN E. Uncertainty quantification and global sensitivity analysis of longitudinal wave propagation in circular bars. application to SHPB device [J]. International Journal of Solids and Structures, 2018, 134: 264–271. DOI: 10.1016/j.ijsolstr.2017.11.005. [8] YANG H S, LI Y L, ZHOU F H. Propagation of stress pulses in a Rayleigh-Love elastic rod [J]. International Journal of Impact Engineering, 2021, 153: 103854. DOI: 10.1016/j.ijimpeng.2021.103854. [9] BRAGOV A M, LOMUNOV A K, LAMZIN D A, et al. Dispersion correction in split-Hopkinson pressure bar: theoretical and experimental analysis [J]. Continuum Mechanics and Thermodynamics, 2022, 34(4): 895–907. DOI: 10.1007/s00161-019-00776-0. [10] RIGBY S E, BARR A D, CLAYTON M. A review of Pochhammer-Chree dispersion in the Hopkinson bar [J]. Proceedings of the Institution of Civil Engineers - Engineering and Computational Mechanics, 2018, 171(1): 3–13. DOI: 10.1680/jencm.16.00027. [11] REN L, YU X M, HE Y, et al. Numerical investigation of lateral inertia effect in dynamic impact testing of UHPC using a Split-Hopkinson pressure bar [J]. Construction and Building Materials, 2020, 246: 118483. DOI: 10.1016/j.conbuildmat.2020.118483. [12] AL-MALIKY N S. Dimension effect on dynamic stress equilibrium in SHPB tests [J]. International Journal of Materials Physics, 2014, 5(1): 15–26. DOI: 10.13140/RG.2.2.15864.19200. [13] PRAKASH G, SINGH NK, GUPTA NK. Flow behaviour of Ti-6Al-4V alloy in a wide range of strain rates and temperatures under tensile, compressive and flexural loads [J]. International Journal of Impact Engineering, 2023, 176: 104549. DOI: 10.1016/j.ijimpeng.2023.104549. [14] WU X Q, YIN Q Y, WEI Y P, et al. Effects of imperfect experimental conditions on stress waves in SHPB experiments [J]. Acta Mechanica Sinica, 2015, 31(6): 827–836. DOI: 10.1007/s10409-015-0439-0. [15] 高光发. 量纲分析理论与应用 [M]. 北京: 科学出版社, 2021: 434–435.GAO G F. Dimensional analysis: theories and applications [M]. Beijing: Science Press, 2021: 434–435. -
计量
- 文章访问数: 274
- HTML全文浏览量: 67
- PDF下载量: 120
- 被引次数: 0