Meticulous analysis of one-dimensional elasto-plastic wave evolution in sandwich rod systems (part Ⅱ): reflection attenuation at the elasto-plastic interface and platform section
-
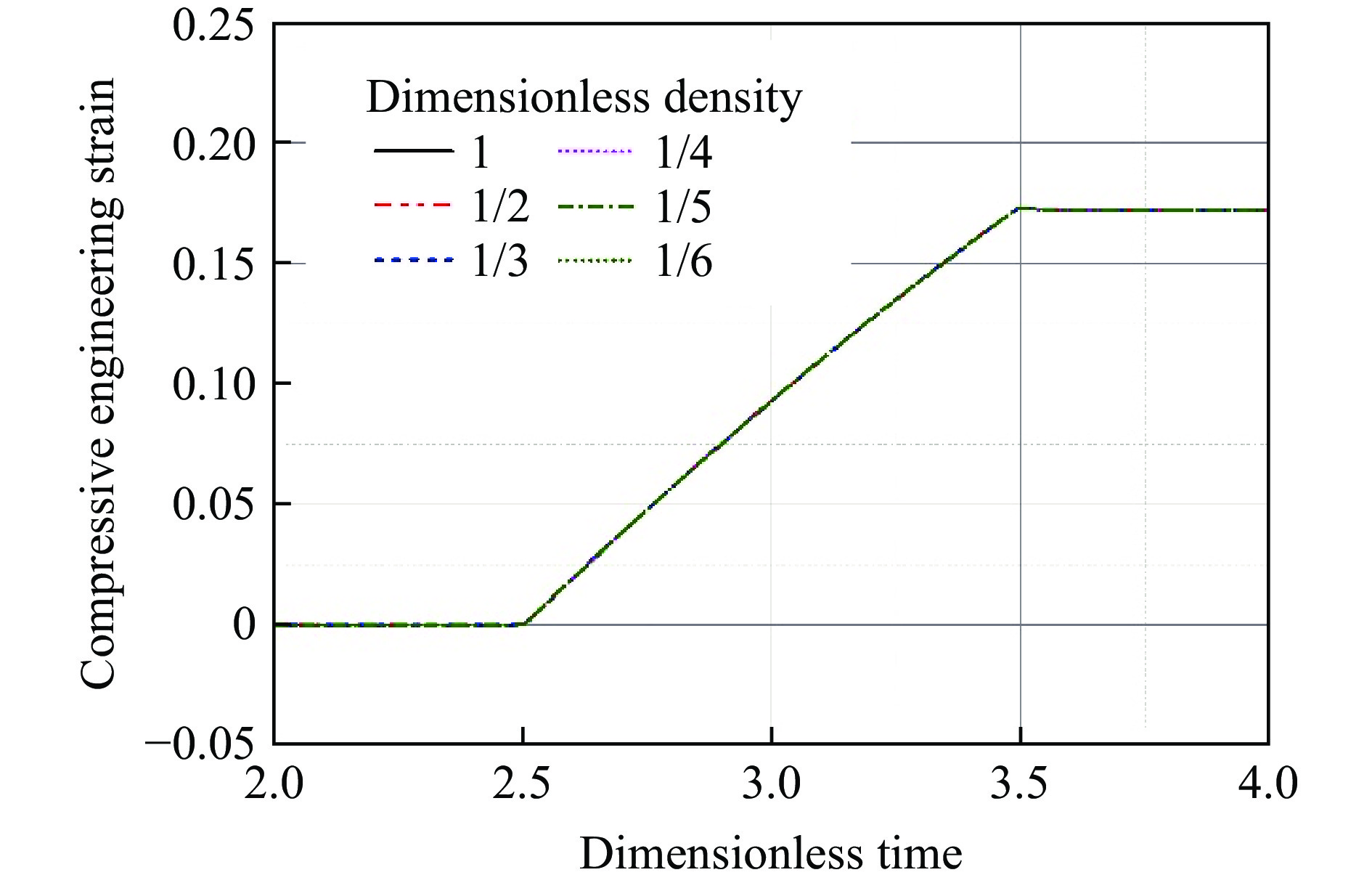
摘要: 相对于入射波加载阶段透反射分析过程，入射波平台段持续时间长且弹塑性传播与演化行为复杂得多，此阶段试件内弹塑性波相互作用的影响非常明显。开展了矩形入射波作用下试件内弹塑性波的相互作用及其在两个界面上的弹塑性透反射行为计算，定量研究了夹心杆系统中反射波的衰减特征。结果表明：强入射波作用下，由于弹塑性波相互作用，试件内形成了曲线型弹塑性交界面，使透射端达到屈服状态的时间明显提前，该弹塑性交界面以大于弹性声速的速度向反射端变速传播；在塑性阶段，反射波的衰减是试件截面积增大引起的广义波阻抗增大和压缩引起的塑性波往返次数增加两个方面导致的衰减量之和。计算显示，试件的密度变化虽然明显影响其波速和广义波阻抗，但两个方面引起的衰减量之和正好接近于零，使得密度变化对透反射波平台段变化的影响可以忽略；塑性模量的增大使得反射波在平台端衰减更快，而试件直径对反射波衰减速度的影响并不是单调的，从4 mm增大到10 mm，反射波衰减速度增大，但增大到12 mm后衰减量反而有所减小。研究结果可为分离式Hopkinson压杆试验透反射波形的深入分析以及精细化试验设计与数据处理提供参考。Abstract: Compared to the reflection and transmission analysis process during the incident wave loading phase, the incident wave plateau phase lasts longer, and the elastic-plastic propagation and evolution behavior are much more complex. The effects of elastic-plastic wave interactions within the specimen during this phase are very pronounced. Using the elastic-plastic incremental wave theory, combined with numerical simulation, the calculations of elastic-plastic wave’s interactions inside the specimens under rectangular incident wave action and its elastic-plastic transmission and reflection behavior at the two interfaces are carried out. The attenuation characteristics of the reflected waves in the sandwich rod system are investigated. The results show that under strong incident wave action, the specimen internally forms a curve-shaped elastic-plastic interface due to the interactions of elastic-plastic waves. This causes the transmission end to reach the yield state significantly earlier. This elastic-plastic interface propagates towards the reflection end at a speed greater than the elastic sound speed. The attenuation of the reflected wave during the plastic phase is the sum of the increase in generalized wave impedance due to the increase in the specimen’s cross-sectional area and the increase in the number of back-and-forth plastic waves caused by compression. Calculations also show that although the change of the specimen density significantly affects its wave speed and wave impedance, the sum of the attenuations caused by these two factors is close to zero. Hence, the effect of density changes on the transmission and reflection wave plateau phase can be ignored. An increase in the plastic modulus causes the reflection wave plateau to attenuate faster, but the effect of the specimen’s diameter is not monotonous. When it increases from 4 mm to 10 mm, the reflection wave attenuation speed increases, but when it further increases to 12 mm, the attenuation amount decreases. This study has certain reference value for in-depth analysis of split Hopkinson pressure bar test for the transmission waveforms as well as for the detailed test design and data processing.
-
图 2 矩形入射波加载段简化线性图
Figure 2. Simplified linear diagram of the rectangular incident wave loading phase
图 3 矩形入射波点G之前的物理平面图
Figure 3. Physical plane before point G of the rectangular incident wave
图 4
$ {\bar t_{AG}} + {\text{d}}\bar t $ 时刻应力波传播物理平面图Figure 4. Physical plane of stress wave propagation at a certain moment
$ {\bar t_{AG}} + {\text{d}}\bar t $ 
图 5 DC段透射波与EF反射波相遇透反射特征
Figure 5. Transmission characteristics when the DC segment transmitted wave meets the EF reflected wave
图 6 矩形入射波时杆2的工程应变
Figure 6. Engineering strain of rod 2 during rectangular wave incidence
图 7 矩形入射波杆2径向面积与轴向应变变化率
Figure 7. Rate of change of radial area and axial strain of rod 2 during rectangular wave incidence
图 8 不同杆2直径时反射波的衰减
Figure 8. Attenuation of reflected waves varied with rod 2 diameters
图 9 无量纲密度为1/4和1/5时透反射物理平面图
Figure 9. Physical plane of transmission at dimensionless densities of 1/4 and 1/5
图 10 矩形波入射时杆2在不同无量纲密度时的工程应变
Figure 10. Engineering strain of rod 2 at different dimensionless densities during rectangular wave incidence
图 11 杆2在不同塑性模量时的矩形入射波工程应变
Figure 11. Engineering strain during rectangular wave incidence at different plastic module
图 12 不同塑性模量时反射波衰减理论与仿真
Figure 12. Theoretical and simulated attenuation of reflected waves varied with different plastic module
图 13 不同塑性模量时矩形入射波的透射波
Figure 13. Transmitted waves of rectangular incident waves varied with different plastic module
表 1 入射增量波在不同直径双线性杆2时两个界面上的应力透反射系数
Table 1. Stress transmission coefficient on two interfaces of the incident incremental wave in bilinear rods of different diameters
杆2直径/mm $ {F}_{\sigma 1,\text{e}}(=-{F}_{\sigma 2,\text{e}}) $ $ {T_{\sigma 1,{\text{e}}}} $ $ {T_{\sigma 1,{\text{p}}}} $ $ {F}_{\sigma 1,\text{p}}(=-{F}_{\sigma 2,\text{p}}) $ $ {T_{\sigma 2,{\text{e}}}} $ $ {T_{\sigma 2,{\text{p}}}} $ 4 −0.859 1.859 0.097 −0.993 0.141 0.152 6 −0.708 1.708 0.097 −0.983 0.292 0.340 8 −0.533 1.533 0.096 −0.971 0.467 0.600 10 −0.355 1.355 0.095 −0.955 0.645 0.930 12 −0.187 1.187 0.094 −0.935 0.813 1.326 
下载: 导出CSV
表 2 矩形波入射时不同直径双线性杆2的特征点参数和首次反射波强度
Table 2. Characteristic point parameters and first reflected wave strength of bilinear rod 2 with different diameters under rectangular wave incidence
杆2直径/mm 点C无量纲应力 点D无量纲应力 首次反射波强度 最大压缩应变 最大截面积膨胀率 4 −0.430 −0.232 −0.091 0.237 0.311 6 −0.468 −0.274 −0.083 0.209 0.264 8 −0.522 −0.340 −0.071 0.175 0.212 10 −0.590 −0.435 −0.057 0.133 0.153 12 −0.674 −0.568 −0.041 0.091 0.100
下载: 导出CSV
表 3 矩形波入射时不同直径双线性杆2截面积的膨胀效应
Table 3. Cross-sectional area expansion effect of bilinear rod 2 with different diameters under rectangular wave incidence
杆2直径/
mm反射波峰值
应力膨胀引起
直接衰减塑性波反射
衰减反射波
总衰减4 −0.935 − 0.0202 − 0.0445 −0.065 6 −0.854 − 0.0385 − 0.0890 −0.127 8 −0.742 − 0.0547 − 0.1305 −0.186 10 −0.601 − 0.0610 − 0.1565 −0.218 12 −0.431 − 0.0569 − 0.1537 −0.211
下载: 导出CSV
-
[1] 高光发. 夹心杆系统中一维弹塑性波演化精细分析(Ⅰ): 典型加载波的透反射[J]. 爆炸与冲击, 2024, 44(8): 081441. DOI: 10.11883/bzycj-2023-0389.GAO G F. Meticulous analysis of one-dimensional elastic-plastic wave evolution in sandwich bar system (part Ⅰ): transmitted and reflected waves for typical loading waves[J]. Explosion and Shock Waves, 2024, 44(8): 081441. DOI: 10.11883/bzycj-2023-0389. [2] BRIZARD D, JACQUELIN E. Uncertainty quantification and global sensitivity analysis of longitudinal wave propagation in circular bars: application to SHPB device [J]. International Journal of Solids and Structures, 2018, 134: 264–271. DOI: 10.1016/j.ijsolstr.2017.11.005. [3] YANG H S, LI Y L, ZHOU F H. Propagation of stress pulses in a Rayleigh-Love elastic rod [J]. International Journal of Impact Engineering, 2021, 153: 103854. DOI: 10.1016/j.ijimpeng.2021.103854. [4] BRAGOV A M, LOMUNOV A K, LAMZIN D A, et al. Dispersion correction in split-Hopkinson pressure bar: theoretical and experimental analysis [J]. Continuum Mechanics and Thermodynamics, 2022, 34(4): 895–907. DOI: 10.1007/s00161-019-00776-0. [5] RIGBY S E, BARR A D, CLAYTON M. A review of pochhammer-chree dispersion in the hopkinson bar [J]. Proceedings of the Institution of Civil Engineers-Engineering and Computational Mechanics, 2018, 171(1): 3–13. DOI: 10.1680/jencm.16.00027. [6] LIU P, HU D A, WU Q K, et al. Sensitivity and uncertainty analysis of interfacial effect in SHPB tests for concrete-like materials [J]. Construction and Building Materials, 2018, 163: 414–427. DOI: 10.1016/j.conbuildmat.2017.12.118. [7] KARIEM M A, BEYNON J H, RUAN D. Misalignment effect in the split Hopkinson pressure bar technique [J]. International Journal of Impact Engineering, 2012, 47: 60–70. DOI: 10.1016/j.ijimpeng.2012.03.006. [8] REN L, YU X M, HE Y, et al. Numerical investigation of lateral inertia effect in dynamic impact testing of UHPC using a Split-Hopkinson pressure bar [J]. Construction and Building Materials, 2020, 246: 118483. DOI: 10.1016/j.conbuildmat.2020.118483. -
计量
- 文章访问数: 167
- HTML全文浏览量: 49
- PDF下载量: 70
- 被引次数: 0