Elastic compensation for dynamic rigid-plastic solutions of structures
-
摘要: 近年来,我国学者以膜力因子法和饱和分析方法相结合为理论工具,对梁、板等结构件在脉冲载荷作用下的塑性大变形行为作了全面深入的研究,为脉冲加载下结构的最终挠度提供了优于历史上各种刚塑性近似解的最佳刚塑性预测公式。然而,由于实际工程应用中金属结构弹塑性动力响应的复杂性和数值模拟的局限性,与考虑材料弹性效应的结果相比,刚塑性解对脉冲加载下结构所预测的最终挠度的误差有多大,是一个亟待解决的关键问题。对这个问题的首阶段研究成果厘清了材料弹性对脉冲加载下结构塑性动态大变形的影响,定量评估了由最佳刚塑性理论解与弹塑性数值模拟得到的最终挠度预测结果之间的差异。在此基础上,提出了补偿弹性效应的策略和方法,即:在已有的最佳刚塑性解预测的挠度基础上添加一个补偿项,将补偿项表达为脉冲载荷强度的效应与结构自身刚度的效应分离的变量函数,并尽量减少待定系数/指数的数量,以求表达式的简洁;根据这些原则在金属结构的主要工程应用领域内选定结构刚度和外载参数的变化范围,对固支梁和固支方板的案例实施拟合与补偿,最后得到了对梁和板增添补偿项后的简单而实用的最终挠度预测公式,其相对误差在3%的范围之内,很适合工程设计应用。文末列表给出了符号与公式的一览,并对梁和方板的结果作了综合和比较。Abstract: In recent years, by combining membrane factor method (MFM) and saturation analysis (SA) as a powerful theoretical tool, scholars in China have made comprehensive studies on the large dynamic plastic deformation of beams, plates and other structures under pulse loading, leading to the best rigid-plastic predictions for the final deflection of the pulse-loaded structures, which are superior to the previously proposed various approximate rigid-plastic solutions. However, due to the complexity of the dynamic elastic-plastic response of structures used in engineering and the limitations of numerical simulations, it is critical to clarify how large is the error generated from the rigid-plastic solutions in predicting the final deflection of pulse-loaded structures compared to the result that takes the elastic effect of material into consideration. Our preliminary study on this issue, which has been published in leading international journals, reveals the effect of material’s elasticity on the large dynamic plastic deformation of structures under pulse loading, and quantitatively evaluates the discrepancies between the final deflection predicted by the best theoretical rigid-plastic solutions and that extracted from elastic-plastic numerical simulations. On this basis, the present paper proposes a strategy to compensate for elastic effect; that is, (1) adding a compensation term to the final deflection predicted by the existing best rigid-plastic solution; (2) expressing the compensation term as an elemental function of variables separation to respectively represent the effects of the pulse intensity and the structural stiffness; and (3) adopting minimum number of undetermined coefficients (or power) in the fitting function to achieve concise formulae. Meanwhile, the variation ranges of structural stiffness and dimensionless load parameter are investigated with reference to metallic structures used in their main application fields. Finally, by implementing the fitting and compensation for the cases of fully-clamped beams and square plates, simple and engineer-friendly formulae for predicting the final deflection of beams and plates are eventually obtained. With the compensation terms being added, the rigid-plastic-solution-based predictions on the final deflection of beams and plates possess a relative error within the range of 3%, which are appropriate and suitable for applications in the engineering design stage. A table at the end of the paper summarizes the major notations and formulae, as well as the comparison between the results on beams and square plates.
-
自20世纪50年代创立结构分析的极限原理以来,采用极限分析方法评估各种工程结构在准静态加载下的极限载荷和初始坍塌机理获得了广泛的工程应用。对于在脉冲载荷或撞击加载下结构的动态响应,绝大部分理论研究也都是基于理想刚塑性的材料假设,通过简化理论模型来获取解析解或半解析解[1-4]。但是,承受脉冲载荷的金属结构在变形初期必然要经历弹性变形阶段,该阶段对结构的变形机制和失效模式所产生的影响仍有待验证。对于脉冲载荷下结构的弹塑性动力响应目前还没有适用的解析方法,而相关的数值模拟又难以获得规律性的认知,因此,定量地评估刚塑性模型所预测的结构最终挠度究竟有多大的误差,是一项极具理论价值和实用意义的工作。
这个问题从1980年代起就引起了冲击动力学学者的注意。Symonds等[5]把弹塑性结构看成是一个由弹簧和质量组成的单自由度系统,求解了它在矩形脉冲和其他形状脉冲作用下的响应,对弹簧刚度无穷大和刚度为有限值的情形加以比较,从而得出了刚塑性预测的位移相对于弹塑性最终位移的误差。他们的结论是,若要刚塑性模型对承受脉冲载荷的弹塑性系统的变形作出合理预测,需要满足2个条件:(1)外载做的功显著大于系统所能储存的最大弹性能(具体地说,如果这二者之比为R,则刚塑性模型预测的最终变形的误差约为1/R);(2)外载脉冲持续的时长要显著小于系统弹性振动的基本周期,否则刚塑性模型预测变形的误差可能发生剧烈波动。Yu[6]对截至1990年代初的相关研究作了评述。
另外,很多研究都揭示出,结构的大变形行为与单自由度的弹簧-质量系统有很大不同。以梁和板这些最基本的结构部件为例,它们在较强的动载作用下,最大挠度将达到或超过自身的厚度,而大挠度诱导产生的轴力/膜力将使它们的承载能力大大增强,因而结构对外载的抵抗力不能简单地用线性弹簧来表征。在这个领域内,过去30年来发展起来的膜力因子法(membrane factor method, MFM)和饱和冲量(saturated impulse)的概念与方法为分析结构大挠度动力响应提供了强有力的理论工具。
自2016年以来,余同希等[7-9]和朱凌等[10]充分利用饱和分析与膜力因子法相结合的理论优势,对各种支承条件下的梁、方板、矩形板在脉冲载荷作用下的动力行为作了透彻的研究,不但获得了优于前人研究的系统理论成果,而且提供了可为工程设计所用的最终挠度预测公式。
在上述系列研究成果中,Tian等分别对简支或固支的梁[11]以及简支或固支的方板[12]作了完整的大变形动力分析。分析采用了膜力因子法,所对应的屈服面精确地反映了弯矩与膜力的相互作用,克服了以往的分析中大都采用方形屈服面只能获得下限解和上限解的局限性。又由于将膜力因子法和饱和分析的应用贯彻到包含瞬态阶段的动力响应全过程,所得到的已是在脉冲载荷作用下梁和方板动力响应的最佳刚塑性解。
但是,随即需要回答一个关键问题:对于脉冲载荷作用下的结构,与考虑材料弹性的结果相比,刚塑性模型所预测的最终挠度的误差有多大?这方面的首阶段研究成果[13-14]的分析方法和主要结论将在本文中首先概述;在此基础上,将提出从已有的最佳刚塑性解预测的挠度出发、针对弹性效应作出补偿的策略;接着,将厘定拟合与补偿的参数范围;然后,分别以固支梁和固支方板为案例,经过数值拟合给出补偿项的数学形式,同时给出加入补偿项后的最终挠度的预测公式及相应的误差范围,可供工程应用;最后,对理论方法作深入讨论,并提出下一步研究的方向。
1. 梁和板的塑性动力响应中的弹性效应概述
Hu等[13]为了定量评估梁动力响应中的弹性效应,首先考虑了梁在矩形脉冲载荷作用下的极限状态,如图1所示,将发生大挠度的梁视为一根发生大变形的塑性弦。
参照这样的极限状态,对能量比
R≡Up/Uemax (塑性功Up 与结构所能存储的最大弹性应变能Uemax 的比值)和特征时间比τ*≡tT/Te(脉冲时长tT与结构基本振动周期Te的比值)作出准确的定义。然后,将刚塑性解预测的最终挠度与有限元法模拟得到的弹塑性最终挠度之间的相对差异定义为最终挠度预测的相对偏差Dη,即:Dη=ηrpf−ηepfηepf (1) 式中:
ηrpf 为刚塑性解预测的最终挠度,其上标rp代表刚塑性解,下标f表示为最终值,也是饱和值;ηepf 为有限元法模拟得到的弹塑性最终挠度,其上标ep代表弹塑性解。分析计算结果发现,Dη与能量比R之间的关联如图2[13]所示。值得注意的是,对于简支梁和固支梁,最终挠度的相对偏差Dη的绝对值可以分别以1/(3R)和1/(2R)作为上界,这显著地小于从单自由度系统得出的1/R。对于大多数工程应用的情况,最终挠度刚塑性预测的相对偏差将保持在10%以内。同时,由于饱和冲量现象的存在,“外载脉冲的时长tT要显著小于结构振动的基本周期Te”这一先前的结论也不再构成对梁的刚塑性解的适用性的限制。这2条新结论都颠覆了业界过往的认知。
Hu等[14]将结构动力响应中弹性效应的研究拓展到二维结构部件,把发生大挠度的方板的极限状态视作一块塑性膜,计及膜力和弯矩对能量耗散的影响,定义了能量比R和特征时间比τ*,揭示了它们同最终挠度的相对偏差Dη的关联。同时为便于工程应用,也可以将最终挠度的相对偏差Dη随无量纲载荷幅值λ≡p0/pY(定义为矩形脉冲的载荷幅值p0与准静态坍塌载荷pY之比)的变化直接表达出来,如图3所示。与梁的情形相同,由于饱和冲量现象的存在,“外载脉冲的时长要显著小于结构振动的基本周期”这一先前的结论也不再构成对方板的刚塑性解的适用性的限制。
Hu等[14]还对梁和方板的弹性效应作了比较。如图3所示,板的弹性效应要比梁的更显著,因此在工程设计中采用刚塑性预测最终变形时需要进行补偿,这正是本文的主题。另一个关键的发现是,各种情形下的挠度预测的相对偏差都同一个无量纲参数ζ相关,它代表了将材料性质与结构几何参数揉合在一起的结构刚度。对于梁和方板,这个结构刚度参数分别为:
ζbeam=hL√EY (2) ζplate≡ha√EY(1−μ) (3) 式中:E、μ和Y分别为材料的杨氏模量、泊松比和屈服应力;对于梁,h和L分别代表厚度和半长;对于方板,h和a分别代表板厚和半边长。如果一根梁的长度同一块方板的边长相等,而其他参数相同,从式(2)和(3)可知,当材料的泊松比μ=0.30时,ζplate比ζbeam要大20%左右。
图4展示了当E/Y=1000及a/h=45时,不同泊松比下固支方板刚塑性解预测的最终挠度的相对偏差随无量纲载荷幅值λ的变化关系。从图4可以看出,对于泊松比μ=0.15, 0.30, 0.45,3条最终挠度相对偏差曲线之间存在差异,随着泊松比μ的增大,最终挠度的相对偏差减小,表明泊松比μ对方板的弹性效应存在一定影响。需要说明的是,由于μ=0.15, 0.30, 0.45时,本例中的ζplate分别为0.762, 0.840和0.948,相互较接近,因此3条偏差曲线间的差异较小,这符合Hu等[14]给出的固支方板最终挠度相对偏差随ζplate的变化规律。
综上所述,Hu等[13-14]分别对梁和方板这2种典型的构件,定量地评估了由最佳的刚塑性理论分析与弹塑性数值模拟得到的最终挠度预测结果之间的相对偏差,厘清了弹性效应对结构塑性动力响应的影响,阐明了刚塑性理论模型在实际工程应用中的适用性。这些结果不但刷新了对结构冲击动力学一些基本问题的认知,而且可以有望推进相应理论结果的工程应用。
2. 对刚塑性模型预测挠度的补偿策略
在引言中已经提到,Tian等[11]采用将膜力因子法与饱和分析结合起来的方法,得出了刚塑性简支和固支梁在均布矩形脉冲作用下的完全解,包括得到了饱和挠度(同时也是刚塑性意义下的最终挠度和最大挠度)、饱和时间和饱和冲量。由于采用了精确屈服面,并考虑了瞬态响应阶段,所以这个刚塑性解是优于先前任何刚塑性解的最佳解。Tian等[12]把这套分析方法推广到方板,同样获得了刚塑性的最佳解。
Tian等[11-12]的一个重要发现是,当脉冲幅值较大时,梁和方板的无量纲最终挠度与无量纲脉冲幅值之间呈现线性关系。这里的无量纲最终挠度定义为梁/板中点的最终挠度w0f除以梁/板的厚度h,即
ηf≡w0f/h ;而无量纲脉冲幅值定义为矩形脉冲压力的幅值p0 除以梁/板的准静态坍塌压力pY ,即λ≡p0/pY 。根据Tian等[11]的结果,采用无量纲脉冲幅值λ(它与Tian等[11]采用的
p0 之间的关系是:对于固支梁λ=p0/4 ,对于简支梁λ=p0/2 ),图5(a)显示了梁在矩形脉冲作用下载荷与最终挠度之间的关系。当对于固支梁和简支梁的λ≥3时,ηf 与λ之间的关系可以用以下直线拟合:ηrpf=C1λ−Cb (4) 对于固支梁,式(4)中的C1和Cb分别取为1.10和0.414;对于简支梁,C1和Cb分别取为0.55和0.183。
类似地,根据Tian等[12]的结果,采用无量纲脉冲幅值λ(它与Tian等[12]采用的p0之间的关系是:对于固支方板
λ=p0/12 ,对于简支方板λ=p0/6 ),图5(b)显示了方板在矩形脉冲作用下载荷与最终挠度之间的关系。当对于固支方板和简支方板的λ≥2时,ηf 与λ之间的关系可以用以下直线拟合:ηrpf=C2λ−Cp (5) 对于固支方板,式(5)中的C2和Cp分别取为2.16和1.456。对于简支方板,C2和Cp分别取为1.08和0.374。
另一方面,如同第1节中介绍的,也采用有限元方法对梁和方板对矩形脉冲的动力响应作了弹塑性模拟,从而得到了梁/板中点在各种条件下的最终挠度
ηepf 。值得注意的是,式(4)~(5)中的
ηrpf 都仅仅与无量纲载荷参数λ有关;但将ηrpf 同有限元模拟得出的ηepf 相比较时,Hu等[13-14]发现二者之差Δη≡ηepf−ηrpf 与2个参数相关:一个仍是无量纲载荷参数λ,另一个是结构刚度参数ζ,对于梁和方板分别由前面的式(2)和式(3)给出。注意,这里所说的Δη 是绝对偏差,不同于式(1)定义的相对偏差。依据上述分析,本文中提出的策略是将梁或板的最终挠度的最佳预测
η*f 表示为2项之和,即:η∗f=ηrpf(λ)+Δ∗η(λ,ζ) (6) 式中:
ηrpf(λ) 采用已有的结果,即式(4)和(5),而补偿项Δ∗η(λ,ζ) 来自对Δη≡ηepf−ηrpf 离散数据所作的、用初等函数表达的拟合。为了方便工程设计估算,我们的目标是,拟合时尽量采用较简单的函数形式和尽量少的待定系数(或幂指数),以求表达式的简洁,同时将梁或板的最终挠度的最佳预测η*f 与弹塑性有限元模拟得到的最终位移ηepf 的相对偏差控制在工程应用可以接受的3%之内。3. 最终挠度补偿的预期适用范围
如同所有的拟合公式一样,对最终挠度预测的补偿所追求的是在某个参数域内的最优。下面将结合方板的主要工程应用领域,来确定我们需要考虑的参数域;参照式(6),也就是需要给出λ和ζ这2个参数的变化范围。
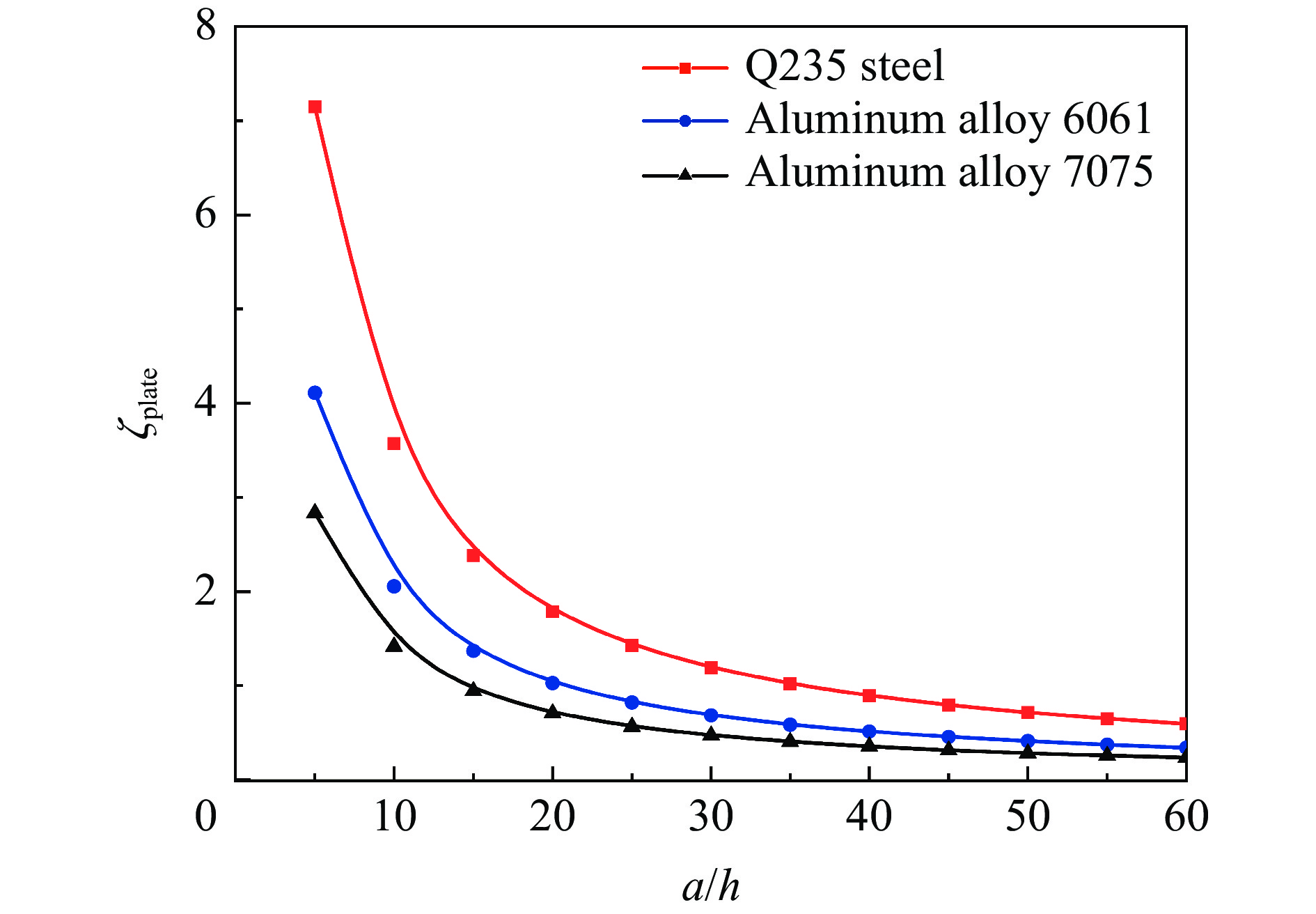
在机械、汽车、航空航天和船舶海洋结构中最常用的金属材料是低碳钢和铝合金。它们的材料性质见表1。表2列出了上述工程领域中金属板的半边长与板厚之比(a/h)的常用范围。可以将这些数据代入式(3)计算方板的结构刚度ζplate。在5≤a/h≤60的范围内,ζplate随a/h的变化如图6所示。
表 1 低碳钢和铝合金的材料性质Table 1. Material properties of mild steel and aluminum alloys材料 杨氏模量E/GPa 泊松比μ 屈服应力Y/MPa 低碳钢Q235 210 0.3 235 铝合金6061 71 0.3 240 铝合金7075 71 0.3 505 表 2 金属板的半边长与板厚之比 的常用范围Table 2. Commonly-used ranges of half length-to-plate thickness ratio for metallic plates工程领域 a/h 船舶海洋结构 10~125 汽车与运载机械 150~900 航空航天结构 80~1500 从图6可以看出,随着a/h的增大,ζplate大体上趋近于0.5。因此,对于最常用到的情形,我们可以按照a/h≥10的低碳钢方板和a/h≥5的6061铝合金方板确定ζplate的取值范围为0.5≤ζplate≤4。至于梁,适当放宽取值范围到0.5≤ζbeam≤6。
另外,载荷参数λ的取值范围可以简单直接地确定。很显然,
λ≡p0/pY ≤1属于低载情形,只产生弹性变形,因而无须考虑。同时,从图5可以发现,ηrpf 与λ间的线性关系在λ≡p0/pY ≥1.5的范围内都是大致成立的;所以,下面作拟合时只考虑λ≥1.5。4. 对刚塑性梁预测的挠度的补偿
采用第2节中设定的补偿策略和第3节中给出的参数
ζbeam≡hL√EY 和λ≡p0/pY 的变化范围,对承受矩形脉冲的固支梁,按照刚塑性解所预测的最终挠度与弹塑性有限元模拟得到的最终挠度之间的绝对偏差Δη≡ηepf−ηrpf 进行拟合,并将拟合得到的、以初等函数表达的Δ∗η(λ,ζ) 作为一个补偿项,对刚塑性解所预测的最终挠度进行修正,按式(6)给出固支梁的最终挠度的最佳预测η*f 。这一预测保证了它同弹塑性模拟得到的最终挠度之间的相对偏差保持在3%以内,即:D∗η=η∗f−ηepfηepf≤3% (7) 为简洁起见,本节中将ζbeam简记为ζ。取补偿项
Δ∗η(λ,ζ) 为λ和ζ的分离变量函数,即Δ∗η(λ,ζ)=ϕ(λ)Ψ(ζ) ;经初步拟合之后,发现可以将Ψ(ζ) 取为ζ的幂函数,同时将ϕ(λ) 取为λ的一次式。在这些条件下,拟合的结果为:Δ∗η=(−0.08λ+0.29)ζ−0.9 (8) 将式(4)、(8)代入式(6),得到补偿后固支梁的最终挠度预测为:
η∗f=1.10λ−0.414+(−0.08λ+0.29)ζ−0.9 (9) 式(9)预测的最终挠度与弹塑性模拟结果间的相对偏差如图7所示。可以看到,它满足:
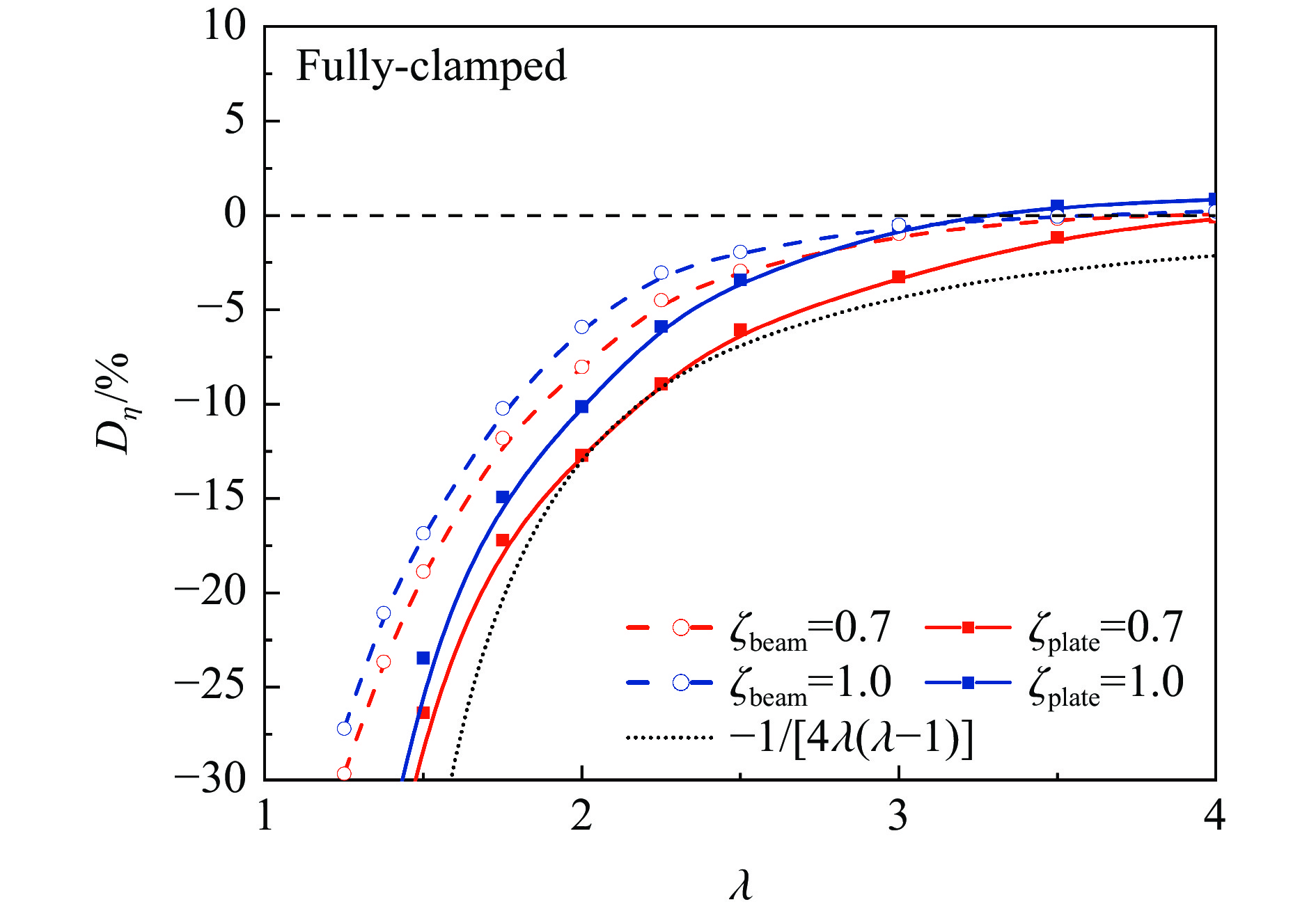
D∗η=η∗f−ηepfηepf≤3% 同弹塑性有限元模拟得到的固支梁最终挠度相比,补偿前后的相对偏差Dη发生了显著的变化,如图8所示。很明显,适当的补偿不但使得最终挠度的相对偏差明显减小,而且这些偏差都朝着Dη正值移动。这正是我们所希望的,因为符号为正的、小的Dη值意味着把理论预测值用于设计估算将略偏于安全。
 图 8 补偿前(实线)和按式(8)作补偿后(虚线)与弹塑性有限元模拟得到的固支梁最终挠度之间的相对偏差Figure 8. The relative discrepancies in the final deflection of fully-clamped beams between rigid-plastic predictions (solid lines displaying before compensation, and broken lines displaying after compensation given by Eq.(8)) and elastic-plastic simulation results
图 8 补偿前(实线)和按式(8)作补偿后(虚线)与弹塑性有限元模拟得到的固支梁最终挠度之间的相对偏差Figure 8. The relative discrepancies in the final deflection of fully-clamped beams between rigid-plastic predictions (solid lines displaying before compensation, and broken lines displaying after compensation given by Eq.(8)) and elastic-plastic simulation results5. 对刚塑性方板预测的挠度的补偿
采用与第4节中对固支梁最终挠度的补偿相类似的方法,将梁的结构刚度
ζbeam≡hL√EY 替换为板的结构刚度ζplate≡ha√EY(1−μ) ,就可以获得对固支方板最终挠度的补偿结果。为简洁起见,以ζ代表ζplate。补偿方案1。仍取补偿项
Δ∗η(λ,ζ) 为λ和ζ的分离变量函数,Δ∗η(λ,ζ)=ϕ(λ)Ψ(ζ) ,其中Ψ(ζ) 取为ζ的幂函数,同时将ϕ(λ) 取为λ的一次式。在这些条件下,拟合的结果为:Δ∗η=(−0.17λ+0.67)ζ−0.4 (10) 将式(5)、(10)代入式(6),得到补偿后固支方板的最终挠度预测为:
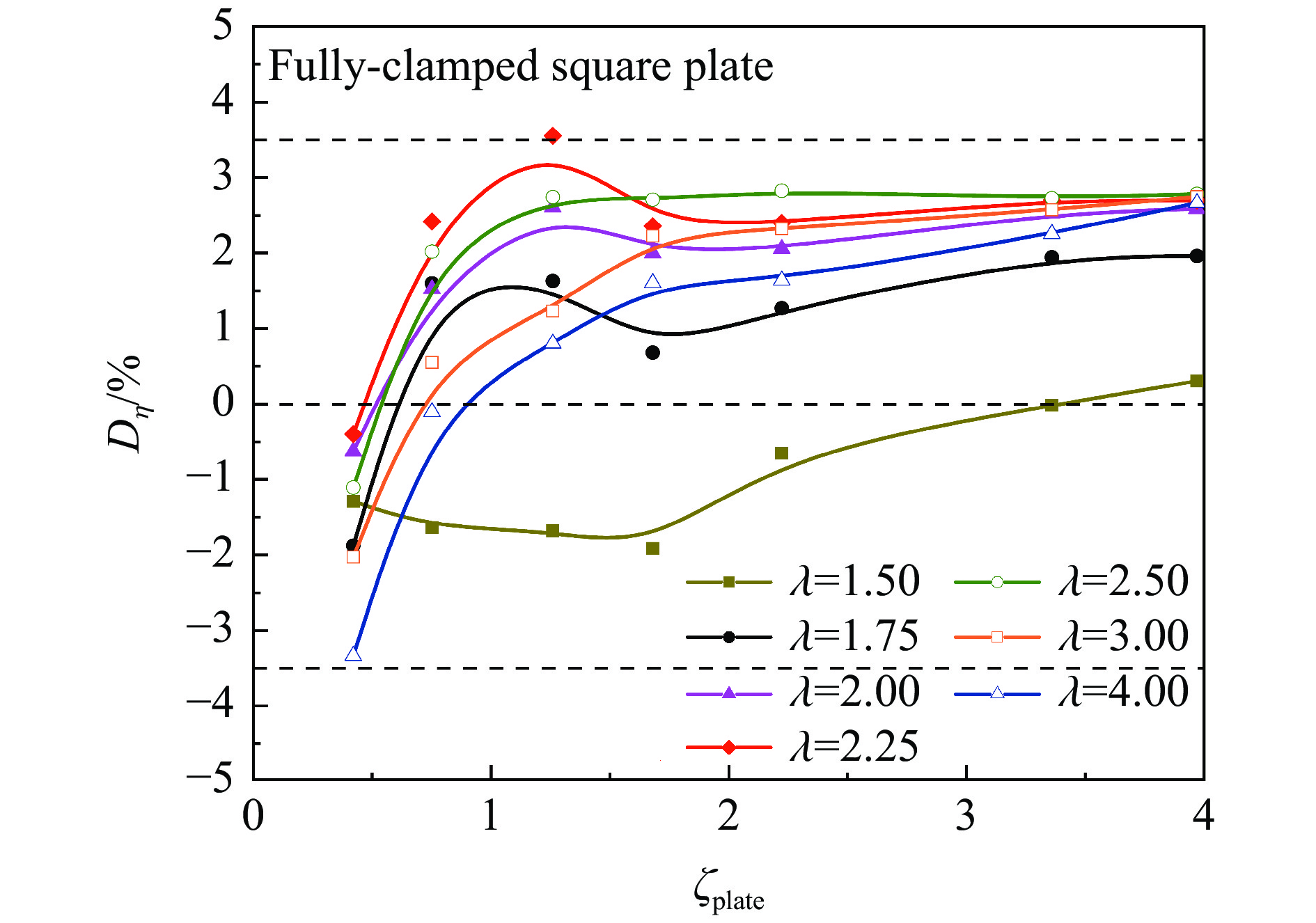
η∗f=2.160λ−1.456+(−0.17λ+0.67)ζ−0.4 (11) 式(11)预测的最终挠度与弹塑性模拟结果间的相对偏差如图9所示。可以看到,它的精确度稍低,只在3.5%范围之内。
鉴于补偿方案1的相对偏差Dη大于预设的3%,为了减小相对偏差Dη,在补偿方案2中,仍然取分离变量的拟合函数
Δ∗η(λ,ζ)=ϕ(λ)Ψ(ζ) ,其中的Ψ(ζ) 取为ζ的幂函数,但将ϕ(λ) 取为λ的二次式。在这些条件下,拟合的结果为:Δ∗η=(0.03λ2−0.33λ+0.85)ζ−0.4 (12) 将式(5)、(12)代入式(6),得到补偿后的最终挠度预测为:
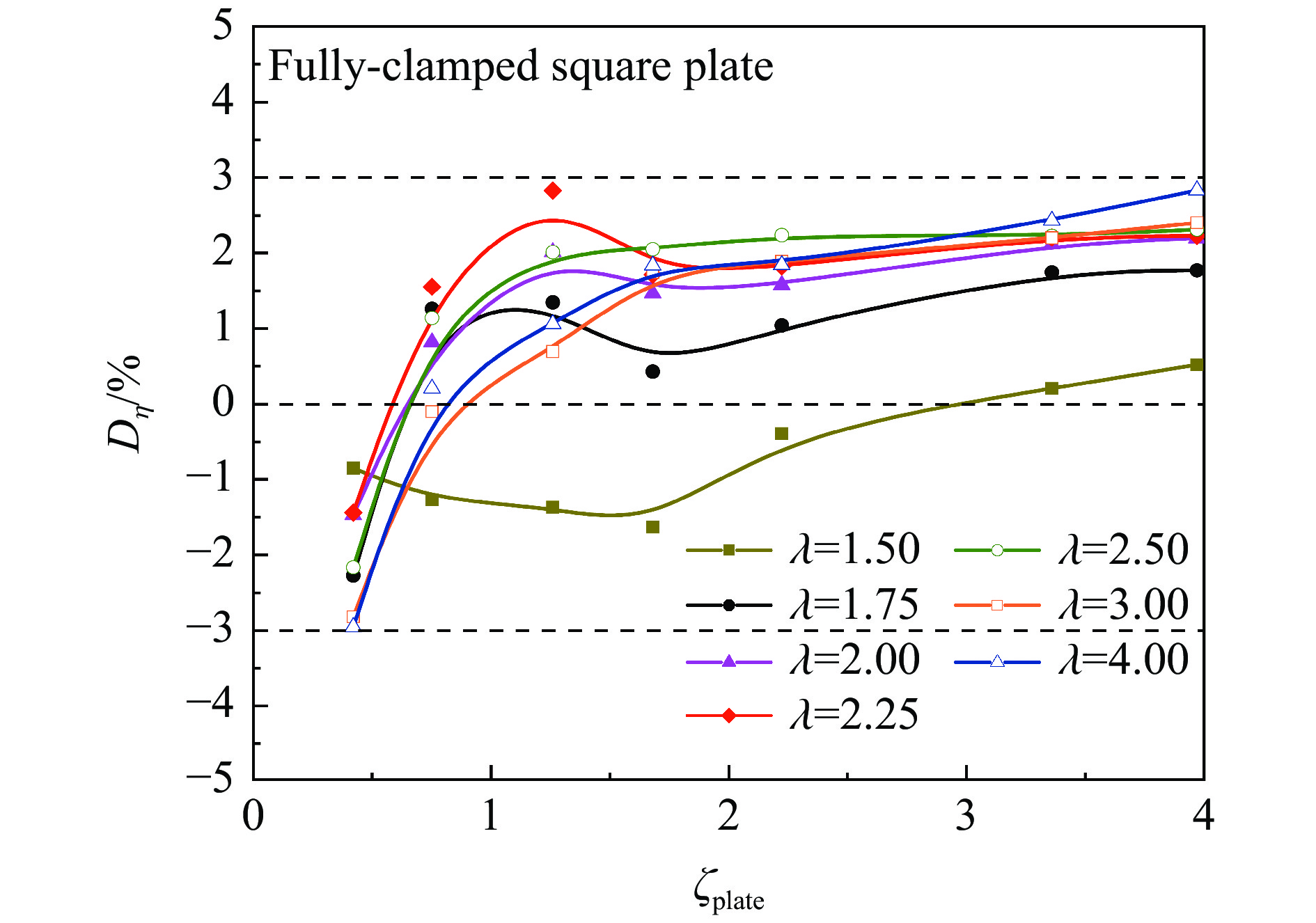
η∗f=2.160λ−1.456+(0.03λ2−0.33λ+0.85)ζ−0.4 (13) 式(13)预测的最终挠度与弹塑性模拟结果间的相对偏差如图10所示。可以看到,它满足:
D∗η=η∗f−ηepfηepf≤3% 6. 讨论与结语
针对脉冲载荷作用下梁和方板的最终挠度预测,为了对弹性效应引起的偏差进行拟合与补偿,强调了必须采用最佳的刚塑性动力学解来同有限元模拟结果比较。这是因为,数十年来,对梁、板等结构已经构建出了多种多样的刚塑性近似解,虽然它们也能对结构的最终变形作出估计,但是由于这些解采用了多种近似假定,它们对最终变形的预测包含了各种原因引起的误差,除弹性效应引起的误差外,还可能有来自近似屈服条件或忽略瞬态响应阶段等原因产生的误差。因此,如果刚塑性解本身就带有太多近似,那么它们并不适合用来分析弹性效应,也不能够作为构建补偿的基础。
如前文所述,借助了饱和分析和膜力因子法,近年来获得了优于先前任何刚塑性解的最佳解[11-12]。同弹塑性有限元模拟结果相比,这些最佳刚塑性解仍然不可避免地显现出偏差,但这种偏差主要来自弹性效应(对于低碳钢等应变率敏感材料,刚塑性解通常都未能较准确地计入应变率效应,也会带来偏差),因此所得到的偏差随参量变化的数据集合为补偿弹性效应奠定了可靠的基础。本文便是在这样的基础上探讨了补偿的策略和方法,并对梁和方板的案例加以实施。
在对偏差进行拟合与补偿的过程中,还注意到弹塑性有限元模拟得到的最终挠度本身的精确度是不容易控制的。影响模拟得到的最终挠度的精确度的因素很多,诸如单元的种类和大小、时间步长的选取,尤其是在结构动态变形的尾段,塑性变形已经饱和但弹性振动仍在持续,如何从弹性振动的时间序列中提取最终挠度,依然具有相当大的不确定性。这也反映到文献[13-14]画出的偏差Dη曲线某些段落显得并不平顺。从文献[13-14]的数据分析估计,弹塑性有限元模拟得到的最终挠度本身可能带有1%~2%量级的误差,因此过分追求拟合和补偿的精确度是没有必要的。
能量比R(即塑性耗散能与结构所能储存的最大弹性能之比)是表征结构动态响应的重要力学量。在对刚塑性梁和板推导R表达式的过程中,发现它仅仅与无量纲载荷参数λ和结构刚度参数ζ相关;因此,这2个关键参数完全是从理论推导中自然出现的,在深入分析中聚焦于这2个参数具有充分的力学依据。
依据上述基本分析,合理地选择了补偿策略,它包括以下要素:(1)补偿项只含2个无量纲参数λ和ζ,它们的变化范围根据工程上常用的材料性质和结构尺度加以确定;(2)选取分离变量的补偿函数
Δ∗η(λ,ζ)=ϕ(λ)Ψ(ζ) ,从力学上看,ϕ(λ) 代表外加载荷的强度的效应,而Ψ(ζ) 反映结构自身的刚度的影响;(3)为便于在设计估算中使用,拟合时对ϕ(λ) 和Ψ(ζ) 采用简单的函数形式和尽量少的待定系数/指数;(4)考虑到有限元模拟本身存在一定数值误差,结合工程设计的需要,把补偿后的最终挠度的相对精度设定在3%之内,避免过度追求精度而牺牲了预测公式的简明性。将本文的主要符号、公式和补偿结果进行整理见表3,同时也对固支梁和固支方板的结果作出了对比。
表 3 主要结果整理及固支梁与固支方板的比较Table 3. Summary of main results as well as comparison of fully-clamped beams and plates项目 固支梁(矩形截面) 固支方板 几何参数 长度2L,宽度b,厚度h 边长2a,厚度h 材料参数 杨氏模量E,屈服应力Y 杨氏模量E,泊松比μ,屈服应力Y 塑性极限弯矩 Mp = Ybh2/4 M0 = Yh2/4 准静态坍塌压力 线载荷pY = 4Mp/(bL) = Yh2/L 面载荷pY = 12M0/a2 = 3Yh2/a2 无量纲压力 λ ≡ p0/pY 结构刚度ζ ζbeam≡hL√EY ζplate≡ha√EY(1−μ) 最佳刚塑性解预测的最终挠度 ηrpf≡wrp0fh=1.10λ−0.414 ηrpf≡wrp0fh=2.16λ−1.456 刚塑性解加上弹性补偿后预测的最终挠度 η*f=1.10λ−0.414+(−0.08λ+0.29)ζ−0.9 η*f=2.160λ−1.456+(0.03λ2−0.33λ+0.85)ζ−0.4 弹塑性模拟得到的最终挠度 ηepf≡wep0fh 上述二者之间的相对偏差 D*η=η*f−ηepfηepf≤3% 在本文中,对脉冲载荷作用下梁和板最终挠度的预测仅限于矩形脉冲情形。对于结构受到非矩形脉冲的情形,需要尝试结合不同的脉冲等效技术[15-18],先将非矩形脉冲(如三角形脉冲、爆炸脉冲等)等效转化为矩形脉冲,再应用本文的结果。但是,本文的补偿策略同脉冲等效相结合是否确实能够合理地预测非矩形脉冲载荷作用下结构的最终挠度,是需要仔细检验的,并在必要时作进一步的修正。此外,如同上面已提到的,对于率敏感材料,需要评估和补偿应变率效应引起的最终变形预测上的偏差。这些都将是下一步的研究目标。
-
图 8 补偿前(实线)和按式(8)作补偿后(虚线)与弹塑性有限元模拟得到的固支梁最终挠度之间的相对偏差
Figure 8. The relative discrepancies in the final deflection of fully-clamped beams between rigid-plastic predictions (solid lines displaying before compensation, and broken lines displaying after compensation given by Eq.(8)) and elastic-plastic simulation results
表 1 低碳钢和铝合金的材料性质
Table 1. Material properties of mild steel and aluminum alloys
材料 杨氏模量E/GPa 泊松比μ 屈服应力Y/MPa 低碳钢Q235 210 0.3 235 铝合金6061 71 0.3 240 铝合金7075 71 0.3 505 表 2 金属板的半边长与板厚之比 的常用范围
Table 2. Commonly-used ranges of half length-to-plate thickness ratio for metallic plates
工程领域 a/h 船舶海洋结构 10~125 汽车与运载机械 150~900 航空航天结构 80~1500 表 3 主要结果整理及固支梁与固支方板的比较
Table 3. Summary of main results as well as comparison of fully-clamped beams and plates
项目 固支梁(矩形截面) 固支方板 几何参数 长度2L,宽度b,厚度h 边长2a,厚度h 材料参数 杨氏模量E,屈服应力Y 杨氏模量E,泊松比μ,屈服应力Y 塑性极限弯矩 Mp = Ybh2/4 M0 = Yh2/4 准静态坍塌压力 线载荷pY = 4Mp/(bL) = Yh2/L 面载荷pY = 12M0/a2 = 3Yh2/a2 无量纲压力 λ ≡ p0/pY 结构刚度ζ ζbeam≡hL√EY ζplate≡ha√EY(1−μ) 最佳刚塑性解预测的最终挠度 ηrpf≡wrp0fh=1.10λ−0.414 ηrpf≡wrp0fh=2.16λ−1.456 刚塑性解加上弹性补偿后预测的最终挠度 η*f=1.10λ−0.414+(−0.08λ+0.29)ζ−0.9 η*f=2.160λ−1.456+(0.03λ2−0.33λ+0.85)ζ−0.4 弹塑性模拟得到的最终挠度 ηepf≡wep0fh 上述二者之间的相对偏差 D*η=η*f−ηepfηepf≤3% -
[1] JOHNSON W. Impact strength of materials [M]. London: Edward Arnord, 1983. [2] JONES N. Structural impact [M]. 2nd ed. Cambridge: Cambridge University Press, 2011. [3] STRONGE W J, YU T X. Dynamic models for structural plasticity [M]. Berlin: Springer-Verlag, 2012. [4] 余同希, 邱信明. 冲击动力学 [M]. 北京: 清华大学出版社, 2011. [5] SYMONDS P S, FRYE C W G. On the relation between rigid-plastic and elastic-plastic predictions of response to pulse loading [J]. International Journal of Impact Engineering, 1988, 7(2): 139–149. DOI: 10.1016/0734-743X(88)90022-X. [6] YU T X. Elastic effect in the dynamic plastic response of structures [M]//JONES N, WIERZBICKI T. Structural Crashworthiness and Failure. London: Springer-Verlage, 1993: 341–384. DOI: 10.1201/9781482262544. [7] 余同希, 朱凌, 许骏. 结构冲击动力学进展(2010−2020) [J]. 爆炸与冲击, 2021, 41(12): 121401. DOI: 10.11883/bzycj-2021-0113.YU T X, ZHU L, XU J. Progress in structural impact dynamics during 2010−2020 [J]. Explosion and Shock Waves, 2021, 41(12): 121401. DOI: 10.11883/bzycj-2021-0113. [8] 余同希, 朱凌, 陈发良. 饱和冲量与膜力因子法: 强动载荷下结构塑性大变形的分析和预测方法 [M]//陈建康, 白树林. 材料的非线性力学性能研究进展. 北京: 机械工业出版社, 2021: 17–29. [9] 余同希, 田岚仁, 朱凌. 强脉冲载荷作用下结构塑性大变形的最大挠度直接预测 [J]. 力学学报, 2023, 55(5): 1113–1123. DOI: 10.6052/0459-1879-22-607.YU T X, TIAN L R, ZHU L. Direct prediction of maximum deflection for plastically deformed structures under intense dynamic pulse [J]. Chinese Journal of Theoretical and Applied Mechanics, 2023, 55(5): 1113–1123. DOI: 10.6052/0459-1879-22-607. [10] 朱凌, 田岚仁, 李德聪, 等. 饱和冲量及其等效方法在舱室内爆炸中的应用 [J]. 中国舰船研究, 2021, 16(2): 99–107. DOI: 10.19693/j.issn.1673-3185.01876.ZHU L, TIAN L R, LI D C, et al. Saturated impulse and application of saturation equivalent method in cabin explosion [J]. Chinese Journal of Ship Research, 2021, 16(2): 99–107. DOI: 10.19693/j.issn.1673-3185.01876. [11] TIAN L R, CHEN F L, ZHU L, et al. Saturated analysis of pulse-loaded beams based on Membrane Factor Method [J]. International Journal of Impact Engineering, 2019, 131: 17–26. DOI: 10.1016/j.ijimpeng.2019.04.021. [12] TIAN L R, CHEN F L, ZHU L, et al. Large deformation of square plates under pulse loading by the combination of Saturated Analysis and Membrane Factor Method [J]. International Journal of Impact Engineering, 2020, 140: 103546. DOI: 10.1016/j.ijimpeng.2020.103546. [13] HU Q J, ZHU L, YU T X. Elastic effects on the dynamic plastic deflection of pulse-loaded beams [J]. International Journal of Impact Engineering, 2023, 176: 104550. DOI: 10.1016/j.ijimpeng.2023.104550. [14] HU Q J, ZHU L, YU T X. Elastic effect on the final deflection of rigid-plastic square plates under pulse loading [J]. Thin-Walled Structures, 2023, 193: 111238. DOI: 10.1016/j.tws.2023.111238. [15] YOUNGDAHL C K. Correlation parameters for eliminating the effect of pulse shape on dynamic plastic deformation [J]. Journal of Applied Mechanics, 1970, 37(3): 744–752. DOI: 10.1115/1.3408605. [16] YOUNGDAHL C K. Influence of pulse shape on the final plastic deformation of a circular plate [J]. International Journal of Solids and Structures, 1971, 7(9): 1127–1142. DOI: 10.1016/0020-7683(71)90057-6. [17] ZHU L, TIAN L R, CHEN F L, et al. A new equivalent method for complex-shaped pulse loading based on saturation analysis and membrane factor method [J]. International Journal of Impact Engineering, 2021, 158: 104018. DOI: 10.1016/j.ijimpeng.2021.104018. [18] CHEN F L, TIAN L R, YU T X, et al. Complete solution of large plastic deformation of square plates under exponentially decaying pulse loading [J]. Acta Mechanica Solida Sinica, 2021, 34(6): 922–936. DOI: 10.1007/s10338-021-00280-6. 期刊类型引用(0)
其他类型引用(2)
-








 下载:
下载:












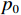







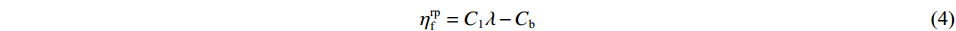







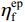



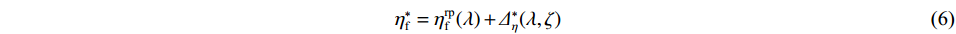










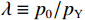























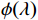







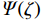


 下载:
下载: