细观非均匀介质中的耦合波动传播

陆建华; 袁良柱; 谢雨珊; 陈美多; 王鹏飞; 徐松林

doi:10.11883/bzycj-2023-0438

细观非均匀介质中的耦合波动传播

doi: 10.11883/bzycj-2023-0438

陆建华^1,,
袁良柱¹,
谢雨珊¹,
陈美多¹,
王鹏飞¹,
徐松林^{1, 2, ,}

1.
中国科学技术大学中国科学院材料力学行为和设计重点实验室，安徽合肥 230027
2.
中国地震局地震预测研究所高压物理与地震科技联合实验室，北京 100036

基金项目: 国家自然科学基金（11672286，11872361）；高压物理与地震科技联合实验室开放基金（2019HPPES01）；中国石油与中国科学院重大战略合作项目（2015A-4812）；中央高校基本科研业务费专项资金（WK2480000008）

详细信息

作者简介:
陆建华（1997－　），男，博士研究生，lujianhua@mail.ustc.edu.cn

通讯作者:
徐松林（1971－　），男，博士，研究员，博士生导师，slxu99@ustc.edu.cn

中图分类号: O347
计量
- 文章访问数: 267
- HTML全文浏览量: 70
- PDF下载量: 93
- 被引次数: 0
出版历程
- 收稿日期: 2023-12-12
- 修回日期: 2024-02-29
- 网络出版日期: 2024-03-12
- 刊出日期: 2024-09-20

Coupled wave propagation in meso-scale heterogeneous medium

1.
CAS Key Laboratory for Mechanical Behavior and Design of Materials, University of Science and Technology of China, Hefei 230027, Anhui, China
2.
United Laboratory of High-Pressure Physics and Earthquake Science, Institute of Earthquake Forecasting, China Earthquake Administration, Beijing 100036, China

摘要

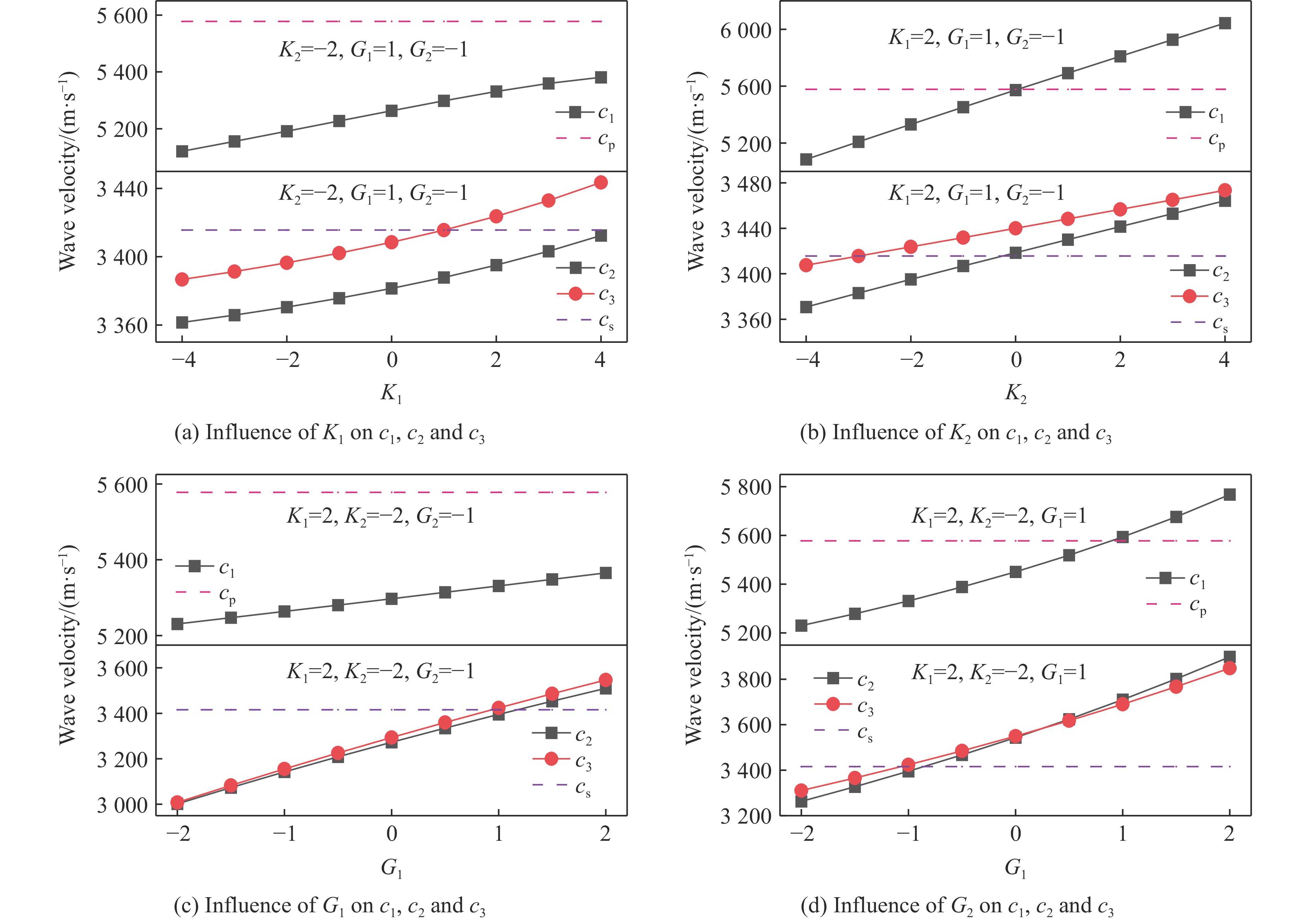
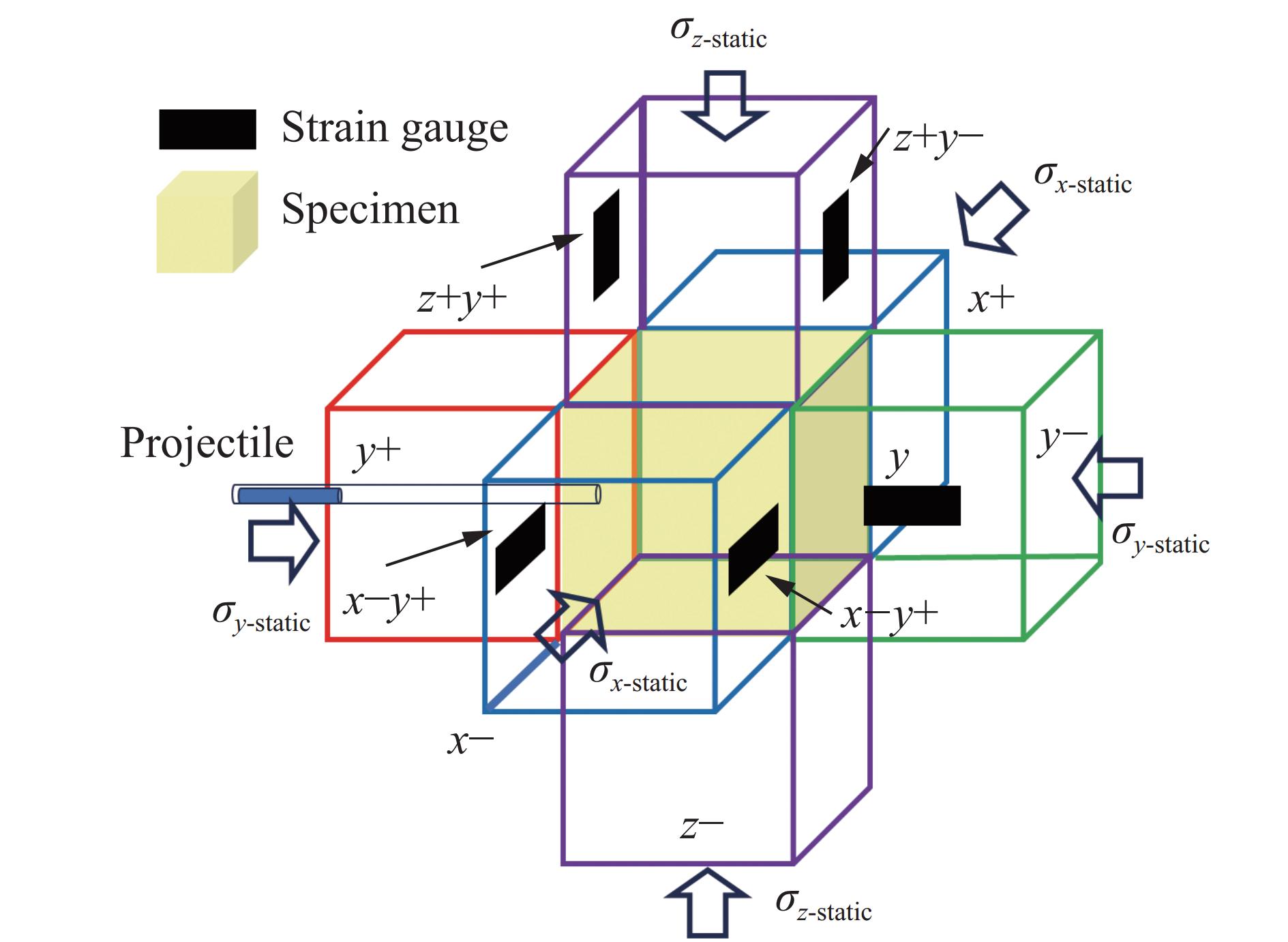
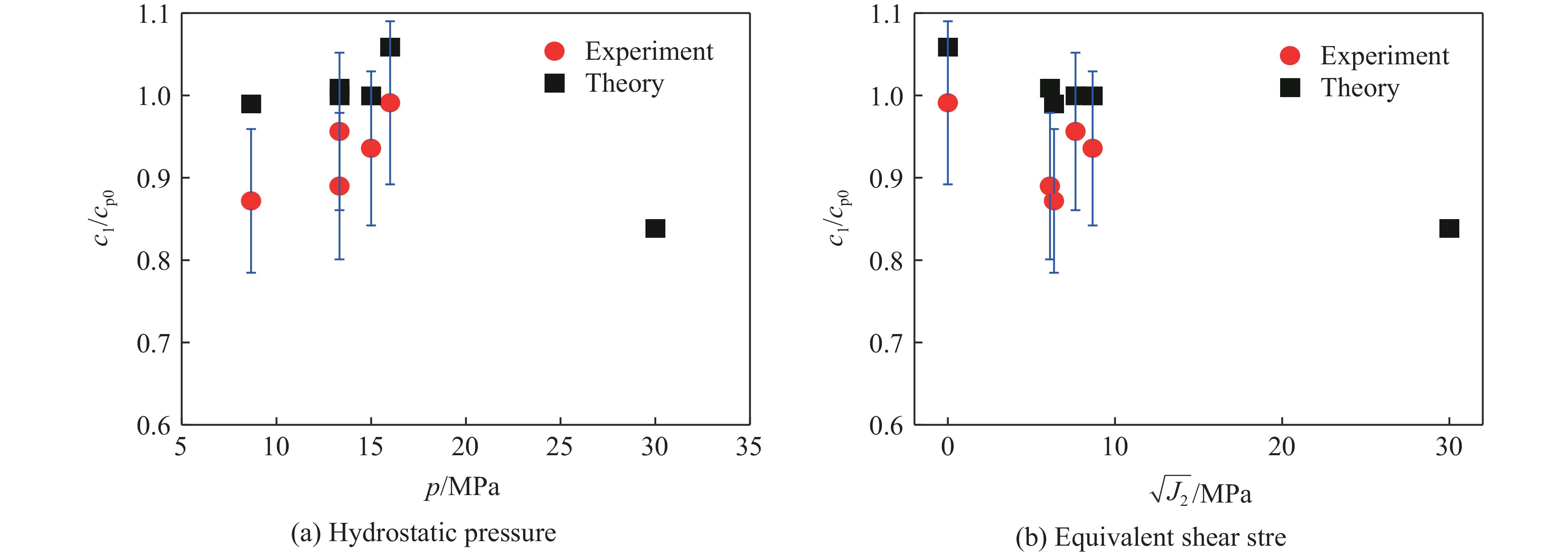
摘要: 非均匀介质在自然界中十分常见，针对细观非均匀介质的波动力学行为和非均匀性描述的研究具有重要意义并充满挑战。建立了反映细观非均匀材料压剪耦合特性的一般压剪耦合本构关系，提出了描述材料非均匀性的耦合系数，并建立了广义波动方程。广义波动方程数值分析表明，耦合系数的正负、取值和组合与应力/应变张量共同影响耦合波动传播过程。作为算例，给出了一阶近似的压剪耦合参数确定的本构关系以及3个压剪耦合特征波速的表达式，并利用有限差分法得到了耦合压缩波和剪切波的传播过程。研究了4个非均匀性耦合系数对应力状态、耦合波速和波传播过程的影响。耦合压缩波速反映了剪切对压缩的耦合效应和体积压实效应2种机制的竞争，耦合剪切波速反映了压缩对剪切的耦合效应和介质持续畸变带来的剪切弱化效应2种机制的竞争。这些机制可通过压剪耦合参数的不同组合来实现。应用真三轴实验系统测量了花岗岩、由砂浆制成的模型材料、具有粗骨料的水泥砂浆制成的材料3种非均匀介质在不同压剪应力下的纵波波速。结果表明，体积压实效应普遍存在，而非均匀程度越高，材料伸缩的同时完成切向的畸变导致压缩波的速度显著降低，剪切对纵波波速的影响越占据主导。理论计算结果与实验结果整体趋势基本一致。本研究可为非均匀材料的波速和动态力学性能研究提供物理机制方面的解释。
- 非均匀性 /
- 压剪耦合效应 /
- 广义波动方程 /
- 本构关系 /
- 波速
Abstract: Heterogeneous media are very common in nature. Due to the complex internal structure, the heterogeneous compressive shear coupled stress field is inside heterogeneous media, which leads to a mutual influence of compression and shear waves. The study of wave mechanics behavior and description of heterogeneity in heterogeneous media is of great significance and full of challenges. This article establishes a general constitutive relationship that reflects the compression shear coupling characteristics of heterogeneous materials, proposes coupling coefficients to describe material heterogeneity, combines momentum conservation law to establish a generalized wave equation, and provides a general method for solving the generalized wave equation. As an example, expressions for the three characteristic wave velocities of compression shear coupling under the first-order compression shear coupling constitutive relationship are provided, and the finite difference method is employed to obtain the propagation process of coupled compression and shear waves. The effects of four heterogeneous coupling coefficients on stress state, coupled wave velocity, and wave propagation process are studied. The positive and negative values of coupling parameters and their combinations reflect the structural characteristics of heterogeneous media and also determine the properties of compression shear coupling waves. For heterogeneous media with high-pressure effects, shear dilation effects, and shear weakening effects, the coupled compression wave velocity is lower than the elastic compression wave velocity corresponding to uniform media, and the coupled shear wave velocity is higher than the elastic shear wave velocity. The effect of shear on compression delays the propagation of compressive stress, while compression promotes the propagation of shear. Coupled compression wave velocity is the result of the competition between the coupling effect of shear on compression and the volume compaction effect. Coupled shear wave velocity is the result of the competition between the coupling effect of compression on shear and the shear weakening effect caused by continuous distortion of the medium. These mechanisms could be achieved through different combinations of compression shear coupling parameters. A true triaxial experimental testing system was used to measure the longitudinal wave velocity of granite, model materials made of mortar, and materials made of cement mortar with coarse aggregates under different compressive and shear stresses. The results indicate that for heterogeneous media, the longitudinal wave velocity decreases with the increase of static water pressure and equivalent shear stress, and the shear expansion and shear weakening effects dominate. The experimental results and theoretical results have the same trend. The conclusion of this study is expected to provide a physical mechanism explanation for the phenomenon of the variation of wave velocity with stress state in heterogeneous materials.
- heterogeneity /
- compression-shear coupling effect /
- generalized wave equation /
- constitutive relationship /
- wave velocity

HTML全文

图 1 耦合参数对静水压力和等效剪应力的影响

Figure 1. Influence of coupling parameters on hydrostatic pressure and equivalent shear stress

下载: 全尺寸图片幻灯片

图 2 耦合参数对耦合压缩波速和耦合剪切波速的影响

Figure 2. Influence of coupling parameters on coupled compression wave velocity and coupled shear wave velocity

下载: 全尺寸图片幻灯片

图 3 耦合参数对压剪耦合波传播的影响

Figure 3. Influence of coupling parameters on the propagation of compressive-shear coupled waves

下载: 全尺寸图片幻灯片

图 4 真三轴实验设备的实物和示意图

Figure 4. Photo and schematic diagram of the true triaxial experimental equipment

下载: 全尺寸图片幻灯片

图 5 y+杆侧面和截面的示意图

Figure 5. Schematic diagrams of the side and section of the y+ bar

下载: 全尺寸图片幻灯片

图 6 真三轴测试系统中应变片的位置

Figure 6. Positions of strain gauges in the true triaxial testing system

下载: 全尺寸图片幻灯片

图 7 在x、y和z轴上的围压分别为20、12和8 MPa时，子弹撞击水泥砂浆材料记录的波形

Figure 7. Waveforms recorded by the projectile impacting the cement mortar material at confining pressures of 20, 12, and 8 MPa on the x-axis, y-axis, and z-axis, respectively

下载: 全尺寸图片幻灯片

图 8 花岗岩试样在不同静水压力和等效剪应力下的纵波波速

Figure 8. Longitudinal wave velocities in granite under different hydrostatic pressures and equivalent shear stresses

下载: 全尺寸图片幻灯片

图 9 MMM试样在不同静水压力和等效剪应力下的纵波波速

Figure 9. Longitudinal wave velocities in MMM under different hydrostatic pressures and equivalent shear stresses

下载: 全尺寸图片幻灯片

图 10 MMMA试样在不同静水压力和等效剪应力下的纵波波速

Figure 10. Longitudinal wave velocities in MMMA under different hydrostatic pressures and equivalent shear stresses

下载: 全尺寸图片幻灯片

表 1 3个试样的参数

Table 1. Parameters for three specimens

试样材料	x方向长度/mm	y方向长度/mm	z方向长度/mm	质量/g	密度/(g·cm⁻³)	杨氏模量/GPa	泊松比
花岗岩	50.10±0.34	50.14±0.34	50.18±0.34	321	2.64	70.0	0.20
MMM	49.70±0.48	50.02±0.34	49.64±0.37	263	2.12	16.5	0.15
MMMA	50.12±0.34	50.20±0.34	50.20±0.34	292	2.31	45.0	0.25

下载: 导出CSV

表 2 试样的围压条件

Table 2. Confining pressure conditions of the specimen

序号	试样材料	压应力/MPa			静水压力/MPa	等效剪应力/MPa
序号	试样材料	x方向	y方向	z方向	静水压力/MPa	等效剪应力/MPa
1	花岗岩	16	16	16	16.00	0
2		5	16	5	8.67	6.35
3		15	5	20	13.33	7.64
4		20	5	20	15.00	8.66
5		20	12	8	13.33	6.11
6	MMM	5	5	5	5.00	0
7		16	5	16	12.33	6.35
8		5	16	5	8.67	6.35
9		16	16	16	16.00	0
10	MMMA	5	5	5	5.00	0
11		16	16	16	16.00	0
12		16	5	16	12.33	6.35
13		5	16	5	8.67	6.35
14		20	12	8	13.33	6.11

下载: 导出CSV

参考文献(40)

[1]	LAN H X, MARTIN C D, HU B. Effect of heterogeneity of brittle rock on micromechanical extensile behavior during compression loading [J]. Journal of Geophysical Research: Solid Earth, 2010, 115(B1): B01202. DOI: 10.1029/2009JB006496.
[2]	袁良柱, 陆建华, 苗春贺, 等. 基于分数阶模型的牡蛎壳动力学特性研究 [J]. 爆炸与冲击, 2023, 43(1): 011101. DOI: 10.11883/bzycj-2022-0318. YUAN L Z, LU J H, MIAO C H, et al. Dynamic properties of oyster shells based on a fractional-order model [J]. Explosion and Shock Waves, 2023, 43(1): 011101. DOI: 10.11883/bzycj-2022-0318.
[3]	SHIROLE D, HEDAYAT A, WALTON G. Illumination of damage in intact rocks by ultrasonic transmission-reflection and digital image correlation [J]. Journal of Geophysical Research: Solid Earth, 2020, 125(7): e2020JB019526. DOI: 10.1029/2020JB019526.
[4]	SHIROLE D, WALTON G, HEDAYAT A. Experimental investigation of multi-scale strain-field heterogeneity in rocks [J]. International Journal of Rock Mechanics and Mining Sciences, 2020, 127: 104212. DOI: 10.1016/j.ijrmms.2020.104212.
[5]	徐松林, 周李姜, 黄俊宇, 等. 岩石类脆性材料动态压剪耦合特性研究 [J]. 振动与冲击, 2016, 35(10): 9–17, 23. DOI: 10.13465/j.cnki.jvs.2016.10.002. XU S L, ZHOU L J, HUANG J Y, et al. Investigation of dynamic coupled behavior of rock materials under combined compression and shear loading [J]. Journal of Vibration and Shock, 2016, 35(10): 9–17, 23. DOI: 10.13465/j.cnki.jvs.2016.10.002.
[6]	ZHOU L J, XU S L, SHAN J F, et al. Heterogeneity in deformation of granite under dynamic combined compression/shear loading [J]. Mechanics of Materials, 2018, 123: 1–18. DOI: 10.1016/j.mechmat.2018.04.013.
[7]	MIAO C H, XU S L, SONG Y P, et al. Influence of stress state on dynamic breakage of quartz glass spheres subjected to lower velocity impacting [J]. Powder Technology, 2022, 397: 11708. DOI: 10.1016/j.powtec.2021.117081.
[8]	CHEN M, XU S L, YUAN L Z, et al. Influence of stress state on dynamic behaviors of concrete under true triaxial confinements [J]. International Journal of Mechanical Sciences, 2023, 253: 108399. DOI: 10.1016/j.ijmecsci.2023.108399.
[9]	谢雨珊, 陆建华, 徐松林, 等. Mo-ZrC梯度金属陶瓷的冲击响应行为 [J]. 爆炸与冲击, 2023, 43(3): 033101. DOI: 10.11883/bzycj-2022-0374. XIE Y S, LU J H, XU S L, et al. On impact properties of Mo-ZrC gradient metal ceramics [J]. Explosion and Shock Waves, 2023, 43(3): 033101. DOI: 10.11883/bzycj-2022-0374.
[10]	HUANG J Y, LU L, FAN D, et al. Heterogeneity in deformation of granular ceramics under dynamic loading [J]. Scripta Materialia, 2016, 111: 114–118. DOI: 10.1016/j.scriptamat.2015.08.028.
[11]	HUANG J Y, XU S L, HU S S. Numerical investigations of the dynamic shear behavior of rough rock joints [J]. Rock Mechanics and Rock Engineering, 2014, 47(5): 1727–1743. DOI: 10.1007/s00603-013-0502-8.
[12]	MIAO C H, XU S L, YUAN L Z, et al. Experimental investigation of failure diffusion in brittle materials subjected to low-speed impact [J]. International Journal of Mechanical Sciences, 2023, 259: 108632. DOI: 10.1016/j.ijmecsci.2023.108632.
[13]	SHAN J F, XU S L, LIU Y G, et al. Dynamic breakage of glass sphere subjected to impact loading [J]. Powder Technology, 2018, 330: 317–329. DOI: 10.1016/j.powtec.2018.02.009.
[14]	TANG Z P, XU S L, DAI X Y, et al. S-wave tracing technique to investigate the damage and failure behavior of brittle materials subjected to shock loading [J]. International Journal of Impact Engineering, 2005, 31(9): 1172–1191. DOI: 10.1016/j.ijimpeng.2004.07.005.
[15]	TING T C T, NAN N. Plane waves due to combined compressive and shear stresses in a half space [J]. Journal of Applied Mechanics, 1969, 36(2): 189–197. DOI: 10.1115/1.3564606.
[16]	LI Y C, TING T C T. Plane waves in simple elastic solids and discontinuous dependence of solution on boundary conditions [J]. International Journal of Solids and Structures, 1983, 19(11): 989–1008. DOI: 10.1016/0020-7683(83)90024-0.
[17]	SONG Q Z, TANG Z P. Combined stress waves with phase transition in thin-walled tubes [J]. Applied Mathematics and Mechanics, 2014, 35(3): 285–296. DOI: 10.1007/s10483-014-1791-7.
[18]	WANG B, ZHANG K, CUI S T, et al. Mechanism of shear attenuation near the interface under combined compression and shear impact loading [J]. Wave Motion, 2017, 73: 96–103. DOI: 10.1016/j.wavemoti.2017.06.003.
[19]	RENAUD A, HEUZÉ T, STAINIER L. On loading paths followed inside plastic simple waves in two-dimensional elastic-plastic solids [J]. Journal of the Mechanics and Physics of Solids, 2020, 143: 104064. DOI: 10.1016/j.jmps.2020.104064.
[20]	PLONA T J. Observation of a second bulk compressional wave in a porous medium at ultrasonic frequencies [J]. Applied Physics Letters, 1980, 36(4): 259–261. DOI: 10.1063/1.91445.
[21]	LIU Q R, KATSUBE N. The discovery of a second kind of rotational wave in a fluid-filled porous material [J]. The Journal of the Acoustical Society of America, 1990, 88(2): 1045–1053. DOI: 10.1121/1.399853.
[22]	BEN-DAVID O, FINEBERG J. Static friction coefficient is not a material constant [J]. Physical Review Letters, 2011, 106(25): 254301. DOI: 10.1103/PhysRevLett.106.254301.
[23]	PASSELÈGUE F X, SCHUBNEL A, NIELSEN S, et al. From sub-Rayleigh to supershear ruptures during stick-slip experiments on crustal rocks [J]. Science, 2013, 340(6137): 1208–1211. DOI: 10.1126/science.1235637.
[24]	RUBINSTEIN S M, COHEN G, FINEBERG J. Detachment fronts and the onset of dynamic friction [J]. Nature, 2004, 430(7003): 1005–1009. DOI: 10.1038/nature02830.
[25]	RUBINSTEIN S M, COHEN G, FINEBERG J. Dynamics of precursors to frictional sliding [J]. Physical Review Letters, 2007, 98(22): 226103. DOI: 10.1103/PhysRevLett.98.226103.
[26]	XIA K W, ROSAKIS A J, KANAMORI H. Laboratory earthquakes: the sub-Rayleigh-to-supershear rupture transition [J]. Science, 2004, 303(5665): 1859–1861. DOI: 10.1126/science.1094022.
[27]	XIA K W, ROSAKIS A J, KANAMORI H, et al. Laboratory earthquakes along inhomogeneous faults: directionality and supershear [J]. Science, 2005, 308(5722): 681–684. DOI: 10.1126/science.110819.
[28]	ZOU Y T, ZHANG W, CHEN T, et al. Thermally induced anomaly in the shear behavior of magnetite at high pressure [J]. Physical Review Applied, 2018, 10(2): 024009. DOI: 10.1103/PhysRevApplied.10.024009.
[29]	WANG D J, LIU T, CHEN T, et al. Anomalous sound velocities of antigorite at high pressure and implications for detecting serpentinization at mantle wedges [J]. Geophysical Research Letters, 2019, 46(10): 5153–5160. DOI: 10.1029/2019GL082287.
[30]	LI B S, WOODY K, KUNG J. Elasticity of MgO to 11 GPa with an independent absolute pressure scale: implications for pressure calibration [J]. Journal of Geophysical Research: Solid Earth, 2006, 111(B11): B11206. DOI: 10.1029/2005jb004251.
[31]	ZOU Y T, LI M, DENG L W, et al. Acoustic velocities, elasticity, and pressure-induced elastic softening in compressed neodymium [J]. Mechanics of Materials, 2021, 155: 103776. DOI: 10.1016/j.mechmat.2021.103776.
[32]	CAI N, CHEN T, QI X T, et al. Sound velocities of the 23 Å phase at high pressure and implications for seismic velocities in subducted slabs [J]. Physics of the Earth and Planetary Interiors, 2019, 288: 1–8. DOI: 10.1016/j.pepi.2019.01.006.
[33]	CAI N, QI X T, CHEN T, et al. Enhanced visibility of subduction slabs by the formation of dense hydrous phase A [J]. Geophysical Research Letters, 2021, 48(19): e2021GL095487. DOI: 10.1029/2021GL095487.
[34]	LU J H, XU S L, MIAO C H, et al. The theory of compression-shear coupled composite wave propagation in rock [J]. Deep Underground Science and Engineering, 2022, 1(1): 77–86. DOI: 10.1002/dug2.12012.
[35]	LU J H, XU S L, LI Y, et al. Investigations on the compression-shear coupled stress waves propagating in heterogeneous rock [J]. Mechanics of Materials, 2023, 186: 104786. DOI: 10.1016/j.mechmat.2023.104786.
[36]	徐松林, 王鹏飞, 赵坚, 等. 基于三维Hopkinson杆的混凝土动态力学性能研究 [J]. 爆炸与冲击, 2017, 37(2): 180–185. DOI: 10.11883/1001-1455(2017)02-0180-06. XU S L, WANG P F, ZHAO J, et al. Dynamic behavior of concrete under static triaxial loading using 3D-Hopkinson bar [J]. Explosion and Shock Waves, 2017, 37(2): 180–185. DOI: 10.11883/1001-1455(2017)02-0180-06.
[37]	徐松林, 王鹏飞, 单俊芳, 等. 真三轴静载作用下混凝土的动态力学性能研究 [J]. 振动与冲击, 2018, 37(15): 59–67. DOI: 10.13465/j.cnki.jvs.2018.15.008. XU S L, WANG P F, SHAN J F, et al. Dynamic behavior of concrete under static tri-axial loadings [J]. Journal of Vibration and Shock, 2018, 37(15): 59–67. DOI: 10.13465/j.cnki.jvs.2018.15.008.
[38]	徐松林, 单俊芳, 王鹏飞, 等. 三轴应力状态下混凝土的侵彻性能研究 [J]. 爆炸与冲击, 2019, 39(7): 071101. DOI: 10.11883/bzycj-2019-0034. XU S L, SHAN J F, WANG P F, et al. Penetration performance of concrete under triaxial stress [J]. Explosion and Shock Waves, 2019, 39(7): 071101. DOI: 10.11883/bzycj-2019-0034.
[39]	XU S L, SHAN J F, ZHANG L, et al. Dynamic compression behaviors of concrete under true triaxial confinement: an experimental technique [J]. Mechanics of Materials, 2020, 140: 103220. DOI: 10.1016/j.mechmat.2019.103220.
[40]	ZHANG L, SHAN J F, MIAO C H, et al. The cratering performance of concrete target under true triaxial confinements [J]. International Journal of Mechanical Sciences, 2021, 210: 106714. DOI: 10.1016/j.ijmecsci.2021.106714.