Pressure-time formula for underwater explosion based on pressure-impulse curve
-
摘要: 根据材料受冲击载荷时的压力-冲量函数,推导得到了适用于水下爆炸冲击载荷的压力时程公式。通过水下爆炸实验方法测量不同药量、不同距离的压力时程曲线,使用MATLAB软件对实验数据进行拟合,由此计算冲击波冲量和能量参数,并与通用的Cole与Орленко理论计算结果进行对比,验证拟合曲线的准确性。相较于Cole和Орленко理论,新方法得到的压力衰减曲线更接近实验值。计算水下爆炸冲击波的比冲量和比冲击波能时,新模型具有较高的计算精度,其中:比冲量与实验值的误差不超过4%,与Орленко理论相比,精度提高了5%~10%;比冲击波能与实验值的误差不超过1%,计算精度与通用理论相当。Abstract: Theoretical study on the attenuation law of underwater explosion shock waves plays a crucial role in predicting the underwater explosion power. The attenuation law of underwater explosion shock waves could be analyzed from the shock waves pressure-time curve. The pressure-impulse (p-I) model has usually been used to assess damage on material and structure. In this paper, the pressure-time formula is analytically derived according to the p-I model. To verify the accuracy of the formula, the pressure-time relation curve was obtained by experiments with various explosive charges and distances. The experimental data was fitted in accordance with the theoretical equations of Cole and Орленко, and also with the developed pressure-time formula. The parameters of the formula are thus obtained. The accuracy could be described by the fitting coefficient R-squared (R2) value. The R-squared value of the developed pressure-time formula exceeds 0.988, which is greater than the R-squared values calculated by the equations of Cole and Орленко. In order to further validate the formula, the impulse and energy of underwater explosion shock waves are derived from the developed pressure-time formula, experimental data, and the equations of Cole and Орленко, respectively. Compared with the experimental results, the error of impulse derived from the developed pressure-time remains below 4%, which is 5%−10% lower than the errors of impulse between the experimental results and the equations of Cole and Орленко. The energy of the shock waves is similarly analyzed. Compared with the experimental results, the error of energy derived from the developed pressure-time remains below 1%, approximately the same as the errors of energy between the experimental results and the equations of Cole and Орленко. Compared with the calculation by equations of Cole and Орленко, it is seen that there is better correlation between the developed pressure-time formula and the experimental results. The developed pressure-time formula can be used to calculate the impulse and energy of underwater explosion shock waves.
-
材料和结构在经受爆炸等冲击载荷时,压力-冲量(p-I)曲线是评价其损伤程度的重要指标。起初,p-I曲线被用来评价建筑物受爆炸载荷时的破坏程度和人员的伤亡程度;近年来,它逐渐被用来评价结构和材料的损伤程度[1-2]。
美国对不同截面形状和材料的梁进行了大量的爆炸毁伤实验[3],得到了满足一定关系的p-I曲线。汪明[1]、Zhang等[4]分别对不同爆炸载荷下的钢柱和夹层玻璃窗进行了研究,得到了相应的p-I曲线,其爆炸损伤参数与梁结构的爆炸损伤参数不同。p-I曲线只有压力和时间2个量纲,在相同载荷、相同材料结构工况下,可认为爆炸损伤参数与时间无关,从而通过微积分理论将其转化成压力-时间公式。
自19世纪以来,尤其是二战时期,为了满足海洋军事的需要,科学家们对水下爆炸问题进行了大量的研究[5-6]。在军事上,水下爆炸研究大多是关于炸药爆炸对舰船、潜艇等结构的损伤效应[6]。水下爆炸的损伤效应主要体现在冲击波和气泡脉动两方面[7],而在冲击波的理论研究中,压力衰减规律一直是研究的重点内容。
关于炸药水下爆炸冲击波的压力分布及其时间变化规律,诸多学者基于冲击波传播理论和实验研究给出大量的模型。Cole[8]基于冲击波传播理论推导出了冲击波的压力衰减指数形式;Орленко[9]基于Cole的指数衰减公式,给出了冲击波压力在衰减后半段的反比形式。Cole和Орленко理论都是冲击波衰减规律的常用理论,前者适用于压力衰减前半段,后者适用于压力衰减后半段。Kiciński等[10]总结了诸多学者的冲击波压力衰减模型,提出当炸药的尺寸以及炸药所处深度不同时,经验公式的参数值会有所不同。Stiepanow对Cole公式做了进一步研究,得到了可模拟TNT炸药水下爆炸冲击波压力衰减全过程的经验公式,包括指数衰减段、倒数衰减段和倒数衰减后段3个部分[11]。Keil[12]提出了冲击波的压力衰减表达式,并结合实验研究给出了经验参数。Ming等[13]、Reid[14]、Rajendran等[15]在Keil[12]的研究基础上,对经验参数进行了修正。Geers等[16]为了提高精度、减小模型与实验之间的误差,提出了一种双指数衰减模型,但其形式复杂,参数较多。
前面诸多研究主要基于冲击波传播理论,结合实验研究得到经验公式,对水下爆炸压力的衰减特性进行研究,然而大多忽视了爆炸的损伤效应,较少从爆炸损伤的角度分析压力的衰减特性。本文中,基于材料的冲击波损伤机理,推导一种新的压力衰减规律的数学表达式,采用新表达式对实验数据进行拟合,比较拟合、Cole理论、Орленко理论和实验得到的比冲量以及比冲击波能,分析新公式的计算精度。
1. 理论推导
在材料的损伤效应研究中,采用p-I图研究材料爆炸损伤的重要参数,材料的p-I公式[2-4]可表达为:
(p−a)(I−b)=c (1) 式中:a为准静态载荷作用下材料达到某一损伤程度的临界压力,b为动态载荷作用下材料达到损伤破坏的临界冲量,c 为与损伤程度和材料相关的常数[17-19]。
将式(1)应用在水下爆炸研究中时,由于水的不可压缩性,可认为a、b、c均为与时间无关的常数。水承受负压时,其稳定性会发生变化,当负压达到某个阈值时,其内部会出现蒸汽泡,并发生空化[20-21]。水受到冲击载荷时,其状态会发生短暂改变,之后又很快恢复。因此,对于水来说,a为准静态载荷作用下水达到与冲击载荷作用后相同状态的临界压力,通常为负值,数值的大小与药量、距离和水变化后的状态相关;爆炸载荷为强冲击载荷,b近似等于冲击波冲量。
在水下爆炸的参数计算中,I可表示为:
I=∫pdt (2) 式中:t为时间。
将式(1)等号两边同时对时间t进行微分,结合式(2)可得:
p=−c(p−a)2d(p−a)dt (3) 将式(3)两边同时乘以dt/p,并对t积分可得:
t=ca2[ln(1−ap)+1p/a−1]+C (4) 式中:C为积分常数。
将式(4)转化成无量纲量的形式:
k=ta2c=f(p/a)=ln(1−ap)+1p/a−1 (5) 在Cole和Орленко的理论中,冲击波压力衰减表达式为:
p={pme−tθ0≤t<θpm0.368θtθ≤t≤(5∼10)θ (6) 式中:pm为冲击波峰值压力,
θ 为压力从pm下降到pm/e时所需要的时间。通常pm≫ a,当p=pm时,式(4)中ln(1−a/p)+1/(p/a−1)≈0 ,t ≈ 0,因此在后续拟合中,可认为C=0。比较式(5)和式(6)可以看出,式(5)的第1项为压力关于时间的指数表达式,第2项为反比表达式。 式(5)结合了Орленко和Cole理论的优点,在描述冲击波压力衰减规律时具有更高的精度。
2. 水下爆炸实验
2.1 实验材料
进行水下爆炸实验所需的材料和设备包括:黑索今炸药(RDX)、雷管、PCB压力传感器、恒流源、示波器、起爆器。
2.2 实验装置
基于水下爆炸罐开展水下爆炸实验,水下爆炸罐是一个上端开口的圆柱体,直径5.0 m,壁厚5 mm,高5.0 m。药包和PCB传感器置于水面以下3.0 m处,传感器与药包的距离r取1.0或1.5 m,实验装置见图1。
采用式(4)拟合实验结果时,p代表入射波的压力,不需要添加结构,测出冲击波在水中的压力时程曲线即可。水下爆炸实验采用的炸药分别为9 g RDX、12 g RDX和19 g RDX。
2.3 实验结果
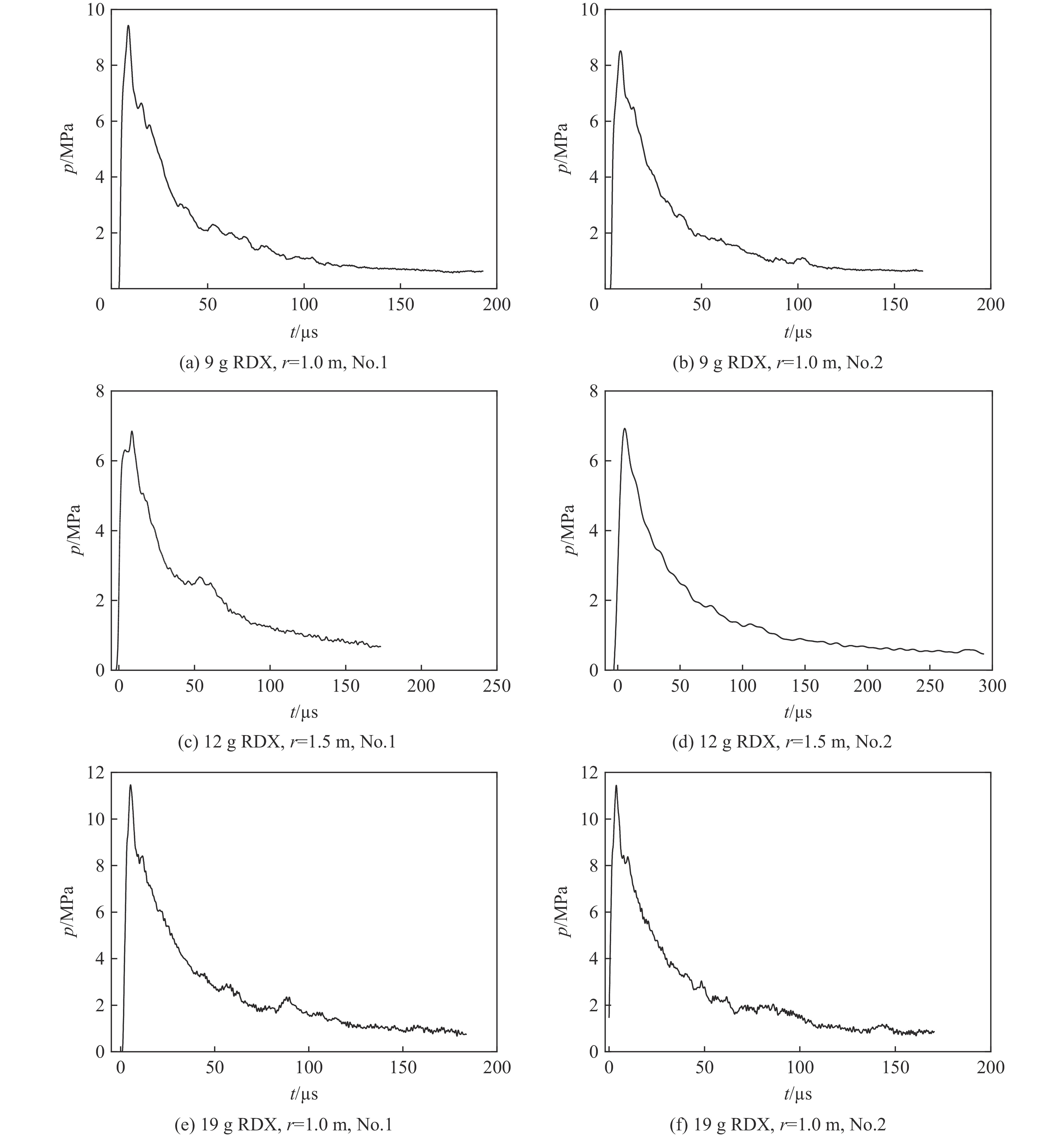
水下爆炸实验采集的数据受噪声、杂波、起爆信号等干扰,为此,先采用1 MHz的低通滤波获取有效信号;基于采样点的频率,使用低通FFT(fast Fourier transform)滤波器,截止频率设置为1 MHz[22]。图2显示了滤波后9 g RDX、12 g RDX和19 g RDX的水下爆炸压力时程曲线,同一质量炸药的实验重复2次。从图2可直接读取水下爆炸冲击波的峰值压力[23]。
冲击波的冲量I为压力对时间的积分[24],可表示为:
I=∫6.7θ0p(t)dt (7) 比冲击波能[18]可表示为:
Es=4πr2mρwCw∫6.7θ0p2(t)dt (8) 式中:
ρw 为水的密度,取1000 kg/m3;Cw 为水中的声速,取1450 m/s;m为RDX的质量;Es为比冲击波能。3. 水下爆炸时程曲线衰减段的MATLAB拟合
3.1 水下爆炸时程曲线衰减段的模拟结果
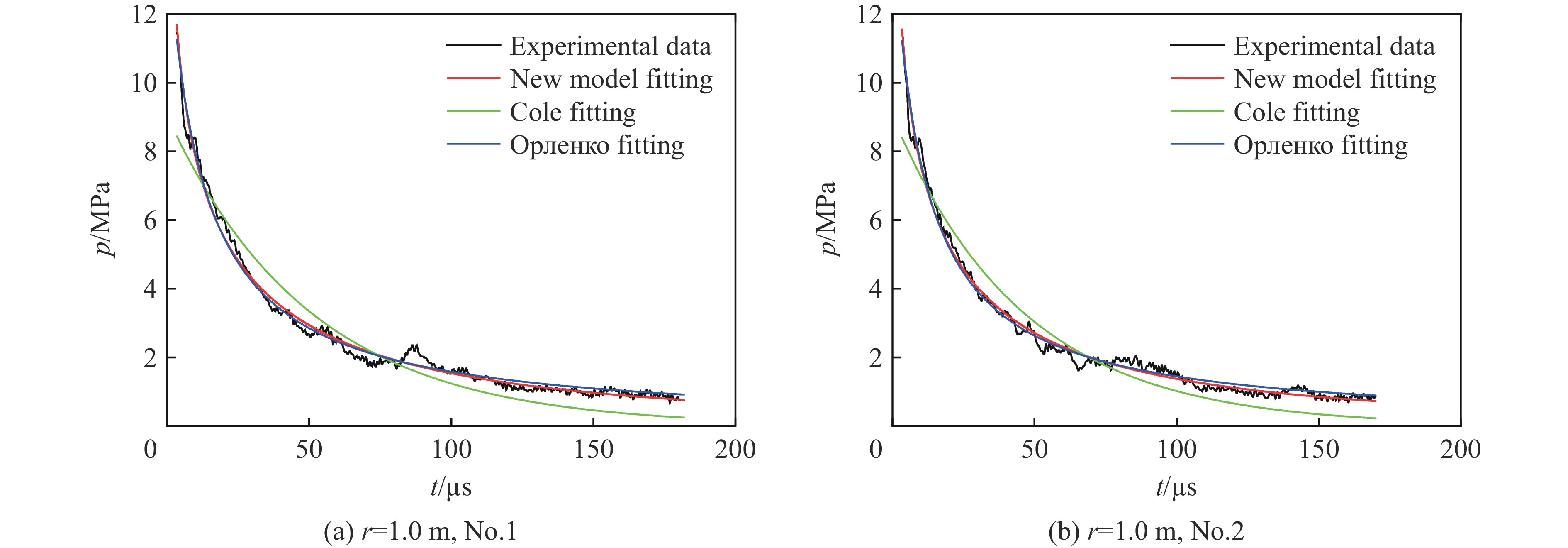
为了验证新模型的有效性,采用MATLAB软件基于式(5)对实验的压力衰减过程进行拟合,得到拟合曲线和a、c两个常数。采用新模型、Cole模型、Орленко模型对9 g RDX、12 g RDX和19 g RDX实验进行拟合,结果如图3~5所示。
拟合曲线的精度由可决系数R2决定:
R2=1−n∑i=1(yi−ˆyi)2n∑i=1(yi−ˉy)2 (9) 式中:
yi 为实验值,ˆyi 表示拟合结果,ˉy 为实验值的平均值,i为数据点的序号,n为数据点总数。R2的取值范围为0~1,数值越大,拟合精度越高。3种拟合方法的R2列于表1。从表1可以看出,新模型的R2均大于0.988,大多数结果大于0.99,说明新模型的模拟值与实验值接近,计算方法有效。相较于Cole和Орленко理论,新模型的R2更接近1,拟合精度更高,说明新模型能更准确地描述水下爆炸冲击波的衰减规律。
表 1 3种拟合方法的精度Table 1. Accuracy of three fitting methods炸药 组号 R2 a/kPa c/(kPa2·s) 新模型 Cole理论 Орленко理论 9 g RDX 1 0.9882 0.9282 0.9854 −0.097 0.053 2 0.9923 0.9363 0.9881 −0.089 0.043 12 g RDX 1 0.9936 0.9327 0.9861 −0.210 0.135 2 0.9963 0.9306 0.9861 −0.200 0.133 19 g RDX 1 0.9897 0.9274 0.9895 −0.180 0.131 2 0.9908 0.9286 0.9900 −0.160 0.094 3.2 冲击波参数评价
水下爆炸损伤效应的主要参数是I和Es。分别计算新模型、Cole-Орленко理论和实验的I与Es,验证新模型对冲击波参数模拟的准确性。由于Cole和Орленко理论分别适用于冲击波衰减的前半段和后半段,因此为计算完整的冲击波冲量与比冲击波能变化过程,将两种理论结合在一起计算。Cole-Орленко理论在计算冲量和比冲击波能时,将常用的Cole和Орленко理论代入式(7)~(8),直接积分可得:
I=pmθ(1−1e+ln6.7e) (10) Es=4πθr2p2mmρwCw(12−12e2−16.7e2) (11) 新模型中,基于实验数据,通过式(7)~(8)积分得到冲击波参数。表2和表3分别列出了冲量和比冲击波能的实验与理论结果,其中δ1为新模型与实验结果的误差,δ2为Cole-Орленко模型与实验结果的误差,负数表示理论值小于实验值。
表 2 冲量的理论值与实验值Table 2. Theoretical and experimental values of impulse炸药 组号 I/(Pa·s) δ1(I)/% δ2(I)/% 实验 新模型 Cole-Орленко模型 9 g RDX 1 325.862 320.943 325.575 −1.510 −0.088 2 282.572 279.108 325.575 −1.226 15.280 12 g RDX 1 376.875 375.155 326.037 −0.456 −13.490 2 349.426 348.407 326.037 −0.292 −6.694 19 g RDX 1 439.536 439.484 431.403 −0.012 −1.850 2 400.488 400.228 431.403 −0.065 7.719 表 3 比冲击波能的理论值与实验值Table 3. Theoretical and experimental values of specific shock wave energy炸药 组号 Es/(MJ·kg−1) δ1(Es)/% δ2(Es)/% 实验 新模型 Cole-Орленко模型 9 g RDX 1 1.057 1.051 0.929 −0.578 −12.110 2 0.902 0.899 0.929 −0.370 2.993 12 g RDX 1 1.171 1.168 1.106 −0.256 −5.551 2 1.087 1.082 1.106 −0.460 1.748 19 g RDX 1 0.840 0.836 0.772 −0.429 −8.095 2 0.757 0.754 0.772 −0.396 1.982 由表2~3可以看出,新模型计算的冲量和比冲击波能具有更高的精度。从冲量来看,新模型结果与实验结果更接近,误差不超过5%,远低于Cole-Орленко模型结果;从比冲击波能来看,新模型结果与Cole-Орленко模型结果相差不大,新模型与实验结果之间的误差略小,并且误差不超过1%,而Cole-Орленко模型结果与实验结果的误差波动较大。
4. 结 论
通过对损伤效应的p-I公式进行推导,得到水下爆炸的压力时程公式。结合Cole和Орленко模型的优势,提出了一个新模型来描述水下爆炸的冲击波衰减过程,并通过水下爆炸实验得到新模型的经验参数。与Cole和Орленко模型相比,新模型与实验数据的近似程度更高,可决系数超过0.988。
-
表 1 3种拟合方法的精度
Table 1. Accuracy of three fitting methods
炸药 组号 R2 a/kPa c/(kPa2·s) 新模型 Cole理论 Орленко理论 9 g RDX 1 0.9882 0.9282 0.9854 −0.097 0.053 2 0.9923 0.9363 0.9881 −0.089 0.043 12 g RDX 1 0.9936 0.9327 0.9861 −0.210 0.135 2 0.9963 0.9306 0.9861 −0.200 0.133 19 g RDX 1 0.9897 0.9274 0.9895 −0.180 0.131 2 0.9908 0.9286 0.9900 −0.160 0.094 表 2 冲量的理论值与实验值
Table 2. Theoretical and experimental values of impulse
炸药 组号 I/(Pa·s) δ1(I)/% δ2(I)/% 实验 新模型 Cole-Орленко模型 9 g RDX 1 325.862 320.943 325.575 −1.510 −0.088 2 282.572 279.108 325.575 −1.226 15.280 12 g RDX 1 376.875 375.155 326.037 −0.456 −13.490 2 349.426 348.407 326.037 −0.292 −6.694 19 g RDX 1 439.536 439.484 431.403 −0.012 −1.850 2 400.488 400.228 431.403 −0.065 7.719 表 3 比冲击波能的理论值与实验值
Table 3. Theoretical and experimental values of specific shock wave energy
炸药 组号 Es/(MJ·kg−1) δ1(Es)/% δ2(Es)/% 实验 新模型 Cole-Орленко模型 9 g RDX 1 1.057 1.051 0.929 −0.578 −12.110 2 0.902 0.899 0.929 −0.370 2.993 12 g RDX 1 1.171 1.168 1.106 −0.256 −5.551 2 1.087 1.082 1.106 −0.460 1.748 19 g RDX 1 0.840 0.836 0.772 −0.429 −8.095 2 0.757 0.754 0.772 −0.396 1.982 -
[1] 汪明. 爆炸荷载作用下钢结构损伤机理及砌体墙破碎过程研究 [D]. 天津: 天津大学, 2010: 42–58.WANG M. Damage mechanism of steel structures and fragmentation process of masonry walls under blast loading [D]. Tianjin: Tianjin University, 2010: 42–58. [2] 汪维. 钢筋混凝土构件在爆炸载荷作用下的毁伤效应及评估方法研究 [D]. 长沙: 国防科学技术大学, 2012: 121–136.WANG W. Study on damage effects and assessments method of reinforced concrete structural members under blast loading [D]. Changsha: National University of Defense Technology, 2012: 121–136. [3] Ma G W, SHI H J, SHU D W. P–I diagram method for combined failure modes of rigid-plastic beams [J]. International Journal of Impact Engineering, 2007, 34(6): 1081–1094. [4] ZHANG X H, HAO H, MA G W. Parametric study of laminated glass window response to blast loads [J]. Engineering Structures, 2013, 56: 1707–1717. DOI: 10.1016/j.engstruct.2013.08.007. [5] 王奕鑫, 马宏昊, 沈兆武, 等. 水下爆炸中水面效应以及药包形状对冲击波的影响 [J]. 中国测试, 2018, 44(10): 7–13, 30. DOI: 10.11857/j.issn.1674-5124.2018.10.002.WANG Y X, MA H H, SHEN Z W, et al. Effect of water surface and shape of charge on shock wave in underwater explosion [J]. China Measurement & Test, 2018, 44(10): 7–13, 30. DOI: 10.11857/j.issn.1674-5124.2018.10.002. [6] 金键, 朱锡, 侯海量, 等. 水下爆炸载荷下舰船响应与毁伤研究综述 [J]. 水下无人系统学报, 2017, 25(6): 396–409. DOI: 10.11993/j.issn.2096-3920.2017.05.002.JIN J, ZHU X, HOU H L, et al. Review of dynamic response and damage mechanism of ship structure subjected to underwater explosion load [J]. Journal of Unmanned Undersea Systems, 2017, 25(6): 396–409. DOI: 10.11993/j.issn.2096-3920.2017.05.002. [7] 段超伟, 宋浦, 胡宏伟, 等. 水下爆炸气泡动态特性的研究进展 [J]. 爆破, 2022, 39(1): 140–151. DOI: 10.3963/j.issn.1001-487X.2022.01.021.DUAN C W, SONG P, HU H W, et al. Research progress on dynamic characteristics of underwater explosion bubbles [J]. Blasting, 2022, 39(1): 140–151. DOI: 10.3963/j.issn.1001-487X.2022.01.021. [8] COLE R H. Underwater explosions [M]. Princeton: Princeton University Press, 1948. [9] Л П 奥尔连科. 爆炸物理学 [M]. 孙承纬, 译. 北京: 科学出版社, 2011: 596–625.ОРЛЕНКО Л П. Explosion physics [M]. Translated by SUN C W. Beijing: Science Press, 2011: 596–625. [10] KICIŃSKI R, SZTUROMSKI B. Pressure wave caused by trinitrotoluene (TNT) underwater explosion: short review [J]. Applied Sciences, 2020, 10(10): 3433. DOI: 10.3390/app10103433. [11] 辛春亮, 薛再清, 涂建, 等. TrueGrid和LS-DYNA动力学数值计算详解 [M]. 北京: 机械工业出版社, 2019: 251–254. [12] KEIL A H. Introduction to underwater explosion research [R]. Portsmouth: Norfolk Naval Ship Yard, 1956. [13] MING F R, ZHANG A M, XUE Y Z, et al. Damage characteristics of ship structures subjected to shockwaves of underwater contact explosions [J]. Ocean Engineering, 2016, 117: 359–382. DOI: 10.1016/j.oceaneng.2016.03.040. [14] REID W D. Response of surface ships to underwater explosions [R]. Canberra: Defence Science and Technology Organization, 1996. [15] RAJENDRAN R, NARASIMHAN K. Deformation and fracture behaviour of plate specimens subjected to underwater explosion: a review [J]. International Journal of Impact Engineering, 2006, 32(12): 1945–1963. DOI: 10.1016/j.ijimpeng.2005.05.013. [16] GEERS T L, HUNTER K S. An integrated wave-effects model for an underwater explosion bubble [J]. The Journal of the Acoustical Society of America, 2002, 111(4): 1584–1601. DOI: 10.1121/1.1458590. [17] SHI Y C, HAO H, LI Z X. Numerical derivation of pressure-impulse diagrams for prediction of RC column damage to blast loads [J]. International Journal of Impact Engineering, 2008, 35(11): 1213–1227. DOI: 10.1016/j.ijimpeng.2007.09.001. [18] HUANG X, BAO H R, HAO Y F, et al. Damage assessment of two-way RC slab subjected to blast load using mode approximation approach [J]. International Journal of Structural Stability and Dynamics, 2017, 17(1): 1750013. DOI: 10.1142/S0219455417500134. [19] FALLAH A S, LOUCA L A. Pressure-impulse diagrams for elastic-plastic-hardening and softening single-degree-of-freedom models subjected to blast loading [J]. International Journal of Impact Engineering, 2007, 34(4): 823–842. DOI: 10.1016/j.ijimpeng.2006.01.007. [20] 郑庆. 水的拉伸强度 [J]. 科学, 1992, 44(5): 35–38.ZHENG Q. Tensile strength of water [J]. Science, 1992, 44(5): 35–38. [21] 黄西成. 内爆与外爆加载下壳体的力学状态及破坏模式分析 [D]. 绵阳: 中国工程物理研究院, 2010: 16–26.HUANG X C. Analysis of mechanical states and failure modes of shells subjected to implosive and explosive loadings [D]. Mianyang: China Academy of Engineering Physics, 2010: 16–26. [22] 陈继平. 含有活性气体的高能炸药爆炸性能和起爆机理研究 [D]. 合肥: 中国科学技术大学, 2023: 39–50.CHEN J P. Study on explosion property and initiation mechanism of high explosive containing active gas [D]. Hefei: University of Science and Technology of China, 2023: 39–50. [23] 黄亮亮, 王林桂, 张西良, 等. 空气层对RDX水下爆炸性能影响的实验研究 [J]. 工程爆破, 2021, 27(5): 32–38. DOI: 10.19931/j.EB.20200120.HUANG L L, WANG L G, ZHANG X L, et al. Experimental study on the influence of air layer on the underwater explosion properties of RDX [J]. Engineering Blasting, 2021, 27(5): 32–38. DOI: 10.19931/j.EB.20200120. [24] BJARNHOLT G. Suggestions on standards for measurement and data evaluation in the underwater explosion test [J]. Propellants, Explosives, Pyrotechnics, 1980, 5(2/3): 67–74. DOI: 10.1002/prep.19800050213. -








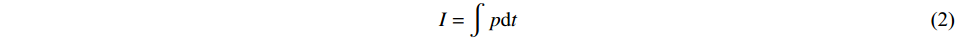







 下载:
下载:

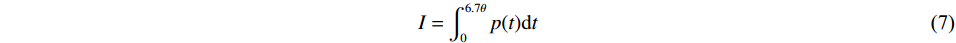













 下载:
下载: