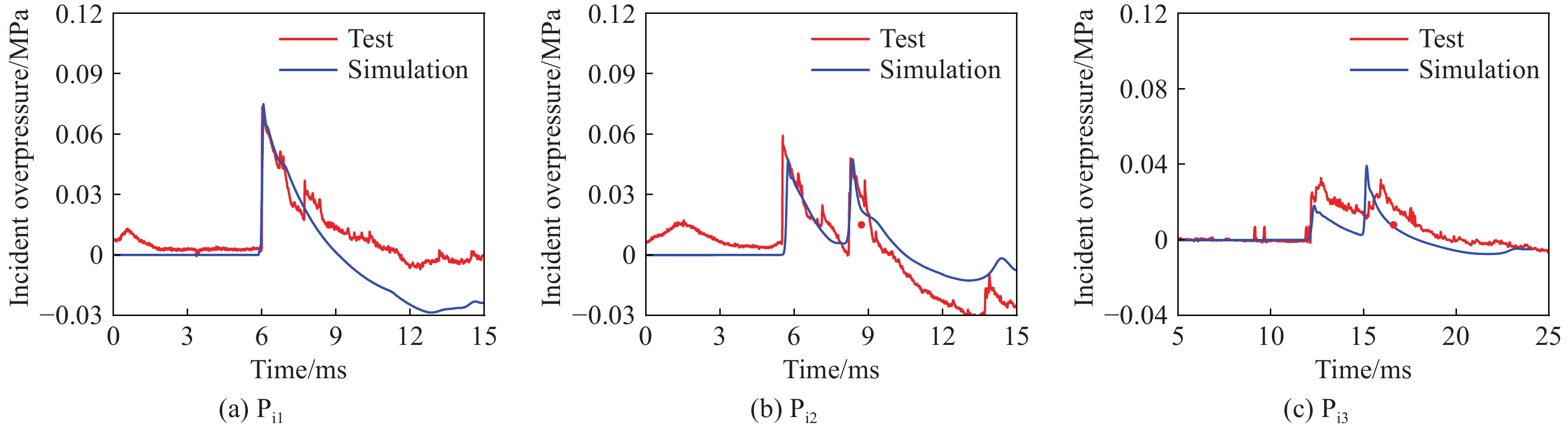
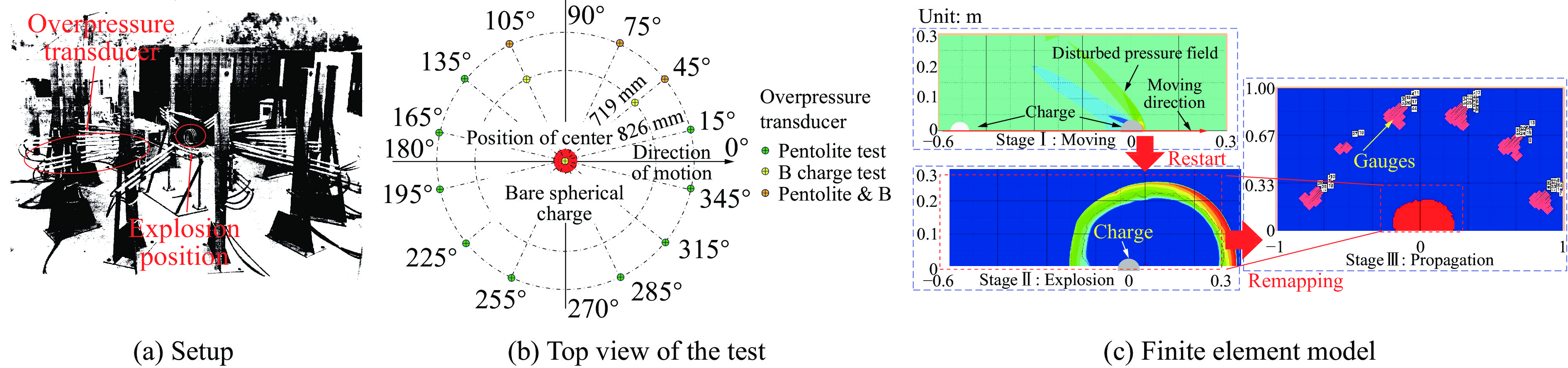
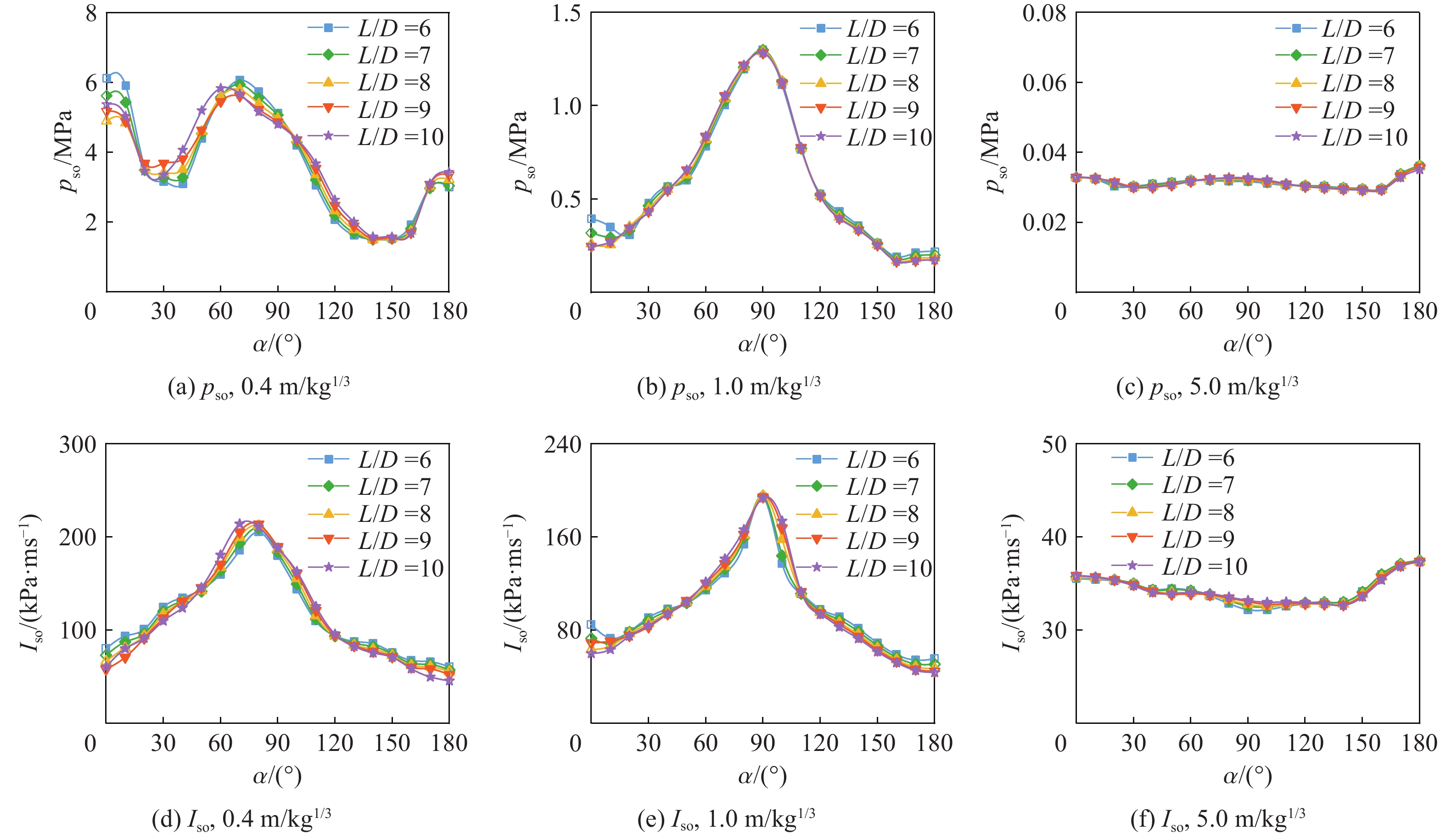
Calculation model for the blast wave load by explosion of air-moving cylindrical charges
-
摘要: 为预测战斗部的爆炸威力，对柱形装药运动爆炸的入射和反射冲击波峰值超压和最大冲量开展数值仿真研究。首先，基于AUTODYN有限元分析程序提出了“三阶段”装药运动爆炸有限元分析方法，通过与已有静止和运动爆炸试验结果对比，验证了方法的可靠性。然后，考虑装药运动速度、长径比、比例距离、方位角和刚性反射等影响因素，开展了运动爆炸工况下200组柱形装药的数值模拟。结果表明：相较于静爆，动爆冲击波场整体前移，波阵面强度在装药运动方向增强而在反方向减弱，该影响与装药运动速度正相关。最后，针对柱形装药空中自由场运动爆炸和垂直于目标迎爆面运动爆炸的典型工况，分别提出了装药运动爆炸入射和反射冲击波峰值超压以及最大冲量的计算模型。该模型与2种战斗部柱形TNT装药运动爆炸工况的数值模拟结果符合良好，能较好地计算柱形装药空中运动爆炸冲击波荷载。Abstract: Ammunition warheads are typically cylindrical charges that detonate at the moving stage. To accurately calculate the blast wave power field and the blast loadings acting on the structure of an air-moving cylindrical charge explosion, the peak overpressure and maximal impulse of the incident and reflected blast waves in the air-moving cylindrical charge explosion were numerically simulated. Firstly, a three-stage finite element analysis method for the explosion of air-moving cylindrical charges was proposed based on the AUTODYN finite element analysis program, and the reliability of the method was verified by comparing the simulated and test data of existing charges air static and moving explosion tests. Then, numerical simulations were conducted for 200 sets of scenarios of air-moving cylindrical charge explosions, considering factors such as charge-moving velocity, length-to-diameter ratio, scaled distance, azimuth angle, and rigid reflection. The distribution characteristics of the moving explosion blast wave field, and incident and reflected blast wave loadings were quantitatively analyzed. The results indicate that the blast wave field of a moving explosion is moved forward compared to the static explosion, and the wavefront strength is enhanced in the direction of charge movement and weakened in the opposite direction. This effect is positively correlated with the charge moving velocity, while the influence of changing the length-to-diameter ratio is small on the blast wave field. Furthermore, for the typical scenarios of air-moving cylindrical charge explosions in a free field and in a reflected field where the cylindrical charge was perpendicular to the target surface, calculation models for the peak overpressure and maximal impulse of the incident and reflected blast waves of the explosion of air-moving cylindrical charges were proposed. Finally, through carrying out numerical simulations of 40 sets of scenarios for the explosion of two simplified moving cylindrical TNT charges of prototype warheads, and comparing data of calculation models and simulations, the applicability of the proposed calculation model was validated. The results indicate that the calculation model is good at evaluating the blast wave loading of air-moving cylindrical charge explosion, which can also provide a certain reference for predicting the moving explosive power of warheads.
-
Key words:
- cylindrical charge /
- air moving explosion /
- peak overpressure /
- maximal impulse /
- calculation model
-
图 1 柱形装药空中动爆有限元模型
Figure 1. Finite element model of cylindrical charges air moving explosion
图 2 局部和全域模型中不同网格尺寸下的空气压力时程曲线
Figure 2. Air pressure-time histories corresponding to different mesh sizes in local and global models
图 10 装药运动扰动压力场和有限元模型
Figure 10. The disturbed pressure field of moving charge and finite element model
图 11 不同速度下扰动压力场对入射冲击波超压时程的影响
Figure 11. Influence of the disturbed pressure field on incident overpressure-time histories with different velocities
图 12 不同时刻柱形装药动爆冲击波压力云图（L/D=6, Ma=4）
Figure 12. Instantaneous blast wave pressure contours of cylindrical charges air moving explosion at different times (L/D=6, Ma=4)
图 13 不同装药长径比的冲击波压力云图（Ma=0）
Figure 13. Instantaneous blast wave pressure contours of cylindrical charges air moving explosion with different length-to-diameter ratios (Ma=0)
图 14 典型比例距离处不同长径比装药的冲击波峰值超压及最大冲量与方位角的关系（Ma=0）
Figure 14. Blast wave peak overpressure and maximal impulse vs. azimuth angle of charges with different length-to-diameter ratios at typical scaled distances (Ma=0)
图 15 不同运动速度工况下柱形装药的动爆冲击波压力云图（0.1 ms）
Figure 15. Instantaneous blast wave pressure contours of cylindrical charges air moving explosion with different velocities (0.1 ms)
图 17 典型比例距离处的冲击波峰值超压和最大冲量与方位角的关系（L/D=6）
Figure 17. Blast wave peak overpressure and maximal impulse vs. azimuth angle of charges with different velocities at typical scaled distances (L/D=6)
图 18 不同运动速度工况下入射冲击波峰值超压（L/D=6）
Figure 18. Peak overpressure of blast wave with different moving velocities (L/D=6)
图 19 典型方位角入射冲击波峰值超压（L/D=6）
Figure 19. Peak overpressure of blast wave under typical azimuth angles (L/D=6)
图 20 不同运动速度工况下入射冲击波的最大冲量（L/D=6）
Figure 20. Maximal impulse of blast wave under typical azimuth angles with different moving velocities (L/D=6)
图 21 典型方位角下入射冲击波的最大冲量（L/D=6）
Figure 21. Maximal impulse of blast wave under typical azimuth angles (L/D=6)
图 22 反射场和自由场有限元模型（单位：m）
Figure 22. Finite element models of reflection and free fields (unit: m)
图 23 不同垂直比例距离处的冲击波超压反射系数（L/D=6）
Figure 23. Reflection coefficients of blast overpressure at different vertical scaled distances (L/D=6)
图 24 23 kg装药的动爆冲击波荷载
Figure 24. Blast wave loadings of 23 kg charges air moving explosion
图 25 280 kg装药的动爆冲击波荷载
Figure 25. Blast wave loadings of 280 kg charges air moving explosion
表 1 扰动压力场影响下入射冲击波峰值超压的相对误差
Table 1. Relative deviations of peak incident overpressure influenced by the disturbance pressure field
Ma Z/(m·kg−1/3) δd/% α=0° α=45° α=90° α=135° α=180° 1 0.3 2.88 0.76 0.97 0.18 9.08 0.4 0.40 0.19 0.03 0.41 6.46 0.5 2.63 0.71 0.42 0.35 2.94 4 0.3 16.41 0.93 7.67 1.40 29.46 0.4 7.75 1.10 4.57 5.15 14.64 0.5 3.66 2.63 2.71 8.14 5.78 
下载: 导出CSV
表 2 峰值超压的拟合系数
Table 2. Coefficients of the fitted formula for peak overpressure
k A1,k,1 A1,k,2 A1,k,3 A2,k,1 A2,k,2 A2,k,3 A3,k,1 A3,k,2 A3,k,3 1 −2287 −60 1782 2769 250 −1686 −291 −40 182 2 −262 −74 484 −893 212 −205 491 −50 −51 3 −873 39 829 732 −160 −685 −152 50 102 4 −1781 89 1304 1854 −233 −932 −242 47 85 j Aj,1 Aj,2 Aj,3 Aj,4 Aj,5 1 −926 −308 721 −4 236 2 1497 858 −558 26 −559 3 −224 −133 111 −7 70
下载: 导出CSV
表 3 比冲量的拟合系数
Table 3. Coefficients of the fitted formula for the scaled impulse
k B1,k,1 B1,k2 B1,k,3 B2,k,1 B2,k,2 B2,k,3 B3,k,1 B3,k,2 B3,k,3 1 −74 31 47 28 −26 12 68 6 −40 2 928 7 −740 −1249 −2 743 521 1 −244 3 525 −1 −414 −676 −5 413 267 2 −129 4 74 −2 −49 −270 −8 167 179 3 −87 j Bj,1 Bj,2 Bj,3 Bj,4 Bj,5 1 372 214 −148 3 −181 2 −277 −72 108 −2 46 3 40 36 −10 0 −17
下载: 导出CSV
-
[1] US Department of Defense. UFC 3-340-02 Structures to resist the effects of accidental explosions, with change 2 [S]. Washington: US Department of Defense, 2014. [2] US Department of the Army. Fundamentals of protective design for conventional weapons: TM 5-855-1 [S]. Washington: US Department of the Army, 1986. [3] 中华人民共和国国家质量监督检验检疫总局, 中国国家标准化管理委员会. 爆破安全规程: GB 6722—2014 [S]. 北京: 中国标准出版社, 2015.General Administration of Quality Supervision, Inspection and Quarantine of the People’s Republic of China, Standardization Administration of the People’s Republic of China. Safety regulations for blasting: GB 6722—2014 [S]. Beijing: Standards Press of China, 2015. [4] STONER R G, BLEAKNEY W. The attenuation of spherical shock waves in air [J]. Journal of Applied Physics, 1948, 19(7): 670–678. DOI: 10.1063/1.1698189. [5] BRODE H L. Numerical solutions of spherical blast waves [J]. Journal of Applied Physics, 1955, 26(6): 766–775. DOI: 10.1063/1.1722085. [6] BAKER W E. Explosions in air [M]. Austin: University of Texas Press, 1974: 6–10. [7] HENRYCH J. The dynamics of explosion and its use [M]. Amsterdam: Elsevier Science Publishing Company, 1979: 218. [8] MILLS C A. The design of concrete structures to resist explosions and weapon effects [C]//1st International Conference on Concrete for Hazard Protections. Edinburgh: European Cement Association, 1987: 11–15. [9] ANASTACIO A C, KNOCK C. Radial blast prediction for high explosive cylinders initiated at both ends [J]. Propellants, Explosives, Pyrotechnics, 2016, 41(4): 682–687. DOI: 10.1002/prep.201500302. [10] KNOCK C, DAVIES N, REEVES T. Predicting blast waves from the axial direction of a cylindrical charge [J]. Propellants, Explosives, Pyrotechnics, 2015, 40(2): 169–179. DOI: 10.1002/prep.201300188. [11] KNOCK C, DAVIES N. Predicting the peak pressure from the curved surface of detonating cylindrical charges [J]. Propellants, Explosives, Pyrotechnics, 2011, 36(3): 203–209. DOI: 10.1002/prep.201000001. [12] KNOCK C, DAVIES N. Predicting the impulse from the curved surface of detonating cylindrical charges [J]. Propellants, Explosives, Pyrotechnics, 2011, 36(2): 105–109. DOI: 10.1002/prep.201000002. [13] XIAO W F, ANDRAE M, GEBBEKEN N. Effect of charge shape and initiation configuration of explosive cylinders detonating in free air on blast-resistant design [J]. Journal of Structural Engineering, 2020, 146(8): 04020146. DOI: 10.1061/(asce)st.1943-541x.0002694. [14] GAO C, KONG X Z, FANG Q, et al. Numerical investigation on free air blast loads generated from center-initiated cylindrical charges with varied aspect ratio in arbitrary orientation [J]. Defence Technology, 2022, 18(9): 1662–1678. DOI: 10.1016/j.dt.2021.07.013. [15] 王明涛, 程月华, 吴昊. 柱形装药空中爆炸冲击波荷载研究 [J]. 爆炸与冲击, 2024, 44(4): 043201. DOI: 10.11883/bzycj-2023-0197.WANG M T, CHENG Y H, WU H. Study on blast loadings of cylindrical charges air explosion [J]. Explosion and Shock Waves, 2024, 44(4): 043201. DOI: 10.11883/bzycj-2023-0197. [16] ARMENDT B F, SPERRAZZA J. Air blast measurements around moving explosive charges, Part Ⅲ: AD0114950 [R]. Aberdeen: Army Ballistics Research Laboratory, 1956. [17] PATTERSON J D, WENIG J. Air blast measurements around moving explosive charges: AD0033173 [R]. Aberdeen: Army Ballistics Research Laboratory, 1954. [18] ARMENDT B F, SPERRAZZA J. Air blast measurements around moving explosive charges, Part Ⅱ: AD0114950 [R]. Aberdeen: Army Ballistics Research Laboratory, 1955. [19] 蒋海燕, 李芝绒, 张玉磊, 等. 运动装药空中爆炸冲击波特性研究 [J]. 高压物理学报, 2017, 31(3): 286–294. DOI: 10.11858/gywlxb.2017.03.010.JIANG H Y, LI Z R, ZHANG Y L, et al. Characteristics of air blast wave field for explosive charge moving at different velocities [J]. Chinese Journal of High Pressure Physics, 2017, 31(3): 286–294. DOI: 10.11858/gywlxb.2017.03.010. [20] 陈龙明, 李志斌, 陈荣. 装药动爆冲击波特性研究 [J]. 爆炸与冲击, 2020, 40(1): 013201. DOI: 10.11883/bzycj-2019-0029.CHEN L M, LI Z B, CHEN R. Characteristics of dynamic explosive shock wave of moving charge [J]. Explosion and Shock Waves, 2020, 40(1): 013201. DOI: 10.11883/bzycj-2019-0029. [21] 聂源, 蒋建伟, 李梅. 球形装药动态爆炸冲击波超压场计算模型 [J]. 爆炸与冲击, 2017, 37(5): 951–956. DOI: 10.11883/1001-1455(2017)05-0951-06.NIE Y, JIANG J W, LI M. Overpressure calculation model of sphere charge blasting with moving velocity [J]. Explosion and Shock Waves, 2017, 37(5): 951–956. DOI: 10.11883/1001-1455(2017)05-0951-06. [22] XU Q P, SU J J, LI Z R, et al. Air blast pressure characteristics of moving charge [J]. Journal of Physics: Conference Series, 2020, 1507: 032052. DOI: 10.1088/1742-6596/1507/3/032052. [23] 周至柔, 蒋海燕, 苏健军. 动爆冲击波场空间位置分布规律研究 [J]. 兵器装备工程学报, 2023, 44(2): 15–21. DOI: 10.11809/bqzbgcxb2023.02.003.ZHOU Z R, JIANG H Y, SU J J. Study on spatial position distribution of a dynamic detonation shock wave field [J]. Journal of Ordnance Equipment Engineering, 2023, 44(2): 15–21. DOI: 10.11809/bqzbgcxb2023.02.003. [24] MA X J, KONG D R, SHI Y C. Experimental and numerical investigation of blast loads induced by moving charge explosion [J]. Structures, 2023, 47: 2037–2049. DOI: 10.1016/j.istruc.2022.12.042. [25] CHEN H J, YIN J P, LI X D, et al. A numerical study on the blast wave distribution and propagation characteristics of cylindrical explosive in motion [J]. Mathematical Problems in Engineering, 2022, 2022: 6446164. DOI: 10.1155/2022/6446164. [26] 王振宁, 尹建平, 伊建亚, 等. 柱形装药近地动爆冲击波周向传播规律研究 [J]. 爆炸与冲击, 2023, 43(6): 063201. DOI: 10.11883/bzycj-2022-0313.WANG Z N, YIN J P, YI J Y, et al. A study on the circumferential propagation law of the shock waves produced by the near ground dynamic explosion of cylindrical charge [J]. Explosion and Shock Waves, 2023, 43(6): 063201. DOI: 10.11883/bzycj-2022-0313. [27] SIMOENS B, LEFEBVRE M H, MINAMI F. Influence of different parameters on the TNT-equivalent of an explosion [J]. Central European Journal of Energetic Materials, 2011, 8(1): 53–67. [28] SIMOENS B, LEFEBVRE M. Influence of the shape of an explosive charge: quantification of the modification of the pressure field [J]. Central European Journal of Energetic Materials, 2015, 12(2): 195–213. [29] 罗兴柏, 张玉令, 丁玉奎. 爆炸力学理论教程 [M]. 北京: 国防工业出版社, 2016: 132–139. -
计量
- 文章访问数: 215
- HTML全文浏览量: 52
- PDF下载量: 110
- 被引次数: 0