军民用防护结构会面临新型钻地弹高精度、深侵彻、大当量打击的严峻威胁。钻地弹通过精确制导系统侵彻至预定位置，而后引爆弹药对防护结构进行毁伤破坏。如何提高防护结构的抗侵彻能力，达到侵不穿、炸不坏的要求，是防护工程领域关注的热点问题。

为同时抵抗钻地弹侵彻和爆炸毁伤破坏效应，防护结构通常采用成层式结构，主要包括遮弹层、分配层和结构层^[1]，如图1所示。遮弹层主要抵抗钻地弹的侵彻，迫使弹体在该层爆炸，一般采用钢筋混凝土、块石等材料；分配层一般采用粗砂、中砂等松散材料，主要用于分散弹药爆炸产生的荷载，使爆炸荷载分布在更大范围，防止爆心点下方结构层发生局部破坏，确保防护结构内部人员和设备安全；结构层主要抵抗侵彻过程中通过分配层传递下来的爆炸荷载以及重力荷载。成层式结构的优点是各层功能明确，即遮弹层主要抵抗弹体侵彻，分配层主要用于分配（分散）爆炸荷载，结构层主要抗爆炸荷载。

图  1  成层式结构示意图

Figure  1.  Schematic diagram of layered protective structure

下载: 全尺寸图片 幻灯片

为提高遮弹层的抗侵彻能力并减小其厚度，学者们^[2-3]开展了大量的研究工作，可总结为2种途径：一是提高遮弹层材料强度，如采用高强混凝土以及纤维增强高性能混凝土等；二是通过高强度材料的复合形成非均质遮弹层，可迫使弹体偏航、变形、甚至破碎，有效降低侵彻深度，如采用大粒径块石混凝土、刚玉块石混凝土、含球形颗粒（刚玉、特种陶瓷、高强钢等）的高强度混凝土等^{[2, 4-7]}。

从可靠防护主体结构角度出发，减小并可靠控制弹药爆炸产生的爆炸波经过分配层后作用于主体结构的荷载是关键。传统成层式结构分配层通常采用就地取材的中粗砂，但其性能差异大且对爆炸波的衰减性能不够高，导致难以可靠控制作用于主体结构上的荷载。多孔泡沫材料如泡沫混凝土^[8]、泡沫空心球^[9]、泡沫陶瓷空心球^[10]、聚氨酯泡沫^[11]等具有高孔隙率、低密度和低波阻抗等特点，对爆炸波的消减效果优异。其中泡沫混凝土材料性能稳定可控且施工方便，已有研究^[12]逐渐将泡沫混凝土用于成层式结构中的分配层。基于平面装药模拟核爆荷载技术，李砚召等^[13]进行了遮弹层/不同分配层的缩比模型试验，试验数据表明，以泡沫混凝土为代表的软材料分配层对核爆炸荷载具有很好的消波作用。张景飞等^[14]、高全臣等^[15]和杜玉兰等^[16]进行了小比例尺混凝土/泡沫混凝土/混凝土成层式结构在接触爆炸和浅埋爆炸下的试验研究，结果表明，由于泡沫混凝土低波阻抗性质，成层式结构能量分布上移，使得作用于结构上的荷载和能量大幅减小。

已有小比例尺缩比爆炸试验表明，以泡沫混凝土为分配层的成层式结构具有良好的抗爆性能，然而受限于试验数据及相应的理论或数值模拟分析，爆炸波在上述结构中的传播衰减机制尚不明确，无法系统阐明其与以中粗砂为分配层传统成层式结构的不同，且未能建立相应的设计计算方法，难以推广应用于防护结构的设计计算。

本文中首先对以泡沫混凝土为夹层的成层式防护结构（以下简称组合式防护结构）开展预制孔装药爆炸试验，测定特定位置处的爆炸波时程曲线和组合式防护结构损伤破坏情况。在此基础上，基于开发的Kong-Fang混凝土材料模型^[17-19]和LS-DYNA中的无网格的光滑粒子伽辽金（smoothed particle Galerkin, SPG）算法^[20]对爆炸波在组合式防护结构中的传播衰减规律和和损伤破坏机理进行系统分析，以明确泡沫混凝土在组合式防护结构中的能量调控机制。

1.   组合式防护结构预制孔装药爆炸试验

为明确钻地弹对组合式防护结构的毁伤破坏效应以及爆炸波在其中的传播衰减机制，采用预制孔装药爆炸模拟钻地弹先侵彻后爆炸，开展了组合式防护结构抗爆性能试验。

1.1   试验概况

图2给出了组合式防护结构预制孔装药爆炸试验的示意图，圆柱形组合式防护结构直径为2.1 m，外围采用厚16 mm的Q235钢箍约束，并在顶部和中心位置进行局部加强。组合式防护结构第1层为厚1.4 m的CF120超高性能混凝土（配合比见表1）遮弹层，上表面中心预留直径105 mm、深度860 mm的圆柱形预制孔用以填充TNT炸药模拟钻地弹的侵彻爆炸作用；第2层为厚0.5 m的C5泡沫混凝土（配合比见表2）层；第3层为厚0.5 m的C40钢筋混凝土主体结构层。

图  2  组合式防护结构预制孔装药爆炸试验示意图

Figure  2.  Schematic diagram of blast test on composite protective structure

下载: 全尺寸图片 幻灯片

表  1  CF120超高性能混凝土配合比（单位：kg/m³）

Table  1.  Mix proportion of CF120 ultra-high performance concrete (unit: kg/m³)

高强 水泥	活性 粉末	砂	石子		钢纤维	水	减水剂
			5～10 mm	10～16 mm
523	230	623	407	611	25	122	21

下载: 导出CSV 

| 显示表格

表  2  泡沫混凝土配合比

Table  2.  Mix proportion of foam concrete

强度等级	设计密度/（kg∙m−3）	粉煤灰/（kg∙m−3）	矿渣/（kg∙m−3）	硅酸钠溶液/（kg∙m−3）	氢氧化钠固体/（kg∙m−3）	水/（kg∙m−3）	泡沫/（L∙m−3）
C1	500	202	202	188	14	72	600
C3	900	362	362	339	25	133	406
C5	1200	484	484	452	33	182	108
C10	1400	564	564	528	38	213	65

下载: 导出CSV 

| 显示表格

圆柱形TNT药柱直径为98 mm，长为554 mm，采用尾部中心点的起爆方式。同时为得到爆炸波在组合式防护结构中的传播衰减规律，在结构中特定位置预埋4个PVDF（聚偏氟乙烯）应力传感器（图3），其中2个传感器位于遮弹层中，另外2个位于主体结构表面。遮弹层中传感器具体位置为预制孔底部中心正下方0.4 m处（编号1-1）及同一平面上距离0.52 m处（编号1-2）；主体结构层中传感器具体位置为正中心处（编号3-1）及同一平面上距离0.52 m处（编号3-2）。从防护结构抗爆角度，往往重点关注沿靶体法向的爆炸压缩波。因此，PVDF应力传感器在布置时要求其表面与靶体（装药）轴线垂直，以此测得沿靶体（装药）法向的爆炸压缩波应力时程曲线。

图  3  PVDF应力传感器的埋置

Figure  3.  Location of PVDF transducers

下载: 全尺寸图片 幻灯片

1.2   试验结果

图4(a)给出了爆炸后组合式防护结构顶面的破坏情况，可以看出遮弹层顶部损伤破坏严重，径向裂缝一直扩展到靶体边界，CF120混凝土部分碎块被抛掷出靶体。为明确组合式防护结构内部的损伤破坏情况，试验后将结构沿径向剖开（图4(b)），可以看到CF120混凝土遮弹层顶部出现漏斗坑，漏斗坑直径与靶体直径相当；预制孔装药附近混凝土出现粉碎破坏，粉碎区周围的混凝土发生破碎；遮弹层底部由于反射拉伸波作用产生震塌裂缝。而C5泡沫混凝土层仅出现少量竖向裂纹，C40钢筋混凝土主体结构层完好无损。

图  4  试验后组合式防护结构靶体破坏情况

Figure  4.  Post-test failure in composite protective structure

下载: 全尺寸图片 幻灯片

图5给出了试验得到的各测点应力时程曲线，遮弹层中1-1处实测应力峰值为235 MPa，1-2处实测应力峰值为156 MPa；主体结构层上3-1处实测应力峰值为2.1 MPa，3-2处未能测到有效数据。由此可初步看出，泡沫混凝土层能够有效衰减爆炸荷载，从而降低作用在主体结构上的荷载。

图  5  试验测得的应力时程曲线及与数值模拟结果的对比

Figure  5.  Comparisons of stress-time histories between test data and numerical prediction

下载: 全尺寸图片 幻灯片

2.   数值模型及试验验证

组合式防护结构预制孔装药爆炸试验存在测试困难、数据有限、试验周期长等诸多问题，数值模拟成为机理分析的主要工具。装药对组合式防护结构破坏效应的数值模拟涉及到爆轰产物的膨胀飞散、混凝土类材料的大变形及损伤破坏、爆炸波在不同介质界面处透反射等问题，合理的材料模型和合适的数值算法对上述问题的准确模拟十分重要。

在爆炸荷载作用近区，混凝土材料受高压和高应变率作用并表现出近似流体特性，经典的损伤塑性模型难以较好描述该状态下混凝土的力学行为。因此，目前普遍采用的冲击爆炸荷载下的混凝土材料模型为流体弹塑性模型，即分别采用强度面和状态方程描述混凝土偏量和球量行为。Kong等^[17]基于混凝土压力-体积应变试验数据和三轴压缩试验数据，对比分析了常用的HJC模型、RHT模型、K&C模型和Kong-Fang模型（混凝土材料流体弹塑性模型）等4种流体弹塑性模型对状态方程和最大强度面的预测效果，发现Kong-Fang模型整体上优于另外3种常用模型。Kong-Fang模型具有良好预测效果的原因在于：以往模型高围压试验数据较少，Kong-Fang模型在参数标定时补充了高围压下的相关试验数据；并且Kong-Fang模型通过关联流动法则考虑球量对偏量的影响，与高围压下混凝土材料的力学行为更为相符。

对装药爆炸下混凝土材料损伤破坏的数值模拟通常采用有限元结合单元删除的方法，孔祥振等^[21]对上述方法进行了系统评估，指出上述方法中的单元删除准则对混凝土结构损伤破坏、应力波传播和结构抗力的预测结果影响非常大，应谨慎使用。最近，高矗等^[22]利用Kong-Fang模型^[17-19]和LS-DYNA的多物质ALE算法^[23]开展了混凝土中爆炸波衰减规律的数值模拟研究，结果表明基于Kong-Fang模型的多物质ALE算法可有效描述爆轰产物的膨胀飞散、混凝土的损伤破坏及爆炸波衰减规律。然而，由于多物质ALE算法中的一个单元可能存在多种物质，当单元内不同物质的差异较大时会得到非物理的结果，从而无法准确模拟爆炸波在不同介质界面处透反射规律^[24]。

对装药爆炸下混凝土材料损伤破坏的另一种模拟方法为无网格法，由于SPG方法^[20]可较好地处理大变形和多物质界面等问题，该方法通过粒子间的键基断裂来描述材料的损伤破坏，有效克服了粒子法收敛性差、拉伸不稳定和有限元法单元删除导致的动量、能量不守恒等问题。基于此，采用Kong-Fang模型和SPG方法，建立数值模型、对相关材料参数进行标定并开展网格敏感性分析，进一步利用前述试验数据对数值模型进行验证。

2.1   数值模型

组合式防护结构预制孔装药爆炸试验的数值模型如图6所示，CF120混凝土遮弹层、C5泡沫混凝土层、C40钢筋混凝土主体结构层以及钢箍等固体采用SPG方法描述，炸药和空气等流体采用欧拉结构化网格（S-ALE）^[25]建模，网格域大小为1 m×1 m×2 m，外围为透射边界。流体和固体间通过LS-DYNA中的流固耦合相互作用，组合式防护结构各层界面及与钢箍间界面的相互作用采用自动面面接触算法描述，炸药和钢箍位置尺寸等均与试验保持一致。SPG算法的整体计算效率与传统有限元方法相比偏低，且只支持三维建模。考虑到经泡沫混凝土层到达C40钢筋混凝土主体结构上的爆炸荷载较小（见图5(c)），主体结构层完好无损（见图4(b)），内部的钢筋（按构造配筋）尚未屈服。因此，在对C40钢筋混凝土主体结构层进行建模时，忽略处于未屈服状态的钢筋的影响，以此来提升计算效率。

图  6  组合式防护结构预制孔装药爆炸数值模型

Figure  6.  Numerical model of blast test on composite protective structure

下载: 全尺寸图片 幻灯片

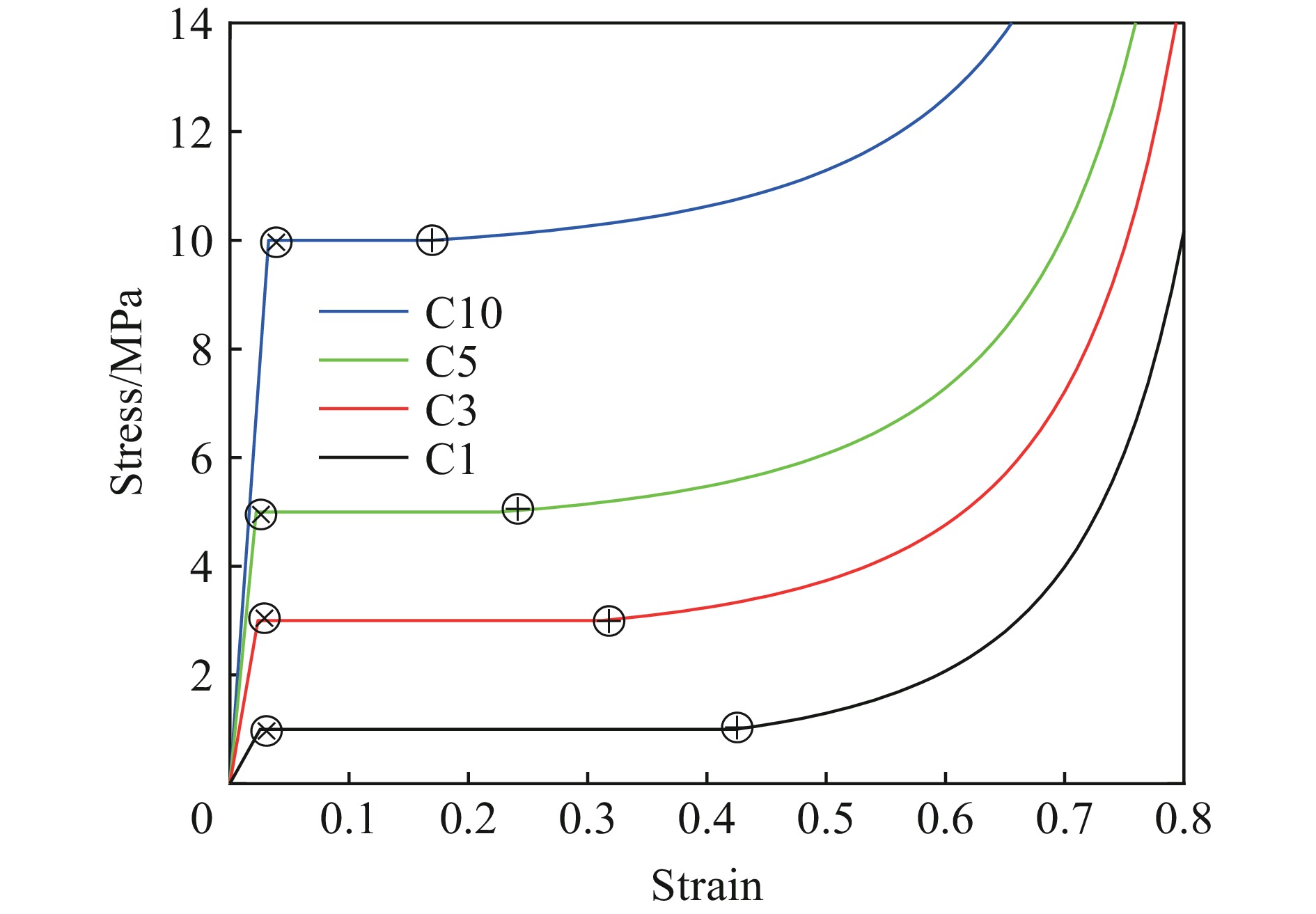
CF120超高性能混凝土和C40混凝土的实测抗压强度分别为134和54 MPa，由Kong-Fang混凝土材料模型描述，模型参数主要包括强度面参数和状态方程参数。高强混凝土和普通混凝土的上述参数参见文献[22]，其他参数采用Kong-Fang模型自动生成算法得到^[18-19]。泡沫混凝土采用Soil and Foam模型描述，图7给出了典型泡沫混凝土的应力应变曲线，其中，标记点“$\otimes$”处的横坐标和纵坐标分别代表泡沫混凝土的屈服应变和屈服应力，标记点“⊕”处的横坐标代表泡沫混凝土的密实应变，标记“$\otimes$”和“⊕”之间应力应变关系的水平直线段称为屈服平台。可以看出泡沫混凝土具有较长的屈服平台，具体模型描述及典型泡沫混凝土（C1、C3、C5和C10）的参数参见文献[26]。

图  7  典型泡沫混凝土应力-应变曲线

Figure  7.  Stress-strain curves of typical foam concrete

下载: 全尺寸图片 幻灯片

TNT炸药和空气分别采用Jones-Wilkins-Lee状态方程和多项式状态方程描述^[22]。钢箍采用屈服应力为210 MPa的理想弹塑性材料。

2.2   网格敏感性分析

合适的网格尺寸可兼顾数值模拟计算精度和效率，利用上述建立的数值模型进行网格敏感性分析。图8~9分别给出了网格尺寸为10、20和40 mm时数值模拟预测得到的测点1-1和1-2应力时程曲线和遮弹层的损伤破坏情况，可以看出，网格尺寸为10和20 mm时测点波形和靶体损伤的预测结果几乎没有差异，为提高计算效率，后续数值模拟均采用尺寸为20 mm的网格。

图  8  不同网格尺寸预测得到的应力时程曲线

Figure  8.  Stress-time histories predicted by using different mesh sizes

下载: 全尺寸图片 幻灯片

图  9  不同网格尺寸预测得到的CF120混凝土遮弹层损伤破坏云图

Figure  9.  Numerically predicted damage in CF120 concrete bursting layer by using different mesh sizes

下载: 全尺寸图片 幻灯片

2.3   数值模拟结果及与试验对比

基于上述数值模型及网格尺寸，对试验进行了精细化数值模拟，通过对比分析数值模拟预测的靶体损伤情况和测点应力时程曲线与试验结果，验证数值模型的可靠性。

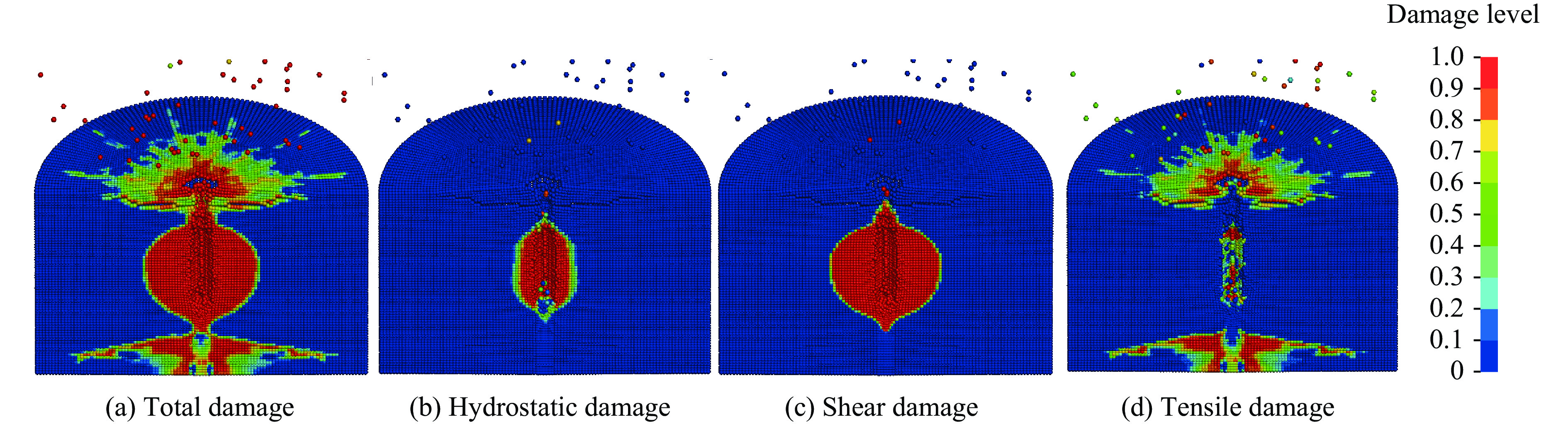
Kong-Fang模型考虑了3种损伤机制，即孔隙坍塌引起的静水压缩损伤、剪切裂缝引起的剪切损伤和拉伸裂缝引起的拉伸损伤，并通过引入与应力状态相关的权重因子考虑3种损伤的相互作用^[19]。图10分别给出数值模拟预测得到的CF120混凝土遮弹层总损伤、静水压缩损伤、剪切损伤和拉伸损伤云图。可以看出，数值模拟预测的遮弹层损伤破坏情况可分为4个区域：(1) 顶部成坑区，对比图10(a)和(d)与试验结果（图4）可发现，数值模拟得到的成坑区域、裂缝分布及尺寸与试验结果基本一致，该区域主要为拉伸损伤，由爆炸压缩波传至遮弹层上表面反射拉伸波导致；(2) 装药附近破碎区，由图10(a)～(c)可知，预制孔装药近区出现明显的剪切损伤和体积压缩损伤耦合破坏，试验中表现为装药附近混凝土出现粉碎破坏，且粉碎区周围的混凝土发生破碎，试验和数值模拟得到的的破碎区域范围比较吻合；(3) 底部震塌区，由图10(a)和(d)可以看出底部震塌区主要为拉伸损伤，由爆炸压缩波传至遮弹层下表面反射拉伸波导致，其范围与试验结果基本一致；(4) 侧面径向裂缝区，由图10(d)可知，该区域主要为拉伸损伤，由钢箍的约束效应引起，预测的裂缝位置和形状与试验结果吻合较好。

图  10  CF120混凝土遮弹层损伤云图

Figure  10.  Numerically predicted damage in the CF120 concrete bursting layer

下载: 全尺寸图片 幻灯片

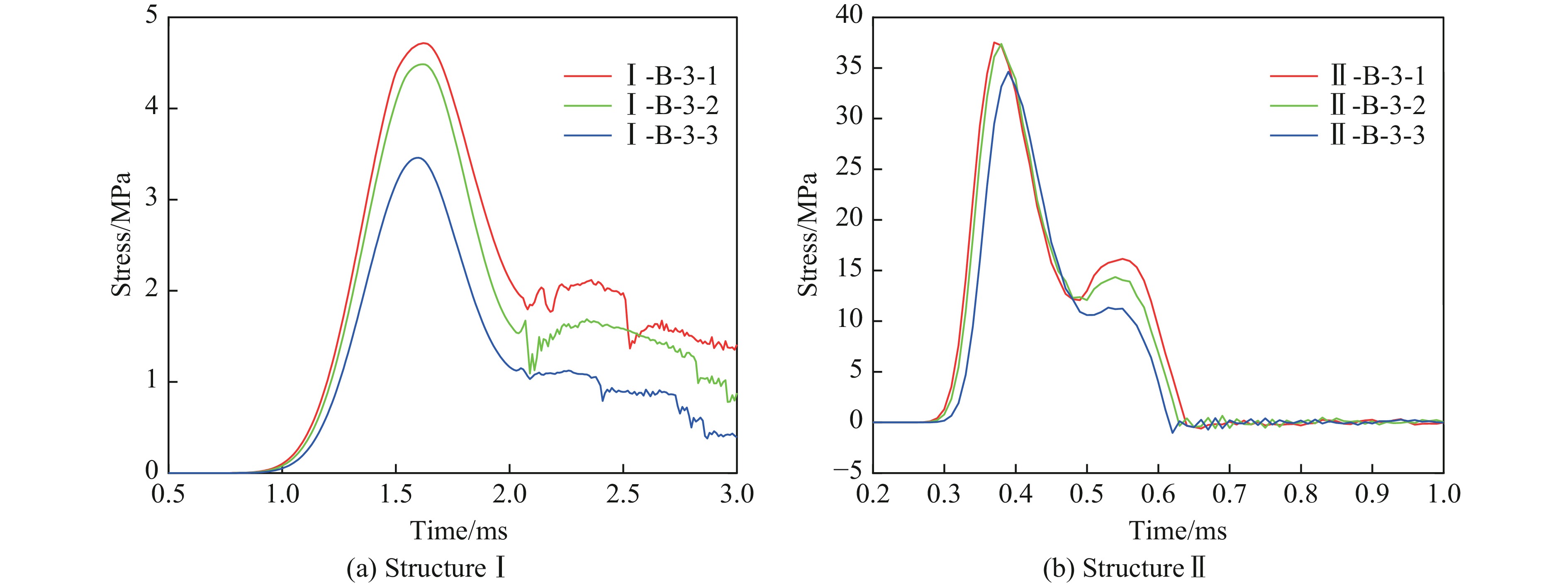
图5给出了数值模拟预测的测点应力时程曲线与试验结果的对比。可以看出，遮弹层中的测点1-1和1-2的峰值和正压持时的数值预测结果与试验数据均吻合非常好；C40钢筋混凝土结构层上表面测点2-1正压持时的数值预测结果与试验数据吻合较好，但峰值较试验值偏高，考虑到爆炸波在不同介质界面的复杂透反射以及试验测试的离散性，认为此结果可靠。

综上所述，数值模拟预测的混凝土遮弹层各区域损伤破坏情况以及爆炸波传播衰减情况均与试验结果吻合较好，验证了Kong-Fang模型结合SPG算法的可靠性。

3.   组合式防护结构的抗爆机制

基于上述经验证的数值模型，进一步分析弹药爆炸作用下组合式防护结构的损伤破坏及对应力波传播的衰减规律。

注意到实际防护结构中不存在边界钢箍，因此在第2节建立的数值模型的基础上将钢箍去掉并改为透射边界，主体结构层下表面为自由边界。为揭示组合式防护结构的抗爆机制，分别对有泡沫混凝土层的结构（编号Ⅰ）和无泡沫混凝土层（用等厚度CF120混凝土替代）的结构（编号Ⅱ）进行装药爆炸的数值模拟。从上到下各层依次编号为1～3，层1和层2界面、层2和层3界面依次编号A和B，如图11所示。

图  11  组合式防护结构数值模型及测点布置示意图

Figure  11.  Numerical model of composite protective structure subjected to explosion and locations of the gauges

下载: 全尺寸图片 幻灯片

3.1   弹药对组合式防护结构的毁伤破坏效应

图12给出了数值模拟预测的结构Ⅰ遮弹层损伤云图，对比图10可以看出，将侧面钢箍去掉并施加透射边界条件后，遮弹层中侧面径向裂缝消失，顶部漏斗坑直径减小且底部震塌区破坏程度降低。上述结果表明，试验中有限靶体径向尺寸和钢箍约束对结构损伤破坏影响显著。因此对于实际问题的数值模拟及分析应采用透射边界以模拟半无限情形。另外，可以看出，在装药近区预测的损伤破坏情况几乎没有差异。这表明结构径向尺寸和钢箍约束对近区的压剪损伤破坏影响较小。

图  12  结构Ⅰ遮弹层损伤云图

Figure  12.  Numerically predicted damage in the CF120 concrete bursting layer of structure Ⅰ

下载: 全尺寸图片 幻灯片

图13给出了数值模拟预测的结构Ⅱ遮弹层损伤云图。与结构Ⅰ相比，开坑破坏及装药近区压剪破坏基本相同，而底部震塌区完全消失。这是由于泡沫混凝土层的存在会使爆炸波在界面A处反射拉伸波，进而使得爆炸能量向上部遮弹层转移，造成震塌破坏。

图  13  结构Ⅱ遮弹层损伤云图

Figure  13.  Numerically predicted damage in the CF120 concrete bursting layer of structure Ⅱ

下载: 全尺寸图片 幻灯片

图14给出了组合式结构Ⅰ和结构Ⅱ中主体结构层的损伤云图，可以看出有泡沫混凝土层时（结构Ⅱ）主体结构层完好无损，无泡沫混凝土层时（结构Ⅱ）主体结构层底部发生较为严重的拉伸损伤破坏。这是由爆炸波在主体结构层内侧自由表面反射拉伸波导致的。

图  14  2种组合式结构（结构Ⅰ和 Ⅱ）中主体结构层的损伤云图

Figure  14.  Numerically predicted damage in the structure layers of structures Ⅰ and Ⅱ

下载: 全尺寸图片 幻灯片

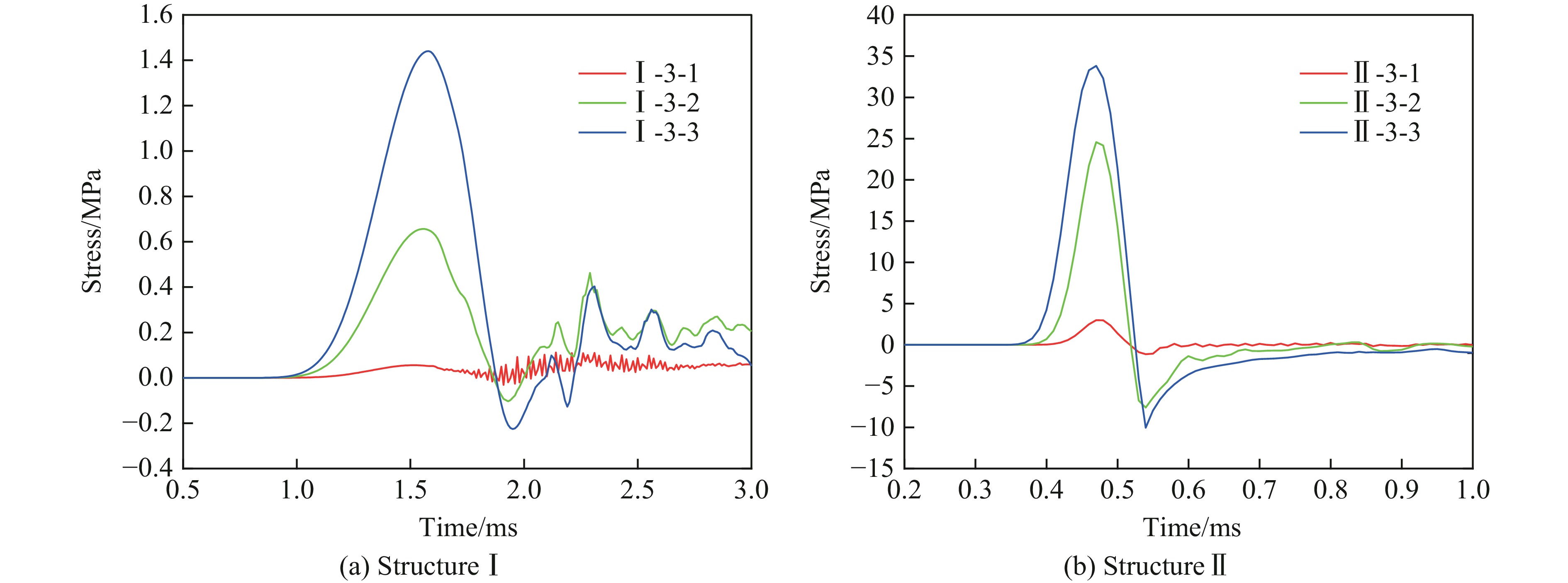
图15给出了组合式结构Ⅰ和Ⅱ主体结构层3处测点的波形曲线，测点位置如图11所示，可以看出组合式结构Ⅰ中3处测点的应力峰值远低于结构Ⅱ，且结构Ⅱ测点3-1和3-2的拉应力已超过C40混凝土的拉伸强度，因此出现图14所示的拉伸损伤破坏。上述分析表明，在遮弹层和主体结构层中设置泡沫混凝土层可以有效削弱爆炸波对主体结构层的作用，具体抗爆消波机制将在后续结合能量分布及爆炸波的传播进一步分析。

图  15  2种组合式结构（结构Ⅰ和Ⅱ）中主体结构层测点的应力时程曲线

Figure  15.  Stress-time histories of gauges in structural layers of structures Ⅰ and Ⅱ

下载: 全尺寸图片 幻灯片

3.2   爆炸能量在组合式防护结构中的分布

组合式防护结构在弹药爆炸作用下的损伤破坏与爆炸能量在各层的分布密切相关^[27]，为弄清组合式防护结构的抗爆机制，以下对爆炸能量的分布规律进行分析。各层的爆炸能量是指组合式防护结构靶体中某一层所吸收的爆炸总能量，包括动能和内能。

图16给出了上述2种结构中各层能量的对比。可以看出，2种结构大部分能量均分布在上部混凝土遮弹层（层1）中；对于有泡沫混凝土层的组合式结构Ⅰ，各层能量占结构总能量的比例分别99.905%（层Ⅰ-1）、0.091%（层Ⅰ-2）、0.004%（层Ⅰ-3）；而对于无泡沫混凝土层的结构Ⅱ，各层能量占结构总能量的比例分为99.489%（层Ⅱ-1）、0.395%（层Ⅱ-2）、0.117%（层Ⅱ-3）。

图  16  2种组合式结构（结构Ⅰ和Ⅱ）中各层能量对比

Figure  16.  Comparison of blast energy distribution in each layer of structures Ⅰ and Ⅱ

下载: 全尺寸图片 幻灯片

对比2种结构各层的能量，可发现含泡沫混凝土层的组合式防护结构Ⅰ的各层能量与结构Ⅱ对应各层能量的比分别为1.091（层1）、0.250（层2）和0.041（层3）。由此可见，泡沫混凝土层的存在使得传入主体结构层的能量大幅降低，其能量仅为无泡沫混凝土层时主体结构层能量的4.1%。

上述分析表明，在遮弹层和主体结构层之间加入泡沫混凝土层会改变防护结构各层能量分布，爆炸能量向上部遮弹层转移，使得主体结构层的能量大幅度降低。

3.3   爆炸波在组合式防护结构中的传播衰减

泡沫混凝土层会改变防护结构中各层能量分布，而各层能量分布与爆炸波在各层介质中的传播衰减以及在界面处的透反射密切相关。因此对爆炸波在组合式防护结构中的传播衰减规律进行分析。

图17（a）给出了结构Ⅰ界面A处层2（编号Ⅰ-A-2）3处测点的爆炸波波形，测点具体位置见图11，图17（b）给出了结构Ⅱ相应位置处的爆炸波波形。对比可以看出，有泡沫混凝土层的结构Ⅰ爆炸波峰值相比结构Ⅱ显著降低，且持续时间增大。这主要是由于爆炸波传播到2种不同介质界面时会发生透射和反射，由于CF120超高性能混凝土的波阻抗（1.18×10⁷ N·s/m³）远大于C5泡沫混凝土的（5.21×10⁵ N·s/m³），波阻抗失配程度非常高，从而使得爆炸波大部分以符号相反的拉伸波反射回CF120混凝土中，仅少部分透射进入C5泡沫混凝土层。

图  17  2种组合式结构（结构Ⅰ和Ⅱ）界面A处测点应力时程曲线

Figure  17.  Stress-time histories of three gauges at interface A in structures Ⅰ and Ⅱ

下载: 全尺寸图片 幻灯片

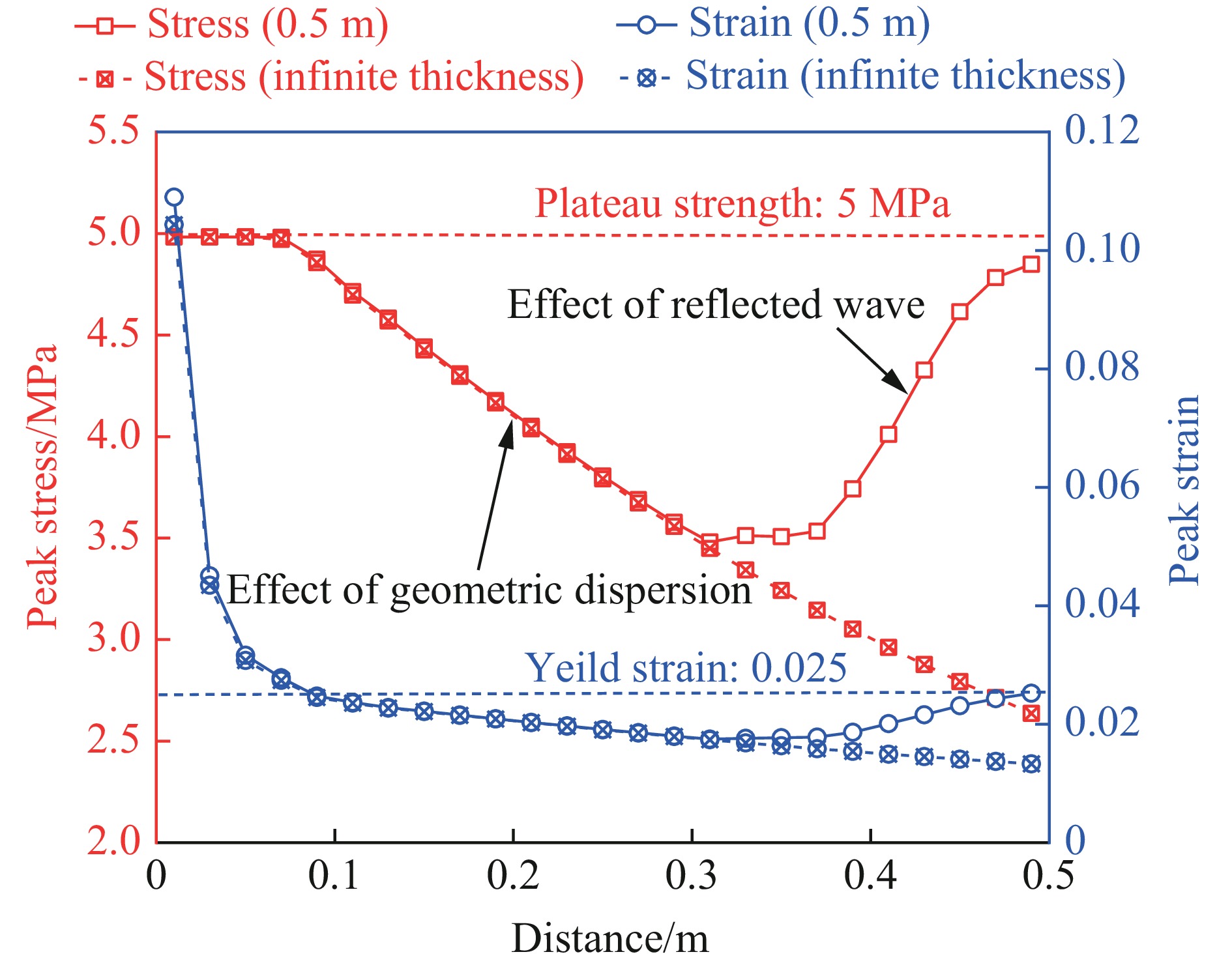
为分析爆炸波在泡沫混凝土层中的传播衰减规律，图18给出了0.5 m厚泡沫混凝土层沿中心轴线方向的应力、应变峰值分布。可以看出，在0～0.08 m范围内，泡沫混凝土的峰值应变超过屈服应变0.025（见图7），此时应力峰值恰为C5泡沫混凝土的平台应力5 MPa。随着爆炸波继续在泡沫混凝土层中传播，爆炸波衰减为弹性波（峰值应变小于屈服应变），同时由于几何弥散效应，应力峰值随传播距离增大而逐渐减小。若忽略下部主体结构层并将泡沫混凝土层视为无限厚，应力峰值和应变峰值随距离增大均将持续减小（图18中红色和绿色虚线）。

图  18  0.5 m厚C5泡沫混凝土层沿中心轴线方向的爆炸波应力、应变峰值分布情况

Figure  18.  Peak stress and peak strain of explosion wave along the central axis of 0.5 m thick C5 foam concrete layer

下载: 全尺寸图片 幻灯片

泡沫混凝土层下部为钢筋混凝土主体结构层，爆炸波传播至两层界面（图11中界面B）时将再次发生透射与反射。图19给出了主体结构层上表面3个测点的爆炸波时程曲线，测点具体位置见图11。对比可以看出，含泡沫混凝土层的结构Ⅰ相比不含泡沫混凝土层的结构Ⅱ的峰值应力大幅减小，且持续时间增长。另外，结合图18中无限厚泡沫混凝土层计算结果可以看出，爆炸波经过结构Ⅰ的界面B时，透射波应力幅值接近以2倍的入射波大小向主体结构层传播。这是由于C5泡沫混凝土的波阻抗远小于C40钢筋混凝土的（8.30×10⁶ N·s/m³），界面B可近似视为固定边界，此时透射波以接近2倍的入射波大小向另一层传播，同时反射波几乎不改变大小和符号。由于上述的反射波作用，在泡沫混凝土0.3～0.5 m厚度范围内的应力峰值和应变峰值出现增强，如图18所示，但应力峰值和应变峰值均不超过C5泡沫混凝土的屈服应力和屈服应变。

图  19  2种组合式结构（结构Ⅰ和Ⅱ）界面B处测点应力时程曲线

Figure  19.  Stress-time histories of three gauges at interface B in structures Ⅰ and Ⅱ

下载: 全尺寸图片 幻灯片

由上述分析可知，组合式防护结构的抗爆机制在于遮弹层和泡沫混凝土层之间的强波阻抗失配关系，使得爆炸能量大部分耗散在遮弹层中，大幅减少经泡沫混凝土层到达主体结构上的能量和荷载。

4.   结　论

针对以中粗砂为分配层的传统成层式结构难以可靠控制作用于主体结构上荷载的缺陷以及以泡沫混凝土为夹层的组合式防护结构抗爆机制不明等问题，首先开展了组合式防护结构预制孔装药爆炸试验，进一步基于精细化数值模拟明确了爆炸波在组合式防护结构中传播衰减规律和损伤破坏机理，主要结论如下。

(1)利用Kong-Fang模型和SPG方法可准确预测爆炸波在组合式防护结构中传播衰减规律和损伤破坏效应。

(2)在遮弹层和主体结构层之间加入泡沫混凝土层会改变防护结构各层能量分布，爆炸能量向上部遮弹层转移，避免主体结构层在爆炸波作用下发生震塌破坏。泡沫混凝土层的存在使得传入主体结构层的能量大幅降低，其能量仅为无泡沫混凝土层时主体结构层能量的4.1%。

(3)组合式防护结构的抗爆机制在于遮弹层和泡沫混凝土层之间的强波阻抗失配关系，使得爆炸能量大部分耗散在遮弹层中，大幅减小经泡沫混凝土层到达主体结构上的荷载和能量。无泡沫混凝土层时主体结构层爆炸荷载峰值应力为37.8 MPa，泡沫混凝土层的存在使得主体结构层爆炸荷载峰值应力仅为4.8 MPa，不超过C5泡沫混凝土的平台强度5 MPa。