Impact resistance of thickness-graded arrow-shaped honeycomb pedestals with negative Poisson’s ratio
-
摘要: 设计了一种箭形负泊松比的蜂窝基座结构，推导了其胞元结构的力学性能解析公式，并利用有限元方法研究了具有厚度梯度箭形负泊松比蜂窝材料的抗冲击性能。基于功能梯度材料，其基体呈连续梯度变化的概念，以胞元壁厚为自变量，设计了顺厚度梯度、逆厚度梯度型和均匀厚度的蜂窝层，并建立基座模型。在基座质量不变的前提下具体讨论了蜂窝胞元凹角及厚度梯度的不同设置情况对基座抗冲击性能的影响。结果表明，相同梯度设置情况下，胞角的变化会引起蜂窝结构等效弹性模量的变化，进而改变基座的抗冲击性能，而将胞壁厚度较小的蜂窝层放置于迎冲端时，基座整体的应力水平明显降低；将壁厚较大的蜂窝层放置于迎冲端时，基座面板的输出冲击环境能够有效地得到控制。Abstract: An arrow-shaped honeycomb pedestal with negative Poisson’s ratio was designed. An analytical formula was derived for the mechanical properties of the cell structures, and the impact resistance of the thickness-graded arrow-shaped honeycomb materials with negative Poisson's ratio was numerically studied by the explicit dynamic finite element method. Based on the concept of functionally-graded materials, honeycomb layers with pathwise thickness gradient, inverse thickness gradient and uniform thickness were designed, by taking the thickness of the cell wall as the independent variable, the relevant model was established. The influence of thickness gradients on the impact resistance of the pedestal was discussed concretely under the premise of constant pedestal mass. The results show that, under the same gradient setting, the change of cell angle will cause the change of equivalent elastic modulus of the honeycomb structure, thus changing the impact resistance of the pedestal. When the honeycomb layer with a thinner cell wall is placed at the impact end, the stress level of the pedestal is significantly reduced. By placing a honeycomb layer with a thicker cell wall on the impact end, the output impact environment of the pedestal panel can be effectively controlled.
-
Ti-6Al-4V是目前应用最广泛的钛合金，在航空航天、国防工业、医药等领域都有重要应用[1-2]，质轻且比强度高，兼具强度与韧性，综合性能优异，是结构轻量化的重要替代材料，有作为战斗部壳体、破片等的潜在应用价值。冲击加载时，Ti-6Al-4V构件快速形变将导致其局部温度急剧上升，鉴于钛、铝等活性元素在高温环境下将发生剧烈氧化放热反应[2]，构件局部温升可能诱发自燃，发生冲击反应，有助于增强战斗部毁伤威力。为全面评估含Ti-6Al-4V战斗部的毁伤威力，有必要研究Ti-6Al-4V材料的冲击反应条件及反应机理。然而，目前相关研究较匮乏。
所谓冲击反应，是指由冲击引起的材料放热化学反应，如氧化、合金化、相变等[3]。能发生冲击反应且兼具适当强度的材料称为多功能含能结构材料[3]，其通常为脆性材料，如Zr基非晶合金[4-8]、活性金属粉末混合物等[9]。在高速冲击条件下，由脆性多功能含能材料制成的结构一般将碎裂成碎片云，结构破坏模式对结构具体形式较不敏感。撞击速度越高，脆性多功能含能材料形成的碎片个数越多，冲击反应烈度越高[4, 10-12]。然而，若多功能含能结构材料为韧性材料，其结构破坏模式将随结构形式改变而改变，这可能影响其材料的冲击反应能力。需进一步分析撞击速度改变对结构破坏模式以及关联冲击反应能力的影响。
常温状态下，多功能含能结构材料性能稳定，可作为结构材料，因此，高温是冲击反应发生的重要条件之一，如Ti、Al、Mg等活性金属高温环境下极易与氧气发生氧化反应，同时释放大量热量[10, 13-14]。亚稳态且性脆的Zr基非晶合金高速撞击时结构失效生热，促使碎片产生相变与氧化，进一步释放热量[4-8]。Al、Mg粉末与Fe2O3混合物制成的铝热剂碎片高速撞击时形变与失效生热，促使铝热剂反应，进一步释放热量[9]。冲击加载下，材料快速变形、断裂失效以及材料与碰撞界面的高速摩擦等均可快速生成大量热量，提供激发冲击反应的高温环境。氧化、合金化、相变等冲击放热化学反应将进一步释放热量，维持高温环境。
反应物接触表面积是冲击反应烈度的控制参数之一。反应物颗粒尺寸越小，接触表面积越大，反应烈度越高[2, 6]。如W/Zr复合材料撞击破碎形成的颗粒尺寸在200 μm以下时，易点燃形成火星或燃烧形成火球；但更大尺寸的颗粒将难以全部氧化[6]。将铝热剂粉末研磨至纳米尺寸并充分混合制成的破片可提高铝热反应效率[9]。20 μm活性金属粉末混合而成的复合材料，撞击时更易激发金属合金化与氧化反应[14]。即使反应产物（如氧化膜）可能隔离阻断反应过程，但当碎片颗粒尺寸足够小时，生成反应产物的一次反应就可将颗粒全部反应。因此，多功能含能结构材料冲击后形成碎片的尺寸应是控制冲击反应的重要参数。
针对Ti-6Al-4V，其块体在600 ℃以下时与氧气生成约100 nm厚的致密氧化膜，将切断内部Ti-6Al-4V与氧气的接触路径，阻断内部材料的进一步氧化反应[15]。所谓块体，即其最小尺寸在毫米或以上量级。尽管Ti-6Al-4V燃烧产物易开裂脱落[16]，无法切断氧气与内部材料的接触路径，但其块体燃烧条件较为苛刻，启燃温度约3 727 ℃，稳定燃烧温度约2 937 ℃[17]。这说明块体Ti-6Al-4V在高温环境下化学性能仍较为稳定，这也是其作为抗高温结构材料的重要原因之一。
爆炸加载驱动Ti-6Al-4V膨胀环的试验中发现，膨胀环碎裂成毫米尺寸的碎块。加载应变率为102 s−1或材料为粗晶粒时，尽管膨胀环中激发了绝热剪切带，但断面无明显氧化痕迹；细晶粒且加载应变率达104 s−1时，断裂面呈紫蓝色，有熔化痕迹[2]。尽管未激发冲击反应，但说明在足够多的热量供给及足够大的氧气接触表面积时，活性金属Ti-6Al-4V仍存在冲击反应的可能。
鉴于Ti-6Al-4V为韧性材料，本文设计两种Ti-6Al-4V弹体，以期撞击混凝土后获得不同的结构破坏模式；开展两种弹体正侵彻混凝土试验，观察弹体是否发生冲击反应；从宏观和细观两个层面观测侵彻后弹体，分析弹体的破坏模式；基于碎片特征揭示Ti-6Al-4V材料的冲击反应条件与机理，为其作为多功能含能结构材料的应用奠定基础。
1. 两种Ti-6Al-4V弹体侵彻混凝土的试验现象分析
本文设计了两种弹体，如图1所示。一种头部尖卵形，壳体为空心圆柱形，全部由Ti-6Al-4V制成，称为钛合金弹；另一种头部为碳/碳复合材料，壳体为Ti-6Al-4V空心圆柱，称为复合弹。侵彻靶标为无筋混凝土圆柱，外裹钢皮，混凝土无约束抗压强度48 MPa。弹靶几何尺寸见表1。弹体采用口径25 mm滑膛火炮发射，正侵彻混凝土靶体，网靶通断测量弹体着靶速度，高速摄影记录弹靶作用过程，试验布局见图2。
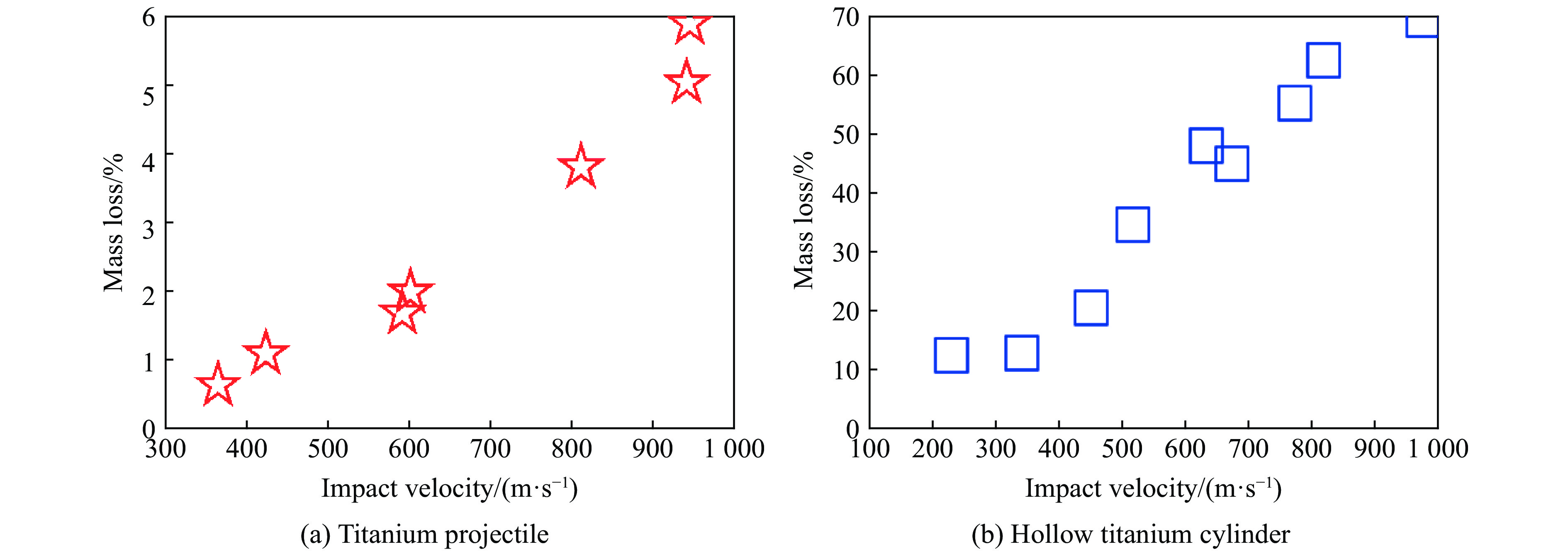
表 1 弹靶几何尺寸Table 1. Dimensions for projectiles and target类别 直径/mm 长度/mm 质量/g 钛合金弹 25.3 180.8 220 复合弹 25.3 206.0 183 混凝土靶 500.0 400.0 − 两种弹体侵彻混凝土靶标的试验结果分别见表2和表3，侵彻前后弹体形状对比分别见图3和图4。由图表可知，钛合金弹侵彻后仍能保持结构基本完整，仅弹体表面有摩擦磨损，质量损失在6%以内；而随着靶速度增加复合弹破坏加剧，复合弹的钛合金空心圆柱发生大变形并撕裂成块，损失质量可高达70%。
表 2 钛合金弹侵彻试验结果Table 2. Experimental results for titanium projectiles弹号 原始弹直径/
mm原始弹长度/
mm原始弹质量/
g实测弹速/
(m·s−1)试后弹质量/
g试后弹长/
mm弹坑尺寸/mm a b c A01 25.26 180.80 220.4 364 219.0 180.7 200 162 83 A02 25.23 180.98 219.8 423 217.4 181.1 320 290 95 A05 25.24 180.84 220.3 591 216.6 178.8 390 310 130 A04 25.26 180.97 221.4 601 217.0 179.3 420 300 120 A07 25.22 180.82 219.5 772 − − 碎散 碎散 碎散 A08 25.24 180.80 220.7 811 212.3 177.0 碎散 碎散 碎散 A12 25.24 180.90 220.5 941 209.4 174.2 碎散 碎散 碎散 A11 25.24 180.78 220.6 945 207.6 171.7 碎散 碎散 碎散 注：（1）a表示靶面弹坑最长长度，b表示弹坑最短长度，c表示弹坑深度；（2）A07弹未找到。 表 3 复合弹侵彻试验结果Table 3. Experimental results for composite projectile弹号 原始弹直径/
mm原始弹长度/
mm原始弹质量/
g钛合金空心
圆柱质量/g实测弹速/
(m·s−1)试后弹质量/
g试后弹长/
mm弹坑尺寸/mm a b c B03 25.25 206.12 183.50 132.20 222 143.0 149.9 无弹坑 无弹坑 无弹坑 B02 25.26 205.74 183.20 131.90 282 132.0 148.1 65 45 4 B01 25.22 205.86 181.70 130.00 341 113.7 134.1 80 60 3 B05 25.27 206.12 184.00 132.80 424 115.6 133.2 160 135 22 B06 25.24 206.32 183.40 132.20 516 105.1 129.0 120 105 33 B04 25.23 205.76 182.00 130.60 574 85.5 106.9 180 155 35 B08 25.24 206.00 182.80 131.80 681 68.7 77.2 230 200 45 B11 25.21 205.96 181.80 131.10 719 72.3 80.2 250 250 45 B09 25.24 206.10 183.50 132.30 813 59.3 82.0 240 230 63 B07 25.23 205.84 181.60 130.70 857 49.0 63.7 300 260 70 B10 25.25 205.86 182.90 131.50 1008 40.4 52.3 290 240 90 
图 3 侵彻前后钛合金弹形状对比Figure 3. Shape variation of titanium projectile before and after penetration test
图 4 侵彻前后复合弹形状对比Figure 4. Shape variation of composite projectile before and after penetration test表4给出了不同着速时钛合金弹侵彻混凝土的高速摄影图像。当着速为364 m/s时（A01），触靶40 μs后，钛合金弹尖有零星火星，形成的火光并不连续；在后续侵彻过程中，A01的零星火星未能形成明亮的火光，在侵彻后约400 μs时火星几乎全部湮灭。当着速增至591 m/s时（A05），钛合金弹尖有明亮火光，连续且对称分布在弹尖位置，反应形成燃烧火球并随侵彻进行持续增大，在约400 μs时充满高速摄影的整个视野，持续反应至约1 ms。在本文研究撞击速度范围内，侵彻混凝土时，钛合金弹发生了冲击化学反应。随撞击速度增加，钛合金弹撞击混凝土靶的化学反应烈度逐渐增加，反应持续时间逐步增长。这说明，即使为韧性材料，相同结构形式时，撞击速度仍可表征多功能含能结构材料的反应烈度。
表 4 同时刻不同着靶速度时钛合金弹撞击混凝土靶的高速摄影图像对比Table 4. High-speed photographies for titanium projectiles penetrating into concrete target at different impact velocities时间/μs A01 A02 A05 A04 A07 A08 A12 A11 364 m/s 423 m/s 591 m/s 601 m/s 772 m/s 811 m/s 941 m/s 945 m/s 0 
40 
100 
200 
400 
800 
1200 
表5给出了不同着速时复合弹侵彻混凝土的高速摄影图像。可以看出，在本文研究着速范围内，复合弹触靶后，头部碳/碳复合材料破碎成粉末，后部钛合金空心圆柱随进。在整个侵彻过程中，高速摄影视野内只能见到碳/碳复合材料和混凝土靶的破碎粉尘，未见反应火星。这说明在本文研究速度范围内，复合弹撞击混凝土未发生冲击反应。
表 5 同时刻不同着靶速度时复合弹撞击混凝土靶的高速摄影图像对比Table 5. High-speed photographies for composite projectiles penetrating into concrete target at different impact velocities时间/μs B03 B02 B01 B05 B06 B04 B08 B07 B10 222 m/s 282 m/s 341 m/s 424 m/s 516 m/s 574 m/s 681 m/s 857 m/s 1 008 m/s 0 
40 
100 
200 
400 
800 
类似撞击速度时，复合弹未发生冲击反应，而钛合金弹有冲击反应，这说明当多功能含能结构材料为韧性材料时，撞击速度不再能统一表征不同结构形式的冲击反应。需进一步分析结构破坏模式、反应机理等，获得激发冲击反应的条件。
2. 两种Ti-6Al-4V弹体的破坏模式宏细观分析
2.1 宏观分析
侵彻后钛合金弹结构完整，仅头部钝化，弹体表面有黑色附着物及平行犁沟，如图3所示。随撞击速度增高，钛合金弹质量损失增加，如图5(a)所示。着靶速度接近1 000 m/s的高速撞击时，随着速增加，钛合金弹质量损失有加速增加的趋势。在本文研究速度范围内，钛合金弹总质量损失仅在6%以下。
图 5 钛合金弹与钛合金空心圆柱的质量损失Figure 5. Mass loss for hollow titanium cylinders and titanium projectiles与钛合金弹不同，撞击混凝土后，复合弹中钛合金空心圆柱产生结构大变形，撕裂成块，呈花瓣状破坏模式，如图4所示。钛合金空心圆柱撞靶后的大变形展现了弹体材料Ti-6Al-4V良好的塑性形变能力。随撞击速度增加，钛合金空心圆柱的剩余质量迅速减少，如图5(b)所示。在本文研究撞击速度范围内，其质量损失可高达70%。试验后回收部分钛合金空心圆柱的碎片，如图6所示。碎片多呈长条状，若将碎片近似为长方体，则最长边与最短边之比约为5。除长度方向外，其余两方向尺度之比约为2。碎片撕裂面一般贯穿整个厚度方向，且空心圆柱壁厚通常为碎片最短边长。碎片形状与爆轰驱动的非控破片形状类似[18]，即长方体碎片三方向平均长度之比约为l1∶l2∶l3=1∶2∶5。因此，针对钛合金空心圆柱，其壁厚仅2.6 mm，碎片其他两方向特征尺寸分别为5.2 mm和13 mm。
2.2 细观分析
2.2.1 Ti-6Al-4V原始细观组织
本文所用两种弹体中Ti-6Al-4V结构均由锻造棒材制成，弹材原始金相组织见图7。由图可知，弹材细观组织为α+β的两相组织，最小尺寸为微米量级。
2.2.2 钛合金弹
取A08的头部横、纵截面，以及A12的壳体中段横截面开展金相分析，弹体外表面附近区域的金相形貌见图8。由图可知，与靶相互作用的弹体头部和壳体外表面绝大部分区域附着熔融区（re-solidification zone, RSZ），而金相试样切割面无熔融区覆盖，见图8(d)。熔融区为氧化物与弹靶碎渣等的混合物，其厚度从弹尖到壳体逐渐减薄，但均在0.1 mm以下。这说明侵彻混凝土过程中，钛合金弹体表面曾经历高温。弹体表面几乎无明显热影响区（heat affected zone, HAZ），在弹尖纵剖面熔融区与未变形组织间发现厚度仅约数微米的热影响区，区域内细观组织有方向趋同的结构变化，类似剪切变形，如图8(b)所示。这说明即使弹体表面温度升高，但至多使弹体表面微米厚度内材料软化。在弹靶摩擦力作用下，软化后材料发生细观组织形变，而所升高的温度不足以促使材料发生明显相变。弹体表面有碎片颗粒即将脱落，见图8(a)和(c)，脱落颗粒形貌与切削碎片类似，尺寸在微米量级。混凝土骨料等硬质颗粒切削软化后弹体表面材料，是弹体表面形成脱落碎片颗粒的重要原因[19-20]。
图 8 钛合金弹侵彻后头部与壳体表面金相形貌（OMZ表示未变形区）Figure 8. Metallograph of outer-surface in titanium projectile after penetration (OMZ represents origainal material zone)鉴于Ti-6Al-4V良好的塑性变形特征与剪切敏感性[2, 21]，在金相分析中特别关注了侵彻诱发的剪切带，其金相形貌见图9，其中SB代表shearing band。由图可知，在A08头部横、纵截面与A12壳体横截面发现了大量剪切带，带宽为微米量级，长度在10−4 m及以上量级。剪切带在弹体表面围成颗粒，极易与弹体脱离。脱落颗粒尺寸在10−4 m量级。
图 9 钛合金弹侵彻后头部和壳体表面剪切带金相形貌Figure 9. Metallograph of shear band (SB) on titanium projectile after penetration综上所述，侵彻混凝土过程中，钛合金弹表面与混凝土高速摩擦，将促使弹体表面数微米厚区域内材料温度升高并软化。在弹靶摩擦力与混凝土骨料等硬质颗粒切削作用下，高温软化的弹体表面区域材料易剪切变形，并形成颗粒碎片与弹体脱离，碎片尺寸为微米量级。弹体表面区域发现大量剪切带，其围成的碎片颗粒尺寸在10−4 m量级。脱离弹体表面的微米至百微米尺寸碎片易高温氧化甚至燃烧，其产物与弹靶碎渣等混合，粘附在弹体表面，形成熔融区。因此，细观组织剪切变形是钛合金弹侵彻混凝土的主要破坏机理，形成的碎片尺寸在微米至百微米量级。
2.2.3 钛合金空心圆柱
侵彻后钛合金空心圆柱撕裂面的金相组织如图10所示。可见，与钛合金弹不同，空心圆柱表面未覆盖熔融区，说明空心圆柱表面未与混凝土高速摩擦。撕裂面“蓝化”，与原始组织有色差，见图10(a)，说明撕裂面释放了大量热量，将其高温氧化。剪切带沿撕裂方向发展，其形貌与钛合金弹中剪切带类似，宽度数微米，见图10(b)。这说明钛合金空心圆柱碎块的撕裂面沿剪切带方向发展。
图 10 侵彻后钛合金空心圆柱金相形貌Figure 10. Metallograph of hollow titanium cylinder after penetration综上所述，侵彻混凝土时，钛合金弹与钛合金空心圆柱的破坏模式差异显著：前者主要以细观组织剪切变形为主要破坏模式，形成尺寸从微米到10−4 m量级的碎片颗粒；而后者撕裂面沿剪切带方向发展，围成大块碎片，尺寸在毫米及以上量级。这两种弹体破坏形成的碎片特征差异显著。下文将分析碎片特征对Ti-6Al-4V材料冲击反应条件的影响。
3. 碎片特征对Ti-6Al-4V材料冲击反应条件的影响
3.1 碎片尺寸及个数
由上文分析可知，钛合金空心圆柱撞靶后形成大块碎片，形状可近似为长方体，三方向长度之比为l1∶l2∶l3=1∶2∶5，l1=2.6 mm。Ti-6Al-4V的密度约为4.44 g/cm3，由钛合金空心圆柱质量损失可以估算其碎片的总数，如图11(a)所示。可见，在本文研究撞击速度范围内，钛合金空心圆柱仅破碎形成至多百余个碎片。
图 11 钛合金弹与钛合金空心圆柱的碎片个数Figure 11. Number of fragments for titanium projectiles and hollow titanium cylinders针对钛合金弹，其质量损失的主要机理为细观组织剪切变形，形成的碎片颗粒尺寸分布在数微米至数百微米区间。为估算形成颗粒碎片的个数，取0.1 mm为钛合金弹撞靶后碎片的特征尺寸。基于钛合金弹横、纵截面金相分析，碎片三方向特征尺度近似相等，故简单假设钛合金弹撞靶后形成碎片为正方体，边长为0.1 mm。根据钛合金弹质量损失同样可估算其碎片个数，如图11(b)所示。由图可知，高速撞击钛合金弹可形成高达3×106个的百微米尺寸碎片，在着靶面将形成碎片云。
当钛合金弹着靶速度在423 m/s及以下时，整弹质量损失仅约1%，形成数十万个百微米尺寸量级的碎片。由表4可知，此时尽管仍有冲击化学反应，但碎片云的燃烧反应持续时间较短。当碎片数量上升至百万量级，形成的碎片云被点燃，冲击反应明显且能持续约1 ms。这说明碎片数量对钛合金弹冲击反应烈度有较大影响。
3.2 不同尺寸碎片供热及供氧效率对比
钛合金弹金相分析表明，其表面未发现明显热影响区，这意味着在侵彻形成的高温环境中，Ti-6Al-4V材料细观组织结构稳定，未发生明显相变。因此，其冲击反应主要为活性元素与氧气发生的氧化或燃烧反应，反应表达式可表示为：
Ti-6Al-4V+O2→TiO+TiO2+Al2O3+V2O5+Heat (1) 反应发生的先决条件是充足的热量输入及足够的氧气供给。
热量与氧气通过反应物质表面输入，其供应效率由单位体积反应物质获得的热量或氧气表征，即
g=γAV (2) 式中：g为反应物质的供热或供氧效率，γ为反应物质的单位表面积热量或氧气输入量，
A 为反应物质表面积，V为反应物质体积。此处反应物质指两种弹体侵彻混凝土靶后形成的Ti-6Al-4V碎片，钛合金弹碎片为边长l=0.1 mm的正方体，而钛合金空心圆柱的碎片为三边长度之比为1∶2∶5的长方体，最短边l1=2.6 mm。因此，式（2）可简化为：gh=17γh5l1 (3) gt=6γtl (4) 式中：下标h和t分别表示钛合金空心圆柱与钛合金弹的参量。可见，两种弹体的供氧或供热效率均与碎片的单位面积供氧或供热效率成正比，与碎片特征尺寸成反比。
由前文分析可知，钛合金弹空心圆柱以塑性变形与裂纹面撕裂为主要生热机制，而钛合金弹除这两种生热机制外，还包括摩擦生热机制。假设钛合金弹与钛合金空心圆柱的塑性变形与裂纹面撕裂两种生热机制的单位供热量相同（即γh=γt），则由于附加了摩擦生热机制，钛合金弹的单位面积供热量大于钛合金空心圆柱的单位面积供热量。这意味着针对供热效率，钛合金弹碎片是钛合金空心圆柱碎片的46倍以上，二者之比由式（4）比式（3）计算得到。类似的，两种弹体在相同供氧环境下，即二者单位表面供氧量相同时，钛合金弹碎片的供氧效率是钛合金空心圆柱碎片的约46倍。因此，钛合金弹形成的百微米尺寸碎片更易与氧气反应。这应是钛合金弹撞击混凝土时发生冲击反应，而钛合金空心圆柱不能激发冲击反应的本质原因。
综上所述，Ti-6Al-4V材料发生冲击反应的必要条件是形成尺寸足够小的碎片，如在本文撞击混凝土的供热与供氧条件下，其冲击反应的碎片尺寸在百微米或更小量级。在特定供氧与供热条件下，存在碎片冲击反应的最大临界尺寸，若碎片尺寸超过该尺寸，无论碎片个数达到多少，均不能发生冲击反应；若碎片尺寸在最大临界尺寸之内，碎片个数越多，冲击反应烈度越高。如本文钛合金弹碎片个数由十万量级上升至百万量级，冲击反应由零星火星向较长时间的持续燃烧转变。
4. 结 论
本文设计并开展了两种结构的Ti-6Al-4V弹体正侵彻混凝土试验，撞击速度在222～1 008 m/s之间。试验发现，钛合金弹存在冲击反应而复合弹无法激发冲击反应。二者破坏模式存在显著差异：钛合金弹侵彻后结构基本完整，仅弹体表面发生摩擦磨损，以细观组织剪切变形为主要失效模式，形成尺寸在10−6至10−4 m量级的颗粒碎片，碎片个数可高达3×106；复合弹的钛合金空心圆柱撕裂成块，撕裂面沿剪切带方向发展，碎片尺寸在毫米或以上量级，碎片个数至多百余个。弹体破坏模式决定了形成的碎片特征。形成足够小的碎片是Ti-6Al-4V材料发生冲击反应的必要条件。特定供氧与供热条件下，存在碎片冲击反应的最大临界尺寸。若碎片尺寸在最大临界尺寸之内，碎片个数越多，冲击反应烈度越高。在本文撞击混凝土的供氧与供热效率下，百微米量级的Ti-6Al-4V碎片可发生冲击反应；该尺寸碎片个数达百万量级时，反应可持续约1 ms。
若确定了冲击加载的供氧和供热效率，可进一步基于化学反应等特征决定材料的冲击反应最大临界尺寸。据此设计结构形式，诱导结构破坏后形成在最大临界尺寸之内的碎片，为冲击反应准备必要条件。此外，可预制在冲击反应最大临界尺寸之内的颗粒混合物，撞击后产生脆性破坏并直接释放颗粒，同样具备冲击反应必要条件。冲击反应的条件与机理研究为多功能含能结构材料的释能应用奠定了基础。
-
图 3 有限元与解析公式计算结果的对比
Figure 3. Comparison between the results by finite element simulation and analytical formula calculation
图 4 厚度梯度型负泊松比蜂窝基座结构
Figure 4. The thickness-graded honeycomb pedestal with negative Poisson’s ratio
图 7 有限元模型及实验基座结构
Figure 7. The finite element model and the corresponding pedestal structure used in test
图 9
θ1 为65°的均匀厚度蜂窝结构von Mises应力云图Figure 9. Von-Mises stress distribution in the layered honeycomb structure with uniform thickness for θ1 = 65°
图 10 胞角
θ1 为65°的蜂窝结构各分层内出现的最大von Mises应力Figure 10. The maximum von-Mises stress in the every layer of the honeycomb structure with θ1 = 65°
图 11 胞角
θ1 为60°的蜂窝结构各分层内出现的最大von Mises应力Figure 11. The maximum von-Mises stress in the every layer of the honeycomb structure with θ1 = 60°
图 12 胞角
θ1 为55°的蜂窝结构各分层内出现的最大von Mises应力Figure 12. The maximum von-Mises stress in the every layer of the honeycomb structure with θ1 = 55°
图 13 各工况下基座的最大von Mises应力
Figure 13. The maximum von Mises stresses in the pedestals under various working conditions
图 15 不同工况下基座面板测点处的加速度时历曲线
Figure 15. Acceleration-time curves at the measuring points of the base panel under different working condition
图 16 不同工况下蜂窝基座面板测点处的冲击谱
Figure 16. Impact spectra at the measuring points of the honeycomb pedestal panels under different working conditions
表 1 厚度梯度基座工况设置
Table 1. Condition settings for thickness gradient pedestals
胞角θ1/(°) 工况 层1厚度/mm 层2厚度/mm 层3厚度/mm 55 均匀厚度 3 3 3 顺厚度梯度 2 3 4 逆厚度梯度 4 3 2 60 均匀厚度 3 3 3 顺厚度梯度 2 3 4 逆厚度梯度 4 3 2 65 均匀厚度 3 3 3 顺厚度梯度 2 3 4 逆厚度梯度 4 3 2 
下载: 导出CSV
-
[1] 王军, 卢立新. 蜂窝纸板面内平台应力表征 [J]. 工程力学, 2012, 29(8): 354–359. DOI: 10.6052/j.issn.1000-4750.2010.11.0816.WANG J, LU L X. Characterization of in-plane platean stress for honeycomb paperboard [J]. Engineering Mechanics, 2012, 29(8): 354–359. DOI: 10.6052/j.issn.1000-4750.2010.11.0816. [2] 张伟. 三维负泊松比多胞结构的轴向压缩性能研究[D]. 大连: 大连理工大学, 2015. [3] CHOI J B, LAKES R S. Analysis of elastic modulus of conventional foams and of re-entrant foam materials with a negative Poisson’s ratio [J]. International Journal of Mechanical Sciences, 1995, 37(1): 51–59. DOI: 10.1016/0020-7403(94)00047-n. [4] LI Y, CORMIER D, WEST H, et al. Non-stochastic Ti-6Al-4V foam structures with negative Poisson’s ratio [J]. Materials Science and Engineering: A, 2012, 558: 579–585. DOI: 10.1016/j.msea.2012.08.053. [5] SCHULTZ J, GRIESE D, SHANKAR P, et al. Optimization of honeycomb cellular meso-structures for high speed impact energy absorption [C] // Proceedings of ASME 2011 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference. Washington: American Society of Mechanical Engineers, 2014: 955-965. DOI: 10.1115/DETC2011-48000. [6] 杨德庆, 马涛, 张梗林. 舰艇新型宏观负泊松比效应蜂窝舷侧防护结构 [J]. 爆炸与冲击, 2015, 35(2): 243–248. DOI: 10.11883/1001-1455(2015)02-0243-06.YANG D Q, MA T, ZHANG G L. A novel auxetic broadside defensive structure for naval ships [J]. Explosion and Shock Waves, 2015, 35(2): 243–248. DOI: 10.11883/1001-1455(2015)02-0243-06. [7] ZHANG X C, AN L Q, DING H M, et al. Influence of cell micro-structure on the in-plane dynamic crushing of honeycombs with negative Poisson’s ratio [J]. Journal of Sandwich Structures and Materials, 2015, 17(1): 26–55. DOI: 10.1177/1099636214554180. [8] KOIZUMI M. FGM activities in Japan [J]. Composites Part B: Engineering, 1997, 28(1−2): 1–4. [9] 何强, 马大为, 张震东, 等. 功能梯度蜂窝材料的面内冲击性能研究 [J]. 工程力学, 2016, 33(2): 172–178. DOI: 10.6052/j.issn.1000-4750.2014.05.0392.HE Q, MA D W, ZHANG Z D, et al. Research on the in-plane dynamic crushing of functionally graded honeycombs [J]. Engineering Mechanics, 2016, 33(2): 172–178. DOI: 10.6052/j.issn.1000-4750.2014.05.0392. [10] 吴鹤翔, 刘颖. 梯度分布对密度梯度金属空心球阵列动力学性能的影响 [J]. 工程力学, 2013, 30(1): 425–431. DOI: 10.6052/j.issn.1000-4750.2011.06.0335.WU H X, LIU Y. The influence of gradient profile on the dynamic properties of density graded metal hollow sphere arrays [J]. Engineering Mechanics, 2013, 30(1): 425–431. DOI: 10.6052/j.issn.1000-4750.2011.06.0335. [11] AJDARI A, CANAVAN P, NAYEB-HASHEMI H, et al. Mechanical properties of functionally graded 2-D cellular structures: a finite element simulation [J]. Materials Science and Engineering: A, 2009, 499(1−2): 434–439. DOI: 10.1016/j.msea.2008.08.040. [12] GUPTA N. A functionally graded syntactic foam material for high energy absorption under compression [J]. Materials Letters, 2007, 61(4−5): 979–982. DOI: 10.1016/j.matlet.2006.06.033. [13] LIANG C, KIERNAN S, GILCHRIST M D. Designing the energy absorption capacity of functionally graded foam materials [J]. Materials Science and Engineering: A, 2009, 507(1−2): 215–225. DOI: 10.1016/j.msea.2008.12.011. [14] 李营, 汪玉, 吴卫国, 等. 船用907A钢的动态力学性能和本构关系 [J]. 哈尔滨工程大学学报, 2015, 36(1): 127–129. DOI: 10.3969/j.issn.1006-7043.201311093.LI Y, WANG Y, WU W G, et al. Dynamic mechanical properties and constitutive relations of marine 907A steel [J]. Journal of Harbin Engineering University, 2015, 36(1): 127–129. DOI: 10.3969/j.issn.1006-7043.201311093. [15] 王军. 浮动冲击平台冲击动力特性研究[D]. 哈尔滨: 哈尔滨工程大学, 2015. [16] German Federal Office for Military Technology and Procurement. Shock resistance specification for bundeswehr ships: BV043[S]. 1985. [17] 卢子兴, 李康. 四边手性蜂窝动态压溃行为的数值模拟 [J]. 爆炸与冲击, 2014, 34(2): 181–187. DOI: 10.11883/1001-1455(2014)02-0181-07.LU Z X, LI K. Numerical simulation on dynamic crushing behaviors of tetrachiral honeycombs [J]. Explosion and Shock Waves, 2014, 34(2): 181–187. DOI: 10.11883/1001-1455(2014)02-0181-07. [18] 王军, 姚熊亮, 杨棣. 浮动冲击平台冲击环境对设备响应的影响 [J]. 爆炸与冲击, 2015, 35(2): 236–242. DOI: 10.11883/1001-1455(2015)02-0236-07.WANG J, YAO X L, YANG D. Impact analysis of shock environment from floating shock platform on equipment response [J]. Explosion and Shock Waves, 2015, 35(2): 236–242. DOI: 10.11883/1001-1455(2015)02-0236-07. -
下载:
计量
- 文章访问数: 5005
- HTML全文浏览量: 1768
- PDF下载量: 89
- 被引次数: 0