A study of parameters of Kong-Fang fluid elastoplastic damage material model for Shandong granite
-
摘要: 岩石类材料的动态力学模型的建立及相应模型参数的确定,对岩石动态力学性能研究及相关仿真计算具有重要意义。以山东五莲地区花岗岩为例,基于Kong-Fang流体弹塑性损伤材料模型(KF模型),通过准静态单轴压缩、劈裂、常规三轴实验及动态分离式霍普金森杆压缩(split Hopkinson pressure bar,SHPB)实验对模型中的强度参数进行了确定,并利用基于分离式霍普金森杆的巴西圆盘(split Hopkinson pressure bar-Brazilian disk,SHPB-BD)实验对应变率相关参数的有效性进行了验证;同时,根据平板撞击实验结果对模型中的状态方程参数进行了拟合。利用实验获得的材料参数值,采用KF模型对花岗岩侵彻实验进行数值模拟,计算得到的弹体侵彻深度及成坑尺寸与实际实验结果误差均小于15%,验证了材料模型及参数值的适用性。
-
关键词:
- Kong-Fang模型 /
- 准静态实验 /
- 动态实验 /
- 平板撞击 /
- 侵彻实验
Abstract: The establishment of the dynamic mechanical model of rock materials and the determination of the relevant model parameters are of great significance to the studies of rock’s dynamic mechanical properties and related simulation calculation. Taking granite in Shandong Province as the experimental object, based on the Kong-Fang fluid elastic-plastic damage material model (KF model), the model parameters are classified into three categories, and the test scheme is then correspondingly determined. The basic strength parameters of the KF model were measured by quasi-static uniaxial compression and unconfined splitting tests. The strength-surface related material parameters were fitted by the results of the conventional triaxial tests under five different confining pressure conditions. In addition, the dynamic split Hopkinson pressure bar (SHPB) tests under several strain rate conditions were carried out to determine the strain-rate related parameters, of which the effectiveness were then verified by the dynamic split Hopkinson pressure bar-Brazilian disk (SHPB-BD) tests results. According to the principle of reverse impact and the Rankine-Hugoniot equation, the plate impact experiments with different impact stress levels were conducted by using a single-stage light gas gun, the state equation parameters in the KF model were fitted according to the impact Hugoniot results of rock samples. To verify the applicability of the material model and the experimentally measured parameter values, the simulation of a penetration process is furtherly conducted. The granite penetration tests were carried out by using a∅ 30 mm caliber gun. The∅ 20 mm bullets penetrated the∅ 1200 mm×800 mm rock targets vertically, which was used to characterize the semi-infinite thickness condition, at an approximately designed speed of 670 m/s. To avoid accidental errors, combined with the high-speed photographic images, three effective penetrate results were obtained. The penetration depth and crater size of the target failure surface were directly measured and scanned by 3D scanner, the experimental average penetration depth, maximum and minimum diameters of the penetration craters were approximately 80.62 mm, 381.47 mm and 263.01 mm, respectively. Using the parameter values obtained from the laboratory experiments, the KF model is then implemented into LS-DYNA through a user-defined material model and used to simulate the penetration test of granite. According to the simulation result of damage distribution and cratering parameters of the target, the calculated penetration depth, maximum and minimum diameters of craters are 80.02 mm, 400 mm and 300 mm, respectively, so the errors between the calculated and the test results are less than 15%, which is acceptable in dynamic problems. The agreement between the numerical and experimental results provides a support to the application of the KF model and the relevant parameter values.-
Key words:
- Kong-Fang model /
- quasi-static experiment /
- dynamic experiment /
- plate impact /
- penetration experiment
-
预测岩石类材料在动态条件下的力学响应及破坏行为一直是数值计算中的重难点,建立合适的动态力学模型并选取适当的材料模型参数十分重要。目前常用的岩石类材料动态模型包括:Holmquist-Johnson-Cook模型(HJC模型)[1]、Karagozian-and-Case模型(K&C模型)[2]、Riedel-Hiermaier-Thoma模型(RHT模型)[3]、Johnson-Holmquist 模型(JH-2 模型)[4]、Talyor-Chen-Kuszmaul 模型(TCK 模型)[5]等,并被应用于如冲击、爆炸、侵彻等多种动态过程的仿真分析中[6-8]。研究表明,这些模型在运用时并不都能得到较理想的效果[9-10],原因在于模型本身存在一定的不足,无法很好地反映岩石材料的某些特征[11-12]。一些学者对现有模型进行了部分修正,并将改进后的模型应用于相关的动态分析中,获得了较好的结果 [10, 13-16]。但建立更为合理的、完善的岩石类材料动态力学模型仍然十分必要。
Kong等[11]基于塑性损伤理论建立了一种新的混凝土动态力学模型(Kong-Fang流体弹塑性损伤材料模型,KF模型),该模型定义了基于3个应力不变量的屈服面,并对压缩和拉伸损伤计算进行了区分。模型中引入了部分关联流动法则,可以较好地描述混凝土材料的剪胀行为。通过多种单元数值试验以及爆炸、冲击等动力学实例的模拟分析,对比HJC、K&C及RHT模型的模拟结果,验证了KF模型的适用性。Huang等[17]进一步对KF模型进行了修正以适用于岩石材料,他们利用大量硬岩实验数据标定了模型中的部分参数,同时采用简明的半经验公式来描述动态增长因子随应变率的变化规律。通过多单元拉压数值试验及岩石劈裂、冲击实例的仿真计算,验证了修正模型对岩石的动态响应具有较好的预测能力。
考虑到天然岩石的力学性质差异较大,本文中将以山东五莲地区的花岗岩对研究对象,采用KF模型,根据模型参数定义,一方面,通过准静态单轴压缩、劈裂、常规三轴及动态分离式霍普金森杆压缩实验对模型中的强度参数进行确定,同时利用动态巴西圆盘实验结果验证应变率相关参数的有效性。另一方面,通过平板撞击实验拟合模型中的状态方程参数;最后结合实测参数值,利用KF模型对花岗岩侵彻试验过程进行仿真,通过对比计算得到的靶体侵彻深度和弹坑尺寸与实际试验的结果,验证材料模型及参数值的适用性。
1. KF模型简介及参数分类
1.1 KF模型简介
KF模型是一种可以描述岩石类材料在高压和高应变率条件下动态响应的计算本构模型[11]。模型主要对屈服面、应变率效应和损伤累积进行了定义,此外,还采用了部分关联流动法则和多项式状态方程来分别描述岩石在低压压缩下的剪胀性和高压压缩下的变形特征。
1.1.1 基于三个应力不变量的屈服面
KF模型采用了压缩子午线的最大强度面σm和残余强度面σr,其表达式分别为[11]:
σm={3[p/(1−D)+T]p≤01.5(p+T)/ψ0<p<fc/3fc+(p−fc/3)/(a1+a2p)p≥fc/3 (1) σr=p/(a1+a2p) (2) 式中:fc和T分别为无侧限单轴压缩强度和拉伸强度;p为静水压力;D为总损伤;a1、a2为与强度面相关的材料常数;ψ为拉压子午线的比值,表示与压力相关的函数。最初,KF模型中定义ψ为p的分段函数,具体根据混凝土的三轴压缩和拉伸实验数据确定[14],后来Huang等[17]考虑到硬岩的压缩性质与混凝土存在差异,对ψ进行了校准以适用于硬岩。
当材料并未达到最大强度面σm或残余强度面σr时,当前破坏面Y则依据当前岩石损伤情况对σm和σr进行插值确定[11]:
Y(σij,D)=√3J2=r′[D(σr−σm)+σm] (3) 式中:J2为偏应力第二不变量;r′为当前子午线与压缩子午线的比值,其值可通过ψ(p)与Lode角计算获得[11],函数形式与RHT模型中的参数R3(θ)(θ为Lode角)计算公式一致。
1.1.2 拉压分离的应变率效应
岩石材料动态强度具有明显的率效应[18],因此KF模型将当前破坏面Y进一步改进为:
Y=ηY(p/η) (4) 式中:η为动态增长因子,其值为岩石动态强度与准静态强度的比值。
考虑到拉压条件下岩石将表现出不同的应变率效应,KF模型中分别定义了压缩强度动态增长因子ηc与拉伸强度动态增长因子ηt,由于原始计算公式较复杂,文献[17]中采用一种半经验的简洁公式对其进行了改进:
ηc=1.0+αeβ(lg˙ε−θ) (5) ηt=1.0+(ηc−1)fc/fcTT (6) 式中:α、β、θ为材料参数,
˙ε 为应变率。1.1.3 状态方程
当压力较高时,岩石材料的强度和结构的影响一般可以忽略[19],此时仅需考虑材料的体积变形,因此模型采用了单独的多项式状态方程来表征岩石在高压条件下的体积压缩行为:
p=k1μ+k2μ2+k3μ3 (7) 式中:k1、k2和k3为状态方程材料参数,μ为体应变。
此外,模型中定义了材料损伤、变形及单元删除。(1)考虑到岩石材料在拉压荷载下的破坏机理不同,分别定义了拉伸损伤Dt和压缩损伤Dc,并根据Dt和Dc计算了岩石材料的总损伤D。在计算损伤时共定义了6个损伤累积相关参数,对于硬岩,文献[17]中给出了这类参数的建议值:d1=0.04、d2=2.3、γ=1、c1=3、c2=6.93、εfrac=0.0015,因此,在本研究中也将这类参数作为常数处理。(2)为了表征岩石在低围压压缩荷载下的剪胀性,基于K&C模型中的塑性势函数,引入了部分关联的塑性流动法则,得到了相应的破坏面与应力更新计算公式。(3)模型中还采纳了两种单元删除准则,分别是基于损伤的拉伸删除准则和基于等效塑性应变的压缩删除准则,以表征岩石在遭受如爆炸等动载荷时材料前端和后端出现的成坑和剥落等破坏特征。由于损伤累积部分的参数为定值,而部分关联塑性流动法则与单元删除准则中没有涉及新的参数,因此本文中不再介绍。
1.2 模型参数分类及确定方法
为了获得待研究花岗岩材料的KF模型参数值,需确定各参数的实验方法。首先,根据模型定义可将待确定参数分为材料基本强度参数、强度相关参数和状态方程相关参数,见表1。材料基本强度参数fc、E和ν的确定可通过无侧限单轴压缩实验进行;对于参数T的确定,由于直接拉伸实验费用高、成功率较低,而巴西劈裂实验制样方便、操作简单、成功率高[20],因此将采用劈裂实验获得的材料劈裂抗拉强度ft来代替参数T;强度面相关的材料常数a1、a2的确定则需要大量三轴压缩实验数据;由式(5)~(6)可知,应变率相关的参数α、β、θ可通过SHPB实验确定,同时可利用SHPB-BD实验结果对拟合参数值的有效性进行验证;对状态方程相关参数的确定,可先通过平板撞击实验获得岩石的冲击雨果纽数据,然后拟合材料的压力-体应变关系曲线(p-μ曲线)获得。
表 1 KF模型参数的分类及实验确定方法Table 1. Parameter classification and experimental determination method of KF model分类 参数 物理意义 确定方法 材料基本强度参数 ft 劈裂拉伸强度 准静态劈裂实验 fc 单轴压缩强度 准静态单轴压缩实验 E 弹性模量 υ 泊松比 强度面相关参数 a1、a2 强度面相关材料常数 准静态常规三轴实验 α、β、θ 应变率相关材料参数 SHPB/ SHPB-BD实验 状态方程相关参数 k1、k2、k3 状态方程参数 平板撞击实验 2. 准静态实验及相关参数确定
2.1 实验样品
选用产自山东五莲地区的粗中粒角闪二长花岗岩,岩石为二长半自形粒状结构,块状构造,主要矿物为斜长石(Pl)、钾长石(Kfs)、石英(Qtz),共占矿物总量的90%。次要矿物为角闪石(Hbl)、黑云母(Bt)、辉石(Px);蚀变及充填矿物为绢云母、绿帘石。岩石的实物照片和显微图像如图1所示。
根据相关的实验规范[21]及实验机对试件的要求,制作各准静态实验的岩石试样:无侧限单轴压缩及常规三轴实验的试件为直径50 mm、高100 mm的标准圆柱形;劈裂实验试件为直径50 mm、高25 mm的圆盘。为了保证实验结果的可靠性,每种实验均开展了重复实验。
2.2 准静态单轴压缩及劈裂实验
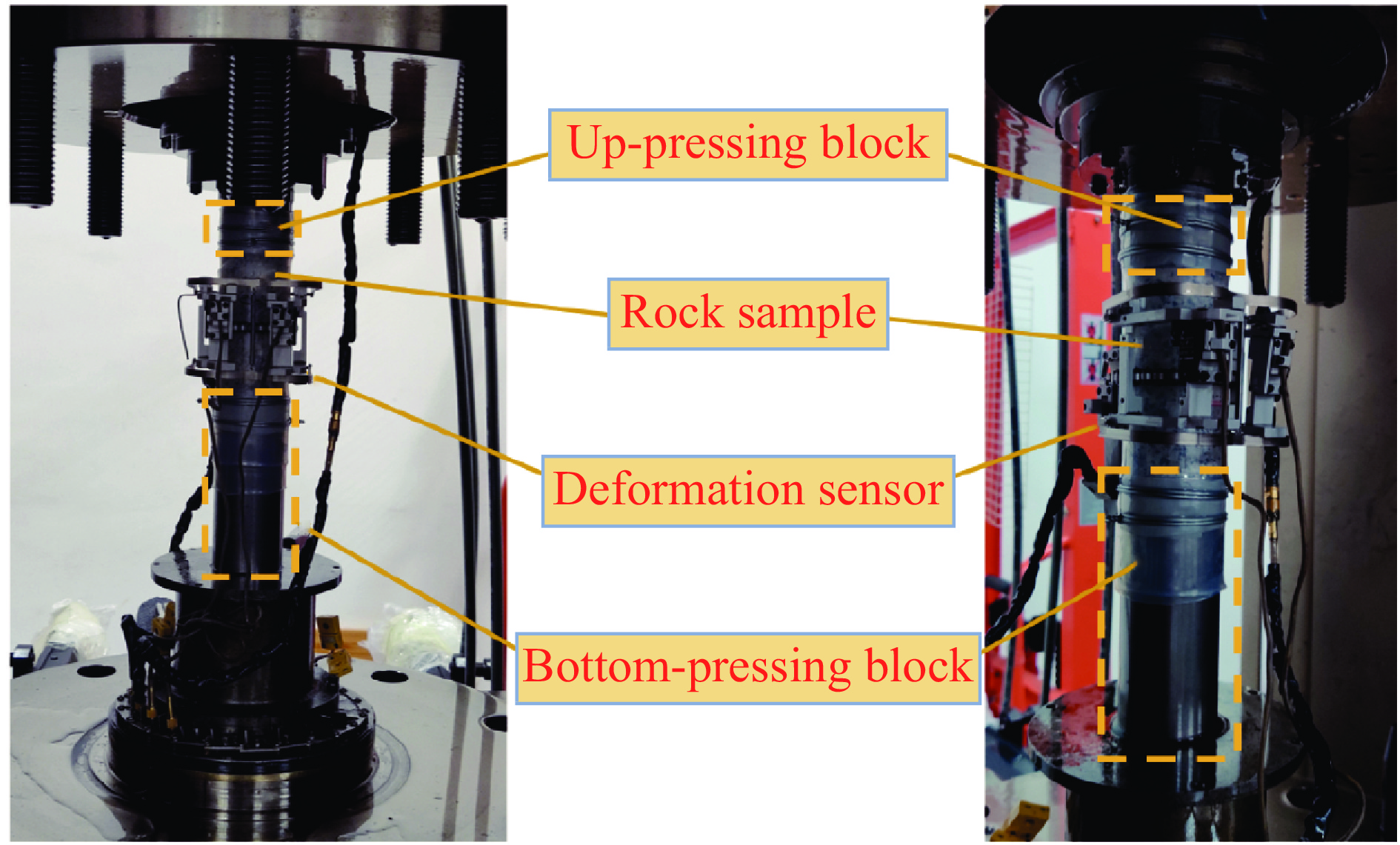
分别利用TAW2000岩石三轴伺服压缩机及WDW-500E微机控制电子式万能试验机对花岗岩试件进行准静态单轴压缩和劈裂实验,如图2所示。实验中均采用变形控制加载,设置变形速率分别为0.06、0.03 mm/min,使得实验过程中试件的应变率为10−5 s−1。
对单轴压缩实验获得的轴向压缩应力-应变曲线进行求导,其中弹性段导数即为试件的弹性模量E,同时采用fc /2处的环向应变和轴向应变来计算各试件的泊松比υ[22]。结合各试件的单轴抗压强度值fc及劈裂拉伸强度ft,利用两倍标准差法剔除异常值,并计算剩余有效数据的平均值作为花岗岩试件的KF模型参数值,结果见表2。
表 2 花岗岩的基本强度参数值Table 2. Basic strength parameters of granite试件 fc /MPa E/GPa υ 试件 ft /MPa UCT-1 125.088 38.076 0.310 ST-1 7.714 UCT-2 133.206 41.82 0.352 ST-2 6.9 UCT-3 128.969 38.843 0.318 ST-3 8.12 UCT-4 133.802 39.534 0.498 ST-4 6.887 UCT-5 133.772 40.759 0.259 ST-5 7.409 KF模型参数值 130.967±3.856 39.806±1.497 0.347±0.091 KF模型参数值 7.406±0.532 2.3 准静态常规三轴实验
利用MTS815型电液伺服岩石力学试验机对花岗岩试件进行准静态常规三轴实验,为了满足参数确定需求,设置了5种不同的围压水平:10、20、30、40、50 MPa。其中10、20 MPa工况各进行1次实验,30、40、50 MPa工况重复3次实验。如图3所示,将试件置于实验平台上,先施加一定的轴向压力防止试件滑动,然后再以1~3 MPa/min的加载速度施加围压至设定值。保持围压约5~10 min,确定不漏油后,以0.06 mm/min的加载速度施加轴压,直至试件破坏。
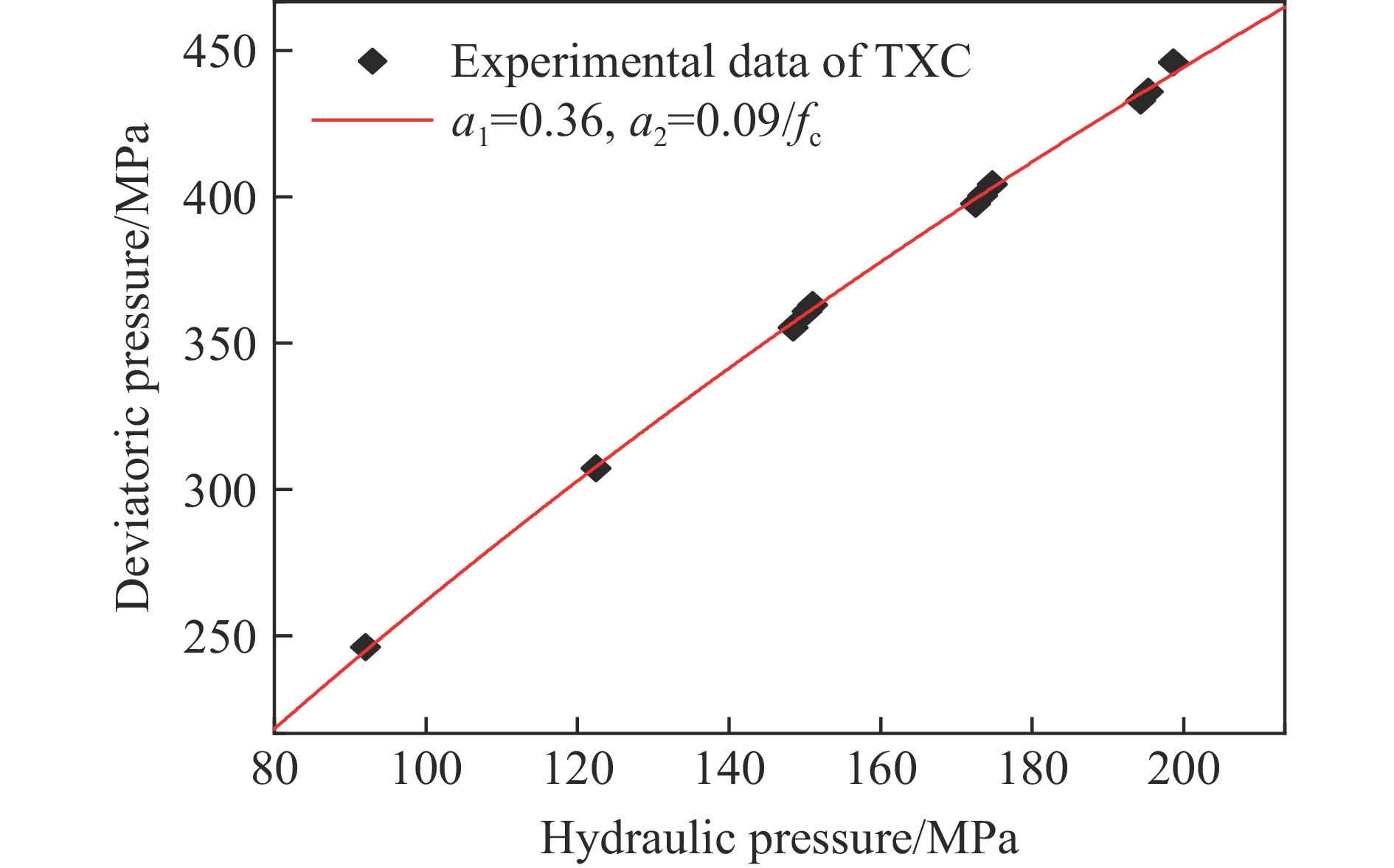
花岗岩试件的常规三轴压缩应力-应变曲线如图4所示。由图4可知,围压实验的重复性较好,岩石的峰值强度随围压的增加而增加。由式(1)可知,确定参数a1、a2只需利用强度面应力值。因此,利用各试件的围压及相应的轴向峰值强度分别计算出各工况下的偏压力(σD=σ1−σ2)和静水压力(p=1/(3(σ1+2σ2))),结果见表3。进一步对a1、a2进行拟合,获得如图5所示的参数拟合值:a1=0.36,a2=0.09/fc。
表 3 不同围压条件下试件的轴向峰值强度、偏压力与静水压力Table 3. The strength, deviatoric pressure and hydrostatic pressure of rock samples under different confining pressures试件 围压/MPa 轴向峰值强度/MPa 静水压力/MPa 偏应力/MPa 10-1 10 256.220 92.073 246.220 20-1 20 327.341 122.447 307.341 30-1 30 385.384 148.461 355.384 30-2 393.095 151.032 363.095 30-3 390.809 150.270 360.809 40-1 40 437.691 172.564 397.691 40-2 440.422 173.474 400.422 40-3 444.189 174.730 404.189 50-1 50 482.936 194.312 432.936 50-2 485.975 195.325 435.975 50-3 495.950 198.650 445.950 3. 动态实验及相关参数确定
3.1 SHPB及SHPB-BD实验
根据相关的实验标准[23-24] ,基于50 mm杆径的分离式霍普金森杆实验系统开展SHPB及SHPB-BD实验。入射杆、透射杆分别长3.0、1.8 m,材料均为马氏体钢,两种实验采用的试件均为
∅ 38 mm×19 mm的圆盘形试样。经过力平衡校验后,计算各有效实验的试件应变率˙ε 、应变ε及应力σ:˙ε = c0(εi−εr−εt)/ls (8) ε = (c0/ls)∫t0(εi−εr−εt)dt (9) σc = A0E0(εi+εr+εt)/(2As) (10) σt = A0E0(εi+εr+εt)/(πDsB) (11) 式中:c0为实验杆的一维弹性波速,εi 、εr 、εt 分别为入射应变、反射应变和透射应变,ls为试样长度,σc为SHPB实验中试样的压缩应力,σt为SHPB-BD实验中试样的拉伸应力,E0为杆的弹性模量,A0为杆的横截面积,As为试样的横截面积,Ds为试样直径,B为试样厚度。
为了确定并检验KF模型中的应变率相关参数α、β、θ,分别进行了14次动态压缩实验(剔除误差较大的一组数据)和15次动态劈裂实验,试件平均应变率为83~360 s−1(动态压缩)和133~245 s−1(动态劈裂)。实验所得的应力-应变曲线如图6所示,从图中可以发现,花岗岩具有一定的应变率效应。提取各实验中平均应变率及峰值强度并计算相应的ηc值与ηt值,结果见表4。利用表4中数据,结合式(5)~(6)对α、β、θ进行拟合及验证。图7中展示了拟合得到的参数值:α=0.06、β=3.47、θ=1.83。图8为利用SHPB-BD实验数据对参数进行验证的结果,由图8可知,拟合得到的参数值有较好的适用性。
表 4 SHPB、SHPB-BD实验的平均应变率及相应的ηc 、ηt 值Table 4. Average strain rates and correspondingηc andηt values obtained from SHPB and SHPB-BD experiments试件 动态压缩强度/MPa ηc 平均应变率/s−1 试件 动态劈裂拉伸强度/MPa ηt 平均应变率/s−1 SHPB-1 159.324 1.217 83 SHPB-BD-1 41.395 5.589 138 SHPB-2 142.925 1.091 105 SHPB-BD-2 37.363 5.045 135 SHPB-3 160.994 1.229 102 SHPB-BD-3 45.456 6.138 133 SHPB-4 156.455 1.195 85 SHPB-BD-4 45.548 6.150 135 SHPB-5 164.434 1.256 122 SHPB-BD-5 52.404 7.076 187 SHPB-6 180.403 1.377 144 SHPB-BD-6 52.654 7.110 183 SHPB-7 145.891 1.114 168 SHPB-BD-7 49.934 6.742 187 SHPB-8 140.933 1.076 187 SHPB-BD-8 49.225 6.647 188 SHPB-9 212.790 1.625 230 SHPB-BD-9 65.320 8.820 241 SHPB-10 260.696 1.991 325 SHPB-BD-10 54.190 7.317 245 SHPB-11 158.481 1.210 258 SHPB-BD-11 70.300 9.492 239 SHPB-12 177.977 1.359 292 SHPB-BD-12 60.436 8.160 243 SHPB-13 215.694 1.647 360 SHPB-BD-13 48.513 6.550 155 SHPB-BD-14 61.372 8.287 199 SHPB-BD-15 71.112 9.602 210 3.2 平板撞击实验
为了获得更干净稳定的信号,基于57 mm口径一级轻气炮,采用反向撞击法开展实验[25]。考虑到获取信号不能受到追赶稀疏波及边侧稀疏波的影响,飞片与靶板尺寸均设计为
∅ 50 mm×10 mm,其中靶体材料为无氧铜。具体的实验原理为:当岩石飞片撞击无氧铜靶时,利用电探针测得飞片的速度w,利用DISAR(displacement interferometer system for any reflector)系统测得靶板背面的自由面质点速度up,Cu。根据应力波原理,结合冲击绝热线方程及Rankine-Hugoniot方程可知,岩石材料的波后质点速度up,rock、波后压力prock、岩石中的冲击波速度us,rock及岩石的体应变μrock [26]分别为:up,rock=w−0.5up,Cu (12) prock = 0.5up,Cuρ0,Cu(c0,Cu+0.5up,CusCu) (13) us,rock = prock/(ρ0,rockup,rock) (14) μrock = up,rock/us,rock (15) 式中:ρ0,Cu为铜的初始密度,ρ0,Cu=8930 kg/m3;c0,Cu、sCu为铜的材料参数, c0,Cu=3940 m/s,sCu=1.49[27];ρ0,rock为花岗岩的初始密度,其值通过密度实验获得:ρ0,rock=2686 kg/m3。
实验时准备并安装好弹丸、电探针、靶板和DISAR系统。利用压缩空气(设计冲击速度小于400 m/s)或压缩氮气(设计冲击速度大于400 m/s)推动弹丸高速撞击靶板。为了拟合花岗岩的prock-μrock曲线,共设计了4种撞击力水平,并各重复3次实验。由式(12)~(15)获得各状态量的结果,见表5。基于实验数据拟合花岗岩试件的prock-μrock曲线,其形式如式(7),拟合结果如图9所示,拟合得到的prock-μrock方程为:
表 5 花岗岩平板撞击实验结果Table 5. Plate impact test results of granite samples实验 w/ (m∙s−1) up,Cu (m∙s−1) up,rock /(m∙s−1) prock /GPa us,rock/ (m∙s−1) μrock G-1 298.249 170.887 212.806 3.103 5430.281 0.039 G-2 298.586 171.614 212.779 3.117 5454.783 0.039 G-3 296.200 175.154 208.623 3.183 5681.906 0.037 G-4 364.353 210.768 258.969 3.856 5543.886 0.047 G-5 362.860 210.444 257.638 3.849 5563.631 0.046 G-6 370.399 217.718 261.540 3.988 5677.582 0.046 G-7 420.639 247.273 297.002 4.553 5708.849 0.052 G-8 425.834 247.173 302.248 4.552 5607.388 0.054 G-9 424.120 246.701 300.769 4.542 5623.706 0.053 G-10 536.010 308.458 381.771 5.743 5601.403 0.068 G-11 527.022 302.785 375.608 5.632 5582.955 0.067 G-12 536.027 312.243 370.879 5.817 5840.622 0.063 prock=45.002μrock+1413.751μrock2−12037.857μrock3 (16) 式中:prock的单位为GPa。因此状态方程相关参数k1、k2、k3的值分别为:k1=45.002,k2=1413.751,k3= −12037.857。
综上所述,花岗岩的KF模型参数已被确定,见表6。
表 6 花岗岩KF模型参数拟合结果Table 6. Fitting results of KF model parameters of granite强度参数 状态方程参数 fc/MPa ft/MPa E/GPa a1 a2/MPa −1 υ α β θ k1/GPa k2/GPa k3/GPa 130.967 7.406 39.806 0.36 0.09/fc 0.347 0.06 3.47 1.83 45.002 1413.751 −12037.857 4. 花岗岩侵彻实验及仿真验证
为了验证实验确定的花岗岩KF模型参数值适用性,开展了花岗岩侵彻实验,并利用代入了实测参数值的KF模型对侵彻实验进行模拟,进一步对比仿真与实验得到的最终侵彻深度与成坑结果。
4.1 侵彻实验
花岗岩侵彻实验基于
∅ 30 mm口径滑膛火炮开展,实验原理是利用火药气体燃烧产生的能量推动弹体侵彻岩石靶体。设计工况为子弹以约670 m/s的速度侵彻半无限厚靶体。考虑到弹体发射的稳定性和岩石靶体取样大小的可操作性,本次实验采用次口径发射技术,利用直径20 mm、CRH为2.5的尖卵形头部杆形弹,外置直径30 mm的三瓣式弹托进行实验,弹体材料为超高强度合金钢30CrMnSiNi2A,力学性能指标及Johnson-Cook本构参数[28]见表7。为了模拟半无限条件,需要合理设计靶体直径以忽略边界效应。当侵彻速度为670 m/s时,由已有的研究成果可知[29],若靶弹径比不小于30,则可以忽略有限边界效应。将满足需求的600 mm×600 mm×800 mm的花岗岩块放置在
∅ 1200 mm×800 mm的钢套筒正中位置,并在空隙处浇筑C40混凝土振捣填实养护28 d制成靶体。实验系统现场布局如图10所示,整个系统主要包括3部分:发射平台、测速系统和高速摄影系统。其中发射平台为口径30 mm的滑膛火炮;测速系统用于获取子弹的侵彻速度,方法是通过在炮口和目标靶之间设置两道已知距离的测速靶纸,当弹体击穿靶纸时将分别输出2个电信号至测速仪,根据信号的时间差即可获得弹体的平均飞行速度。根据测得的飞行速度可进一步调整火药的填装量,以实现设计的发射初速。高速摄像系统用于观察子弹的脱壳情况,并判断弹体入射姿态是否满足垂直入射要求。
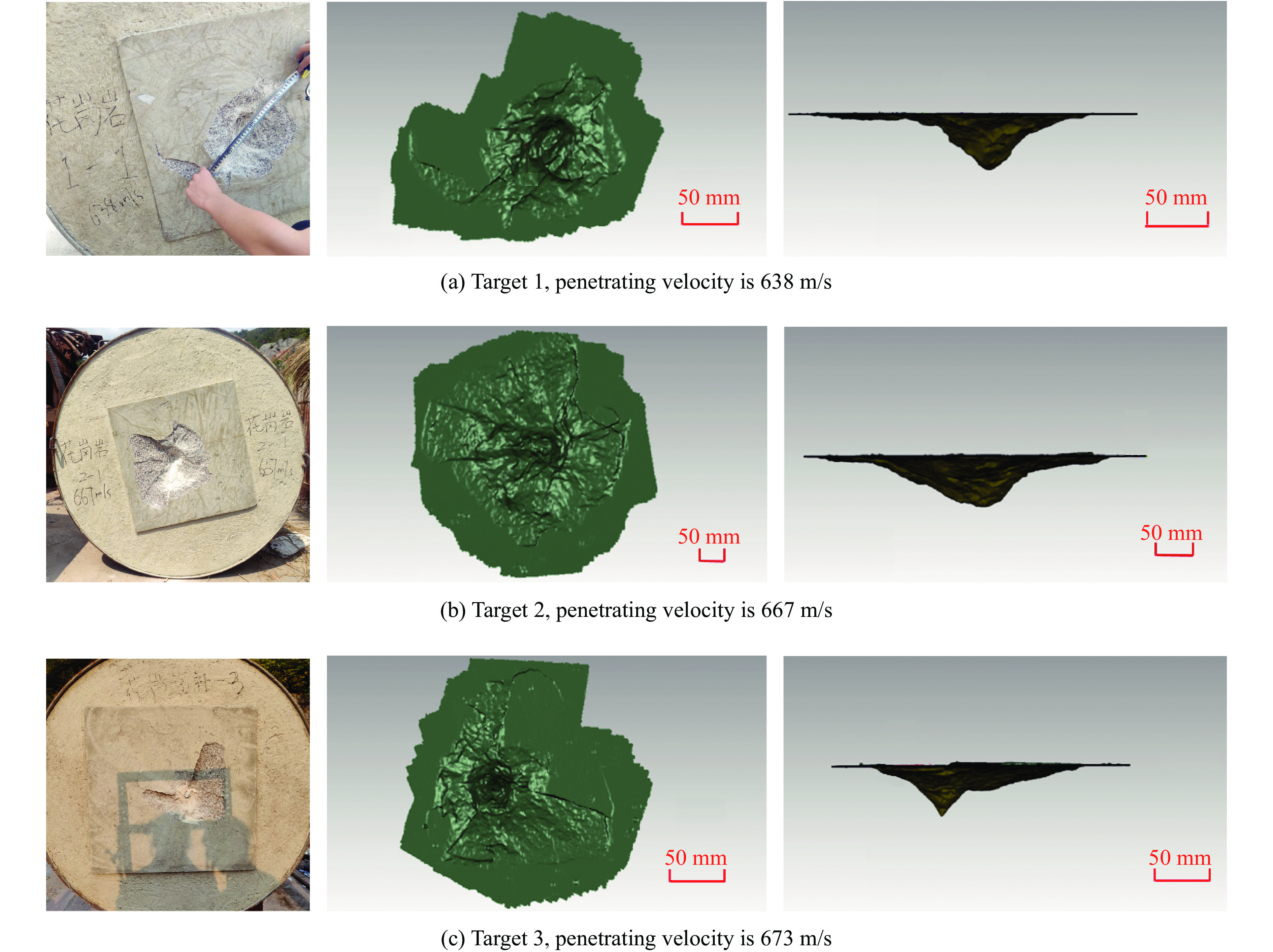
进行多次侵彻实验,根据高速摄影图片选取子弹侵彻着角小于5°的有效侵彻结果,图11为3个有效侵彻实验的弹坑照片与三维扫描结果。利用直尺和三维扫描仪对花岗岩侵彻后的靶体进行现场测量和光学扫描,测量及扫描得到的靶体侵彻深度及成坑参数见表8。
表 8 花岗岩靶成坑参数现场测量及三维扫描结果Table 8. Direct measurement and 3D scanning results of crater parameters of granite targets试验 子弹着角/(°) 侵彻速度/(m∙s−1) 侵彻深度/mm 侵彻弹坑最大直径/mm 侵彻弹坑最小直径/mm 现场测量 三维扫描 现场测量 三维扫描 现场测量 三维扫描 靶1 −3.5 638 75.05 76.29 310 327.98 245 248.36 靶2 −3.5 667 72.19 72.48 430 408.24 275 283.00 靶3 −1.5 673 96.00 91.73 410 402.60 265 261.67 4.2 仿真与实验结果对比
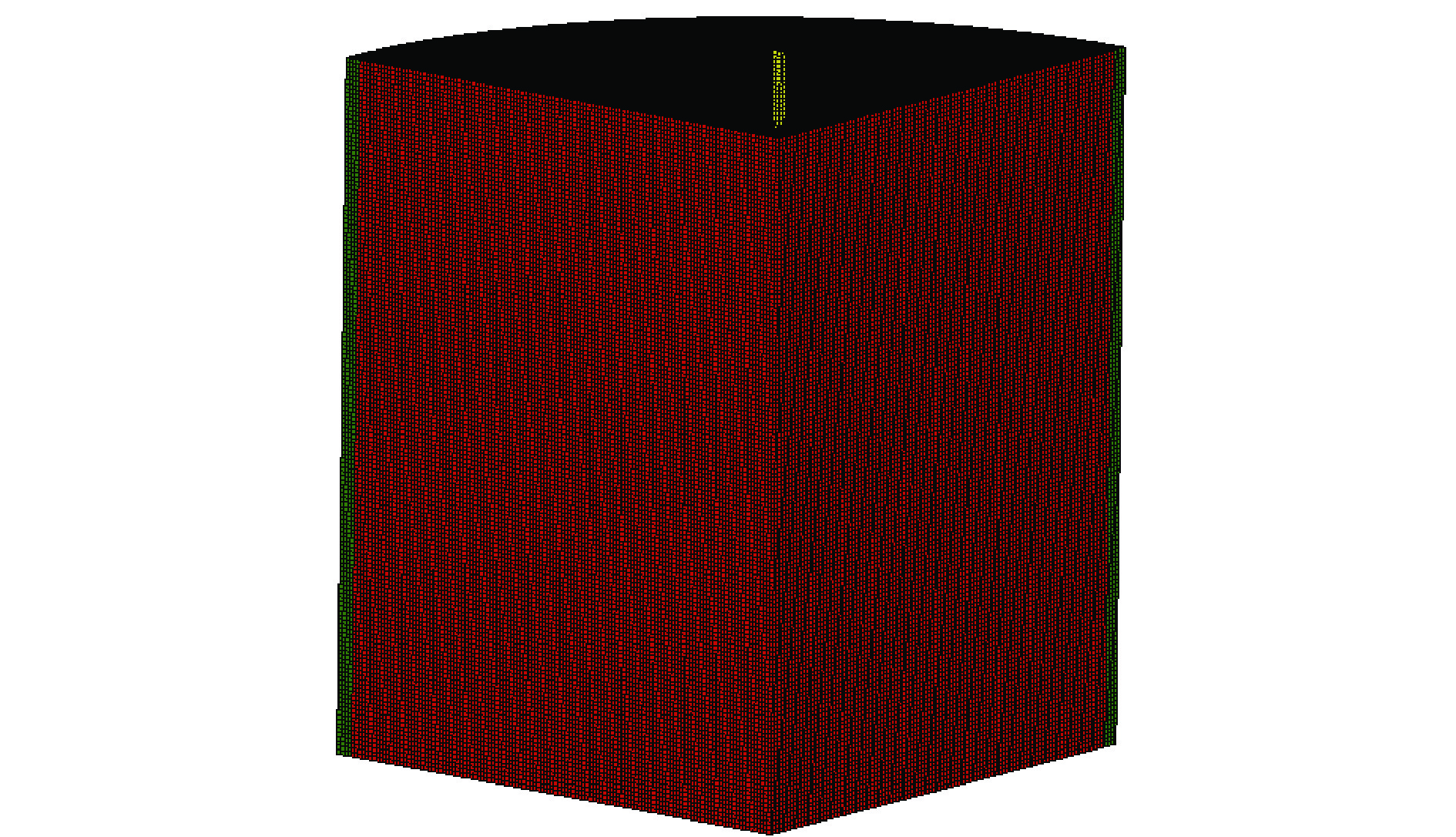
利用LS-DYNA对花岗岩侵彻实验进行仿真。考虑到实验的对称性,同时,为了减少计算成本,采用1/4对称模型进行仿真实验,网格设计如图12所示,将花岗岩靶体简化成了外套一圈薄层铁箍的
∅ 1200 mm×800 mm的圆柱体,弹体则按实验中实用子弹进行建模,模型整体尺寸与实际实验中弹靶尺寸一致。由于3组实验测得的子弹侵彻速度接近,因此模拟时设置弹体在靶体中心处以670 m/s的初速度垂直向下侵彻,弹体与靶体均采用实体单元网格,网格尺寸均设置为1 mm×1 mm×1 mm(弹头处网格做过渡处理)。靶体上表面设置为自由边界,下表面设置为无反射边界,靶体对称面上施加了对称边界。弹体和靶体间的接触采用*CONTACT_ERODING_SURFACE_TO_SURFACE接触算法定义。通过二次开发将KF模型嵌入LS-DYNA软件中,并作为靶体的材料模型,模型参数见表6。使用Johnson-Cook模型作为弹体的材料模型,具体参数见表7。
提取靶体单元的损伤图像并进行对称处理,得到的靶体损伤分布如图13所示。当损伤值为零时,表示单元未损伤,当损伤值为1时,表示单元完全损伤。由图13可知,计算得到的弹坑最大直径为400 mm,最小直径为300 mm。提取侵彻过程中子弹侵彻深度及加速度的历史曲线,如图14所示,可以发现,侵彻深度达到约80 mm时即保持不变,同时弹体的加速度时程曲线也呈现典型的三段式。
由于侵彻实验随机性较大,因此取成坑参数的平均值进行分析,结果见表9。对比实验和数值模拟得到的侵彻弹坑和侵彻深度发现,对于侵彻深度和弹坑最大直径,数值模拟与实验结果的误差小于5%;侵彻弹坑最小直径的模拟结果与实际结果误差较大,但仍小于15%。综上所述,采用KF材料模型,并利用实验获取的参数值得到的数值模拟结果与实验结果吻合较好,验证了材料模型及实测参数值具有较好的适用性。
表 9 实验和数值模拟得到的花岗岩靶侵彻结果比较Table 9. Comparison of penetration results obtained from penetration test and numerical simulation方法 侵彻深度/mm 侵彻弹坑最大直径/mm 侵彻弹坑最小直径/mm 实验 80.62±10.46 381.47±49.60 263.01±14.75 数值模拟 80.02 400.00 300.00 误差/% −0.75 4.86 14.07 5. 结 论
通过多种准静态及动态实验确定了山东五莲地区花岗岩的KF模型参数,并利用KF模型,结合实测参数值,对花岗岩侵彻实验进行了仿真计算,得到以下主要结论。
(1)通过准静态单轴压缩、劈裂及常规三轴实验确定了模型中的部分强度相关参数:fc=130.967 MPa、ft=7.406 MPa、E=39.806 GPa、υ=0.347、a1=0.36、a2=0.09/fc。
(2)通过SHPB实验确定了模型中的应变率相关参数:α=0.06、β=3.47、θ=1.83,并利用SHPB-BD实验结果验证了参数的有效性。
(3)通过平板撞击实验确定了模型中的状态方程相关参数:k1=45.002 GPa、k2=1413.751 GPa、k3=−12037.857 GPa。
(4)利用KF模型及实测参数值对花岗岩侵彻实验进行了模拟,结果表明,数值模拟获得的侵彻深度及成坑最大直径与实验结果的误差小于5%,侵彻弹坑最小直径的模拟结果与实际结果误差小于15%。总体上来说仿真结果与实际结果吻合较好,验证了材料模型及参数值的适用性。
-
表 1 KF模型参数的分类及实验确定方法
Table 1. Parameter classification and experimental determination method of KF model
分类 参数 物理意义 确定方法 材料基本强度参数 ft 劈裂拉伸强度 准静态劈裂实验 fc 单轴压缩强度 准静态单轴压缩实验 E 弹性模量 υ 泊松比 强度面相关参数 a1、a2 强度面相关材料常数 准静态常规三轴实验 α、β、θ 应变率相关材料参数 SHPB/ SHPB-BD实验 状态方程相关参数 k1、k2、k3 状态方程参数 平板撞击实验 表 2 花岗岩的基本强度参数值
Table 2. Basic strength parameters of granite
试件 fc /MPa E/GPa υ 试件 ft /MPa UCT-1 125.088 38.076 0.310 ST-1 7.714 UCT-2 133.206 41.82 0.352 ST-2 6.9 UCT-3 128.969 38.843 0.318 ST-3 8.12 UCT-4 133.802 39.534 0.498 ST-4 6.887 UCT-5 133.772 40.759 0.259 ST-5 7.409 KF模型参数值 130.967±3.856 39.806±1.497 0.347±0.091 KF模型参数值 7.406±0.532 表 3 不同围压条件下试件的轴向峰值强度、偏压力与静水压力
Table 3. The strength, deviatoric pressure and hydrostatic pressure of rock samples under different confining pressures
试件 围压/MPa 轴向峰值强度/MPa 静水压力/MPa 偏应力/MPa 10-1 10 256.220 92.073 246.220 20-1 20 327.341 122.447 307.341 30-1 30 385.384 148.461 355.384 30-2 393.095 151.032 363.095 30-3 390.809 150.270 360.809 40-1 40 437.691 172.564 397.691 40-2 440.422 173.474 400.422 40-3 444.189 174.730 404.189 50-1 50 482.936 194.312 432.936 50-2 485.975 195.325 435.975 50-3 495.950 198.650 445.950 表 4 SHPB、SHPB-BD实验的平均应变率及相应的
ηc 、ηt 值Table 4. Average strain rates and corresponding
ηc andηt values obtained from SHPB and SHPB-BD experiments试件 动态压缩强度/MPa ηc 平均应变率/s−1 试件 动态劈裂拉伸强度/MPa ηt 平均应变率/s−1 SHPB-1 159.324 1.217 83 SHPB-BD-1 41.395 5.589 138 SHPB-2 142.925 1.091 105 SHPB-BD-2 37.363 5.045 135 SHPB-3 160.994 1.229 102 SHPB-BD-3 45.456 6.138 133 SHPB-4 156.455 1.195 85 SHPB-BD-4 45.548 6.150 135 SHPB-5 164.434 1.256 122 SHPB-BD-5 52.404 7.076 187 SHPB-6 180.403 1.377 144 SHPB-BD-6 52.654 7.110 183 SHPB-7 145.891 1.114 168 SHPB-BD-7 49.934 6.742 187 SHPB-8 140.933 1.076 187 SHPB-BD-8 49.225 6.647 188 SHPB-9 212.790 1.625 230 SHPB-BD-9 65.320 8.820 241 SHPB-10 260.696 1.991 325 SHPB-BD-10 54.190 7.317 245 SHPB-11 158.481 1.210 258 SHPB-BD-11 70.300 9.492 239 SHPB-12 177.977 1.359 292 SHPB-BD-12 60.436 8.160 243 SHPB-13 215.694 1.647 360 SHPB-BD-13 48.513 6.550 155 SHPB-BD-14 61.372 8.287 199 SHPB-BD-15 71.112 9.602 210 表 5 花岗岩平板撞击实验结果
Table 5. Plate impact test results of granite samples
实验 w/ (m∙s−1) up,Cu (m∙s−1) up,rock /(m∙s−1) prock /GPa us,rock/ (m∙s−1) μrock G-1 298.249 170.887 212.806 3.103 5430.281 0.039 G-2 298.586 171.614 212.779 3.117 5454.783 0.039 G-3 296.200 175.154 208.623 3.183 5681.906 0.037 G-4 364.353 210.768 258.969 3.856 5543.886 0.047 G-5 362.860 210.444 257.638 3.849 5563.631 0.046 G-6 370.399 217.718 261.540 3.988 5677.582 0.046 G-7 420.639 247.273 297.002 4.553 5708.849 0.052 G-8 425.834 247.173 302.248 4.552 5607.388 0.054 G-9 424.120 246.701 300.769 4.542 5623.706 0.053 G-10 536.010 308.458 381.771 5.743 5601.403 0.068 G-11 527.022 302.785 375.608 5.632 5582.955 0.067 G-12 536.027 312.243 370.879 5.817 5840.622 0.063 表 6 花岗岩KF模型参数拟合结果
Table 6. Fitting results of KF model parameters of granite
强度参数 状态方程参数 fc/MPa ft/MPa E/GPa a1 a2/MPa −1 υ α β θ k1/GPa k2/GPa k3/GPa 130.967 7.406 39.806 0.36 0.09/fc 0.347 0.06 3.47 1.83 45.002 1413.751 −12037.857 基本力学指标 JC本构模型参数 密度/
(kg∙m−3)洛氏硬度
HRC抗拉强度/
MPa延伸率/
%截面收缩率/
%屈服强度A/
MPa硬化系数B/
MPa硬化指数n 应变率敏感
系数c温度软化
系数m7830 45 1380 12 58 1269 810 0.479 0.04 1 表 8 花岗岩靶成坑参数现场测量及三维扫描结果
Table 8. Direct measurement and 3D scanning results of crater parameters of granite targets
试验 子弹着角/(°) 侵彻速度/(m∙s−1) 侵彻深度/mm 侵彻弹坑最大直径/mm 侵彻弹坑最小直径/mm 现场测量 三维扫描 现场测量 三维扫描 现场测量 三维扫描 靶1 −3.5 638 75.05 76.29 310 327.98 245 248.36 靶2 −3.5 667 72.19 72.48 430 408.24 275 283.00 靶3 −1.5 673 96.00 91.73 410 402.60 265 261.67 表 9 实验和数值模拟得到的花岗岩靶侵彻结果比较
Table 9. Comparison of penetration results obtained from penetration test and numerical simulation
方法 侵彻深度/mm 侵彻弹坑最大直径/mm 侵彻弹坑最小直径/mm 实验 80.62±10.46 381.47±49.60 263.01±14.75 数值模拟 80.02 400.00 300.00 误差/% −0.75 4.86 14.07 -
[1] HOLMQUIST T J, JOHNSON G R, COOK W H. A computational constitutive model for concrete subjected to large strains, high strain rates and high pressures [C]//Proceedings of 14th International Symposium. Quebec, Canada: ADPA, 1993: 591–600. [2] MALVAR L J, CRAWFORD J E, WESEVICH J W, et al. A plasticity concrete material model for DYNA3D [J]. International Journal of Impact Engineering, 1997, 19(9/10): 847–873. DOI: 10.1016/S0734-743X(97)00023-7. [3] RIEDEL W, THOMA K, HIERMAIER S, et al. Penetration of reinforced concrete by BETA-B-500 numerical analysis using a new macroscopic concrete model for hydrocodes [C]//Proceedings of the 9th International Symposium on the Interaction of the Effects of Munitions with Structures. Berlin: Fraunhofer Institut für Kurzzeitdynamik, Ernst-Mach-Institut (EMI), 1999: 315–322. [4] JOHNSON G R, HOLMQUIST T J. An improved computational constitutive model for brittle materials [J]. AIP Conference Proceedings, 1994, 309(1): 981–984. DOI: 10.1063/1.46199. [5] TAYLOR L M, CHEN E P, KUSZMAUL J S. Microcrack-induced damage accumulation in brittle rock under dynamic loading [J]. Computer Methods in Applied Mechanics and Engineering, 1986, 55(3): 301–320. DOI: 10.1016/0045-7825(86)90057-5. [6] GRUNWALD C, SCHAUFELBERGER B, STOLZ A, et al. A general concrete model in hydrocodes: verification and validation of the Riedel-Hiermaier-Thoma model in LS-DYNA [J]. International Journal of Protective Structures, 2017, 8(1): 58–85. DOI: 10.1177/2041419617695977. [7] 毕程程. 华山花岗岩HJC本构参数标定及爆破损伤数值模拟 [D]. 合肥: 合肥工业大学, 2018.BI C C. Calibration of HJC constitutive parameters of Huashan granite and its blasting damage numerical simulation [D]. Hefei: Hefei University of Technology, 2018. [8] SAUER C, HEINE A, RIEDEL W. Developing a validated hydrocode model for adobe under impact loading [J]. International Journal of Impact Engineering, 2017, 104: 164–176. DOI: 10.1016/j.ijimpeng.2017.01.019. [9] ZHANG F L, SHEDBALE A S, ZHONG R, et al. Ultra-high performance concrete subjected to high-velocity projectile impact: implementation of K&C model with consideration of failure surfaces and dynamic increase factors [J]. International Journal of Impact Engineering, 2021, 155: 103907. DOI: 10.1016/j.ijimpeng.2021.103907. [10] KONG X Z, FANG Q, WU H, et al. Numerical predictions of cratering and scabbing in concrete slabs subjected to projectile impact using a modified version of HJC material model [J]. International Journal of Impact Engineering, 2016, 95: 61–71. DOI: 10.1016/j.ijimpeng.2016.04.014. [11] KONG X Z, FANG Q, CHEN L, et al. A new material model for concrete subjected to intense dynamic loadings [J]. International Journal of Impact Engineering, 2018, 120: 60–78. DOI: 10.1016/j.ijimpeng.2018.05.006. [12] TU Z G, LU Y. Evaluation of typical concrete material models used in hydrocodes for high dynamic response simulations [J]. International Journal of Impact Engineering, 2009, 36(1): 132–146. DOI: 10.1016/j.ijimpeng.2007.12.010. [13] POLANCO-LORIA M, HOPPERSTAD O S, BØRVIK T, et al. Numerical predictions of ballistic limits for concrete slabs using a modified version of the HJC concrete model [J]. International Journal of Impact Engineering, 2008, 35(5): 290–303. DOI: 10.1016/j.ijimpeng.2007.03.001. [14] KONG X Z, FANG Q, Li Q M, et al. Modified K&C model for cratering and scabbing of concrete slabs under projectile impact [J]. International Journal of Impact Engineering, 2017, 108: 217–228. DOI: 10.1016/j.ijimpeng.2017.02.016. [15] LEPPÄNEN J. Concrete subjected to projectile and fragment impacts: modelling of crack softening and strain rate dependency in tension [J]. International Journal of Impact Engineering, 2006, 32(11): 1828–1841. DOI: 10.1016/j.ijimpeng.2005.06.005. [16] 金乾坤. 混凝土动态损伤与失效模型 [J]. 兵工学报, 2006, 27(1): 10–14. DOI: 10.3321/j.issn:1000-1093.2006.01.003.JIN Q K. Dynamic damage and failure model for concrete materials [J]. Acta Armamentarii, 2006, 27(1): 10–14. DOI: 10.3321/j.issn:1000-1093.2006.01.003. [17] HUANG X P, KONG X Z, CHEN Z Y, et al. A computational constitutive model for rock in hydrocode [J]. International Journal of Impact Engineering, 2020, 145: 103687. DOI: 10.1016/j.ijimpeng.2020.103687. [18] LI X B, LOK T S, ZHAO J. Dynamic characteristics of granite subjected to intermediate loading rate [J]. Rock Mechanics and Rock Engineering, 2005, 38(1): 21–39. DOI: 10.1007/s00603-004-0030-7. [19] WILLMOTT G R, PROUD W G. The shock hugoniot of tuffisitic kimberlite breccia [J]. International Journal of Rock Mechanics and Mining Sciences, 2007, 44(2): 228–237. DOI: 10.1016/j.ijrmms.2006.07.006. [20] 张燕, 于大伟, 叶剑红. 岩石类材料拉伸弹性模量测量方法的对比研究 [J]. 岩土力学, 2018, 39(6): 2295–2303. DOI: 10.16285/j.rsm.2017.2192.ZHANG Y, YU D W, YE J H. Study on measurement methodology of tensile elastic modulus of rock materials [J]. Rock and Soil Mechanics, 2018, 39(6): 2295–2303. DOI: 10.16285/j.rsm.2017.2192. [21] 中国住房和城乡建设部. 工程岩体试验方法标准: GB/T 50266-2013 [S]. 北京: 中国标准出版社, 2008. . [22] 刘佑荣, 唐辉明. 岩体力学 [M]. 北京: 化学工业出版社, 2008. [23] 中国爆破行业协会. 岩石材料动态单轴压缩强度测试方法: T/CSEB 0004-2018 [S]. 北京: 冶金工业出版社, 2018. [24] 中国爆破行业协会. 岩石材料巴西圆盘试样动态拉伸强度测试方法: T/CSEB 0001-2018 [S]. 北京: 冶金工业出版社, 2018. [25] ZHANG Q B, BRAITHWAITE C H, ZHAO J. Hugoniot equation of state of rock materials under shock compression [J]. Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences, 2017, 375(2085): 20160169. DOI: 10.1098/rsta.2016.0169. [26] SHANG J L, SHEN L T, ZHAO J. Hugoniot equation of state of the Bukit Timah granite [J]. International Journal of Rock Mechanics and Mining Sciences, 2000, 37(4): 705–713. DOI: 10.1016/S1365-1609(00)00002-2. [27] MEYERS M A. Dynamic behavior of materials [M]. New York: Wiley, 1994: 133. [28] 李磊, 张先锋, 吴雪, 等. 不同硬度30CrMnSiNi2A钢的动态本构与损伤参数 [J]. 高压物理学报, 2017, 31(3): 239–248. DOI: 10.11858/gywlxb.2017.03.005.LI L, ZHANG X F, WU X, et al. Dynamic constitutive and damage parameters of 30CrMnSiNi2A steel with different hardnesses [J]. Chinese Journal of High Pressure Physics, 2017, 31(3): 239–248. DOI: 10.11858/gywlxb.2017.03.005. [29] 梁斌, 刘彤. 有界混凝土靶尺寸效应对弹丸侵彻的影响研究 [J]. 弹箭与制导学报, 2004, 24(4): 39–41. DOI: 10.3969/j.issn.1673-9728.2004.04.013. 期刊类型引用(1)
1. 杨慧,王可慧,周刚,李明,吴海军,戴湘晖,段建. 不同风化程度花岗岩的动态力学特性及抗侵彻性能. 爆炸与冲击. 2024(10): 49-66 .  本站查看
本站查看其他类型引用(2)
-













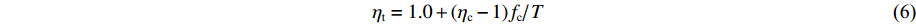


 下载:
下载:




















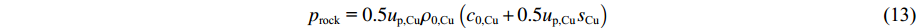













 下载:
下载: