Wave propagation in lattices based on Tersoff potential
-
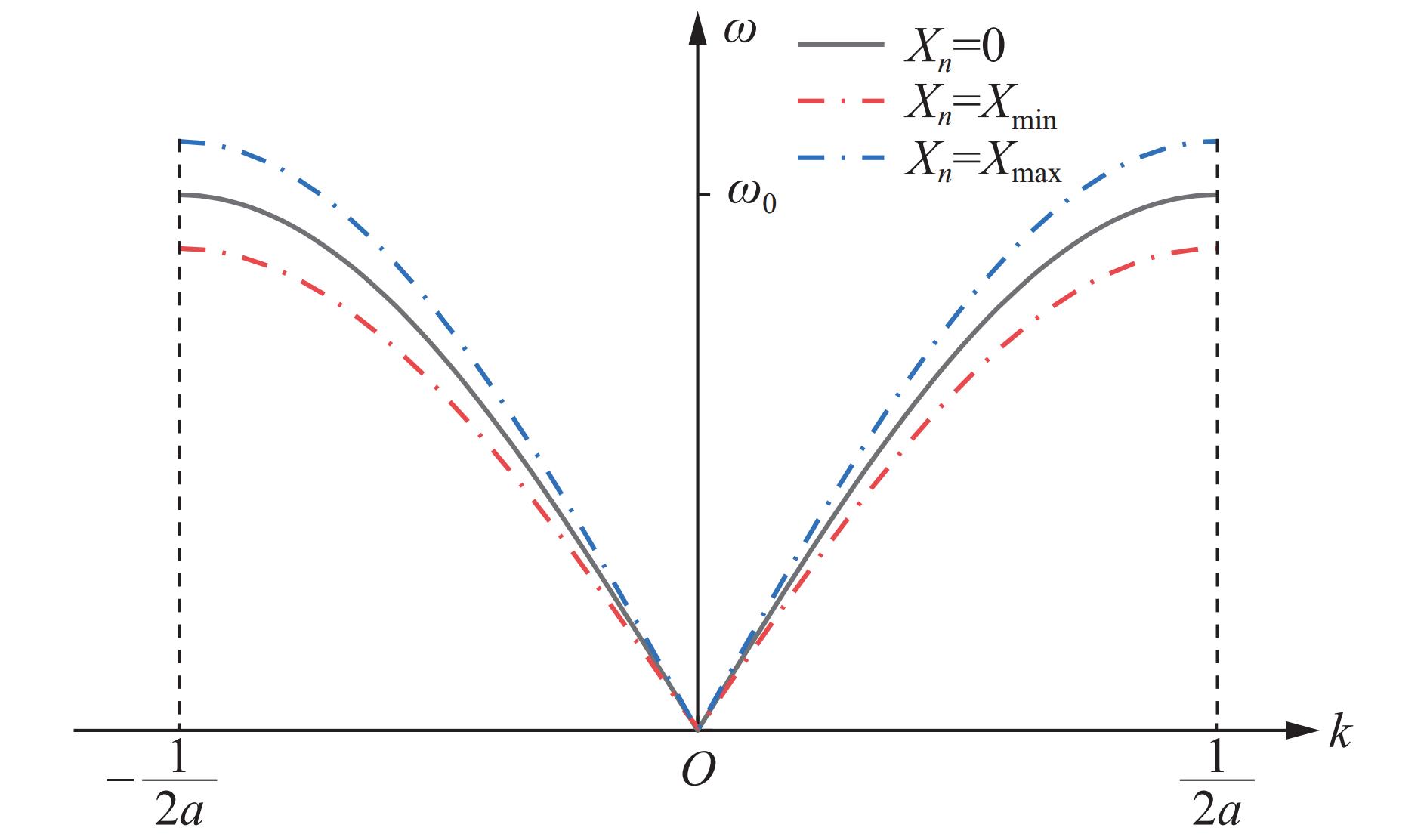
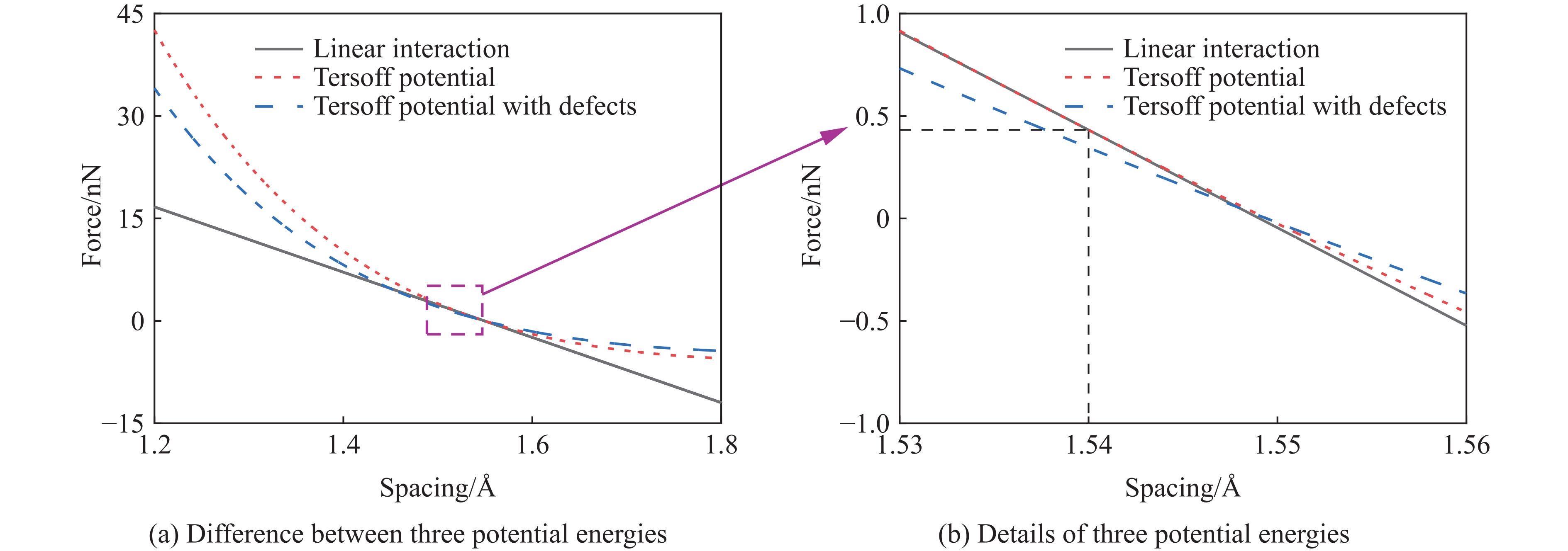
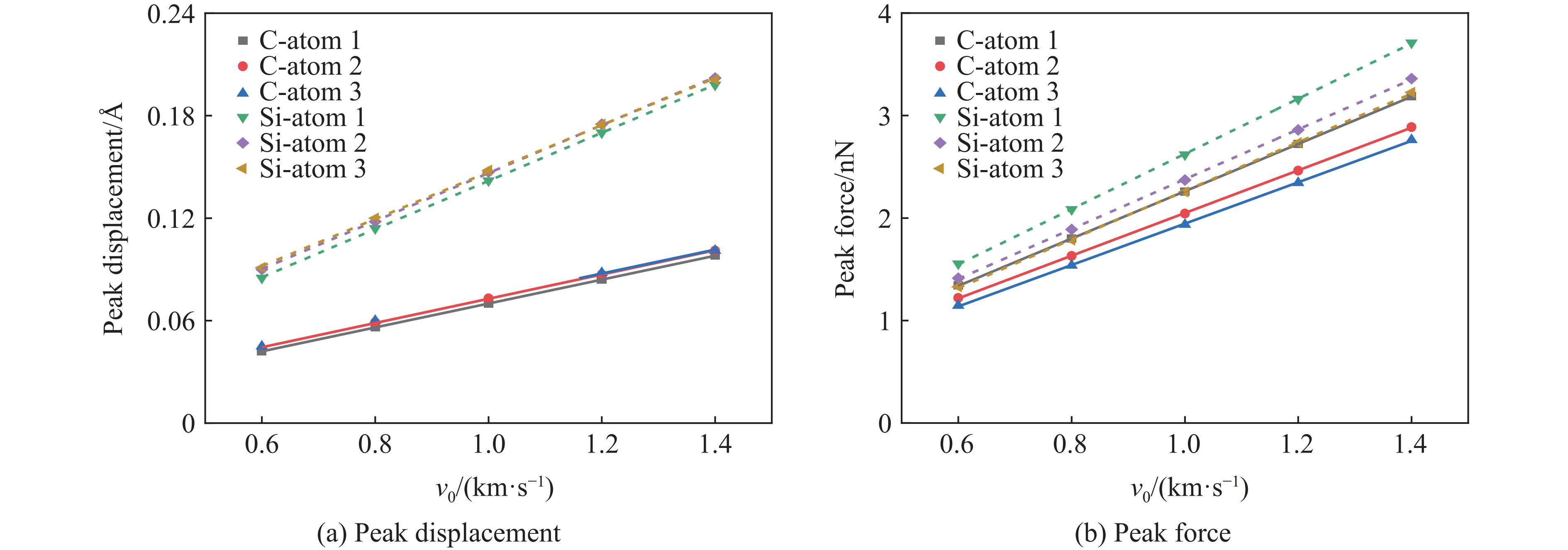
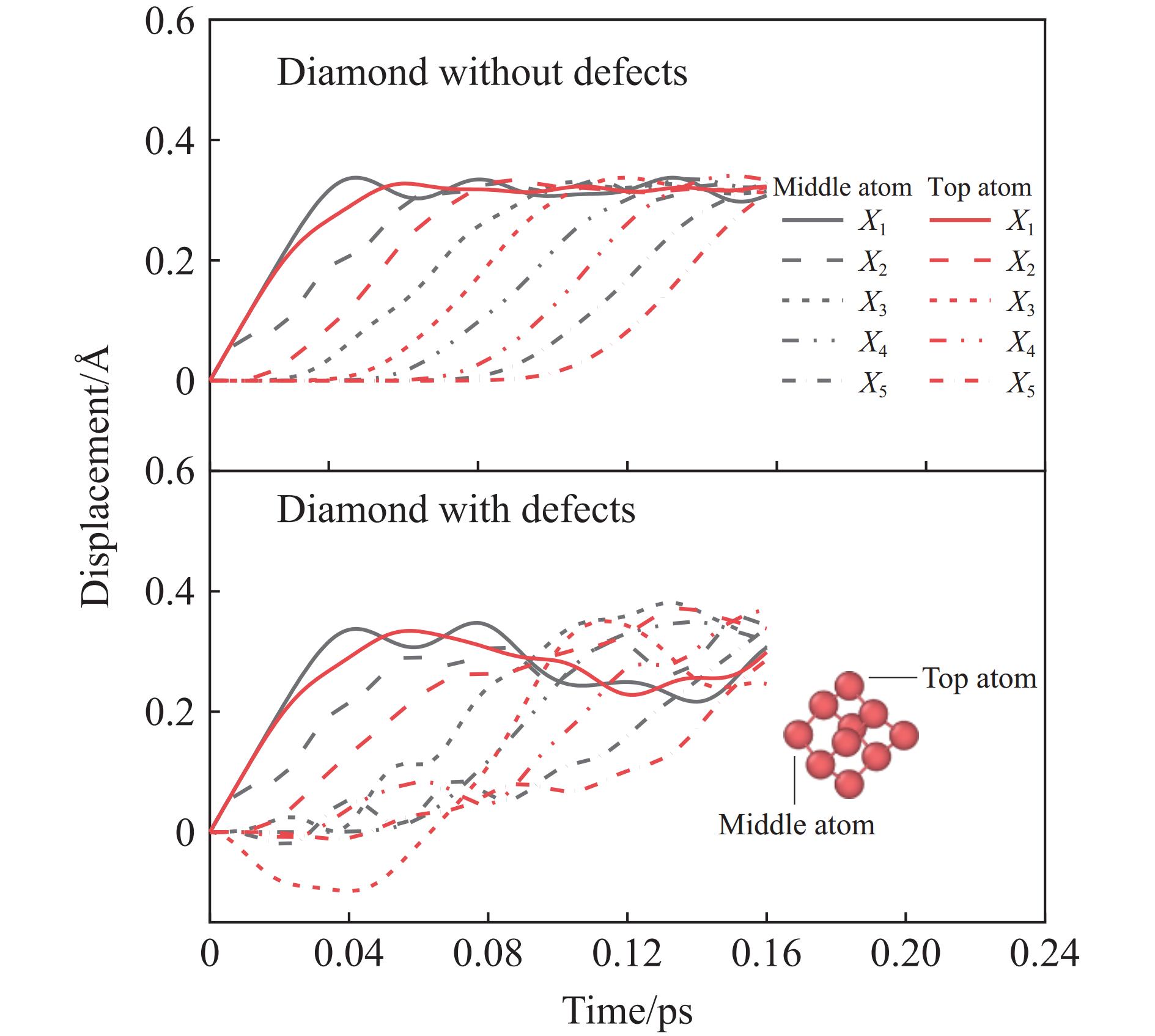
摘要: 在晶格间的Tersoff势作用下分别研究了单晶体系和多晶体系中的波动传播特性。首先，在微振动的情况下，分别基于晶格间线性作用、Tersoff势作用以及含缺陷的Tersoff势作用3种势能函数研究了单晶体系中格波的传播，得到了晶格中的色散关系以及格波波速的表达式。其次，分别以碳晶格和硅晶格为例，运用有限差分方法，研究了3种势能作用下单晶体系中的波动传播过程，对比了压缩和拉伸冲击下晶格的运动差异，并讨论了入射速度对位移峰值和受力峰值的影响，揭示了单晶体系中波动传播与连续介质中波动传播的差异。最后，分别以金刚石和碳化硅为例，采用分子动力学模拟方法，研究了多晶体系中的波动传播特性，讨论了不同空间位置原子的运动差异。结果表明：多晶体系中晶格结构更复杂，其中的波动传播特性与单晶体系存在差异；缺陷的存在对波动传播规律影响显著，这种影响在多晶体系中表现得更加突出。Abstract: The propagation characteristics of waves are the basis for studying the dynamic behavior of materials, and the theoretical study of waves in continuous media at the macro scale has been well developed. With the widespread application of materials and structures at the micro- and nano- scales, the study of wave propagation characteristics at the lattice scale is receiving increasing attention. In this article, the Tersoff potential interaction between lattices is applied to study the wave propagation characteristics in single-crystal and polycrystalline systems. Firstly, in the case of micro-vibration, the propagation of lattice waves in a single-crystal system is studied based on three potential energy functions between lattices: linear interaction, Tersoff potential, and Tersoff potential with defects. The dispersion relationship in the lattice and the expression of lattice wave velocity are obtained. Secondly, taking carbon lattice and silicon lattice as examples, the finite difference method is applied to study the wave propagation process in the single-crystal system under three potential energies. The differences in lattice motion under compressive and tensile impacts are compared, and the influence of incident velocity on the peak displacement and peak force is discussed, which reveals the difference in wave propagation between single-crystal systems and continuous media. Finally, taking diamond and silicon carbide as examples, molecular dynamics simulations are used to study the wave propagation characteristics in polycrystalline systems, and the differences in atomic motion at different spatial positions are discussed. The results indicate that the lattice structure in polycrystalline systems is more complex, and the wave propagation characteristics in polycrystalline systems are different from those in single-crystal systems. The existence of defects has a significant impact on the propagation law of waves, which is more prominent in polycrystalline systems. This study has good reference significance for the study of material dynamics performance at the micro- and nano- scales.
-
Key words:
- lattice dynamics /
- micro-vibration /
- Tersoff potential /
- defect /
- molecular dynamics
-
电热化学发射技术使用等离子体发生器代替常规点火源，将电能转化为等离子体内能来点燃固体发射药。等离子体与固体发射药相互作用不仅能显著缩短发射药点火延迟时间[1-3]，还能消除发射药的温度效应[4]，提高燃速，从而有效提高炮口初速和动能[5]。常规发射通常使用指数燃速公式来描述发射药燃烧特性[6-7]，C.R.Woodley等[8-9]假定电热化学发射时注入的电能只用于增强发射药的内能，引入了电功率增强因子，给出了与输入电功率相关的发射药燃速公式。M.J.Taylor等[10]进一步研究了辐射、发射药侵蚀和电热裂解对燃速的影响，考虑了引起燃速增强的电功率阈值的影响。李海元等[11-12]、A.Brik等[13]利用改进的密闭爆发器对等离子体增强发射药燃速的特性进行了实验研究; 实验表明等离子体作用下，发射药燃气压力变化迅速，燃气压力梯度增大，点火和燃烧特性发生显著变化。因此，等离子体作用下燃气压力梯度对发射药燃速的影响不可忽略。
本文中，拟通过密闭爆发器实验得到燃气压力曲线，分析等离子体作用下固体发射药的燃烧特性。基于指数燃速公式，结合燃气压力梯度和电功率增强对发射药燃速的影响，得到等离子体作用下固体发射药的瞬态燃速公式。
1. 基本原理
1.1 实验装置
图 1所示为等离子体点火密闭爆发器实验装置结构示意图，主要由脉冲成形网络、底喷式等离子体发生器和密闭爆发器等组成。密闭爆发器为耐高压厚壁圆筒，其中一端装有底喷式等离子体发生器，其主体由聚乙烯毛细管组成，一端采用杆状封闭电极，另一端装有敞开式环状电极，通过爆炸丝将两端电极导通。脉冲成形网络由多个模块并联组成，能通过时序设置产生幅值、脉宽可调的脉冲电流。固体发射药试样由硝化棉纸包裹放置于密闭爆发器内。
图 1 等离子体点火密闭爆发器实验装置结构示意图Figure 1. Experimental setup of closed bomb with plasma igniter实验测试系统主要由传感器和数据采集设备组成。燃气压力采用Kistler6215压力传感器测量，发生器两端的电压和电流分别采用电阻分压器和Rogowski线圈测量。采用JV5200瞬态记录仪对脉冲电源和发生器的电参数以及密闭爆发器内的压力信号进行同步采集记录。
1.2 瞬态燃速理论模型
1.2.1 基本假设
忽略密闭爆发器内固体发射药试样燃烧过程中气相压力和温度随空间的变化，即假设任意时刻密闭爆发器内各位置的气相压力和温度均相同。
固体发射药试样燃烧过程满足几何燃烧定律假设：(1)装药的所有颗粒具有均一的理化性质以及完全相同的几何形状和尺寸；(2)所有药粒表面同时着火；(3)所有药粒具有相同的燃烧环境。
由于气相与密闭爆发器壁面存在换热，需考虑试样燃烧过程中的热损失。本文中采用热损失因数修正发射药火药力的方式来计算发射药试样燃烧过程中的热损失。
1.2.2 气相状态方程
考虑点火药的影响，得到密闭爆发器内的气体状态方程[14]：
p{V0−mρp[1−ψ(t)]−αmψ(t)−αigmig(t)}=T[Rmψ(t)+Rigmig(t)] (1) 式中:p为燃气压力，Pa；V0为密闭爆发器容积，m3；m为装药质量，kg；ρp为固体发射药密度，kg/m3；ψ (t)为t时刻发射药已燃体积分数；α为余容，m3/kg；αig为点火药余容，m3/kg；mig(t)为t时刻已燃点火药质量，kg；T为混合气体温度，K；R为发射药燃气气体常数，J/(mol·K)；Rig为点火药燃气气体常数，J/(mol·K)。
1.2.3 气相能量守恒方程
气相能量守恒方程[14]为：
(1−c1)fmψ(t)+figmig(t)+(κ−1)cplEpl(t)=T[Rmψ(t)+Rigmig(t)] (2) 式中:cl为热损失因数；f为发射药火药力，J/kg；fig为点火药火药力，J/kg；κ为燃气比热比；cpl为密闭爆发器中输入等离子体电能的利用率；Epl(t)为t时刻输入等离子体发生器的电能，J。
式(2)通用于常规点火和等离子体点火。常规点火时，Epl(t)=0；等离子体点火时，mig(t)=0。
1.2.4 固体发射药瞬态燃速公式
根据Vieille定律，常规点火时固体发射药燃速公式通常采用指数形式[14]：
u=u1pn1 (3) 式中:u1为燃速系数，n1为燃速指数。
C.R.Woodley等[8]在Vieille定律的基础上引入电功率增强因子，得到等离子体增强发射药燃速公式:
u=u1pn1(1+βePe) (4) 式中:βe为电功率增强因子，MW-1; Pe为输入发生器的电功率，MW。
等离子体点火时，在Woodley燃速公式的基础上，加入燃气压力梯度对发射药燃速的影响[15]，得到等离子体作用下固体发射药瞬态燃速公式：
u=u1pn1[1+A(t)n1u21p2n1+1dpdt](1+βePe) (5) 式中:A(t)为发射药燃烧过程中与压力及火焰结构有关的时间函数。
1.2.5 相关系数处理方法
由气体状态方程和能量守恒方程可知：
p{V0−mρp[1−ψ(t)]−αmψ(t)−αigmig(t)}=(1−c1)fmψ(t)+figmig(t)+(κ−1)cplEpl(t) (6) 首先，通过常规点火实验确定热损失因数。常规点火时, Epl(t)=0。发射药试样完全燃完时刻有：ψ(t) =1, p=pm, pm为实验测得的最高燃气压力。由此可求出发射药燃烧过程中的热损失因数：
c1=1+figmig(t)fm−pmf[V0m−α−mig(t)mαig] (7) 其次，通过等离子体点火实验确定电能利用率。等离子体点火时，mig(t)=0。同样发射药试样完全燃完时刻有:ψ(t) =1, p=pm。假定等离子体点火时热损失因数未发生变化，进一步求出等离子体点火过程中的电能利用率：
cpl=pm(V0−αm)−(1−c1)fm(κ−1)Epl(t) (8) 1.2.6 拟合精度
采用均方误差σ来衡量模拟压力曲线与实验压力曲线间的误差：
σ=√n∑i=1[ps(i)−pt(i)]2n (9) 式中:n为压力曲线上选取不同时刻的点数；ps(i)、pt(i)分别为同一时刻模拟和实验测得的压力。
2. 实验结果与分析
对4/7高固体发射药试样进行了2发常规点火和3发等离子体点火密闭爆发器实验。其中密闭爆发器容积为145 cm3，装药量为36.1 g; 实验中采用单个模块放电，电容约为1 300 μF，电感为40 μH。实验参数与结果如表 1所示，其中第3、4发实验发射药置于密闭爆发器中间，第5发实验发射药置于发生器出口处。表 1中, Uc为电容器放电电压；tig为发射药点火延迟时间(定义为爆发器内压力达到20 MPa的时刻)；tend为发射药完全燃完时刻，即爆发器内压力达到峰值pm对应的时刻。
表 1 实验参数和结果Table 1. Experimental parameters and results编号 点火方式 Uc/kV tig/ms tend/ms pm/MPa 实验1 2号电点火 1.604 5.05 298 实验2 2号电点火 1.673 4.95 300 实验3 等离子体点火 8.3 0.261 2.93 318 实验4 等离子体点火 10.0 0.197 2.44 319 实验5 等离子体点火 10.1 0.167 2.16 329 实验测得的膛内压力曲线如图 2所示，由图可知等离子体点火大大缩短了发射药试样的点火延迟时间和燃烧过程时间；同时，随着等离子体的注入，密闭爆发器内燃气压力不断增大。
实验测得的膛内压力梯度曲线如图 3所示，由图 3可知在发射药燃烧初始时刻，等离子体作用下的燃气压力梯度远大于常规点火，而且等离子体明显影响了燃气的压力梯度，随着发射药的燃烧，影响程度逐渐降低。
图 4所示为常规点火时实验和数值模拟得到的压力曲线，其中数值模拟压力曲线分别由指数燃速公式和瞬态燃速公式推得。对比可知，指数燃速公式和瞬态燃速公式均有较高的精确度；但是在发射药燃烧后期瞬态燃速公式与实验结果更吻合。
图 4 常规点火时实验与数值模拟压力曲线Figure 4. Experimental and simulated pressure-time curvesafter conventional ignition通过分析常规点火和等离子体点火实验相关数据，得到4/7高发射药的电功率增强因子为0.005 MW-1。图 5~7分别给出了第3、4和5发等离子体点火实验时压力与放电功率随时间变化的曲线，其中压力曲线分别通过实验测量、Woodley燃速公式和瞬态燃速公式所得。当加入电功率增强因子后，Woodley燃速公式和瞬态燃速公式得到的压力曲线均符合实验曲线。因此，拟合得到的电功率增强因子适用于4/7高发射药试样。
图 5 第3发实验的压力与输入电功率曲线Figure 5. Pressure-time and electric power-time curves for test 3
图 6 第4发实验的压力与输入电功率曲线Figure 6. Pressure-time and electric power-time curves for test 4
图 7 第5发实验的压力与输入电功率曲线Figure 7. Pressure-time and electric power-time curves for test 5结合图 3中的压力梯度曲线，分析等离子体输入过程中压力梯度与发射药位置和输入电功率的关系。对比第3、4发实验曲线可知，发射药处于相同位置时，早期燃气压力梯度峰值与输入电功率峰值成正比，且压力梯度变化趋势与输入电功率曲线相似。对比第4、5发实验曲线可知，在相似电功率下，缩短发生器与发射药距离提高了电能利用系数，从而增大了燃气压力梯度。因此，缩短等离子体与发射药距离，提高输入电功率均能获得更理想的等离子体增强效应。
对比图 5~7中各压力曲线可知，在燃烧中间段Woodley燃速公式所得压力曲线略低于实验压力曲线。其原因可能是Woodley燃速公式中仅考虑了等离子体注入期间的电增强效应，未考虑放电结束后的增强效应。因此，脉冲放电结束后Woodley燃速公式所得压力曲线与实验有偏差，而在瞬态燃速公式中通过压力梯度项考虑了放电结束后的燃速增强效应，提高了数值模拟精度。
进一步对比各燃速公式的拟合程度，计算得拟合的压力曲线的均方误差如表 2所示。由表 2可知，随着输入电功率的增加以及发生器与发射药间距的减小，Woodley燃速公式均方误差增大；瞬态燃速公式得到的压力均方误差小于Woodley燃速公式，且受到输入电能、电功率和发生器与发射药间距的影响更小。因此，瞬态燃速公式得到的膛压曲线与实验数据符合情况优于Woodley燃速公式，能更真实地反映等离子体作用下密闭爆发器内燃气压力的变化。
表 2 模拟压力曲线与实验压力曲线间的均方误差Table 2. Mean squared errors between simulated pressure curves and test ones编号 σ/MPa Woodley燃速公式 瞬态燃速公式 实验3 4.325 4.294 实验4 9.312 4.910 实验5 13.506 5.715 3. 结论
利用密闭爆发器实验，研究了等离子体增强4/7高固体发射药的燃速特性，实验表明等离子体作用下燃气压力梯度增大，燃速明显增强。根据实验数据，综合考虑燃气压力梯度和燃速增强因子的影响，拟合了4/7高固体发射药等离子体作用下的瞬态燃速公式，计算得到等离子体注入期间的燃速增强因子为0.005 MW-1。瞬态燃速公式与实验压力曲线符合程度比C.R.Woodley等[8]提出的燃速公式更高，能更精确描述固体发射药在等离子体作用下的燃烧过程。实验表明，在低装填密度时，缩短等离子体与发射药的距离和提高电功率均能获得更明显的等离子体增强效应。
-
图 2 Tersoff势下角频率与波数的关系
Figure 2. Relationship between angular frequency and wavenumber under Tersoff potential
图 3 含缺陷的Tersoff势下角频率与波数的关系
Figure 3. Relationship between angular frequency and wavenumber under Tersoff potential with defects
图 4 碳晶格中势力与晶格间距的关系
Figure 4. Relationship between force and lattice spacing in carbon lattices
图 5 不同势作用下碳晶格中波的传播特性
Figure 5. Propagation characteristics of waves in carbon lattice under different potentials
图 6 压缩和拉伸过程中波形的对比
Figure 6. Comparison of waveforms during compression and stretching processes
图 7 含缺陷时压缩和拉伸过程中波形的对比
Figure 7. Comparison of waveforms during compression and stretching processes with defects
图 8 C晶格和Si晶格中波的传播特性
Figure 8. Propagation characteristics of waves in carbon lattice and silicon lattice
图 10 金刚石和碳化硅的分子动力学模型
Figure 10. Molecular dynamics models of diamond and silicon carbide
图 13 压缩和拉伸过程中金刚石中的波传播特性
Figure 13. Wave propagation characteristics of diamond during compression and stretching processes
图 14 金刚石与碳化硅中波传播过程的对比
Figure 14. Comparison of wave propagation processes in diamond and silicon carbide
图 15 多晶体系中入射速度对波传播的影响
Figure 15. Influences of incident velocity on wave propagation in polycrystalline systems
共价键种类 m1 γ λ3/Å–1 C d cosθ0 n1 C―C 3.0 1.0 0 38 049 4.348 4 –0.570 58 0.727 51 Si―Si 3.0 1.0 0 100 390 16.217 0 –0.598 25 0.787 34 共价键种类 β λ2/Å–1 B/eV R/Å D/Å λ1/Å–1 A1/eV C―C 1.572 4×10–7 2.211 90 346.70 1.95 0.15 3.487 9 1 393.6 Si―Si 1.100 0×10–6 1.732 22 471.18 2.85 0.15 2.479 9 1 830.8 
下载: 导出CSV
表 2 2种晶格在平衡位置处的参数
Table 2. Parameters of two types of lattices at equilibrium positions
原子种类 摩尔质量/(g·mol−1) 平衡距离/Å 晶格常数/Å C 12 1.54 3.57 Si 28 2.35 5.43
下载: 导出CSV
-
[1] 徐松林, 刘永贵, 席道瑛. 岩石物理与动力学原理 [M]. 北京: 科学出版社, 2019: 1–21.XU S L, LIU Y G, XI D Y. Rock physics and dynamics principle [M]. Beijing: Science Press, 2019: 1–21. [2] 王礼立. 应力波基础 [M]. 2版. 北京: 国防工业出版社, 2005: 1–28.WANG L L. Foundation of stress waves [M] 2nd ed. Beijing: National Defense Industry Press, 2005: 1–28. [3] 袁良柱, 陆建华, 苗春贺, 等. 基于分数阶模型的牡蛎壳动力学特性研究 [J]. 爆炸与冲击, 2023, 43(1): 011101. DOI: 10.11883/bzycj-2022-0318.YUAN L Z, LU J H, MIAO C H, et al. Dynamic properties of oyster shells based on a fractional-order model [J]. Explosion and Shock Waves, 2023, 43(1): 011101. DOI: 10.11883/bzycj-2022-0318. [4] 袁良柱, 苗春贺, 单俊芳, 等. 冲击下混凝土试样应变率效应和惯性效应探讨 [J]. 爆炸与冲击, 2022, 42(1): 013101. DOI: 10.11883/bzycj-2021-0114.YUAN L Z, MIAO C H, SHAN J F, et al. On strain-rate and inertia effects of concrete samples under impact [J]. Explosion and Shock Waves, 2022, 42(1): 013101. DOI: 10.11883/bzycj-2021-0114. [5] CHEN M D, XU S L, YUAN L Z, et al. Influence of stress state on dynamic behaviors of concrete under true triaxial confinements [J]. International Journal of Mechanical Sciences, 2023, 253: 108399. DOI: 10.2139/ssrn.4332010. [6] JANG S, RABBANI M, OGRINC A L, et al. Tribochemistry of diamond-like carbon: interplay between hydrogen content in the film and oxidative gas in the environment [J]. ACS Applied Materials & Interfaces, 2023, 15(31): 37997–38007. DOI: 10.1021/acsami.3c05316. [7] HU L F, ZHAI X Y, LI J G, et al. Improving the mechanical properties and tribological behavior of sulfobetaine polyurethane based on hydrophobic chains to be applied as artificial meniscus [J]. ACS Applied Materials & Interfaces, 2023, 15(25): 29801–29812. DOI: 10.1021/acsami.3c02940. [8] WANG D Y, WANG P F, WU Y F, et al. Temperature and rate-dependent plastic deformation mechanism of carbon nanotube fiber: experiments and modeling [J]. Journal of the Mechanics and Physics of Solids, 2023, 173: 105241. DOI: 10.1016/j.jmps.2023.105241. [9] 薛晓. 碳纳米管纤维的动静态力学性能研究 [D]. 合肥: 中国科学技术大学, 2020: 53–61. DOI: 10.27517/d.cnki.gzkju.2020.000565.XUE X. Investigation of dynamic and quasi-static mechanical properties of carbon nanotube fibers [D]. Hefei: University of Science and Technology of China, 2020: 53–61. DOI: 10.27517/d.cnki.gzkju.2020.000565. [10] MACHADO M, MOREIRA P, FLORES P, et al. Compliant contact force models in multibody dynamics: evolution of the Hertz contact theory [J]. Mechanism and Machine Theory, 2012, 53: 99–121. DOI: 10.1016/j.mechmachtheory.2012.02.010. [11] BORODICH F M. The Hertz-type and adhesive contact problems for depth-sensing indentation [J]. Advances in Applied Mechanics, 2014, 47: 225–366. DOI: 10.1016/b978-0-12-800130-1.00003-5. [12] YANG F, XIE W H, MENG S H. Impact and blast performance enhancement in bio-inspired helicoidal structures: a numerical study [J]. Journal of the Mechanics and Physics of Solids, 2020, 142: 104025. DOI: 10.1016/j.jmps.2020.104025. [13] PENG Q, LIU X M, WEI Y G. Elastic impact of sphere on large plate [J]. Journal of the Mechanics and Physics of Solids, 2021, 156: 104604. DOI: 10.1016/j.jmps.2021.104604. [14] TANG X, YANG J. Wave propagation in granular material: what is the role of particle shape? [J]. Journal of the Mechanics and Physics of Solids, 2021, 157: 104605. DOI: 10.1016/j.jmps.2021.104605. [15] ALBERDI R, ROBBINS J, WALSH T, et al. Exploring wave propagation in heterogeneous metastructures using the relaxed micromorphic model [J]. Journal of the Mechanics and Physics of Solids, 2021, 155: 104540. DOI: 10.1016/j.jmps.2021.104540. [16] WAYMEL R F, WANG E, AWASTHI A, et al. Propagation and dissipation of elasto-plastic stress waves in two dimensional ordered granular media [J]. Journal of the Mechanics and Physics of Solids, 2018, 120: 117–131. DOI: 10.1016/j.jmps.2017.11.007. [17] LI S F, WANG G. Introduction to micromechanics and nanomechanics [M]. Singapore: World Scientific Publishing Co. Pre. Ltd., 2008. [18] ZUNDEL L, MALONE K, CERDÁN L, et al. Lattice resonances for thermoplasmonics [J]. ACS Photonics, 2023, 10(1): 274–282. DOI: 10.1021/acsphotonics.2c01610. [19] CERDÁN L, ZUNDEL L, MANJAVACAS A. Chiral lattice resonances in 2.5-dimensional periodic arrays with achiral unit cells [J]. ACS Photonics, 2023, 10(6): 1925–1935. DOI: 10.1021/acsphotonics.3c00369. [20] HU Y G, LIEW K M, WANG Q, et al. Nonlocal shell model for elastic wave propagation in single-and double-walled carbon nanotubes [J]. Journal of the Mechanics and Physics of Solids, 2008, 56(12): 3475–3485. DOI: 10.1016/j.jmps.2008.08.010. [21] AGARWAL G, VALISETTY R R, DONGARE A M. Shock wave compression behavior and dislocation density evolution in Al microstructures at the atomic scales and the mesoscales [J]. International Journal of Plasticity, 2020, 128: 102678. DOI: 10.1016/j.ijplas.2020.1026. [22] SAM A, ÁLVAREZ M B, VENEGAS R, et al. Multiscale acoustic properties of nanoporous materials: from microscopic dynamics to mechanics and wave propagation [J]. The Journal of Physical Chemistry C, 2023, 127(15): 7471–7483. DOI: 10.1021/acs.jpcc.3c00060. [23] 薛定谔. 薛定谔讲演录 [M]. 2版. 范岱年, 胡新和, 译. 北京: 北京大学出版社, 2019: 7–8.SCHRÖDINGER E. Lectures of Schrödinger [M]. 2nd ed. Translated by FAN D N, HU X H. Beijing: Peking University Press, 2019: 7–8. [24] TOLOS L, CENTELLES M, RAMOS A. The equation of state for the nucleonic and hyperonic core of neutron stars [J]. Publications of the Astronomical Society of Australia, 2017, 34: e065. DOI: 10.1017/pasa.2017.60. [25] AARABI M, SARKA J, PANDEY A, et al. Quantum dynamical investigation of dihydrogen-hydride exchange in a transition-metal polyhydride complex [J]. The Journal of Physical Chemistry A, 2023, 127(31): 6385–6399. DOI: 10.1021/acs.jpca.3c01863. [26] HO W W, CHOI S. Exact emergent quantum state designs from quantum chaotic dynamics [J]. Physical Review Letters, 2022, 128(6): 060601. DOI: 10.1103/PhysRevLett.128.060601. [27] 黄昆. 固体物理学 [M]. 北京: 人民教育出版社, 1966: 35–43. [28] 王礼立, 胡时胜, 杨黎明, 等. 材料动力学 [M]. 合肥: 中国科学技术大学出版社, 2017: 33–158. [29] BRENNER D W. Tersoff-type potentials for carbon, hydrogen and oxygen [J]. MRS Online Proceedings Library (OPL), 1988, 141: 59. DOI: 10.1557/proc-141-59. [30] TERSOFF J. Modeling solid-state chemistry: interatomic potentials for multicomponent systems [J]. Physical Review B, 1989, 39(8): 5566. DOI: 10.1103/PhysRevB.39.5566. 期刊类型引用(15)
1. 薛颖杰，陈智刚，杨芮，崔晋，康彦姝，付建平. 椭圆截面弹体侵彻多层混凝土靶的数值仿真. 中北大学学报(自然科学版). 2025(01): 35-44 . 
百度学术2. 张丁山，张博，付良，徐笑，李鹏飞. 弹体尾部斜锥面形状对侵彻偏转的影响. 高压物理学报. 2024(01): 145-154 . 百度学术3. 刘泓甫，黄风雷，白志玲，段卓平. 刚性弹体带攻角斜侵彻贯穿混凝土靶板的理论模型. 兵工学报. 2023(08): 2381-2390 . 百度学术4. 朱超，张晓伟，张庆明，张陶. 弹体斜侵彻双层钢板的结构响应和失效研究. 爆炸与冲击. 2023(09): 140-154 . 
本站查看5. 杨璞，李继承，陈建良，张斌，何丽灵，陈刚. 撞击姿态对构型弹体非正侵彻多层间隔钢靶弹道特性的影响规律. 爆炸与冲击. 2023(09): 123-139 . 本站查看6. 张丁山，全嘉林，付良，张博，徐笑. 侵彻弹体尖卵形头部形状对偏转力矩的影响. 火炸药学报. 2023(09): 834-839 . 百度学术7. 姜安邦，李典，李永清，侯海量. 偏转式抗侵彻防护技术研究现状. 材料导报. 2023(24): 99-107 . 百度学术8. 陈柏翰，沈子楷，邹慧辉，王伟光，王可慧. 硬目标内弹体斜侵彻状态的基本演化特性. 兵工学报. 2023(S1): 59-66 . 百度学术9. 陈柏翰，王笠镔，邹慧辉，王伟光，王可慧. 自旋对弹体侵彻效应的影响. 兵工学报. 2023(S1): 117-124 . 百度学术10. 郭松林，高世桥，李泽章，李云彪. 弹引系统攻角侵彻混凝土仿真与试验研究. 兵器装备工程学报. 2022(01): 135-139+205 . 百度学术11. 成丽蓉，汪德武，贺元吉，赵宏伟，杨杰，邓斌. 弹体斜侵彻多层间隔混凝土薄靶姿态偏转机理研究. 北京理工大学学报. 2022(10): 1009-1016 . 百度学术12. 李鹏程，张先锋，刘闯，魏海洋，刘均伟，邓宇轩. 攻角和入射角对弹体侵彻混凝土薄靶弹道特性影响规律研究. 爆炸与冲击. 2022(11): 92-104 . 本站查看13. 廉璞，牟东，青泽，刘军. 侵彻弹药姿态测量技术研究现状及发展. 探测与控制学报. 2021(02): 1-9 . 百度学术14. 李东伟，刘俞平，王筱锋，王昭明，赵双双. 弹形参数对战斗部斜穿甲姿态偏转影响研究. 兵器装备工程学报. 2021(09): 40-44 . 百度学术15. 张健东，武海军，李伟，李金柱，皮爱国. 头部带肋板的异形结构弹体斜贯穿混凝土薄靶实验和数值模拟. 高压物理学报. 2021(06): 186-194 . 百度学术其他类型引用(5)
-
下载:
百度学术
计量
- 文章访问数: 259
- HTML全文浏览量: 96
- PDF下载量: 91
- 被引次数: 20