Stress wave effects and influencing mechanisms on stress-strain curves in the elastic compression stage of SHPB tests based on generalized wave impedance theory
-
摘要: 定量研究分离式霍普金森压杆(split Hopkinson pressure bar,SHPB)试验中弹性压缩阶段试件中的应力波效应是解耦准确材料弹性曲线的基础。在满足平面波假设的基础上,基于广义波阻抗理论,对杆与试件面积不匹配时试件弹性压缩阶段应力波演化造成的结构效应开展了定量理论研究,分析了不同情况下弹性阶段内试件唯象工程及实际材料应力-应变曲线的偏差特征与主要因素,并揭示了影响这种偏差的影响规律及其机理。研究表明:对于线性入射加载波,当无量纲时间为0.5的倍数时,即使其他参数改变,试件唯象与材料实际的应力-应变曲线仍对应相等;试件两端的应力差较大时,若应力差的变化趋于稳定,则试件唯象与材料实际的应力-应变曲线差异较小。计算了不同波动区间内试件的最大应力偏离值及其变化趋势和对应的无量纲时间,研究了入射波是双线性组合波时试件的应力-应变曲线。研究表明:双线性波入射时,2个线性区间可以独立分析,无论如何组合线性区间或应力差如何变化,只要试件两端应力差为近似恒定曲线,对应的试件唯象工程应力-应变曲线都是相对准确的。Abstract: Quantitative investigation of stress wave effects during the elastic compression stage of split Hopkinson pressure bar (SHPB) tests is fundamental for decoupling accurate elastic curve of material. Based on the assumption of plane waves and utilizing the generalized wave impedance theory, a quantitative theoretical analysis of the structural effects caused by the evolution of stress waves during the elastic compression stage of specimens with mismatched bar/specimen cross-sectional areas is conducted. The characteristics and main influencing factors of the deviation between engineering stress-strain curves of specimens during the elastic stage and the actual material stress-strain curves under different conditions are explored. It further reveals the governing rules and mechanisms influencing these deviations. The analysis indicates that for linearly incident loading waves, when the dimensionless time is a multiple of 0.5, even if other parameters change, the engineering stress-strain values of the specimen correspond approximately to the actual material stress-strain values. Even when there is a significant stress difference at both ends of the specimen, if the variation of stress difference tends to stabilize, the difference between the engineering stress-strain curve of the specimen and the actual material stress-strain curve is relatively small. The study calculates the maximum stress deviation value of the specimen and its corresponding dimensionless time, as well as the trend of the maximum stress deviation value of the specimen within different fluctuation intervals. Moreover, the study investigates the scenario where the incident wave is a bilinear combination wave. The results show that when a bilinear incident wave is present, the two linear intervals can be independently analyzed. Regardless of the combination or the variation of stress difference, only when the stress difference at both ends of the specimen reaches an approximately constant curve, the corresponding engineering stress-strain curve of the specimen is relatively accurate. This study provides theoretical references for the refined design of SHPB tests and the accurate data processing.
-
-
[1] LIU F, LI Q M. Strain-rate effect of polymers and correction methodology in a SHPB test [J]. International Journal of Impact Engineering, 2022, 161: 104109. DOI: 10.1016/j.ijimpeng.2021.104109. [2] LIU P, HU D A, WU Q K, et al. Sensitivity and uncertainty analysis of interfacial effect in SHPB tests for concrete-like materials [J]. Construction and Building Materials, 2018, 163: 414–427. DOI: 10.1016/j.conbuildmat.2017.12.118. [3] KARIEM M A, BEYNON J H, RUAN D. Misalignment effect in the split Hopkinson pressure bar technique [J]. International Journal of Impact Engineering, 2012, 47: 60–70. DOI: 10.1016/j.ijimpeng.2012.03.006. [4] NIE H L, MA W F, HE X L, et al. Misalignment tolerance in one-side and symmetric loading Hopkinson pressure bar experiments [J]. Acta Mechanica Solida Sinica, 2022, 35(2): 273–281. DOI: 10.1007/s10338-021-00267-3. [5] GUO Y B, GAO G F, JING L, et al. Dynamic properties of mortar in high-strength concrete [J]. International Journal of Impact Engineering, 2022, 165: 104216. DOI: 10.1016/j.ijimpeng.2022.104216. [6] PANOWICZ R, JANISZEWSKI J, KOCHANOWSKI K. Effects of sample geometry imperfections on the results of split Hopkinson pressure bar experiments [J]. Experimental Techniques, 2019, 43(4): 397–403. DOI: 10.1007/s40799-018-0293-7. [7] BRIZARD D, JACQUELIN E. Uncertainty quantification and global sensitivity analysis of longitudinal wave propagation in circular bars: application to SHPB device [J]. International Journal of Solids and Structures, 2018, 134: 264–271. DOI: 10.1016/j.ijsolstr.2017.11.005. [8] YANG H S, LI Y L, ZHOU F H. Propagation of stress pulses in a Rayleigh-Love elastic rod [J]. International Journal of Impact Engineering, 2021, 153: 103854. DOI: 10.1016/j.ijimpeng.2021.103854. [9] BRAGOV A M, LOMUNOV A K, LAMZIN D A, et al. Dispersion correction in split-Hopkinson pressure bar: theoretical and experimental analysis [J]. Continuum Mechanics and Thermodynamics, 2022, 34(4): 895–907. DOI: 10.1007/s00161-019-00776-0. [10] RIGBY S E, BARR A D, CLAYTON M. A review of Pochhammer-Chree dispersion in the Hopkinson bar [J]. Engineering and Computational Mechanics, 2018, 171(1): 3–13. DOI: 10.1680/jencm.16.00027. [11] REN L, YU X M, HE Y, et al. Numerical investigation of lateral inertia effect in dynamic impact testing of UHPC using a split-Hopkinson pressure bar [J]. Construction and Building Materials, 2020, 246: 118483. DOI: 10.1016/j.conbuildmat.2020.118483. [12] ROTARIU A N, TRANĂ E, MATACHE L. Young’s modulus calculus using split Hopkinson bar tests on long and thin material samples [J]. Materials, 2022, 15(9): 3058. DOI: 10.3390/ma15093058. [13] AGHAYAN S, BIELER S, WEINBERG K. Determination of the high-strain rate elastic modulus of printing resins using two different split Hopkinson pressure bars [J]. Mechanics of Time-Dependent Materials, 2022, 26(4): 761–773. DOI: 10.1007/s11043-021-09511-2. [14] ZHANG Q M, HUANG X M, GUO R, et al. Study on dynamic impact response and optimal constitutive model of Al-Mg-Si aluminum alloy [J]. Materials, 2022, 15(21): 7618. DOI: 10.3390/ma15217618. [15] CHEN J P, TAO W J, HUAN S, et al. Data processing of wave propagation in viscoelastic split Hopkinson pressure bar [J]. AIP Advances, 2022, 12(4): 045210. DOI: 10.1063/5.0083888. [16] ZHOU Z P, GAO D D, LIN G J, et al. Static and dynamic mechanical properties of epoxy nanocomposites reinforced by hybridization with carbon nanofibers and block ionomers [J]. Engineering Fracture Mechanics, 2022, 271: 108638. DOI: 10.1016/j.engfracmech.2022.108638. [17] PRAKASH G, SINGH N K, GUPTA N K. Flow behaviour of Ti-6Al-4V alloy in a wide range of strain rates and temperatures under tensile, compressive and flexural loads [J]. International Journal of Impact Engineering, 2023, 176: 104549. DOI: 10.1016/j.ijimpeng.2023.104549. 期刊类型引用(14)
1. 罗刚,严荔,李文权,杨云生,张宇航,AHMED MD Elias,刘凤玲. 水下爆炸作用下悬浮隧道结构设计与优化. 中国公路学报. 2025(02): 47-59 .  百度学术
百度学术2. 张典典,何晖,石同幸. 爆炸损伤后起波钢筋混凝土梁吸能能力有限元分析. 低温建筑技术. 2024(04): 101-104 .  百度学术
百度学术3. 曾浩,袁鹏程,杨婷,徐慎春,吴成清. 地聚物超高性能混凝土复合板抗接触爆炸试验与数值模拟. 爆炸与冲击. 2024(06): 105-121 .  本站查看
本站查看4. 李爱群,晁磊,刘少波,吴宜峰,杨参天. 泡沫铝复合结构的制备研究进展与展望. 建筑结构. 2024(19): 90-98+168 .  百度学术
百度学术5. 魏广帅,汪维,杨建超,高伟亮. POZD涂覆钢板加固钢筋混凝土板抗爆性能研究. 材料导报. 2023(21): 289-296 .  百度学术
百度学术6. 雷升祥,赵伟,雷宇明. 城市地下空间工程韧性提升研究. 隧道建设(中英文). 2023(10): 1627-1636 .  百度学术
百度学术7. 刘超,孙启鑫,李会驰. 近爆作用下钢筋混凝土π梁防护性能的数值模拟. 振动与冲击. 2022(04): 223-231 .  百度学术
百度学术8. 周宏元,杜文钊,王小娟,张雪健,余尚江,张宏. 地冲击下新型脆断构件防护性能实验研究. 爆炸与冲击. 2022(07): 115-125 .  本站查看
本站查看9. 魏崇一,杨骥,彭春霖,李广帮,廖相巍. 泡沫金属的发展及制备方法. 鞍钢技术. 2022(05): 8-13+23 .  百度学术
百度学术10. 周辉,任辉启,吴祥云,易治,黄魁,穆朝民,王海露. 成层式防护结构中分散层研究综述. 爆炸与冲击. 2022(11): 3-28 .  本站查看
本站查看11. 陈沫衡,张典堂,钱坤,徐阳. 防爆墙材料与结构研究进展. 工程爆破. 2021(05): 93-101 .  百度学术
百度学术12. 张嵩,巴振宁,赵靖轩. 大直径盾构隧道复合泡沫铝保护层抗爆性能研究. 市政技术. 2020(03): 149-152 .  百度学术
百度学术13. 周宏元,李永胜,王小娟,杜建国,余尚江,陈荣华. 地冲击作用下基于泡沫混凝土的地下结构柔性防护. 北京工业大学学报. 2020(06): 533-539 .  百度学术
百度学术14. 刘飞,杨超志,夏明,贾鑫,汪剑辉. 钢筋混凝土板爆炸动态响应研究进展. 防护工程. 2020(05): 1-9 .  百度学术
百度学术其他类型引用(19)
-







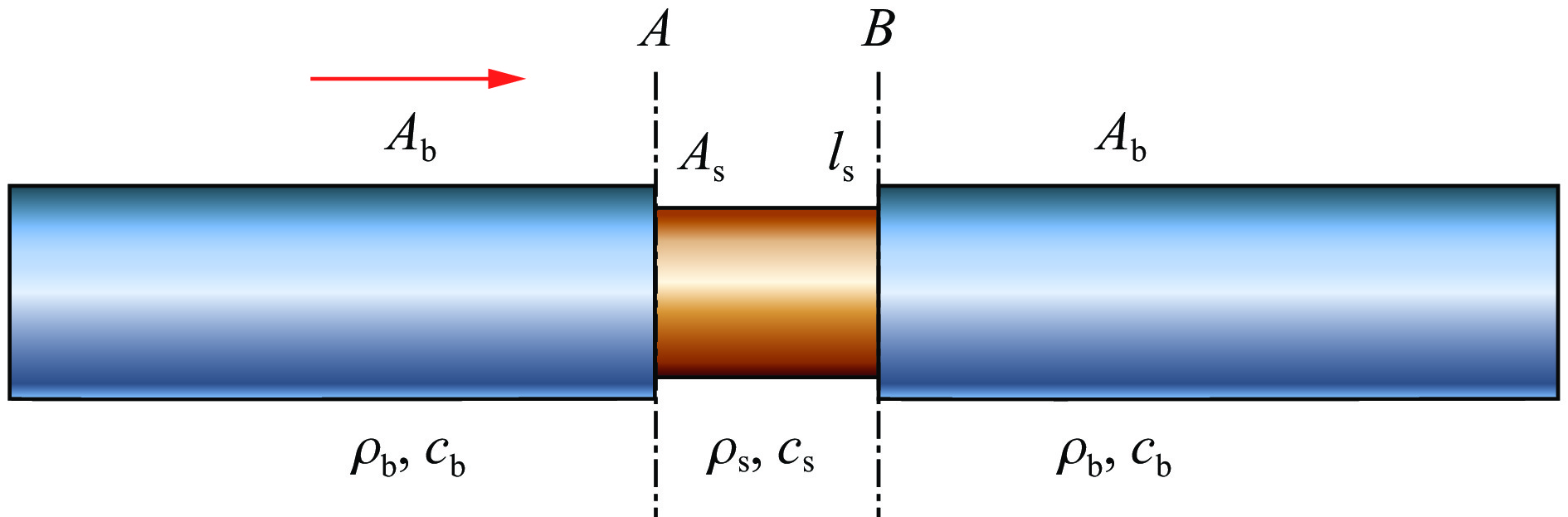
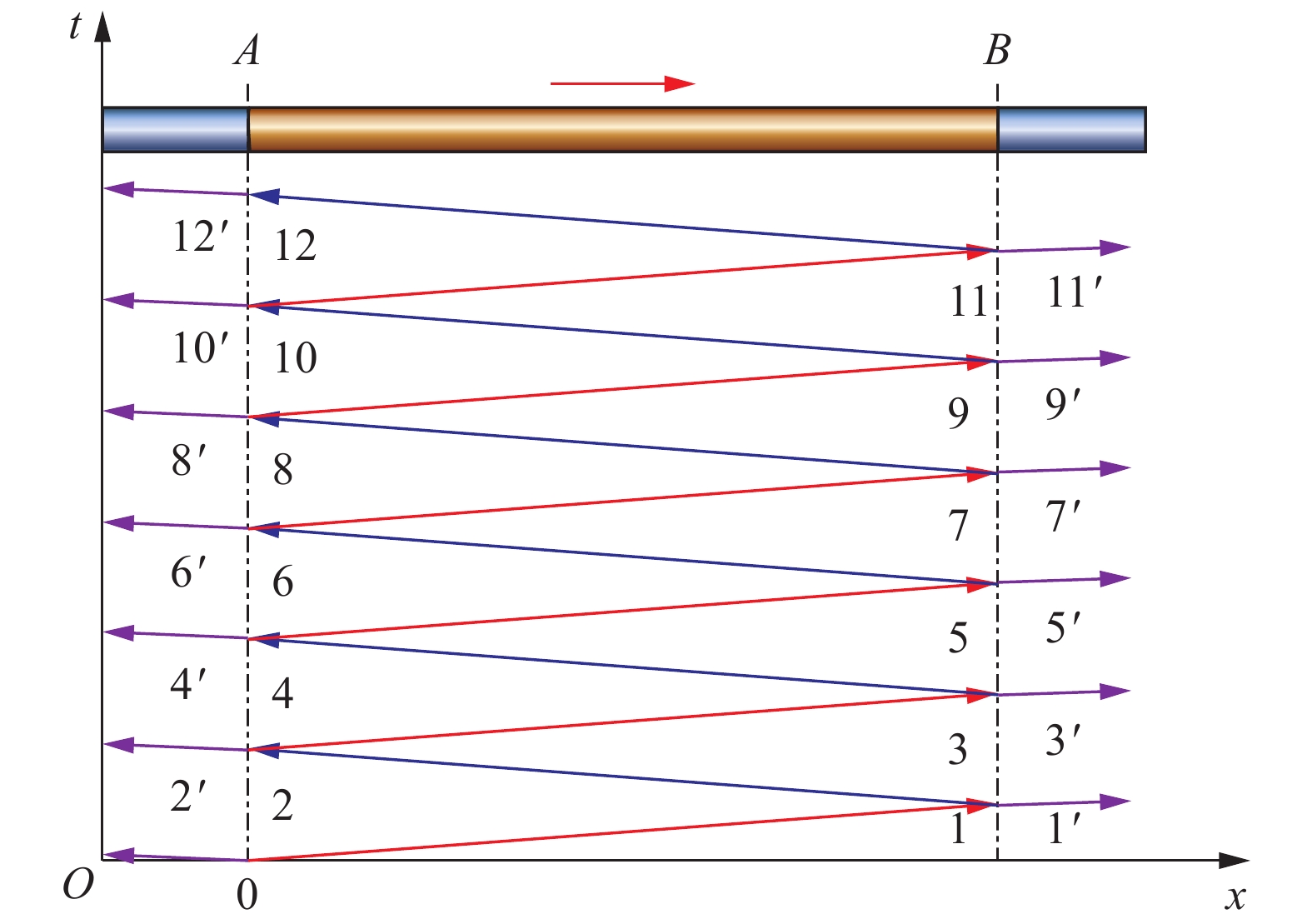
 下载:
下载:

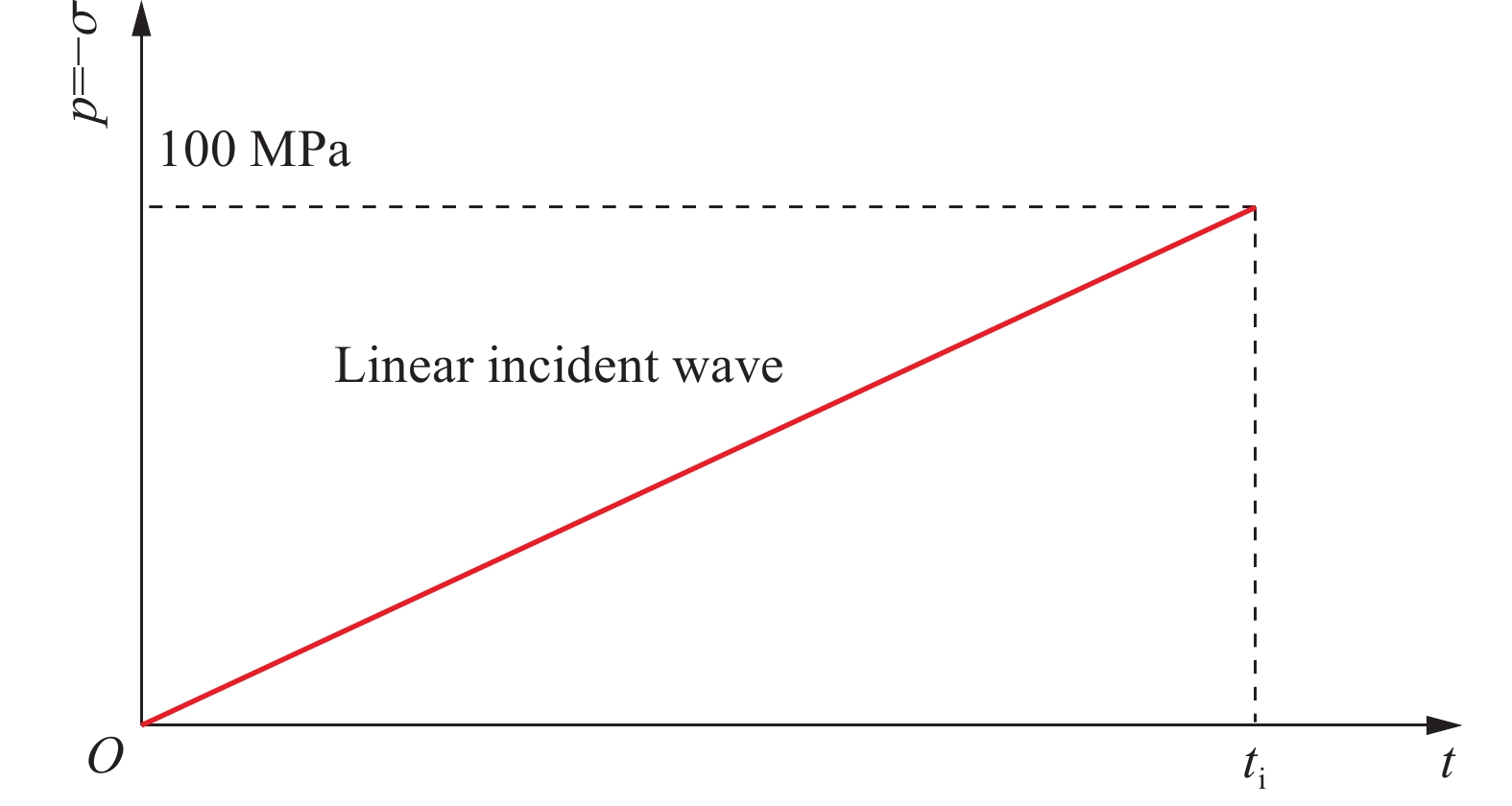
















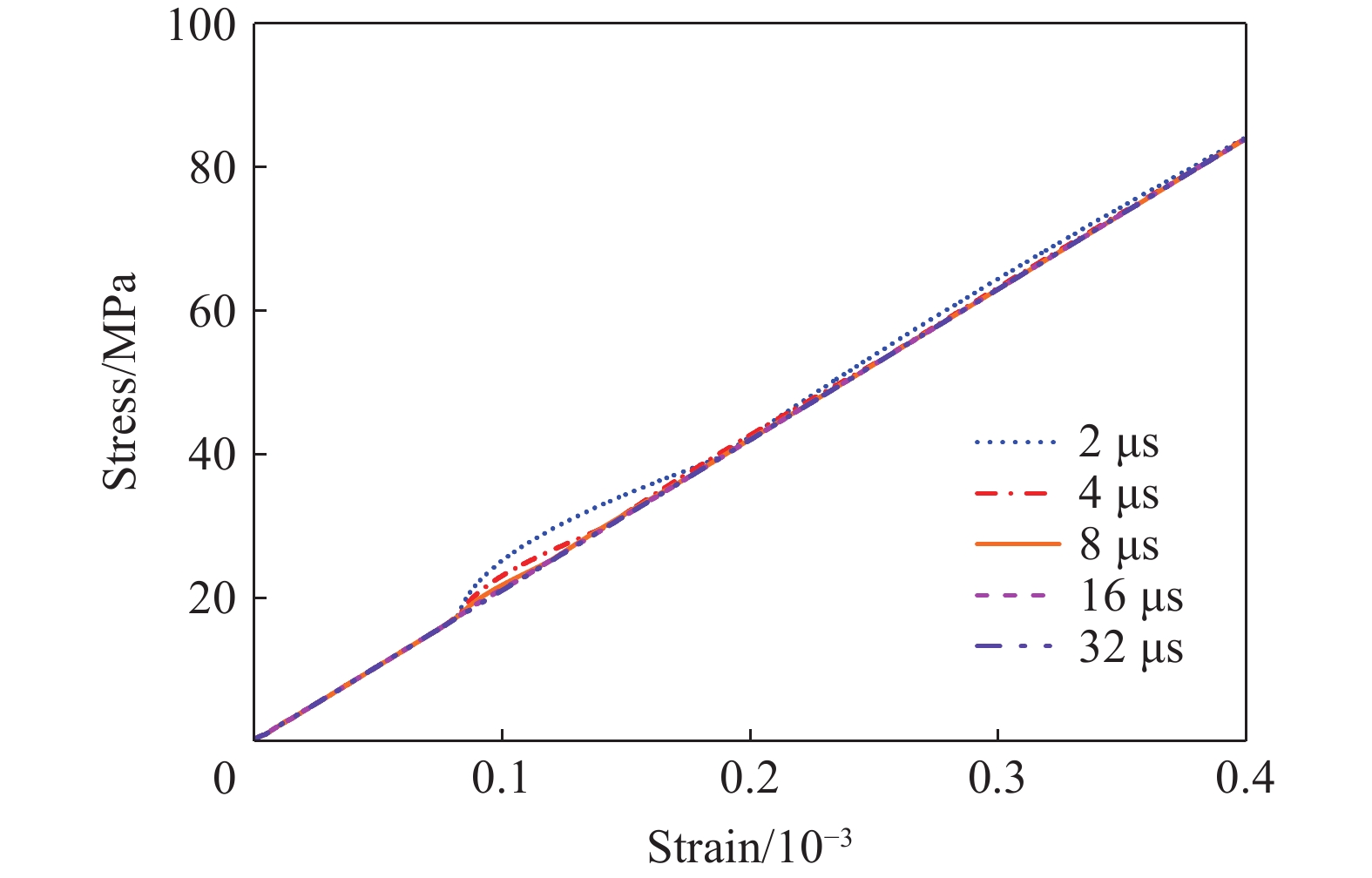
 百度学术
百度学术