多胞子弹冲击泡沫夹芯梁的动力学响应分析

张元瑞; 朱玉东; 王克鸿; 周琦; 虞吉林; 郑志军

doi:10.11883/bzycj-2024-0045

多胞子弹冲击泡沫夹芯梁的动力学响应分析

doi: 10.11883/bzycj-2024-0045

张元瑞^1,,
朱玉东¹,
王克鸿²,
周琦²,
虞吉林¹,
郑志军^{1, 3, ,}

1.
中国科学技术大学近代力学系中国科学院材料力学行为和设计重点实验室，安徽合肥 230027
2.
南京理工大学材料科学与工程学院，江苏南京 210094
3.
中国科学院力学研究所非线性力学国家重点实验室，北京 100190

基金项目: 国家自然科学基金（11872360）

详细信息

作者简介:
张元瑞（1997－　），女，博士，yrzhang1@ustc.edu.cn

通讯作者:
郑志军（1979－　），男，博士，副教授，zjzheng@ustc.edu.cn

中图分类号: O389
计量
- 文章访问数: 159
- HTML全文浏览量: 55
- PDF下载量: 94
- 被引次数: 0
出版历程
- 收稿日期: 2024-01-29
- 修回日期: 2024-04-05
- 网络出版日期: 2024-04-07
- 刊出日期: 2024-09-20

Dynamic response analysis of cellular projectile impacting foam sandwich beam

1.
CAS Key Laboratory of Mechanical Behavior and Design of Materials, Department of Modern Mechanics, University of Science and Technology of China, Hefei 230027, Anhui, China
2.
School of Materials Science and Engineering, Nanjing University of Science and Technology, Nanjing 210094, Jiangsu, China
3.
State Key Laboratory of Nonlinear Mechanics, Institute of Mechanics, Chinese Academy of Science, Beijing 100190, China

摘要

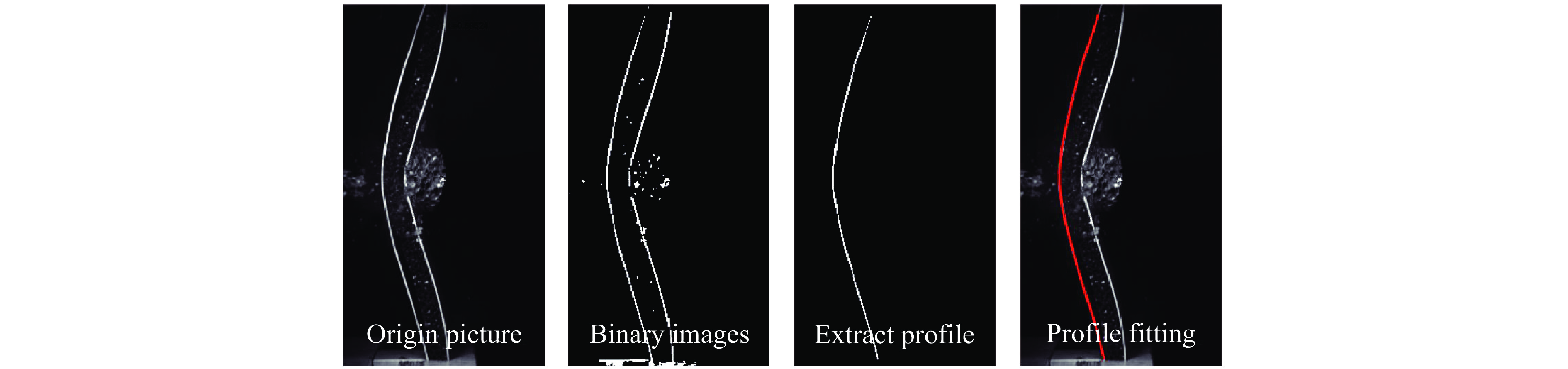
摘要: 为了研究均匀/梯度多胞子弹冲击泡沫夹芯梁的耦合响应过程和多胞子弹对夹芯梁的加载效果，对该冲击过程开展了理论分析、数值模拟和试验研究：通过将泡沫夹芯梁等效为单梁以简化分析，基于多胞子弹的冲击波模型和泡沫夹芯梁的等效单梁响应模型，构建了多胞子弹冲击泡沫夹芯梁的耦合分析模型，给出了冲击过程中各响应阶段的控制方程，并结合龙格-库塔方法对方程进行了数值求解；基于三维Voronoi技术，开展了均匀/梯度多胞子弹冲击泡沫夹芯梁的细观有限元模拟；在多胞子弹的冲击测试平台上进行了试验研究，结合高速摄影技术获取了多胞子弹和泡沫夹芯梁的速度响应。结果表明：耦合分析模型可以准确地预测多胞子弹和泡沫夹芯梁的速度历程曲线以及多胞子弹产生的冲击压强；在初始动量相同但密度分布或初速度不同的多胞子弹冲击下，同一构型的泡沫夹芯梁展现出不同的力学响应，这说明多胞子弹的加载不能简单地等效为脉冲加载，多胞子弹与夹芯梁之间的耦合效应不可忽略；相较于均匀多胞子弹，梯度多胞子弹的冲击压力波形更加尖锐，在其衰减过程中展现出更强的非线性特征。
- 多胞子弹 /
- 泡沫夹芯梁 /
- 耦合分析模型 /
- 有限元模拟 /
- 冲击测试
Abstract: Cellular projectiles are widely used in the impact tests of protective structures, but the actual loads of cellular projectiles acting on the tested sandwich structures are still unclear. To explore the coupling response process between the uniform/graded cellular projectile and the foam sandwich beam and the loading effect of cellular projectiles, theoretical analysis, numerical simulations, and impact tests were carried out. The foam sandwich beam was equivalent to a monolithic beam to simplify the analysis. Based on the shock wave model of the cellular projectile and the equivalent response model of the foam sandwich beam, a coupling analysis model of the cellular projectile impacting the foam sandwich beam was developed, and its governing equations were presented and solved numerically by the Runge-Kutta method. Meso-finite element simulations of a uniform/graded cellular projectile impacting a foam sandwich beam were carried out based on the 3D Voronoi technique. Impact tests were performed on the test platform of cellular projectiles, and the velocity response of the cellular projectiles and the foam sandwich beams was obtained by using a high-speed camera and a digital image processing technique. It is found that the coupling analysis model can accurately predict the velocity history curves of the cellular projectile and the foam sandwich beam, as well as the impact pressure of the cellular projectile. Subjected to cellular projectiles with the same initial momentum but different density distribution or initial velocity, foam sandwich beams with the same configuration present different mechanical response processes, which demonstrates that the impact of cellular projectiles cannot be simply equivalent to impulse loading, and the coupling effect between the projectile and the sandwich beam cannot be ignored. Compared with uniform cellular projectiles, the impact pressure waveform of the graded cellular projectile is sharper and shows stronger nonlinearity during its attenuation. This study clarifies the loading effect of cellular projectiles on foam sandwich beams and lays a theoretical foundation for the optimal design of cellular projectiles simulating blast loads.
- cellular projectile /
- foam sandwich beam /
- coupling analysis model /
- finite element simulation /
- impact test

HTML全文

图 1 梯度多胞子弹冲击泡沫夹芯梁的示意图

Figure 1. Schematic diagrams of a graded cellular projectile impacting a foam sandwich beam

下载: 全尺寸图片幻灯片

图 2 夹芯梁的等效单梁的速度场在冲击过程中的5个相

Figure 2. Five phases of the velocity field of the equivalent monolithic beam for the sandwich beam during the impact process

下载: 全尺寸图片幻灯片

图 3 泡沫铝的准静态单轴压缩应力-应变曲线和和面/背梁材料6061-T6铝的准静态拉伸应力-应变曲线

Figure 3. The quasi-static uniaxial compression stress-strain curves of aluminum foam and the quasi-static uniaxial tensile stress-strain curve of 6061-T6 aluminum

下载: 全尺寸图片幻灯片

图 4 均匀多胞子弹试件以及夹芯梁试件

Figure 4. Test specimens of uniform cellular projectiles and foam sandwich beam

下载: 全尺寸图片幻灯片

图 5 多胞子弹冲击泡沫铝夹芯梁的测试平台

Figure 5. A test platform for cellular projectile impacting a clamped foam sandwich beam

下载: 全尺寸图片幻灯片

图 6 高速摄影图像的数据处理方法

Figure 6. Data processing method of high-speed photography images

下载: 全尺寸图片幻灯片

图 7 多胞子弹的有限元模型及夹芯梁芯层网格敏感性分析

Figure 7. The finite element models of different cellular projectiles and grid sensitivity analysis of sandwich beam core

下载: 全尺寸图片幻灯片

图 8 有限元模拟中多胞子弹冲击泡沫铝夹芯梁的变形过程

Figure 8. The deformation process of the foam sandwich beam under the impact of the cellular projectile in the simulation

下载: 全尺寸图片幻灯片

图 9 UCP-2冲击泡沫夹芯梁的速度历程曲线

Figure 9. The history of the mid-span velocity of the foam sandwich beam impacted by projectile UCP-2

下载: 全尺寸图片幻灯片

图 10 UCP-2冲击下泡沫夹芯梁的跨中挠度和承载历程曲线

Figure 10. The history of the mid-span deflection and the impact pressure of the foam sandwich beam impacted by projectile UCP-2

下载: 全尺寸图片幻灯片

图 11 等初始动量的均匀/梯度多胞子弹冲击下泡沫夹芯梁的跨中速度、跨中挠度和承载历程曲线

Figure 11. The mid-span velocity, deflection, and impact pressure of foam sandwich beam impacted by uniform/graded cellular projectiles with the same initial momentum

下载: 全尺寸图片幻灯片

图 12 UCP-30和UCP-60冲击固支泡沫铝夹芯梁的变形过程

Figure 12. The deformation process of the clamped foam sandwich beams impacted by projectiles UCP-30 and UCP-60

下载: 全尺寸图片幻灯片

图 13 UCP-30和UCP-60冲击下泡沫夹芯梁的速度响应

Figure 13. The velocity response of the foam sandwich beams impacted by projectiles UCP-30 and UCP-60

下载: 全尺寸图片幻灯片

图 14 UCP-30和UCP-60子弹冲击下泡沫夹芯梁的最终变形

Figure 14. The final deformation configurations of the foam sandwich beams impacted by projectiles UCP-30 and UCP-60

下载: 全尺寸图片幻灯片

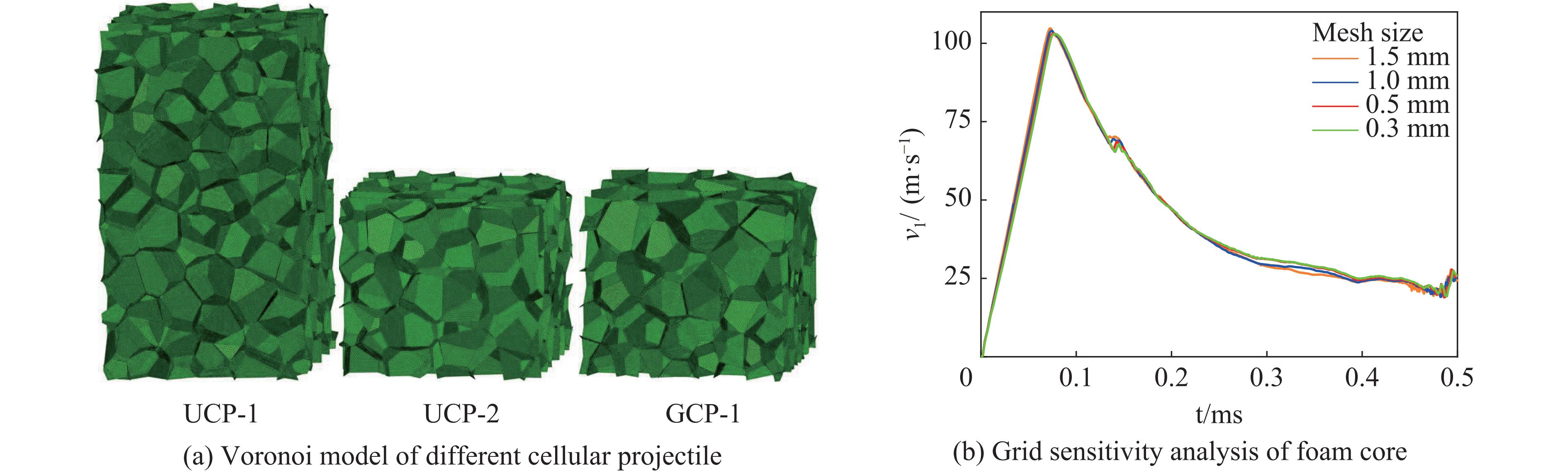
表 1 有限元模拟和实验中多胞子弹的参数

Table 1. Parameters of cellular projectiles in finite element simulations and experimental tests

方法	子弹编号	ρ₀	ρ_e	l₀/mm	v₀/(m·s⁻¹)	I/(N·s)
有限元模拟	UCP-1	0.15	0.15	60	100	2.98
	UCP-2	0.15	0.15	30	200	2.98
	GCP-1	0.20	0.10	30	200	2.98
实验测试	UCP-30	0.11	0.11	30	215	2.00
实验测试	UCP-60	0.11	0.11	60	104	1.94
注：I为冲量.

下载: 导出CSV

参考文献(33)

[1]	GUO H Y, YUAN H, ZHANG J X, et al. Review of sandwich structures under impact loadings: experimental, numerical and theoretical analysis [J]. Thin-Walled Structures, 2024, 196: 111541. DOI: 10.1016/j.tws.2023.111541.
[2]	GAN E C J, REMENNIKOV A, RITZEL D, et al. Approximating a far-field blast environment in an advanced blast simulator for explosion resistance testing [J]. International Journal of Protective Structures, 2020, 11(4): 468–493. DOI: 10.1177/2041419620911133.
[3]	ESPINOSA H D, LEE S, MOLDOVAN N. A novel fluid structure interaction experiment to investigate deformation of structural elements subjected to impulsive loading [J]. Experimental Mechanics, 2006, 46(6): 805–824. DOI: 10.1007/s11340-006-0296-7.
[4]	GAN E C J, REMENNIKOV A, RITZEL D. Investigation of trees as natural protective barriers using simulated blast environment [J]. International Journal of Impact Engineering, 2021, 158: 104004. DOI: 10.1016/j.ijimpeng.2021.104004.
[5]	REID S R, PENG C. Dynamic uniaxial crushing of wood [J]. International Journal of Impact Engineering, 1997, 19(5/6): 531–570. DOI: 10.1016/S0734-743X(97)00016-X.
[6]	RADFORD D D, DESHPANDE V S, FLECK N A. The use of metal foam projectiles to simulate shock loading on a structure [J]. International Journal of Impact Engineering, 2005, 31(9): 1152–1171. DOI: 10.1016/j.ijimpeng.2004.07.012.
[7]	RADFORD D D, FLECK N A, DESHPANDE V S. The response of clamped sandwich beams subjected to shock loading [J]. International Journal of Impact Engineering, 2006, 32(6): 968–987. DOI: 10.1016/j.ijimpeng.2004.08.007.
[8]	RATHBUN H J, RADFORD D D, XUE Z, et al. Performance of metallic honeycomb-core sandwich beams under shock loading [J]. International Journal of Solids and Structures, 2006, 43(6): 1746–1763. DOI: 10.1016/j.ijsolstr.2005.06.079.
[9]	TAGARIELLI V L, DESHPANDE V S, FLECK N A. The dynamic response of composite sandwich beams to transverse impact [J]. International Journal of Solids and Structures, 2007, 44(7/8): 2442–2457. DOI: 10.1016/j.ijsolstr.2006.07.015.
[10]	RUBINO V, DESHPANDE V S, FLECK N A. The dynamic response of end-clamped sandwich beams with a Y-frame or corrugated core [J]. International Journal of Impact Engineering, 2008, 35(8): 829–844. DOI: 10.1016/j.ijimpeng.2007.10.006.
[11]	JING L, WANG Z H, NING J G, et al. The dynamic response of sandwich beams with open-cell metal foam cores [J]. Composites Part B: Engineering, 2011, 42(1): 1–10. DOI: 10.1016/j.compositesb.2010.09.024.
[12]	JING L, WANG Z H, NING J G, et al. The mechanical response of metallic sandwich beams under foam projectile impact loading [J]. Latin American Journal of Solids and Structures, 2011, 8(1): 107–120. DOI: 10.1590/S1679-78252011000100006.
[13]	JING L, WANG Z H, ZHAO L M. The dynamic response of sandwich panels with cellular metal cores to localized impulsive loading [J]. Composites Part B: Engineering, 2016, 94: 52–63. DOI: 10.1016/j.compositesb.2016.03.035.
[14]	JING L, WANG Z H, ZHAO L M. Response of metallic cylindrical sandwich shells subjected to projectile impact: experimental investigations [J]. Composite Structures, 2014, 107: 36–47. DOI: 10.1016/j.compstruct.2013.07.011.
[15]	LI X, LI S Q, WANG Z H, et al. Response of aluminum corrugated sandwich panels under foam projectile impact: experiment and numerical simulation [J]. Journal of Sandwich Structures & Materials, 2017, 19(5): 595–615. DOI: 10.1177/1099636216630503.
[16]	LI X, ZHANG P W, LI S Q, et al. Dynamic response of aluminum honeycomb sandwich panels under foam projectile impact [J]. Mechanics of Advanced Materials and Structures, 2018, 25(8): 637–646. DOI: 10.1080/15376494.2017.1308595.
[17]	ZHAO Z, JING L. The response of clamped sandwich panels with layered-gradient aluminum foam cores to foam projectile impact [J]. Mechanics of Advanced Materials and Structures, 2020, 27(9): 744–753. DOI: 10.1080/15376494.2018.1495790.
[18]	魏建辉, 李旭, 黄威, 等. 高速冲击载荷下梯度金属泡沫夹芯梁的动态响应与失效 [J]. 爆炸与冲击, 2023, 43(5): 053301. DOI: 10.11883/bzycj-2022-0156. WEI J H, LI X, HUANG W, et al. Dynamic response and failure of sandwich beams with graded metal foam core under high-velocity impact [J]. Explosion and Shock Waves, 2023, 43(5): 053301. DOI: 10.11883/bzycj-2022-0156.
[19]	ZHANG J X, ZHU Y Q, LI K K, et al. Dynamic response of sandwich plates with GLARE face-sheets and honeycomb core under metal foam projectile impact: Experimental and numerical investigations [J]. International Journal of Impact Engineering, 2022, 164: 104201. DOI: 10.1016/j.ijimpeng.2022.104201.
[20]	XIAO D B, CHEN X Q, LI Y, et al. The structure response of sandwich beams with metallic auxetic honeycomb cores under localized impulsive loading: experiments and finite element analysis [J]. Materials & Design, 2019, 176: 107840. DOI: 10.1016/j.matdes.2019.107840.
[21]	LI Y, CHEN Z H, XIAO D B, et al. The dynamic response of shallow sandwich arch with auxetic metallic honeycomb core under localized impulsive loading [J]. International Journal of Impact Engineering, 2020, 137: 103442. DOI: 10.1016/j.ijimpeng.2019.103442.
[22]	CHEN Z H, LIU L W, GAO S L, et al. Dynamic response of sandwich beam with star-shaped reentrant honeycomb core subjected to local impulsive loading [J]. Thin-Walled Structures, 2021, 161: 107420. DOI: 10.1016/j.tws.2020.107420.
[23]	YU R P, WANG X, ZHANG Q C, et al. Effects of sand filling on the dynamic response of corrugated core sandwich beams under foam projectile impact [J]. Composites Part B: Engineering, 2020, 197: 108135. DOI: 10.1016/j.compositesb.2020.108135.
[24]	WANG X, YU R P, ZHANG Q C, et al. Dynamic response of clamped sandwich beams with fluid-filled corrugated cores [J]. International Journal of Impact Engineering, 2020, 139: 103533. DOI: 10.1016/j.ijimpeng.2020.103533.
[25]	张元瑞, 朱玉东, 郑志军, 等. 泡沫子弹冲击固支单梁的耦合分析模型 [J]. 力学学报, 2022, 54(8): 2161–2172. DOI: 10.6052/0459-1879-22-223. ZHANG Y R, ZHU Y D, ZHENG Z J, et al. A coupling analysis model of clamped monolithic beam impacted by foam projectiles [J]. Chinese Journal of Theoretical and Applied Mechanics, 2022, 54(8): 2161–2172. DOI: 10.6052/0459-1879-22-223.
[26]	RADFORD D D, MCSHANE G J, DESHPANDE V S, et al. The response of clamped sandwich plates with metallic foam cores to simulated blast loading [J]. International Journal of Solids and Structures, 2006, 43(7/8): 2243–2259. DOI: 10.1016/j.ijsolstr.2005.07.006.
[27]	WANG Z H, JING L, NING J G, et al. The structural response of clamped sandwich beams subjected to impact loading [J]. Composite Structures, 2011, 93(4): 1300–1308. DOI: 10.1016/j.compstruct.2010.05.011.
[28]	QIU X, DESHPANDE V S, FLECK N A. Impulsive loading of clamped monolithic and sandwich beams over a central patch [J]. Journal of the Mechanics and Physics of Solids, 2005, 53(5): 1015–1046. DOI: 10.1016/j.jmps.2004.12.004.
[29]	YUE Z S, WANG X, HE C, et al. Elevated shock resistance of all-metallic sandwich beams with honeycomb-supported corrugated cores [J]. Composites Part B: Engineering, 2022, 242: 110102. DOI: 10.1016/j.compositesb.2022.110102.
[30]	ZHENG Z J, WANG C F, YU J L, et al. Dynamic stress-strain states for metal foams using a 3D cellular model [J]. Journal of the Mechanics and Physics of Solids, 2014, 72: 93–114. DOI: 10.1016/j.jmps.2014.07.013.
[31]	YANG J, WANG S L, DING Y Y, et al. Crashworthiness of graded cellular materials: A design strategy based on a nonlinear plastic shock model [J]. Materials Science and Engineering: A, 2017, 680: 411–420. DOI: 10.1016/j.msea.2016.11.010.
[32]	LI Q M, JONES N. Shear and adiabatic shear failures in an impulsively loaded fully clamped beam [J]. International Journal of Impact Engineering, 1999, 22(6): 589–607. DOI: 10.1016/S0734-743X(99)00013-5.
[33]	ZHANG Y R, ZHU Y D, CHANG B X, et al. Blast-loading simulators: Multiscale design of graded cellular projectiles considering projectile-beam coupling effect [J]. Journal of the Mechanics and Physics of Solids, 2023, 180: 105402. DOI: 10.1016/j.jmps.2023.105402.