Structure and propagation mode of gaseous spinning detonation in rectangular tube
-
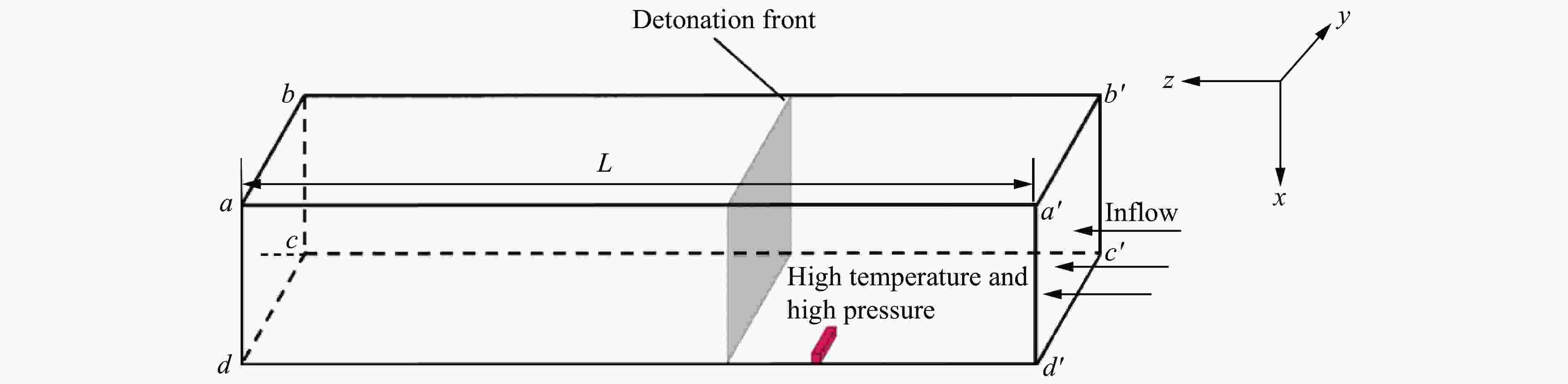
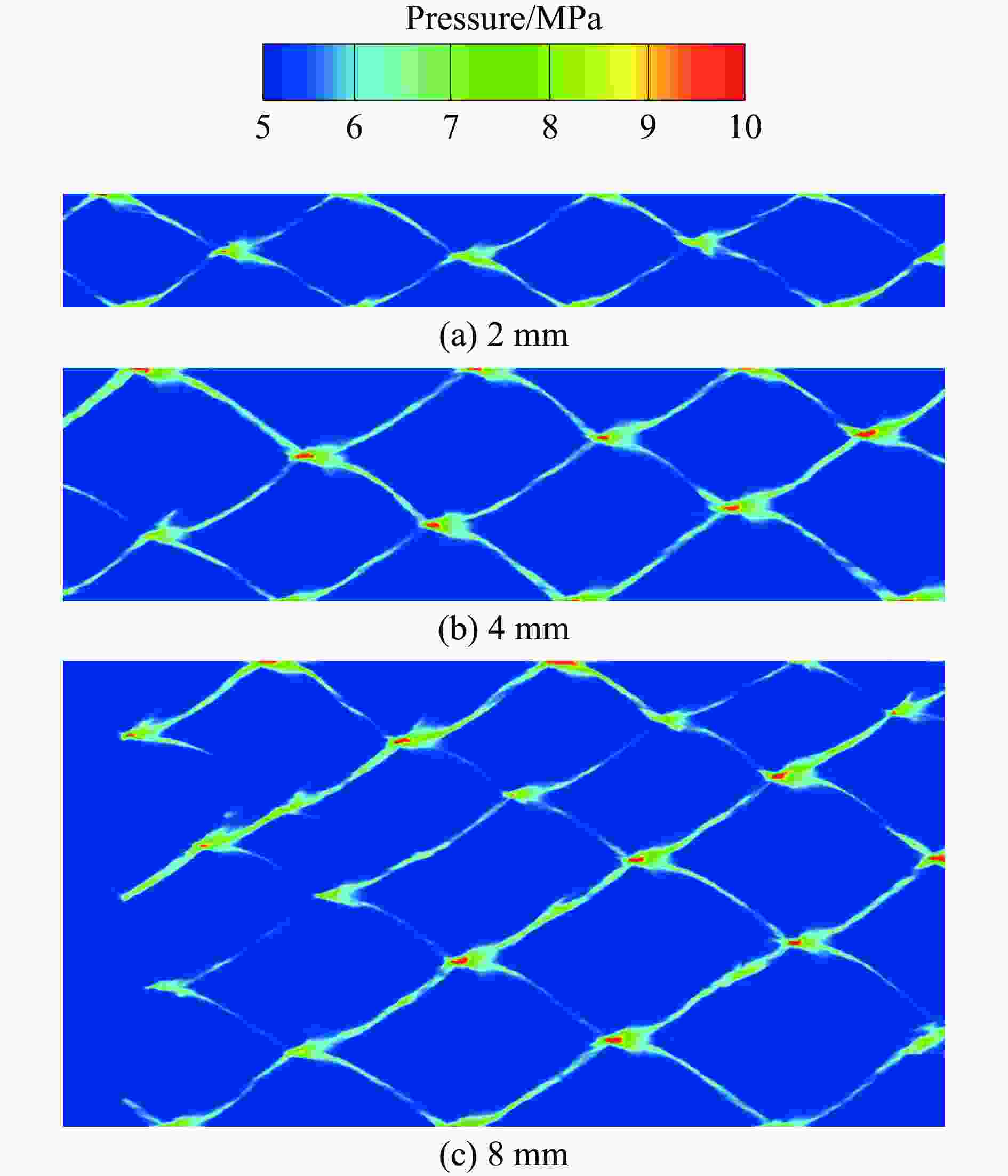
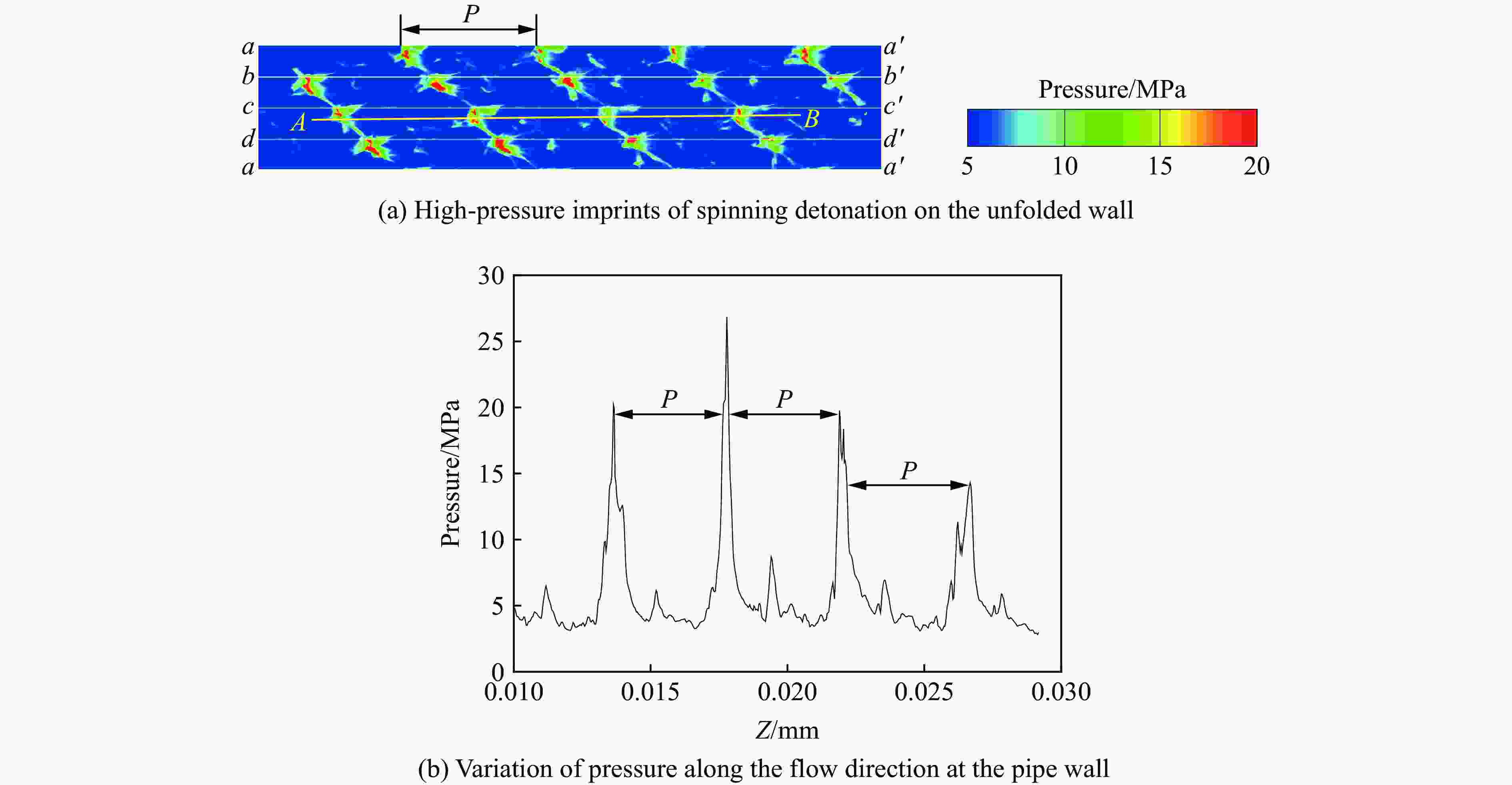
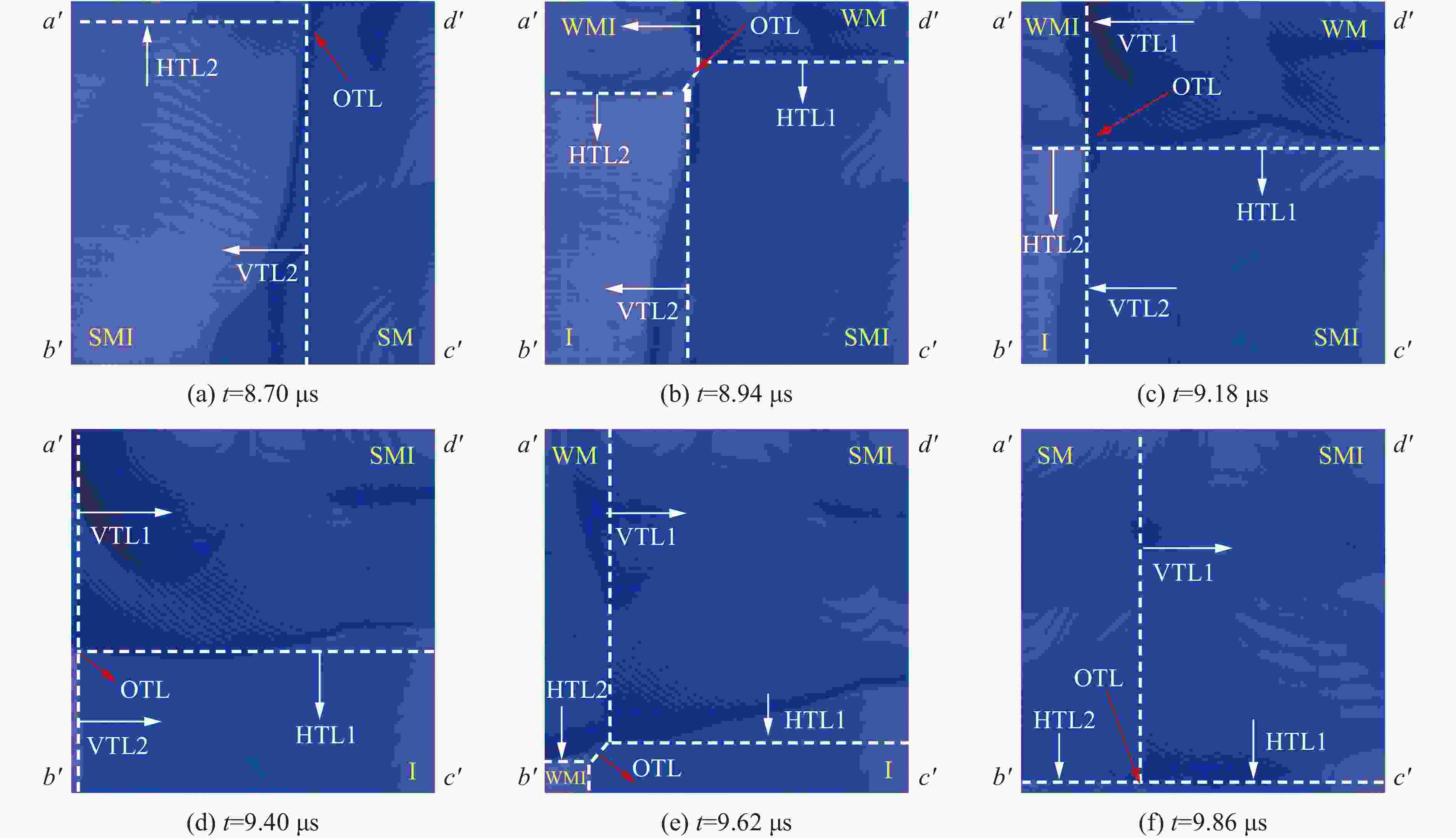
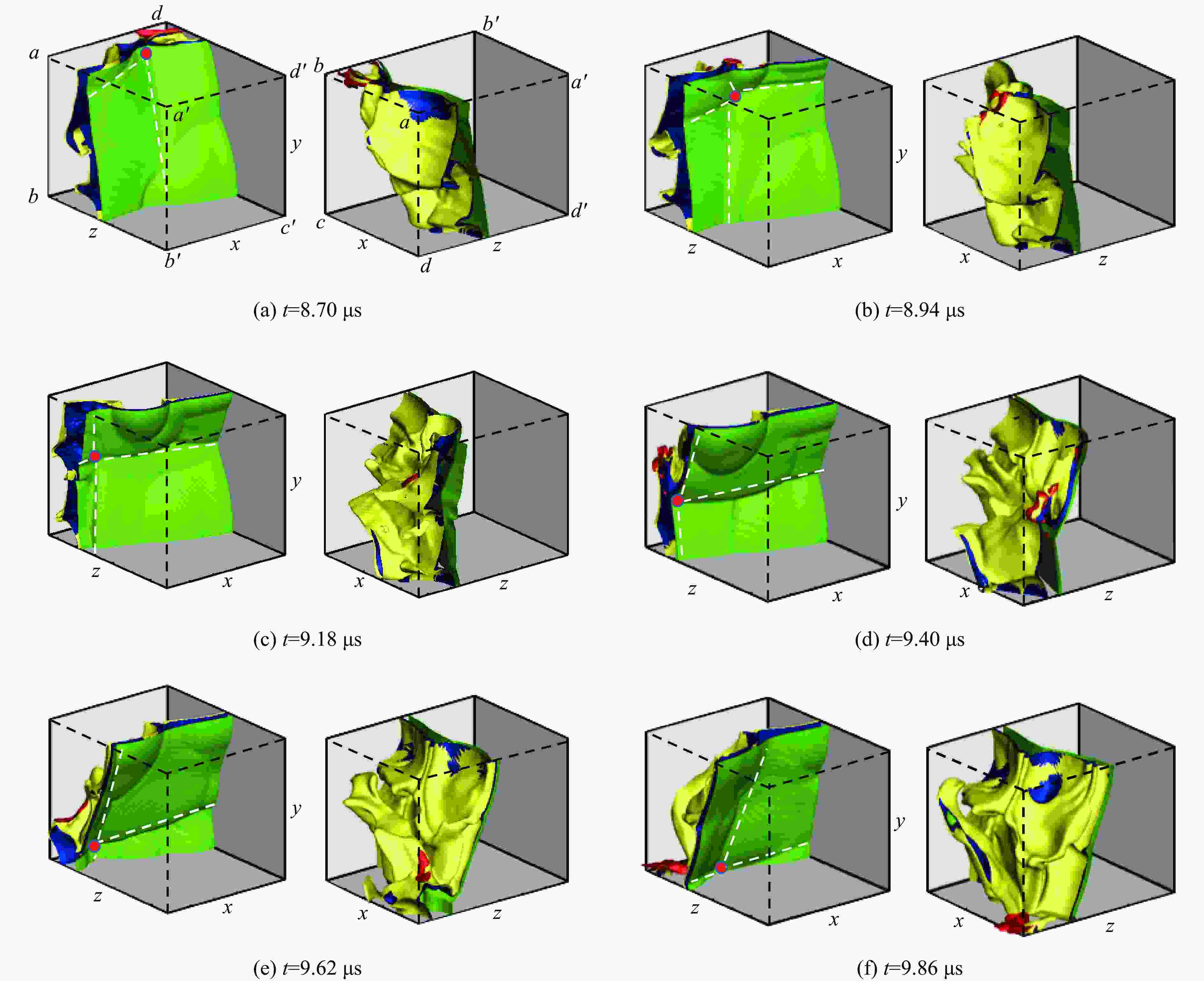
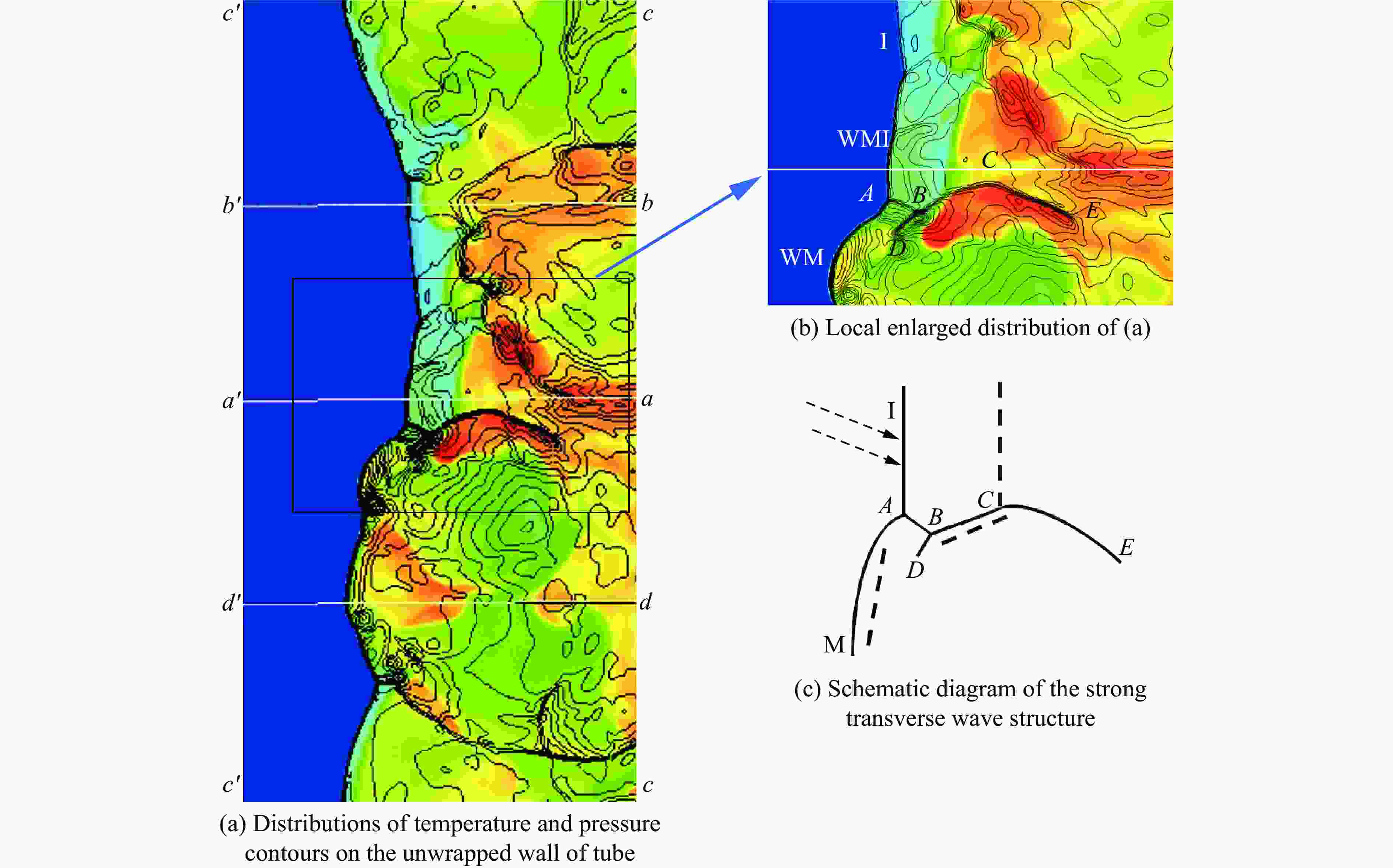
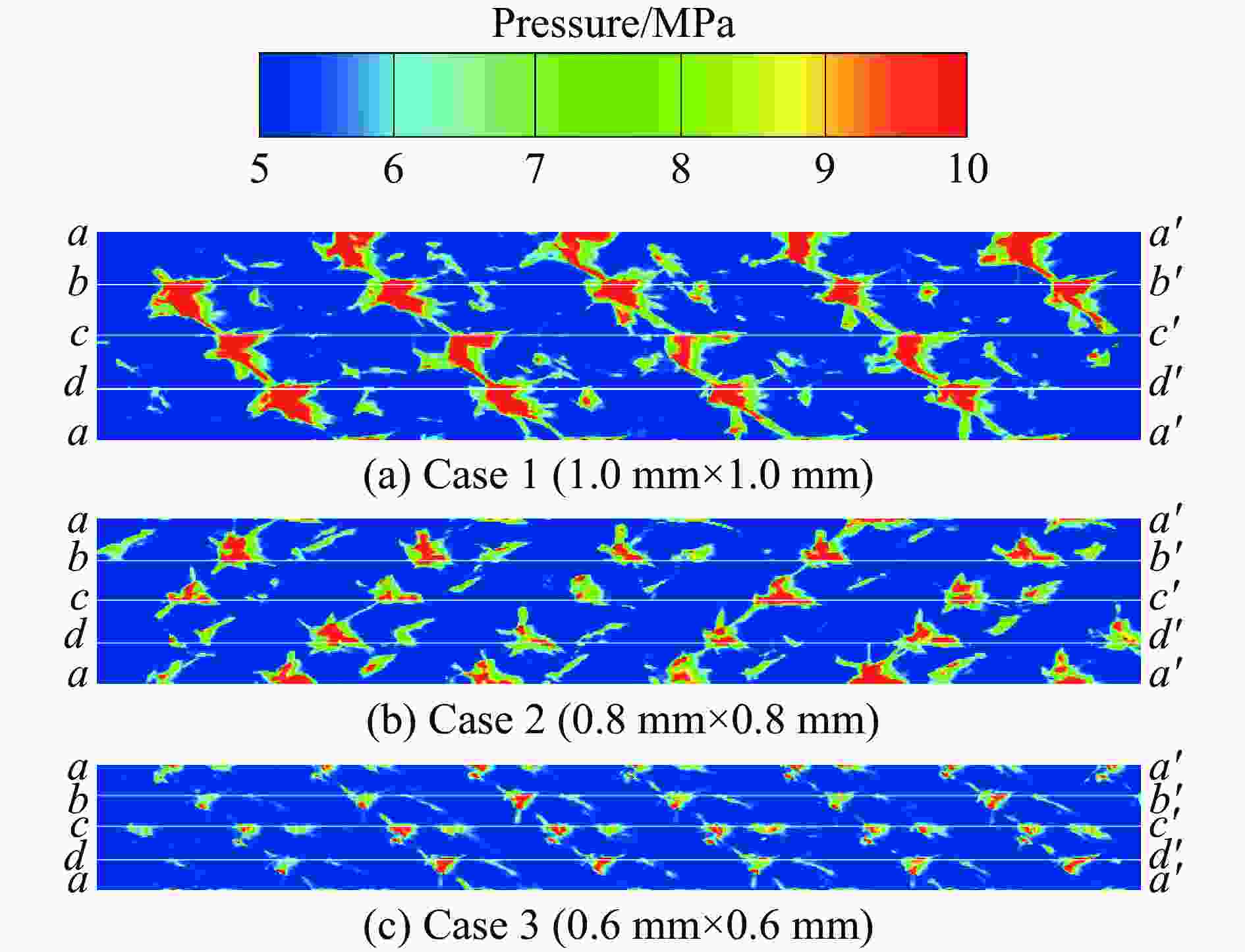
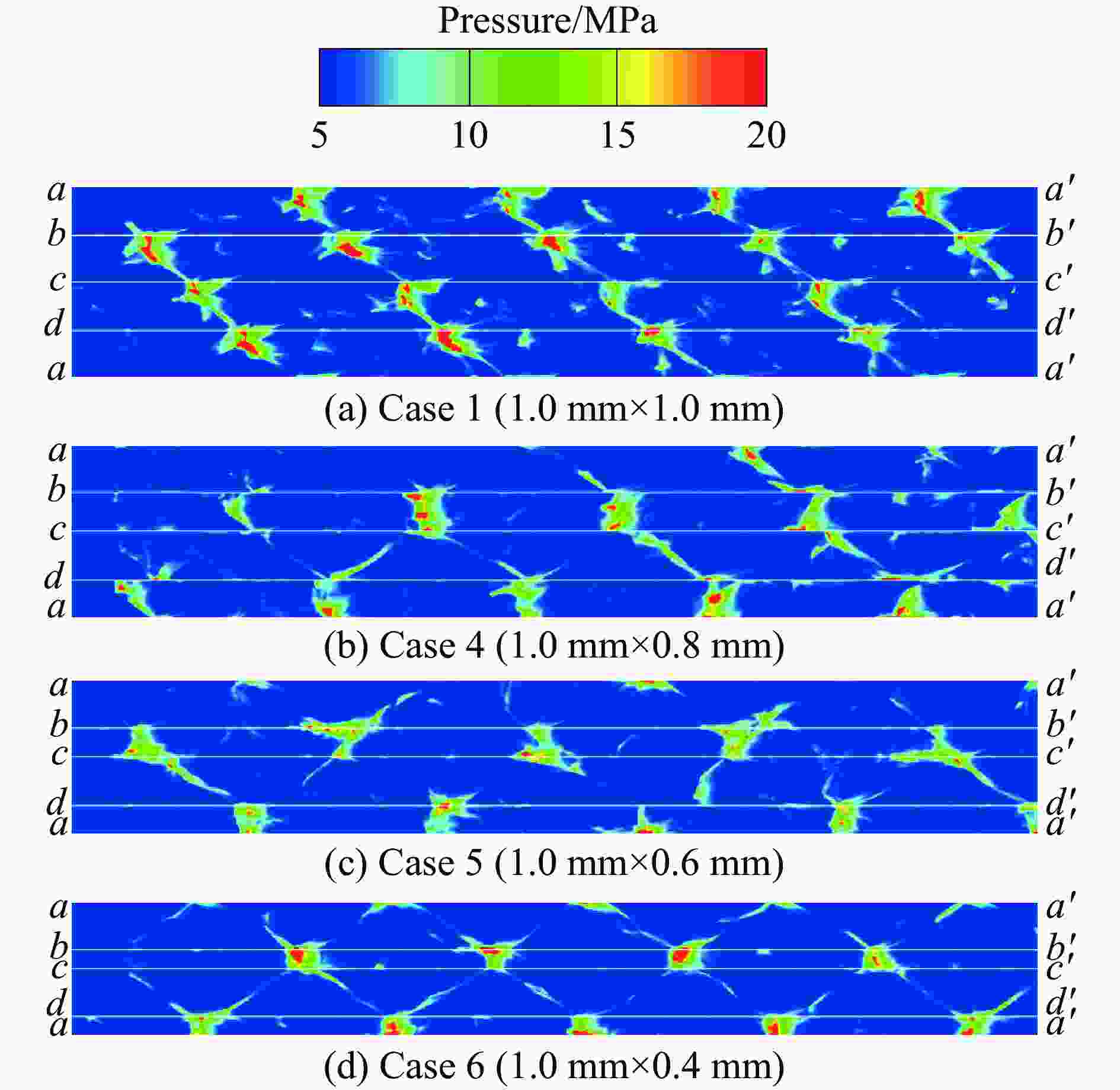
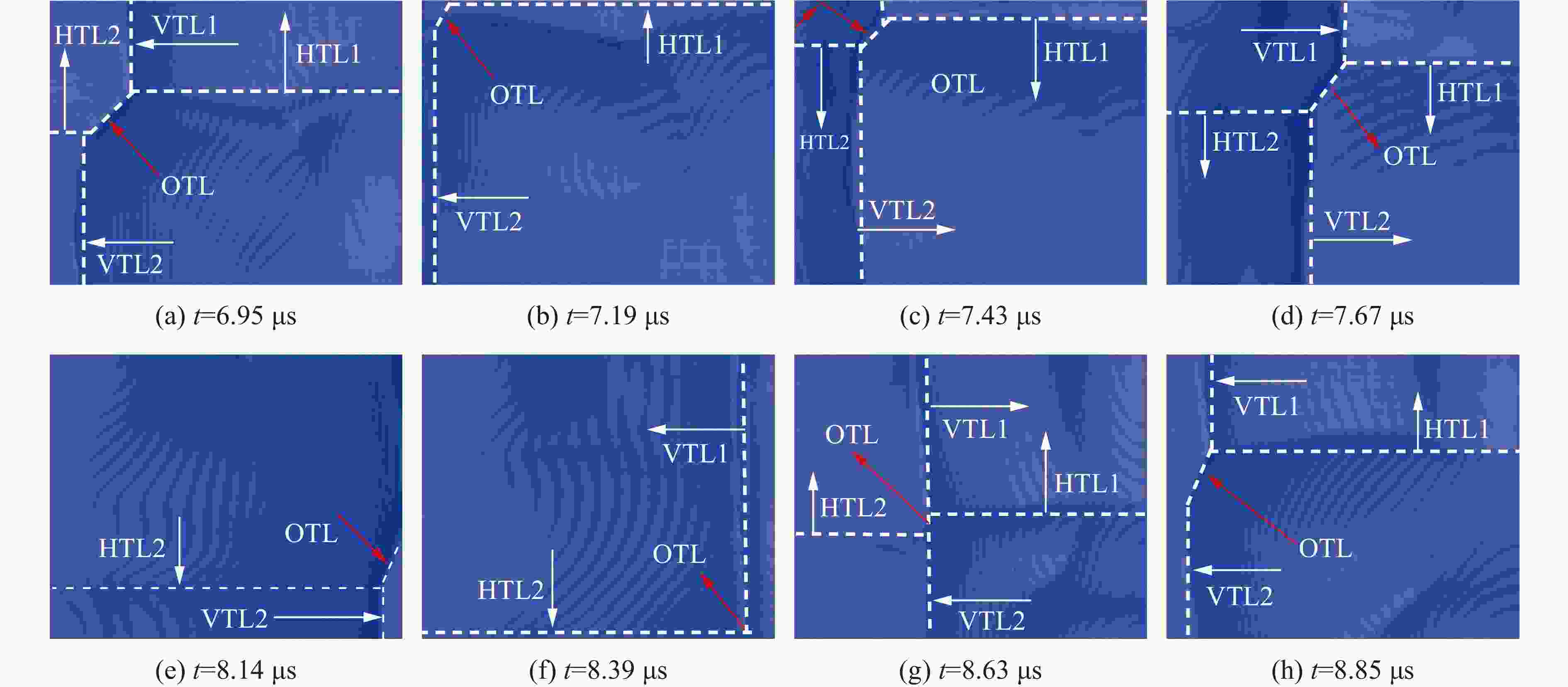
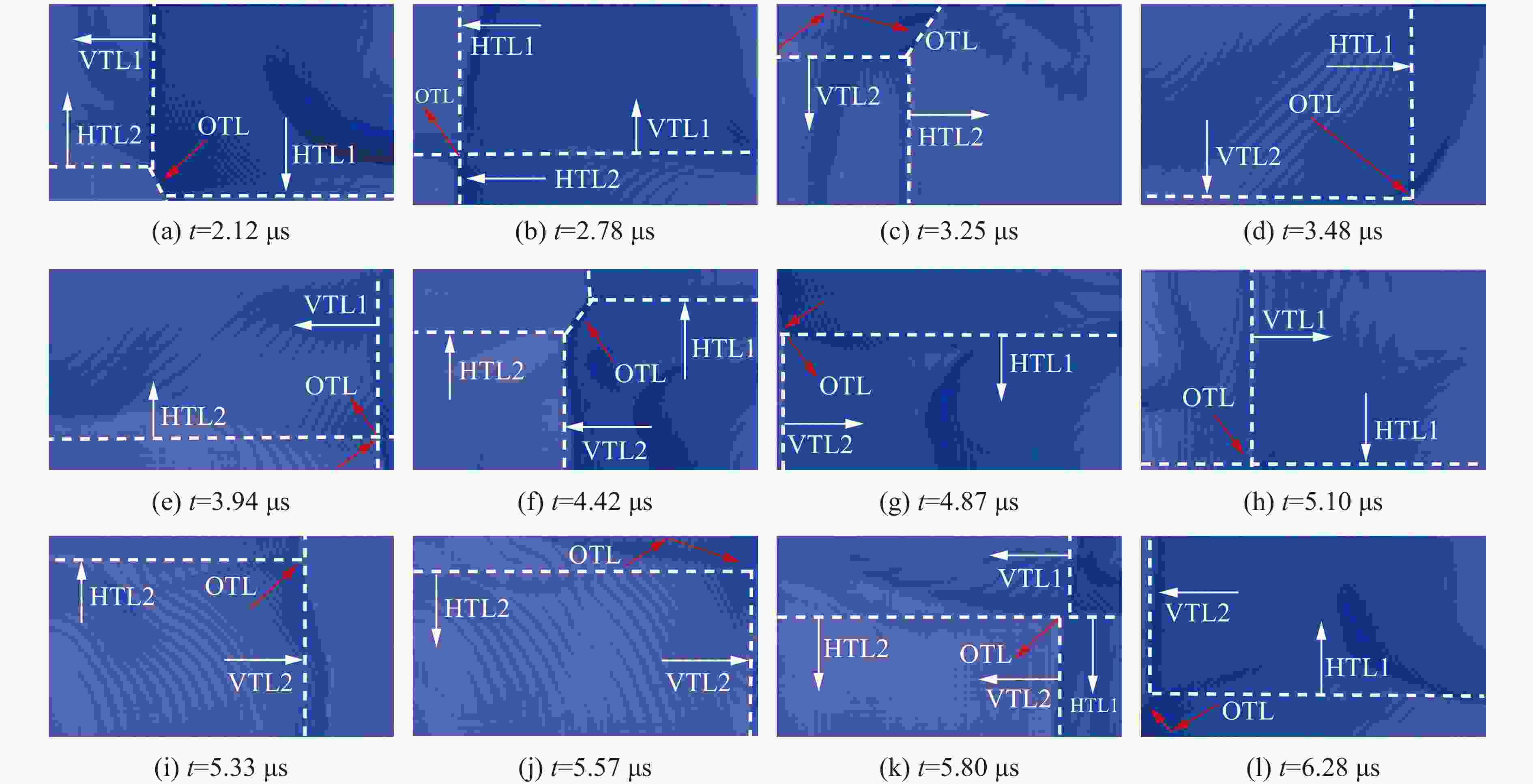
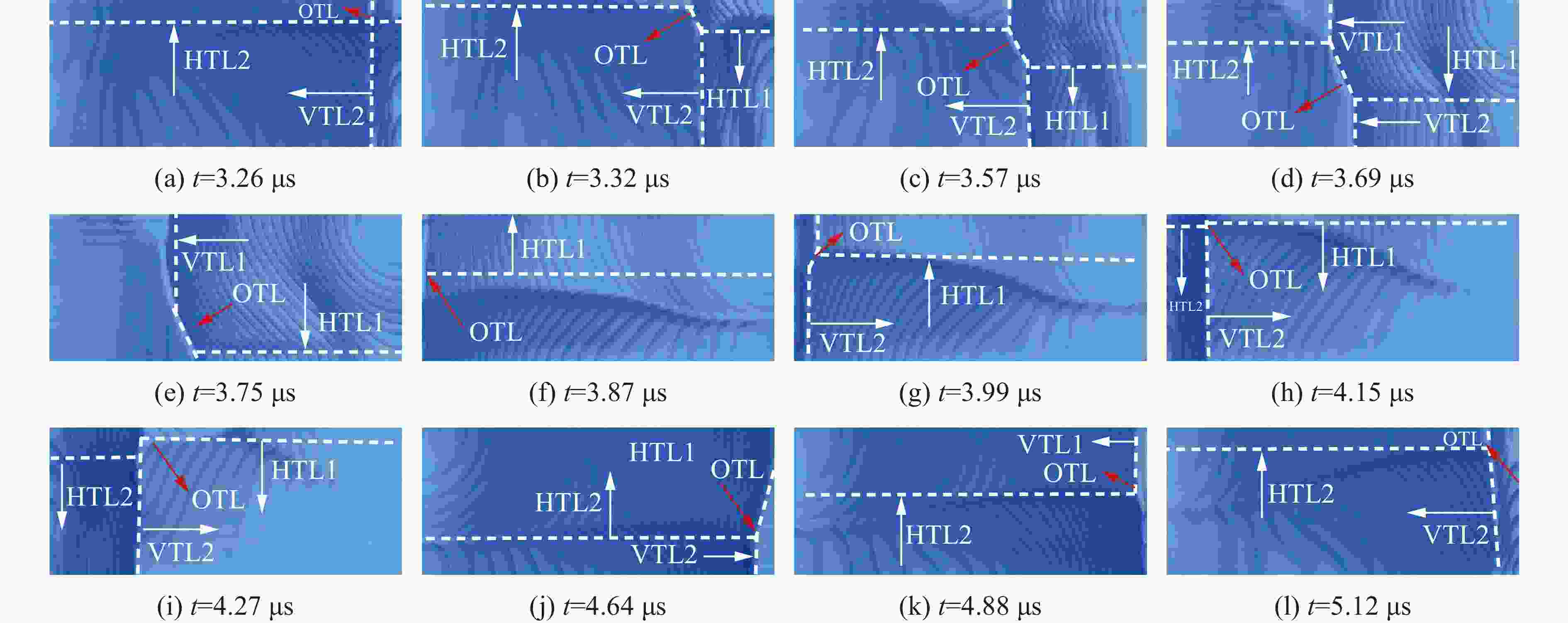
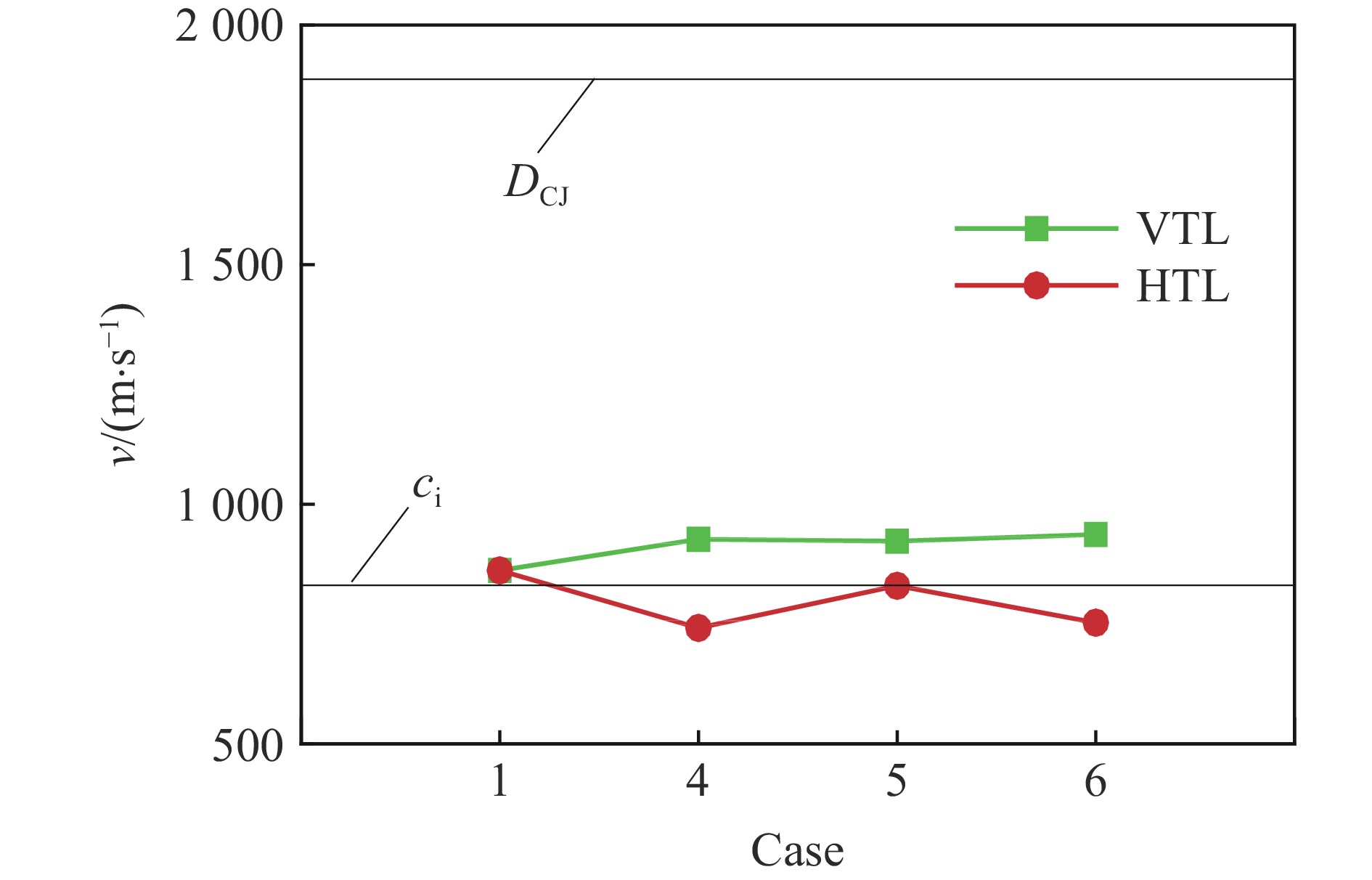
摘要: 为探索极限条件下矩形管道截面长宽比对于螺旋爆轰传播的影响，采用基于五阶WENO有限差分格式和两步总包反应模型的Euler方程，对三维气相螺旋爆轰波在矩形截面管道中的结构及其传播方式进行了数值研究。通过模拟不同管道截面尺寸下爆轰波的三波线运动轨迹、流场分布及高压印记结构，揭示了截面几何尺寸对气相临界爆轰波稳定传播的影响规律。结果表明：螺旋爆轰能在一定范围的小管道截面尺寸内通过横、竖两条三波线及其相互作用形成的斜三波线的运动来维持传播；随着管道截面尺寸长宽比的增加，螺旋爆轰在壁面上形成的高压印记逐渐由倾斜的条带结构变成局部点状分布结构，波阵面上的斜三波线的轨迹也由方管中沿着单一方向的圆周运动逐渐发展为具有转向机制的复杂运动轨迹；当长宽比进一步增加时，三维螺旋爆轰存在向二维结构的单头爆轰结构退化的趋势。Abstract: In order to explore the effect of the aspect ratio of rectangular tube on the propagation of the spinning detonation under the limiting detonation propagating conditions, the structure of the three-dimensional gas-phase spinning detonation wave and its propagation modes in rectangular cross-section tubes are numerically investigated based on Euler equations with a 5th-order WENO finite difference scheme and the two-step global reaction model. A linear stability theory of planar detonation wave based on the normal mode method is firstly adopted to examine the chemical reaction parameters for numerical simulations and then several cases with different aspect ratios in cross-section of rectangular tube are investigated to study the structure and propagation mode of three-dimensional gaseous spinning detonation waves. By recording motions of triple lines, flow-field distributions and high-pressure imprint of detonation wave under different sizes of tube cross-section, the effect of cross-sectional geometry on the stable propagation of gaseous detonation under the limiting detonation propagating condition is revealed. The results show that the spinning detonation propagation can be maintained within a certain range of small tube cross-section size, through the movements of horizontal and vertical triple lines and an oblique triple line that is produced by interaction between both horizontal and vertical triple lines. For a square tube with 1 of aspect ratio in cross-section, the high-pressure imprint of spinning detonation on the wall forms the helical strip pattern. With the increase of the aspect ratio of the cross-section size of the tube, the pattern of a high-pressure imprint formed by the spinning detonation on the channel wall varies from the strip structure to a dotted distribution structure, the trajectory of the oblique triple line on the wave front gradually develops from the circular motion in a single direction to a complex trajectory with varying direction. When the aspect ratio is further increased, there is a tendency for the three-dimensional spinning detonation wave to eventually degenerate into a two-dimensional single-head detonation wave structure.
-
Key words:
- spinning detonation /
- rectangular tube /
- triple line /
- two-step global reaction
-
图 1 表1参数的爆轰波线性稳定性分析结果
Figure 1. The results of linear stability analysis of chemical parameters used in table1
图 4 算例1在网格尺寸为10 μm下的计算结果
Figure 4. Computational results for case 1 with grid size of 10 μm
图 5 算例1（1.0 mm×1.0 mm）中一个典型周期内的三波线运动轨迹
Figure 5. Variation of triple line trajectories at several moments in case 1 (1.0 mm×1.0 mm)
图 6 算例1（1.0 mm×1.0 mm）几个时刻下旋转爆轰三维流场
Figure 6. Three-dimensional flow-field of spinning detonation at several moments in case 1 (1.0 mm×1.0 mm)
图 7 壁面温度云图与压力等值线的平面展开图
Figure 7. Distributions of temperature and pressure contours on the unwrapped wall of tube
图 8 不同管道截面尺寸下展开壁面上的高压印记
Figure 8. High-pressure imprints on the unwrapped wall for different tube cross-section sizes
图 10 工况4（1.0 mm×0.8 mm）下一个典型周期内的三波线运动轨迹
Figure 10. Motion trajectories of triple lines in case 4 (1.0 mm × 0.8 mm) over one period
图 11 工况5（1.0 mm×0.6 mm）下一个典型周期内的三波线运动轨迹
Figure 11. Motion trajectories of triple lines in case 5 (1.0 mm × 0.6 mm) over one period
图 12 工况6（1.0 mm×0.4 mm）下一个典型周期内的三波线运动轨迹
Figure 12. Motion trajectories of triple lines in case 6 (1.0 mm × 0.4 mm) over one period
图 13 不同算例下HTL和VTL的传播速度
Figure 13. Propagating velocities of HTL and VTL for different cases
图 14 不同管道截面长宽比下的三波线（OTL）轨迹示意图
Figure 14. Schematic diagram of OTL trajectories under different tube cross-sectional aspect ratios
表 1 初始条件与化学反应参数
Table 1. Initial conditions and chemical reaction parameters
p0/MPa T0/K A1/[s∙(kg∙m−3)2.2] A2/[s∙(kg∙m−3)2] Ea/RT0 γ q2/RT0 0.1 300 2×107 7×105 21 1.3 24.4 
下载: 导出CSV
表 2 计算工况
Table 2. Computational cases
工况 管道长/mm 管道宽/mm 1 1.0 1.0 2 0.8 0.8 3 0.6 0.6 4 1.0 0.8 5 1.0 0.6 6 1.0 0.4
下载: 导出CSV
-
[1] CAMPBELL C, WOODHEAD D W. CCCCI.—the ignition of gases by an explosion-wave. part I. carbon monoxide and hydrogen mixtures [J]. Journal of the Chemical Society (Resumed), 1926, 129: 3010–3021. DOI: 10.1039/JR9262903010. [2] BONE W A, FRASER R P, WHEELER W H. A photographic investigation of flame movements in gaseous explosions Ⅶ—the phenomenon of spin in detonation [J]. Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences, 1935, 235(746): 29–68. DOI: 10.1038/136974a0. [3] FAY J A. A mechanical theory of spinning detonation [J]. The Journal of Chemical Physics, 1952, 20(6): 942–950. DOI: 10.1063/1.1700655. [4] EDWARDS D H, PARRY D J, JONES A T. The structure of the wave front in spinning detonation [J]. Journal of Fluid Mechanics, 1966, 26(2): 321–336. DOI: 10.1017/S0022112066001265. [5] HUANG Z W, LEFEBVRE M H, VAN TIGGELEN P J. Experiments on spinning detonations with detailed analysis of the shock structure [J]. Shock Waves, 2000, 10(2): 119–125. DOI: 10.1007/s001930050185. [6] DOU H S, TSAI H M, KHOO B C, et al. Simulations of detonation wave propagation in rectangular ducts using a three-dimensional WENO scheme [J]. Combustion and Flame, 2008, 154(4): 644–659. DOI: 10.1016/j.combustflame.2008.06.013. [7] DOU H S, KHOO B C. Effect of initial disturbance on the detonation front structure of a narrow duct [J]. Shock Waves, 2010, 20(2): 163–173. DOI: 10.1007/s00193-009-0240-8. [8] TSUBOI N, ASAHARA M, ETO K, et al. Numerical simulation of spinning detonation in square tube [J]. Shock Waves, 2008, 18(4): 329–344. DOI: 10.1007/s00193-008-0153-y. [9] TSUBOI N, ETO K, HAYASHI A K. Detailed structure of spinning detonation in a circular tube [J]. Combustion and Flame, 2007, 149(1/2): 144–161. DOI: 10.1016/j.combustflame.2006.12.004. [10] TSUBOI N, HAYASHI A K. Numerical study on spinning detonations [J]. Proceedings of the Combustion Institute, 2007, 31(2): 2389–2396. DOI: 10.1016/j.proci.2006.07.262. [11] NAGAO T, ASAHARA M, HAYASHI A K, et al. Numerical analysis of spinning detonation dependency on initial pressure using AUSMDV scheme [C] // 51st AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition. Grapevine: AIAA, 2013. DOI: 10.2514/6.2013-1177. [12] HUANG Y, JI H, LIEN F, et al. Numerical study of three-dimensional detonation structure transformations in a narrow square tube: from rectangular and diagonal modes into spinning modes [J]. Shock Waves, 2014, 24(4): 375–392. DOI: 10.1007/s00193-014-0499-2. [13] SUGIYAMA Y, MATSUO A. Numerical study of acoustic coupling in spinning detonation propagating in a circular tube [J]. Combustion and Flame, 2013, 160(11): 2457–2470. DOI: 10.1016/j.combustflame.2013.06.003. [14] SUGIYAMA Y, MATSUO A. Numerical analysis on acoustic coupling of spinning detonation in a square tube [J]. Journal of Thermal Science and Technology, 2016, 11(1): JTST0010. DOI: 10.1299/jtst.2016jtst0010. [15] WANG C, SHU C W, HAN W H, et al. High resolution WENO simulation of 3D detonation waves [J]. Combustion and Flame, 2013, 160(2): 447–462. DOI: 10.1016/j.combustflame.2012.10.002. [16] 王成, 韩文虎, 宁建国. 三维气相爆轰动态并行计算程序设计与开发 [J]. 计算力学学报, 2012, 29(6): 948–953. DOI: 10.7511/jslx201206022.WANG C, HAN W H, NING J G. Design and development of dynamic parallel computing code for three-dimensional gaseous detonation [J]. Chinese Journal of Computational Mechanics, 2012, 29(6): 948–953. DOI: 10.7511/jslx201206022. [17] WANG C, LI P, GAO Z, et al. Three-dimensional detonation simulations with the mapped WENO-Z finite difference scheme [J]. Computers & Fluids, 2016, 139: 105–111. DOI: 10.1016/j.compfluid.2016.04.016. [18] 沈洋, 申华, 刘凯欣. 矩形通道中三维气相爆轰的三波线结构分析[C]//北京力学会第21届学术年会暨北京振动工程学会第22届学术年会论文集. 北京: 北京力学会, 北京振动工程学会, 2015. DOI: 10.11883/1001-1455(2016)05-0577-06. [19] SHEN Y, SHEN H, LIU K X, et al. Three-dimensional detonation cellular structures in rectangular ducts using an improved CESE scheme [J]. Chinese Physics B, 2016, 25(11): 114702. DOI: 10.1088/1674-1056/25/11/114702. [20] XIAO Q, SOW A, MAXWELL B M, et al. Effect of boundary layer losses on 2D detonation cellular structures [J]. Proceedings of the Combustion Institute, 2021, 38(3): 3641–3649. DOI: 10.1016/j.proci.2020.07.068. [21] SHORT M, SHARPE G J. Pulsating instability of detonations with a two-step chain-branching reaction model: theory and numerics [J]. Combustion Theory and Modelling, 2003, 7(2): 401–416. DOI: 10.1088/1364-7830/7/2/311. [22] SHORT M. Multidimensional linear stability of a detonation wave at high activation energy [J]. SIAM Journal on Applied Mathematics, 1997, 57(2): 307–326. DOI: 10.1137/s0036139995288101. [23] SHORT M, DOLD J W. Linear stability of a detonation wave with a model three-step chain-branching reaction [J]. Mathematical and Computer Modelling, 1996, 24(8): 115–123. DOI: 10.1016/0895-7177(96)00144-6. [24] VASIL’EV A A. Near-limiting regimes of gaseous detonation [J]. Combustion, Explosion and Shock Waves, 1987, 23(3): 358–364. DOI: 10.1007/BF00748799. [25] VOITSEKHOVSKII B V, MITROFANOV V V, TOPCHIYAN M E. Structure of the detonation front in gases(survey) [J]. Combustion, Explosion and Shock Waves, 1969, 5(3): 267–273. DOI: 10.1007/BF00748606. [26] FICKETT W, DAVIS W C. Detonation [M]. Berkeley: University of California Press, 1979. -
计量
- 文章访问数: 257
- HTML全文浏览量: 60
- PDF下载量: 91
- 被引次数: 0