Review on dynamic mechanical analysis and design of graded cellular materials
-
摘要: 多胞材料是一种内部含有大量空穴和胞元的结构,具有轻质、高比吸能等特性,广泛应用于航空航天、交通运输和人体防护等碰撞/爆炸防护领域。引入梯度设计可实现多胞材料的有序耗能和载荷调控,满足不同情形和工况下的防护需求。对梯度多胞材料动态力学行为和设计的研究进展进行了综述,着重介绍了梯度多胞材料/结构在抗冲击、抗爆炸和模拟爆炸载荷加载3个方面的应用案例。首先,介绍了梯度多胞材料的分类,较系统地总结了梯度多胞材料在动态加载下的变形特征、冲击波模型、防护性能等方面的研究,阐明了梯度多胞材料的密度/强度梯度与惯性效应存在的竞争机制。其次,以冲击波模型为力学原理指导梯度多胞材料/结构设计,介绍了梯度多胞材料耐撞性反向设计、多种抗爆炸夹芯结构设计、计及弹靶耦合效应的爆炸载荷模拟器设计等策略,实现了最佳防护效果或载荷精准控制,为抗冲击/抗爆炸防护设计和快速评估提供理论基础和技术支撑。最后,展望了梯度多胞材料在极端环境加载、大能量冲击和强非线性载荷调控等需求下冲击防护领域的应用前景。Abstract: Cellular materials are structures with a large number of internal cavities and cells, which have the properties of lightweight and high specific energy absorption, and they are widely used in the collision/explosion protection, such as aerospace, transportation, and human protection. Introducing a gradient design to cellular materials helps the materials to meet the protection requirements in different scenarios and conditions with the properties of orderly dissipation of energy and manipulation of loads. A review of research advances in the dynamic analysis and design on mechanical behavior of graded cellular materials is presented. Three cases of the applications of graded cellular materials/structures, i.e., impact resistance, blast resistance, and blast-mimicking loading, are elaborated. Firstly, graded cellular materials are briefly described from various aspects, such as natural vs. artificial, layered vs. continuous, strength gradient vs. density gradient, and conventional manufacturing vs. additive manufacturing. The studies of the deformation characteristics, shock wave models, and protective properties of graded cellular materials under dynamic loading are then reviewed systematically. A competitive mechanism of density/strength gradients and inertial effects exists in graded cellular materials to synergistically modulate collapse deformation modes. According to the stress-strain curve characteristics of cellular materials, choosing the appropriate constitutive model could increase the characterization accuracy for its dynamic mechanical behavior. Secondly, the shock wave models are used as a mechanical tool to guide the design of graded cellular materials/structures. Some strategies are elaborated, such as the backward design of graded cellular materials for impact resistance, the design of several types of anti-blast sandwich structures, and the design of blast-load simulators with the projectile-beam coupling effect being taken into account. The optimal protection effect or precise load control had been realized efficiently, which provides a theoretical basis and technical support for the protection design and rapid evaluation of impact/explosion resistance structures. Finally, for the applications in the scenarios of extreme environmental loading, large energy impacts, and strong nonlinear load manipulation, the investigations of graded cellular materials are full of challenges and expectation.
-
表 1 不同冲击工况下梯度多胞材料的动态响应理论模型
Table 1. Theoretical models of the dynamic response for graded cellular materials under different impact scenarios
变形模式 质量块初速度撞击[80, 95] 爆炸加载[112] 单波 $ \left\{ \begin{gathered} \dot \varPhi = \dfrac{v}{{{\varepsilon _{\text{B}}}(\rho (\varPhi ))}} \\ {\sigma _{\text{B}}} = {\sigma _{\text{0}}} + {\rho _{\text{s}}}\rho (\varPhi )\dfrac{{{v^2}}}{{{\varepsilon _{\text{B}}}(\rho (\varPhi ))}} \\ \dot v = \dfrac{{ - {\sigma _{\text{B}}}}}{{m + {\rho _{\text{s}}}\displaystyle\int_0^\varPhi {\rho (X){\rm{d}}X} }} \\ \end{gathered} \right. $ $ \left\{ \begin{gathered} \dot \varPhi = \dfrac{v}{{{\varepsilon _{\text{B}}}(\rho (\varPhi ))}} \\ {\sigma _{\text{B}}} = {\sigma _{\text{0}}} + {\rho _{\text{s}}}\rho (\varPhi )\dfrac{{{v^2}}}{{{\varepsilon _{\text{B}}}(\rho (\varPhi ))}} \\ \dot v = \dfrac{{p(t) - {\sigma _{\text{B}}}}}{{m + {\rho _{\text{s}}}\displaystyle\int_0^\varPhi {\rho (X){\rm{d}}X} }} \\ \end{gathered} \right. $ 双波 $ \left\{ \begin{gathered} {{\dot \varPhi }_1} = \dfrac{{{v_1} - {v_2}}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _1}))}} \\ {{\dot \varPhi }_2} = \dfrac{{ - {v_2}}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _2}))}} \\ {\sigma _{{\text{B,1}}}} = {\sigma _{\text{0}}}(\rho ({\varPhi _1})) + {\rho _{\text{s}}}\rho ({\varPhi _1})\dfrac{{{{({v_1} - {v_2})}^2}}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _1}))}} \\ {\sigma _{{\text{B,2}}}} = {\sigma _{\text{0}}}(\rho ({\varPhi _2})) + {\rho _{\text{s}}}\rho ({\varPhi _2})\dfrac{{{v_2}^2}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _2}))}} \\ {{\dot v}_1} = \dfrac{{ - {\sigma _{{\text{B,1}}}}}}{{m + {\rho _{\text{s}}}\displaystyle\int_0^{{\varPhi _1}} {\rho (X){\text{d}}X} }} \\ {{\dot v}_2} = \dfrac{{{\sigma _{\text{0}}}(\rho ({\varPhi _1})) - {\sigma _{\text{0}}}(\rho ({\varPhi _2}))}}{{{\rho _{\text{s}}}\displaystyle\int_{{\varPhi _1}}^{{\varPhi _2}} {\rho (X){\text{d}}X} }} \\ \end{gathered} \right. $ $ \left\{ \begin{gathered} {{\dot \varPhi }_1} = \dfrac{{{v_1} - {v_2}}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _1}))}} \\ {{\dot \varPhi }_2} = \dfrac{{ - {v_2}}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _2}))}} \\ {\sigma _{{\text{B,1}}}} = {\sigma _{\text{0}}}(\rho ({\varPhi _1})) + {\rho _{\text{s}}}\rho ({\varPhi _1})\dfrac{{{{({v_1} - {v_2})}^2}}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _1}))}} \\ {\sigma _{{\text{B,2}}}} = {\sigma _{\text{0}}}(\rho ({\varPhi _2})) + {\rho _{\text{s}}}\rho ({\varPhi _2})\dfrac{{{v_2}^2}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _2}))}} \\ {{\dot v}_1} = \dfrac{{p(t) - {\sigma _{{\text{B,1}}}}}}{{m + {\rho _{\text{s}}}\displaystyle\int_0^{{\varPhi _1}} {\rho (X){\text{d}}X} }} \\ {{\dot v}_2} = \dfrac{{{\sigma _{\text{0}}}(\rho ({\varPhi _1})) - {\sigma _{\text{0}}}(\rho ({\varPhi _2}))}}{{{\rho _{\text{s}}}\displaystyle\int_{{\varPhi _1}}^{{\varPhi _2}} {\rho (X){\text{d}}X} }} \\ \end{gathered} \right. $ 三波 $ \left\{ \begin{gathered} {{\dot \varPhi }_1} = \dfrac{{{v_1} - {v_2}}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _1}))}} \\ {{\dot \varPhi }_2} = \dfrac{{{v_2} - {v_3}}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _2}))}} \\ {{\dot \varPhi }_3} = \dfrac{{{v_3}}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _3}))}} \\ {\sigma _{{\text{B,1}}}} = {\sigma _{\text{0}}}(\rho ({\varPhi _1})) + {\rho _{\text{s}}}\rho ({\varPhi _1})\dfrac{{{{({v_1} - {v_2})}^2}}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _1}))}} \\ {\sigma _{{\text{B,2}}}} = {\sigma _{\text{0}}}(\rho ({\varPhi _2})) + {\rho _{\text{s}}}\rho ({\varPhi _2})\dfrac{{{{({v_2} - {v_3})}^2}}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _2}))}} \\ {\sigma _{{\text{B,3}}}} = {\sigma _{\text{0}}}(\rho ({\varPhi _3})) + {\rho _{\text{s}}}\rho ({\varPhi _3})\dfrac{{{v_3}^2}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _3}))}} \\ {{\dot v}_1} = \dfrac{{ - {\sigma _{{\text{B,1}}}}(\rho ({\varPhi _1}))}}{{m + {\rho _{\text{s}}}\displaystyle\int_0^{{\varPhi _1}} {\rho (X){\rm{d}}X} }} \\ {{\dot v}_2} = \dfrac{{{\sigma _{\text{0}}}(\rho ({\varPhi _1})) - {\sigma _{\text{0}}}(\rho ({\varPhi _2}))}}{{{\rho _{\text{s}}}\displaystyle\int_{{\varPhi _1}}^{{\varPhi _2}} {\rho (X){\rm{d}}X} }} \\ {{\dot v}_3} = \dfrac{{{\sigma _{{\text{B,3}}}}(\rho ({\varPhi _2})) - {\sigma _{{\text{B,2}}}}(\rho ({\varPhi _3}))}}{{{\rho _{\text{s}}}\displaystyle\int_{{\varPhi _2}}^{{\varPhi _3}} {\rho (X){\rm{d}}X} }} \\ \end{gathered} \right. $ $ \left\{ \begin{gathered} {{\dot \varPhi }_1} = \dfrac{{{v_1} - {v_2}}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _1}))}} \\ {{\dot \varPhi }_2} = \dfrac{{{v_2} - {v_3}}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _2}))}} \\ {{\dot \varPhi }_3} = \dfrac{{{v_3}}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _3}))}} \\ {\sigma _{{\text{B,1}}}} = {\sigma _{\text{0}}}(\rho ({\varPhi _1})) + {\rho _{\text{s}}}\rho ({\varPhi _1})\dfrac{{{{({v_1} - {v_2})}^2}}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _1}))}} \\ {\sigma _{{\text{B,2}}}} = {\sigma _{\text{0}}}(\rho ({\varPhi _2})) + {\rho _{\text{s}}}\rho ({\varPhi _2})\dfrac{{{{({v_2} - {v_3})}^2}}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _2}))}} \\ {\sigma _{{\text{B,3}}}} = {\sigma _{\text{0}}}(\rho ({\varPhi _3})) + {\rho _{\text{s}}}\rho ({\varPhi _3})\dfrac{{{v_3}^2}}{{{\varepsilon _{\text{B}}}(\rho ({\varPhi _3}))}} \\ {{\dot v}_1} = \dfrac{{p(t) - {\sigma _{{\text{B,1}}}}(\rho ({\varPhi _1}))}}{{m + {\rho _{\text{s}}}\displaystyle\int_0^{{\varPhi _1}} {\rho (X){\rm{d}}X} }} \\ {{\dot v}_2} = \dfrac{{{\sigma _{\text{0}}}(\rho ({\varPhi _1})) - {\sigma _{\text{0}}}(\rho ({\varPhi _2}))}}{{{\rho _{\text{s}}}\displaystyle\int_{{\varPhi _1}}^{{\varPhi _2}} {\rho (X){\rm{d}}X} }} \\ {{\dot v}_3} = \dfrac{{{\sigma _{{\text{B,3}}}}(\rho ({\varPhi _2})) - {\sigma _{{\text{B,2}}}}(\rho ({\varPhi _3}))}}{{{\rho _{\text{s}}}\displaystyle\int_{{\varPhi _2}}^{{\varPhi _3}} {\rho (X){\rm{d}}X} }} \\ \end{gathered} \right. $ -
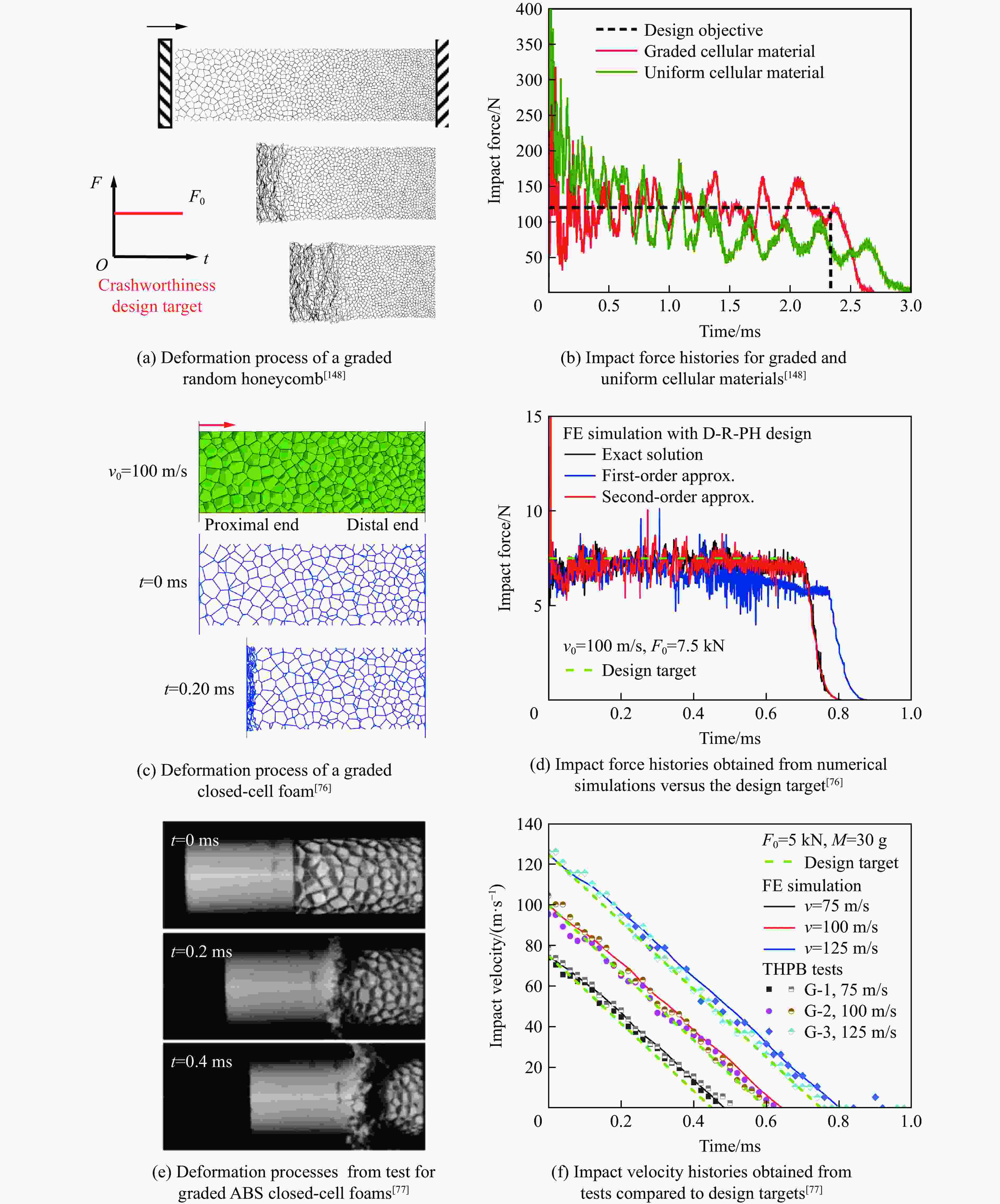
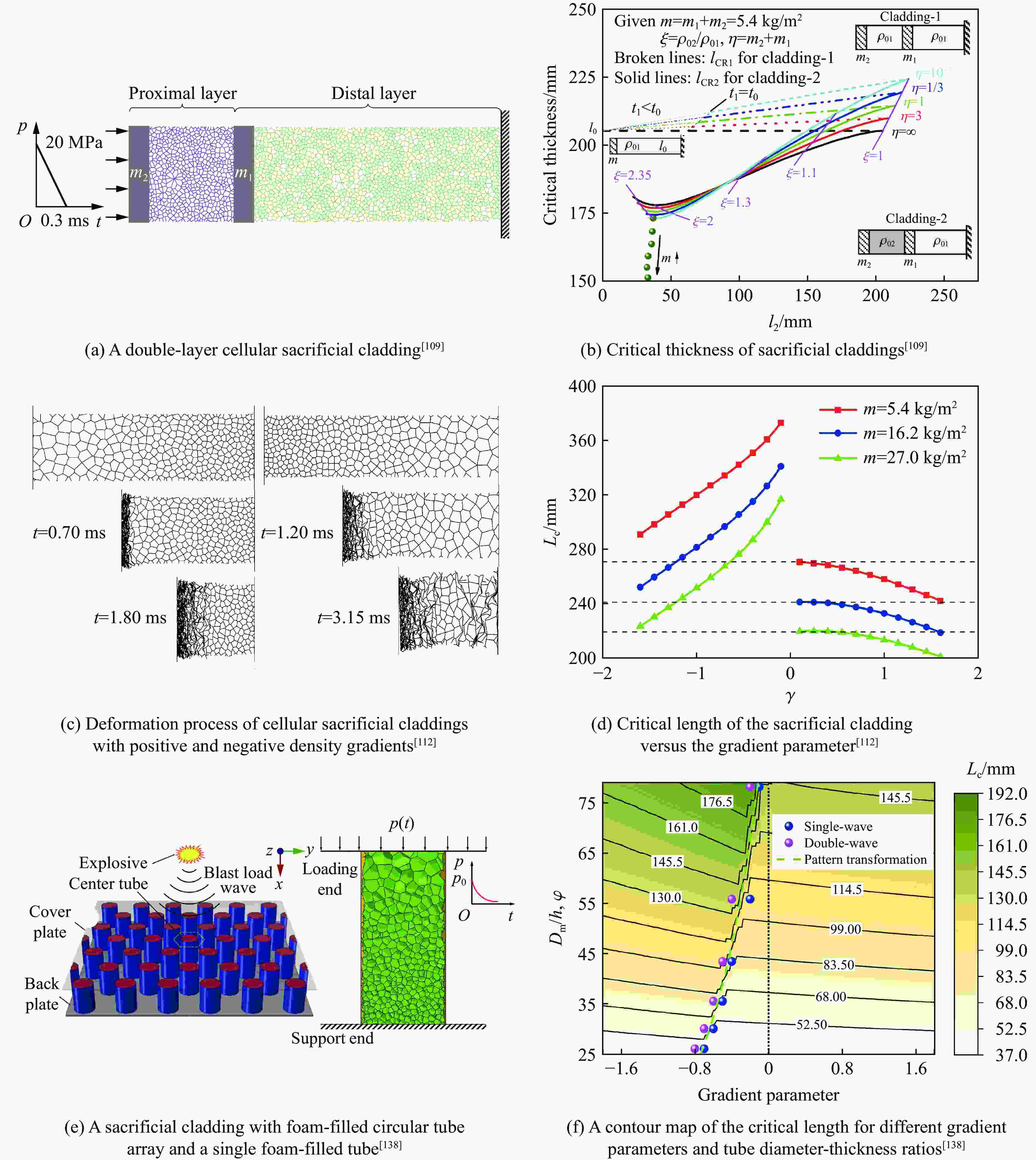
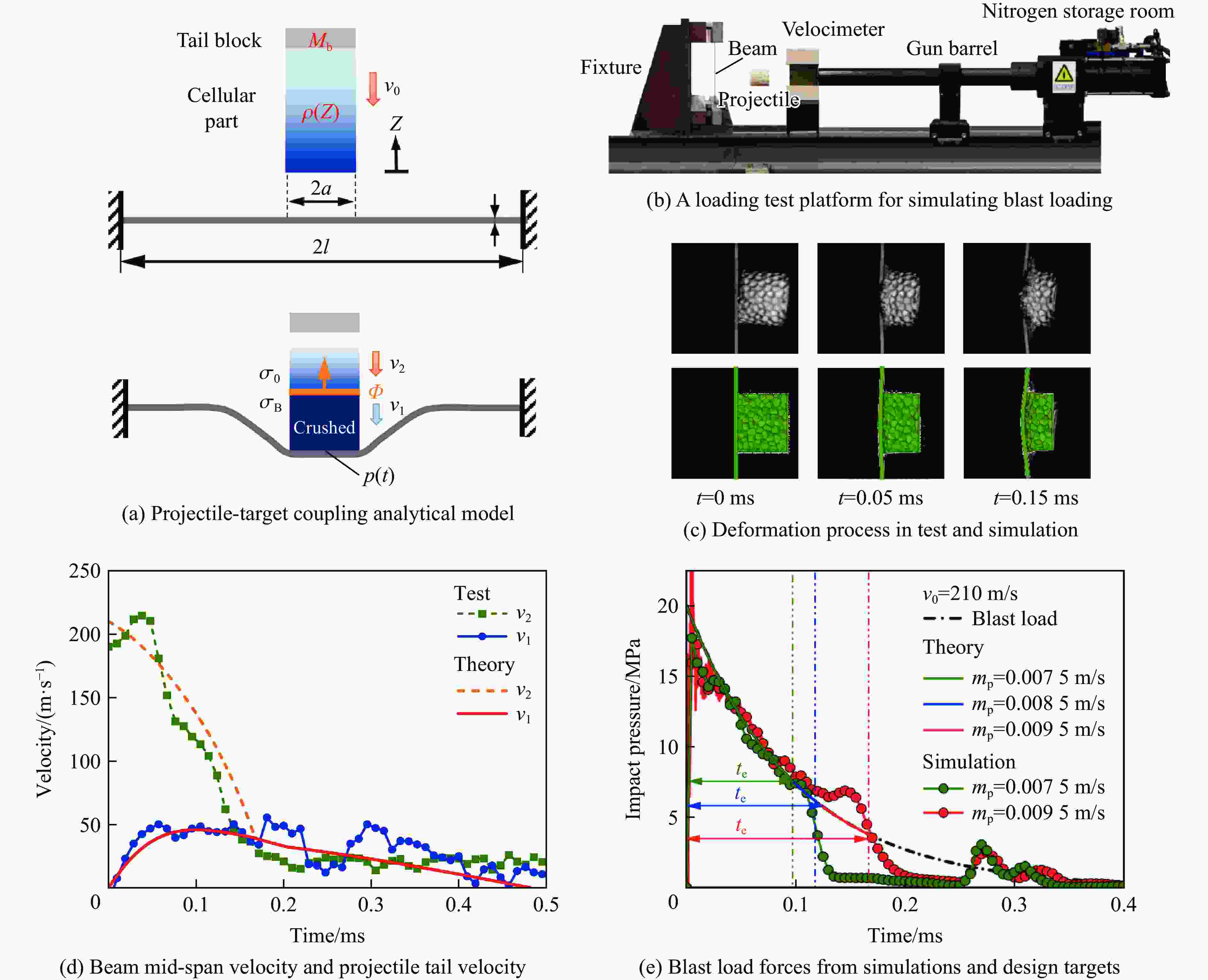
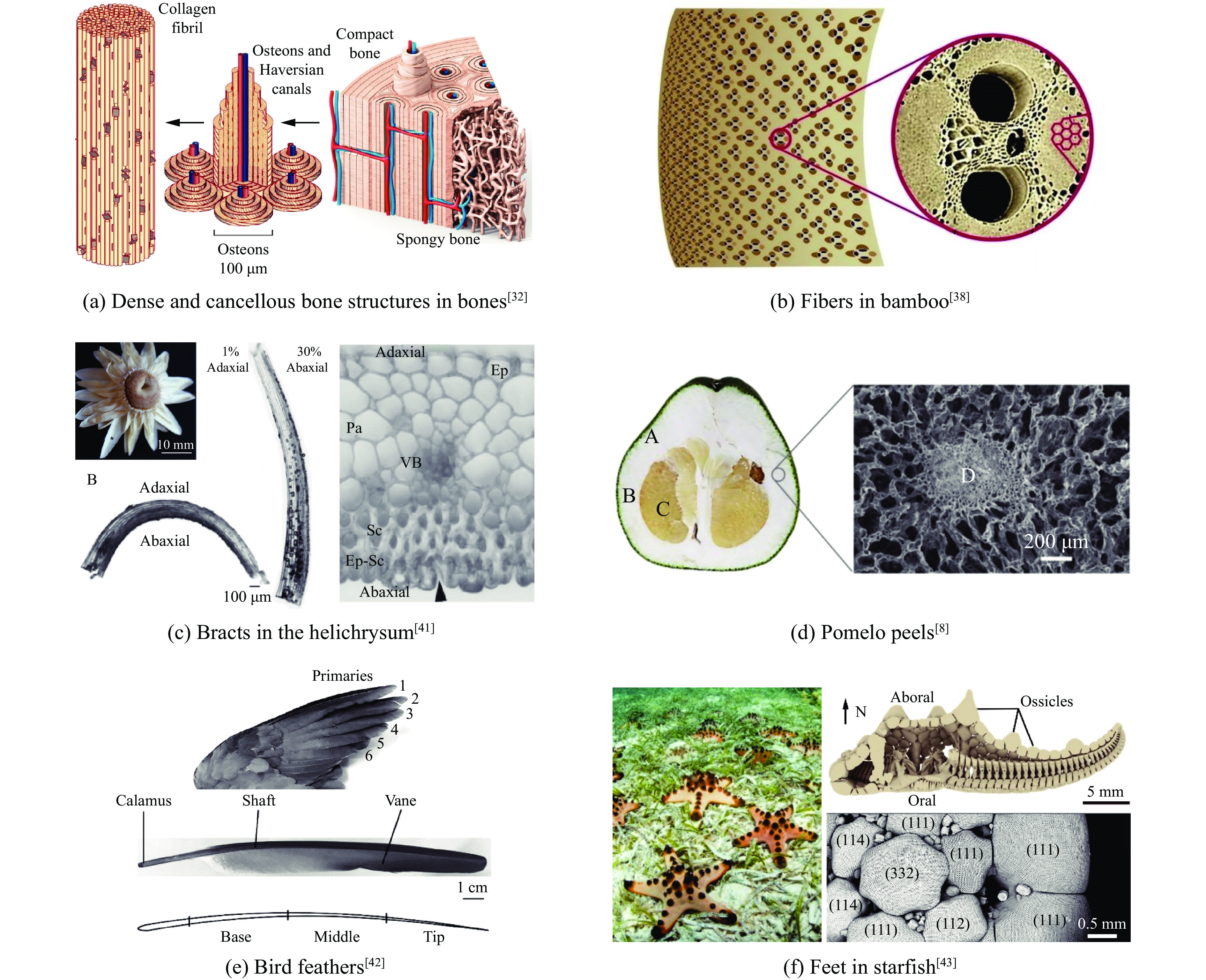
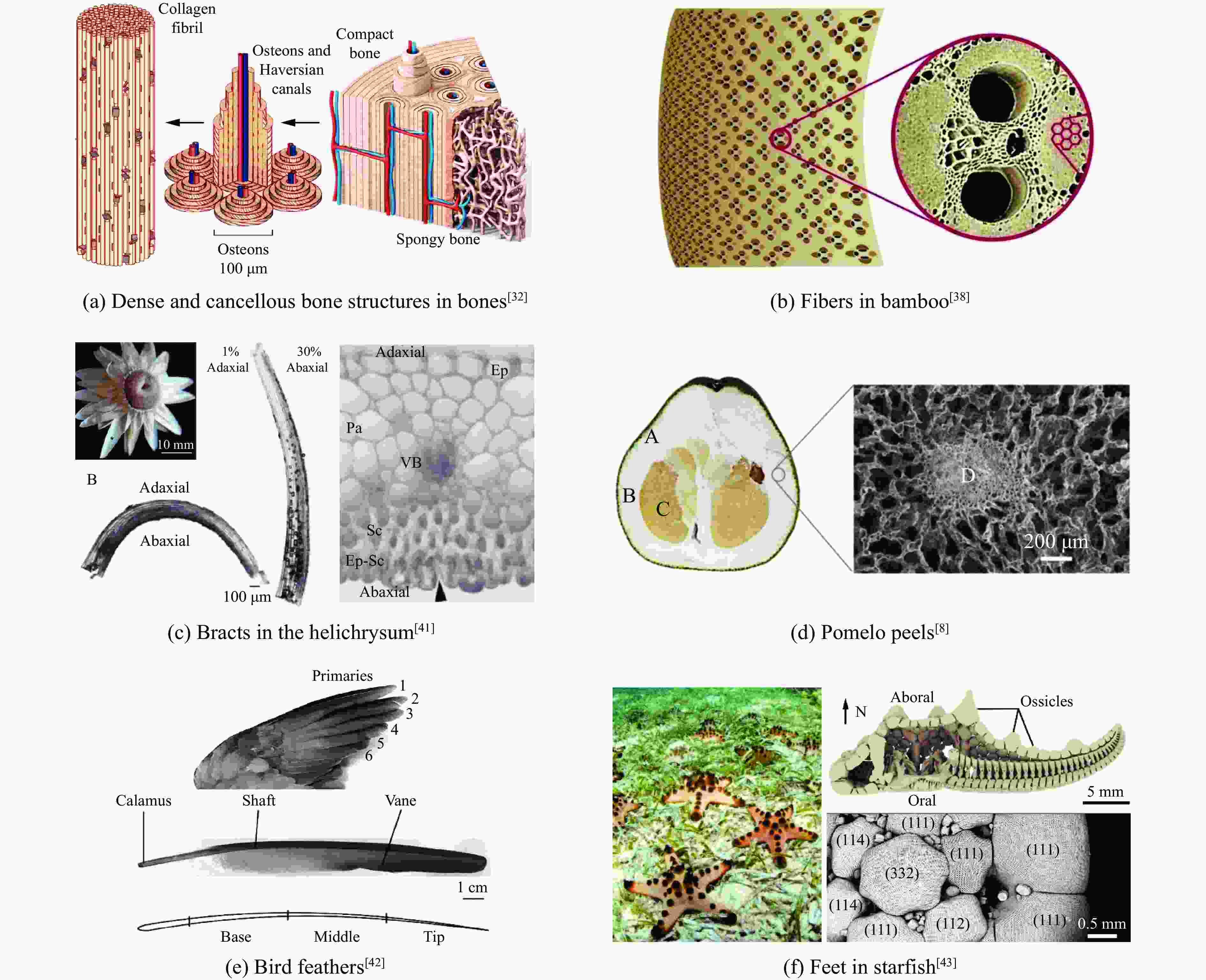
[1] ZHANG Z Q, YANG J L. Improving safety of runway overrun through foamed concrete aircraft arresting system: an experimental study [J]. International Journal of Crashworthiness, 2015, 20(5): 448–463. DOI: 10.1080/13588265.2015.1033971. [2] YANG X F, YANG J L, ZHANG Z Q, et al. A review of civil aircraft arresting system for runway overruns [J]. Progress in Aerospace Sciences, 2018, 102: 99–121. DOI: 10.1016/j.paerosci.2018.07.006. [3] 田斌, 李如江, 赵家骏, 等. 钢板与泡沫铝复合板弹药包装箱的对比研究 [J]. 兵器装备工程学报, 2019, 40(10): 190–194. DOI: 10.11809/bqzbgcxb2019.10.040.TIAN B, LI R J, ZHAO J J, et al. Comparative study of steel plate and foam aluminum composite plate ammunition packaging box [J]. Journal of Ordnance Equipment Engineering, 2019, 40(10): 190–194. DOI: 10.11809/bqzbgcxb2019.10.040. [4] 张凯, 张庆明, 贾伟. 多层圆管夹芯结构的装甲车底板防爆设计 [J]. 安全与环境学报, 2017, 17(3): 969–974. DOI: 10.13637/j.issn.1009-6094.2017.03.030.ZHANG K, ZHANG Q M, JIA W. On the safety design of the multilayer circular-tube structure of the armored vehicle plate under the explosion loading [J]. Journal of Safety and Environment, 2017, 17(3): 969–974. DOI: 10.13637/j.issn.1009-6094.2017.03.030. [5] 张钱城, 郝方楠, 李裕春, 等. 爆炸冲击载荷作用下车辆和人员的损伤与防护 [J]. 力学与实践, 2014, 36(5): 527–539. DOI: 10.6052/1000-0879-13-539.ZHANG Q C, HAO F N, LI Y C, et al. Research progress in the injury and protection to vehicle and passengers under explosive shock loading [J]. Mechanics in Engineering, 2014, 36(5): 527–539. DOI: 10.6052/1000-0879-13-539. [6] REID S R, PENG C. Dynamic uniaxial crushing of wood [J]. International Journal of Impact Engineering, 1997, 19(5/6): 531–570. DOI: 10.1016/S0734-743X(97)00016-X. [7] GROSSER D, LIESE W. On the anatomy of Asian bamboos, with special reference to their vascular bundles [J]. Wood Science and Technology, 1971, 5(4): 290–312. DOI: 10.1007/BF00365061. [8] ZHANG W, YIN S, YU T X, et al. Crushing resistance and energy absorption of pomelo peel inspired hierarchical honeycomb [J]. International Journal of Impact Engineering, 2019, 125: 163–172. DOI: 10.1016/j.ijimpeng.2018.11.014. [9] ASHBY M F. The properties of foams and lattices [J]. Philosophical Transactions of the Royal Society A: Mathematical, Physical and Engineering Sciences, 2006, 364(1838): 15–30. DOI: 10.1098/rsta.2005.1678. [10] CHEN L M, ZHANG J, DU B, et al. Dynamic crushing behavior and energy absorption of graded lattice cylindrical structure under axial impact load [J]. Thin-Walled Structures, 2018, 127: 333–343. DOI: 10.1016/j.tws.2017.10.048. [11] WANG Z G. Recent advances in novel metallic honeycomb structure [J]. Composites Part B: Engineering, 2019, 166: 731–741. DOI: 10.1016/j.compositesb.2019.02.011. [12] BACIGALUPO A, DE BELLIS M L, MISSERONI D. Design of tunable acoustic metamaterials with periodic piezoelectric microstructure [J]. Extreme Mechanics Letters, 2020, 40: 100977. DOI: 10.1016/j.eml.2020.100977. [13] WANG Z G, ZHOU Y, WANG X X, et al. Compression behavior of strut-reinforced hierarchical lattice—experiment and simulation [J]. International Journal of Mechanical Sciences, 2021, 210: 106749. DOI: 10.1016/j.ijmecsci.2021.106749. [14] SUN Z P, GUO Y B, SHIM V P W. Deformation and energy absorption characteristics of additively-manufactured polymeric lattice structures—effects of cell topology and material anisotropy [J]. Thin-Walled Structures, 2021, 169: 108420. DOI: 10.1016/j.tws.2021.108420. [15] XIAO L J, XU X, FENG G Z, et al. Compressive performance and energy absorption of additively manufactured metallic hybrid lattice structures [J]. International Journal of Mechanical Sciences, 2022, 219: 107093. DOI: 10.1016/j.ijmecsci.2022.107093. [16] TAN P J, REID S R, HARRIGAN J J, et al. Dynamic compressive strength properties of aluminium foams. part Ⅱ-‘shock’ theory and comparison with experimental data and numerical models [J]. Journal of the Mechanics and Physics of Solids, 2005, 53(10): 2206–2230. DOI: 10.1016/j.jmps.2005.05.003. [17] ELNASRI I, PATTOFATTO S, ZHAO H, et al. Shock enhancement of cellular structures under impact loading: part Ⅰ experiments [J]. Journal of the Mechanics and Physics of Solids, 2007, 55(12): 2652–2671. DOI: 10.1016/j.jmps.2007.04.005. [18] LIU Y D, YU J L, ZHENG Z J, et al. A numerical study on the rate sensitivity of cellular metals [J]. International Journal of Solids and Structures, 2009, 46(22/23): 3988–3998. DOI: 10.1016/j.ijsolstr.2009.07.024. [19] ZHENG Z J, WANG C F, YU J L, et al. Dynamic stress-strain states for metal foams using a 3D cellular model [J]. Journal of the Mechanics and Physics of Solids, 2014, 72: 93–114. DOI: 10.1016/j.jmps.2014.07.013. [20] SUN Y L, LI Q M, MCDONALD S A, et al. Determination of the constitutive relation and critical condition for the shock compression of cellular solids [J]. Mechanics of Materials, 2016, 99: 26–36. DOI: 10.1016/j.mechmat.2016.04.004. [21] WANG H R, LI S Q, LIU Z F, et al. Investigation on the dynamic response of circular sandwich panels with the bio-inspired gradient core [J]. Thin-Walled Structures, 2020, 149: 106667. DOI: 10.1016/j.tws.2020.106667. [22] LAZARUS B S, CHADHA C, VELASCO-HOGAN A, et al. Engineering with keratin: a functional material and a source of bioinspiration [J]. iScience, 2021, 24(8): 102798. DOI: 10.1016/j.isci.2021.102798. [23] LIU Z Q, MEYERS M A, ZHANG Z F, et al. Functional gradients and heterogeneities in biological materials: design principles, functions, and bioinspired applications [J]. Progress in Materials Science, 2017, 88: 467–498. DOI: 10.1016/j.pmatsci.2017.04.013. [24] LE BARBENCHON L, GIRARDOT J, KOPP J B, et al. Multi-scale foam: 3D structure/compressive behaviour relationship of agglomerated cork [J]. Materialia, 2019, 5: 100219. DOI: 10.1016/j.mtla.2019.100219. [25] LAUNEY M E, BUEHLER M J, RITCHIE R O. On the mechanistic origins of toughness in bone [J]. Annual Review of Materials Research, 2010, 40: 25–53. DOI: 10.1146/annurev-matsci-070909-104427. [26] SARANATHAN V, OSUJI C O, MOCHRIE S G J, et al. Structure, function, and self-assembly of single network gyroid (I4132) photonic crystals in butterfly wing scales [J]. Proceedings of the National Academy of Sciences of the United States of America, 2010, 107(26): 11676–11681. DOI: 10.1073/pnas.0909616107. [27] GIBSON L J, ASHBY M F. Cellular solids: structure and properties [M]. Cambridge: Cambridge University Press, 1988. [28] WESTER T. Nature teaching structures [J]. International Journal of Space Structures, 2002, 17(2/3): 135–147. DOI: 10.1260/026635102320321789. [29] HELFMAN COHEN Y, REICH Y, GREENBERG S. Biomimetics: structure-function patterns approach [J]. Journal of Mechanical Design, 2014, 136(11): 111108. DOI: 10.1115/1.4028169. [30] TAVSAN C, TAVSAN F, SONMEZ E. Biomimicry in architectural design education [J]. Procedia-Social and Behavioral Sciences, 2015, 182: 489–496. DOI: 10.1016/j.sbspro.2015.04.832. [31] AZIZ M S, EL SHERIF A Y. Biomimicry as an approach for bio-inspired structure with the aid of computation [J]. Alexandria Engineering Journal, 2016, 55(1): 707–714. DOI: 10.1016/j.aej.2015.10.015. [32] BUCKWALTER J A, GLIMCHER M J, COOPER R R, et al. Bone biology. Ⅰ: structure, blood supply, cells, matrix, and mineralization [J]. Instructional Course Lectures, 1996, 45: 371–386. [33] NOGATA F, TAKAHASHI H. Intelligent functionally graded material: bamboo [J]. Composites Engineering, 1995, 5(7): 743–751. DOI: 10.1016/0961-9526(95)00037-N. [34] AMADA S, MUNEKATA T, NAGASE Y, et al. The mechanical structures of bamboos in viewpoint of functionally gradient and composite materials [J]. Journal of Composite Materials, 1996, 30(7): 800–819. DOI: 10.1177/002199839603000703. [35] RAY A K, DAS S K, MONDAL S, et al. Microstructural characterization of bamboo [J]. Journal of Materials Science, 2004, 39(3): 1055–1060. DOI: 10.1023/B:JMSC.0000012943.27090.8f. [36] SILVA E C N, WALTERS M C, PAULINO G H. Modeling bamboo as a functionally graded material: lessons for the analysis of affordable materials [J]. Journal of Materials Science, 2006, 41(21): 6991–7004. DOI: 10.1007/s10853-006-0232-3. [37] HABIBI M K, SAMAEI A T, GHESHLAGHI B, et al. Asymmetric flexural behavior from bamboo’s functionally graded hierarchical structure: underlying mechanisms [J]. Acta Biomaterialia, 2015, 16: 178–186. DOI: 10.1016/j.actbio.2015.01.038. [38] WEGST U G K, BAI H, SAIZ E, et al. Bioinspired structural materials [J]. Nature Materials, 2015, 14(1): 23–36. DOI: 10.1038/nmat4089. [39] EDER M, JUNGNIKL K, BURGERT I. A close-up view of wood structure and properties across a growth ring of Norway spruce (Picea abies [L] Karst. ) [J]. Trees, 2009, 23(1): 79-84. DOI: 10.1007/s00468-008-0256-1. [40] SPECK T, BURGERT I. Plant stems: functional design and mechanics [J]. Annual Review of Materials Research, 2011, 41: 169–193. DOI: 10.1146/annurev-matsci-062910-100425. [41] BOROWSKA-WYKRĘT D, RYPIEŃ A, DULSKI M, et al. Gradient of structural traits drives hygroscopic movements of scarious bracts surrounding Helichrysum bracteatum capitulum [J]. Annals of Botany, 2017, 119(8): 1365–1383. DOI: 10.1093/aob/mcx015. [42] DENG K, KOVALEV A, RAJABI H, et al. The damping properties of the foam-filled shaft of primary feathers of the pigeon Columba livia [J]. The Science of Nature, 2021, 109(1): 1. DOI: 10.1007/s00114-021-01773-7. [43] YANG T, CHEN H S, JIA Z A, et al. A damage-tolerant, dual-scale, single-crystalline microlattice in the knobby starfish, Protoreaster nodosus [J]. Science, 2022, 375(6581): 647–652. DOI: 10.1126/science.abj9472. [44] EVANS A G, HUTCHINSON J W, FLECK N A, et al. The topological design of multifunctional cellular metals [J]. Progress in Materials Science, 2001, 46(3/4): 309–327. DOI: 10.1016/S0079-6425(00)00016-5. [45] DESHPANDE V S, FLECK N A, ASHBY M F. Effective properties of the octet-truss lattice material [J]. Journal of the Mechanics and Physics of Solids, 2001, 49(8): 1747–1769. DOI: 10.1016/S0022-5096(01)00010-2. [46] YAO R Y, PANG T, HE S Y, et al. A bio-inspired foam-filled multi-cell structural configuration for energy absorption [J]. Composites Part B: Engineering, 2022, 238: 109801. DOI: 10.1016/j.compositesb.2022.109801. [47] ZHANG Y, HE S Y, LIU J G, et al. Density gradient tailoring of aluminum foam-filled tube [J]. Composite Structures, 2019, 220: 451–459. DOI: 10.1016/j.compstruct.2019.04.026. [48] BROTHERS A H, DUNAND D C. Density-graded cellular aluminum [J]. Advanced Engineering Materials, 2006, 8(9): 805–809. DOI: 10.1002/adem.200600074. [49] 张新春, 刘颖. 密度梯度蜂窝材料动力学性能研究 [J]. 工程力学, 2012, 29(8): 372–377. DOI: 10.6052/j.issn.1000-4750.2010.12.0872.ZHANG X C, LIU Y. Research on the dynamic crushing of honeycombs with density gradient [J]. Engineering Mechanics, 2012, 29(8): 372–377. DOI: 10.6052/j.issn.1000-4750.2010.12.0872. [50] LIU H, NG B F. Dynamic response of density-graded foam subjected to soft impact [J]. Composite Structures, 2022, 284: 115145. DOI: 10.1016/j.compstruct.2021.115145. [51] PAGLIOCCA N, YOUSSEF G, KOOHBOR B. In-plane mechanical and failure responses of honeycombs with syntactic foam cell walls [J]. Composite Structures, 2022, 295: 115866. DOI: 10.1016/j.compstruct.2022.115866. [52] JEFFERSON A J, SCHNEIDER J, SCHIFFER A, et al. Dynamic crushing of tailored honeycombs realized via additive manufacturing [J]. International Journal of Mechanical Sciences, 2022, 219: 107126. DOI: 10.1016/j.ijmecsci.2022.107126. [53] 吴鹤翔, 刘颖. 梯度变化对密度梯度蜂窝材料力学性能的影响 [J]. 爆炸与冲击, 2013, 33(2): 163–168. DOI: 10.11883/1001-1455(2013)02-0163-06.WU H X, LIU Y. Influences of density gradient variation on mechanical performances of density-graded honeycomb materials [J]. Explosion and Shock Waves, 2013, 33(2): 163–168. DOI: 10.11883/1001-1455(2013)02-0163-06. [54] FENG G Z, LI S, XIAO L J, et al. Energy absorption performance of honeycombs with curved cell walls under quasi-static compression [J]. International Journal of Mechanical Sciences, 2021, 210: 106746. DOI: 10.1016/j.ijmecsci.2021.106746. [55] ZENG Y, SUN L J, YAO H H, et al. Fabrication of alumina ceramics with functional gradient structures by digital light processing 3D printing technology [J]. Ceramics International, 2022, 48(8): 10613–10619. DOI: 10.1016/j.ceramint.2021.12.275. [56] BAI L, GONG C, CHEN X H, et al. Mechanical properties and energy absorption capabilities of functionally graded lattice structures: experiments and simulations [J]. International Journal of Mechanical Sciences, 2020, 182: 105735. DOI: 10.1016/j.ijmecsci.2020.105735. [57] JIN M X, FENG Q X, FAN X J, et al. Investigation on the mechanical properties of TPMS porous structures fabricated by laser powder bed fusion [J]. Journal of Manufacturing Processes, 2022, 76: 559–574. DOI: 10.1016/j.jmapro.2022.02.035. [58] QIU N, ZHANG J Z, YUAN F Q, et al. Mechanical performance of triply periodic minimal surface structures with a novel hybrid gradient fabricated by selective laser melting [J]. Engineering Structures, 2022, 263: 114377. DOI: 10.1016/j.engstruct.2022.114377. [59] ZHANG J F, CHEN X H, SUN Y X, et al. Design of a biomimetic graded TPMS scaffold with quantitatively adjustable pore size [J]. Materials & Design, 2022, 218: 110665. DOI: 10.1016/j.matdes.2022.110665. [60] AL-KETAN O, ABU AL-RUB R K. Multifunctional mechanical metamaterials based on triply periodic minimal surface lattices [J]. Advanced Engineering Materials, 2019, 21(10): 1900524. DOI: 10.1002/adem.201900524. [61] FENG G Z, LI S, XIAO L J, et al. Mechanical properties and deformation behavior of functionally graded TPMS structures under static and dynamic loading [J]. International Journal of Impact Engineering, 2023, 176: 104554. DOI: 10.1016/j.ijimpeng.2023.104554. [62] AL-KETAN O, ABU AL-RUB R K. MSLattice: a free software for generating uniform and graded lattices based on triply periodic minimal surfaces [J]. Material Design & Processing Communications, 2021, 3(6): e205. DOI: 10.1002/mdp2.205. [63] KOOHBOR B, RAVINDRAN S, KIDANE A. In situ deformation characterization of density-graded foams in quasi-static and impact loading conditions [J]. International Journal of Impact Engineering, 2021, 150: 103820. DOI: 10.1016/j.ijimpeng.2021.103820. [64] KOOHBOR B, KIDANE A. Design optimization of continuously and discretely graded foam materials for efficient energy absorption [J]. Materials & Design, 2016, 102: 151–161. DOI: 10.1016/j.matdes.2016.04.031. [65] KOOHBOR B, KIDANE A, LU W Y, et al. Investigation of the dynamic stress-strain response of compressible polymeric foam using a non-parametric analysis [J]. International Journal of Impact Engineering, 2016, 91: 170–182. DOI: 10.1016/j.ijimpeng.2016.01.007. [66] ZHANG J H, CHEN L, WU H, et al. Experimental and mesoscopic investigation of double-layer aluminum foam under impact loading [J]. Composite Structures, 2020, 241: 110859. DOI: 10.1016/j.compstruct.2019.04.031. [67] ZHANG J J, WEI H, WANG Z H, et al. Dynamic crushing of uniform and density graded cellular structures based on the circle arc model [J]. Latin American Journal of Solids and Structures, 2015, 12(6): 1102–1125. DOI: 10.1590/1679-78251630. [68] FAN J H, ZHANG J J, WANG Z H, et al. Dynamic crushing behavior of random and functionally graded metal hollow sphere foams [J]. Materials Science and Engineering: A, 2013, 561: 352–361. DOI: 10.1016/j.msea.2012.10.026. [69] ZHANG J J, WANG Z H, ZHAO L M. Dynamic response of functionally graded cellular materials based on the Voronoi model [J]. Composites Part B: Engineering, 2016, 85: 176–187. DOI: 10.1016/j.compositesb.2015.09.045. [70] LIANG M Z, LI X Y, LIN Y L, et al. Dynamic compressive behaviors of two-layer graded aluminum foams under blast loading [J]. Materials, 2019, 12(9): 1445. DOI: 10.3390/ma12091445. [71] LIU Y, WU H X, WANG B. Gradient design of metal hollow sphere (MHS) foams with density gradients [J]. Composites Part B: Engineering, 2012, 43(3): 1346–1352. DOI: 10.1016/j.compositesb.2011.11.057. [72] KARAGIOZOVA D, ALVES M. Compaction of a double-layered metal foam block impacting a rigid wall [J]. International Journal of Solids and Structures, 2014, 51(13): 2424–2438. DOI: 10.1016/j.ijsolstr.2014.03.012. [73] KARAGIOZOVA D, ALVES M. Stress waves in layered cellular materials—dynamic compaction under axial impact [J]. International Journal of Mechanical Sciences, 2015, 101/102: 196–213. DOI: 10.1016/j.ijmecsci.2015.07.024. [74] 张健, 赵桂平, 卢天健. 梯度泡沫金属的冲击吸能特性 [J]. 工程力学, 2016, 33(8): 211–220. DOI: 10.6052/j.issn.1000-4750.2014.09.0755.ZHANG J, ZHAO G P, LU T J. Energy absorption behaviour of density-graded metallic foam under impact loading [J]. Engineering Mechanics, 2016, 33(8): 211–220. DOI: 10.6052/j.issn.1000-4750.2014.09.0755. [75] YANG J, WANG S L, DING Y Y, et al. Crashworthiness of graded cellular materials: a design strategy based on a nonlinear plastic shock model [J]. Materials Science and Engineering: A, 2017, 680: 411–420. DOI: 10.1016/j.msea.2016.11.010. [76] CHANG B X, ZHENG Z J, ZHANG Y L, et al. Crashworthiness design of graded cellular materials: an asymptotic solution considering loading rate sensitivity [J]. International Journal of Impact Engineering, 2020, 143: 103611. DOI: 10.1016/j.ijimpeng.2020.103611. [77] CHANG B X, ZHENG Z J, ZHANG Y R, et al. Crashworthiness design of graded cellular materials: experimental verification of the backward design strategy [J]. International Journal of Impact Engineering, 2023, 171: 104366. DOI: 10.1016/j.ijimpeng.2022.104366. [78] ZHANG Y R, ZHU Y D, CHANG B X, et al. Blast-loading simulators: multiscale design of graded cellular projectiles considering projectile-beam coupling effect [J]. Journal of the Mechanics and Physics of Solids, 2023, 180: 105402. DOI: 10.1016/j.jmps.2023.105402. [79] WANG X K, ZHENG Z J, YU J L, et al. Impact resistance and energy absorption of functionally graded cellular structures [J]. Applied Mechanics and Materials, 2011, 69: 73–78. DOI: 10.4028/www.scientific.net/AMM.69.73. [80] ZHENG J, QIN Q H, WANG T J. Impact plastic crushing and design of density-graded cellular materials [J]. Mechanics of Materials, 2016, 94: 66–78. DOI: 10.1016/j.mechmat.2015.11.014. [81] SHEN C J, LU G, YU T X, et al. Dynamic response of a cellular block with varying cross-section [J]. International Journal of Impact Engineering, 2015, 79: 53–64. DOI: 10.1016/j.ijimpeng.2014.08.017. [82] SHEN C J, LU G, RUAN D, et al. Propagation of the compaction waves in a cellular block with varying cross-section [J]. International Journal of Solids and Structures, 2016, 88/89: 319–336. DOI: 10.1016/j.ijsolstr.2016.01.014. [83] ZHANG J J, LU G X, RUAN D, et al. Experimental observations of the double shock deformation mode in density graded honeycombs [J]. International Journal of Impact Engineering, 2019, 134: 103386. DOI: 10.1016/j.ijimpeng.2019.103386. [84] RAPAKA S D, PANDEY M, ANNABATTULA R K. Effect of combined gradation in cross-sectional area and density on the dynamic compressive behavior of foams for moderate impact velocities [J]. Mechanics of Materials, 2022, 172: 104381. DOI: 10.1016/j.mechmat.2022.104381. [85] SHEN C J, YU T X, LU G. Double shock mode in graded cellular rod under impact [J]. International Journal of Solids and Structures, 2013, 50(1): 217–233. DOI: 10.1016/j.ijsolstr.2012.09.021. [86] DUAN Y, DING Y, LIU Z Y, et al. Effects of cell size vs. cell-wall thickness gradients on compressive behavior of additively manufactured foams [J]. Composites Science and Technology, 2020, 199: 108339. DOI: 10.1016/j.compscitech.2020.108339. [87] DUAN Y, ZHAO X H, DU B, et al. Quasi-static compressive behavior and constitutive model of graded foams [J]. International Journal of Mechanical Sciences, 2020, 177: 105603. DOI: 10.1016/j.ijmecsci.2020.105603. [88] DUAN Y, ZHAO X H, LIU Z Y, et al. Dynamic response of additively manufactured graded foams [J]. Composites Part B: Engineering, 2020, 183: 107630. DOI: 10.1016/j.compositesb.2019.107630. [89] BAI L, GONG C, CHEN X H, et al. Quasi-static compressive responses and fatigue behaviour of Ti-6Al-4V graded lattice structures fabricated by laser powder bed fusion [J]. Materials & Design, 2021, 210: 110110. DOI: 10.1016/j.matdes.2021.110110. [90] DE WAAL L, LU G X, ZHANG J J, et al. Dynamic behaviour of graded origami honeycomb [J]. International Journal of Impact Engineering, 2021, 157: 103976. DOI: 10.1016/j.ijimpeng.2021.103976. [91] YANG J X, CHEN X H, SUN Y X, et al. Compressive properties of bidirectionally graded lattice structures [J]. Materials & Design, 2022, 218: 110683. DOI: 10.1016/j.matdes.2022.110683. [92] ZAMANI M H, HEIDARI-RARANI M, TORABI K. Optimal design of a novel graded auxetic honeycomb core for sandwich beams under bending using digital image correlation (DIC) [J]. Composite Structures, 2022, 286: 115310. DOI: 10.1016/j.compstruct.2022.115310. [93] 王海任, 李世强, 刘志芳, 等. 爆炸载荷下双向梯度仿生夹芯圆板的力学行为 [J]. 爆炸与冲击, 2021, 41(4): 043201. DOI: 10.11883/bzycj-2020-0132.WANG H R, LI S Q, LIU Z F, et al. Mechanical behaviors of bi-directional gradient bio-inspired circular sandwich plates under blast loading [J]. Explosion and Shock Waves, 2021, 41(4): 043201. DOI: 10.11883/bzycj-2020-0132. [94] LIU H, ZHANG E T, NG B F. In-plane dynamic crushing of a novel honeycomb with functionally graded fractal self-similarity [J]. Composite Structures, 2021, 270: 114106. DOI: 10.1016/j.compstruct.2021.114106. [95] WANG X K, ZHENG Z J, YU J L. Crashworthiness design of density-graded cellular metals [J]. Theoretical and Applied Mechanics Letters, 2013, 3(3): 031001. DOI: 10.1063/2.1303101. [96] ZENG H B, PATTOFATTO S, ZHAO H, et al. Impact behaviour of hollow sphere agglomerates with density gradient [J]. International Journal of Mechanical Sciences, 2010, 52(5): 680–688. DOI: 10.1016/j.ijmecsci.2009.11.012. [97] MATSUMOTO Y, BROTHERS A H, STOCK S R, et al. Uniform and graded chemical milling of aluminum foams [J]. Materials Science and Engineering: A, 2007, 447(1/2): 150–157. DOI: 10.1016/j.msea.2006.10.049. [98] POLLIEN A, CONDE Y, PAMBAGUIAN L, et al. Graded open-cell aluminium foam core sandwich beams [J]. Materials Science and Engineering: A, 2005, 404(1/2): 9–18. DOI: 10.1016/j.msea.2005.05.096. [99] HASSANI A, HABIBOLAHZADEH A, BAFTI H. Production of graded aluminum foams via powder space holder technique [J]. Materials & Design, 2012, 40: 510–515. DOI: 10.1016/j.matdes.2012.04.024. [100] HE S Y, ZHANG Y, DAI G, et al. Preparation of density-graded aluminum foam [J]. Materials Science and Engineering: A, 2014, 618: 496–499. DOI: 10.1016/j.msea.2014.08.087. [101] HE S Y, LV Y N, CHEN S T, et al. Gradient regulation and compressive properties of density-graded aluminum foam [J]. Materials Science and Engineering: A, 2020, 772: 138658. DOI: 10.1016/j.msea.2019.138658. [102] ZHANG Y, ZANG X Y, WANG K, et al. Fabrication of functionally radial graded metallic foam [J]. Materials Letters, 2020, 264: 127292. DOI: 10.1016/j.matlet.2019.127292. [103] JIANG H, COOMES A, ZHANG Z N, et al. Tailoring 3D printed graded architected polymer foams for enhanced energy absorption [J]. Composites Part B: Engineering, 2021, 224: 109183. DOI: 10.1016/j.compositesb.2021.109183. [104] ABDELMAGEED M, CANTWELL W, ZAKI W. Energy absorption and mechanical response of graded face-centered cubic structures [J]. International Journal of Mechanical Sciences, 2024, 273: 109232. DOI: 10.1016/j.ijmecsci.2024.109232. [105] YU S X, SUN J X, BAI J M. Investigation of functionally graded TPMS structures fabricated by additive manufacturing [J]. Materials & Design, 2019, 182: 108021. DOI: 10.1016/j.matdes.2019.108021. [106] BI S R, CHEN E Z, GAITANAROS S. Additive manufacturing and characterization of brittle foams [J]. Mechanics of Materials, 2020, 145: 103368. DOI: 10.1016/j.mechmat.2020.103368. [107] MASKERY I, ABOULKHAIR N T, AREMU A O, et al. A mechanical property evaluation of graded density Al-Si10-Mg lattice structures manufactured by selective laser melting [J]. Materials Science and Engineering: A, 2016, 670: 264–274. DOI: 10.1016/j.msea.2016.06.013. [108] XIAO L J, SONG W D. Additively-manufactured functionally graded Ti-6Al-4V lattice structures with high strength under static and dynamic loading: experiments [J]. International Journal of Impact Engineering, 2018, 111: 255–272. DOI: 10.1016/j.ijimpeng.2017.09.018. [109] LIAO S F, ZHENG Z J, YU J L, et al. A design guide of double-layer cellular claddings for blast alleviation [J]. International Journal of Aerospace and Lightweight Structures, 2013, 3(1): 109–133. DOI: 10.3850/S201042862013000550. [110] LIAO S F, ZHENG Z J, YU J L. On the local nature of the strain field calculation method for measuring heterogeneous deformation of cellular materials [J]. International Journal of Solids and Structures, 2014, 51(2): 478–490. DOI: 10.1016/j.ijsolstr.2013.10.019. [111] DING Y Y, ZHENG Z J, WANG Y G, et al. Impact resistance and design of graded cellular cladding [J]. International Journal of Applied Mechanics, 2018, 10(10): 1850107. DOI: 10.1142/s1758825118501077. [112] 蔡正宇, 丁圆圆, 王士龙, 等. 梯度多胞牺牲层的抗爆炸分析 [J]. 爆炸与冲击, 2017, 37(3): 396–404. DOI: 10.11883/1001-1455(2017)03-0396-09.CAI Z Y, DING Y Y, WANG S L, et al. Anti-blast analysis of graded cellular sacrificial cladding [J]. Explosion and Shock Waves, 2017, 37(3): 396–404. DOI: 10.11883/1001-1455(2017)03-0396-09. [113] DING Y Y, ZHENG Y X, ZHENG Z J, et al. Blast alleviation of sacrificial cladding with graded and uniform cellular materials [J]. Materials, 2020, 13(24): 5616. DOI: 10.3390/ma13245616. [114] WANG P, WANG X K, ZHENG Z J, et al. Stress distribution in graded cellular materials under dynamic compression [J]. Latin American Journal of Solids and Structures, 2017, 14(7): 1251–1272. DOI: 10.1590/1679-78253428. [115] 虞吉林, 余同希, 周风华. 材料和结构的动态吸能 [M]. 合肥: 中国科学技术大学出版社, 2015.YU J L, YU T X, ZHOU F H. Dynamic energy absorption of materials and structures [M]. Hefei: University of Science and Technology of China Press, 2015. [116] CHEN J Y, ZHANG P, CHENG Y S, et al. On the crushing response of the functionally graded metallic foams based on 3D Voronoi model [J]. Thin-Walled Structures, 2020, 157: 107085. DOI: 10.1016/j.tws.2020.107085. [117] LIU Y, WU H X, LU G X, et al. Dynamic properties of density graded thin-walled metal hollow sphere arrays [J]. Mechanics of Advanced Materials and Structures, 2013, 20(6): 478–488. DOI: 10.1080/15376494.2011.627642. [118] SHEN C J, LU G, YU T X. Dynamic behavior of graded honeycombs—a finite element study [J]. Composite Structures, 2013, 98: 282–293. DOI: 10.1016/j.compstruct.2012.11.002. [119] KARAGIOZOVA D, ZHANG J J, CHEN P W, et al. Response of graded Miura-Ori metamaterials to quasi-static and dynamic in-plane compression [J]. Journal of Aerospace Engineering, 2022, 35(4). DOI: 10.1061/(asce)as.1943-5525.0001416. [120] REID S R, REDDY T Y. Experimental investigation of inertia effects in one-dimensional metal ring systems subjected to end impact— Ⅰ. fixed-ended systems [J]. International Journal of Impact Engineering, 1983, 1(1): 85–106. DOI: 10.1016/0734-743X(83)90014-3. [121] HARRIGAN J J, REID S R, TAN P J, et al. High rate crushing of wood along the grain [J]. International Journal of Mechanical Sciences, 2005, 47(4/5): 521–544. DOI: 10.1016/j.ijmecsci.2004.12.013. [122] ZHENG Z J, LIU Y D, YU J L, et al. Dynamic crushing of cellular materials: continuum-based wave models for the transitional and shock modes [J]. International Journal of Impact Engineering, 2012, 42: 66–79. DOI: 10.1016/j.ijimpeng.2011.09.009. [123] PATTOFATTO S, ELNASRI I, ZHAO H, et al. Shock enhancement of cellular structures under impact loading: part Ⅱ analysis [J]. Journal of the Mechanics and Physics of Solids, 2007, 55(12): 2672–2686. DOI: 10.1016/j.jmps.2007.04.004. [124] ZHENG Z J, YU J L, WANG C F, et al. Dynamic crushing of cellular materials: a unified framework of plastic shock wave models [J]. International Journal of Impact Engineering, 2013, 53: 29–43. DOI: 10.1016/j.ijimpeng.2012.06.012. [125] LOPATNIKOV S L, GAMA B A, JAHIRUL HAQUE M, et al. Dynamics of metal foam deformation during Taylor cylinder-Hopkinson bar impact experiment [J]. Composite Structures, 2003, 61(1/2): 61–71. DOI: 10.1016/S0263-8223(03)00039-4. [126] LOPATNIKOV S L, GAMA B A, HAQUE M J, et al. High-velocity plate impact of metal foams [J]. International Journal of Impact Engineering, 2004, 30(4): 421–445. DOI: 10.1016/S0734-743X(03)00066-6. [127] LOPATNIKOV S L, GAMA B A, GILLESPIE JR J W. Modeling the progressive collapse behavior of metal foams [J]. International Journal of Impact Engineering, 2007, 34(3): 587–595. DOI: 10.1016/j.ijimpeng.2005.12.004. [128] HARRIGAN J J, REID S R, YAGHOUBI A S. The correct analysis of shocks in a cellular material [J]. International Journal of Impact Engineering, 2010, 37(8): 918–927. DOI: 10.1016/j.ijimpeng.2009.03.011. [129] WANG S L, DING Y Y, WANG C F, et al. Dynamic material parameters of closed-cell foams under high-velocity impact [J]. International Journal of Impact Engineering, 2017, 99: 111–121. DOI: 10.1016/j.ijimpeng.2016.09.013. [130] DING Y Y, WANG S L, ZHAO K, et al. Blast alleviation of cellular sacrificial cladding: a nonlinear plastic shock model [J]. International Journal of Applied Mechanics, 2016, 8(4): 1650057. DOI: 10.1142/s1758825116500575. [131] DING Y Y, WANG S L, ZHENG Z J, et al. Dynamic crushing of cellular materials: a unique dynamic stress-strain state curve [J]. Mechanics of Materials, 2016, 100: 219–231. DOI: 10.1016/j.mechmat.2016.07.001. [132] BARNES A T, RAVI-CHANDAR K, KYRIAKIDES S, et al. Dynamic crushing of aluminum foams: part Ⅰ—experiments [J]. International Journal of Solids and Structures, 2014, 51(9): 1631–1645. DOI: 10.1016/j.ijsolstr.2013.11.019. [133] GAITANAROS S, KYRIAKIDES S. Dynamic crushing of aluminum foams: part Ⅱ—analysis [J]. International Journal of Solids and Structures, 2014, 51(9): 1646–1661. DOI: 10.1016/j.ijsolstr.2013.11.020. [134] GAITANAROS S, KYRIAKIDES S. On the effect of relative density on the crushing and energy absorption of open-cell foams under impact [J]. International Journal of Impact Engineering, 2015, 82: 3–13. DOI: 10.1016/j.ijimpeng.2015.03.011. [135] WANG S L, ZHENG Z J, ZHU C F, et al. Crushing and densification of rapid prototyping polylactide foam: meso-structural effect and a statistical constitutive model [J]. Mechanics of Materials, 2018, 127: 65–76. DOI: 10.1016/j.mechmat.2018.09.003. [136] MA G W, YE Z Q. Energy absorption of double-layer foam cladding for blast alleviation [J]. International Journal of Impact Engineering, 2007, 34(2): 329–347. DOI: 10.1016/j.ijimpeng.2005.07.012. [137] SHEN C J, LU G, YU T X. Investigation into the behavior of a graded cellular rod under impact [J]. International Journal of Impact Engineering, 2014, 74: 92–106. DOI: 10.1016/j.ijimpeng.2014.02.015. [138] ZHANG H, CHANG B X, PENG K F, et al. Anti-blast analysis and design of a sacrificial cladding with graded foam-filled tubes [J]. Thin-Walled Structures, 2023, 182: 110313. DOI: 10.1016/j.tws.2022.110313. [139] LIU H, DING S R, NG B F. Impact response and energy absorption of functionally graded foam under temperature gradient environment [J]. Composites Part B: Engineering, 2019, 172: 516–532. DOI: 10.1016/j.compositesb.2019.05.072. [140] GUPTA V, KIDANE A, SUTTON M. Closed-form solution for shock wave propagation in density-graded cellular material under impact [J]. Theoretical and Applied Mechanics Letters, 2021, 11(5): 100288. DOI: 10.1016/j.taml.2021.100288. [141] RAPAKA S D, PANDEY M, ANNABATTULA R K. Theoretical analysis on the dynamic compressive behavior of cellular solids with non-linear variation in cross-sectional area [J]. International Journal of Impact Engineering, 2021, 155: 103921. DOI: 10.1016/j.ijimpeng.2021.103921. [142] CHENG Q, YIN J F, WEN J H, et al. Triply periodic minimal surface structures: energy absorption performance under impact loading and their graded design [J]. Mechanics of Advanced Materials and Structures. DOI: 10.1080/15376494.2024.2311237. [143] CUI L, KIERNAN S, GILCHRIST M D. Designing the energy absorption capacity of functionally graded foam materials [J]. Materials Science and Engineering: A, 2009, 507(1/2): 215–225. DOI: 10.1016/j.msea.2008.12.011. [144] AJDARI A, NAYEB-HASHEMI H, VAZIRI A. Dynamic crushing and energy absorption of regular, irregular and functionally graded cellular structures [J]. International Journal of Solids and Structures, 2011, 48(3/4): 506–516. DOI: 10.1016/j.ijsolstr.2010.10.018. [145] ZHANG X, ZHANG H. Optimal design of functionally graded foam material under impact loading [J]. International Journal of Mechanical Sciences, 2013, 68: 199–211. DOI: 10.1016/j.ijmecsci.2013.01.016. [146] YANG J, WANG S L, ZHENG Z J, et al. Impact resistance of graded cellular metals using cell-based finite element models [J]. Key Engineering Materials, 2016, 703: 400–405. DOI: 10.4028/www.scientific.net/KEM.703.400. [147] LIU H, ZHANG Z Q, LIU H, et al. Theoretical investigation on impact resistance and energy absorption of foams with nonlinearly varying density [J]. Composites Part B: Engineering, 2017, 116: 76–88. DOI: 10.1016/j.compositesb.2017.02.012. [148] 常白雪, 郑志军, 赵凯, 等. 梯度多胞材料耐撞性设计的简化模型和渐近解 [J]. 中国科学: 物理学 力学 天文学, 2018, 48(9): 094615. DOI: 10.1360/SSPMA2018-00162.CHANG B X, ZHENG Z J, ZHAO K, et al. A simplified model and its asymptotic solution for the crashworthiness design of graded cellular material [J]. Scientia Sinica Physica, Mechanica & Astronomica, 2018, 48(9): 094615. DOI: 10.1360/SSPMA2018-00162. [149] 余同希, 卢国兴, 张雄. 能量吸收: 结构与材料的力学行为和塑性分析 [M]. 北京: 科学出版社, 2019. [150] RAVINDRAN S, KOOHBOR B, MALCHOW P, et al. Experimental characterization of compaction wave propagation in cellular polymers [J]. International Journal of Solids and Structures, 2018, 139/140: 270–282. DOI: 10.1016/j.ijsolstr.2018.02.003. [151] LIU J G, HOU B, LU F Y, et al. A theoretical study of shock front propagation in the density graded cellular rods [J]. International Journal of Impact Engineering, 2015, 80: 133–142. DOI: 10.1016/j.ijimpeng.2015.02.001. [152] 常白雪, 郑志军, 赵凯, 等. 具有恒定冲击载荷的梯度泡沫金属材料设计 [J]. 爆炸与冲击, 2019, 39(4): 041101. DOI: 10.11883/bzycj-2018-0431.CHANG B X, ZHENG Z J, ZHAO K, et al. Design of gradient foam metal materials with a constant impact load [J]. Explosion and Shock Waves, 2019, 39(4): 041101. DOI: 10.11883/bzycj-2018-0431. [153] LI D, HOU H L, CHEN C H, et al. Experimental study on the combined damage of multi-layered composite structures subjected to close-range explosion of simulated warheads [J]. International Journal of Impact Engineering, 2018, 114: 133–146. DOI: 10.1016/j.ijimpeng.2017.12.007. [154] 赵著杰, 侯海量, 李典. 填充多胞元抗冲击防护结构动力学特性及防护性能研究进展 [J]. 中国舰船研究, 2021, 16(3): 96–111. DOI: 10.19693/j.issn.1673-3185.02053.ZHAO Z J, HOU H L, LI D. Research progress on dynamic characteristics and protective performance of multicellular filled impact resistant protective structure [J]. Chinese Journal of Ship Research, 2021, 16(3): 96–111. DOI: 10.19693/j.issn.1673-3185.02053. [155] ZHOU H Y, WANG Y H, WANG X J, et al. Energy absorption of graded foam subjected to blast: a theoretical approach [J]. Materials & Design, 2015, 84: 351–358. DOI: 10.1016/j.matdes.2015.06.124. [156] XIA Y, WU C Q, LIU Z X, et al. Protective effect of graded density aluminium foam on RC slab under blast loading—an experimental study [J]. Construction and Building Materials, 2016, 111: 209–222. DOI: 10.1016/j.conbuildmat.2016.02.092. [157] YIN C Y, JIN Z Y, CHEN Y, et al. The underwater blast resistance of sacrificial claddings with stepwise graded cellular cores [J]. Journal of Offshore Mechanics and Arctic Engineering, 2017, 139(2): 021602. DOI: 10.1115/1.4034922. [158] LIANG M Z, LI Z B, LU F Y, et al. Theoretical and numerical investigation of blast responses of continuous-density graded cellular materials [J]. Composite Structures, 2017, 164: 170–179. DOI: 10.1016/j.compstruct.2016.12.065. [159] LAN X K, FENG S S, HUANG Q, et al. Blast response of continuous-density graded cellular material based on the 3D Voronoi model [J]. Defence Technology, 2018, 14(5): 433–440. DOI: 10.1016/j.dt.2018.06.003. [160] LIANG M Z, LI X Y, LIN Y L, et al. Compaction wave propagation in layered cellular materials under air-blast [J]. International Journal of Applied Mechanics, 2019, 11(1): 1950003. DOI: 10.1142/S1758825119500030. [161] PENG C X, TRAN P. Bioinspired functionally graded gyroid sandwich panel subjected to impulsive loadings [J]. Composites Part B: Engineering, 2020, 188: 107773. DOI: 10.1016/j.compositesb.2020.107773. [162] NOVAK N, BOROVINŠEK M, AL-KETAN O, et al. Impact and blast resistance of uniform and graded sandwich panels with TPMS cellular structures [J]. Composite Structures, 2022, 300: 116174. DOI: 10.1016/j.compstruct.2022.116174. [163] RADFORD D D, DESHPANDE V S, FLECK N A. The use of metal foam projectiles to simulate shock loading on a structure [J]. International Journal of Impact Engineering, 2005, 31(9): 1152–1171. DOI: 10.1016/j.ijimpeng.2004.07.012. [164] CHEN A, KIM H, ASARO R J, et al. Non-explosive simulated blast loading of balsa core sandwich composite beams [J]. Composite Structures, 2011, 93(11): 2768–2784. DOI: 10.1016/j.compstruct.2011.05.027. [165] LI L, ZHANG Q C, ZHANG R, et al. A laboratory experimental technique for simulating combined blast and impact loading [J]. International Journal of Impact Engineering, 2019, 134: 103382. DOI: 10.1016/j.ijimpeng.2019.103382. [166] RADFORD D D, FLECK N A, DESHPANDE V S. The response of clamped sandwich beams subjected to shock loading [J]. International Journal of Impact Engineering, 2006, 32(6): 968–987. DOI: 10.1016/j.ijimpeng.2004.08.007. [167] RADFORD D D, MCSHANE G J, DESHPANDE V S, et al. The response of clamped sandwich plates with metallic foam cores to simulated blast loading [J]. International Journal of Solids and Structures, 2006, 43(7/8): 2243–2259. DOI: 10.1016/j.ijsolstr.2005.07.006. [168] 敬霖, 王志华, 赵隆茂, 等. 撞击载荷下泡沫铝夹芯梁的塑性动力响应 [J]. 爆炸与冲击, 2010, 30(6): 561–568. DOI: 10.11883/1001-1455(2010)06-0561-08.JING L, WANG Z H, ZHAO L M, et al. Dynamic plastic response of foam sandwich beams subjected to impact loading [J]. Explosion and Shock Waves, 2010, 30(6): 561–568. DOI: 10.11883/1001-1455(2010)06-0561-08. [169] 宋延泽, 王志华, 赵隆茂, 等. 撞击载荷下泡沫铝夹层板的动力响应 [J]. 爆炸与冲击, 2010, 30(3): 301–307. DOI: 10.11883/1001-1455(2010)03-0301-07.SONG Y Z, WANG Z H, ZHAO L M, et al. Dynamic response of foam sandwich plates subjected to impact loading [J]. Explosion and Shock Waves, 2010, 30(3): 301–307. DOI: 10.11883/1001-1455(2010)03-0301-07. [170] JING L, WANG Z H, NING J G, et al. The mechanical response of metallic sandwich beams under foam projectile impact loading [J]. Latin American Journal of Solids and Structures, 2011, 8(1): 107–120. DOI: 10.1590/S1679-78252011000100006. [171] JING L, WANG Z H, NING J G, et al. The dynamic response of sandwich beams with open-cell metal foam cores [J]. Composites Part B: Engineering, 2011, 42(1): 1–10. DOI: 10.1016/j.compositesb.2010.09.024. [172] XIE Q H, JING L, WANG Z H, et al. Deformation and failure of clamped shallow sandwich arches with foam core subjected to projectile impact [J]. Composites Part B: Engineering, 2013, 44(1): 330–338. DOI: 10.1016/j.compositesb.2012.04.070. [173] JING L, WANG Z H, ZHAO L M. Response of metallic cylindrical sandwich shells subjected to projectile impact—experimental investigations [J]. Composite Structures, 2014, 107: 36–47. DOI: 10.1016/j.compstruct.2013.07.011. [174] JING L, WANG Z H, ZHAO L M. The dynamic response of sandwich panels with cellular metal cores to localized impulsive loading [J]. Composites Part B: Engineering, 2016, 94: 52–63. DOI: 10.1016/j.compositesb.2016.03.035. [175] LI X, LI S Q, WANG Z H, et al. Response of aluminum corrugated sandwich panels under foam projectile impact—experiment and numerical simulation [J]. Journal of Sandwich Structures & Materials, 2017, 19(5): 595–615. DOI: 10.1177/1099636216630503. [176] LI X, ZHANG P W, LI S Q, et al. Dynamic response of aluminum honeycomb sandwich panels under foam projectile impact [J]. Mechanics of Advanced Materials and Structures, 2018, 25(8): 637–646. DOI: 10.1080/15376494.2017.1308595. [177] 叶楠, 张伟, 黄威, 等. PVC夹芯板在冲击载荷下的动态响应与失效模式 [J]. 爆炸与冲击, 2017, 37(1): 37–45. DOI: 10.11883/1001-1455(2017)01-0037-09.YE N, ZHANG W, HUANG W, et al. Dynamic response and failure mode of PVC sandwich plates subjected to impact loading [J]. Explosion and Shock Waves, 2017, 37(1): 37–45. DOI: 10.11883/1001-1455(2017)01-0037-09. [178] YE N, ZHANG W, LI D C, et al. Dynamic response and failure of sandwich plates with PVC foam core subjected to impulsive loading [J]. International Journal of Impact Engineering, 2017, 109: 121–130. DOI: 10.1016/j.ijimpeng.2017.06.005. [179] XIAO D B, CHEN X Q, LI Y, et al. The structure response of sandwich beams with metallic auxetic honeycomb cores under localized impulsive loading—experiments and finite element analysis [J]. Materials & Design, 2019, 176: 107840. DOI: 10.1016/j.matdes.2019.107840. [180] LI Y, CHEN Z H, XIAO D B, et al. The Dynamic response of shallow sandwich arch with auxetic metallic honeycomb core under localized impulsive loading [J]. International Journal of Impact Engineering, 2020, 137: 103442. DOI: 10.1016/j.ijimpeng.2019.103442. [181] 熊飞扬, 高松林, 李晓彬, 等. 局部冲击载荷作用下星形蜂窝夹芯梁的动态响应研究 [J]. 武汉理工大学学报(交通科学与工程版), 2020, 44(2): 388–392. DOI: 10.3963/j.issn.2095-3844.2020.02.036.XIONG F Y, GAO S L, LI X B, et al. Study on dynamic response of star honeycomb sandwich beam under local impact load [J]. Journal of Wuhan University of Technology (Transportation Science & Engineering), 2020, 44(2): 388–392. DOI: 10.3963/j.issn.2095-3844.2020.02.036. [182] CHEN Z H, LIU L W, GAO S L, et al. Dynamic response of sandwich beam with star-shaped reentrant honeycomb core subjected to local impulsive loading [J]. Thin-Walled Structures, 2021, 161: 107420. DOI: 10.1016/j.tws.2020.107420. [183] 张元瑞, 朱玉东, 郑志军, 等. 泡沫子弹冲击固支单梁的耦合分析模型 [J]. 力学学报, 2022, 54(8): 2161–2172. DOI: 10.6052/0459-1879-22-223.ZHANG Y R, ZHU Y D, ZHENG Z J, et al. A coupling analysis model of clamped monolithic beam impacted by foam projectiles [J]. Chinese Journal of Theoretical and Applied Mechanics, 2022, 54(8): 2161–2172. DOI: 10.6052/0459-1879-22-223. [184] LI L, HAN B, HE S Y, et al. Shock loading simulation using density-graded metallic foam projectiles [J]. Materials & Design, 2019, 164: 107546. DOI: 10.1016/j.matdes.2018.107546. -






 下载:
下载: