Theoretical calculation of shock compression properties of MESMs with electronic thermal motion effect
-
摘要: 为了更好地描述疏松态金属材料的冲击压缩特性，基于托马斯-费米原子统计模型，研究金属晶体中电子热行为对系统内粒子数、内能、压强等参数的影响，修改了描述疏松金属材料的Wu-Jing模型中的参数R的计算方法。结合混合物的冷能叠加原理，得到考虑电子相影响的疏松态混合物物态方程。并对不同配比的密实态W/Cu合金、不同疏松度的Al/Ni合金的典型多功能含能结构材料进行计算，获得其冲击压力-比容关系及冲击波速度-粒子速度关系，计算结果与实验结果吻合较好。结果表明，本文中模型对未反应条件下的金属材料冲击压缩特性预测较好；疏松材料的冲击压力-粒子速度关系并不呈现出密实材料的近似线性关系，其冲击压缩过程分为压实前和压实后2个明显的阶段；多功能含能结构材料的冲击压缩特性受材料孔隙率、材料配比等影响明显。Abstract: In this work, based on the Thomas-Fermi statistical model, we modified the calculation method of the Wu-Jing parameters R and investigated the effect of the electronic thermal motion on such parameters as the particle number, the internal energy, and the pressure, inside the metallic crystal structure so as to truly describe the shock compression properties of porous metal materials. A new equation of state was developed for porous materials in which the contribution of the electronic phases was considered explicitly under the condition of different porosity. The relationship between pressure and particle velocity, shock wave velocity and particle velocity was obtained for typical MESMs, for instance different components of W/Cu alloy (dense) and different dense degrees of Al/Ni alloy. Compared with existing models, the equation of state established in this paper is better fitted with the experimental results. The results show that this model can be used to predict the shock compression properties of metal materials under unreacted conditions. The us-up relationship of porous materials does not exhibit an approximate linear relationship as solid materials, due to the shock compression characteristics that are divided into two distinct phases before and after compaction. The shock compression characteristics of multi-functional energetic structure materials are obviously affected by the porosity and material ratio.
-
Key words:
- MESMs /
- equation of state /
- electronic thermal motion of impact /
- porous materials
1) “第十一届全国爆炸力学学术会议”推荐论文 -
图 1 不同配比合金的压力与比容关系
Figure 1. Relationship between pressure and specific volume for the alloy with different content ratios
图 2 不同配比合金的us-up拟合曲线
Figure 2. us-up fitted curve of alloy with different content ratios
图 3 不同理论密实度时Al/Ni的us-up拟合关系
Figure 3. us-up fitted curves of Al/Ni materials with different dense degrees
-
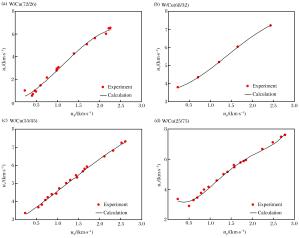
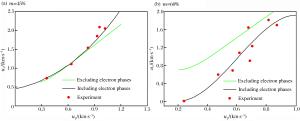
[1] 许世昌. 双层含能药型罩射流成型机理及侵彻性能研究[D]. 南京: 南京理工大学, 2015. http://cdmd.cnki.com.cn/Article/CDMD-10288-1015358660.htm [2] JORDAN J L, DICK R D, FERRANTI L, et al. Equation of state of aluminum-iron oxide (Fe2O3) epoxy composite: Modeling and experiment[C]//Proceedings of the Conference of the American Physical Society Topical Group on Shock Compression of Condense Matter. Baltimore, Maryland, United States, 2005. http://meetings.aps.org/link/BAPS.2005.SHOCK.T3.1 [3] JORDAN J L, HERBOLD E B, SUTHERLAND G, et al. Shock equation of state of multi-constituent epoxy-metal particulate composites[J]. Journal of Applied Physics, 2011, 109(1):013531.DOI: 10.1063/1.3531579. [4] EAKINS D E, THADHANI N N. Mechanistic aspects of shock-induced reactions in Ni+Al powder mixtures[C]//Proceedings of the Conference of the American Physical Society Topical Group on Shock Compression of Condensed Matter. Waikoloa, Hawaii, United states, 2007. http://meetings.aps.org/link/BAPS.2007.SHOCK.C6.4 [5] XU X, THADHANI N N. Investigation of shock-induced reaction behavior of as-blended and ball-milled Ni plus Ti powder mixtures using time-resolved stress measurements[J]. Journal of Applied Physics, 2004, 96(4):2000-2009. doi: 10.1063/1.1773380 [6] 张先锋, 赵晓宁, 乔良.反应金属冲击反应过程的理论分析[J].爆炸与冲击, 2010, 30(2):145-151. doi: 10.11883/1001-1455(2010)02-0145-07ZHANG Xianfeng, ZHAO Xiaoning, QIAO Liang. Theory analysis on shock-induced chemical reaction of reactive metal[J]. Explosion and Shock Waves, 2010, 30(2):145-151. doi: 10.11883/1001-1455(2010)02-0145-07 [7] 史安顺. 多功能含能结构材料冲击压缩特性及其反应行为研究[D]. 南京: 南京理工大学, 2013. http://cdmd.cnki.com.cn/Article/CDMD-10288-1013165207.htm [8] 经福谦.实验物态方程导引[M].北京:科学出版社, 1986. [9] 汤文辉.物态方程理论及计算概论[M].长沙:国防科技大学出版社, 1999. [10] WU Qiang, JING Fuqian. Thermodynamic equation of state and application to Hugoniot predictions for porous materials[J]. Journal of Applied Physics, 1996, 80(8):4343-4351.DOI: 10.1063/1.363391. [11] CARROLL M M, HOLT A C. Static and dynamic pore-collapse relations for ductile porous materials[J]. Journal of Applied Physics, 1972, 43(4):1626-1636. doi: 10.1063/1.1661372 [12] ZEL'DOVICH Y B, RAIZER Y P, HAYES W D, et al. Physics of shock waves and high-temperature hydrodynamic phenomena[M]. New York: Dover Publications, 2002. -
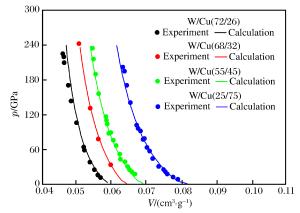
下载:
图(3) / 表(1)
计量
- 文章访问数: 5271
- HTML全文浏览量: 1954
- PDF下载量: 163
- 被引次数: 0