基于饱和响应时间的封闭空间内爆炸载荷等效方法研究

孔祥韶; 周沪; 郑成; 吴卫国

doi:10.11883/bzycj-2018-0183

基于饱和响应时间的封闭空间内爆炸载荷等效方法研究

doi: 10.11883/bzycj-2018-0183

孔祥韶^{1, 2,},
周沪^{1, 2},
郑成^{1, 2},
吴卫国^{1, 2, ,}

1.
武汉理工大学高性能船舶技术教育部重点实验室，湖北武汉 430063
2.
武汉理工大学交通学院，湖北武汉 430063

基金项目: 装备预研教育部联合基金青年人才项目（6141A02033108）；国家自然科学基金（11502180）

详细信息

作者简介:
孔祥韶（1983－　），男，博士，副教授, kongxs@whut.edu.cn

通讯作者:
吴卫国（1960－　），男，硕士，教授, mailjt@163.com

中图分类号: O383
计量
- 文章访问数: 5980
- HTML全文浏览量: 1844
- PDF下载量: 75
- 被引次数: 0
出版历程
- 收稿日期: 2018-05-27
- 修回日期: 2019-01-25
- 刊出日期: 2019-09-01

An equivalent calculation method for confined-blast load based on saturated response time

KONG Xiangshao^{1, 2
,},
ZHOU Hu^{1, 2},
ZHENG Cheng^{1, 2},
WU Weiguo^{1, 2
, ,}

1.
Key Laboratory of High Performance Ship Technology, Wuhan University of Technology, Ministry of Education, Wuhan 430063, Hibei, China
2.
School of Transportation, Wuhan University of Technology, Wuhan 430063, Hibei, China

摘要

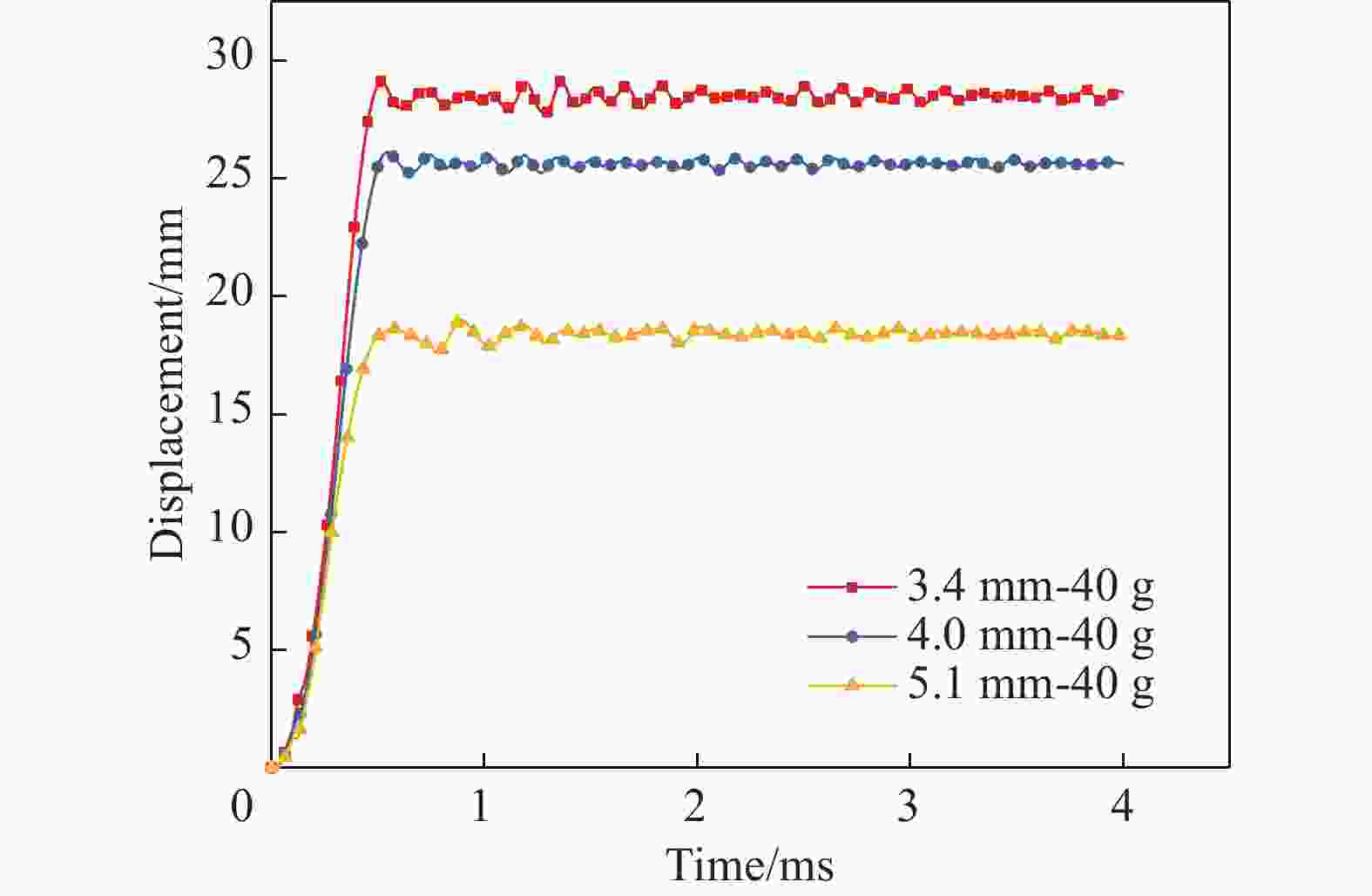
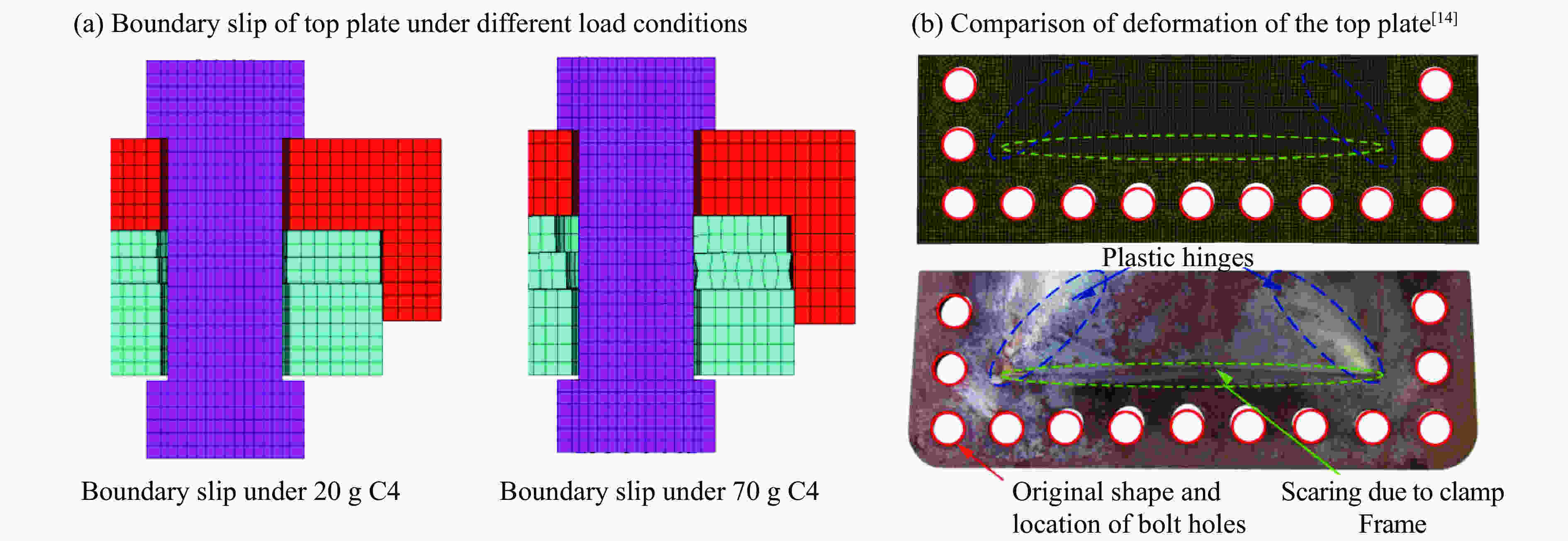
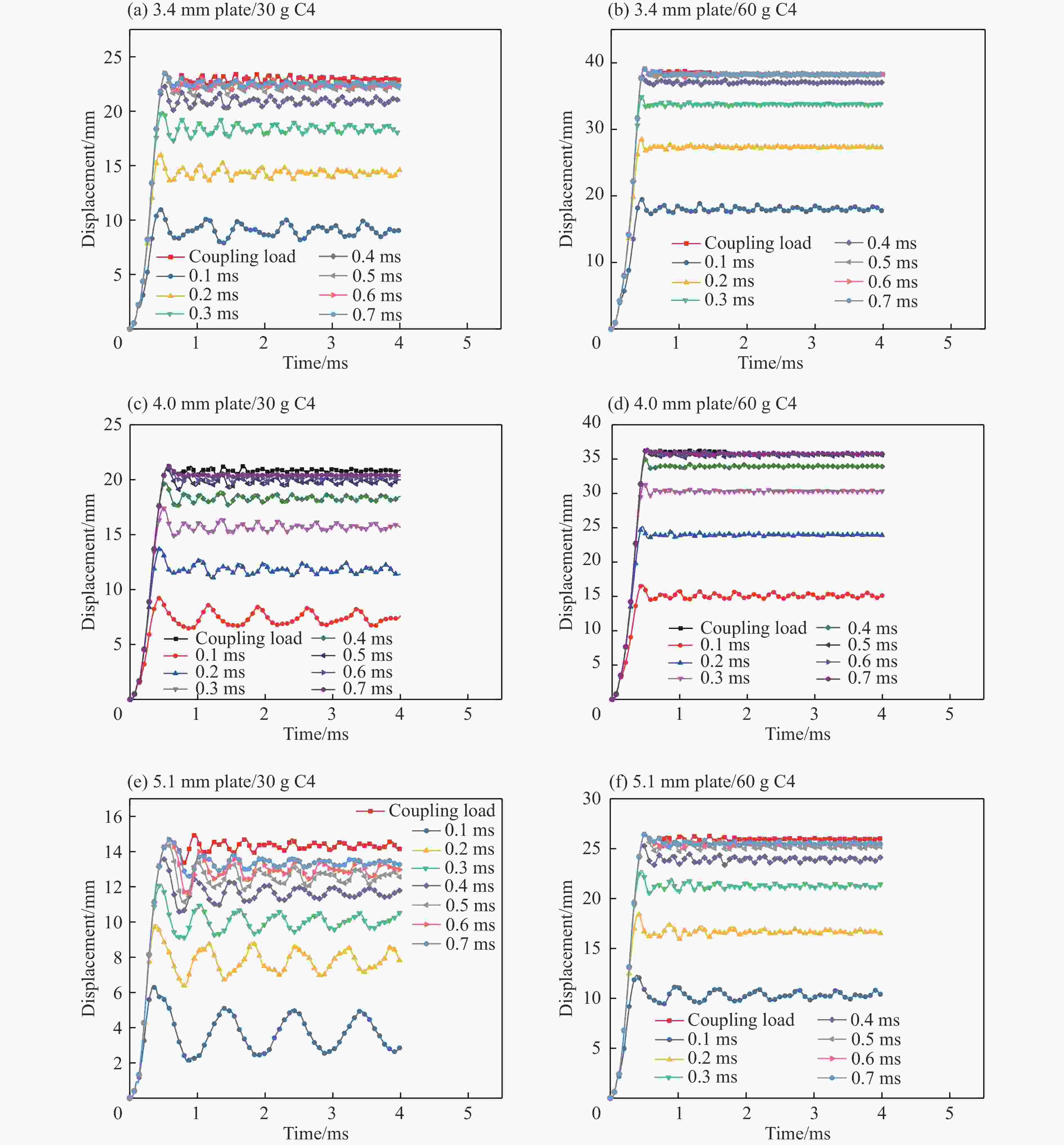
摘要: 针对舱内爆炸载荷形式复杂、作用时间长、缺乏有效的简化描述方法的问题，首先采用显式动力学计算程序开展了内爆载荷作用下钢板动态响应的数值计算，在与试验结果对比验证的基础上分析了金属板的内爆载荷饱和冲量。通过对216种不同爆炸载荷加载时长与金属板响应关系的分析，提出了内爆炸载荷作用下结构最大变形所对应的饱和时间计算经验公式，并给出了饱和时间的无量纲系数建议值。考虑到内爆载荷初始冲击波的影响，结合爆炸载荷饱和作用时间的规律，提出了封闭空间爆炸载荷的矩形载荷等效方法，对比了18组简化载荷与耦合载荷分别作用下钢板的动力响应，验证了等效方法的有效性。
- 舱内爆炸 /
- 金属板 /
- 饱和冲量 /
- 等效方法
Abstract: Due to the complex form and long duration of the load from a confined explosion, it is usually difficult to propose an uniform simplified formula to describe the confined blast load effectively, which can be applied in the predicting the dynamic response of structures. In present paper, the explicit dynamic code Autodyn was employed to predict the response of steel plates under confined blast load numerically. The effectiveness of the numerical method was validated by comparing the numerical and experimental results, and then the characteristic of saturated impulse of steel plate was analyzed. The numerical simulations of 216 plates, which experience different load durations, were conducted. Based on analyzing the relationship between the duration of confined blast load and the subsequent dynamic response of these steel plates, a simplified formula was deduced to determine the saturated time that corresponding to the maximum deformation of plate, and a guide value of the parameter of the dimensionless saturated time was presented. Considering the influence of initial shock wave of confined explosion, combined with the law of saturation action time of explosion load, a rectangular load equivalent method for the confined blast load is proposed. The dynamic response of metal plate under 18 groups of simplified and fully-coupling load is compared, and the effectiveness of the equivalent method is verified.
- confined-blast load /
- steel plate /
- saturated impulse /
- equivalent method

HTML全文

图 1 内爆试验装置^{[4, 14]}

Figure 1. Experimental setup of confined explosion ^{[4, 14]}

下载: 全尺寸图片幻灯片

图 2 1/4对称模型及坐标轴

Figure 2. 1/4 symmetry model and coordinate system

下载: 全尺寸图片幻灯片

图 3 模型焊缝细节

Figure 3. Local detail model of welding line

下载: 全尺寸图片幻灯片

图 4 计算模型

Figure 4. Computational model

下载: 全尺寸图片幻灯片

图 5 三种板厚在40 g药量下顶板中心点响应计算

Figure 5. Response of the center point of top plates with three kinds of thickness under 40 g explosive

下载: 全尺寸图片幻灯片

图 6 数值计算值与试验值对比

Figure 6. Comparison between computational and experimental results

下载: 全尺寸图片幻灯片

图 7 不同载荷下侧板连接处变形

Figure 7. Deformation of the side plate at the joint under different loads

下载: 全尺寸图片幻灯片

图 8 顶板边界滑移与变形

Figure 8. Boundary slip and deformation of the top plate

下载: 全尺寸图片幻灯片

图 9 不同加载时长工况中点响应对比

Figure 9. Comparison of central point responses of plates with different loading times

下载: 全尺寸图片幻灯片

图 10 不同加载时长下响应峰值时刻对比

Figure 10. Response peak time comparison under different loading times

下载: 全尺寸图片幻灯片

图 11 爆炸载荷与等效矩形载荷

Figure 11. Explosive load and equivalent rectangular load

下载: 全尺寸图片幻灯片

图 12 等效载荷施加示意图

Figure 12. Sketch of applied equivalent load

下载: 全尺寸图片幻灯片

图 13 全耦合载荷与简化载荷计算对比

Figure 13. Comparison of fully coupled load and simplified load calculation

下载: 全尺寸图片幻灯片

图 14 简化矩形载荷作用下顶板中心点位移计算结果对比

Figure 14. Comparison of center point displacements of top plates under simplified rectangular load

下载: 全尺寸图片幻灯片

表 1 Johnson-Cook模型材料参数

Table 1. Material parameters of Johnson-Cook model

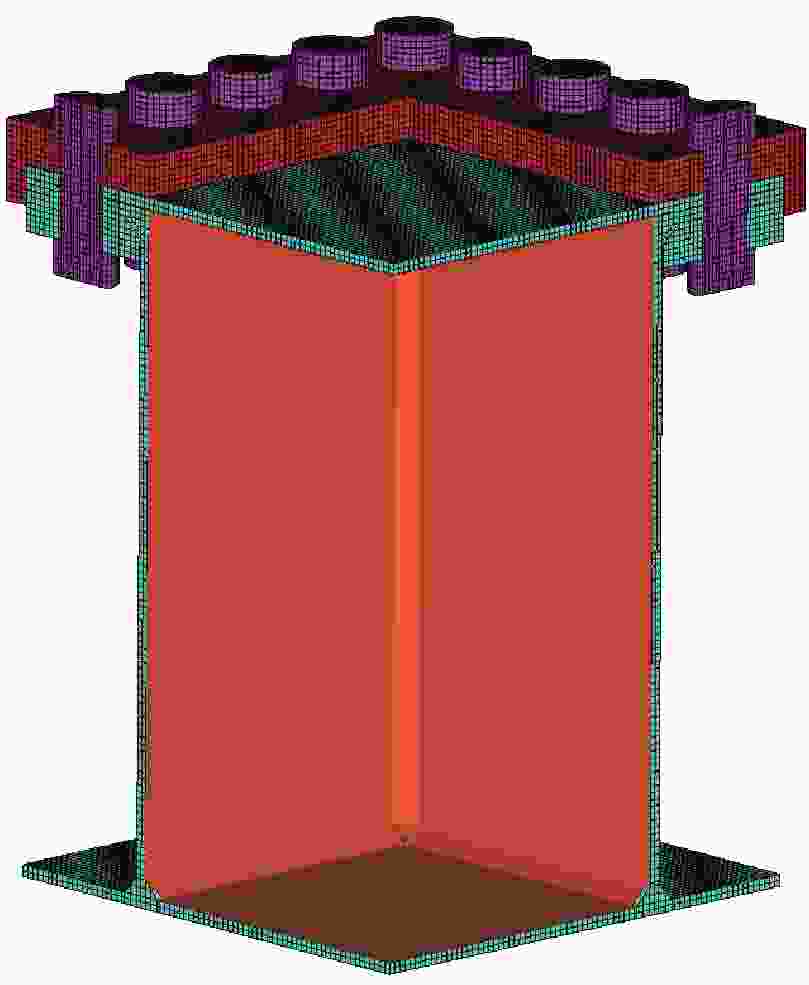
板厚/mm	材料	A/MPa	B/MPa	n	C	m
3.4	低碳钢	233.47	480.37	0.356 5	0.036 9	0.665 5
4.0	低碳钢	221.67	361.35	0.474 6	0.048 1	0.665 5
5.1	300WAsteel	263.58	519.64	0.384 3	0.025 9	0.665 5

下载: 导出CSV

表 2 夹持结构材料参数

Table 2. Material parameters of holding device

部件	状态方程	刚体约束	强度模型	体积模量/GPa	剪切模量/GPa
上压板	Rigid	No	−	159	81.8
下压板	Rigid	Yes	−	159	81.8
螺栓	Linear	−	Elastic	159	81.8

下载: 导出CSV

表 3 C4炸药JWL状态方程参数

Table 3. JWL EOS parameters of explosive C4

ρ/(g·cm⁻³)	A/GPa	B/GPa	R₁	R₂	w	E/(GJ·m⁻³)
1.601	609.8	12.95	4.500	1.400	0.250	9.000

下载: 导出CSV

表 4 无量纲饱和冲量参数

Table 4. Parameters of dimensionless saturation impulse

板厚/mm	饱和时间/ms	板长/m	材料密度/（g·cm⁻³）	屈服强度/MPa	λ
3.4	0.6	0.2	7.83	233.47	16.4
4.0	0.6	0.2	7.83	221.67	16.0
5.1	0.6	0.2	7.83	263.58	17.4

下载: 导出CSV

表 5 等效矩形载荷换算

Table 5. Equivalent rectangular load

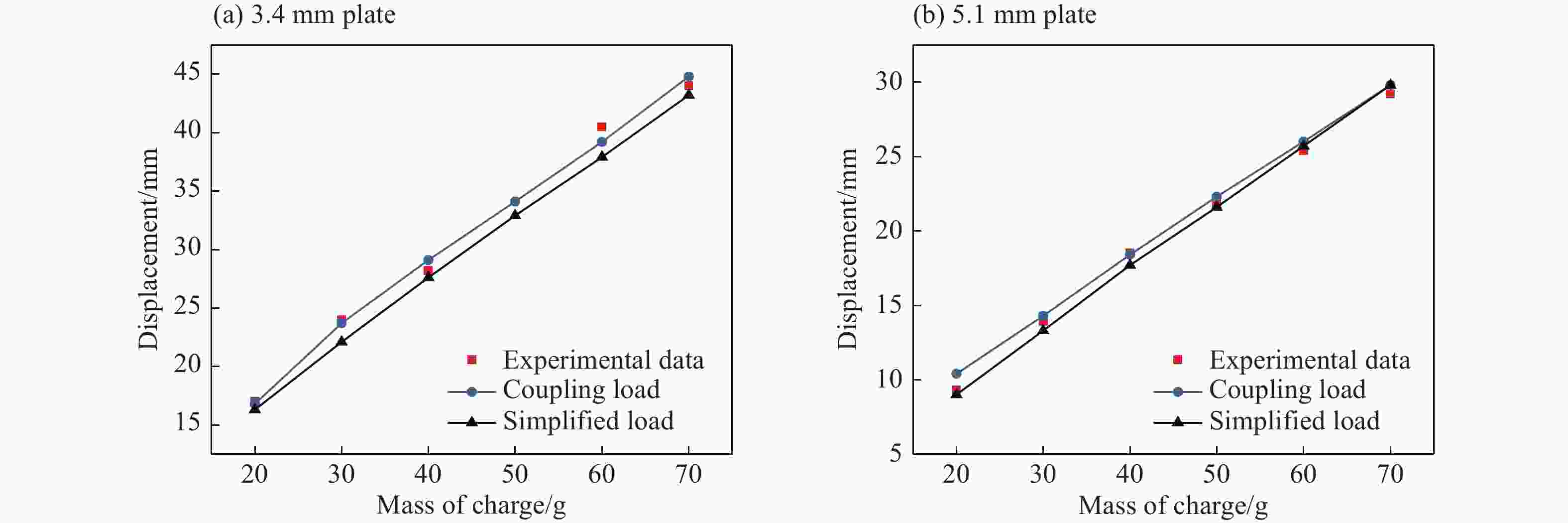
板厚/mm	药量/g	饱和冲量/（Pa·s）	等效压力/kPa	等效时间/μs
3.4	20	2 312.63	4 133.46	559.49
	30	3 198.58	5 808.93	550.63
	40	4 035.65	7 363.20	548.08
	50	4 856.17	8 910.56	544.99
	60	5 617.45	10 378.59	541.25
	70	6 369.51	11 877.10	536.28
4.0	20	2 358.52	4 190.00	562.89
	30	3 273.74	5 916.10	553.36
	40	4 136.70	7 534.59	549.03
	50	4 975.16	9 137.39	544.48
	60	5 762.89	10 656.95	540.76
	70	6 560.27	12 250.34	535.52
5.1	20	2 434.13	4 266.77	570.49
	30	3 401.43	6 059.57	561.33
	40	4 315.98	7 753.58	556.64
	50	5 204.07	9 435.92	551.52
	60	6 053.82	11 069.43	546.90
	70	6 916.35	12 757.55	542.14

下载: 导出CSV

参考文献(18)

[1]	EDRI I, SAVIR Z, FELDGUN V R, et al. On blast pressure analysis due to a partially confined explosion: I: experimental studies [J]. International Journal of Protective Structures, 2011, 2: 1–20. DOI: 10.1260/2041-4196.2.1.1.
[2]	HU Y, WU C, Lukaszewicz M, et al. Characteristics of confined blast loading in unvented structures [J]. International Journal of Protective Structures, 2011, 2(1): 21–44. DOI: 10.1260/2041-4196.2.1.21.
[3]	杨科之, 杨秀敏, 王年桥. 内爆荷载作用下结构等效静载计算方法 [J]. 解放军理工大学学报(自然科学版), 2002, 3(4): 31–33. YANG Kezhi, YANG Xiumin, WANG Nianqiao. Equivalent static load calculation method of structure subjected to internal explosion [J]. Journal of PLA University of Science and Technology(Natural Science Edition), 2002, 3(4): 31–33.
[4]	GERETTO C, YUEN S C K, NURICK G N. An experimental study of the effects of degrees of confinement on the response of square mild steel plates subjected to blast loading [J]. International Journal of Impact Engineering, 2015, 79: 32–44. DOI: 10.1016/j.ijimpeng.2014.08.002.
[5]	KUHL A L, REICHENBACH H. Combustion effects in confined explosions [J]. Proceedings of the Combustion Institute, 2009, 32(2): 2291–2298. DOI: 10.1016/j.proci.2008.05.001.
[6]	KUHL A L, BELL J B, BECKNER V E, et al. Spherical combustion clouds in explosions [J]. Shock Waves, 2013, 23(3): 233–249. DOI: 10.1007/s00193-012-0410-y.
[7]	ZHAO Y P, YU T X, FANG J. Large dynamic plastic deflection of a simply supported beam subjected to rectangular pressure pulse [J]. Archive of Applied Mechanics, 1994, 64(3): 223–232.
[8]	ZHAO Y P, YU T X, FANG J. Saturation impulses for dynamically loaded structures with finite-deflections [J]. Structural Engineering & Mechanics, 1995, 3(6): 583–592.
[9]	ZHU L, YU T X. Saturated impulse for pulse-loaded elastic-plastic square plates [J]. International Journal of Solids & Structures, 1997, 34(14): 1709–1718.
[10]	NURICK G N, MARTIN J B. Deformation of thin plates subjected to impulsive loading: a review Part II: experimental studies [J]. International Journal of Impact Engineering, 1989, 8(2): 171–186. DOI: 10.1016/0734-743X(89)90015-8.
[11]	NURICK G N, MARTIN J B. Deformation of thin plates subjected to impulsive loading: a review: Part I: theoretical considerations [J]. International Journal of Impact Engineering, 1989, 8(2): 159–170. DOI: 10.1016/0734-743X(89)90014-6.
[12]	Dragos J, WU C Q, Oehlers D J. Simplification of fully confined blasts for structural response analysis [J]. Engineering Structures, 2013, 56(11): 312–326.
[13]	PICKERD V, BORNSTEIN H, MCCARTHY P, et al. Analysis of the structural response and failure of containers subjected to internal blast loading [J]. International Journal of Impact Engineering, 2016, 95: 40–53. DOI: 10.1016/j.ijimpeng.2016.04.010.
[14]	GERETTO C. The effects of different degrees of confinement on the deformation of square plates subjected to blast loading [D]. Western Cape: University of Cape Town, 2012: 101−203.
[15]	JOHNSON G R, COOK W H. Fracture characteristics of three metals subjected to various strains, strain rates, temperatures and pressures [J]. Engineering Fracture Mechanics, 1985, 21(1): 31–48. DOI: 10.1016/0013-7944(85)90052-9.
[16]	ZHENG C, KONG X S, WU W G, et al. Experimental and numerical studies on the dynamic response of steel plates subjected to confined blast loading [J]. International Journal of Impact Engineering, 2018, 113: 144–160. DOI: 10.1016/j.ijimpeng.2017.11.013.
[17]	URTIEW P A, HAYES B. Parametric study of the dynamic JWL-EOS for detonation products [J]. Combustion, Explosion, and Shock Waves, 1991, 27(4): 505–514.
[18]	XU W Z, KONG X S, ZHENG C, et al. Numerical method for predicting the blast wave in partially confined chamber [J]. Mathematical Problems in Engineering, 2018, 2018(5): 1–17.