On velocity attenuation of a truncated cone-shaped projectile vertically penetrating through liquid
-
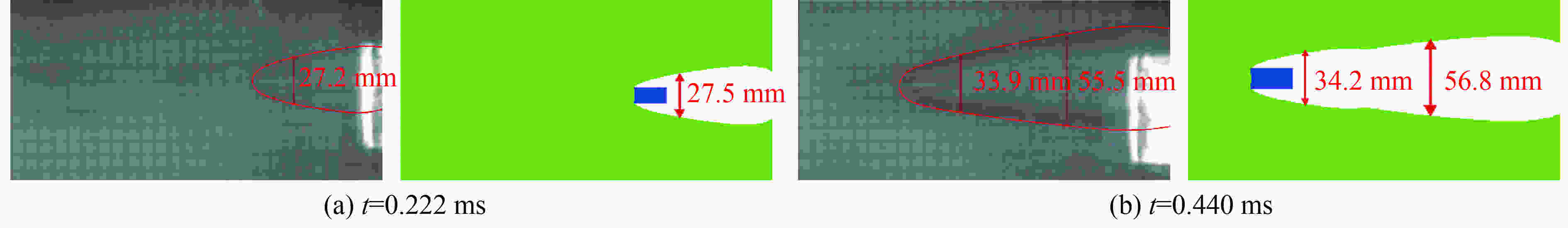
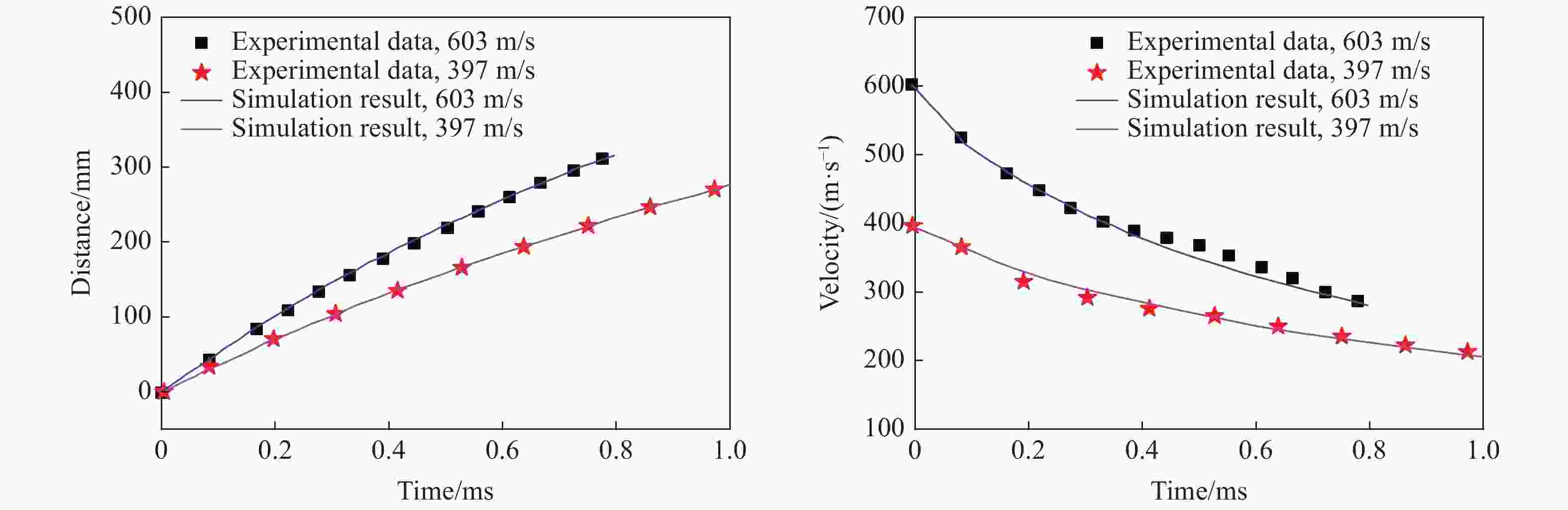
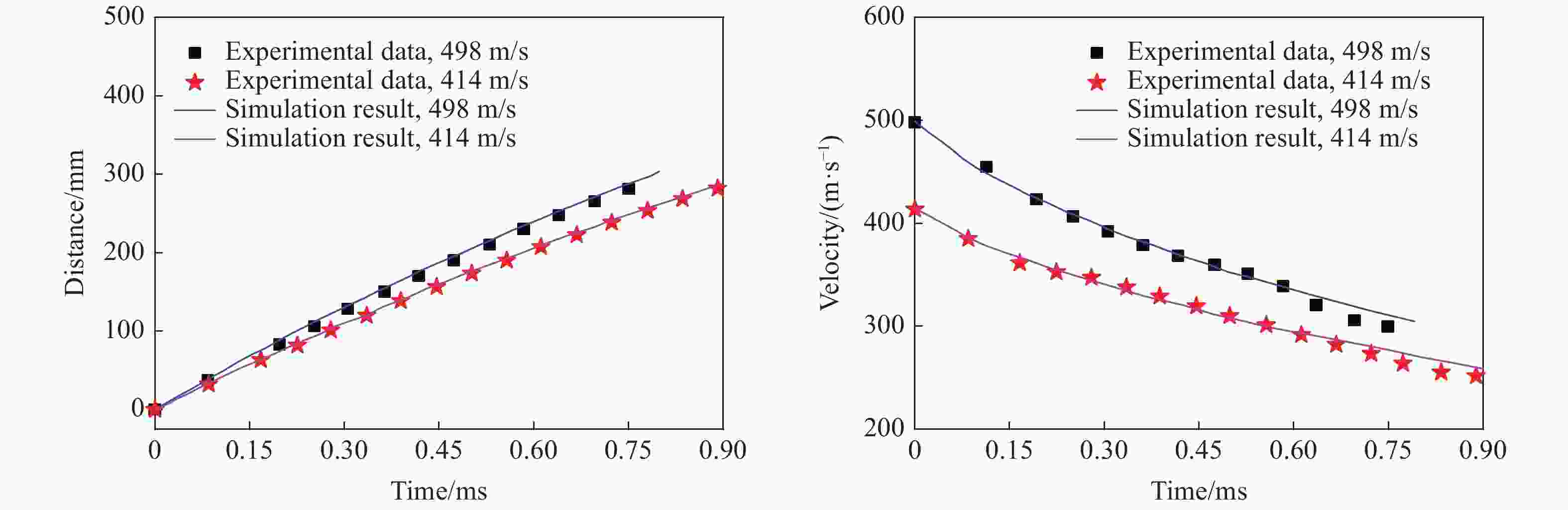
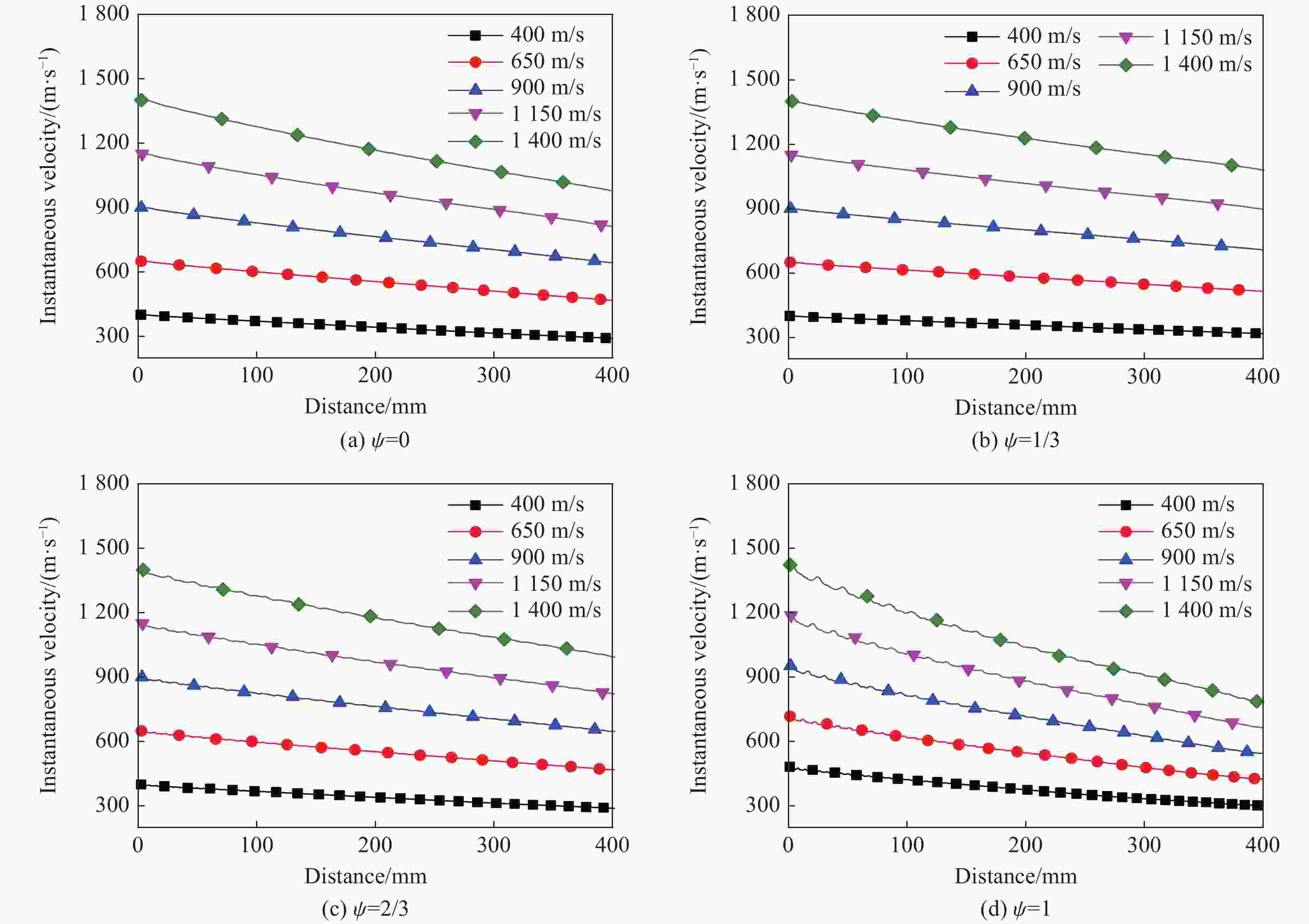
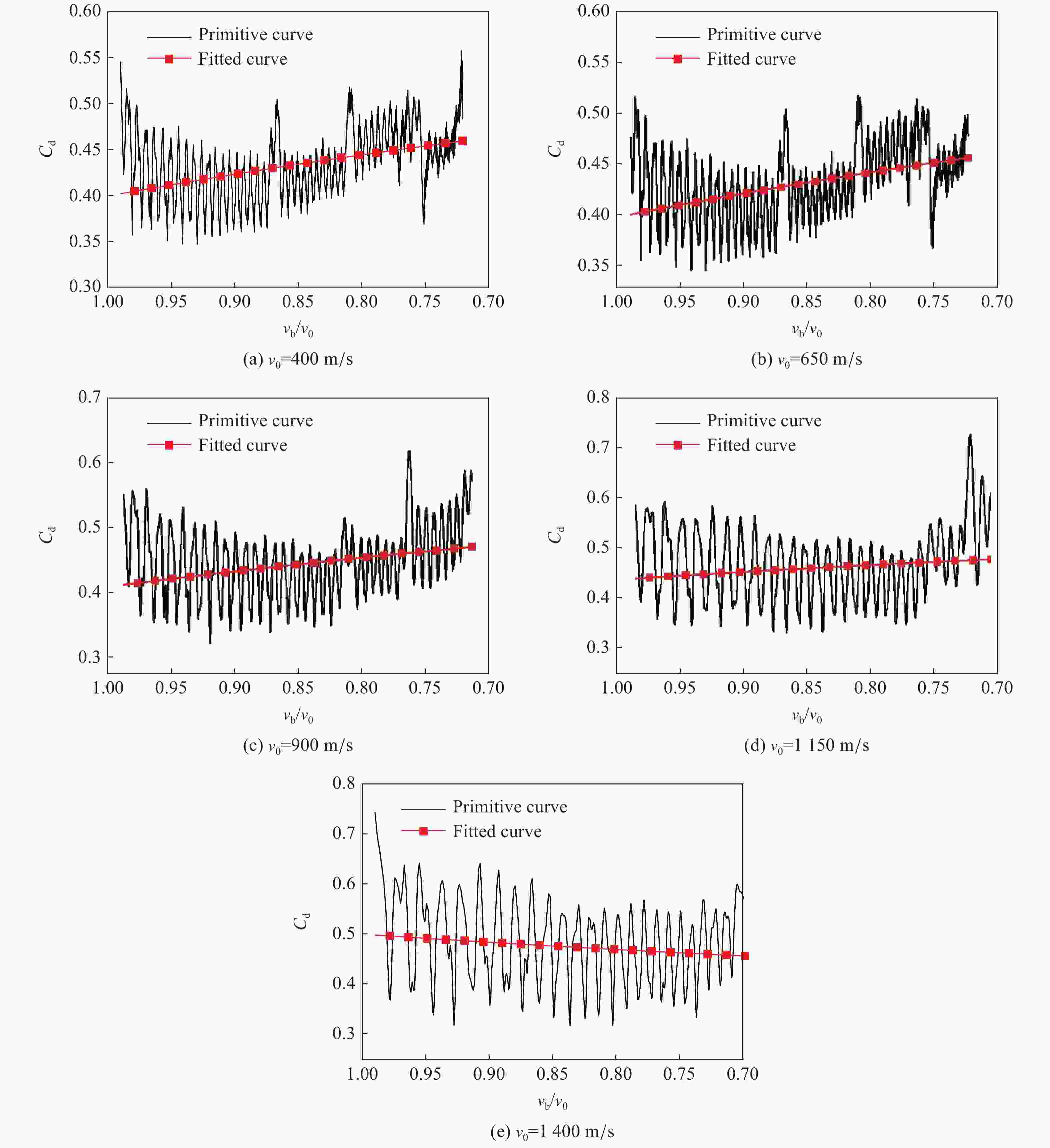
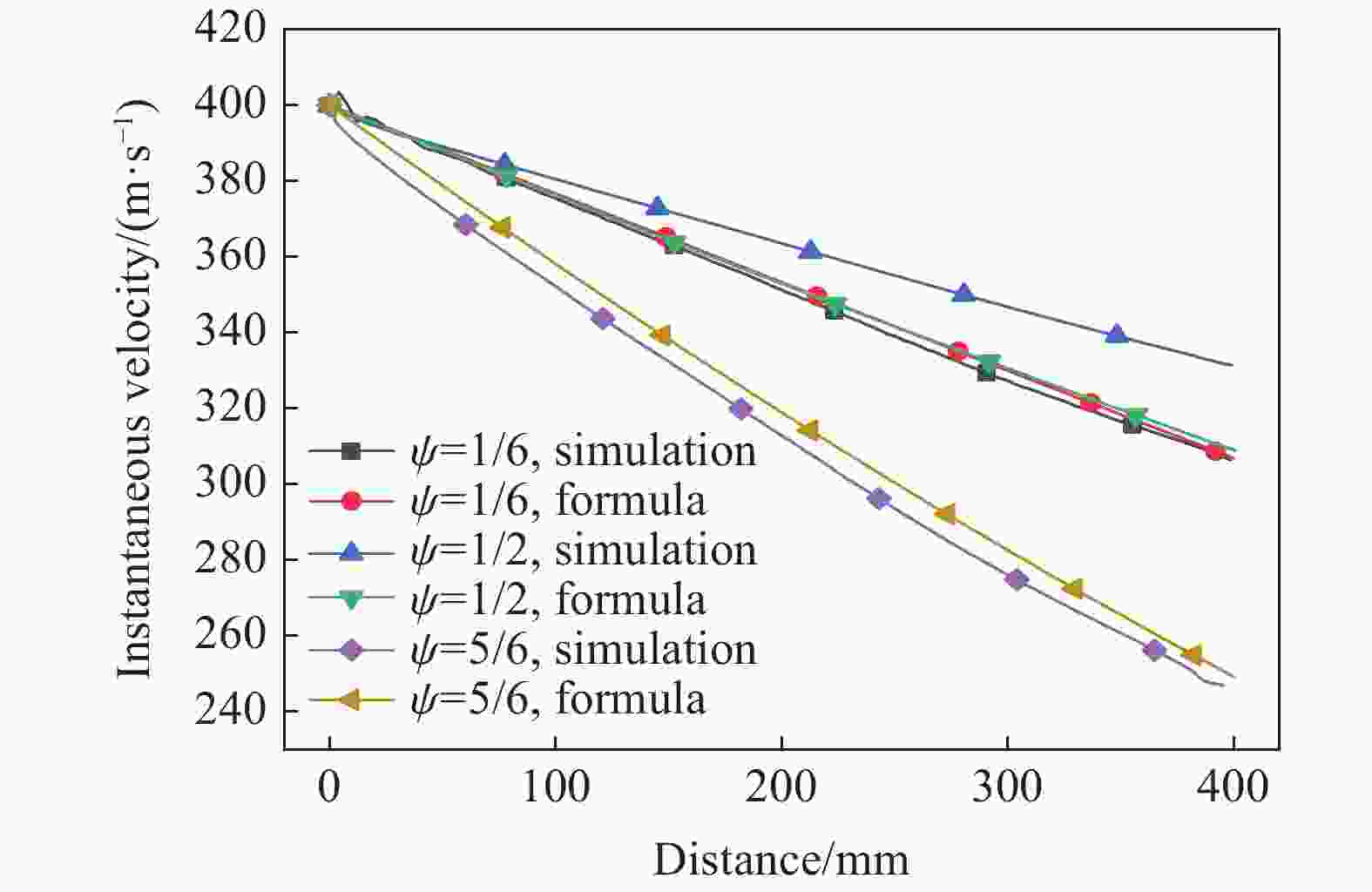
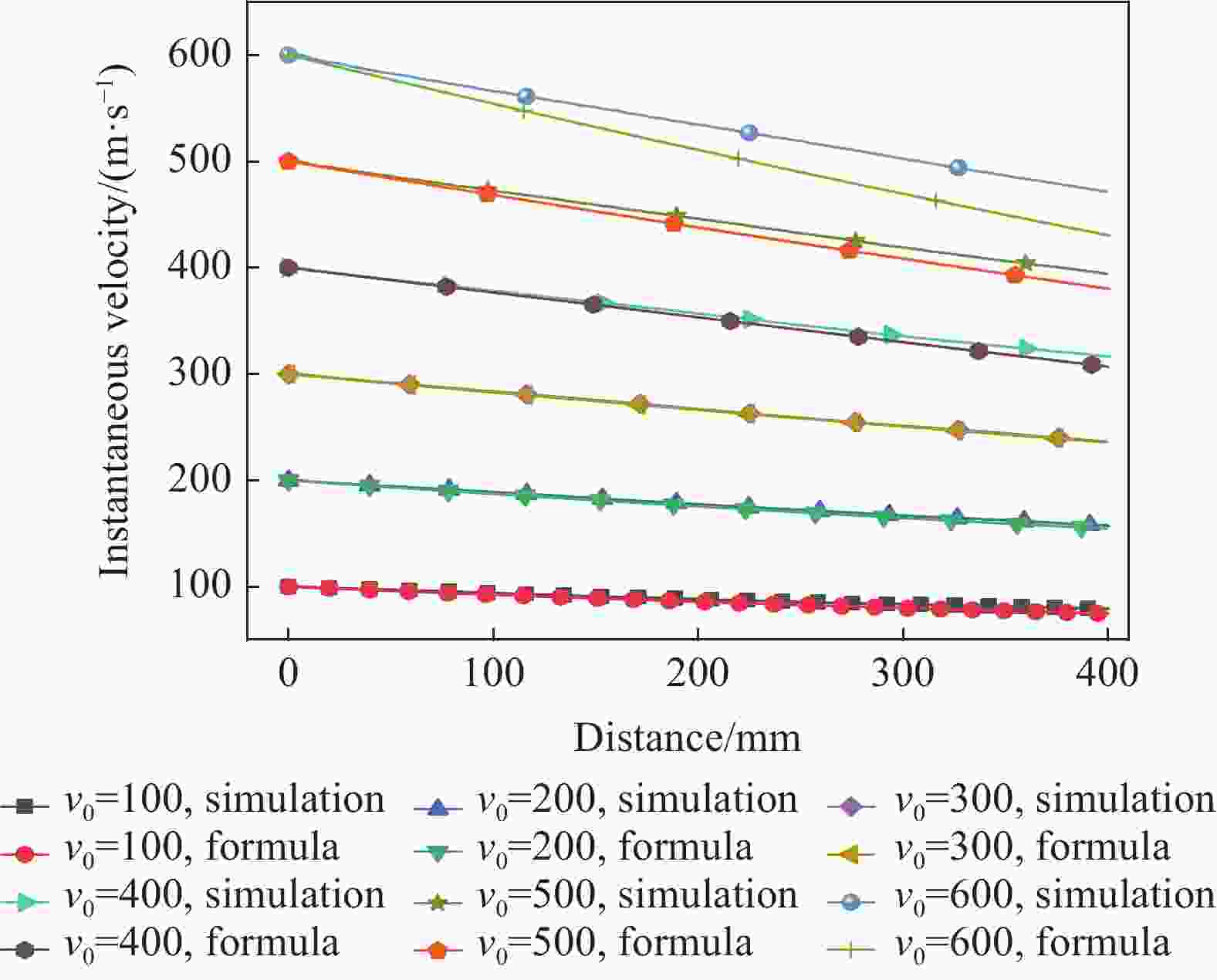
摘要: 水面舰船被动防护体系中液舱的主要功能之一是阻止高速弹体(爆炸破片)对内部重要结构、设备和人员的威胁,高速弹体打击液舱的过程包含着复杂的能量传递与耗散。为了分析弹体形状对其在液体介质中运动速度衰减的影响, 开展了一系列不同头形因数的截锥形弹体在不同入水速度下弹体垂直侵彻液体介质过程的数值模拟,得到了垂直侵彻液体介质时弹体速度衰减特性,发现高速弹体在液体介质中运动的阻力因数与弹体形状和无量纲速度有关。基于对系列数值模拟计算结果的拟合分析,提出了计及头形因数的截锥形弹体 垂直侵彻液体介质时的速度衰减经验公式,通过开展数值算例分析验证了公式计算结果的可靠性。本文中提出的经验公式可实现对高速弹体在液体介质中速度衰减的准确快速计算,为舰船防护液舱结构设计提供一定的参考。Abstract: One of the main functions of liquid tanks in the passive protective systems of surface warships is to prevent the damage by high-velocity projectiles (explosive fragments) to important internal structures, equipments and personnels. The process of high-velocity projectile penetrating through a liquid tank involves complex energy transfer and dissipation. To explore the influences of the head shape of a projectile and its water-entry velocity on the velocity attenuation of the projectile in fluid, a series of truncated cone-shaped projectiles with different head shape factors were developed to numerically simulate the processes of the truncated cone-shaped projectiles vertically penetrating through the fluid at different initial water-entry velocities. The velocity attenuation characteristics were obtained for the projectiles vertically penetrating through the fluid. The above results display that the resistance factor of a high-velocity projectile moving in the fluid is related with the projectile shape and the ratio of the instantaneous velocity of the projectile to its initial water-entry velocity. Based on the numerical simulations and the corresponding fitting results, an empirical formula was proposed by considering the projectile head factor to predict the velocity attenuation of the truncated cone-shaped projectiles vertically penetrating through the fluid. And a series of calculation examples were carried out to verify the formula. These calculation examples show that the formula is feasible and can be used to accurately calculate the velocity attenuation of high-velocity projectiles in fluid media and it is helpful for the structural design of the protective tanks of warships.
-
${A_1}{\rm{/GPa}}$ ${A_{\rm{2}}}{\rm{/GPa}}$ ${A_3}{\rm{/GPa}}$ ${B_0}$ ${B_1}$ ${T_1}{\rm{/GPa}}$ ${T_2}{\rm{/GPa}}$ $ {\rho }_{0}\rm{/(kg}·{\rm{m}}^{\rm{-3}}\rm{)}$ 2.2 9.54 14.57 0.28 0.28 2.2 0 1 000 表 2 数值计算工况
Table 2. Numerical calculation conditions
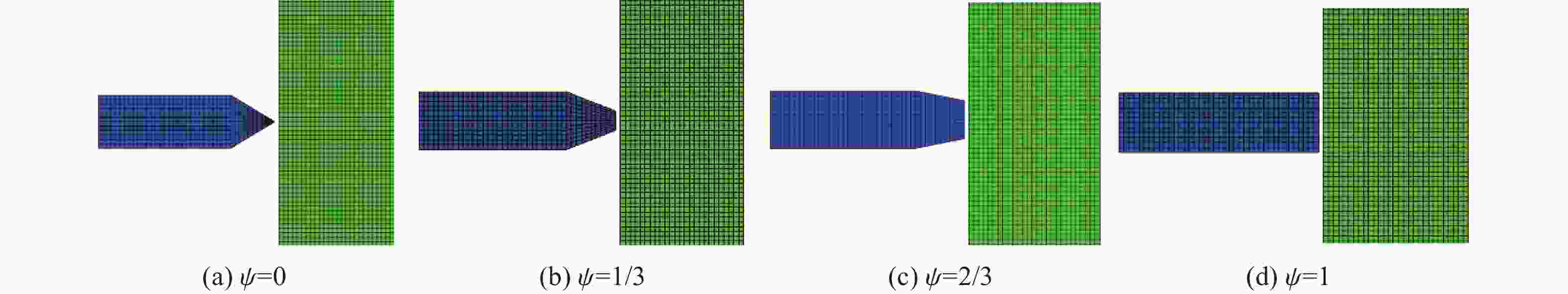
工况 $\psi $ v0/(m·s−1) 工况 $\psi $ v0/(m·s−1) 工况 $\psi $ v0/(m·s−1) 工况 $\psi $ v0/(m·s−1) 1-1 0 400 2-1 1/3 400 3-1 2/3 400 4-1 1 400 1-2 0 650 2-2 1/3 650 3-2 2/3 650 4-2 1 650 1-3 0 900 2-3 1/3 900 3-3 2/3 900 4-3 1 900 1-4 0 1 150 2-4 1/3 1 150 3-4 2/3 1 150 4-4 1 1 150 1-5 0 1 400 2-5 1/3 1 400 3-5 2/3 1 400 4-5 1 1 400 表 3 不同工况下的参数
${a_0}$ 和${a_1}$ 的数值Table 3. Values of parameters
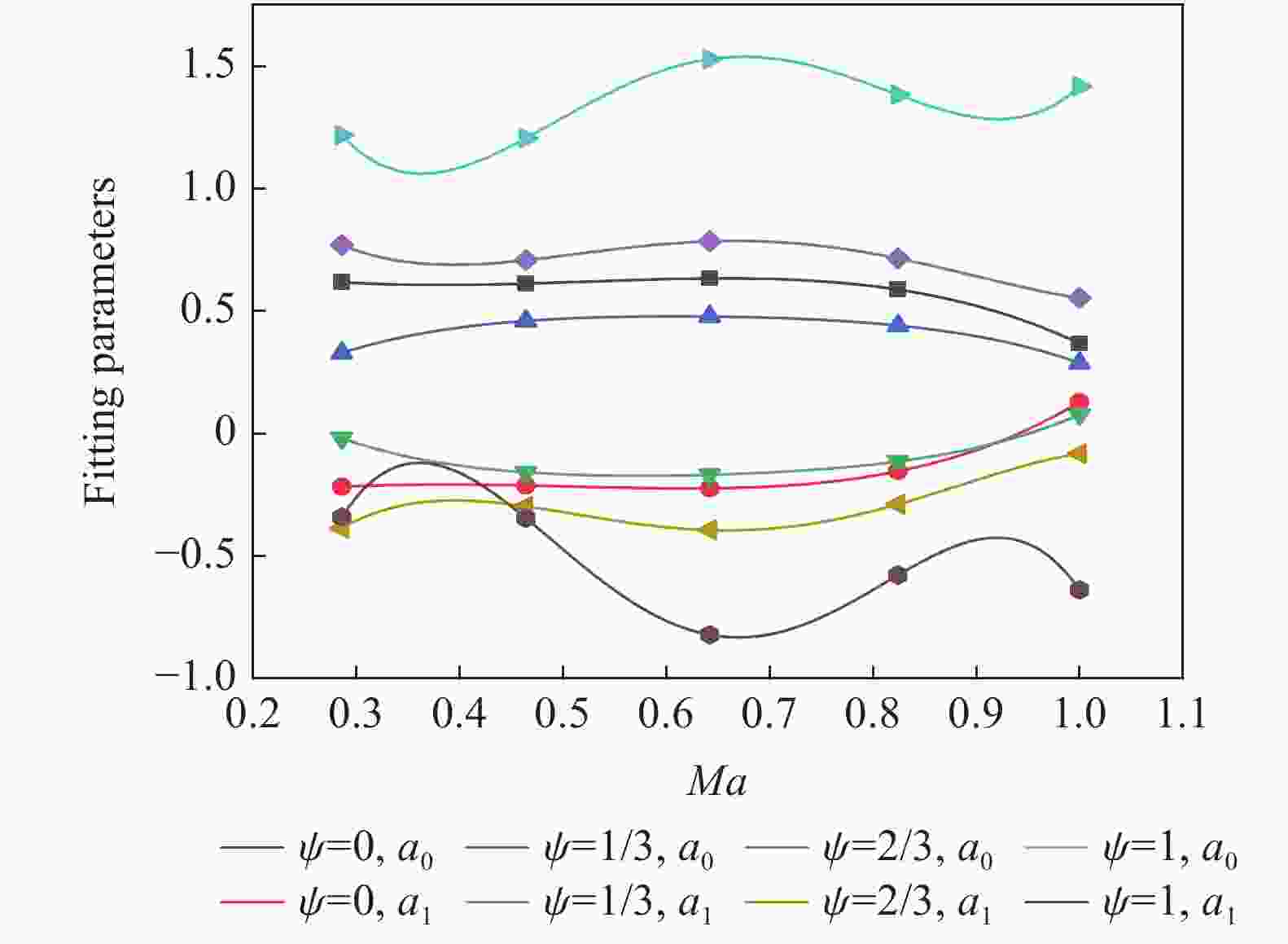
${a_0}$ and${a_1}$ under different working conditions$\psi $ 拟合参数 v0/(m·s−1) 400 650 900 1150 1400 0 a0 0.617 0.612 0.633 0.587 0.367 a1 −0.217 −0.212 −0.223 −0.153 0.128 1/3 a0 0.329 0.459 0.477 0.440 0.288 a1 −0.019 −0.158 −0.168 −0.113 0.077 2/3 a0 0.768 0.707 0.784 0.714 0.553 a1 −0.387 −0.297 −0.394 −0.289 −0.082 1 a0 1.216 1.204 1.527 1.382 1.416 a1 −0.344 −0.345 −0.823 −0.580 −0.640 表 4 参数
${a_0}$ 、${a_1}$ 的拟合结果Table 4. Fitting results of parameters
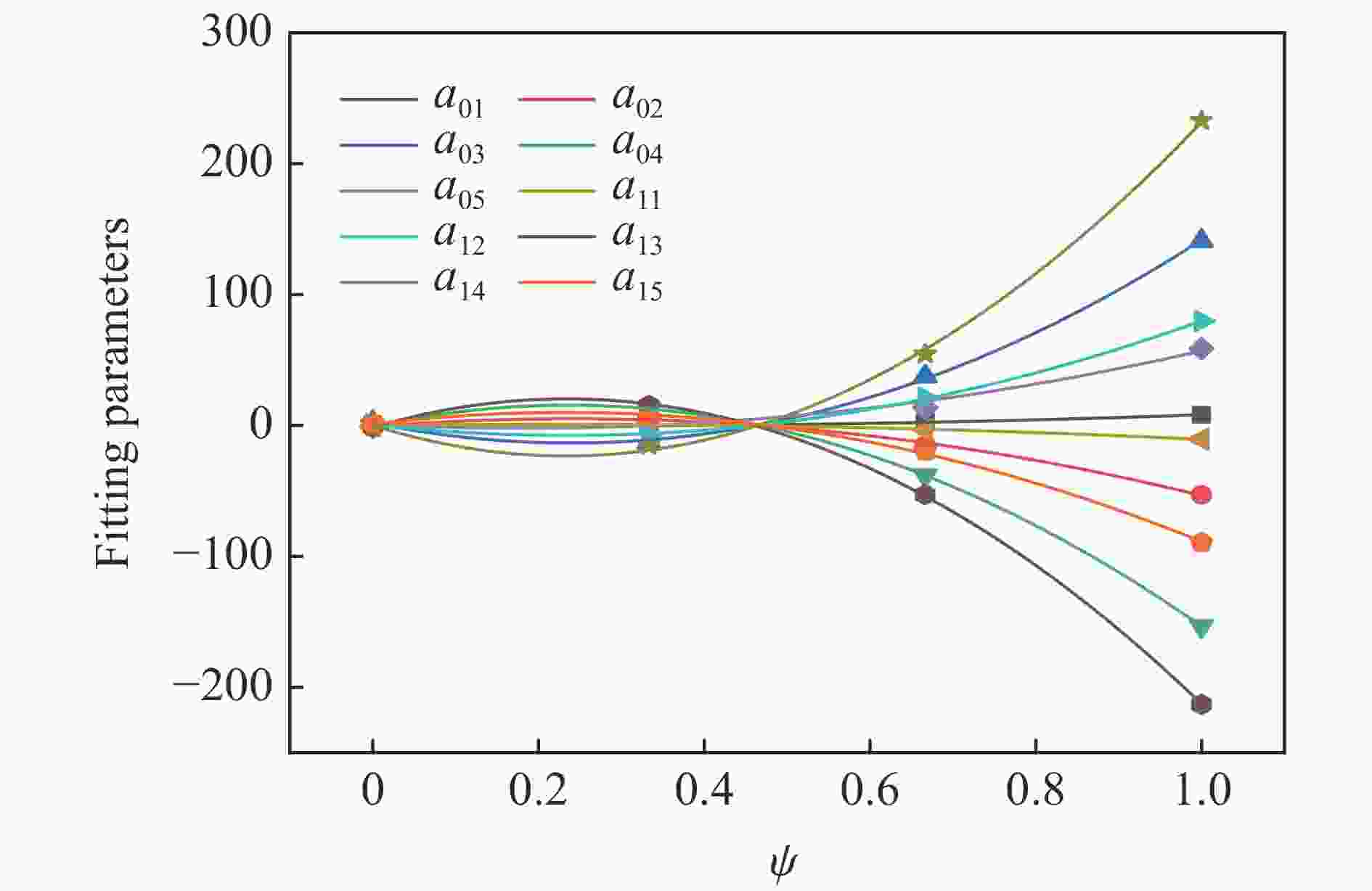
${a_0}$ and${a_1}$ $\psi $ a01 a02 a03 a04 a05 a11 a12 a13 a14 a15 0 0.847 −1.459 2.575 −0.707 −0.887 −0.376 0.918 −1.096 −1.059 1.743 1/3 −0.611 6.078 −13.025 12.901 5.055 1.060 −7.042 15.285 −15.196 5.971 2/3 2.810 −15.067 37.573 −38.342 13.579 −3.246 21.137 −52.932 54.463 −19.504 1 8.033 −53.017 141.081 −153.418 58.737 −10.547 79.685 −213.159 232.929 −89.548 表 5
${a_{ij}}$ 系列参数的拟合结果Table 5. Fitting results of
${a_{ij}}$ series parameters${a_{ij}}$ ${a_{ij}}_0$ ${a_{ij}}_1$ ${a_{ij}}_2$ ${a_0}_1$ 0.693 −7.536 15.031 ${a_{02}}$ −0.864 49.585 −102.332 ${a_{03}}$ 1.908 −128.117 267.957 ${a_{04}}$ −0.654 136.681 −289.498 ${a_{05}}$ 0.814 −32.000 88.221 ${a_1}_1$ −0.239 9.212 −19.657 ${a_1}_2$ 0.628 −70.273 149.620 ${a_1}_3$ −1.463 185.983 −397.312 ${a_1}_4$ 0.187 −201.803 433.298 ${a_1}_5$ 1.001 77.280 −167.088 -
[1] 孔祥韶. 爆炸载荷及复合多层防护结构响应特性研究[D]. 武汉: 武汉理工大学, 2013: 1-26.DOI: 10.7666/d.Y2364126. [2] LEE M, LONGORIA R G, WILSON D E. Cavity dynamics in high-speed water entry [J]. Physics of Fluids, 1997, 9(3): 540–550. DOI: 10.1063/1.869472. [3] 李营, 张磊, 朱海清, 等. 爆炸破片在液舱中的速度衰减特性研究 [J]. 中国造船, 2016, 57(1): 127–137. DOI: 10.3969/j.issn.1000-4882.2016.01.014.LI Y, ZHANG L, ZHU H Q, et al. Velocity attenuation of blast fragments in water tank [J]. Shipbuilding of China, 2016, 57(1): 127–137. DOI: 10.3969/j.issn.1000-4882.2016.01.014. [4] 沈晓乐, 朱锡, 侯海量, 等. 高速破片侵彻防护液舱试验研究 [J]. 中国舰船研究, 2011, 6(3): 12–15. DOI: 10.3969/j.issn.1673-3185.2011.03.003.SHEN X L, ZHU X, HOU H L, et al. Experimental study on penetration properties of high velocity fragment into safety liquid cabin [J]. Chinese Journal of Ship Research, 2011, 6(3): 12–15. DOI: 10.3969/j.issn.1673-3185.2011.03.003. [5] 郭子涛. 弹体入水特性及不同介质中金属靶的抗侵彻性能研究[D]. 哈尔滨: 哈尔滨工业大学, 2012: 21-36.DOI: 10.7666/d.D241209. [6] ZHAO B L, ZHAO J G, CUI C Y, et al. Growth model of cavity generated by the projectile impacting liquid-filled tank [J]. Defence Technology, 2020, 16(3): 609–616. DOI: 10.1016/j.dt.2019.09.013. [7] ZHANG Y, LI X B, LI S Y. Research on the velocity attenuation characteristics of the fragments during high-speed water entry [C] // Proceedings of the 37th International Conference on Ocean, Offshore and Arctic Engineering. Madrid: ASME, 2018.DOI: 10.1115/OMAE2018-78665. [8] VARAS D, ZAERA R, LÓPEZ-PUENTE J. Numerical modelling of partially filled aircraft fuel tanks submitted to Hydrodynamic Ram [J]. Aerospace Science and Technology, 2012, 16(1): 19–28. DOI: 10.1016/j.ast.2011.02.003. [9] BIRKHOFF G, CAYWOOD T E. Fluid flow patterns [J]. Journal of Applied Physics, 1949, 20(7): 646–659. DOI: 10.1063/1.1698450. [10] 孔祥韶, 吴卫国, 刘芳, 等. 舰船舷侧防护液舱对爆炸破片的防御作用研究 [J]. 船舶力学, 2014, 18(8): 996–1004. DOI: 10.3969/j.issn.1007-7294.2014.08.015.KONG X S, WU W G, LIU F, et al. Research on protective effect of guarding fluid cabin under attacking by explosion fragments [J]. Journal of Ship Mechanics, 2014, 18(8): 996–1004. DOI: 10.3969/j.issn.1007-7294.2014.08.015. [11] LECYSYN N, BONY-DANDRIEUX A, APRIN L, et al. Experimental study of hydraulic ram effects on a liquid storage tank: analysis of overpressure and cavitation induced by a high-speed projectile [J]. Journal of Hazardous Materials, 2010, 178(1/2/3): 635–643. DOI: 10.1016/j.jhazmat.2010.01.132. [12] JOHNSON G R, COOK W H. A constitutive model and data for metals subjected to large strains, high strain rates and high temperatures [C] // Proceedings of the 7th International Symposium on Ballistics. Netherlands: The Hague, 1983. [13] 陈刚, 陈忠富, 陶俊林, 等. 45钢动态塑性本构参量与验证 [J]. 爆炸与冲击, 2005, 25(5): 451–456. DOI: 10.11883/1001-1455(2005)05-0451-06.CHEN G, CHEN Z F, TAO J L, et al. Investigation and validation on plastic constitutive parameters of 45 steel [J]. Explosion and Shock Waves, 2005, 25(5): 451–456. DOI: 10.11883/1001-1455(2005)05-0451-06. [14] 李晓杰, 张程娇, 王小红, 等. 水的状态方程对水下爆炸影响的研究 [J]. 工程力学, 2014, 31(8): 46–52. DOI: 10.6052/j.issn.1000-4750.2013.03.0180.LI X J, ZHANG C J, WANG X H, et al. Numerical study on the effect of equations of state of water on underwater explosions [J]. Engineering Mechanics, 2014, 31(8): 46–52. DOI: 10.6052/j.issn.1000-4750.2013.03.0180. -







 下载:
下载: