Relationship between the blood-vessel coupling characteristics and the propagation of pulse waves
-
摘要: 脉搏波既不可简单地理解为可压缩血液流体中的压力纵波，也不可简单地理解为沿固体血管传播的涨缩位移横波，而是超乎普通想象的流-固耦合和纵波-横波耦合的复杂波。从分析耦合本构关系的新途径出发，本文中提出了一个流-固耦合/纵波-横波耦合的串联模型，可为解读“位数形势”中医脉诊提供更丰富的信息。结果表明，脉搏波耦合系统的等效体积压缩模量Ks以及相应的耦合系统脉搏波传播速度cs主要依赖于两个无量纲参数：血液-血管模量比Kb(p)/E(p)和薄壁血管径厚比D(p)/h0，它们因人而异、因人的不同脉搏位置而异。文中定量分析了它们对cs的影响，显示人体的Kb/E值在103数量级，从而cs值在100～101 m/s数量级，以适应人体生理生化反应。由临床有创测量，证实脉搏体积横波与脉搏压力纵波是相耦合地以相同速度传播；还显示脉搏波是在其波阵面上具有氧合生化反应的“生物波”。此外，还讨论了“脉压放大”现象与非线性本构关系和与血管分叉处加载增强反射之间的关系，并讨论了Lewis关于重搏波形成的假设。Abstract: Pulse waves cannot be understood simply as pressure waves (longitudinal waves) propagating in compressible blood fluid, nor as radially expanding-contracting displacement waves (transverse waves) propagating along solid blood vessels, but rather as complex waves with fluid-solid coupling and longitudinal wave-transverse wave coupling beyond ordinary imagination. Starting from a new approach to analyze the coupling constitutive relation, a series model is proposed, providing more information for traditional Chinese medicine (TCM) pulse diagnosis in terms of the “position, rate, shape and potential”. It is shown that the equivalent volumetric compression modulus Ks and the corresponding pulse wave propagation velocity cs of the coupling pulse wave system, mainly depend on two dimensionless parameters: the ratio of the blood modulus to the vessel modulus, Kb(p)/E(p) and the ratio of the diameter to the thickness, D(p)/h0, of thin-walled blood vessels, which may vary from person to person and from different pulse locations for the same person. The influences of them on the cs are quantitatively analyzed, showing that for human body the magnitude of Kb/E is in the order of 103 so that the magnitude of cs is in the order of 100–101 m/s to adapt to the human physio-biochemical reactions. By clinical invasive measurements, it is confirmed that the pulse volume transverse wave and the pulse pressure longitudinal wave are coupled and propagate at the same speed, and it is shown that the pulse wave is actually a “biological wave” with oxygenation and biochemical reactions on the wave front. Furthermore, the relations of the “pulse pressure amplification” with the nonlinear constitutive relation and with the load enhanced reflection at the bifurcation of blood vessels, as well as the Lewis’s hypothesis about the formation of dicrotic wave are discussed.
-
图 5
${D / {{h_0} = 10}}$ 时，耦合系数$\alpha $ 随${{{K_{\rm{b}}}} / E}$ 的变化Figure 5. Relation between coupling coefficient
$\alpha $ and${{{K_{\rm{b}}}} / E}$ for${D/ {{h_0} = 10}}$ 
图 6
${{{K_{\rm{b}}}} / E} = {\rm{1}} \times {\rm{1}}{0^{\rm{3}}}$ 时，耦合系数$\alpha $ 随${D / {{h_0}}}$ 的变化Figure 6. Relation between coupling coefficient
$\alpha $ and${D / {{h_0}}}$ for${{{K_{\rm{b}}}} / E} = {\rm{1}} \times {\rm{1}}{0^{\rm{3}}}$ -
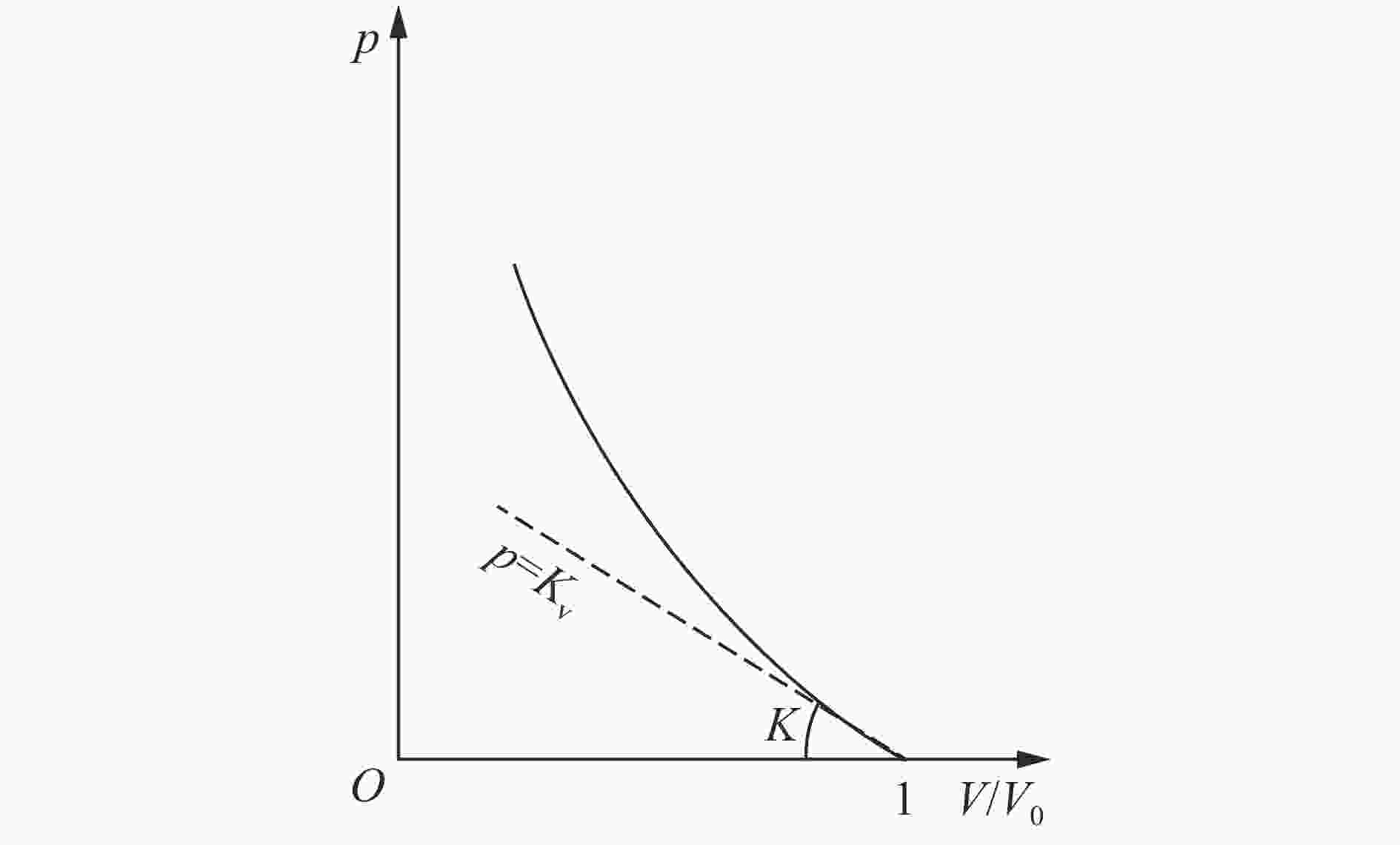
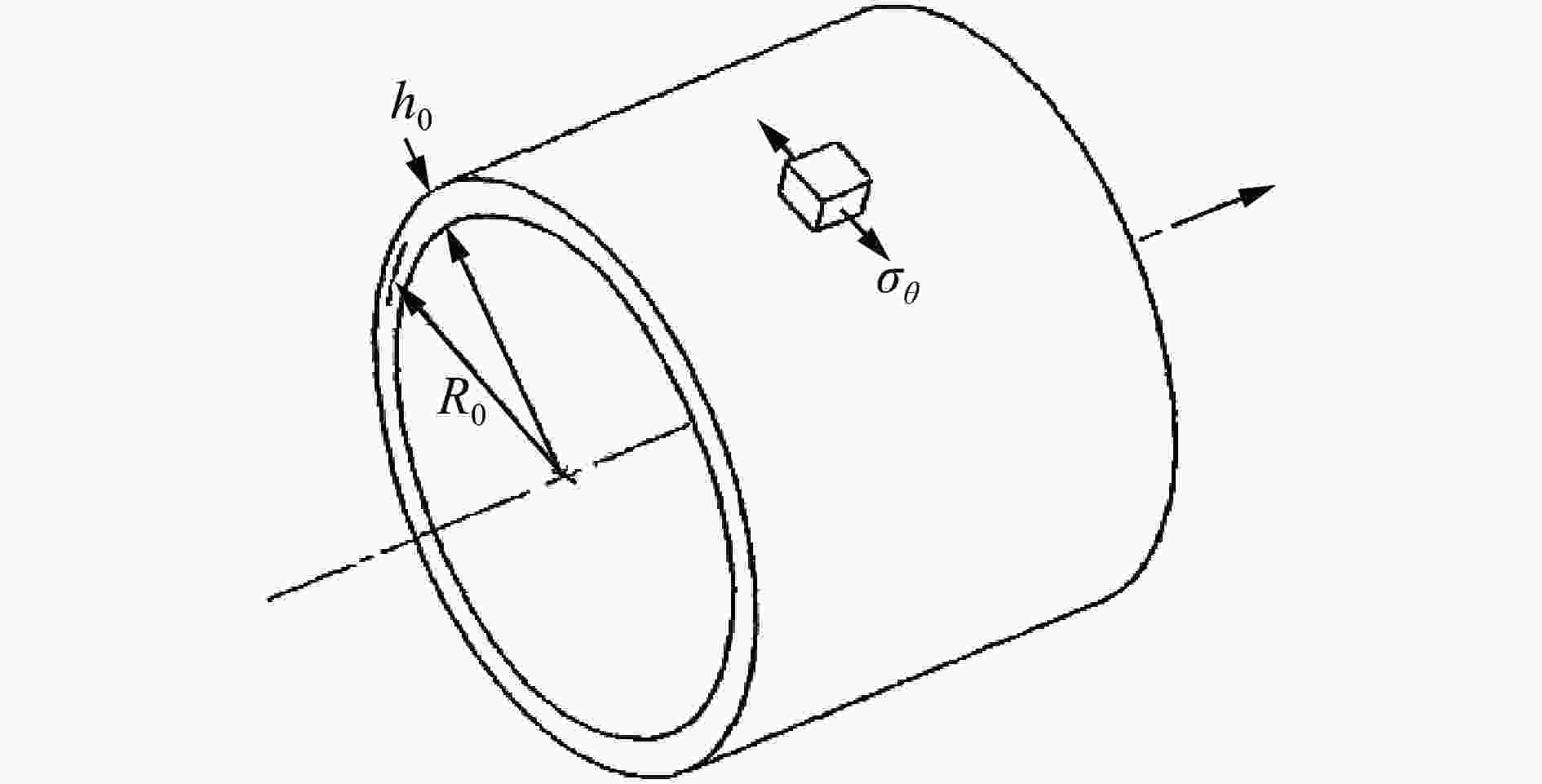
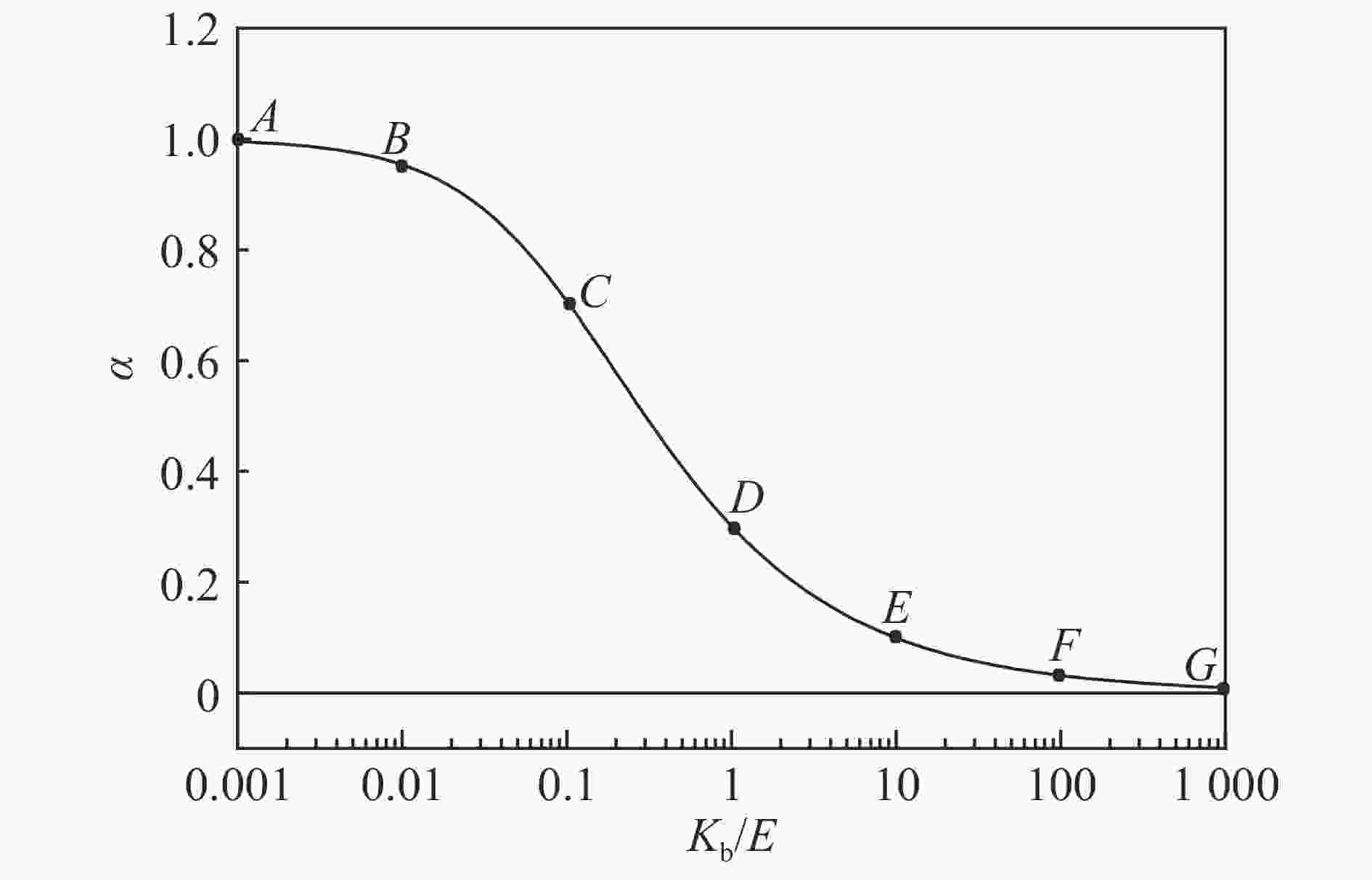
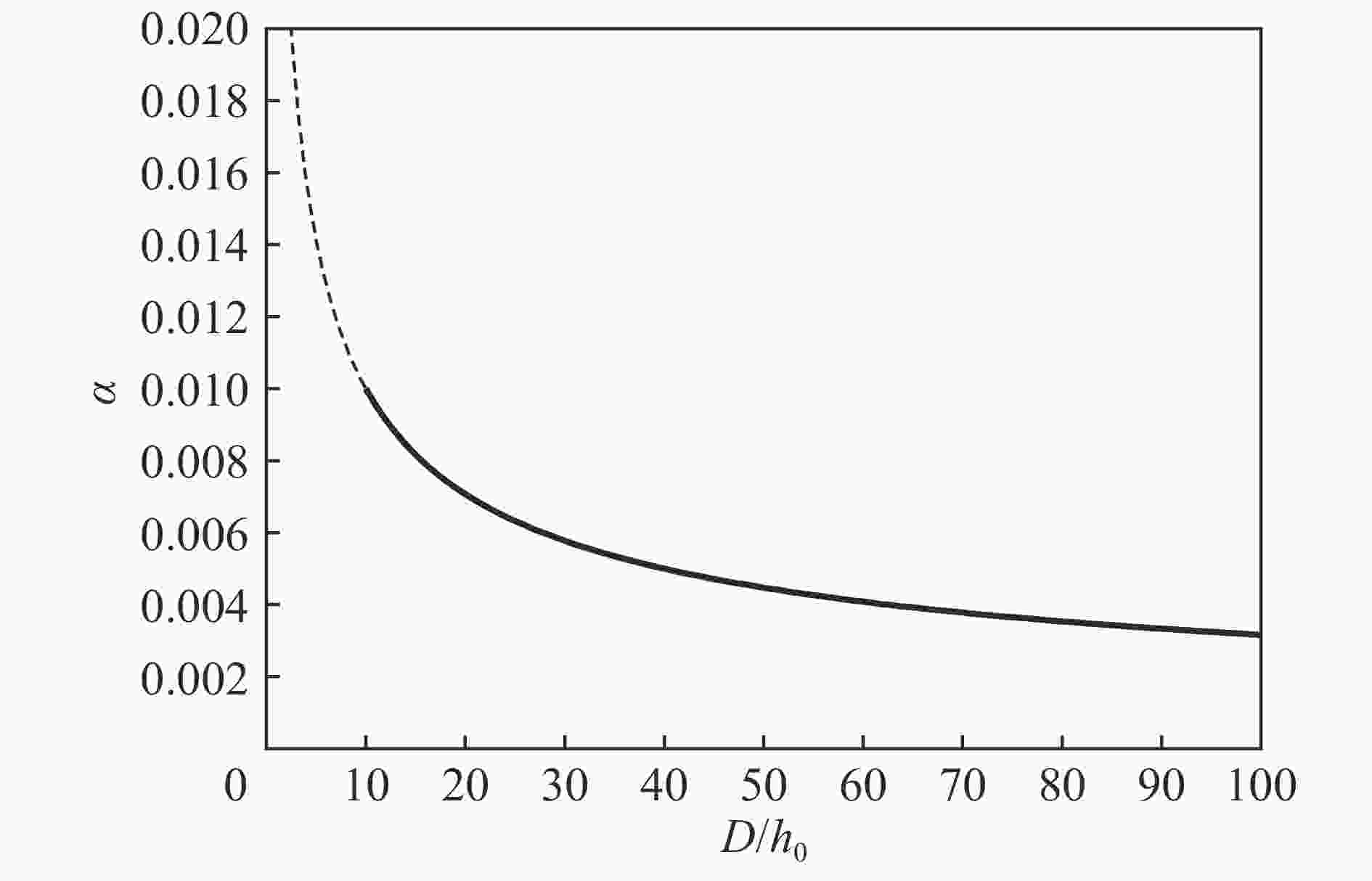
WANG L L, WANG H. Mechanics modeling and inverse analyses of pulse wave system from the view-point of traditional Chinese medicine [C]//Proceedings of the ASME 2016, 35th International Conference on Ocean, Offshore and Arctic Engineering. Busan, South Korea: ASME, 2016. DOI: 10.1115/OMAE2016-55106. 王礼立, 王晖. 脉搏波系统的力学模型及反演兼对若干中医学问题的讨论 [J]. 力学学报, 2016, 48(6): 1416–1424. DOI: 10.6052/0459-1879-15-322.WANG L L, WANG H. Mechanics modeling and inverse analyses of pulse waves system with discussions on some concepts in the traditional Chinese medicine [J]. Chinese Journal of Theoretical and Applied Mechanics, 2016, 48(6): 1416–1424. DOI: 10.6052/0459-1879-15-322. 王琦. 中医体质学 [M]. 北京: 人民卫生出版社, 2009.WANG Q. Constitutionology of Chinese medicine [M]. Beijing: People’s Medical Publishing House, 2009. 王晖. 体质的中医保健 [M]. 宁波: 宁波出版社, 2009.WANG H. Traditional Chinese medicine health care of body constitutions [M]. Ningbo: Ningbo Press, 2009. 王礼立. 应力波基础 [M]. 2版. 北京: 国防工业出版社, 2005.WANG L L. Foundation of stress waves [M]. 2nd ed. Beijing: National Defense Industry Press, 2005. HU C S, CHUNG Y F, YEH C C, et al. Temporal and spatial properties of arterial pulsation measurement using pressure sensor array [J]. Evidence-Based Complementary and Alternative Medicine, 2012, 2012: 745127. DOI: 10.1155/2012/745127. XUE Y, SU Y, ZHANG C, et al. Full-field wrist pulse signal acquisition and analysis by 3D Digital Image Correlation [J]. Optics and Lasers in Engineering, 2017, 98: 76–82. DOI: 10.1016/j.optlaseng.2017.05.018. MANCIA G, DE BACKER G, DOMINICZAK A, et al. 2007 Guidelines for the management of arterial hypertension: the task force for the management of arterial hypertension of the European Society of Hypertension (ESH) and of the European Society of Cardiology (ESC) [J]. Journal of Hypertension, 2007, 25(6): 1105–1187. DOI: 10.1097/HJH.0b013e3281fc975a. 中国高血压防治指南修订委员会, 高血压联盟(中国), 中华医学会心血管病学分会, 等. 中国高血压防治指南(2018年修订版) [J]. 中国心血管杂志, 2019, 24(1): 24–56. DOI: 10.3969/j.issn.1007-5410.2019.01.002.China Hypertension Prevention and Control Guidelines Revision Committee, Hypertension Alliance (China), Chinese Society of Cardiology, et al. 2018 Chinese guidelines for the management of hypertension [J]. Chinese Journal of Cardiovascular Medicine, 2019, 24(1): 24–56. DOI: 10.3969/j.issn.1007-5410.2019.01.002. MOENS A J. Die pulskurve [M]. Leiden: Brill, 1878. KORTEWEG D J. Ueber die Fortpflanzungsgeschwindigkeit des schalles in elastischen Röhren [J]. Annalen der Physik, 1878, 241(12): 525–542. DOI: 10.1002/andp.18782411206. HUGHES D J, BABBS C F, GEDDES L A, et al. Measurements of Young’s modulus of elasticity of the canine aorta with ultrasound [J]. Ultrasonic Imaging, 1979, 1(4): 356–367. DOI: 10.1177/016173467900100406. FUNG Y C. Biomechanics: circulation [M]. New York: Springer, 1997. MA Y J, CHOI J, HOURLIER-FARGETTE A, et al. Relation between blood pressure and pulse wave velocity for human arteries [J]. Proceedings of the National Academy of Sciences of the United States of America, 2018, 115(44): 11144–11149. DOI: 10.1073/pnas.1814392115. TIMOSHENKO S P, GOODIER J N. Theory of elasticity [M]. 3rd ed. London: McGraw-Hill Book Company, 1970. HUGHES D J, FEARNOT N E, BABBS C F, et al. Continuous measurement of aortic radius change in vivo with an intra-aortic ultrasonic catheter [J]. Medical and Biological Engineering and Computing, 1985, 23(3): 197–202. DOI: 10.1007/BF02446857. URICK R J. A sound velocity method for determining the compressibility of finely divided substances [J]. Journal of Applied Physics, 1947, 18(11): 983–987. DOI: 10.1063/1.1697584. WANG S H, LEE L P, LEE J S. A linear relation between the compressibility and density of blood [J]. The Journal of the Acoustical Society of America, 2001, 109(1): 390–396. DOI: 10.1121/1.1333419. LAURENT S, GIRERD X, MOURAD J J, et al. Elastic modulus of the radial artery wall material is not increased in patients with essential hypertension [J]. Arteriosclerosis and Thrombosis: A Journal of Vascular Biology, 1994, 14(7): 1223–1231. DOI: 10.1161/01.ATV.14.7.1223. 王礼立, 王晖, 杨黎明, 等. 论脉搏波客观化和定量化研究的症结所在 [J]. 中华中医药杂志, 2017, 32(11): 4855–4863.WANG L L, WANG H, YANG L M, et al. Crux of objectification and quantification of pulse waves [J]. China Journal of Traditional Chinese Medicine and Pharmacy, 2017, 32(11): 4855–4863. WEBSTER J G. Design of pulse Oximeters [M]. Boca Raton: CRC Press, 1997. LEWIS T. The factors influencing the prominence of the dicrotic wave [J]. The Journal of Physiology, 1906, 34(6): 414–429. DOI: 10.1113/jphysiol.1906.sp001165. -
下载:
图(7)
计量
- 文章访问数: 6762
- HTML全文浏览量: 2723
- PDF下载量: 137
- 被引次数: 0