Dynamic response and parameter analysis of concrete-filled steel tubular structure under lateral impact loading
-
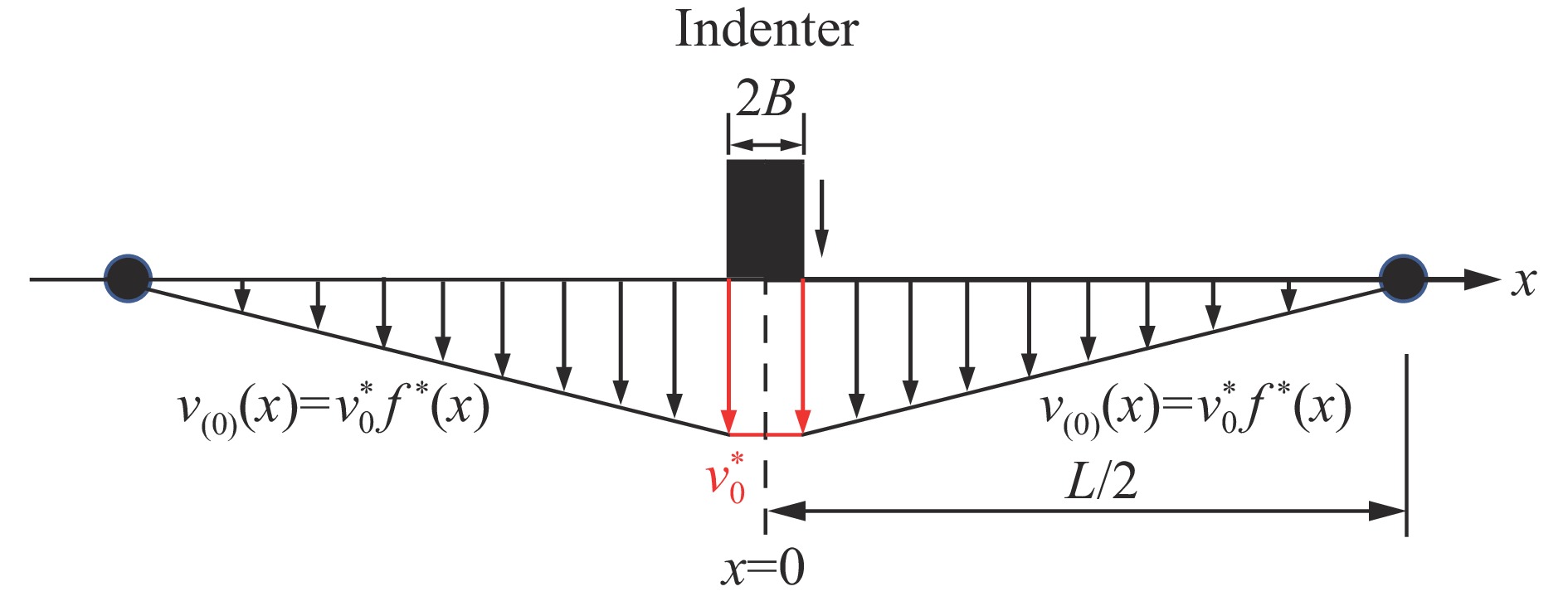
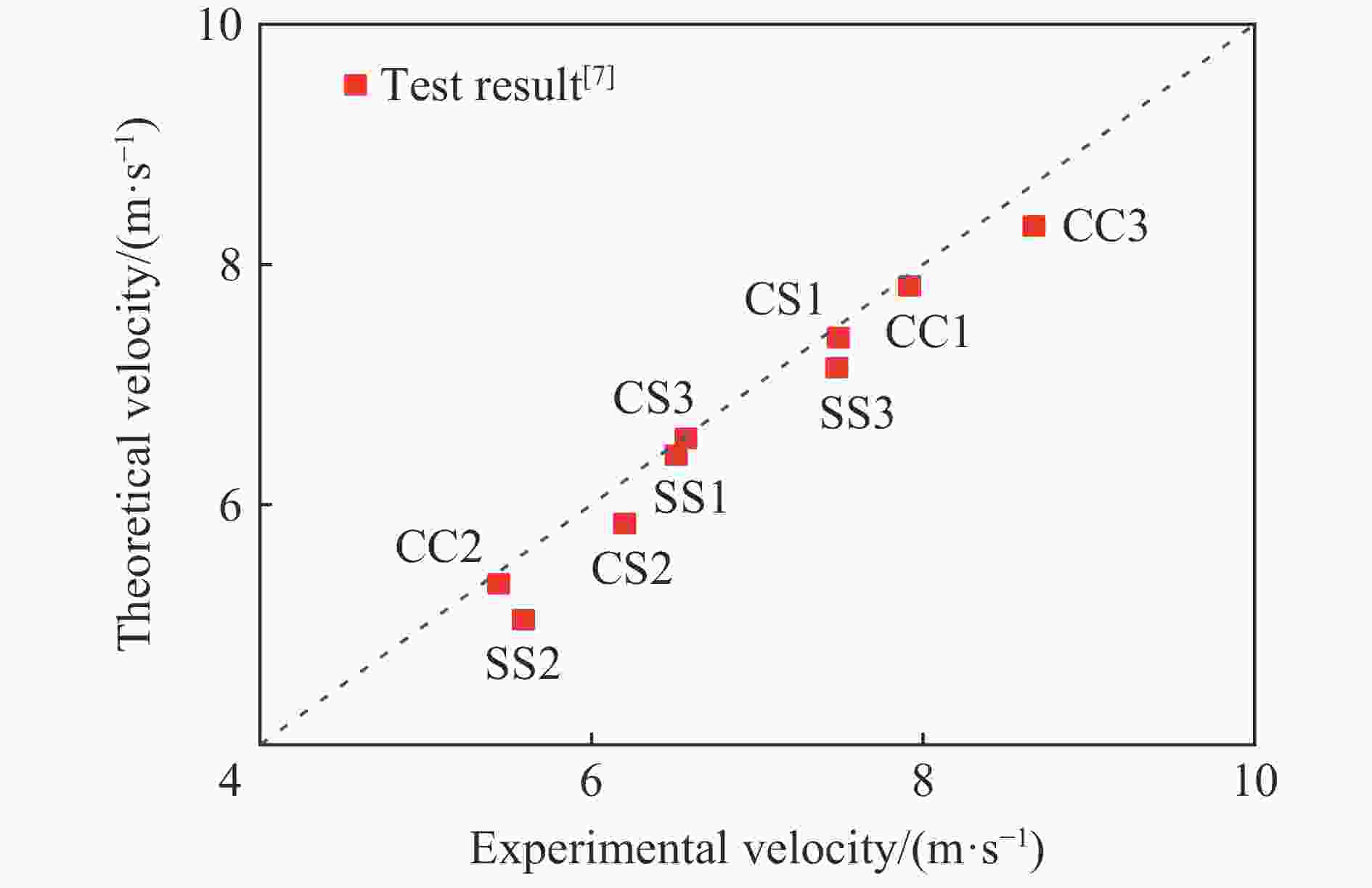
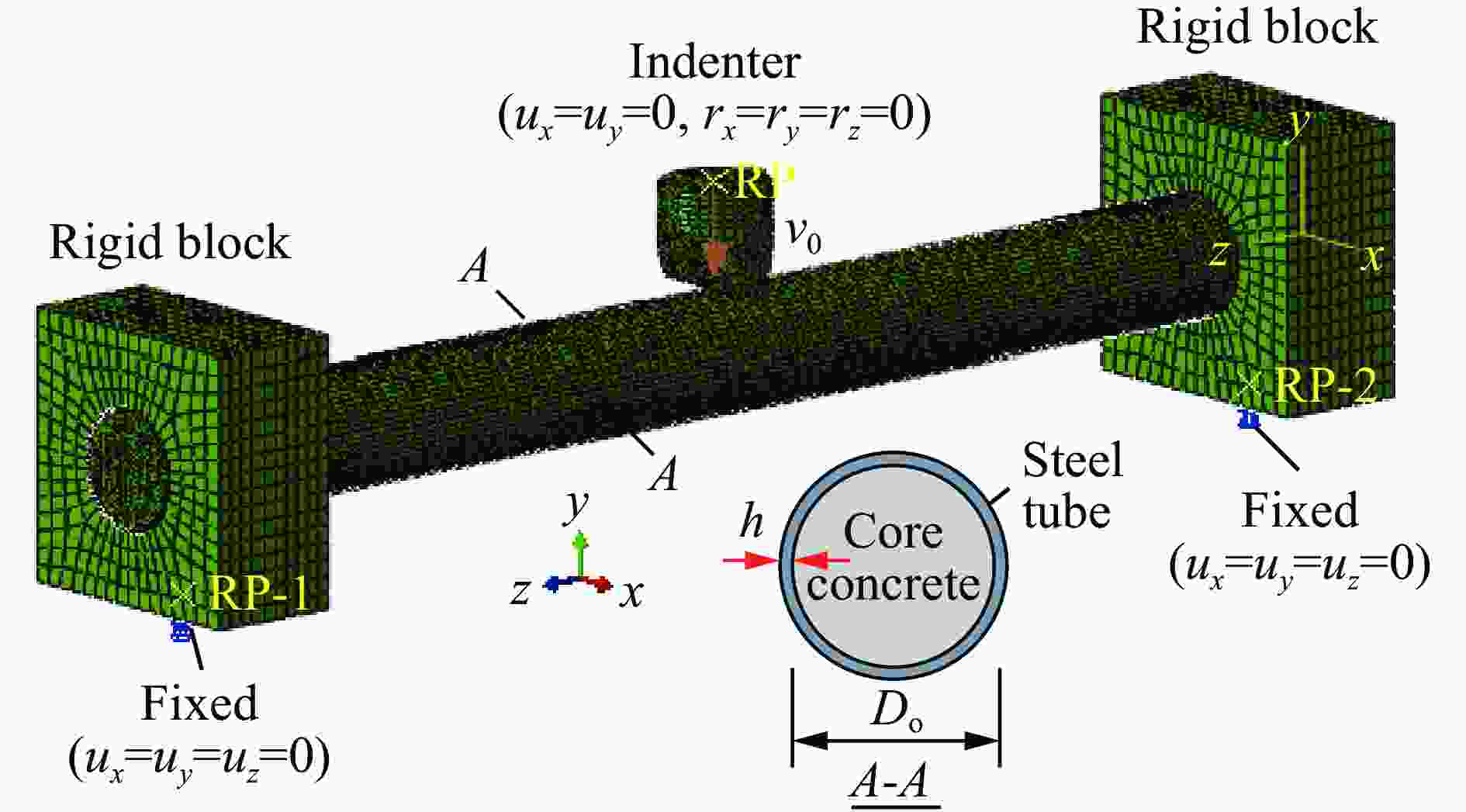
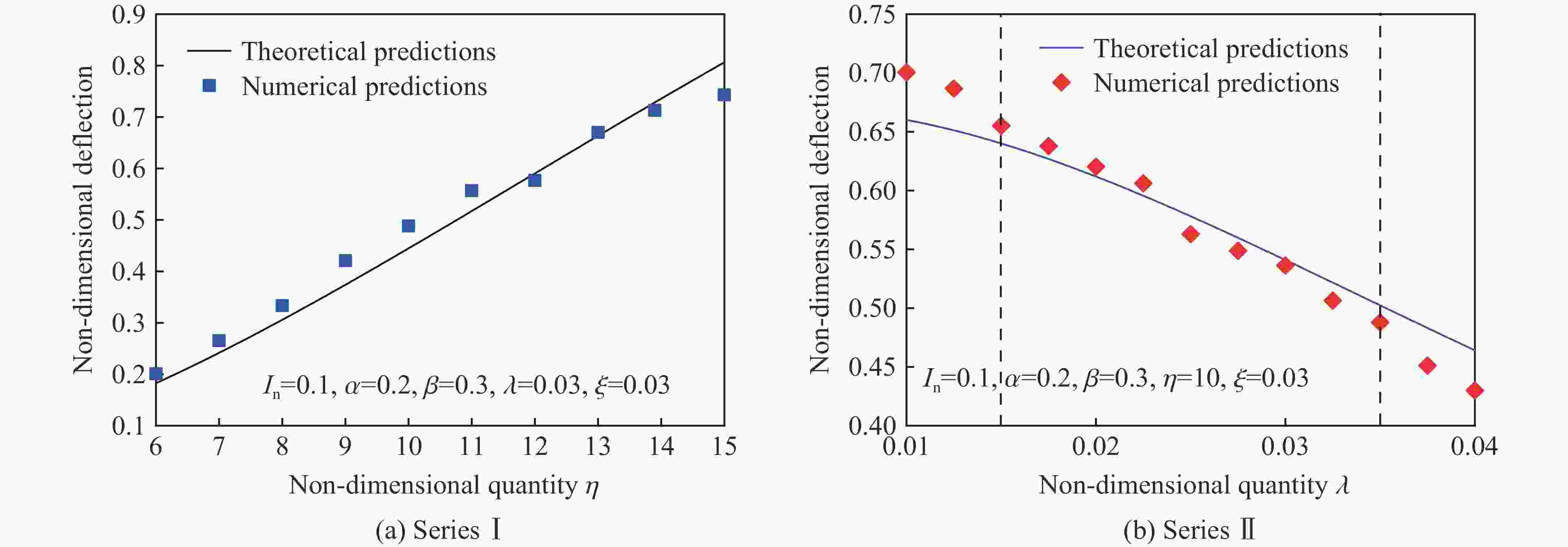
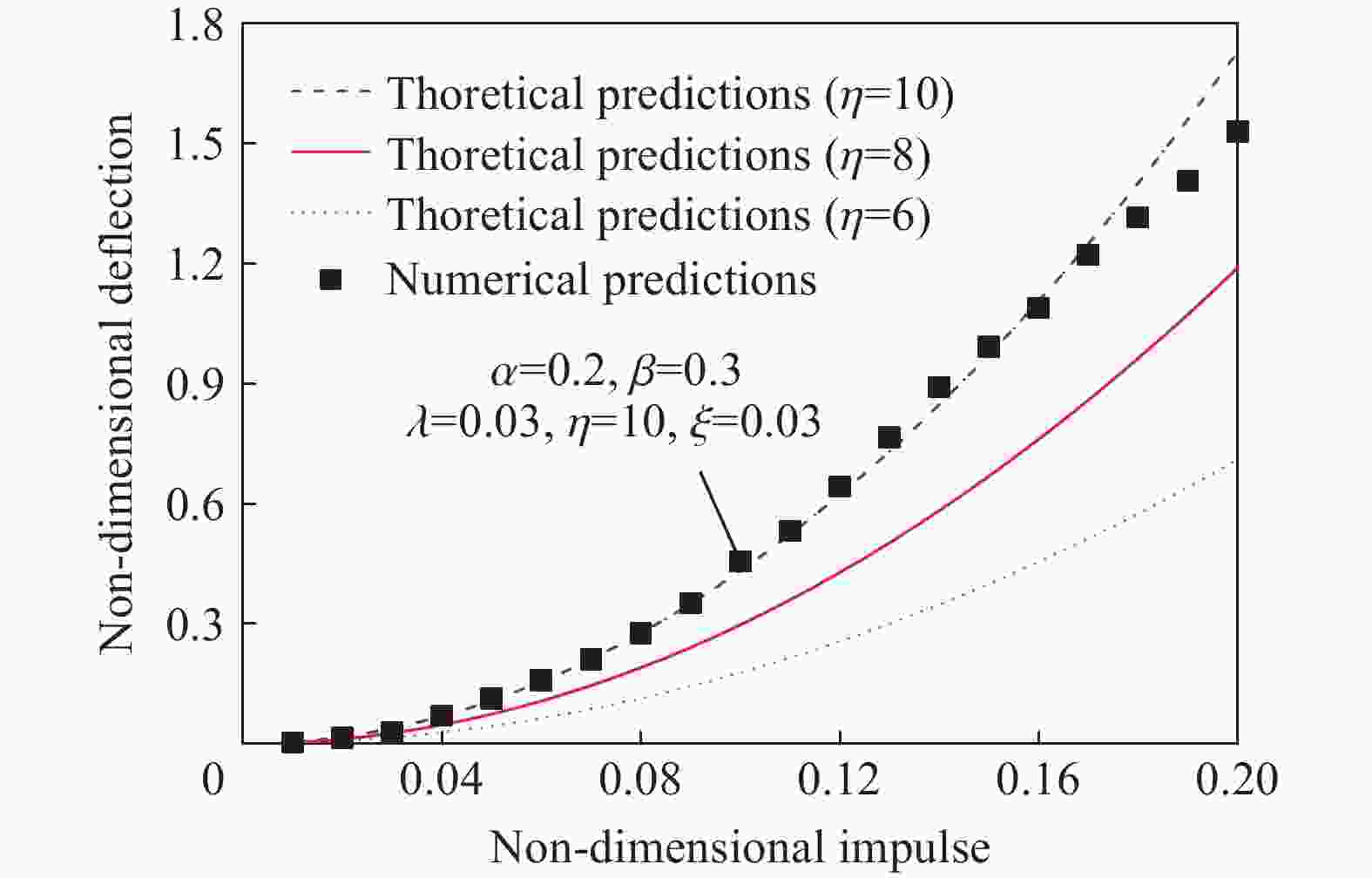
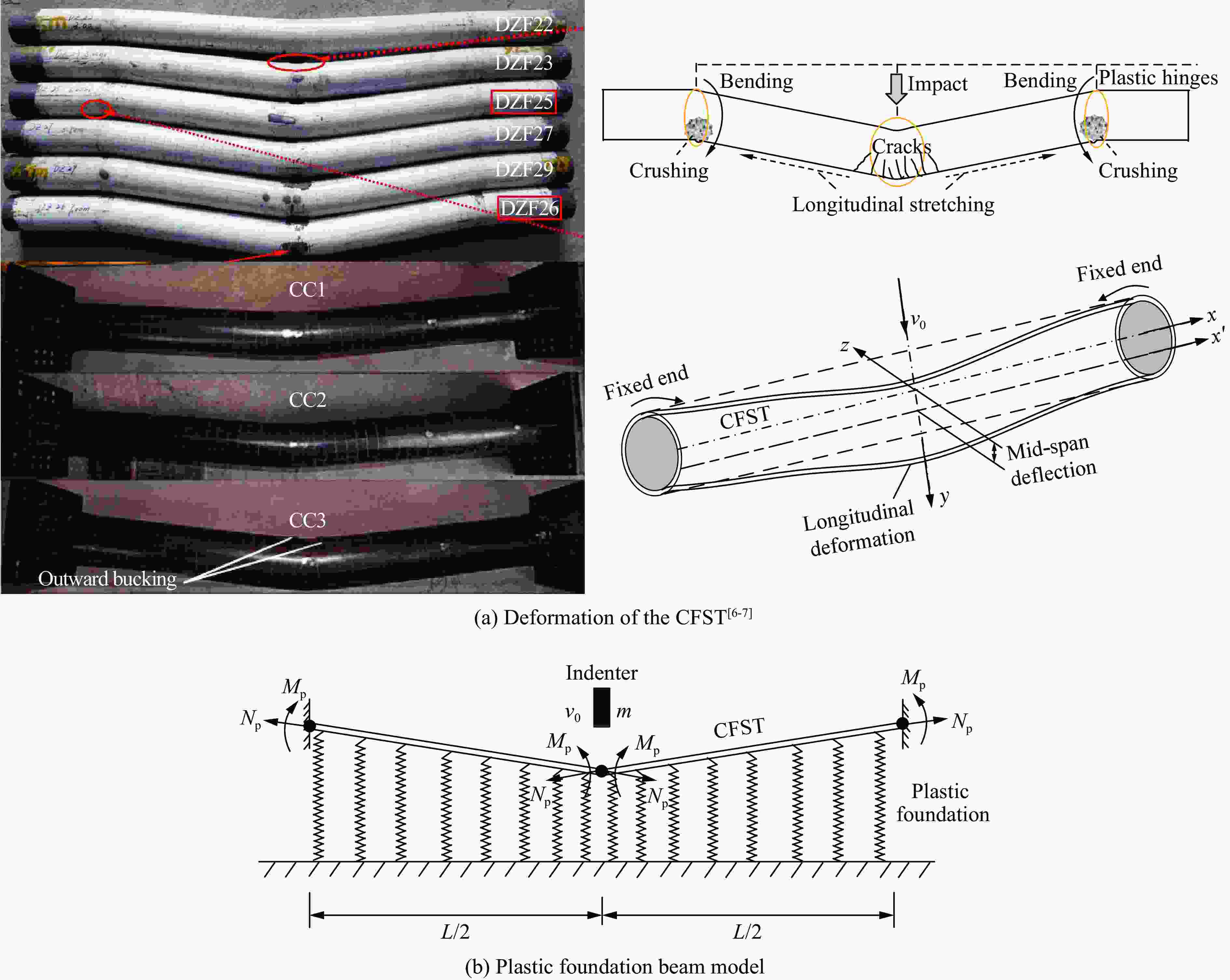
摘要: 采用刚塑性结构模态分析法和数值模拟方法，对侧向冲击载荷作用下的圆截面钢管混凝土结构进行了塑性动力分析。将钢管混凝土等效为刚塑性地基梁模型，给出了钢管混凝土构件跨中侧向变形的模态解析解，得到了冲击载荷作用下影响结构最终侧向变形的无量纲参数。利用ABAQUS/Explicit软件建立了钢管混凝土结构在侧向冲击作用下的动态响应数值模型并进行了计算分析，将理论预测值和数值模拟结果与试验结果进行了交叉对比。结合量纲分析和数值模型对影响构件最终变形的几何、物理参数及初始冲量进行了分析。结果表明：理论预测值和数值模拟结果与试验结果吻合较好，结构的塑性变形与理论假定的塑性铰分布一致。构件几何参数中，长径比和径厚比对其侧向最终变形有较大影响；冲击头相对宽度可改变构件的变形模态；相比于几何参数，钢管和混凝土芯层的物理参数对构件跨中挠度的影响较小；结构的侧向变形与初始冲量成二次幂相关。最后给出了理论分析参数的适用范围。刚塑性响应模态解可较好地预测钢管混凝土结构在侧向冲击载荷作用下的塑性变形行为。Abstract: By employing the mode approximation method for rigid-plastic structural dynamic behavior and numerical simulation, a dynamic response analysis was conducted on circular-section concrete-filled steel tubular (CFST) structures subjected to lateral impact loadings. The mechanical model of the CFST structure was equivalently represented as a rigid-plastic foundation beam model according to its plastic behavior. Under the linear velocity field assumption and the geometric similarity, the equivalently initial velocity for mode approximation of the structure was derived and compared with the existing experimental data. An analytical solution for the plastic lateral deformation at the mid-span of the CFST with two fixed ends by the rigid-plastic mode approximation method was provided, yielding non-dimensional geometric and physical parameters that influenced the ultimate lateral plastic deformation. A numerical model of the CFST structure under lateral impact was established using ABAQUS/Explicit. The theoretical and numerical predictions were both compared with existing experimental global deformations. Dimensional analysis and numerical modeling were combined to analyze the geometric and physical parameters, as well as the initial impact impulse, which influence the plastic deformation of the CFST structure. The results demonstrate a good agreement between the theoretical, numerical results, and experimental data, confirming that the plastic deformations of the structure align with the assumed distribution of plastic hinges. For geometric variables, the ratio of length to diameter and ratio of thickness to diameter exert a significant influence on the final lateral deformation. The relative width of the indenter can alter the deformation shape of the structure. The physical parameters of the steel tube and core concrete have less impact on the deflection at the mid-span compared with the geometric variables. The final lateral deformation of the CFST structure exhibits a quadratic correlation with the initial impact impulse. Finally, the applicable range of all the theoretical analysis variables is given according to the corresponding parameter analysis. The proposed mode solutions for rigid-plastic response provide a reliable prediction of the plastic deformation behavior of the CFST structures under lateral impact loadings.
-
图 1 钢管混凝土结构的变形和力学模型
Figure 1. Schematic diagrams of CFST structure deformation and mechanical model
图 2 等效初速度时刻构件的速度场分布
Figure 2. Velocity field of the mechanical model at the time of the equivalent initial velocity
图 6 理论和试验挠度的对比
Figure 6. Comparison between theoretical and experimental mid-span deflections
图 7 模态解几何参数对构件跨中挠度的影响
Figure 7. Effect of geometric parameters in mode solution on the mid-span nondimensional deflection of CFST
图 8 几何参数数值模拟结果与理论预测值的对比（组Ⅰ和Ⅱ）
Figure 8. Comparison between theoretical and numerical results of geometric parameters (seriesⅠand Ⅱ)
图 9 不同冲击头宽度下构件的变形和塑性损伤
Figure 9. Deformation and damage of steel tube and core concrete under different indenter sizes
图 10 物理参数
$\alpha $ 和$\beta $ 对构件跨中挠度的影响（表2第Ⅳ组）Figure 10. Impact of physical parameters
$\alpha $ and$\beta $ on the mid-span nondimensional deflection (series Ⅳ in Table 2)
图 11 初始冲量
${I_{\text{n}}}$ 对构件跨中挠度的影响（组Ⅴ）Figure 11. Impact of initial impulse
${I_{\text{n}}} $ on the mid-span nondimensional deflection (series Ⅴ)表 1 试验构件参数
Table 1. Parameters of test members
编号 原文编号 m/kg L/m v0/(m·s−1) Do/mm h/mm fs/MPa fc/MPa $ w_{\text{f}}^{\text{*}} / {\rm{mm}}$ 来源 1 CC1 465 1.74 9.10 180 3.65 247 65.74 57.00 文献[7] 2 CC2 920 1.74 6.40 180 3.65 247 65.74 60.00 3 CC3 465 1.74 9.67 180 3.65 247 65.74 72.00 4 DBF14 229.8 1.2 3.96 120 1.70 232 33.73 19.44 文献[5] 5 DBF16 229.8 1.2 4.14 120 1.70 232 47.5 25.66 6 DZF22 229.8 1.2 7.67 120 3.50 298 47.5 39.42 7 DZF23 229.8 1.2 9.90 120 3.50 298 47.5 63.78 8 DZF24 229.8 1.2 10.19 120 3.50 298 47.5 65.40 9 DZF25 229.8 1.2 8.93 120 3.50 298 47.5 72.42 10 DZF28 229.8 1.2 11.54 120 3.50 298 47.5 79.42 11 DZF30 229.8 1.2 11.63 120 3.50 298 47.5 82.30 12 DHF35 229.8 1.2 10.84 120 4.50 290 47.5 33.06 13 DHF36 229.8 1.2 14.48 120 4.50 290 47.5 73.24 14 DHF37 229.8 1.2 14.00 120 4.50 290 47.5 56.20 15 DHF39 229.8 1.2 11.71 120 4.50 290 47.5 38.30 16 DHF40 229.8 1.2 12.52 120 4.50 290 47.5 48.10 
下载: 导出CSV
表 2 有限元算例参数
Table 2. Parameters of the FE model
组别 m0/kg v0/(m·s−1) Do/mm h/mm fc/MPa ρc/(kg·m−3) B/mm Ⅰ 920 16～6.75 300～120 3.6～9.0 60 2 440 27 Ⅱ 920 8.1～11.2 180 1.8～10.8 60 2 440 27 Ⅲ 920 9.4 180 5.4 60 2 440 9～450 Ⅳ 920 9.4 180 5.4 40～80 2 300～2 600 27 Ⅴ 150～1000 2.8～14.3 180 5.4 60 2 440 27
下载: 导出CSV
-
[1] HAH L H, LI W, BJORHOVDE R. Developments and advanced applications of concrete-filled steel tubular (CFST) structures: members [J]. Journal of Constructional Steel Research, 2014, 100: 211–228. DOI: 10.1016/j.jcsr.2014.04.016. [2] 余同希, 朱凌, 许骏. 结构冲击动力学进展 (2010−2020) [J]. 爆炸与冲击, 2021, 41(12): 121401. DOI: 10.11883/bzycj-2021-0113.YU T X, ZHU L, XU J. Progress in structural impact dynamics during 2010−2020 [J]. Explosion and Shock Waves, 2021, 41(12): 121401. DOI: 10.11883/bzycj-2021-0113. [3] FAN W, XU X, ZHANG Z, et al. Performance and sensitivity analysis of UHPFRC-strengthened bridge columns subjected to vehicle collisions [J]. Engineering Structures, 2018, 173: 251–268. DOI: 10.1016/j.engstruct.2018.06.113. [4] XU M, GAO S, GUO L H, et al. Study on collapse mechanism of steel frame with CFST-columns under column-removal scenario [J]. Journal of Constructional Steel Research, 2018, 141: 275–286. DOI: 10.1016/j.jcsr.2017.11.020. [5] 王蕊. 钢管混凝土结构构件在侧向撞击下的动力响应及其损伤破坏的研究 [D]. 太原: 太原理工大学, 2008: 19–49.WANG R. Study on the dynamic response and damage failure of concrete filled steel tube under lateral impact [D]. Taiyuan, Shanxi, China: Taiyuan University of Technology, 2008: 19–49. [6] WANG R, HAN L H, HOU C C. Behavior of concrete filled steel tubular (CFST) members under lateral impact: experiment and FEA model [J]. Journal of Constructional Steel Research, 2013, 80: 188–201. DOI: 10.1016/j.jcsr.2012.09.003. [7] HAN L H, HOU C C, ZHAO X L, et al. Behaviour of high-strength concrete filled steel tubes under transverse impact loading [J]. Journal of Constructional Steel Research, 2014, 92: 25–39. DOI: 10.1016/j.jcsr.2013.09.003. [8] 王潇宇, DEMARTINO C, 徐金俊, 等. 侧向冲击作用下钢管混凝土柱动力响应试验研究及计算方法 [J]. 土木工程学报, 2017, 50(12): 28–36. DOI: 10.15951/j.tmgcxb.2017.12.004.WANG X Y, DEMARTINO C, XU J J, et al. Dynamic response of concrete filled steel tube column under lateral impact load: experimental study and calculation method [J]. China Civil Engineering Journal, 2017, 50(12): 28–36. DOI: 10.15951/j.tmgcxb.2017.12.004. [9] ZHU A Z, XU W, GAO K, et al. Lateral impact response of rectangular hollow and partially concrete-filled steel tubular columns [J]. Thin-Walled Structures, 2018, 130: 114–131. DOI: 10.1016/j.tws.2018.05.009. [10] 王文达, 陈振福, 纪孙航. 长期持荷工况下钢管混凝土构件的抗撞击性能 [J]. 爆炸与冲击, 2021, 41(8): 083106. DOI: 10.11883/bzycj-2020-0204.WANG W D, CHEN Z F, JI S H, Impact resistance of concrete-filled steel tubular members under long-term loading [J]. Explosion and Shock Waves, 2021, 41(8): 083106. DOI: 10.11883/bzycj-2020-0204. [11] 王路明, 刘艳辉, 赵世春, 等. 侧向低速冲击作用下钢管混凝土构件开裂评估模型及影响因素研究 [J]. 土木工程学报, 2022, 55(3): 7–17, 35. DOI: 10.15951/j.tmgcxb.2022.03.001.WANG L M, LIU Y H, ZHAO S C, et al. Study on evaluation and influencing factors for cracking of concrete-filled steel tubular members subjected to lateral low-velocity impact [J]. China Civil Engineering Journal, 2022, 55(3): 7–17, 35. DOI: 10.15951/j.tmgcxb.2022.03.001. [12] 瞿海雁, 李国强, 孙建运, 等. 侧向冲击作用下钢管混凝土构件的简化分析模型 [J]. 同济大学学报 (自然科学版), 2011, 39(1): 35–41. DOI: 0253-374X(2011)01-0035-07.QU H Y, LI G Q, SUN J Y, et al. Simplified analysis model of circular concrete filled steel tube specimen under lateral impact [J]. Journal of Tongji University (Natural science), 2011, 39(1): 35–41. DOI: 0253-374X(2011)01-0035-07. [13] BAMBACH M R, JAMA H, ZHAO X L, et al. Hollow and concrete filled steel hollow sections under transverse impact loads [J]. Engineering Structures, 2008, 30(10): 2859–2870. DOI: 10.1016/j.engstruct.2008.04.003. [14] WANG Y, QIAN X, LIEW J Y R, et al. Impact of cement composite filled steel tubes: an experimental, numerical and theoretical treatise [J]. Thin-Walled Structures, 2015, 87: 76–88. DOI: 10.1016/j.tws.2014.11.007. [15] 余同希, 华云龙. 结构塑性动力学引论 [M]. 合肥: 中国科技大学出版社, 1994: 88−89. [16] MARTIN J B, SYMONDS P S. Mode approximations for impulsively loaded rigid plastic structures [J]. Journal of the Engineering Mechanics Division, 1965, 92(5): 61. DOI: 10.1061/JMCEA3.0001036. [17] YU T X, STRONGE W J. Large deflections of a rigid-plastic beam-on-foundation from impact [J]. International Journal of Impact Engineering, 1990, 9(1): 115–126. DOI: 10.1016/0734-743x(90)90025-q. [18] 于博丽, 冯根柱, 李世强, 等. 横向爆炸载荷下薄壁圆管的动态响应 [J]. 爆炸与冲击, 2019, 39(10): 103101. DOI: 10.11883/bzycj-2018-0295.YU B L, FENG G Z, LI S Q, et al. Dynamic response of thin-wall circular tubes under transverse blast loading [J]. Explosion and Shock Waves, 2019, 39(10): 103101. DOI: 10.11883/bzycj-2018-0295. [19] WALTERS R M, JONES N. An approximate theoretical study of the dynamic plastic behavior of shells [J]. International Journal of Non-Linear Mechanics, 1972, 7(3): 255–273. DOI: 10.1016/0020-7462(72)90049-2. [20] 张煜航, 陈青青, 张杰, 等. 混凝土三维细观模型的建模方法与力学特性分析 [J]. 爆炸与冲击, 2019, 39(5): 054205. DOI: 10.11883/bzycj-2018-0408.ZHANG Y H, CHEN Q Q, ZHANG J, et al. 3D mesoscale modeling method and dynamic mechanical [J]. Explosion and Shock Waves, 2019, 39(5): 054205. DOI: 10.11883/bzycj-2018-0408. -
计量
- 文章访问数: 295
- HTML全文浏览量: 126
- PDF下载量: 67
- 被引次数: 0