Blast resistance of reinforced concrete arches subjected to underwater explosions
-
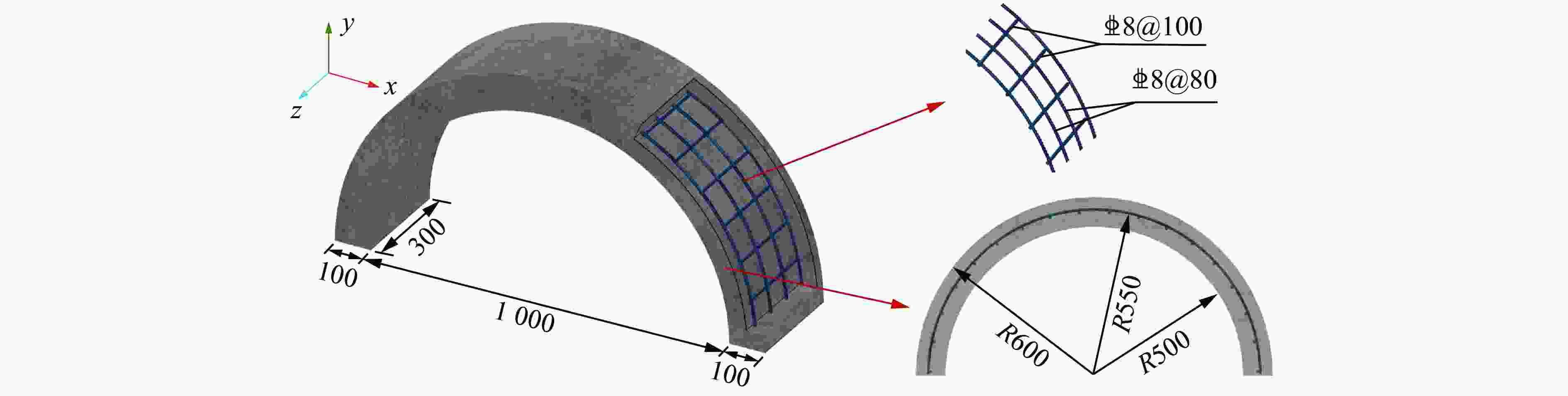
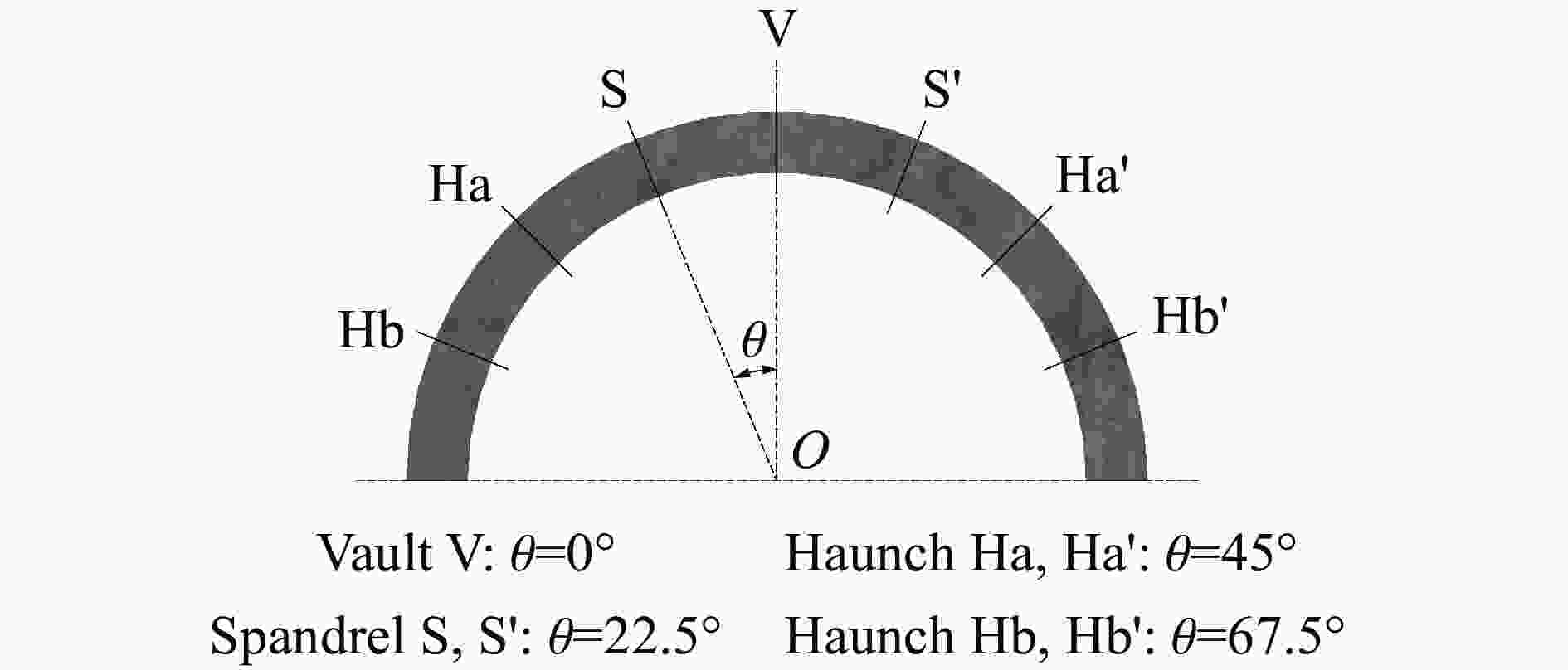
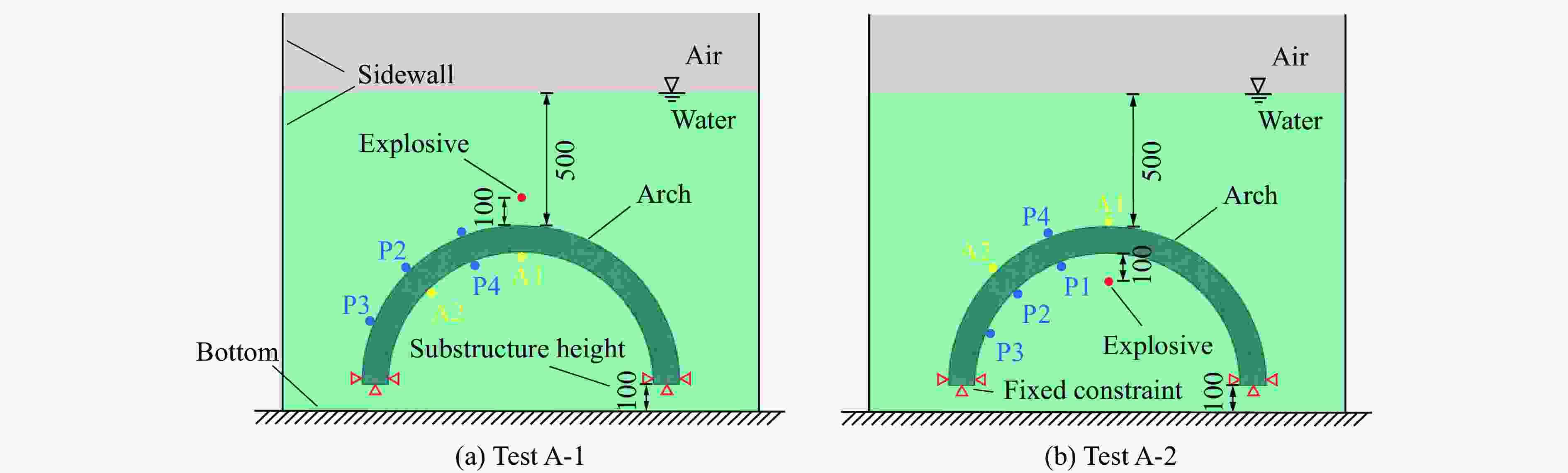
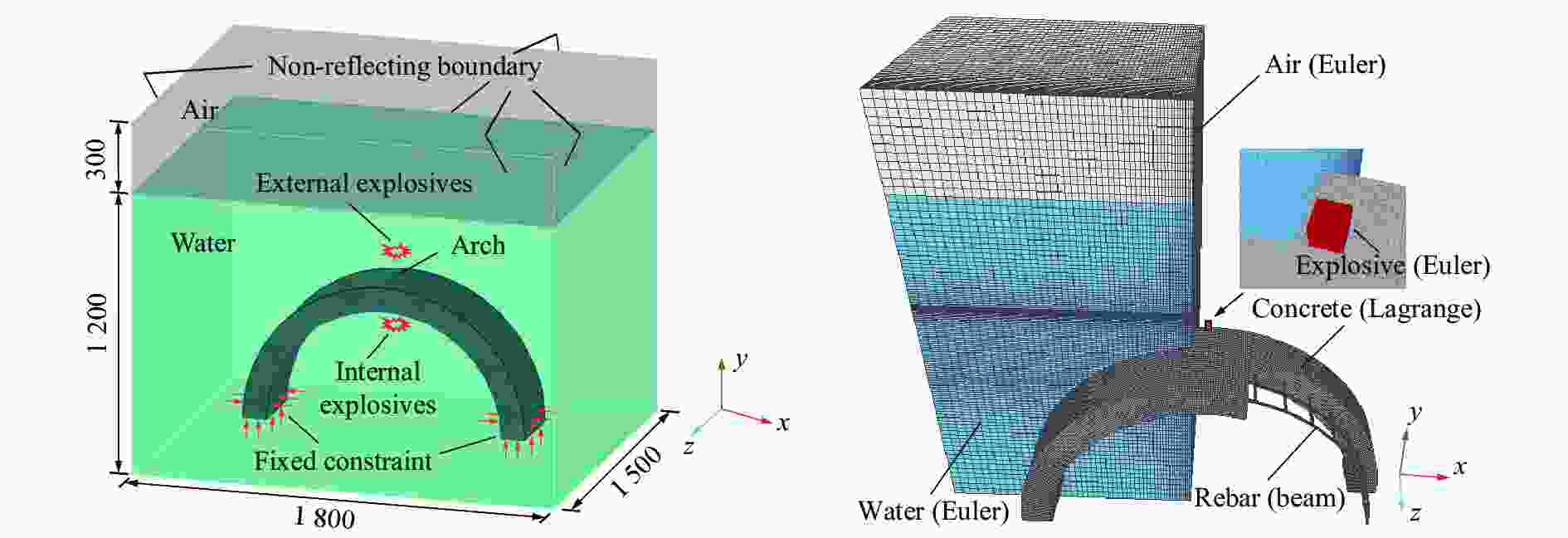
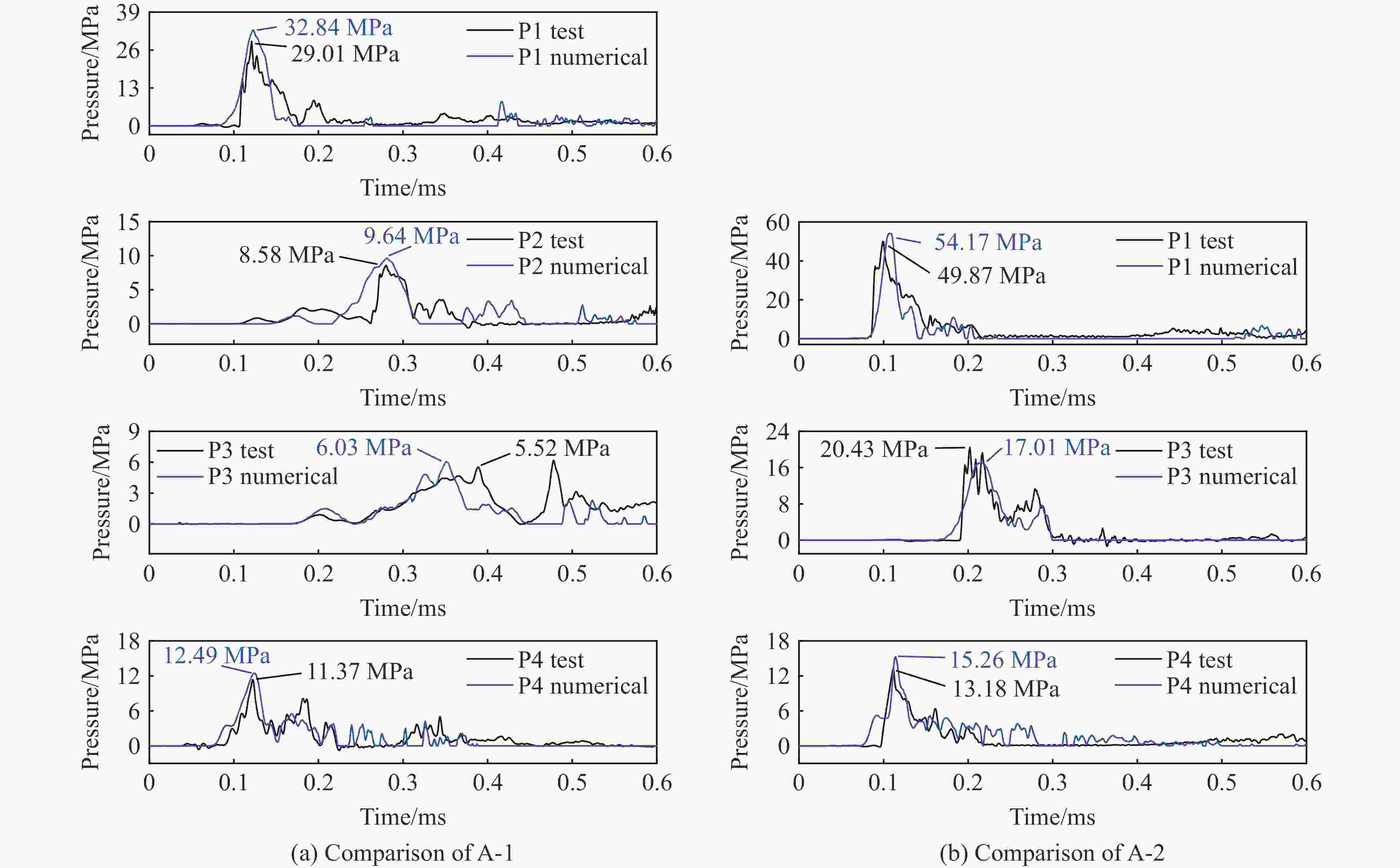
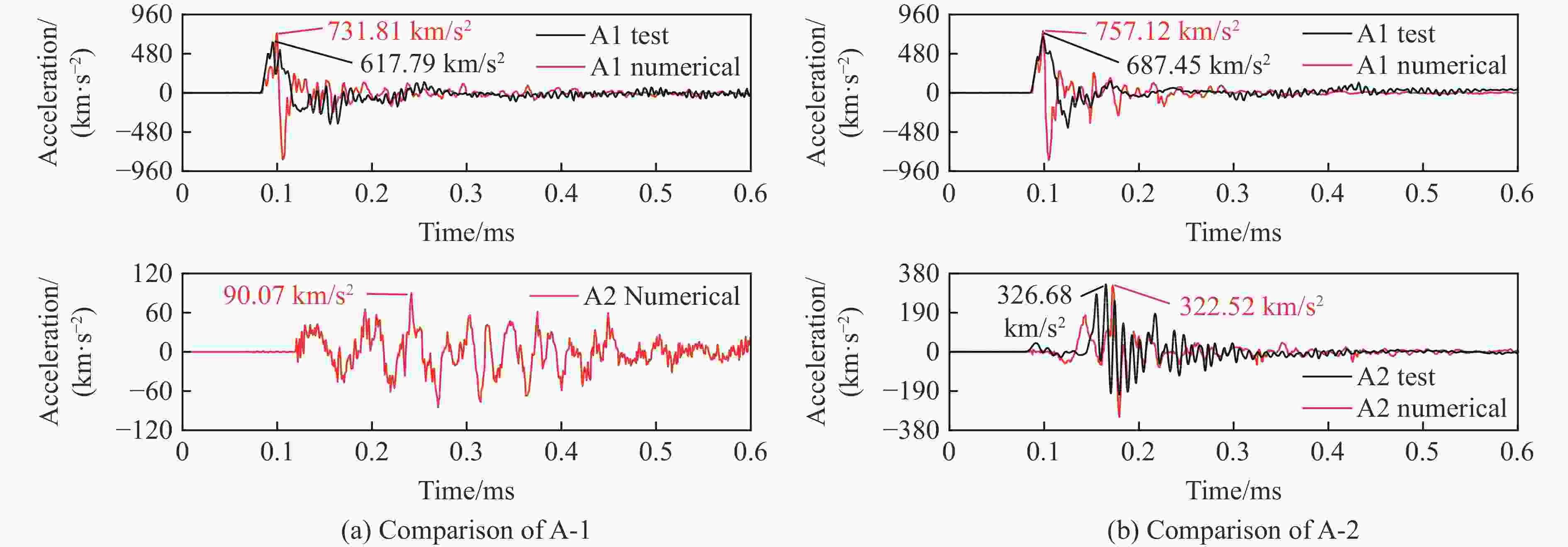
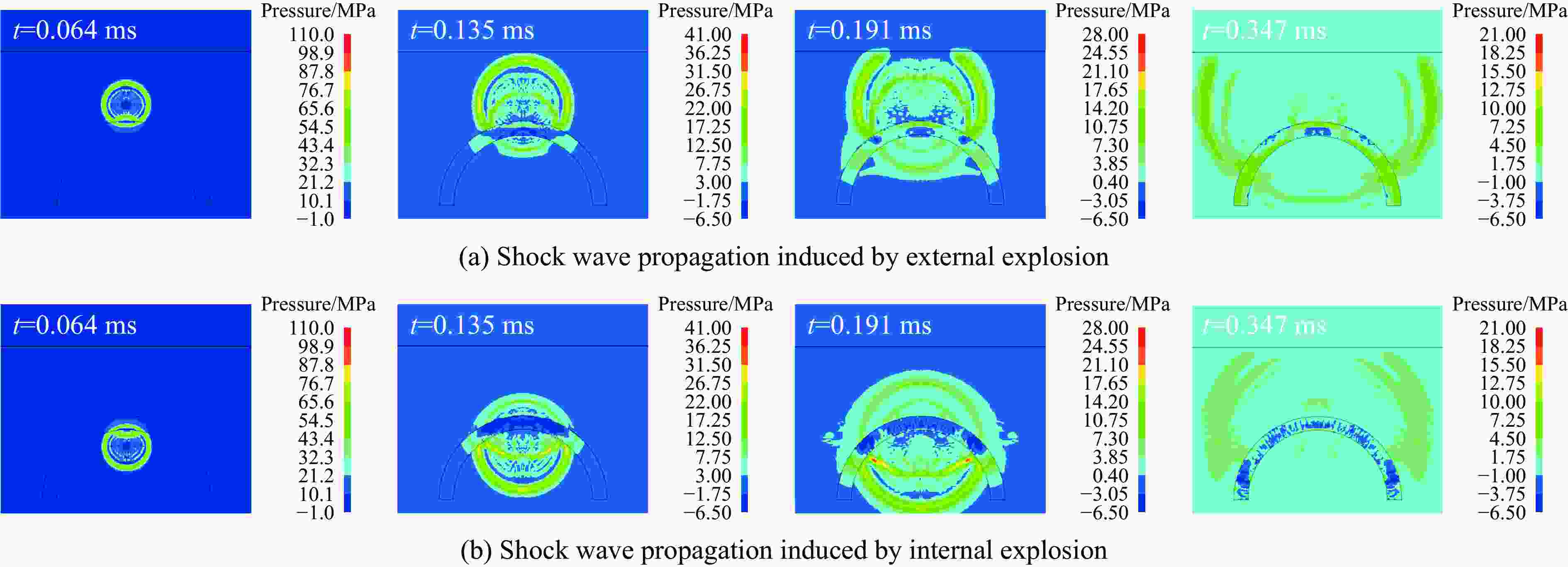
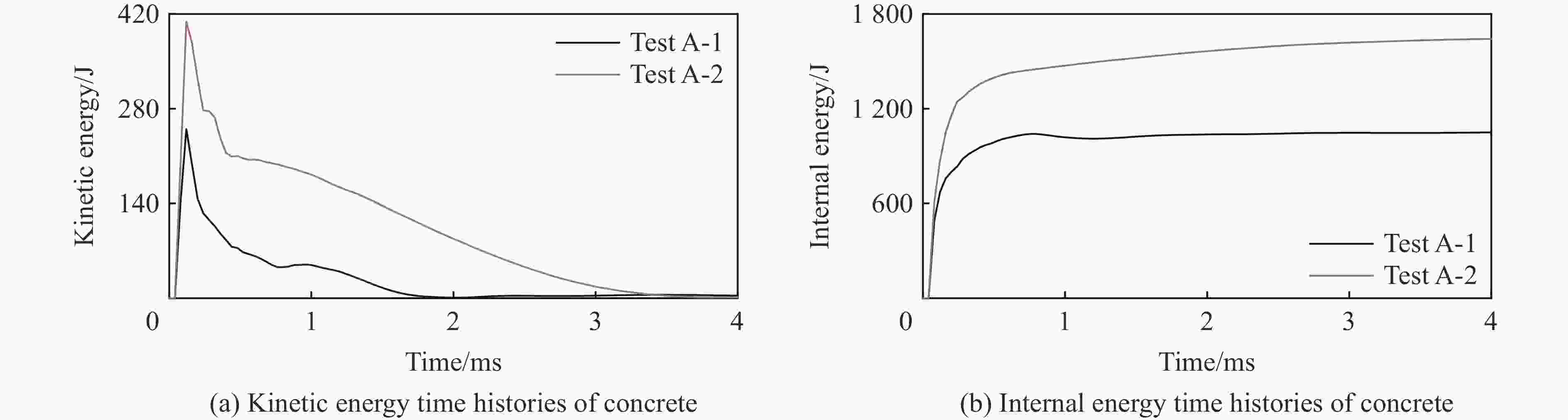
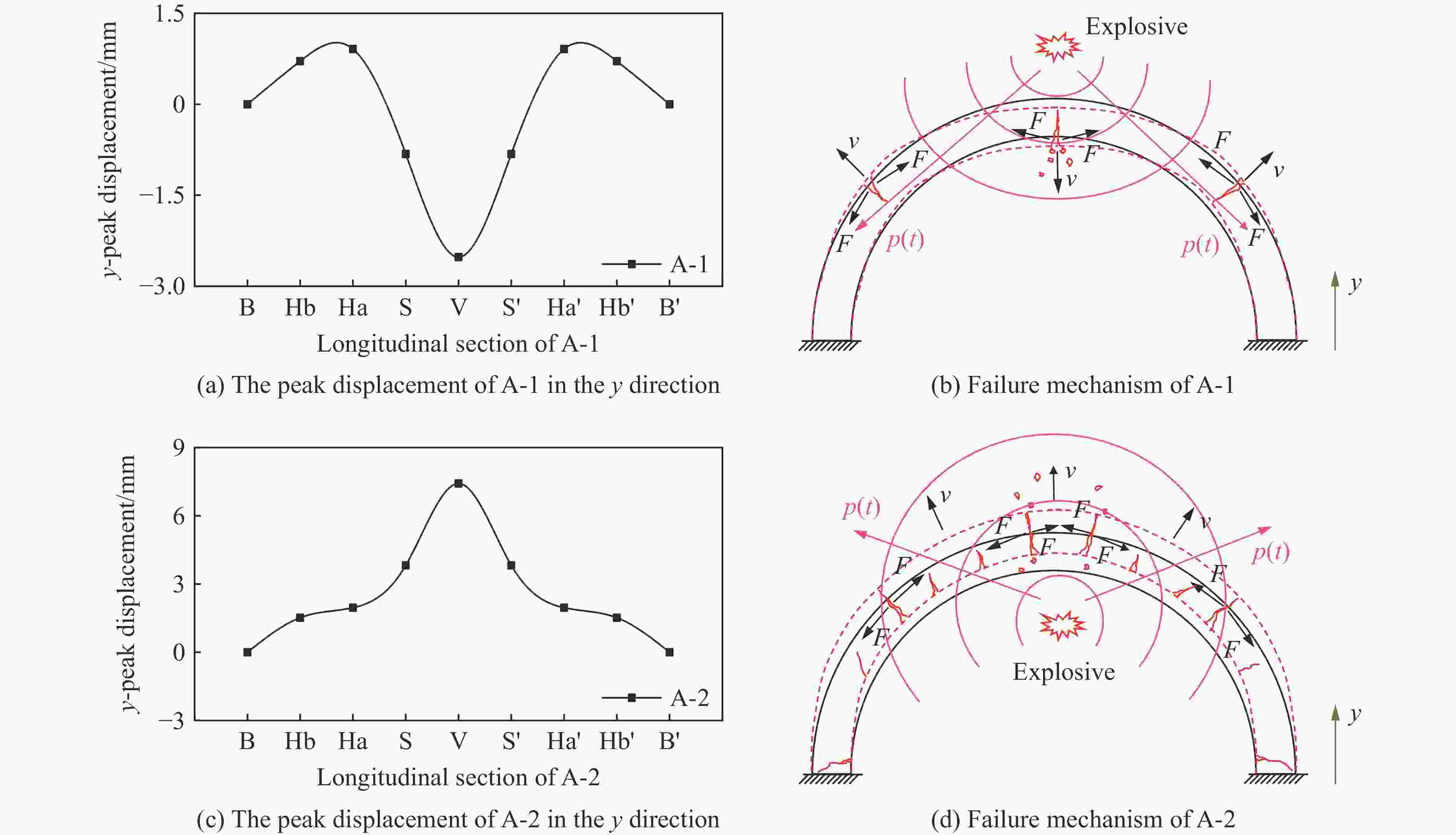
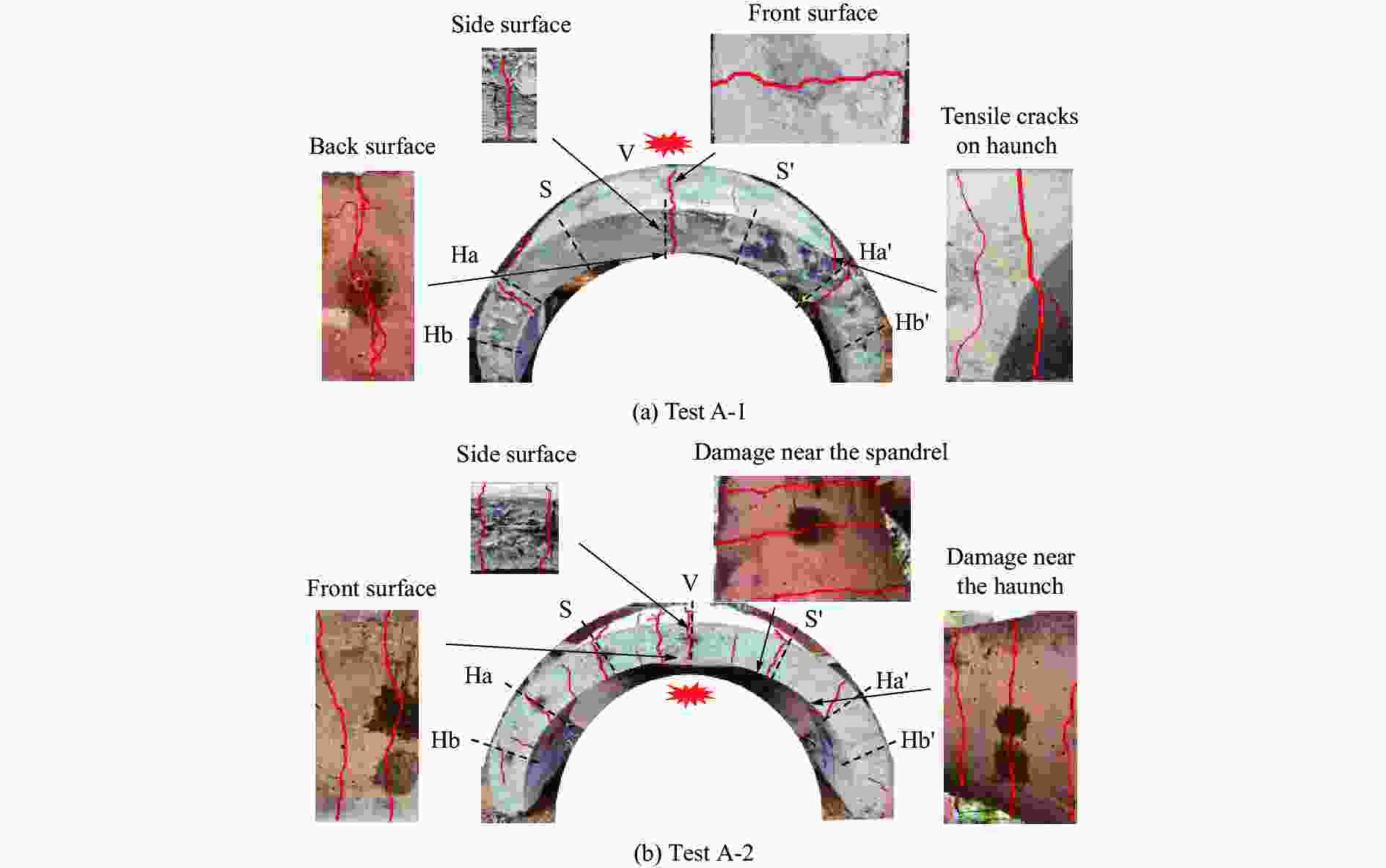
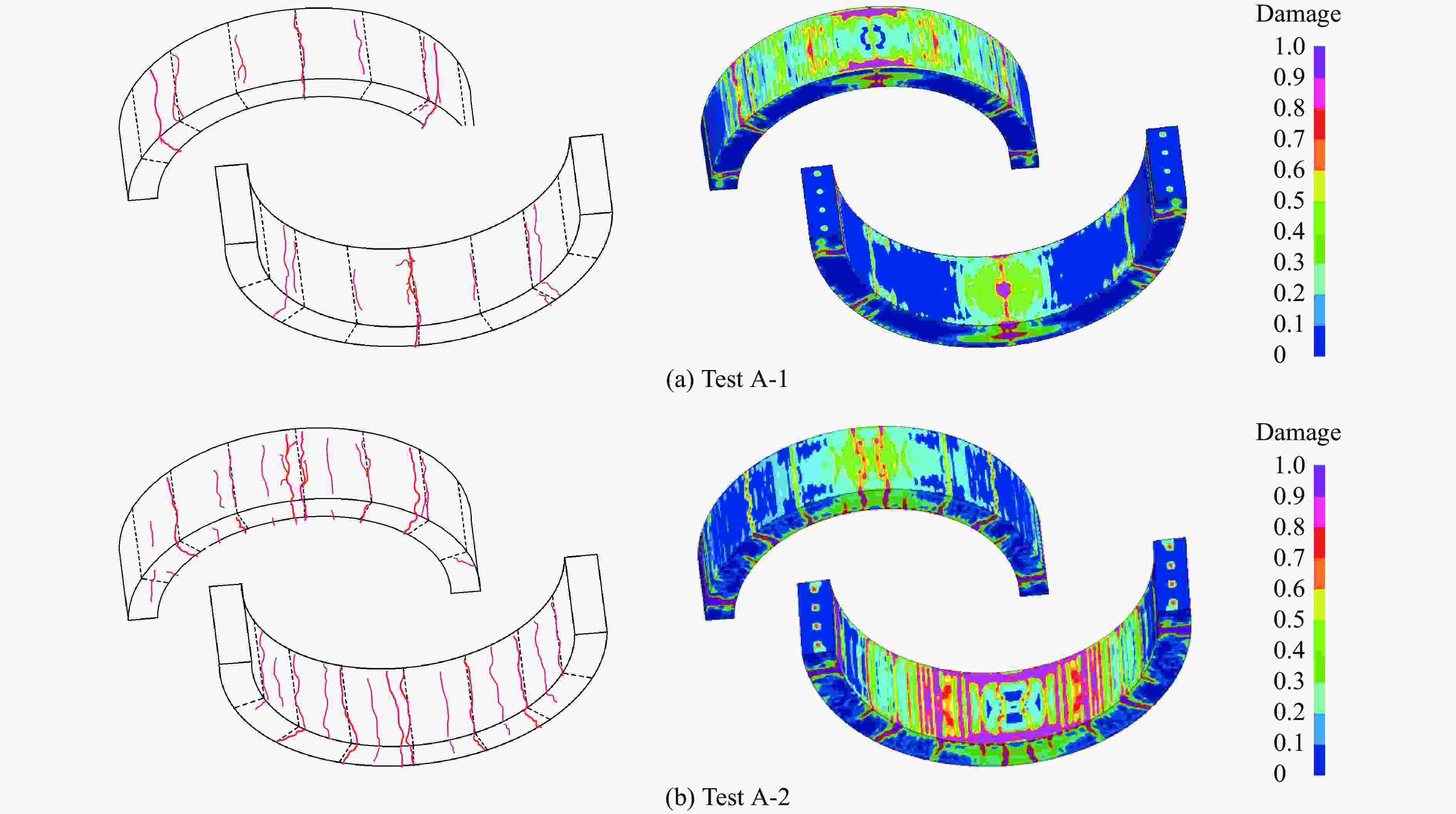
摘要: 为探究水下爆炸荷载作用下钢筋混凝土拱的动力响应特性和破坏特征,制作了两个钢筋混凝土拱试件,并开展了水下爆炸试验。试验分为拱外爆炸和拱内爆炸两组,采用10 g乳化炸药,试验时爆源距结构面最小距离为10 cm(起爆点位于拱结构正上方和正下方),通过传感器记录爆炸试验中钢筋混凝土拱典型断面处的水压力及加速度时程曲线。基于Arbitrary Lagrange-Euler (ALE)算法,建立了空气-水-炸药-钢筋混凝土拱等多介质动态耦合作用模型,将数值模拟结果与试验结果对比,验证了数值方法的可靠性。采用验证后的数值模型进一步研究了拱外及拱内爆炸荷载作用下钢筋混凝土拱的动力响应差异。结果表明:相同炸药当量下,内部爆炸有更多的能量作用于混凝土拱,使结构的动力响应更强烈;外部爆炸下,拱顶、拱腰处产生较大裂缝;内部爆炸时,迎爆面裂缝数量明显增多,拱肩位置出现裂缝。钢筋混凝土拱形结构抵抗外部爆炸荷载的能力明显强于内部爆炸荷载。Abstract: As common structures in hydraulic engineering, the arch structures may suffer from explosion load during their operation life. In order to explore the dynamic response characteristics and failure features of reinforced concrete arches subjected to underwater explosions, two reinforced concrete arch specimens were fabricated and underwater explosion tests were carried out. The tests consisted of two groups: external explosion and internal explosion. 10 g emulsion explosives were used, and the minimum distance between the explosion source and the structure was 10 cm (the explosives were placed directly above or below the arch). The time history curves of water pressure and structural acceleration at typical sections of the arches during the explosion tests were recorded. Based on the Arbitrary Lagrange-Euler (ALE) algorithm, a multi-material dynamic coupling model, including air, water, explosive, and reinforced concrete arch was established. The initiation of explosive, the propagation of shock wave, the interaction between fluid and solid, and the dynamic response of the structure were considered in the numerical model. The reliability of the numerical model was verified by comparing the numerical results and the experimental results. With the calibrated numerical model, the difference of dynamic response of reinforced concrete arches under external explosion and internal explosion was further studied. The results show that more energy acts on the concrete arch, and the structural response is stronger when subjected to internal explosion. Large cracks occur at the vault and waist induced by external explosion. Compared with the external explosion, the number of cracks significantly increases under internal explosion, and cracks also appear at the spandrel. The ability of reinforced concrete arch to resist external explosive loads is significantly stronger than that of internal explosive loads although the explosive weight is the same. For concrete arches that are vulnerable to external explosions, high strength concrete or reinforced reinforcement can be appropriately used in the arch vault and waist. For concrete arches that may be subjected to internal explosions, protective nets can be set to make the explosion occur at a longer distance away from the structures, or high strength materials can be used to resist the overall deformation.
-
表 1 每立方米混凝土配料
Table 1. Concrete ingredients per cubic meter
材料 用量/kg 配合比例 规格 水泥 428 1 P.O 42.5 天然河砂 728 1.7 0~3 mm粒径 碎石 1047 2.45 5~15 mm粒径 水 167 0.39 普通自来水 减水剂 5.56 0.013 聚羧酸型 表 2 炸药参数
Table 2. Explosive parameters
ρ0/(kg·m−3) D/(m·s−1) pCJ/ GPa m/GPa n/GPa R1 R2 ω Ee/MPa 1630 6930 21 373.77 3.75 4.15 0.9 0.35 6000 表 3 RHT混凝土参数
Table 3. Concrete parameters of RHT model
参数 取值 参数 取值 参数 取值 抗压强度fc/MPa 45 失效压缩应变率εT/s−1 3×1025 罗德角相关系数B 0.0105 密度ρ0/(kg·m−3) 2314 侵蚀体积应变εero 2.0 压缩应变率指数βC 0.026 初始孔隙度α0 1.1884 多项式参数A1/GPa 35.27 拉伸应变率指数βT 0.031 孔隙度指数αP 3 多项式参数A2/GPa 39.58 压缩屈服面参数GC* 0.53 拉压强度比ft* 0.102 多项式参数A3/GPa 9.04 拉伸屈服面参数GT* 0.7 剪压强度比fs* 0.18 状态方程参数B0 1.22 剪切模量缩减系数X 0.5 剪切模量Gel/GPa 17.21 状态方程参数B1 1.22 损伤参数D1 0.04 破碎压力pcr/MPa 30 状态方程参数T1/GPa 35.27 损伤指数D2 1 压实压力pco/GPa 6 状态方程参数T2/GPa 0 最小失效应变εmin 0.01 参考拉伸应变率ε0C/s−1 3×10−5 失效面参数A 1.6 残余面参数Af 1.6 参考压缩应变率ε0T/s−1 3×10−6 失效面指数N 0.61 残余面指数Nf 0.61 失效拉伸应变率εC/s−1 3×1025 拉压子午比参数Q0 0.6805 -
[1] 任伟, 盖轶婷, 张岗. 纤维复合材料加固初应力下的钢筋混凝土拱分析 [J]. 上海交通大学学报, 2015, 49(2): 232–238. DOI: 10.16183/j.cnki.jsjtu.2015.02.016.REN W, GAI Y T, ZHANG G. Analysis of RC arch strengthen by bonded FRP under initial stress [J]. Journal of Shanghai Jiao Tong University, 2015, 49(2): 232–238. DOI: 10.16183/j.cnki.jsjtu.2015.02.016. [2] 周健南, 金丰年, 范华林, 等. 震后地下拱结构的抗冲击波动载能力评估 [J]. 工程力学, 2012, 29(2): 159–164,171.ZHOU J N, JIN F N, FAN H L, et al. Residual dynamic resistance of seismic damaged underground arch [J]. Engineering Mechanics, 2012, 29(2): 159–164,171. [3] ZHANG X, WANG P, JIANG M R, et al. CFRP strengthening reinforced concrete arches: strengthening methods and experimental studies [J]. Composite Structures, 2015, 131: 852–867. DOI: 10.1016/j.compstruct.2015.06.034. [4] 杨绿峰, 陈致. 钢筋混凝土拱承载力分析的齐次广义屈服函数 [J]. 工程力学, 2023, 40(4): 71–79. DOI: 10.6052/j.issn.1000-4750.2021.09.0734.YANG L F, CHEN Z. Homogeneous generalized yield function for bearing capacity analysis of reinforced concrete arch [J]. Engineering Mechanics, 2023, 40(4): 71–79. DOI: 10.6052/j.issn.1000-4750.2021.09.0734. [5] TANG Z X, ZHOU Y Z, FENG J, et al. Concrete protective arches reinforced with BFRP bars: construction and quasi-static structural performances [J]. Tunnelling and Underground Space Technology, 2021, 108: 103731. DOI: 10.1016/j.tust.2020.103731. [6] TANG L Z, YU L, LUO X, et al. Shaking table test on the seismic response and reinforcement measures of double-arch tunnels in mountainous areas [J]. Tunnelling and Underground Space Technology, 2023, 139: 105232. DOI: 10.1016/j.tust.2023.105232. [7] CHEN W S, HAO H. Numerical study of a new multi-arch double-layered blast-resistance door panel [J]. International Journal of Impact Engineering, 2012, 43: 16–28. DOI: 10.1016/j.ijimpeng.2011.11.010. [8] 周忠欣, 金丰年, 袁小军, 等. 侧向点爆炸作用下地下拱结构的动力响应 [J]. 爆炸与冲击, 2018, 38(3): 639–646. DOI: 10.11883/bzycj-2016-0295.ZHOU Z X, JIN F N, YUAN X J, et al. Dynamic response of underground arch structure under lateral point blast loads [J]. Explosion and Shock Waves, 2018, 38(3): 639–646. DOI: 10.11883/bzycj-2016-0295. [9] LIU G K, WANG W, LIU R C, et al. Deriving formulas of loading distribution on underground arch structure surface under close-in explosion [J]. Engineering Failure Analysis, 2020, 115: 104608. DOI: 10.1016/j.engfailanal.2020.104608. [10] 吴克刚, 胡玉峰, 宋殿义, 等. 钢筋混凝土拱抗爆性能的数值模拟研究 [J]. 采矿技术, 2018, 18(6): 153–156. DOI: 10.3969/j.issn.1671-2900.2018.06.051.WU K G, HU Y F, SONG D Y, et al. Numerical simulation study on explosion resistance of reinforced concrete arch [J]. Mining Technology, 2018, 18(6): 153–156. DOI: 10.3969/j.issn.1671-2900.2018.06.051. [11] WANG P, JIANG M R, ZHOU J N, et al. Spalling in concrete arches subjected to shock wave and CFRP strengthening effect [J]. Tunnelling and Underground Space Technology, 2018, 74: 10–19. DOI: 10.1016/j.tust.2018.01.009. [12] XIAO Y, ZHU W Q, WU W C, et al. Damage modes and mechanism of RC arch slab under contact explosion at different locations [J]. International Journal of Impact Engineering, 2022, 170: 104360. DOI: 10.1016/j.ijimpeng.2022.104360. [13] 霍庆, 王逸平, 刘光昆, 等. 地下拱形结构侧顶爆炸的破坏模式及影响因素 [J]. 兵工学报, 2021, 42(S1): 105–116. DOI: 10.3969/j.issn.1000-1093.2021.S1.014.HUO Q, WANG Y P, LIU G K, et al. Failure mode and influencing factors of underground arched structure subjected to side top blast [J]. Acta Armamentarii, 2021, 42(S1): 105–116. DOI: 10.3969/j.issn.1000-1093.2021.S1.014. [14] 陈昊, 卢浩, 孙善政, 等. 常规武器二次爆炸作用下浅埋钢筋混凝土拱结构破坏规律 [J]. 爆炸与冲击, 2023, 43(8): 085104. DOI: 10.11883/bzycj-2022-0260.CHEN H, LU H, SUN S Z, et al. Failure law of shallow buried reinforced concrete arch structure under secondary explosion of conventional weapons [J]. Explosion and Shock Waves, 2023, 43(8): 085104. DOI: 10.11883/bzycj-2022-0260. [15] 周听清. 爆炸动力学及其应用 [M]. 合肥: 中国科学技术大学出版社, 2001: 108–118. [16] 闫秋实, 宁素瑜, 杜修力, 等. 水中近场爆炸作用下钢筋混凝土桩毁伤效应研究 [J]. 北京工业大学学报, 2019, 45(2): 153–159. DOI: 10.11936/bjutxb2017110011.YAN Q S, NING S Y, DU X L, et al. Damage effect for a typical reinforced concrete pile under the near field explosion in water [J]. Journal of Beijing University of Technology, 2019, 45(2): 153–159. DOI: 10.11936/bjutxb2017110011. [17] 刘靖晗, 唐廷, 韦灼彬, 等. 水下爆炸对高桩码头毁伤效应的试验研究 [J]. 爆炸与冲击, 2020, 40(11): 105–11408. DOI: 10.11883/bzycj-2019-0467.LIU J H, TANG T, WEI Z B, et al. Experimental research in damage effects of high-piled wharf under underwater explosion [J]. Explosion and Shock Waves, 2020, 40(11): 105–114. DOI: 10.11883/bzycj-2019-0467. [18] ZHAO H N, ZHAO X H, FANG H Y, et al. Experimental investigation of steel fiber reinforced concrete slabs subjected to underwater contact explosions [J]. Ocean Engineering, 2023, 281: 114664. DOI: 10.1016/j.oceaneng.2023.114664. [19] 贺铭, 张阿漫, 刘云龙. 近场水下爆炸气泡与双层破口结构的相互作用 [J]. 爆炸与冲击, 2020, 40(11): 111402. DOI: 10.11883/bzycj-2020-0110.HE M, ZHANG A M, LIU Y L. Interaction of the underwater explosion bubbles and nearby double-layer structures with circular holes [J]. Explosion and Shock Waves, 2020, 40(11): 111402. DOI: 10.11883/bzycj-2020-0110. [20] YANG G D, WANG G H, LU W B, et al. Cross-section shape effects on anti-knock performance of RC columns subjected to air and underwater explosions [J]. Ocean Engineering, 2019, 181: 252–266. DOI: 10.1016/j.oceaneng.2019.04.031. [21] WEN Y B, CHI H, LAI Z C, et al. Experimental and numerical investigation on saturated concrete subjected to underwater contact explosion [J]. Construction and Building Materials, 2023, 384: 131465. DOI: 10.1016/j.conbuildmat.2023.131465. [22] LIU Z P, LI H, LI L. Anti-explosion performance analysis of marine interdiction system based on ALE method [J]. Heliyon, 2023, 9(3): e13873. DOI: 10.1016/j.heliyon.2023.e13873. [23] HERNANDEZ C, MARANON A, ASHCROFT I A, et al. A computational determination of the Cowper-Symonds parameters from a single Taylor test [J]. Applied Mathematical Modelling, 2013, 37(7): 4698–4708. DOI: 10.1016/j.apm.2012.10.010. [24] YUAN S J, HAO H, ZONG Z H, et al. A study of RC bridge columns under contact explosion [J]. International Journal of Impact Engineering, 2017, 109: 378–390. DOI: 10.1016/j.ijimpeng.2017.07.017. [25] LSTC. LS-DYNA keyword user’s manual: VOLUME II material models [M]. Livermore: Livermore Software Technology Corporation, 2017. [26] WEBSTER K G. Investigation of close proximity underwater explosion effects on a Ship-Like structure using the multi-material Arbitrary Lagrangian Eulerian finite element method [D]. Blacksburg: Virginia Polytechnic Institute and State University, 2007: 223–233. [27] HAI L, REN X D. Computational investigation on damage of reinforced concrete slab subjected to underwater explosion [J]. Ocean Engineering, 2020, 195: 106671. DOI: 10.1016/j.oceaneng.2019.106671. [28] LEE E L, HORNIG H C, KURY J W. Adiabatic expansion of high explosive detonation products: UCRL-50422 [R]. Livermore: University of California Radiation Lab, 1968. DOI: 10.2172/4783904. [29] RIEDEL W, THOMA K, HIERMAIER S, et al. Penetration of reinforced concrete by BETA-B-500 numerical analysis using a new macroscopic concrete model for hydrocodes [C]//Proceedings of the 9th International Symposium on the Effects of Munitions with Structures. Berlin, 1999: 315–322. -







 下载:
下载: