二次爆炸作用下钢筋混凝土梁动力响应的数值模拟

张海鹏; 潘钻峰; 司豆豆

doi:10.11883/bzycj-2024-0021

二次爆炸作用下钢筋混凝土梁动力响应的数值模拟

doi: 10.11883/bzycj-2024-0021

1.
同济大学土木工程学院，上海 200092

基金项目: 国家自然科学基金项目（52078368）

详细信息

作者简介:
张海鹏（1992－　），男，博士研究生，zhpeng@tongji.edu.cn

通讯作者:
潘钻峰（1981－　），男，博士，教授，zfpan@tongji.edu.cn

中图分类号: O383
计量
- 文章访问数: 165
- HTML全文浏览量: 38
- PDF下载量: 88
- 被引次数: 0
出版历程
- 收稿日期: 2024-01-08
- 修回日期: 2024-05-25
- 网络出版日期: 2024-05-28

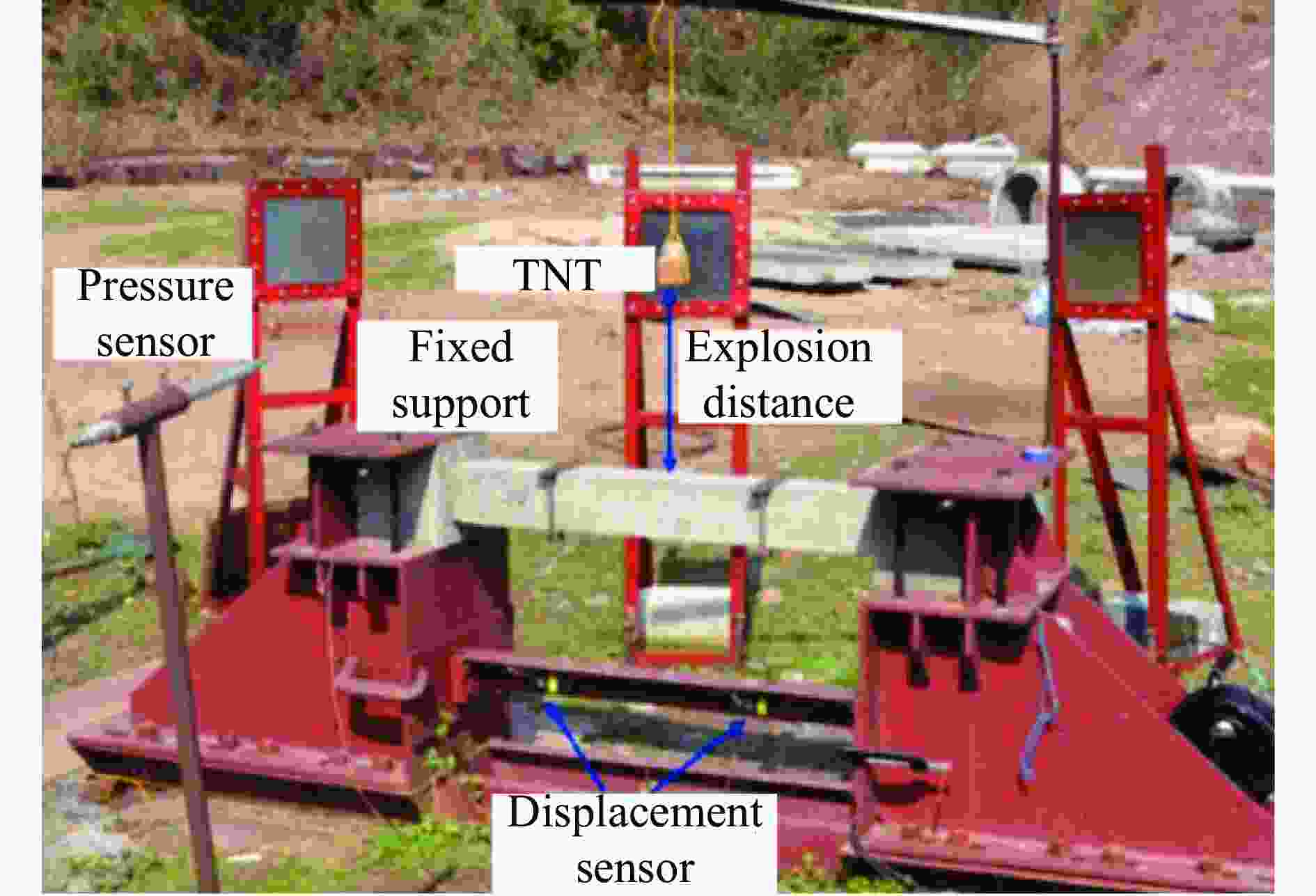
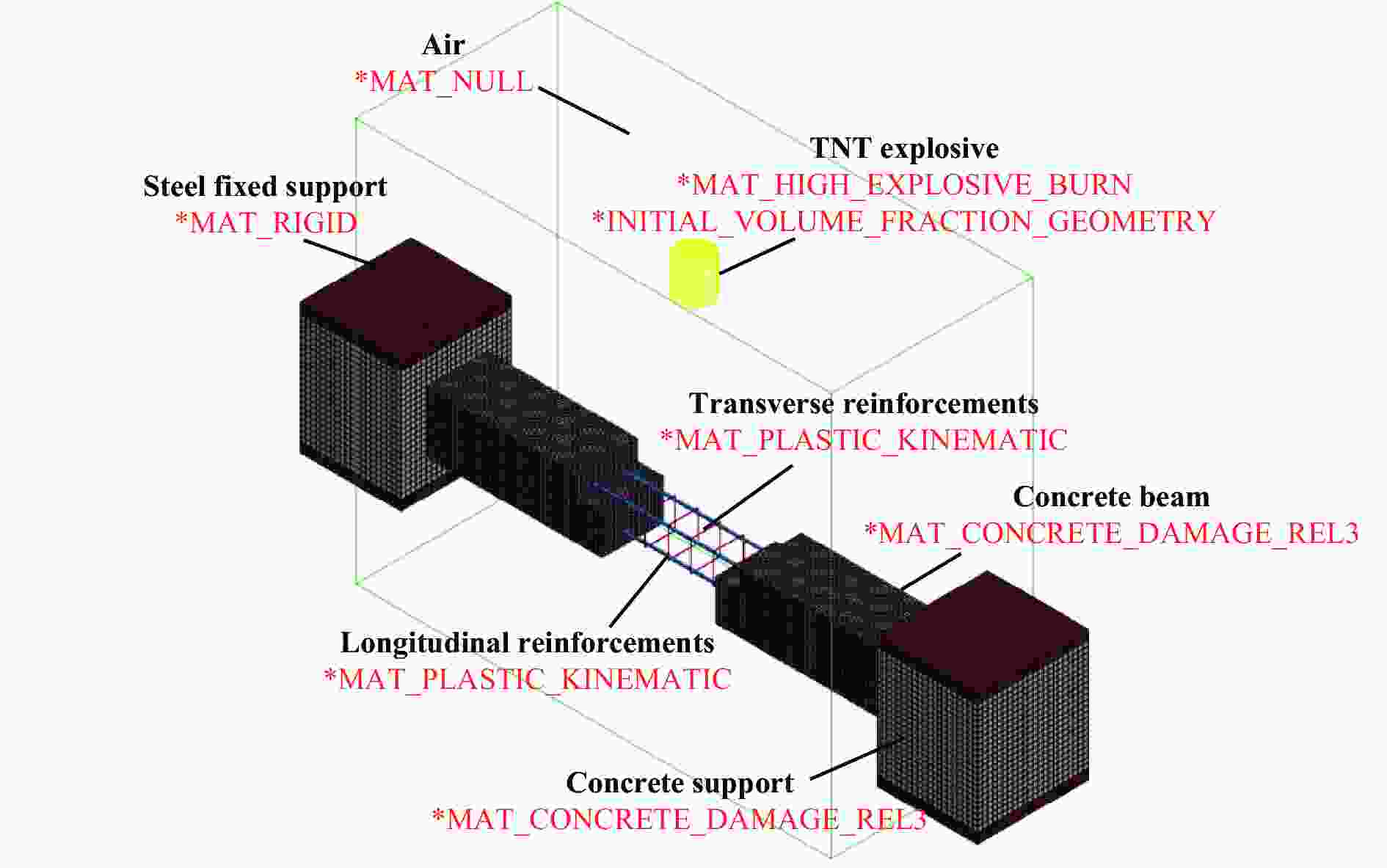
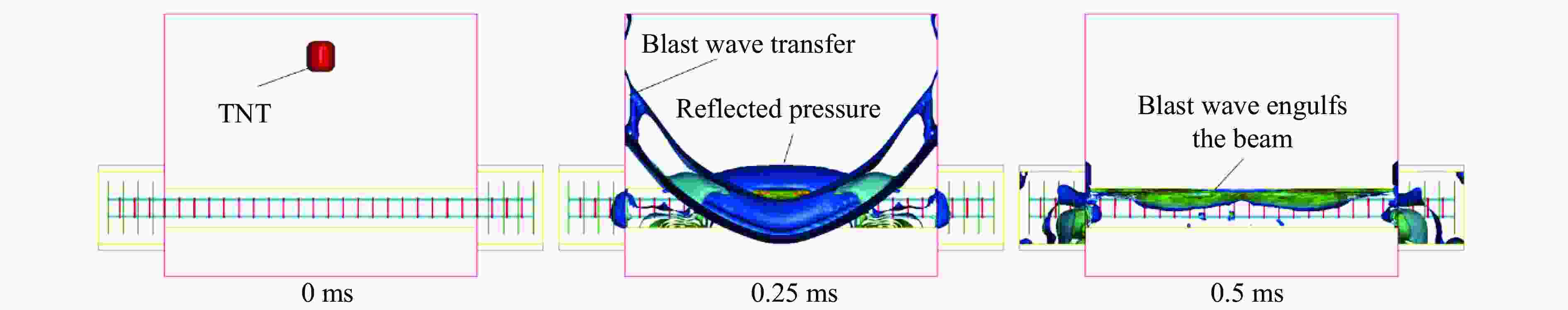
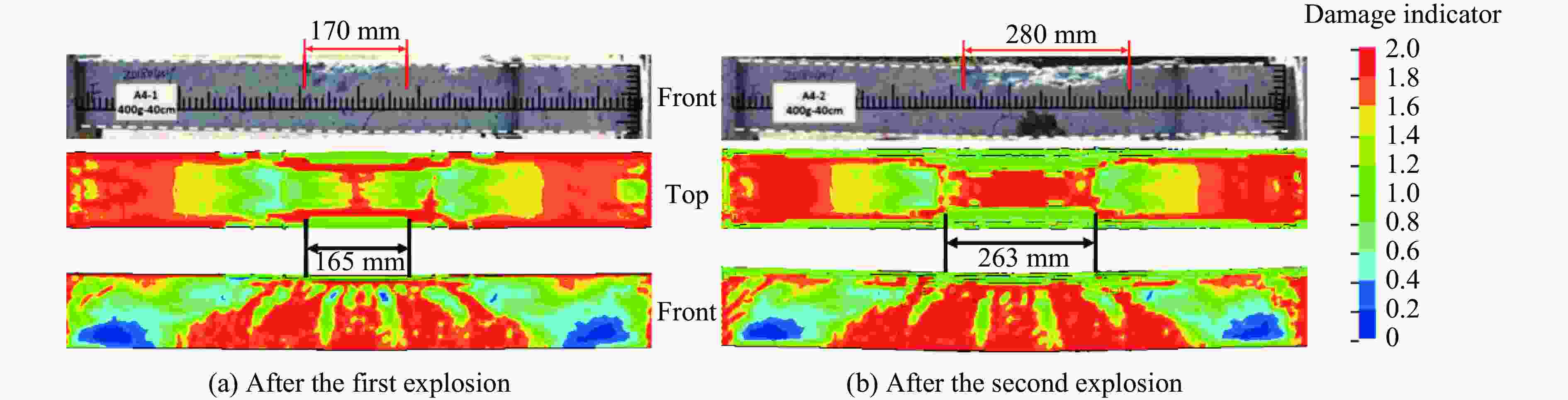
Simulation on reinforced concrete beam subjected to secondary explosion

1.
College of Civil Engineering, Tongji University, Shanghai 200092, China

摘要

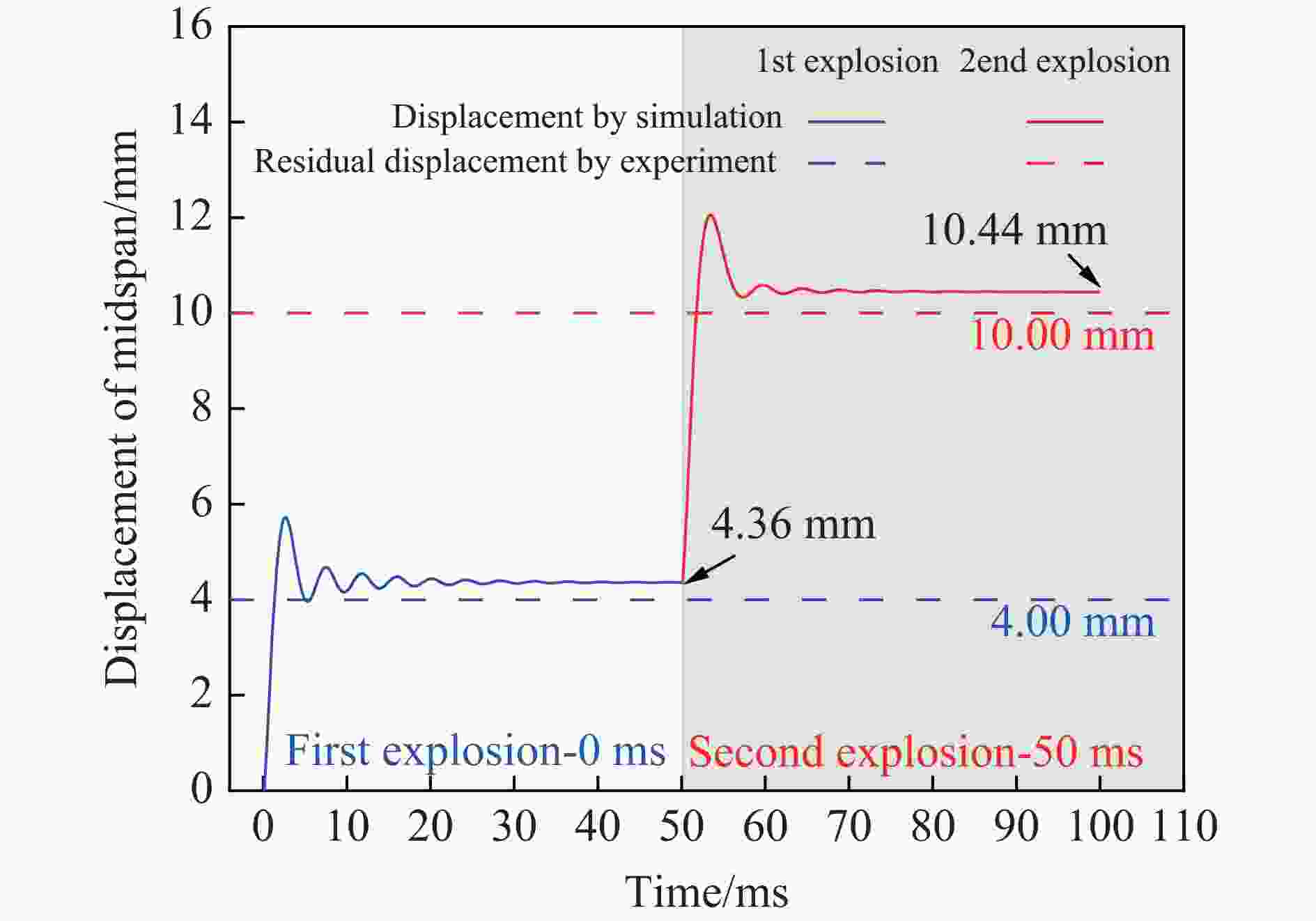
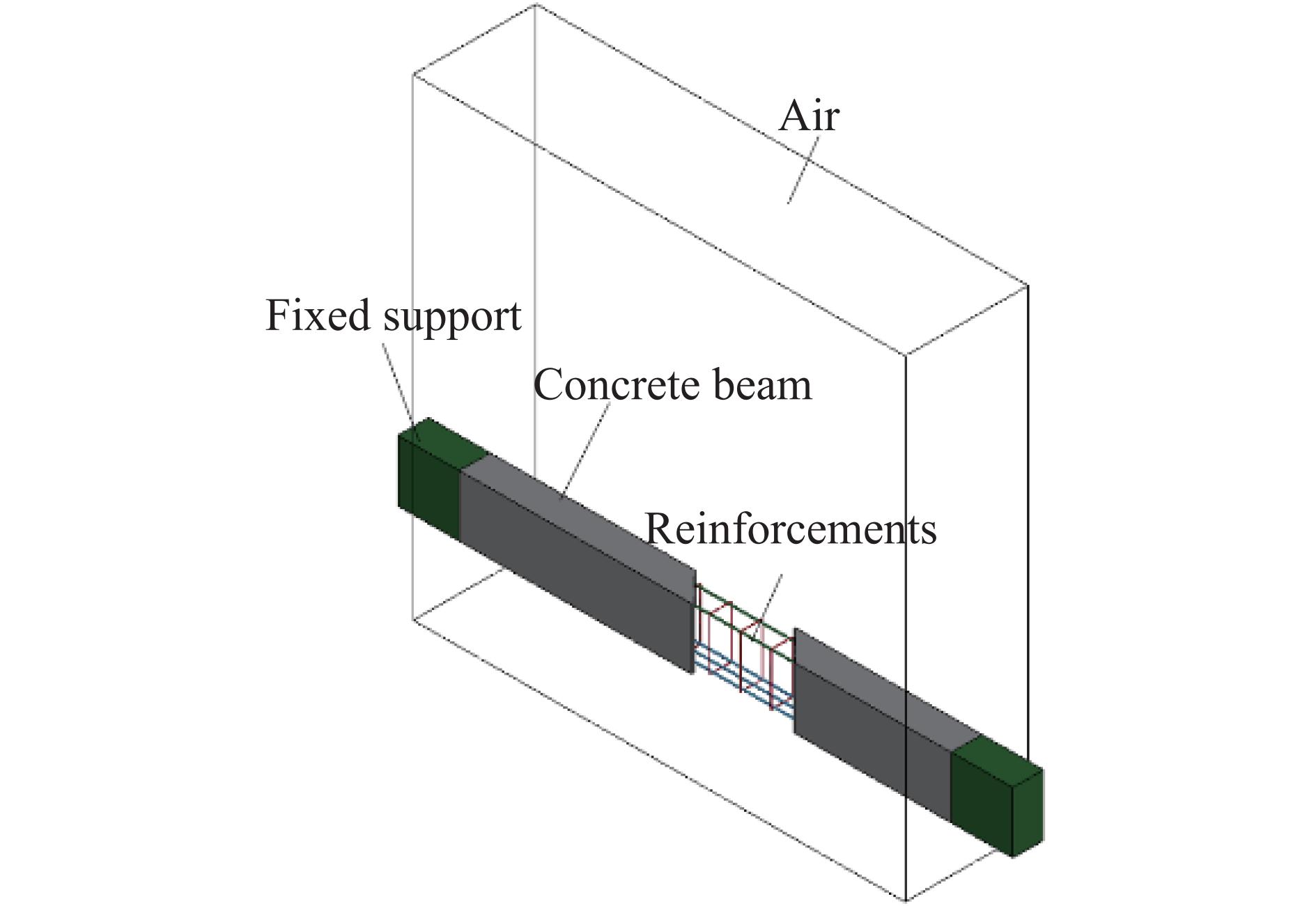
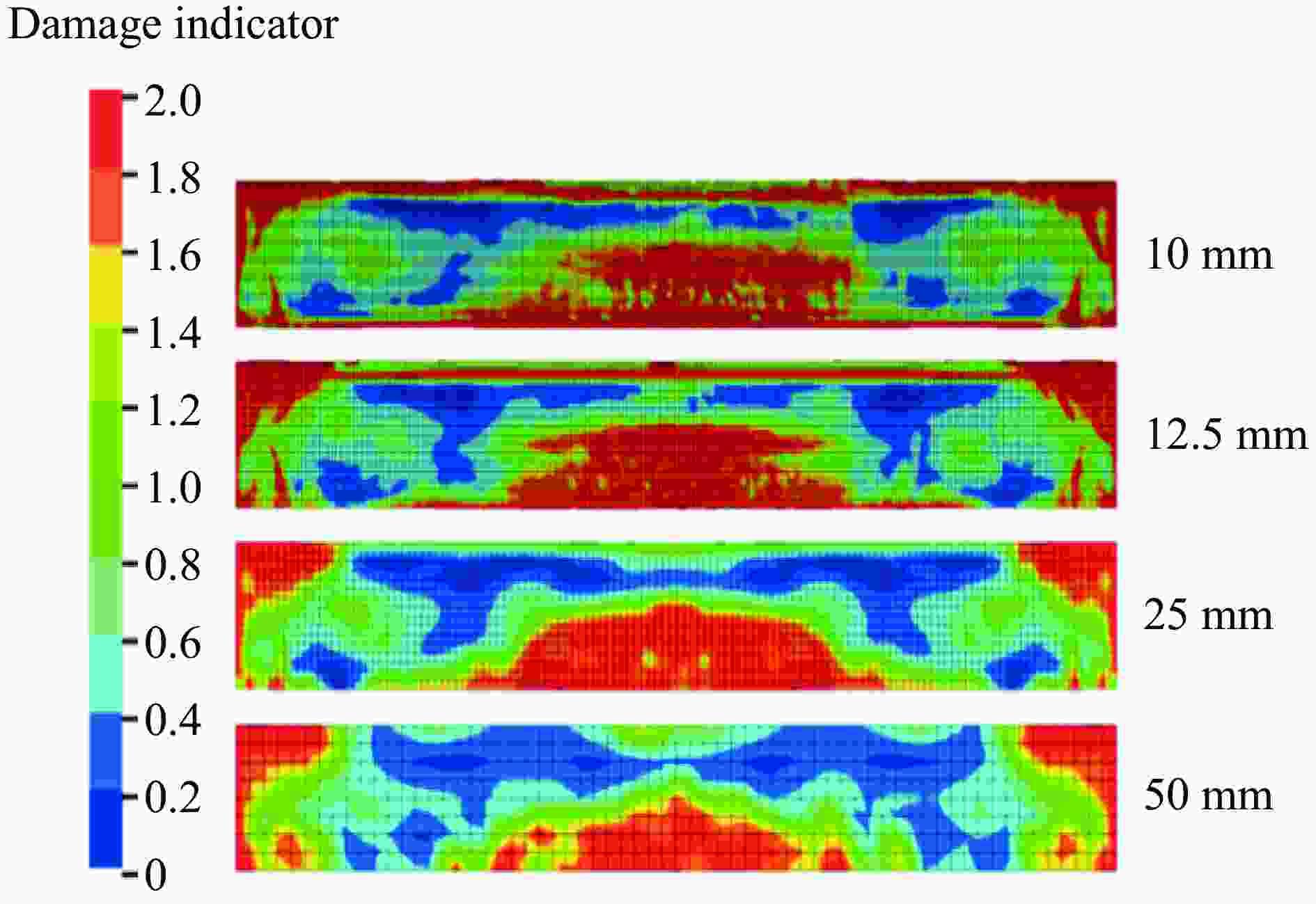
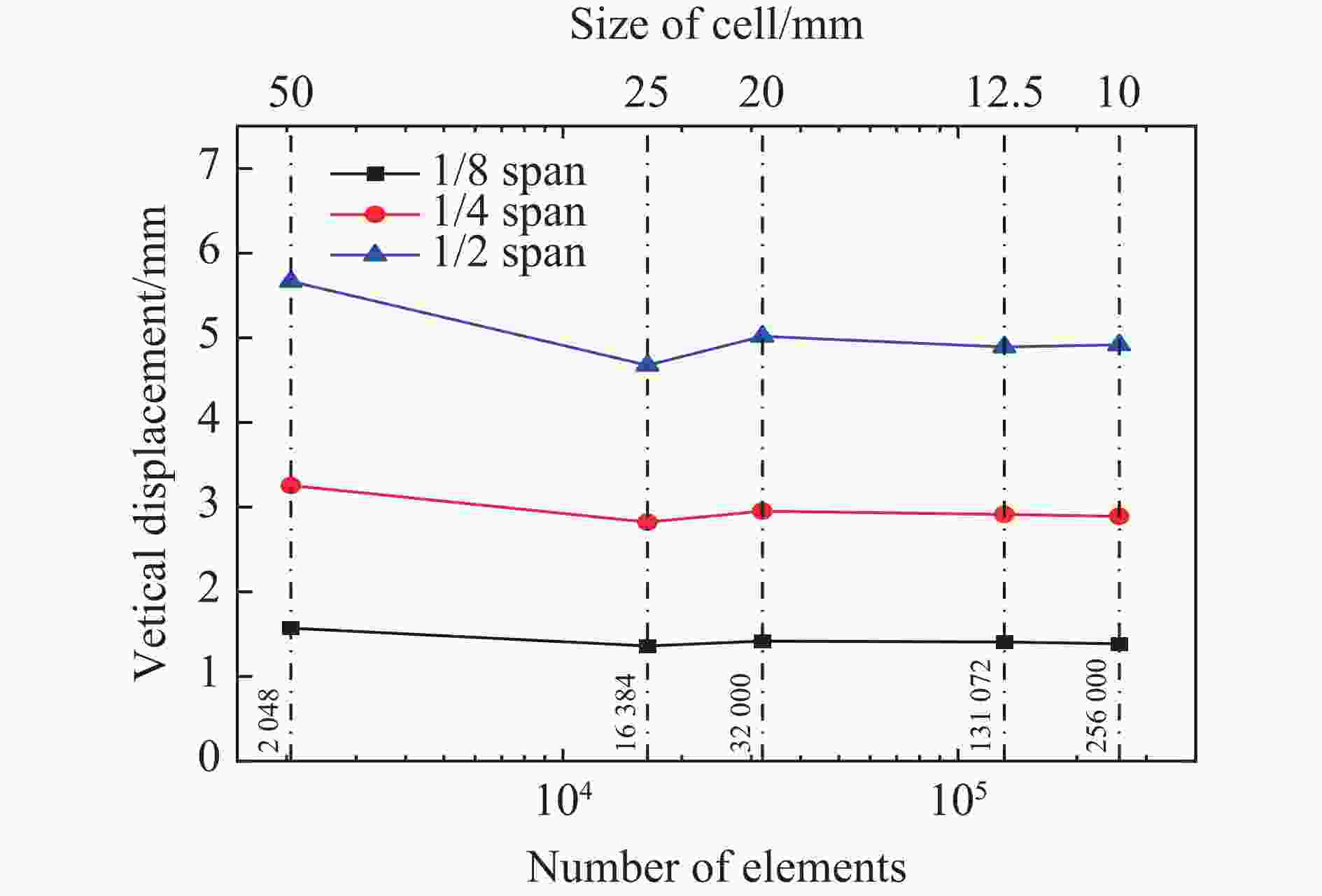
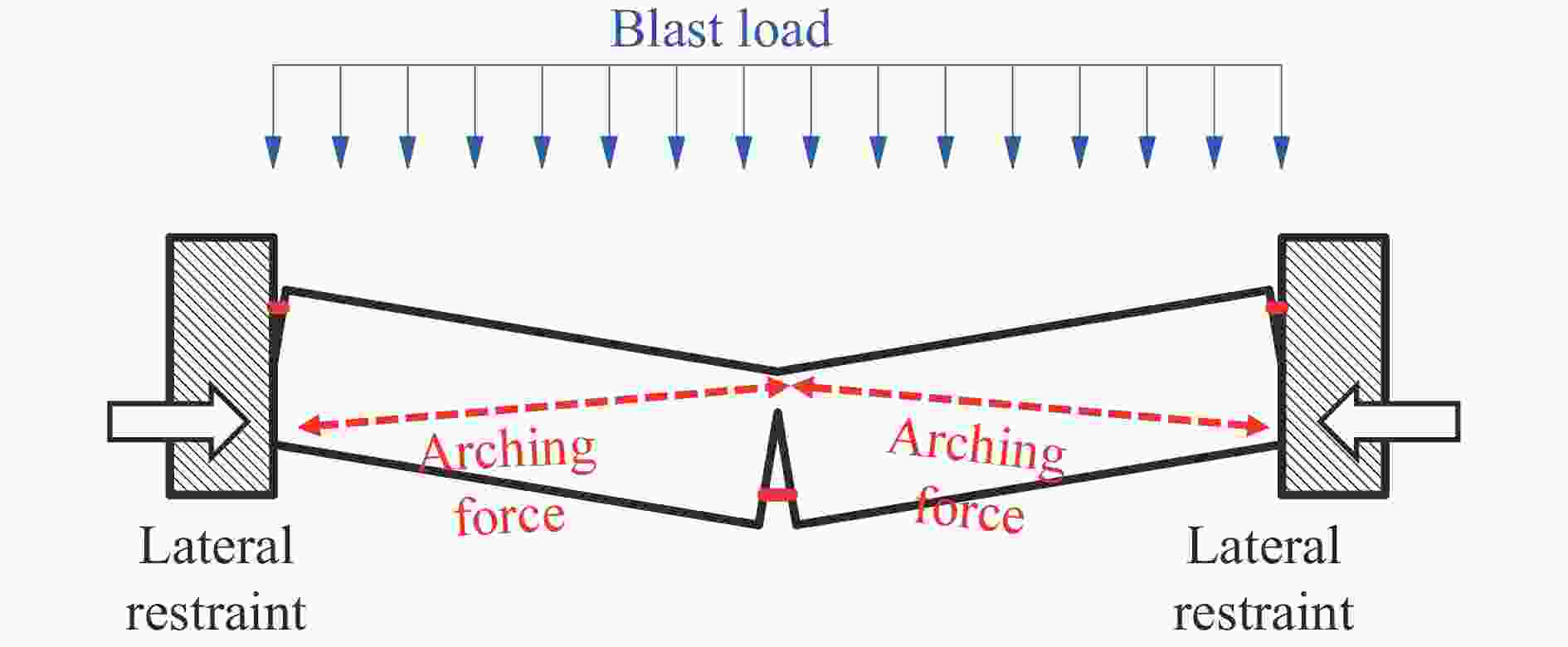
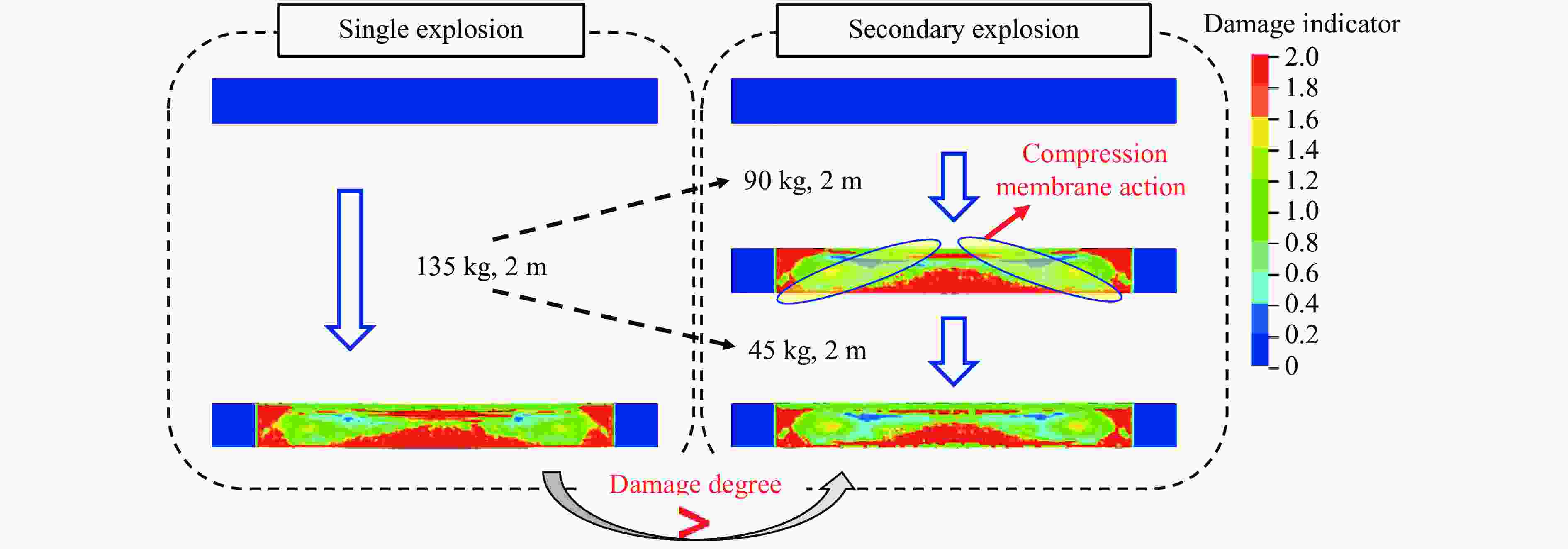
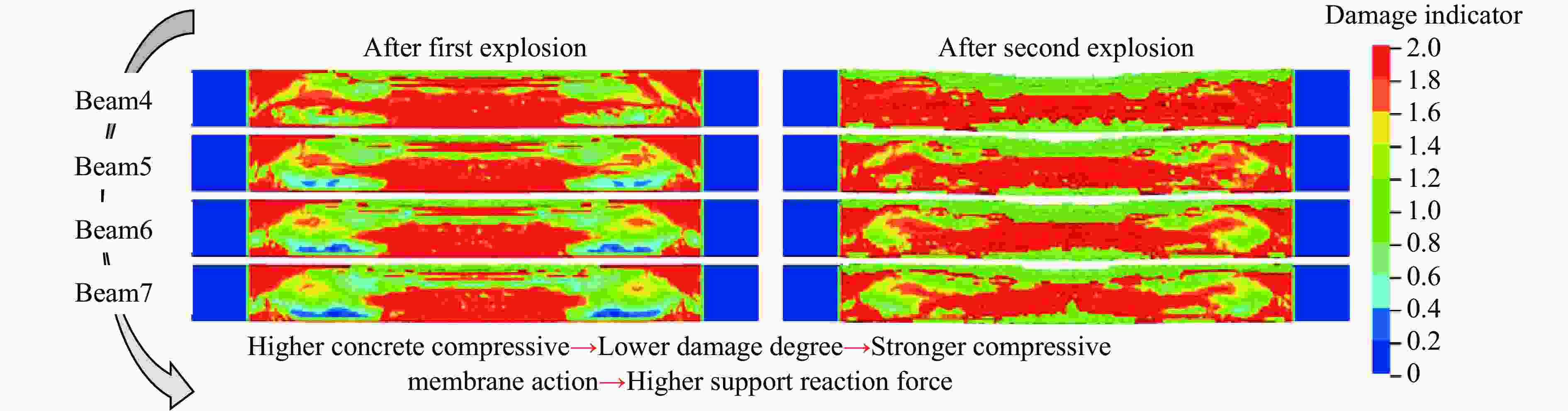
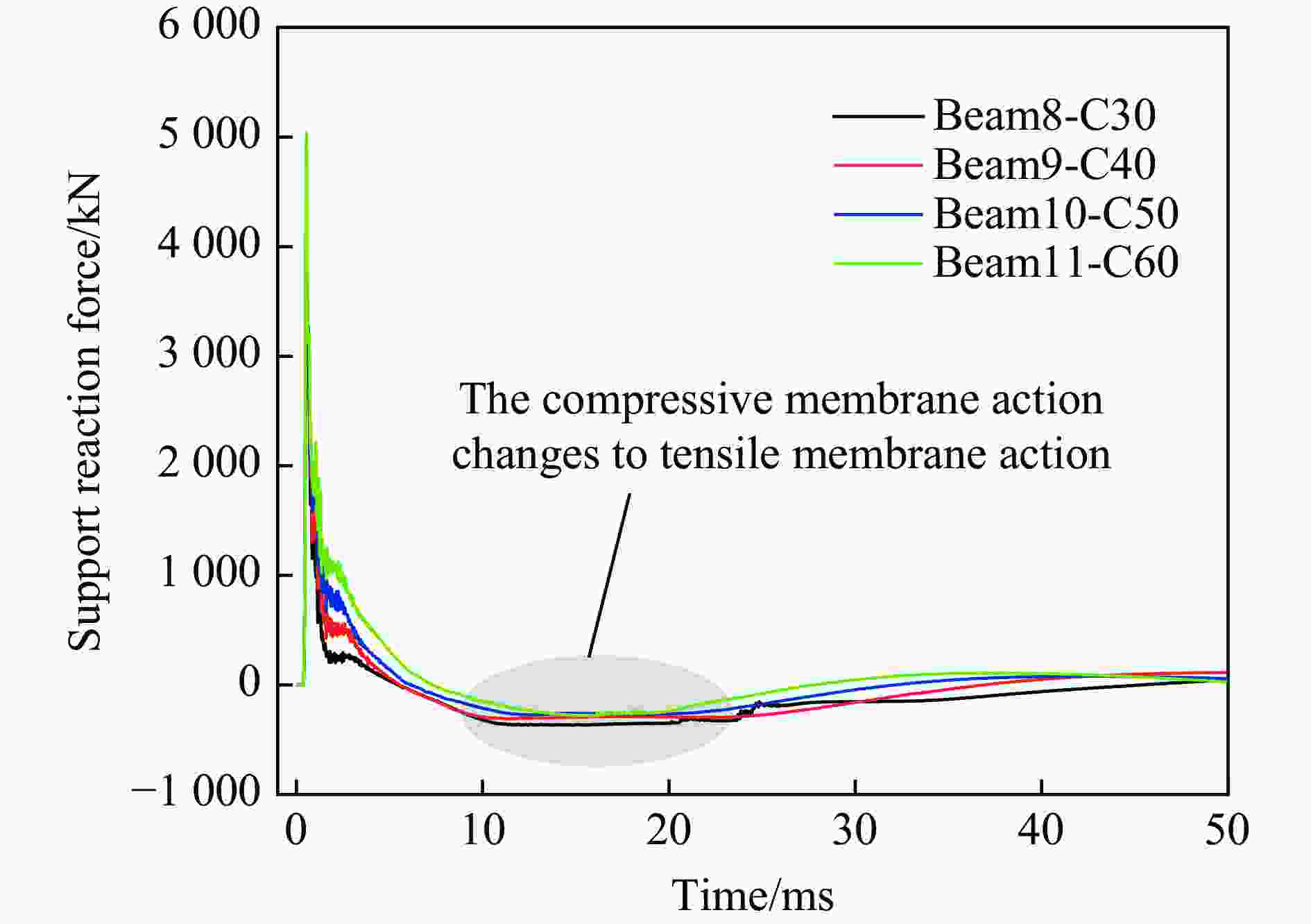
摘要: 为了探究钢筋混凝土梁在二次爆炸作用下的毁伤效应，开展了相关数值分析研究：利用LS-DYNA中的流固耦合算法和完全重启动技术，对钢筋混凝土梁二次爆炸试验进行了数值模拟，分析结果与试验结果基本一致，验证了模拟方法和材料模型参数的正确性；在此基础上，对二次爆炸场景进行扩展，对典型足尺钢筋混凝土梁进行建模分析，探究了爆炸场景、混凝土强度、纵筋配筋率和箍筋配筋率对二次爆炸作用下钢筋混凝土梁损伤破坏模式和动力响应的影响。结果表明：由于压力膜效应的存在，在保持爆炸总当量不变的前提下，单次爆炸对钢筋混凝土梁构件造成的损伤比连续两次爆炸造成的累积损伤更严重；提高混凝土强度可以显著提高二次爆炸作用下钢筋混凝土梁的抗爆性能；提高纵筋配筋率对梁抗爆性能的提升效果不明显；而对混凝土梁支座部位采用箍筋加密措施可以降低钢筋混凝土梁在爆炸作用下的剪切破坏程度，提高钢筋混凝土梁在二次爆炸作用下的抗爆性能。进一步计算得到了所涉及二次爆炸场景下两种不同设计参数钢筋混凝土梁的等损伤曲线，建立了相应的损伤程度分区图。
- 钢筋混凝土 /
- 爆炸荷载 /
- 二次爆炸 /
- 数值模型 /
- 流固耦合 /
- 动力响应
Abstract: Terrorist attacks and local wars occur frequently, which makes the risk of buildings subjected to multiple explosions increasing. Most of the existing research focuses on the single explosion scenario, and there are few studies on the damage effect of reinforced concrete structures under multiple explosions. In order to study the damage effect of reinforcement concrete beams under secondary explosion and offset the shortcomings of the existing research, relevant numerical analysis was carried out. The damage parameters of K&C concrete constitutive model were modified firstly. And the arbitrary Lagrangian-Eulerian method for FSI (fluid-structure interaction) was used to simulate the secondary explosion experiment of reinforced concrete beam with the full restart function of LS-DYNA. The numerical analysis results were well consistent with the test results, verifying the effectiveness of the simulation method and the modified constitutive model. On this basis, the secondary explosion simulation conditions were expanded. The effects of various parameters, including scaled distance, concrete compressive strength, longitudinal reinforcement ratio and transverse reinforcement details, on the damage effect of typical size reinforcement concrete beams under secondary explosion were further analyzed. The results show that due to the compressive membrane action of reinforcement concrete beam, keeping the total equivalent TNT weight of the explosion unchanged, the damage of RC component caused by one single explosion is more serious than the cumulative damage caused by two successive explosions. The concrete compressive strength has a more significant effect on the blast resistance performance of RC beams under secondary explosion, the higher the concrete strength, the lower the damage degree of the beam under the secondary explosion. Increasing the longitudinal reinforcement ratio has no obvious effect on improving the blast resistance performance of the beam and reducing the transverse reinforcement spacing can effectively decrease the shear failure degree of reinforcement concrete beam which makes the blast resistance performance of RC beams under secondary explosion and near explosion improved. The iso-damage curves of reinforcement concrete beams with two different design parameters are further calculated and the corresponding damage degree zoning maps are established.
- reinforced concrete /
- blast load /
- secondary explosion /
- numerical model /
- fluid-structure interaction /
- dynamic response

HTML全文

图 1 K&C默认损伤曲线与修正后损伤曲线对比

Figure 1. Comparison of damage function between the K&C model default and modified parameters

下载: 全尺寸图片幻灯片

图 2 文献[23]中二次爆炸试验场地布置

Figure 2. Sketch of the field test in Ref. [23]

下载: 全尺寸图片幻灯片

图 3 有限元模型

Figure 3. The FE model

下载: 全尺寸图片幻灯片

图 4 爆炸波等压面

Figure 4. The isobaric surface of the explosion wave

下载: 全尺寸图片幻灯片

图 5 损伤云图

Figure 5. The damage contours

下载: 全尺寸图片幻灯片

图 6 试验和有限元计算的得到梁跨中挠度时程曲线对比

Figure 6. Comparisons of deflection time history at mid-span between experiment and numerical simulation

下载: 全尺寸图片幻灯片

图 7 足尺梁有限元分析模型

Figure 7. The FE model of full-size RC beam

下载: 全尺寸图片幻灯片

图 8 不同网格尺寸混凝土的损伤

Figure 8. Damage calculated with different mesh size

下载: 全尺寸图片幻灯片

图 9 不同网格尺寸梁各位置处最大挠度

Figure 9. The deflection of beam with different mesh size

下载: 全尺寸图片幻灯片

图 10 FEMA 426中不同类型的爆炸场景^[24]

Figure 10. Different types of explosion scenarios in FEMA 426

下载: 全尺寸图片幻灯片

图 11 不同RC梁配筋构造

Figure 11. Reinforcement detailing of RC beam

下载: 全尺寸图片幻灯片

图 12 梁跨中位移时程曲线

Figure 12. Deflection time history at mid-span

下载: 全尺寸图片幻灯片

图 13 爆炸荷载作用下典型钢筋混凝土构件压力膜效应

Figure 13. Compression membrane action in a typical reinforced concrete member under blast loading

下载: 全尺寸图片幻灯片

图 14 总当量相同时单次爆炸作用和二次爆炸作用损伤云图对比

Figure 14. The damage contours of single explosion and secondary explosion under the same total TNT equivalent

下载: 全尺寸图片幻灯片

图 15 梁跨中位移时程曲线：Beam4-Beam7

Figure 15. Deflection time history at mid-span: Beam4-Beam7

下载: 全尺寸图片幻灯片

图 16 Beam4-Beam7支座反力时程曲线

Figure 16. Reaction force time history of Beam4-Beam7

下载: 全尺寸图片幻灯片

图 17 RC梁Beam4～Beam7在二次爆炸作用下的损伤云图

Figure 17. The damage contours of Beam4-Beam7 under secondary explosion

下载: 全尺寸图片幻灯片

图 18 比例距离为0.141 m/kg^1/3时RC梁Beam8～Beam11损伤云图

Figure 18. The damage contours of Beam8-Bema11 with scaled distance 0.141 m/kg^1/3

下载: 全尺寸图片幻灯片

图 19 梁跨中位移时程曲线：Beam8-Beam11

Figure 19. Deflection time history at mid-span: Beam8-Beam11

下载: 全尺寸图片幻灯片

图 20 Beam8-Beam11支座反力时程时程曲线

Figure 20. Reaction force time history of Beam8-Beam11

下载: 全尺寸图片幻灯片

图 21 梁跨中位移时程曲线：Beam4、Beam12、Beam13

Figure 21. Deflection time history at mid-span: Beam4, Beam12 and Beam13

下载: 全尺寸图片幻灯片

图 22 梁跨中位移时程曲线：Beam4、Beam14～Beam16

Figure 22. Deflection time history at mid-span: Beam4, Beam14-16

下载: 全尺寸图片幻灯片

图 23 Beam4，Beam14～Beam16损伤云图

Figure 23. The damage contours of Beam4, Beam14-Beam16

下载: 全尺寸图片幻灯片

图 24 Beam4，Beam14-Beam16支座反力时程曲线

Figure 24. Reaction force time history of Beam4, Beam14-Beam16 under secondary explosion

下载: 全尺寸图片幻灯片

图 25 梁跨中位移时程曲线：Beam8、Beam17、Beam18

Figure 25. Deflection time history at mid-span: Beam8, Beam17 and Beam18

下载: 全尺寸图片幻灯片

图 26 比例距离为0.141m/kg^1/3时RC梁Beam8、Beam17、Beam18损伤云图

Figure 26. The damage contours of Beam8, Bema17 and Beam18 with scaled distance 0.141m/kg^1/

下载: 全尺寸图片幻灯片

图 27 不同损伤程度阈值下钢筋混凝土梁二次爆炸等损伤曲线

Figure 27. The iso-damage curves of RC beams under secondary explosion with different damage levels

下载: 全尺寸图片幻灯片

图 28 二次爆炸作用下钢筋混凝土梁损伤程度分区图

Figure 28. Zoning map of the damage degree of the RC beams under secondary explosion

下载: 全尺寸图片幻灯片

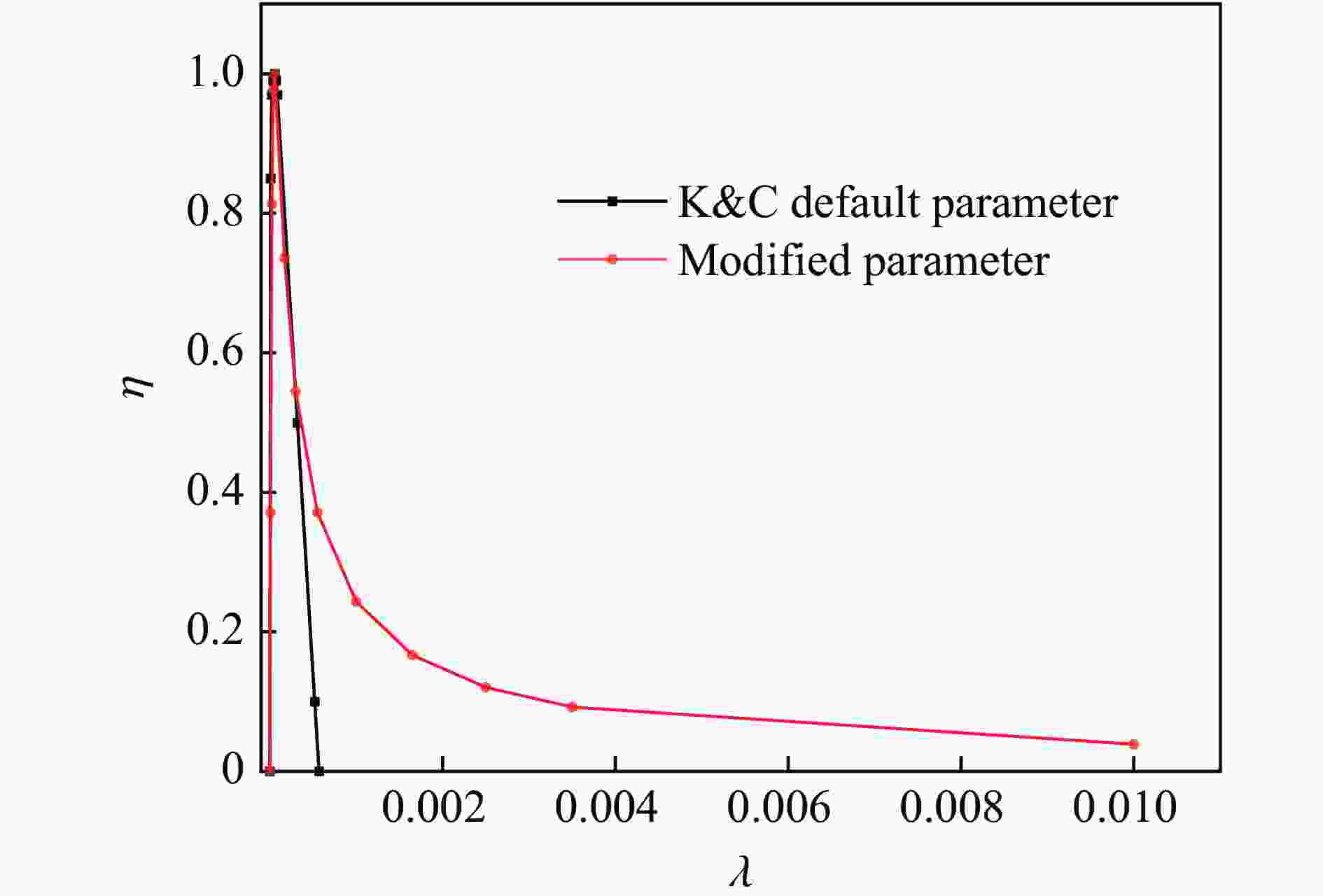
表 1 参数(η, λ)具体取值

Table 1. Damage parameters (η, λ) of K&C model

上升段		下降段
λ	η	λ	η
0	0	1.70×10⁻⁴	0.73617
8.00×10⁻⁶	0.37026	3.00×10⁻⁴	0.54456
2.40×10⁻⁵	0.81341	5.50×10⁻⁴	0.37119
4.00×10⁻⁵	0.97668	1.00×10⁻³	0.24346
5.60×10⁻⁵	1.00000	1.65×10⁻³	0.16694
		2.50×10⁻³	0.12059
		3.50×10⁻³	0.09209
		1.00×10⁻²	0.03876

下载: 导出CSV

表 2 MAT_HIGH_EXPLOSIVE_BURN材料模型及EOS_JWL状态方程输入参数

Table 2. Input parameters for MAT_HIGH_EXPLOSIVE_BURN and EOS_JWL

密度/ (kg·m⁻³)	爆速/(m·s)	爆压/Pa	A/Pa	B/Pa	R₁	R₂	ω	E₀/Pa	初始相对体积
1630	6930	2.1×10¹⁰	3.712×10¹¹	3.23×10⁹	4.15	0.95	0.32	7×10⁹	1

下载: 导出CSV

表 3 MAT_NULL材料模型及EOS_LINEAR_POLYNOMIAL状态方程输入参数

Table 3. Input parameters for MAT_NULL and EOS_LINEAR_POLYNOMIAL

密度/(kg·m⁻³)	粘滞系数/(Pa·s)	C₀, C₁, C₂, C_3, C₆	C₄, C₅	E₀/Pa	初始相对体积
1.29	2×10⁻⁵	0	0.4	2.5×10⁵	1

下载: 导出CSV

表 4 足尺钢筋混凝土梁设计参数及爆炸工况

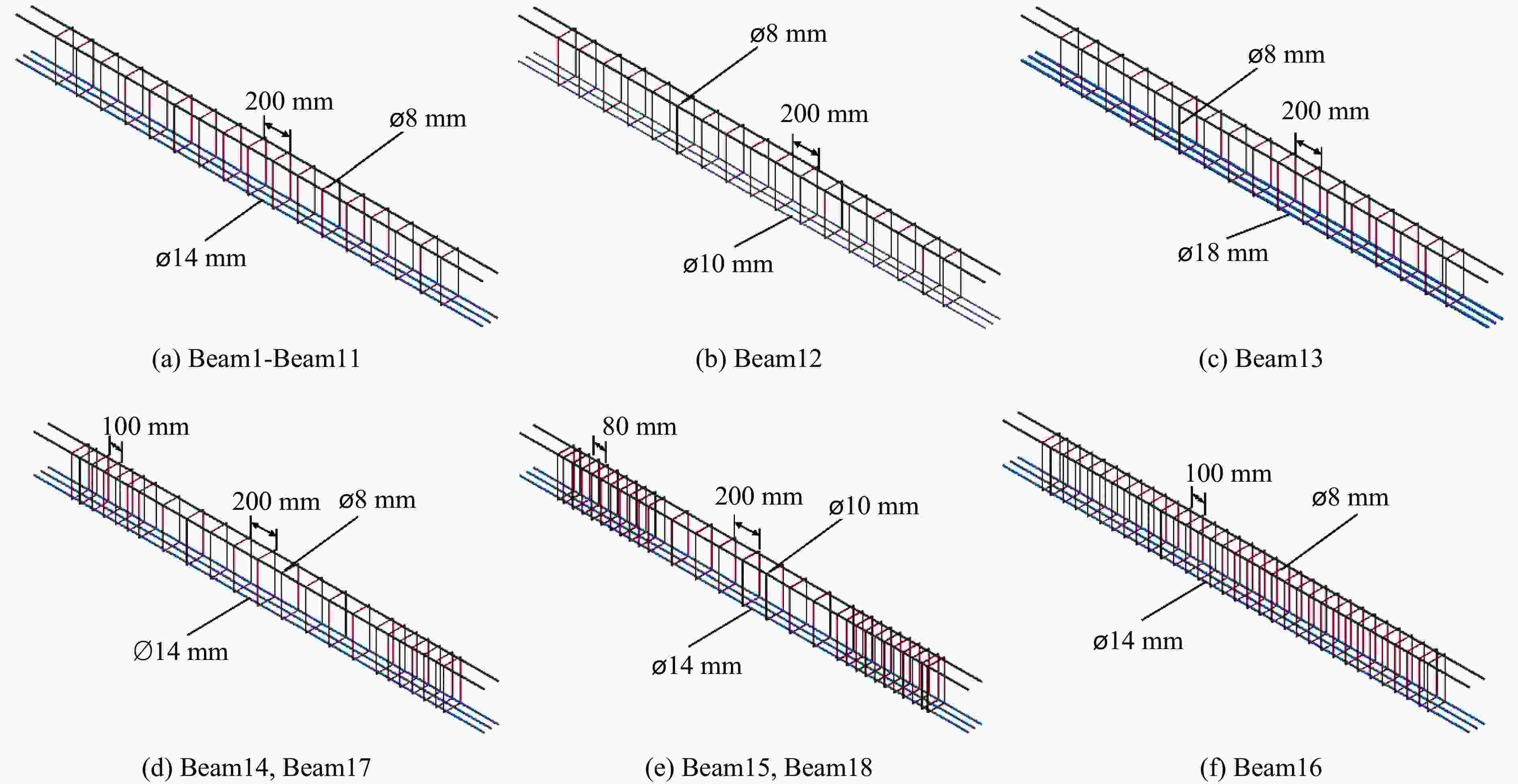
Table 4. The details of the full-size RC beams and the explosive scenarios

编号	(装药量/kg, 爆距/m)		混凝土强度等级	纵筋	箍筋
编号	第1次	第2次	混凝土强度等级	纵筋	箍筋
Beam1	(45, 2)	(45, 2)	C30	314	8@200mm
Beam2	(90, 2)	(45, 2)	C30	314	8@200mm
Beam3	(135, 2)	(45, 2)	C30	314	8@200mm
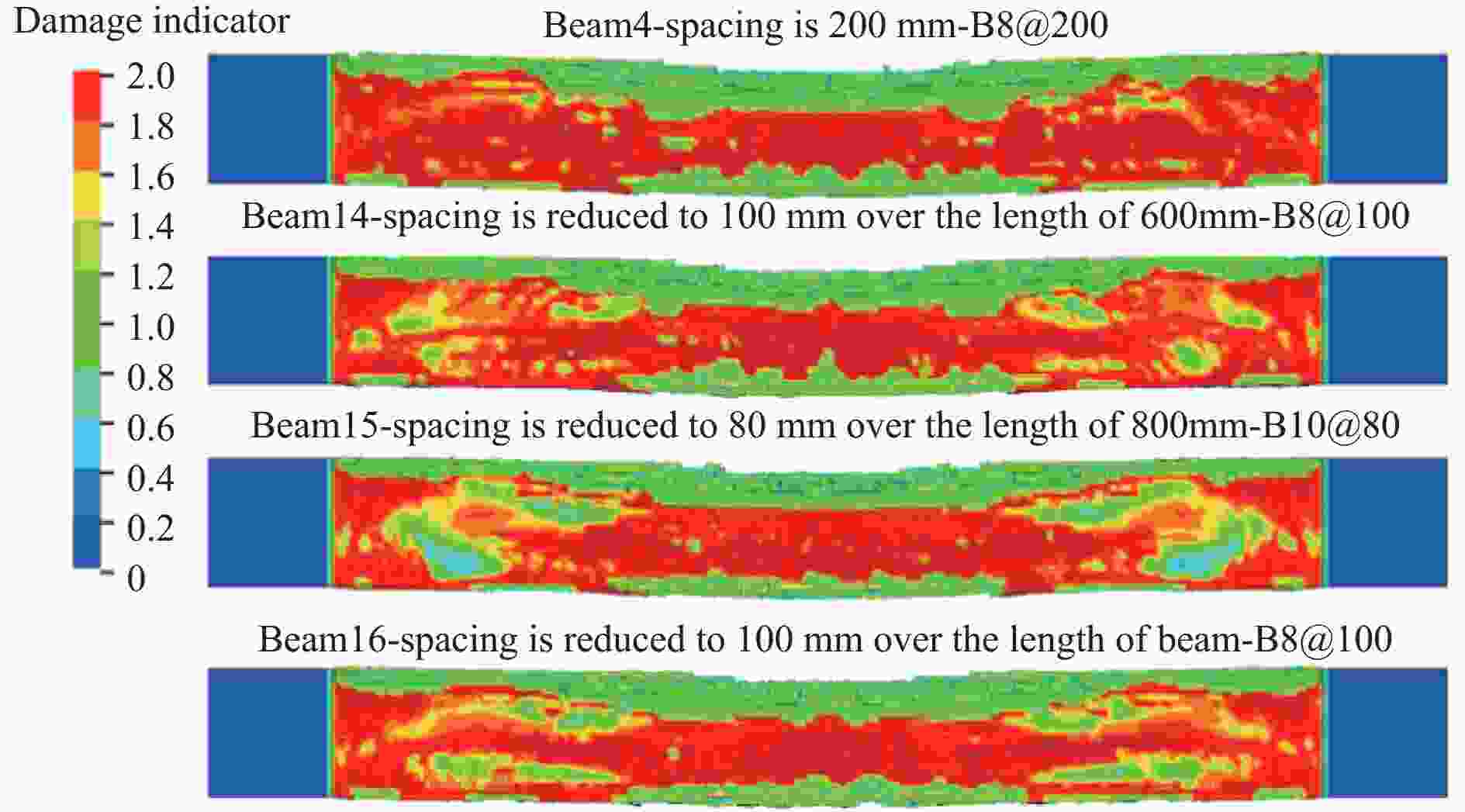
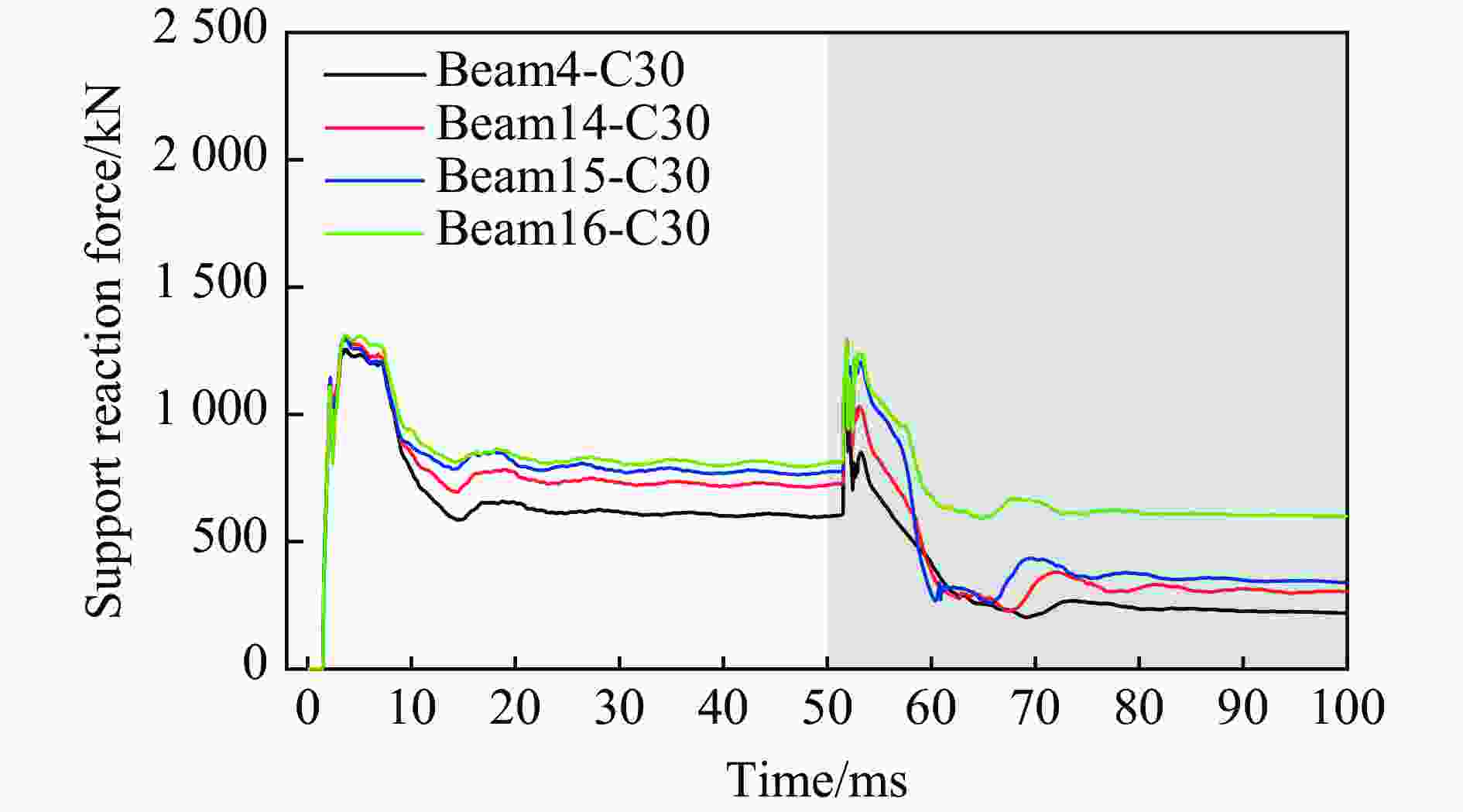
Beam4	(45, 1)	(45, 1)	C30	314	8@200mm
Beam5	(45, 1)	(45, 1)	C40	314	8@200mm
Beam6	(45, 1)	(45, 1)	C50	314	8@200mm
Beam7	(45, 1)	(45, 1)	C60	314	8@200mm
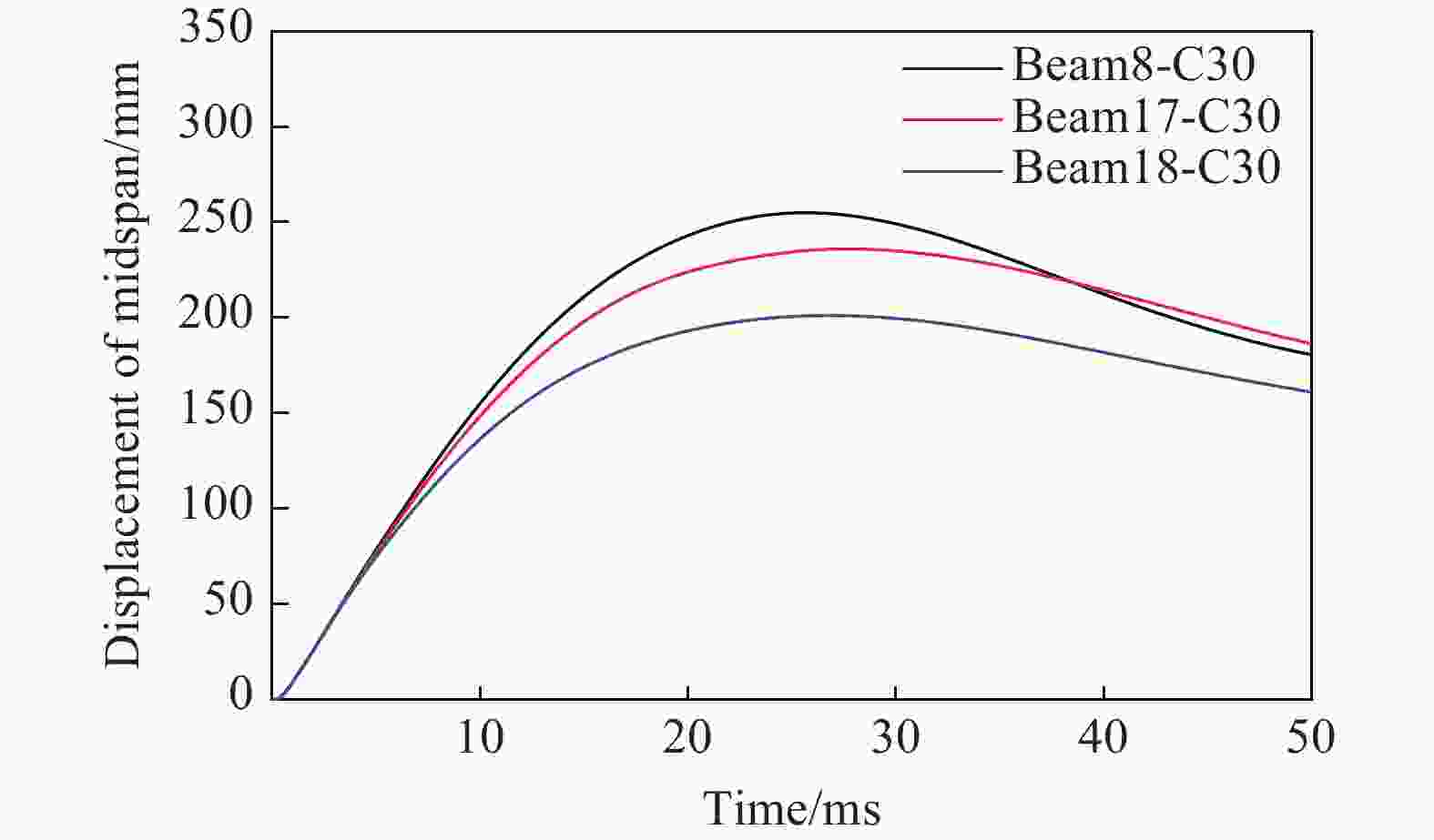
Beam8	(45, 0.5)	−	C30	314	8@200mm
Beam9	(45, 0.5)	−	C40	314	8@200mm
Beam10	(45, 0.5)	−	C50	314	8@200mm
Beam11	(45, 0.5)	−	C60	314	8@200mm
Beam12	(45, 1)	(45, 1)	C30	310	8@200mm
Beam13	(45, 1)	(45, 1)	C30	318	8@200mm
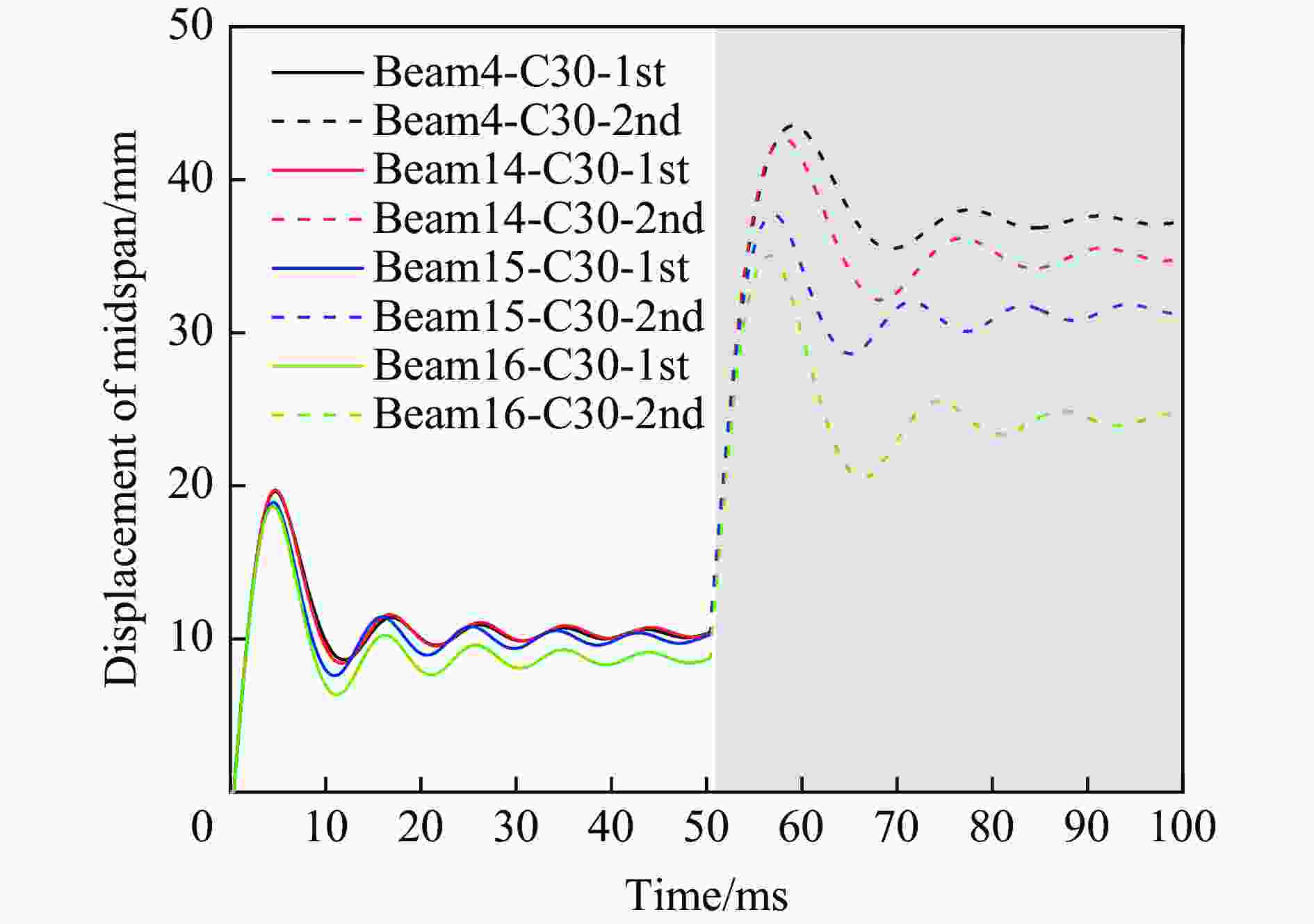
Beam14	(45, 1)	(45, 1)	C30	314	梁端箍筋加密8@100mm
Beam15	(45, 1)	(45, 1)	C30	314	梁端箍筋加密10@80mm
Beam16	(45, 1)	(45, 1)	C30	314	8@100mm
Beam17	(45, 0.5)	−	C30	314	梁端箍筋加密8@100mm
Beam18	(45, 0.5)	−	C30	314	梁端箍筋加密10@80mm

下载: 导出CSV

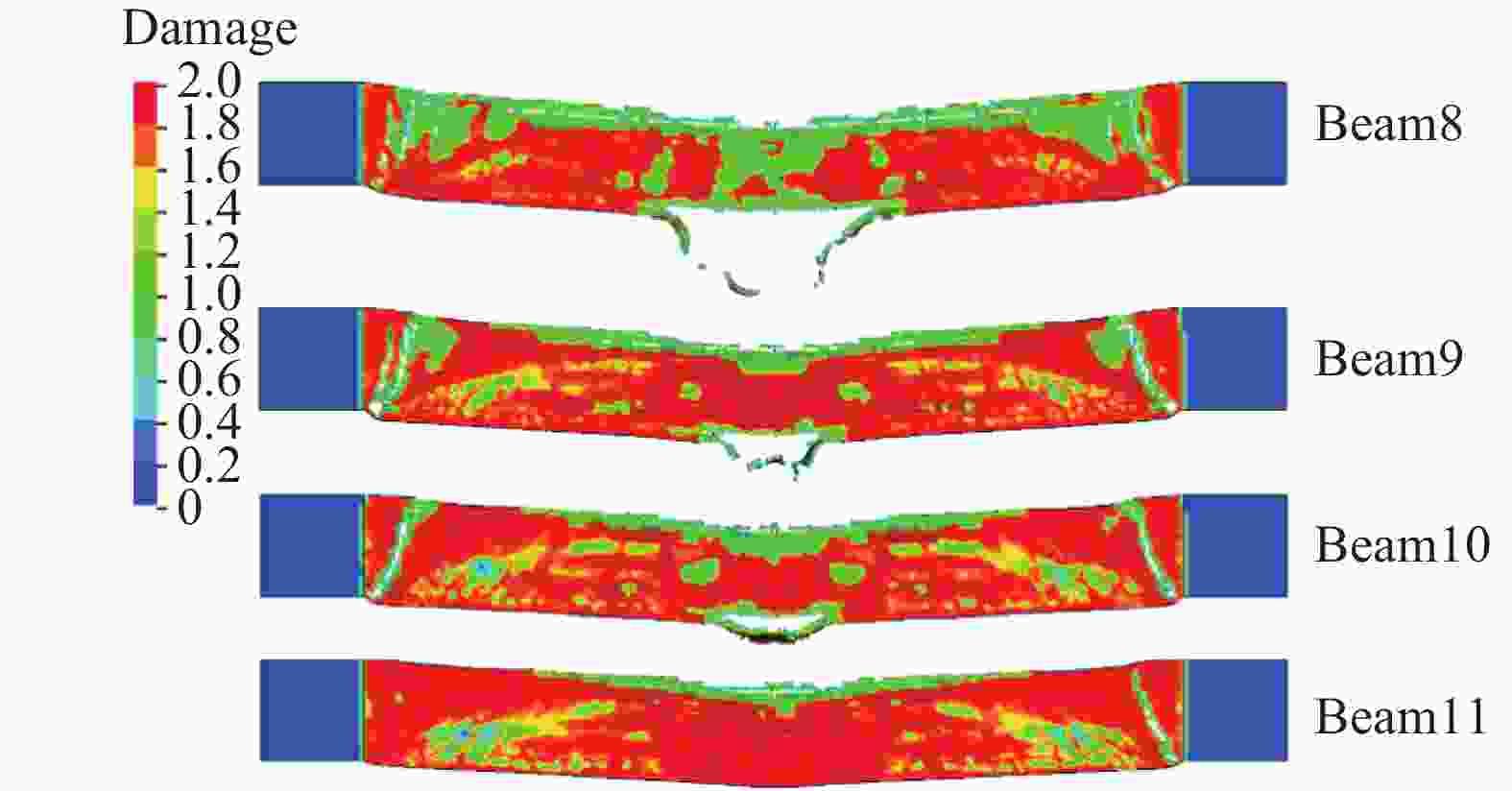
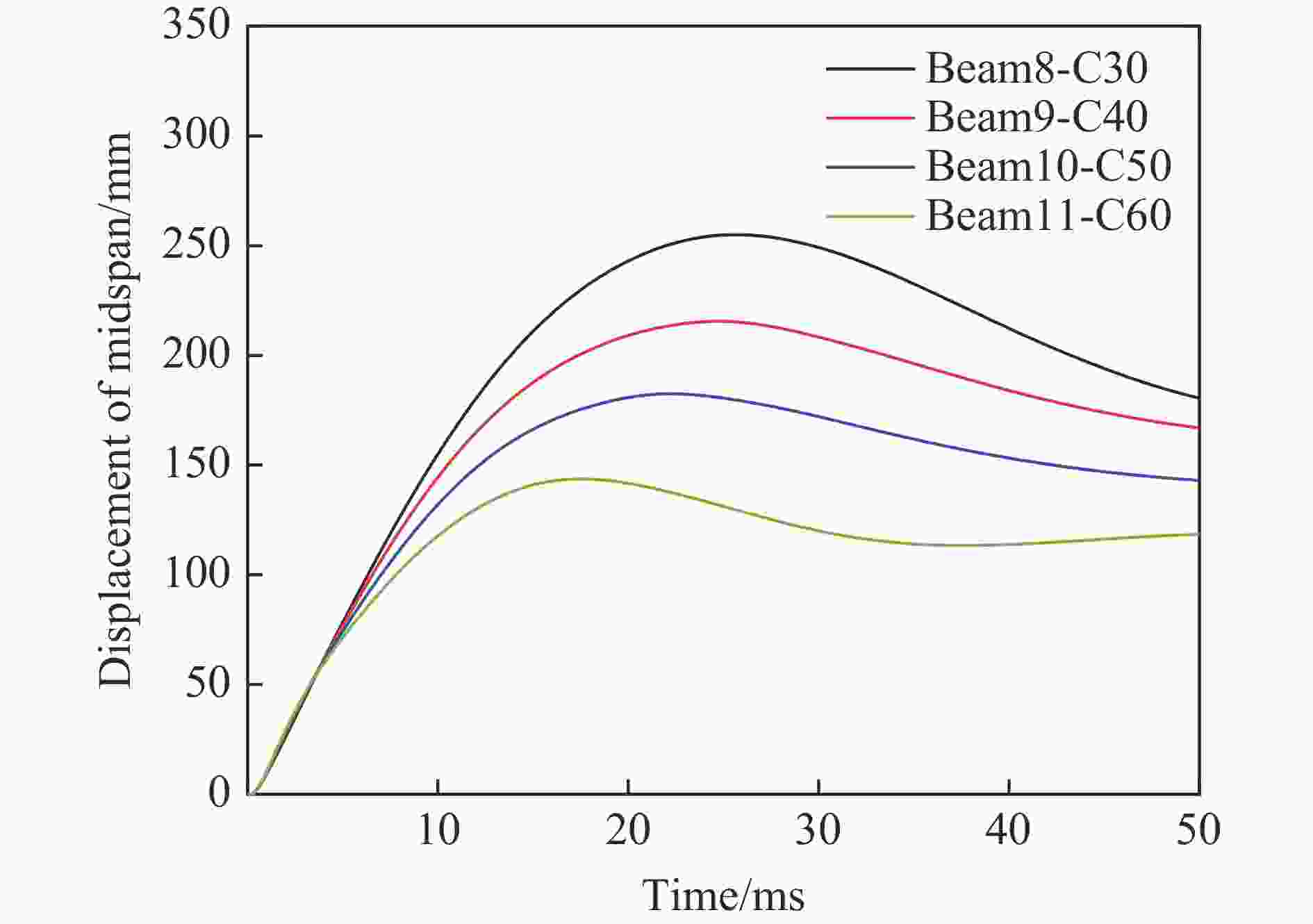
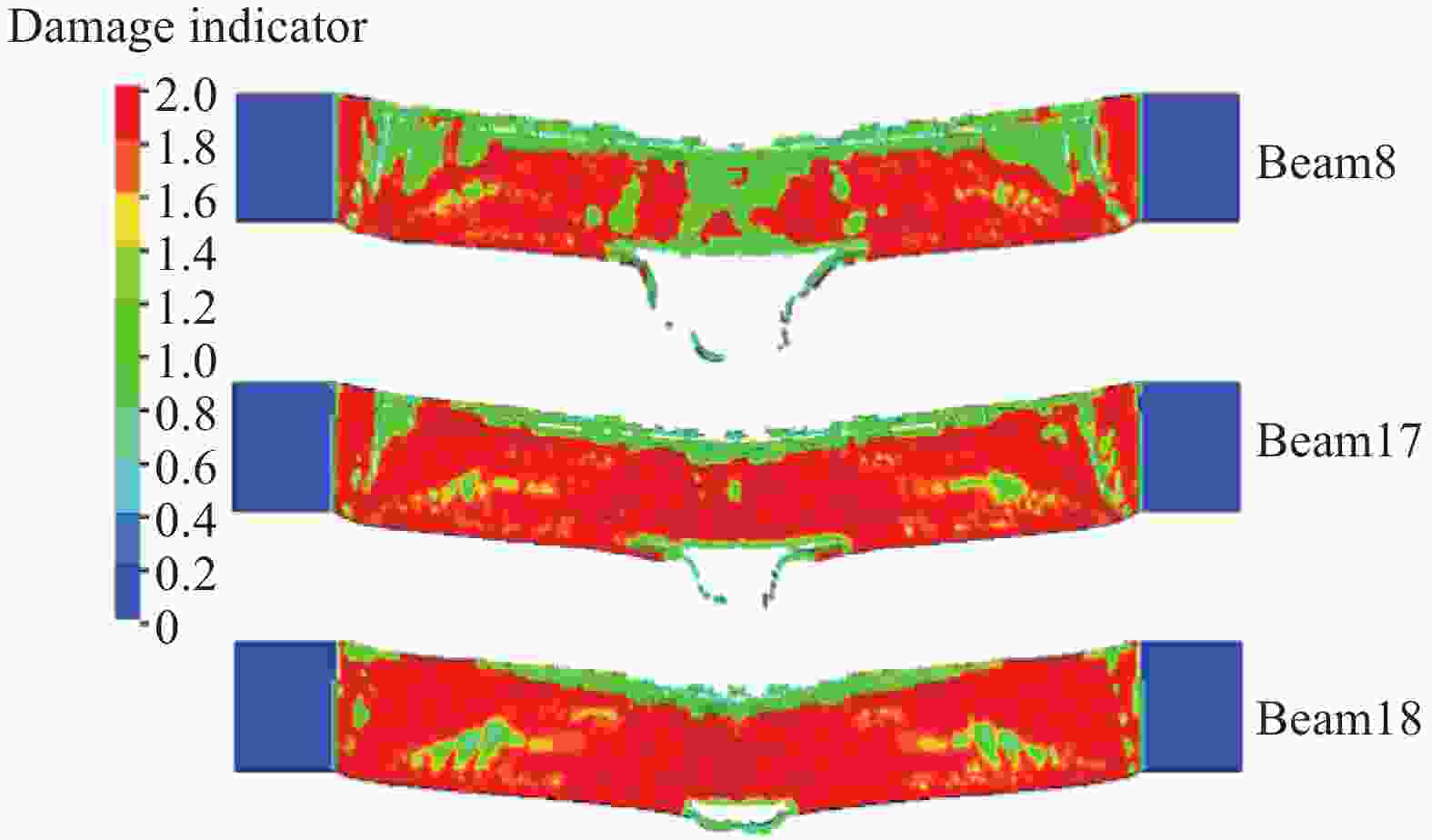
表 5 RC梁损伤的计算结果

Table 5. Numerical results of damage of beams

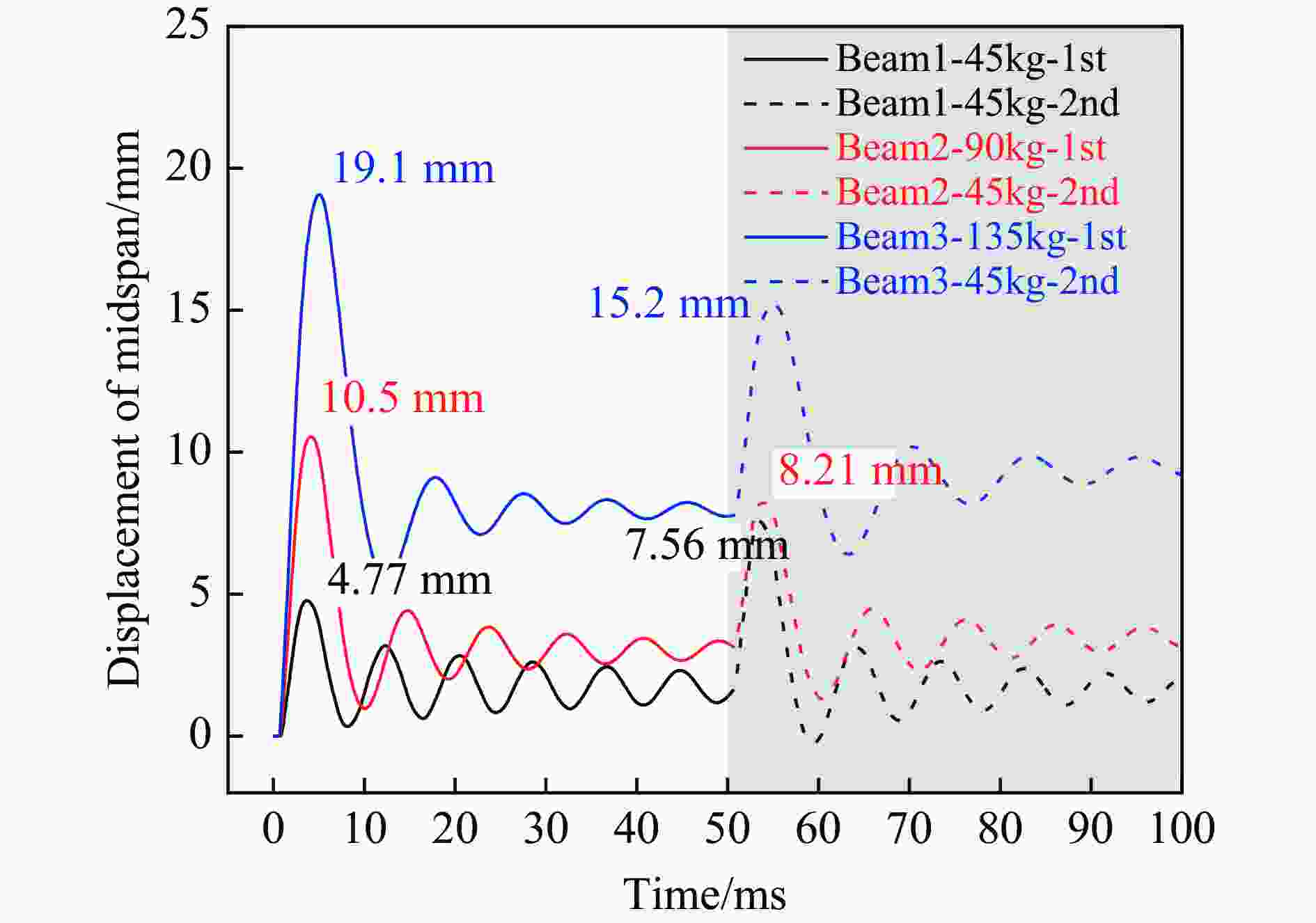
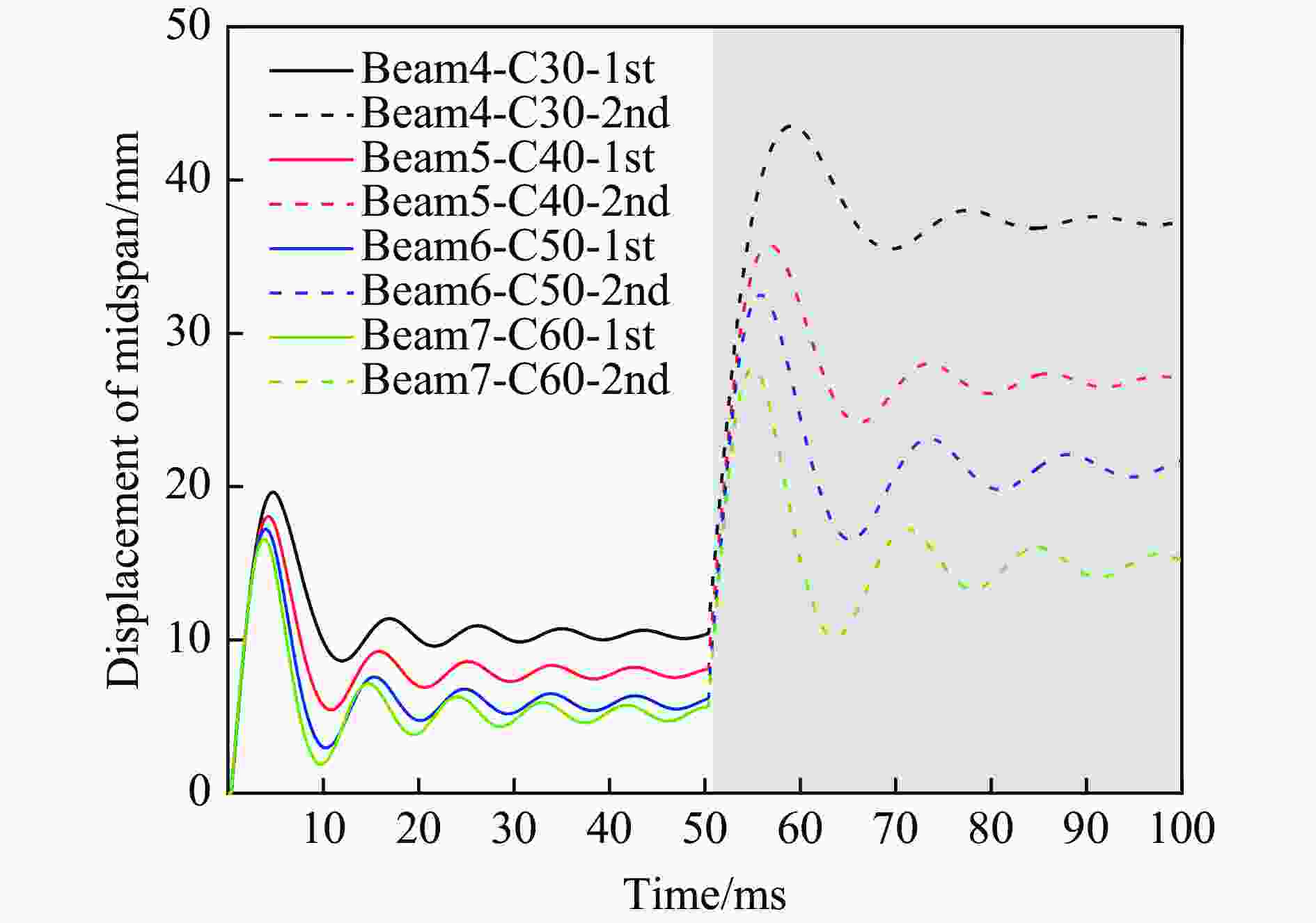
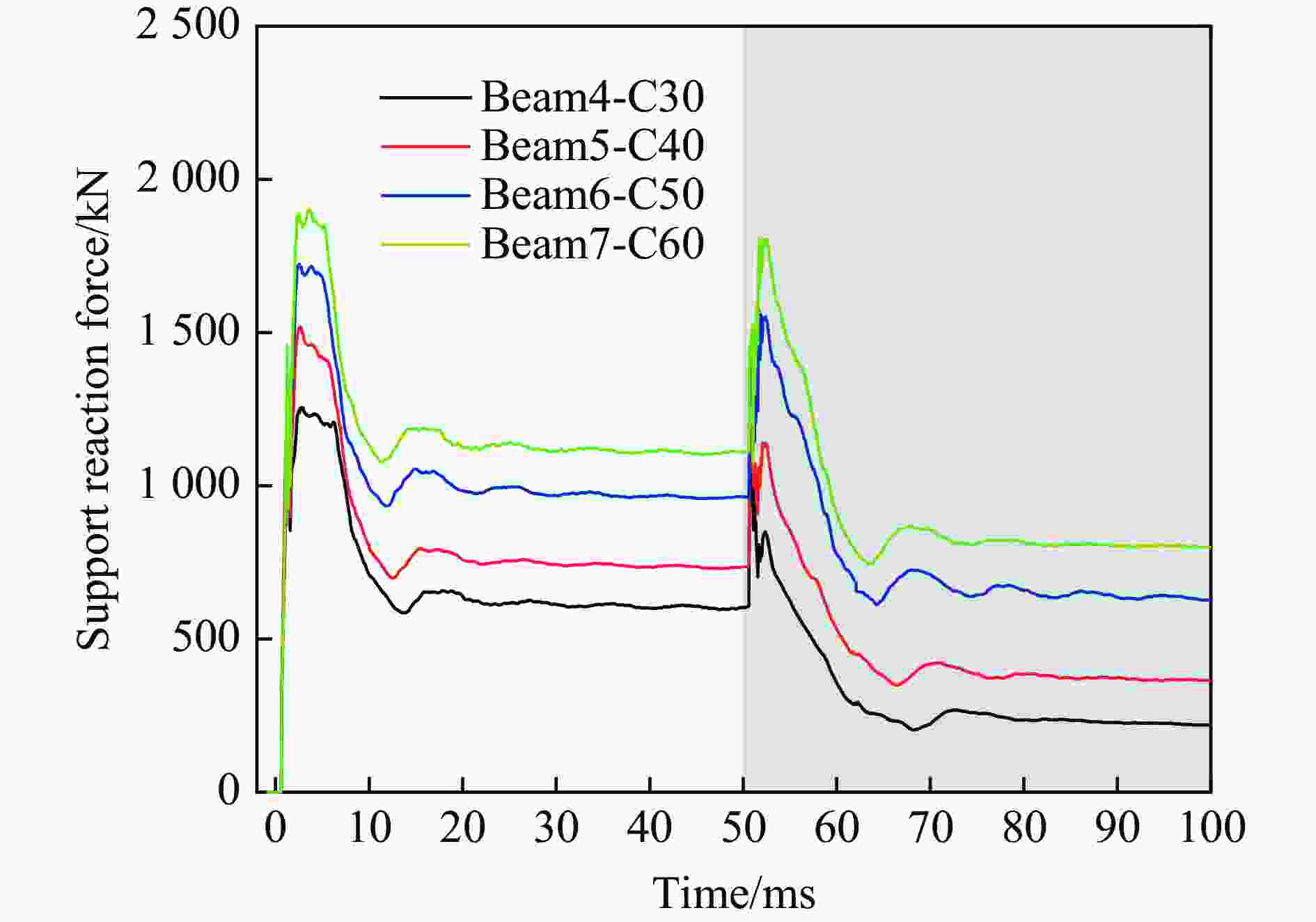
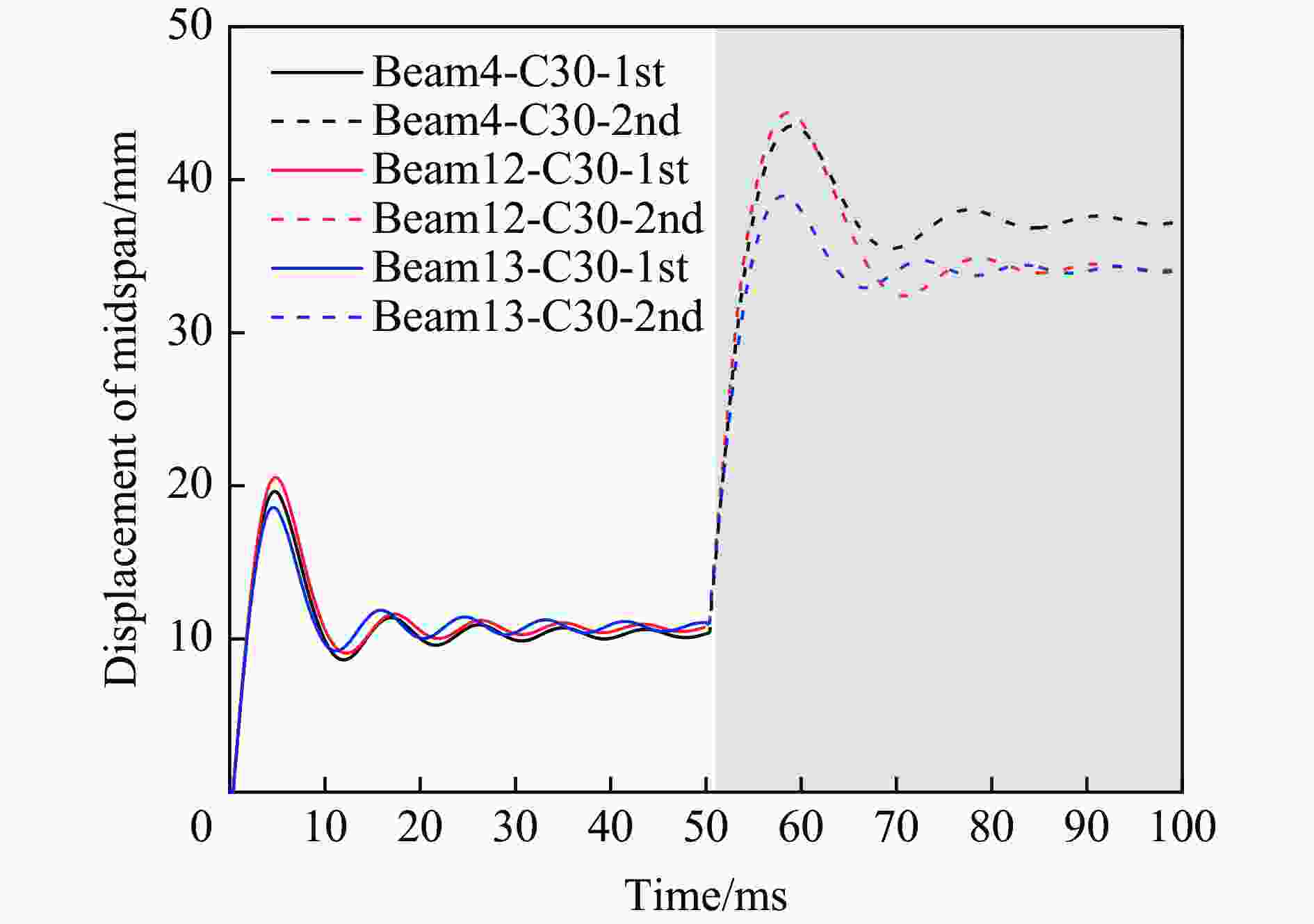
编号	爆次	X_m/mm	θ_max/(°)	损伤程度	编号	爆次	X_m/mm	θ_max/(°)	损伤程度
Beam1	二次	7.562	0.271	轻度损伤	Beam10	单次	182.493	6.507	完全破坏
Beam2	二次	8.214	0.294	轻度损伤	Beam11	单次	143.675	5.131	完全破坏
Beam3	二次	15.188	0.544	轻度损伤	Beam12	二次	44.363	1.588	中度损伤
Beam4	二次	43.522	1.558	中度损伤	Beam13	二次	38.934	1.394	中度损伤
Beam5	二次	35.765	1.281	中度损伤	Beam14	二次	42.670	1.528	中度损伤
Beam6	二次	32.488	1.163	中度损伤	Beam15	二次	37.709	1.350	中度损伤
Beam7	二次	27.713	0.992	轻度损伤	Beam16	二次	35.057	1.255	中度损伤
Beam8	单次	254.972	9.054	完全破坏	Beam17	单次	235.966	8.389	完全破坏
Beam9	单次	215.498	7.671	完全破坏	Beam18	单次	201.104	7.164	完全破坏

下载: 导出CSV

表 6 两种方法得到二次爆炸作用下梁的跨中最大位移和支座最大转角计算结果

Table 6. Numerical results of maximum midspan displacement and maximum bearing rotation angle by ALE and CONWEP

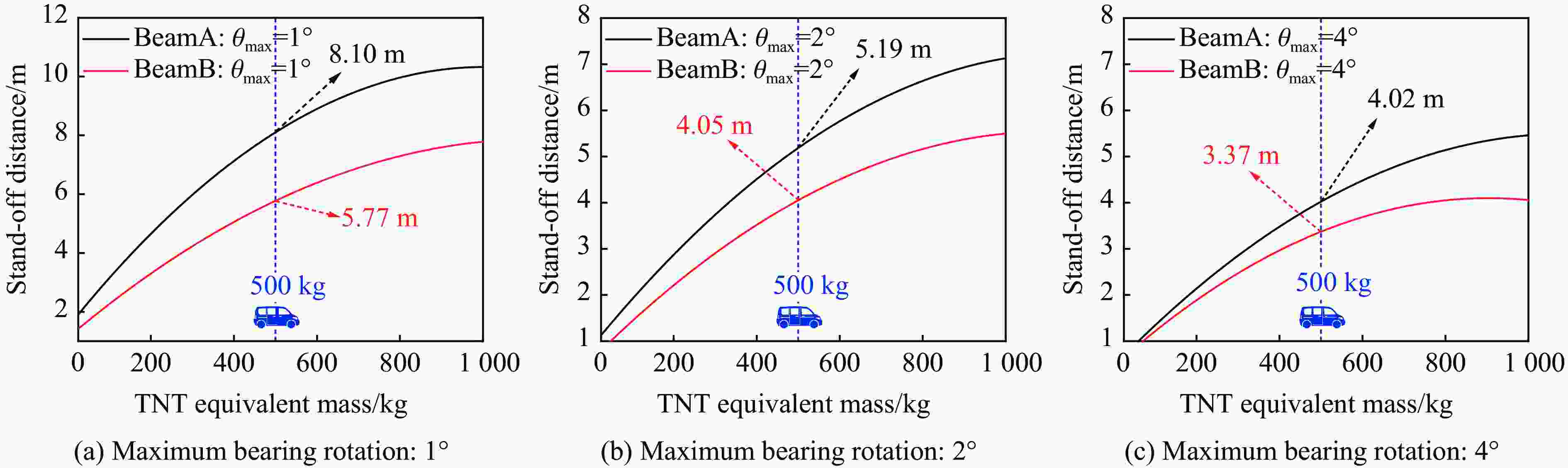
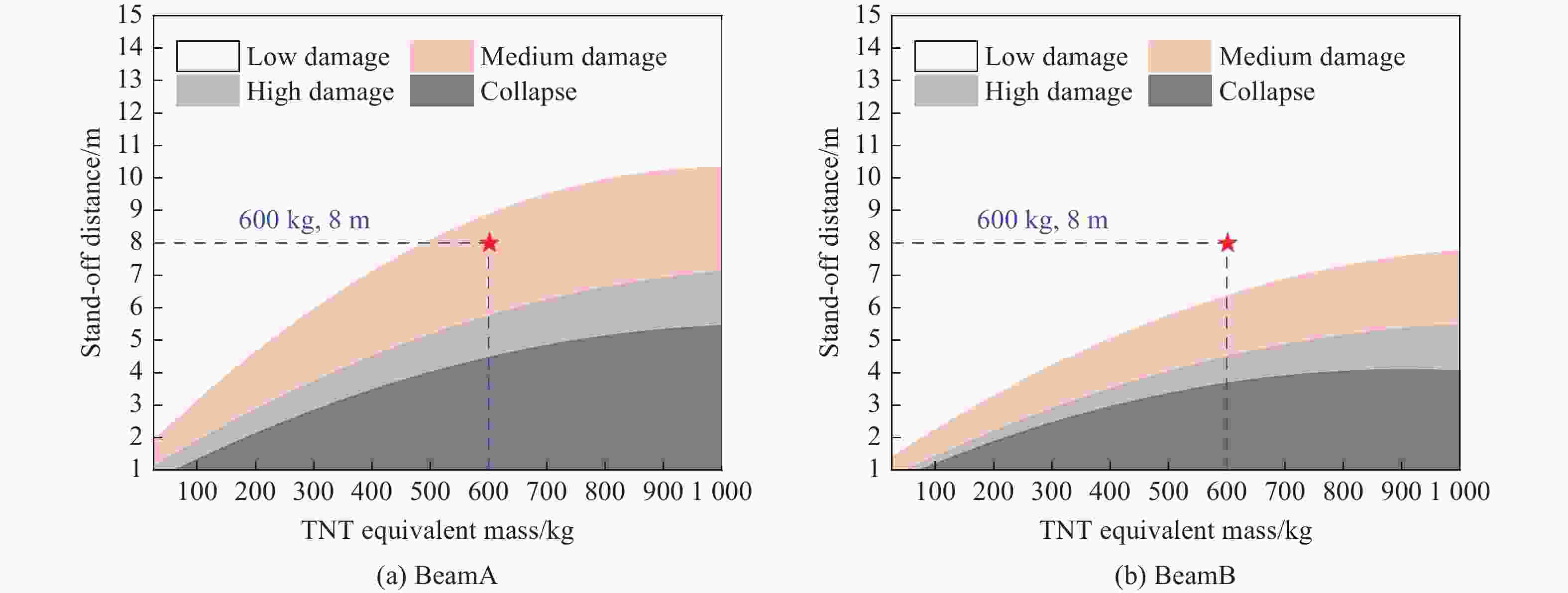
梁编号	跨中最大位移/mm		支座最大转角/(°)		梁编号	跨中最大位移/mm		支座最大转角/(°)
梁编号	ALE	CONWEP	ALE	CONWEP	梁编号	ALE	CONWEP	ALE	CONWEP
Beam4	43.522	46.933	1.558	1.680	Beam7	27.713	29.761	0.992	1.066
Beam5	35.765	38.608	1.281	1.382	Beam14	42.670	43.524	1.528	1.558
Beam6	32.488	33.628	1.163	1.204	Beam15	37.709	40.237	1.350	1.441

下载: 导出CSV

表 7 不同损伤阈值下等损伤曲线计算参数

Table 7. Calculation parameters of iso-damage curves under different damage levels

BeamA				BeamB
θ_max/(°)	a₁/(m·kg⁻¹)	a₂/(m·kg⁻²)	b/m	θ_max/(°)	a₁/(m·kg⁻¹)	a₂/(m·kg⁻²)	b/m
1	0.01766	−8.81×10⁻⁶	1.4724	1	0.01194	−5.28×10⁻⁶	1.1229
2	0.01105	−4.77×10⁻⁶	0.8542	2	0.00898	−4.05×10⁻⁶	0.5772
4	0.00926	−4.26×10⁻⁶	0.4573	4	0.00808	−4.47×10⁻⁶	0.4493

下载: 导出CSV

参考文献(31)

[1]	ABEDINI M, MUTALIB A A. Investigation into damage criterion and failure modes of RC structures when subjected to extreme dynamic loads [J]. Archives of Computational Methods in Engineering, 2020, 27(2): 501–515. DOI: 10.1007/s11831-019-09317-z.
[2]	SIELICKI P W, ŚLOSARCZYK A, SZULC D. Concrete slab fragmentation after bullet impact: an experimental study [J]. International Journal of Protective Structures, 2019, 10(3): 380–389. DOI: 10.1177/2041419619854764.
[3]	MAZURKIEWICZ L, MAŁACHOWSKI J, BARANOWSKI P. Optimization of protective panel for critical supporting elements [J]. Composite Structures, 2015, 134: 493–505. DOI: 10.1016/j.compstruct.2015.08.069.
[4]	WOODSON S C, KIGER S A. Stirrup requirements for blast-resistant slabs [J]. Journal of Structural Engineering, 1988, 114(9): 2057–2069. DOI: 10.1061/(ASCE)0733-9445(1988)114:9(2057).
[5]	KYEI C, BRAIMAH A. Effects of transverse reinforcement spacing on the response of reinforced concrete columns subjected to blast loading [J]. Engineering Structures, 2017, 142: 148–164. DOI: 10.1016/j.engstruct.2017.03.044.
[6]	汪维. 钢筋混凝土构件在爆炸载荷作用下的毁伤效应及评估方法研究 [D]. 长沙: 国防科技大学, 2012: 37–57. WANG W. Study on damage effects and assessments method of reinforced concrete structural members under blast loading [D]. Changsha: Graduate School of National University of Defense Technology, 2012: 37–57.
[7]	师燕超, 李忠献. 爆炸荷载作用下钢筋混凝土柱的动力响应与破坏模式 [J]. 建筑结构学报, 2008, 29(4): 112–117. DOI: 10.3321/j.issn:1000-6869.2008.04.015. SHI Y C, LI Z X. Dynamic responses and failure modes of RC columns under blast loading [J]. Journal of Building Structures, 2008, 29(4): 112–117. DOI: 10.3321/j.issn:1000-6869.2008.04.015.
[8]	高超, 宗周红, 伍俊. 爆炸荷载下钢筋混凝土框架结构倒塌破坏试验研究 [J]. 土木工程学报, 2013, 46(7): 9–20. DOI: 10.15951/j.tmgcxb.2013.07.012. GAO C, ZONG Z H, WU J. Experimental study on progressive collapse failure of RC frame structures under blast loading [J]. China Civil Engineering Journal, 2013, 46(7): 9–20. DOI: 10.15951/j.tmgcxb.2013.07.012.
[9]	邓国强, 杨秀敏. 钻地弹重复打击效应现场试验研究 [J]. 防护工程, 2012, 34(5): 1–5. DENG G Q, YANG X M. Experimental investigation into damage effects of repeated attacks of precision-guided penetration weapons [J]. Protective Engineering, 2012, 34(5): 1–5.
[10]	李恩奇, 何浩东, 蒋鸣, 等. 考虑倒塌因素的建筑物多弹累积毁伤效应分析 [J]. 防护工程, 2013, 35(4): 59–64. LI E Q, HE H D, JIANG M, et al. Numerical analysis of damage and collapse process of RC frame structures under repeated attacks [J]. Protective Engineering, 2013, 35(4): 59–64.
[11]	章毅, 方秦, 陈力, 等. 多次爆炸荷载作用下梁的抗爆性能分析 [J]. 兵工学报, 2009, 30(S2): 182–187. ZHANG Y, FANG Q, CHEN L, et al. Blast resistant properties of reinforced concrete and steel beams subjected to multiple blast loads [J]. Acta Armamentarii, 2009, 30(S2): 182–187.
[12]	杨大兴, 马林建, 马淑娜, 等. 常规武器对钢筋混凝土梁二次爆炸效应分析 [J]. 防护工程, 2012, 34(6): 38–41. YANG D X, MA L J, MA S N, et al. An analysis of the damage effects of a second conventional weapon explosion on reinforced concrete beams [J]. Protective Engineering, 2012, 34(6): 38–41.
[13]	陈昊, 卢浩, 孙善政, 等. 常规武器二次爆炸作用下浅埋钢筋混凝土拱结构破坏规律 [J]. 爆炸与冲击, 2023, 43(8): 085104. DOI: 10.11883/bzycj-2022-0260. CHEN H, LU H, SUN S Z, et al. Failure law of shallow buried reinforced concrete arch structure under secondary explosion of conventional weapons [J]. Explosion and Shock Waves, 2023, 43(8): 085104. DOI: 10.11883/bzycj-2022-0260.
[14]	WILLIAMS G D, WILLIAMSON E B. Response of reinforced concrete bridge columns subjected to blast loads [J]. Journal of Structural Engineering, 2011, 137(9): 903–913. DOI: 10.1061/(ASCE)ST.1943-541X.0000440.
[15]	SHI Y C, HAO H, LI Z X. Numerical derivation of pressure–impulse diagrams for prediction of RC column damage to blast loads [J]. International Journal of Impact Engineering, 2008, 35(11): 1213–1227. DOI: 10.1016/j.ijimpeng.2007.09.001.
[16]	王银, 孔祥振, 方秦, 等. 弹体对混凝土材料先侵彻后爆炸损伤破坏效应的数值模拟研究 [J]. 爆炸与冲击, 2022, 42(1): 013301. DOI: 10.11883/bzycj-2021-0132. WANG Y, KONG X Z, FANG Q, et al. Numerical investigation on damage and failure of concrete targets subjected to projectile penetration followed by explosion [J]. Explosion and Shock Waves, 2022, 42(1): 013301. DOI: 10.11883/bzycj-2021-0132.
[17]	WU Y C, CRAWFORD J E. Numerical modeling of concrete using a partially associative plasticity model [J]. Journal of Engineering Mechanics, 2015, 141(12): 04015051. DOI: 10.1061/(ASCE)EM.1943-7889.0000952.
[18]	李猛深, 李杰, 李宏, 等. 爆炸荷载下钢筋混凝土梁的变形和破坏 [J]. 爆炸与冲击, 2015, 35(2): 177–183. DOI: 10.11883/1001-1455(2015)02-0177-07. LI M S, LI J, LI H, et al. Deformation and failure of reinforced concrete beams under blast loading [J]. Explosion and Shock Waves, 2015, 35(2): 177–183. DOI: 10.11883/1001-1455(2015)02-0177-07.
[19]	MOHAMMED T A. Reinforced concrete structural members under impact loading [D]. Toledo: University of Toledo, 2011.
[20]	KONG X Z, FANG Q, LI Q M, et al. Modified K&C model for cratering and scabbing of concrete slab under projectile impact [J]. International Journal of Impact Engineering, 2017, 108: 217–228. DOI: 10.1016/j.ijimpeng.2017.02.016.
[21]	张学杰. 爆炸荷载作用下FRP加固钢筋混凝土柱动态响应精细化分析及损伤评估方法研究 [D]. 天津: 天津大学, 2020: 80–90. ZHANG X J. Research on methods for refined dynamic response analysis and damage assessment of FRP strengthened RC columns subjected to blast loading [D]. Tianjin: Tianjin University, 2020: 80–90.
[22]	Livermore Software Technology Corporation. Keyword user’s manual Volume II Material Models: LS-DYNA R11 [R]. California: Livermore Software Technology Corporation, 2010.
[23]	陈旭光. 建筑物在侵爆作用下的累积毁伤评估 [D]. 长沙: 国防科技大学, 2019: 51–83. CHEN X G. Cumulative damage assessment of buildings under penetration and explosion [D]. Changsha: Graduate School of National University of Defense Technology, 2019: 51–83.
[24]	Federal Emergency Management Agency. Reference manual to mitigate potential terrorist attacks against buildings: FEMA-426 [R]. Washington: U. S. Department of Homeland Security, 2011.
[25]	Federal Emergency Management Agency. Risk assessment: FEMA-452 [R]. Washington: U. S. Department of Homeland Security, 2005.
[26]	KELLIHER D, SUTTON-SWABY K. Stochastic representation of blast load damage in a reinforced concrete building [J]. Structural Safety, 2012, 34(1): 407–417. DOI: 10.1016/j.strusafe.2011.08.001.
[27]	CAMPIDELLI M, TAIT M J, EL-DAKHAKHNI W W, et al. Numerical strategies for damage assessment of reinforced concrete block walls subjected to blast risk [J]. Engineering Structures, 2016, 127: 559–582. DOI: 10.1016/j.engstruct.2016.08.032.
[28]	ALMUSTAFA M K, NEHDI M L. Novel hybrid machine learning approach for predicting structural response of RC beams under blast loading [J]. Structures, 2022, 39: 1092–1106. DOI: 10.1016/j.istruc.2022.04.007.
[29]	US Army Corps of Engineers. Structures to resist the effects of accidental explosions TM5-1300 [S]. Washington: Departments of the Army, the Navy, and the Air Force, 1990.
[30]	US Department of Defense. Structures to resist the effects of accidental explosions: UFC 3-340-02 [R]. Washington: The US Department of the Army, 2008.
[31]	中国工程建设标准化协会. T/CECS 736-2020 民用建筑防爆设计标准 [S]. 北京: 中国建筑工业出版社, 2020. China Association for Engineering Construction Standardization. T/CECS 736-2020 Standard for blast protection design of civil buildings [S]. Beijing: China Architecture & Building Press, 2020.