基于简易冲击分解模型的爆轰驱动硅橡胶数值模拟及实验解读

刘军; 殷建伟; 张凤国

doi:10.11883/bzycj-2024-0070

基于简易冲击分解模型的爆轰驱动硅橡胶数值模拟及实验解读

doi: 10.11883/bzycj-2024-0070

北京应用物理与计算数学研究所，北京 100094

基金项目: 国家自然科学基金（12101062，12271054）

详细信息

作者简介:
刘　军（1981－　），男，硕士，研究员，caepcfd@126.com

中图分类号: O368
计量
- 文章访问数: 45
- HTML全文浏览量: 7
- PDF下载量: 29
- 被引次数: 0
出版历程
- 收稿日期: 2024-03-11
- 修回日期: 2024-06-14
- 网络出版日期: 2024-06-17

Numerical simulation and experimental interpretation of detonation driven silicone rubber based on simple shock decomposition model

Institute of Applied Physics and Computational Mathematics, Beijing 100094, China

摘要

摘要: 为了反映爆轰驱动下硅橡胶发生冲击分解反应的物理过程，提出了一种简易的硅橡胶冲击分解模型。基于该模型，对爆轰驱动含硅橡胶夹层钢板实验进行了模拟，并分析解读了钢板的自由面速度。结果表明，实验中硅橡胶发生了冲击分解反应，导致钢板的自由面速度曲线出现了首次起跳中间速度平台及首次起跳速度峰值降低的现象。受硅橡胶冲击分解影响，首次入射波压力将在临界冲击分解压力附近弛豫一段时间，再继续升高至最高压力。该压力波作用于钢板的自由面后，出现了自由面速度在中间速度平台停留一段时间，之后继续升高至速度峰值的现象。硅橡胶冲击分解后的气相物质可压缩性较高，首次加载波内较多的能量被用于压缩气体做功，导致首次波传播至自由面时能量衰减、峰值压力降低，首次起跳速度峰值降低。
- 硅橡胶 /
- 爆轰驱动 /
- 冲击分解 /
- 分解压力
Abstract: Silicone rubber has been widely used as a typical sandwich-structure or cushion-structure material in various high pressure loading environments. Under pressure loading of up to tens of GPa, silicone rubber may undergo shock decomposition reaction, and the decomposition products contain gas-solid mixture. Numerical simulation without the shock decomposition of silicone rubber can’t interpret some complex physical phenomena observed in detonation driven experiment. In order to illustrate the shock decomposition effect of silicone rubber, a simple shock decomposition model for silicone rubber is proposed based on the existing physical knowledge. By using the simple shock decomposition model for silicone rubber, the simulations of the experiment setup of detonation driven silicone rubber foam are carried out, and the simulated free surface velocities are compared with the experiments. The results show that the shock decomposition of silicone rubber can reasonably interpret the two grotesque phenomena observed in the experiment. During the shock decomposition process, the first incident pressure of silicone rubber would relax around the critical shock decomposition pressure for a period of time. As a result, the free surface velocity of steel plate exhibits a platform as observed in the experiment during the first take-off process. The compressibility of gas phase products of silicone rubber after shock decomposition is much higher than the solid/fluid materials, so more energy in the first incident wave is consumed to compress gas products to do work, leading to energy attenuation and peak pressure reduction when the first incident wave propagates to the outer surface of steel plate. Consequently, the peak value of the first take-off free surface velocity of steel plate decreases. Insight into the dynamic behavior of silicone rubber at high pressures is particularly valuable for predicting their response to extreme conditions, and it contributes to a deeper understanding of such experimental phenomena and to the proposal of a more refined shock decomposition model for silicone rubber.
- silicone rubber /
- detonation driven /
- shock decomposition /
- decomposition pressure

HTML全文

图 1 爆轰驱动双层钢板实验的二维平面示意图^[22]

Figure 1. Schematic diagram of the detonation-driven steel plates experiment^[22]

下载: 全尺寸图片幻灯片

图 2 爆轰驱动双层钢板实验的简化计算模型和局部网格划分情况

Figure 2. Simplified calculation model of experimental setup with the details of mesh division

下载: 全尺寸图片幻灯片

图 3 起爆后不同时刻模拟的钢板密度分布

Figure 3. Simulated density distributions of steel at different times after initiation

下载: 全尺寸图片幻灯片

图 4 $ {\varnothing } $30 mm位置上S₃和S₄的自由面速度

Figure 4. Free surface velocity of S₄, S₃ at $ {\varnothing } $30 mm

下载: 全尺寸图片幻灯片

图 5 硅橡胶泡沫1/2厚度处的压力、密度和内能

Figure 5. Simulated pressure, density and internal energy in the middle position of silicone rubber foam

下载: 全尺寸图片幻灯片

图 6 考虑冲击分解后硅橡胶一侧测点S₃处的自由面速度

Figure 6. Free surface velocity of S₃ on silicone side with shock decomposition

下载: 全尺寸图片幻灯片

图 7 硅橡胶泡沫1/2厚度处的压力和内能时程曲线

Figure 7. Time histories of simulated pressure and internal energy in the middle position of silicone rubber

下载: 全尺寸图片幻灯片

表 1 45钢的计算参数^[26-28]

Table 1. Calculation parameters of 45 steel^[26-28]

ρ₀^[26]/(g·cm⁻³)	c₀^[26]/(km·s⁻¹)	S₁^[26]	S₂	S₃	γ₀^[26]	λ^[26]	G₀^[27-28]/GPa	Y₀^[27-28]/GPa	T_m0^[26]/K	α₀	α_s/GPa	η/(Pa·s)	D_cut
7.85	4.57	1.49	0	0	2.17	0.43	81.8	0.355	2380	1.0001	0.3	10	0.10

下载: 导出CSV

表 2 硅橡胶（泡沫）的计算参数^[7,11]

Table 2. Calculation parameters of silicone rubber (foam)^[7,11]

ρ₀^[7]/(g·cm⁻³)	c₀^[7]/(km1·s⁻¹)	S₁^[7]	S₂	S₃	γ₀^[7]	λ	ρ₀₀^[11]/(g·cm⁻³)
1.04	1.63	1.66	0	0	1.5	0	0.92

下载: 导出CSV

表 3 RHT-901炸药的计算参数^[31-32]

Table 3. Calculation parameters of RHT-901^[31-32]

ρ₀^[31]/(g·cm⁻³)	P_CJ^[31]/GPa	D_CJ^[31]/(km·s⁻¹)	A/GPa	B/GPa	R₁	R₂	ω	E^[32]/(kJ·cm⁻³)
1.7	28.0	7.8	737.4	16.94	4.8	0.58	0.34	8.5

下载: 导出CSV

参考文献(37)

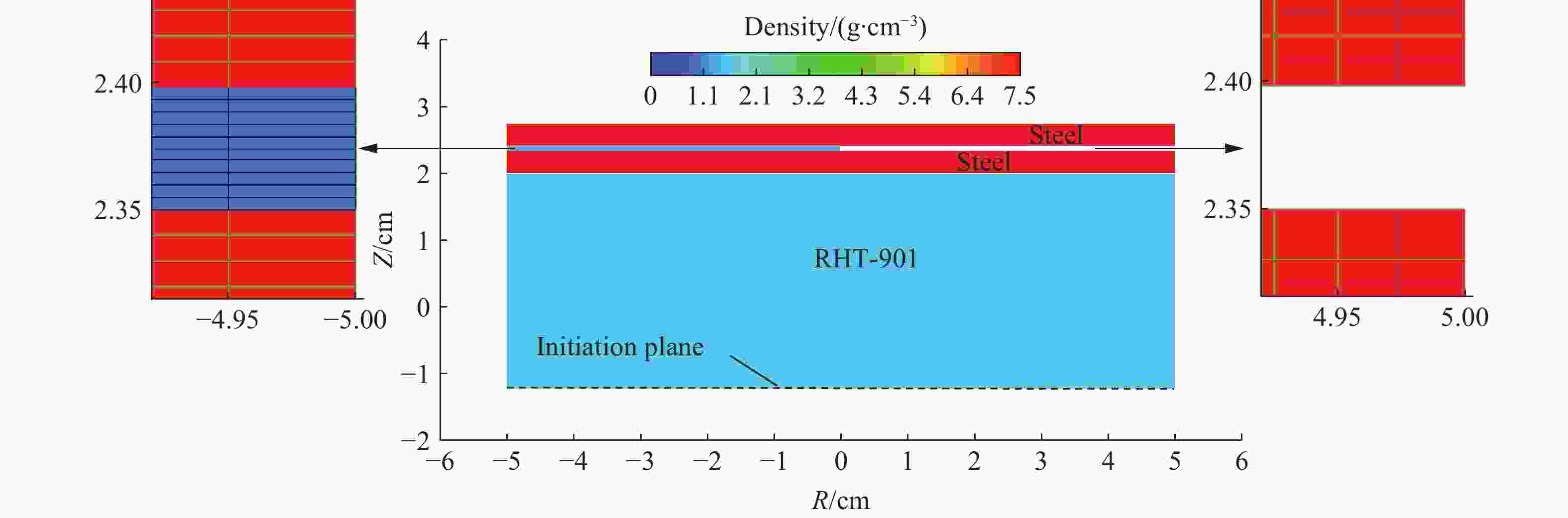
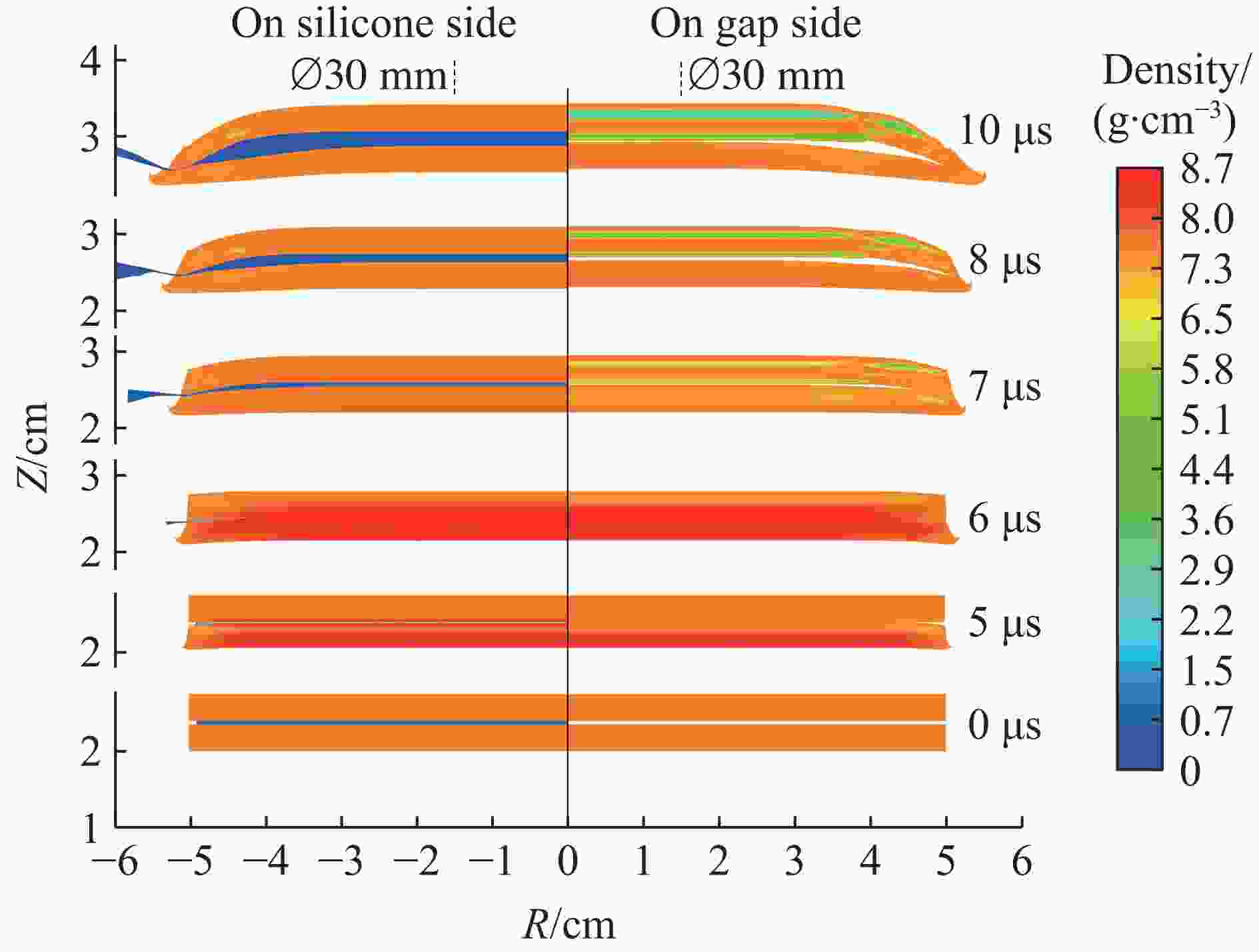
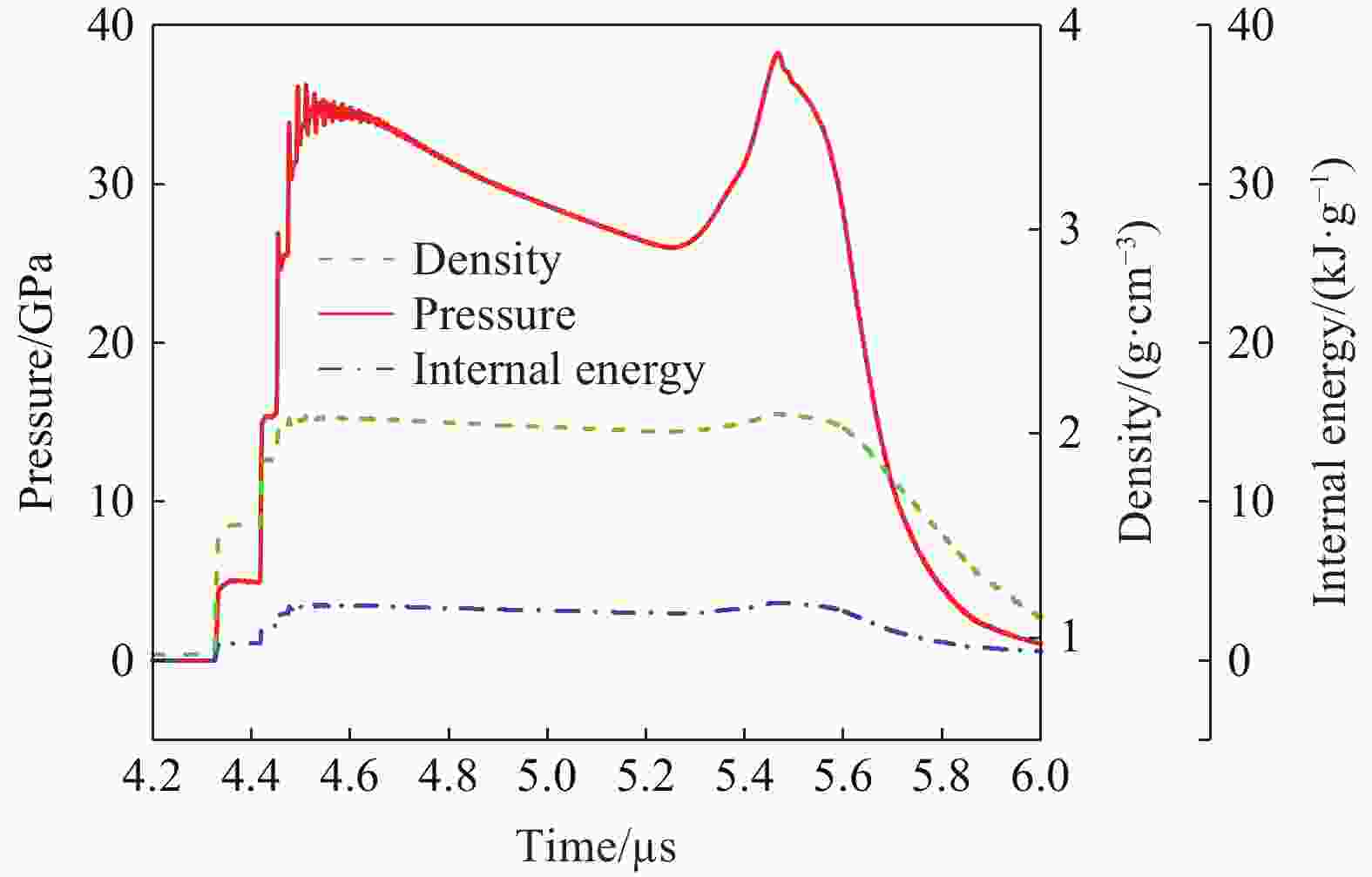
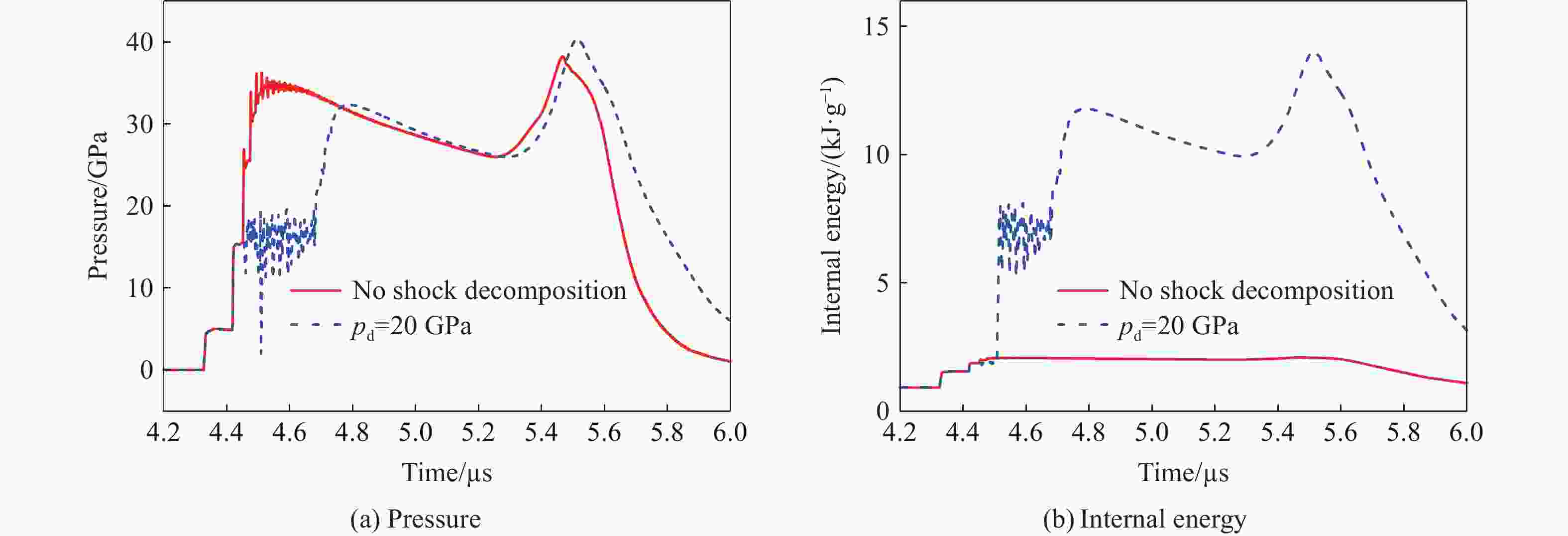
[1]	LANDROCK A H. Handbook of plastic foams: types, properties, manufacture and applications [M]. Park Ridge: Noyes Publications, 1995.
[2]	MAITI A, WEISGRABER T H, GEE R H. Modeling the mechanical and aging properties of silicone rubber and foam-stockpile-historical & additively manufactured materials: LLNL-TR-661699 [R]. Livermore: LLNL, 2014.
[3]	SANBORN B, SONG B, SMITH S. Pre-strain effect on frequency-based impact energy dissipation through a silicone foam pad for shock mitigation [J]. Journal of Dynamic Behavior of Materials, 2016, 2(1): 138–145. DOI: 10.1007/s40870-015-0043-1.
[4]	DOWELL F. Simple EOS for the silicone rubber Sylgard 184: LA-10164-MS [R]. United States: Los Alamos National Lab, 1984.
[5]	CARTER W J, MARSH S P. Hugoniot equation of state of polymers: LA-13006-MS [R]. USA: Los Alamos National Lab, 1995.
[6]	KONDO K, YASUMOTO Y, SUGIURA H, et al. Multiple shock reverberations in a layer structure observed by particle-velocity and pressure gauges [J]. Journal of Applied Physics, 1981, 52(2): 772–776. DOI: 10.1063/1.328761.
[7]	WINTER R E, WHITEMAN G, HAINING G S, et al. Measurement of equation of state of silicone elastomer [J]. AIP Conference Proceedings, 2004, 706(1): 679–684.
[8]	DATTELBAUM D M, JENSEN J D, SCHWENDT A M, et al. A novel method for static equation-of state-development: Equation of state of a cross-linked poly(dimethylsiloxane) (PDMS) network to 10 GPa [J]. The Journal of Chemical Physics, 2005, 122(14): 144903. DOI: 10.1063/1.1879872.
[9]	曾鉴荣, 刘勇, 杜保国, 等. 低密度硅橡胶冲击雨贡纽曲线测量 [J]. 高压物理学报, 1996, 10(4): 299–303. DOI: 10.11858/gywlxb.1996.04.010. ZENG J R, LIUY, DU B G, et al. Measurement of shock-Hugoniot curve of low-density silastic [J]. Chinese Journal of High Pressure Physics, 1996, 10(4): 299–303. DOI: 10.11858/gywlxb.1996.04.010.
[10]	王青松. 泡沫态硅橡胶冲击绝热线的近似计算 [J]. 高压物理学报, 2010, 24(2): 120–124. DOI: 10.11858/gywlxb.2010.02.007. WANG Q S. Calculation of the Hugoniot of silicon rubber foam [J]. Chinese Journal of High Pressure Physics, 2010, 24(2): 120–124. DOI: 10.11858/gywlxb.2010.02.007.
[11]	李欣竹, 吴强, 张汉钊, 等. 泡沫硅橡胶冲击压缩性实验研究 [J]. 高压物理学报, 1998, 12(4): 291–297. DOI: 10.11858/gywlxb.1998.04.008. LI X Z, WU Q, ZHANG H Z, et al. Study on the shock compression of foam silicon rubber [J]. Chinese Journal of High Pressure Physics, 1998, 12(4): 291–297. DOI: 10.11858/gywlxb.1998.04.008.
[12]	MORRIS C E, FRITZ J N, MCQUEEN R G. The equation of state of polytetrafluoroethylene to 80 GPa [J]. The Journal of Chemical Physics, 1984, 80(10): 5203–5218. DOI: 10.1063/1.446591.
[13]	DATTELBAUM D M, COE J D, RIGG P A, et al. Shockwave response of two carbon fiber-polymer composites to 50 GPa [J]. Journal of Applied Physics, 2014, 116: 194308. DOI: 10.1063/1.4898313.
[14]	KERLEY G I. Equation of state and constitutive models for numerical simulations of dust impacts on the solar probe: KTS09-1 [R]. Maryland: Johns Hopkins University Applied Physics Laboratory, 2013.
[15]	HAMDANI S, LONGUET C, PERRIN D, et al. Flame retardancy of silicone-based materials [J]. Polymer Degradation and Stability, 2009, 94(4): 465–495. DOI: 10.1016/j.polymdegradstab.2008.11.019.
[16]	GRASSIE N, MACFARLANE I G. The thermal degradation of polysiloxanes- I. Poly(dimethylsiloxane) [J]. European Polymer Journal, 1978, 14(11): 875–884. DOI: 10.1016/0014-3057(78)90084-8.
[17]	CAMINO G, LOMAKIN S M, LAZZARI M. Polydimethylsiloxane thermal degradation Part 1. Kinetic aspects [J]. Polymer, 2001, 42(6): 2395–2402. DOI: 10.1016/S0032-3861(00)00652-2.
[18]	CAMINO G, LOMAKIN S M, LAGEARD M. Thermal polydimethylsiloxane degradation. Part 2. The degradation mechanisms [J]. Polymer, 2002, 43(7): 2011–2015. DOI: 10.1016/S0032-3861(01)00785-6.
[19]	JOVANOVIC J D, GOVEDARICA M N, DVORNIC P R, et al. The thermogravimetric analysis of some polysiloxanes [J]. Polymer Degradation and Stability, 1998, 61(1): 87–93. DOI: 10.1016/S0141-3910(97)00135-3.
[20]	RADHAKRISHNAN T S. New method for evaluation of kinetic parameters and mechanism of degradation from pyrolysis-GC studies: thermal degradation of polydimethylsiloxanes [J]. Journal of Applied Polymer Science, 1999, 73(3): 441–450. DOI: 10.1002/(SICI)1097-4628(19990718)73:3<441::AID-APP16>3.0.CO;2-J.
[21]	HAYASHIDA K, TSUGE S, OHTANI H. Flame retardant mechanism of polydimethylsiloxane material containing platinum compound studied by analytical pyrolysis techniques and alkaline hydrolysis gas chromatography [J]. Polymer, 2003, 44(19): 5611–5616. DOI: 10.1016/S0032-3861(03)00622-0.
[22]	李涛, 刘明涛, 王晓燕, 等. 装配垫层与间隙对爆轰加载下金属飞片运动特征的影响 [J]. 高压物理学报, 2018, 32(4): 044202. DOI: 10.11858/gywlxb.20170576. LI T, LIU M T, WANG X Y, et al. Effects of explosive device with foam cushion and air clearance on kinetic characteristic of steel flyer under detonation loading [J]. Chinese Journal of High Pressure Physics, 2018, 32(4): 044202. DOI: 10.11858/gywlxb.20170576.
[23]	刘军, 孙致远, 张凤国, 等. 间隙对爆轰加载下金属飞片运动特征影响的模拟分析 [J]. 爆炸与冲击, 2023, 43(4): 042201. DOI: 10.11883/bzycj-2022-0239. LIU J, SUN Z Y, ZHANG F G, et al. Simulation analysis of the effect of clearance on motion characteristic of metal flyer under detonation loading [J]. Explosion and Shock Waves, 2023, 43(4): 042201. DOI: 10.11883/bzycj-2022-0239.
[24]	WHIRLEY R G, ENGELMANN B E. DYNA2D: A nonlinear, explicit, two-dimensional finite element code for solid mechanics: User manual: UCRL-MA-110630 [R]. USA: Lawrence Livermore National Lab. , 1992.
[25]	孙承纬. 炸药平面波透镜的有效药量 [C]//爆轰研究论文集(第3卷). 绵阳: 中国工程物理研究院流体物理研究所, 1998: 307–316.
[26]	STEINBERG D J. Equation of state and strength properties of selected materials: UCRL-MA-106439 [R]. USA: Lawrence Livermore National Laboratory Report, 1991.
[27]	张林, 张祖根, 秦晓云, 等. D6A、921和45钢的动态破坏与低压冲击特性 [J]. 高压物理学报, 2003, 17(4): 305–310. DOI: 10.11858/gywlxb.2003.04.011. ZHANG L, ZHANG Z G, QIN X Y, et al. Dynamic fracture and mechanical property of D6A, 921 and 45 steels under low shock pressure [J]. Chinese Journal of High Pressure Physics, 2003, 17(4): 305–310. DOI: 10.11858/gywlxb.2003.04.011.
[28]	胡昌明, 贺红亮, 胡时胜. 45号钢的动态力学性能研究 [J]. 爆炸与冲击, 2003, 23(2): 188–192. DOI: 10.11883/1001-1455(2003)02-0188-5. HU C M, HE H L, HU S S. A study on dynamic mechancial behaviors of 45 steel [J]. Explosion and Shock Waves, 2003, 23(2): 188–192. DOI: 10.11883/1001-1455(2003)02-0188-5.
[29]	HERRMANN W. Constitutive equation for the dynamic compaction of ductile porous materials [J]. Journal of Applied Physics, 1969, 40(6): 2490–2499. DOI: 10.1063/1.1658021.
[30]	CARROLL M, HOLT A C. Suggested modification of the P-α model for porous materials [J]. Journal of Applied Physics, 1972, 43(2): 759–761. DOI: 10.1063/1.1661203.
[31]	DOBRATZ B M, CRAWFORD P C. LLNL explosives handbook properties of chemical explosives and explosive simulants: UCRL-52997 [R]. Livermore: LLNL, 1985.
[32]	董海山, 周芬芬. 高能炸药及相关物性能 [M]. 北京: 科学出版社, 1989.
[33]	DOBRATZ B M. Properties of chemical explosives and explosive simulants: UCRL-51319 [R]. Livermore: Lawrence Livermore National Lab. , 1972.
[34]	PARTOM Y. Detonation products EOS by specifying gamma (V) for the principal isentrope [J]. Journal of Energetic Materials, 2011, 29(3): 193–208. DOI: 10.1080/07370652.2010.514888.
[35]	PARTOM Y. Calibrating gruneisen-gamma(V) in the framework of the adiabatic-gamma(V) EOS for detonation products [J]. Journal of Energetic Materials, 2012, 30(3): 252–264. DOI: 10.1080/07370652.2011.573524.
[36]	YANG D, ZHANG W, JIANG B Z, et al. Silicone rubber ablative composites improved with zirconium carbide or zirconia [J]. Composites Part A: Applied Science and Manufacturing, 2013, 44: 70–77. DOI: 10.1016/j.compositesa.2012.09.002.
[37]	BELYAEV A F, BOBOLEV V K, KOROTKOV A I, et al. Shock-initiated detonation: determination of critical initiation pressures: SAND-76-6016 [R]. USA, 1976.