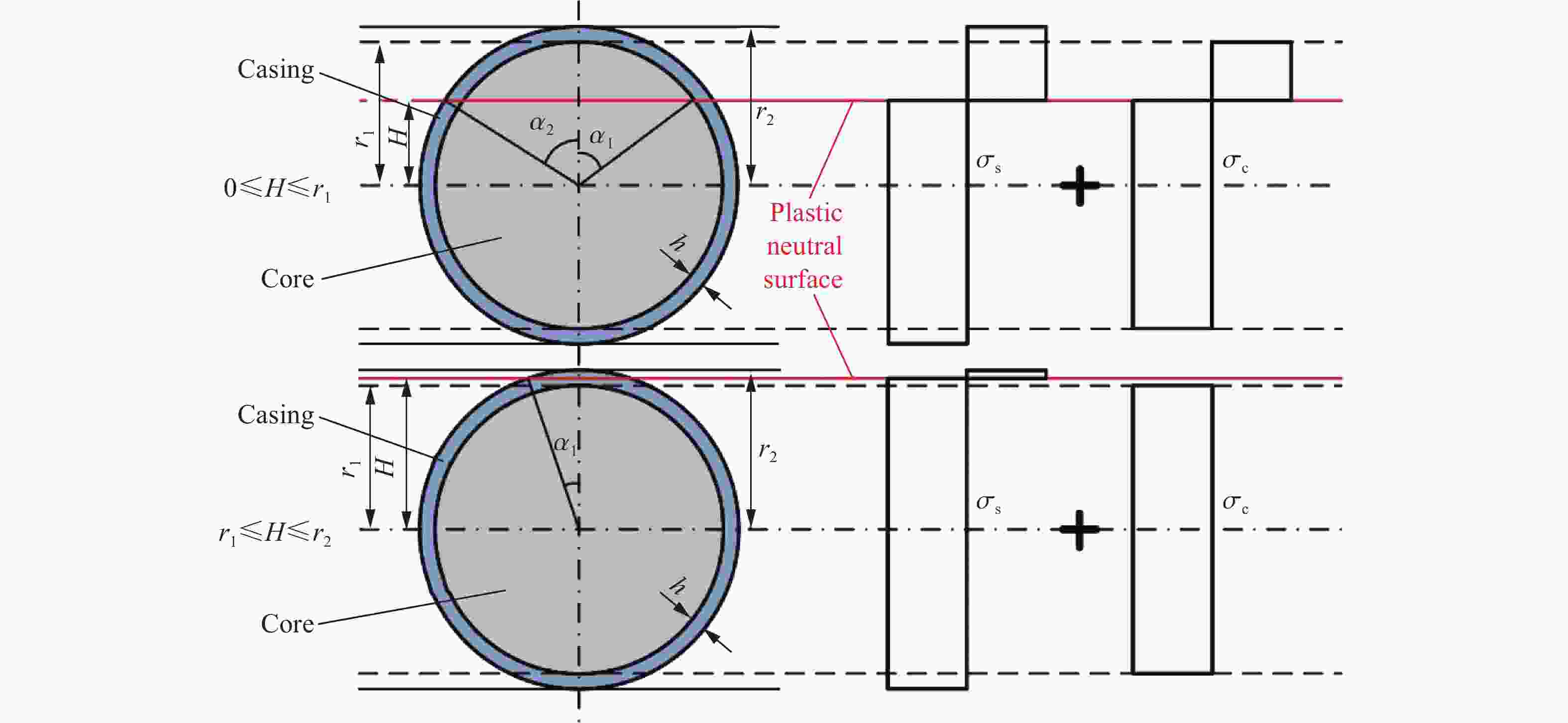
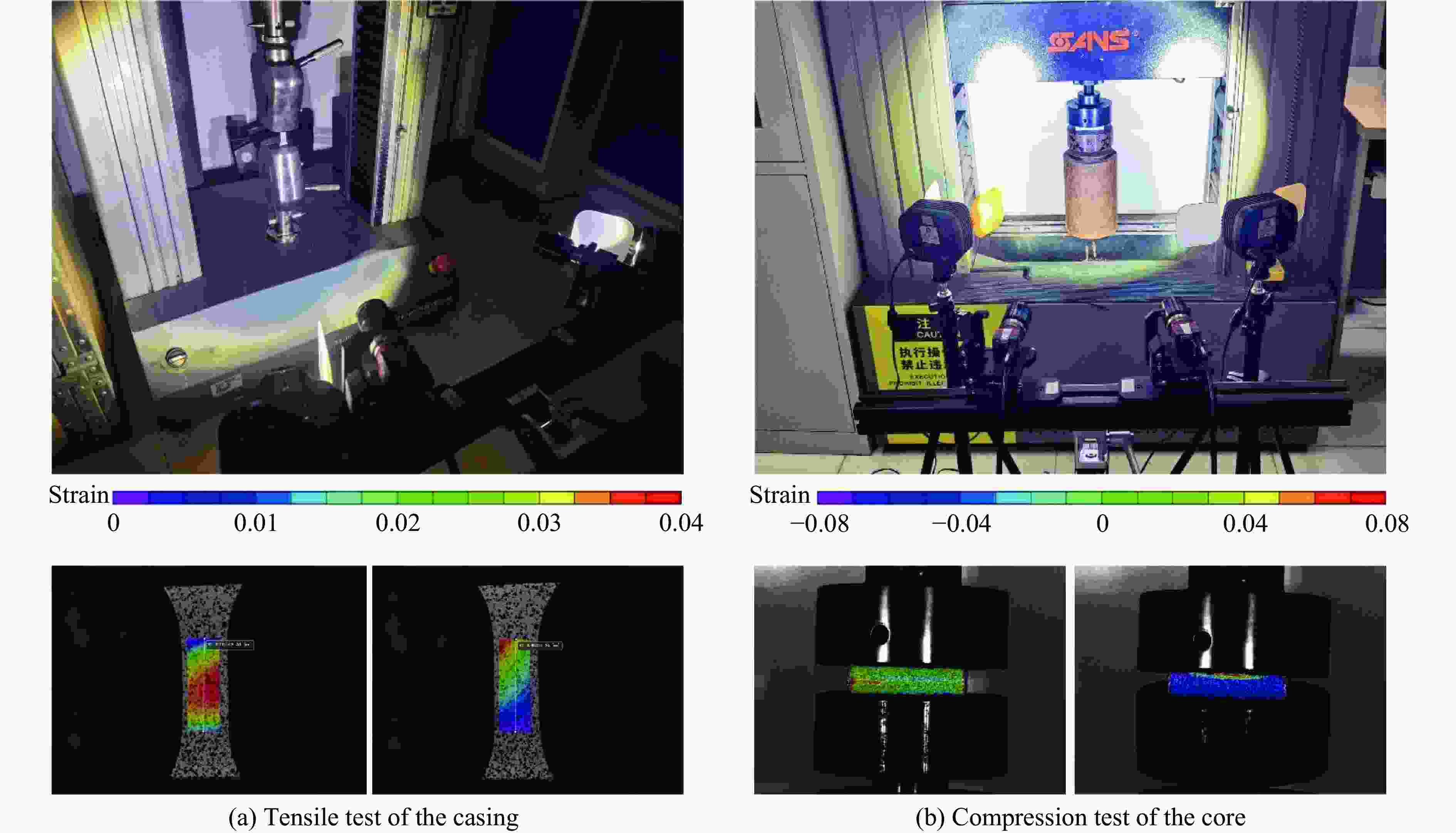
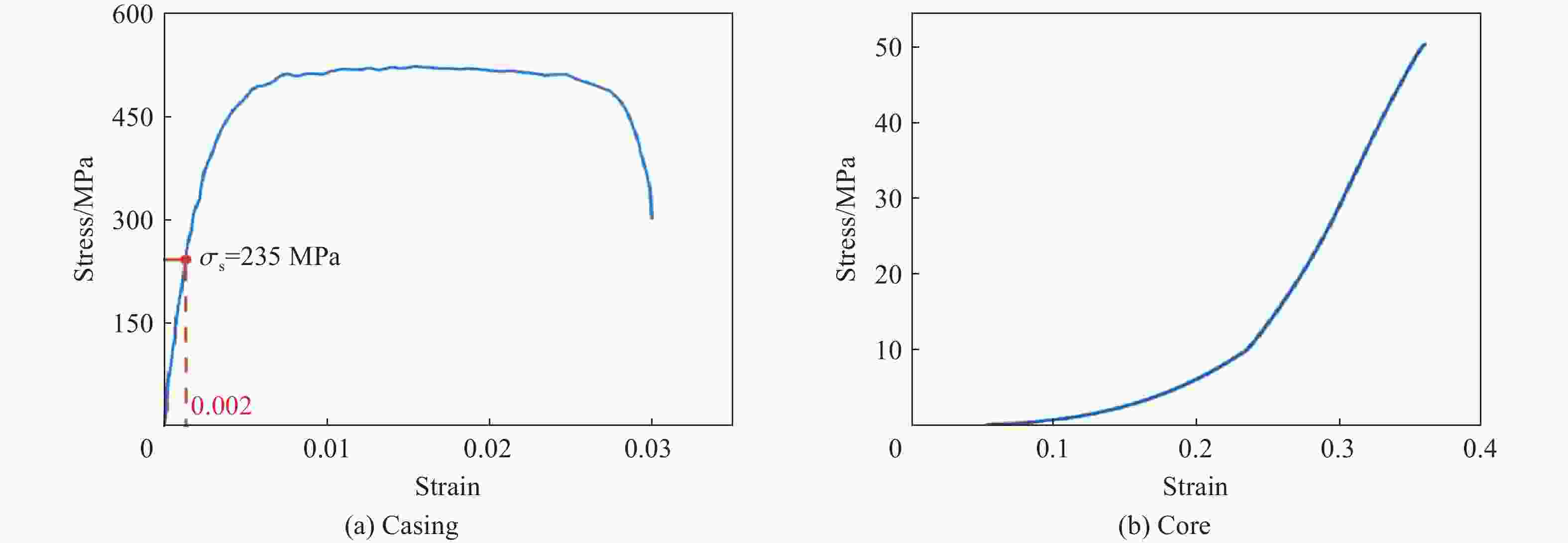
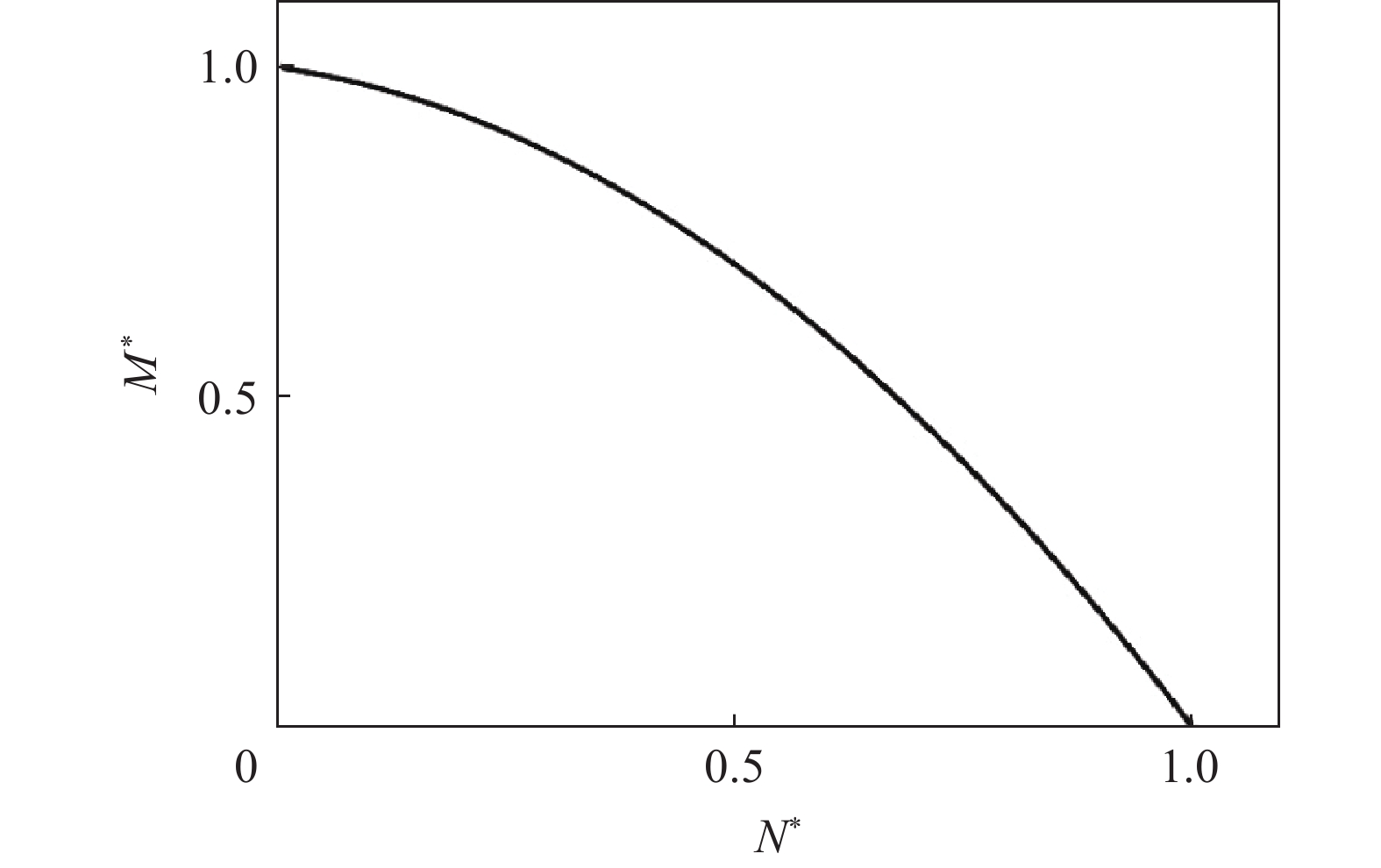
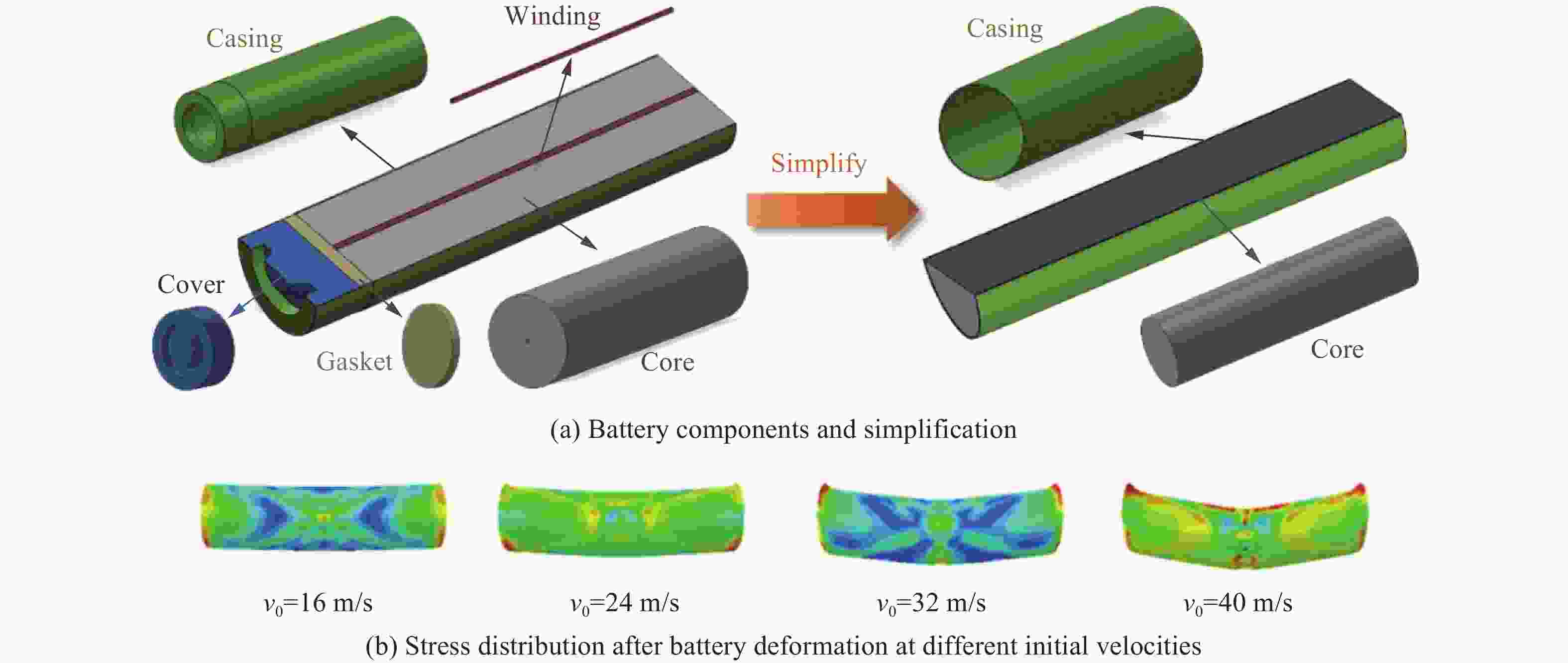
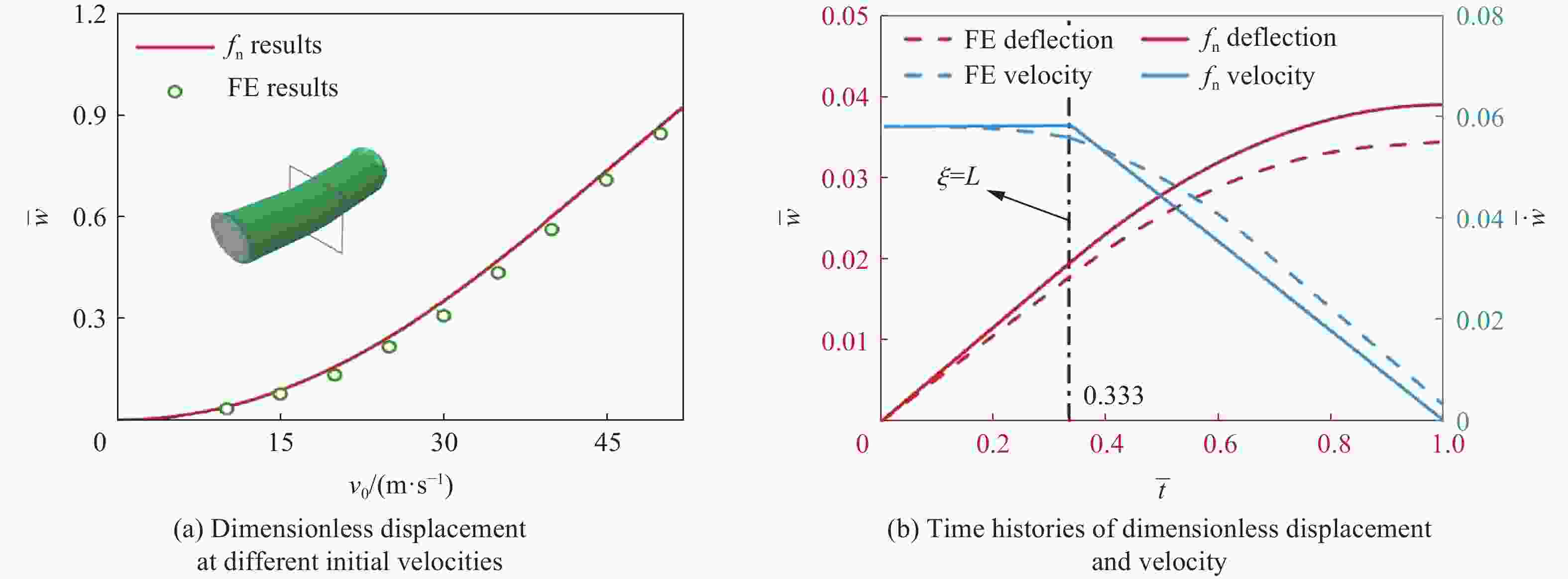
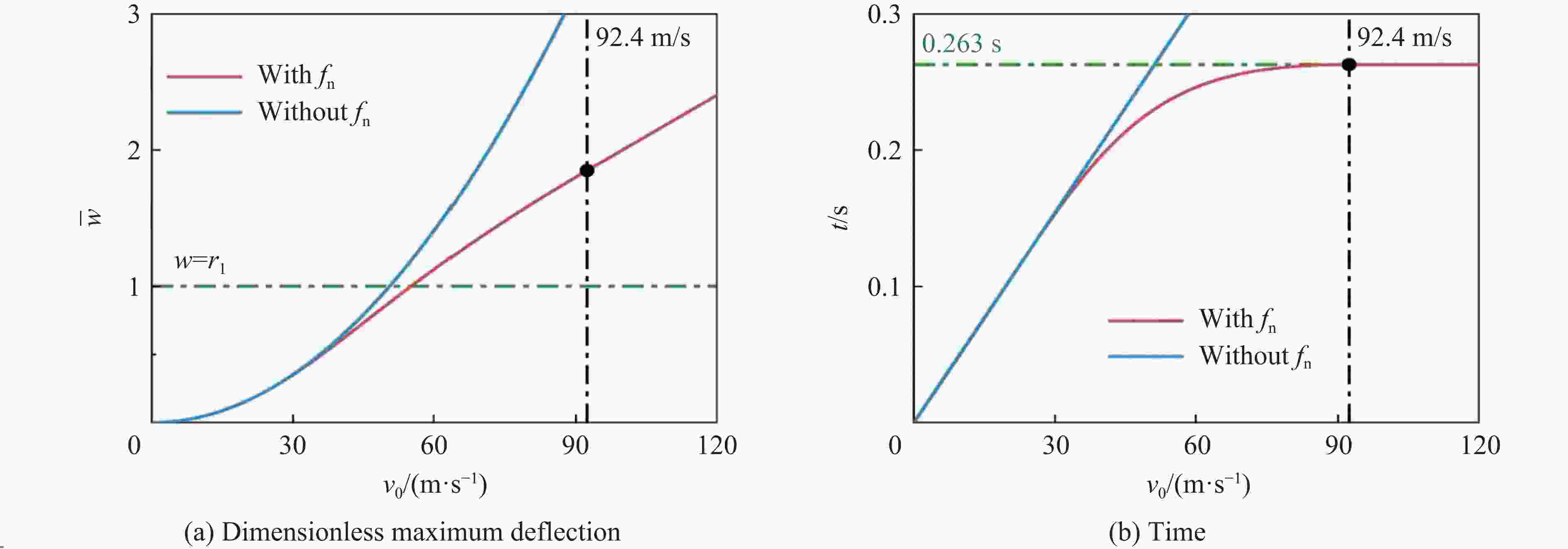
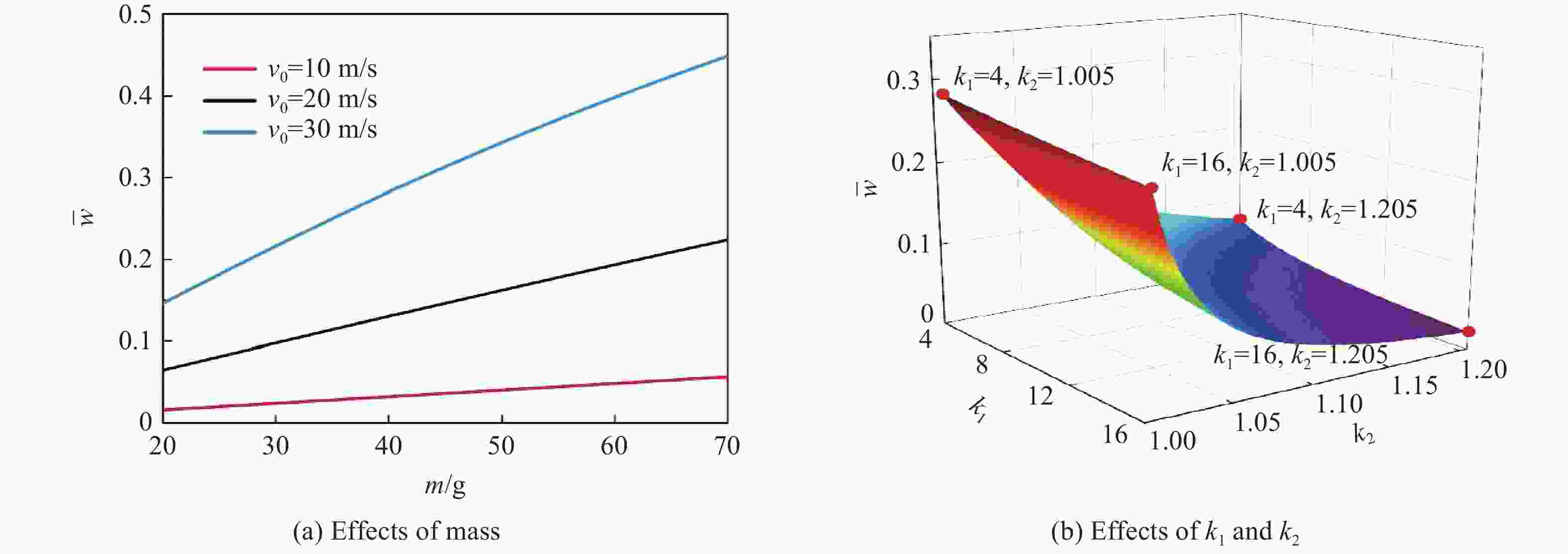
| [1] |
HUANG Z X, ZHANG X C, Liu N N, et al. Failure mechanisms and acoustic responses of cylindrical lithium-ion batteries under compression loadings [J]. Engineering Failure Analysis, 2024, 163: 108594. DOI: 10.1016/j.engfailanal.2024.108594.
|
| [2] |
XU J, LIU B H, HU D Y. State of charge dependent mechanical integrity behavior of 18650 lithium-ion batteries [J]. Scientific Reports, 2016, 6: 21829. DOI: 10.1038/srep21829.
|
| [3] |
WANG W W, YANG S, LIN C. Clay-like mechanical properties for the jellyroll of cylindrical lithium-ion cells [J]. Applied Energy, 2017, 196: 249–258. DOI: 10.1016/j.apenergy.2017.01.062.
|
| [4] |
SAHRAEI E, CAMPBELL J, WIERZBICKI T. Modeling and short circuit detection of 18650 Li-ion cells under mechanical abuse conditions [J]. Journal of Power Sources, 2012, 220: 360–372. DOI: 10.1016/j.jpowsour.2012.07.057.
|
| [5] |
GILAKI M, AVDEEV I. Impact modeling of cylindrical lithium-ion battery cells: a heterogeneous approach [J]. Journal of Power Sources, 2016, 328: 443–451. DOI: 10.1016/j.jpowsour.2016.08.034.
|
| [6] |
ZHANG H J, ZHOU M Z, HU L L, et al. Mechanism of the dynamic behaviors and failure analysis of lithium-ion batteries under crushing based on stress wave theory [J]. Engineering Failure Analysis, 2020, 108: 104290. DOI: 10.1016/j.engfailanal.2019.104290.
|
| [7] |
ZHANG X C, ZHANG T, LIU N N, et al. Dynamic crushing behaviors and failure of cylindrical lithium-ion batteries subjected to impact loading [J]. Engineering Failure Analysis, 2023, 154: 107653. DOI: 10.1016/j.engfailanal.2023.107653.
|
| [8] |
ZHU J E, LUO H L, LI W, et al. Mechanism of strengthening of battery resistance under dynamic loading [J]. International Journal of Impact Engineering, 2019, 131: 78–84. DOI: 10.1016/j.ijimpeng.2019.05.003.
|
| [9] |
WANG L B, LI J P, CHEN J Y, et al. Revealing the internal short circuit mechanisms in lithium-ion batteries upon dynamic loading based on multiphysics simulation [J]. Applied Energy, 2023, 351: 121790. DOI: 10.1016/j.apenergy.2023.121790.
|
| [10] |
ZHOU D, LI H G, LI Z H, et al. Toward the performance evolution of lithium-ion battery upon impact loading [J]. Electrochimica Acta, 2022, 432: 141192. DOI: 10.1016/j.electacta.2022.141192.
|
| [11] |
陈发良, 余同希. 正多边形板的塑性动力响应: 小挠度分析和大挠度分析 [J]. 爆炸与冲击, 1991, 11(2): 106–116. DOI: 10.11883/1001-1455(1991)02-0106-11.CHEN F L, YU T X. Dynamic plastic response of regular polygonal plates [J]. Explosion and Shock Waves, 1991, 11(2): 106–116. DOI: 10.11883/1001-1455(1991)02-0106-11.
|
| [12] |
CHEN F L, YU T X. Analysis of large deflection dynamic response of rigid-plastic beams [J]. Journal of Engineering Mechanics, 1993, 119(6): 1293–1301. DOI: 10.1061/(ASCE)0733-9399(1993)119:6(1293).
|
| [13] |
QIN Q H, WANG T J. A theoretical analysis of the dynamic response of metallic sandwich beam under impulsive loading [J]. European Journal of Mechanics-A/Solids, 2009, 28(5): 1014–1025. DOI: 10.1016/j.euromechsol.2009.04.002.
|
| [14] |
XIANG C P, QIN Q H, WANG M S, et al. Low-velocity impact response of sandwich beams with a metal foam core: Experimental and theoretical investigations [J]. International Journal of Impact Engineering, 2019, 130: 172–183. DOI: 10.1016/j.ijimpeng.2019.04.014.
|
| [15] |
谌勇, 唐平, 汪玉, 等. 刚塑性圆板受水下爆炸载荷时的动力响应 [J]. 爆炸与冲击, 2005, 25(1): 90–96. DOI: 10.11883/1001-1455(2005)01-0090-07.CHEN Y, TANG P, WANG Y, et al. Dynamic response analysis of rigid-plastic circular plate under underwater blast loading [J]. Explosion and Shock Waves, 2005, 25(1): 90–96. DOI: 10.11883/1001-1455(2005)01-0090-07.
|
| [16] |
张新春, 王俊瑜, 汪玉林, 等. 基于膜力因子法的方形锂离子电池冲击动力响应研究 [J]. 应用数学和力学, 2022, 43(11): 1203–1213. DOI: 10.21656/1000-0887.430289.ZHANG X C, WANG J Y, WANG Y L, et al. Impact responses of prismatic lithium-ion battery based on the membrane factor method [J]. Applied Mathematics and Mechanics, 2022, 43(11): 1203–1213. DOI: 10.21656/1000-0887.430289.
|
| [17] |
ZHANG X C, HUANG Z X, WANG Y L, et al. Dynamic responses of cylindrical lithium-ion battery under localized impact loading [J]. Mechanics of Advanced Materials and Structures, 2024. DOI: 10.1080/15376494.2024.2359648.
|
| [18] |
YU T X, STRONGE W J. Large deflections of a rigid-plastic beam-on-foundation from impact [J]. International Journal of Impact Engineering, 1990, 9(1): 115–126. DOI: 10.1016/0734-743X(90)90025-Q.
|
| [19] |
XIA Y, WIERZBICKI T, SAHRAEI E, et al. Damage of cells and battery packs due to ground impact [J]. Journal of Power Sources, 2014, 267: 78–97. DOI: 10.1016/j.jpowsour.2014.05.078.
|
| [20] |
JIANG W Z, LIU Y, WANG B. Dynamic responses of metal sandwich beams under high velocity impact considering time inhomogeneity of core deformation [J]. International Journal of Impact Engineering, 2017, 110: 311–323. DOI: 10.1016/j.ijimpeng.2017.05.010.
|
| [21] |
XU J, LIU B H, WANG X Y, et al. Computational model of 18650 lithium-ion battery with coupled strain rate and SOC dependencies [J]. Applied Energy, 2016, 172: 180–189. DOI: 10.1016/j.apenergy.2016.03.108.
|
| [22] |
WANG L B, CHEN J Y, LI J P, et al. A novel anisotropic model for multi-stage failure threshold of lithium-ion battery subjected to impact loading [J]. International Journal of Mechanical Sciences, 2022, 236: 107757. DOI: 10.1016/j.ijmecsci.2022.107757.
|






 下载:
下载: