Effects of loading pressure and gap dimension on the formation of gap jet under strong dynamic loading
-
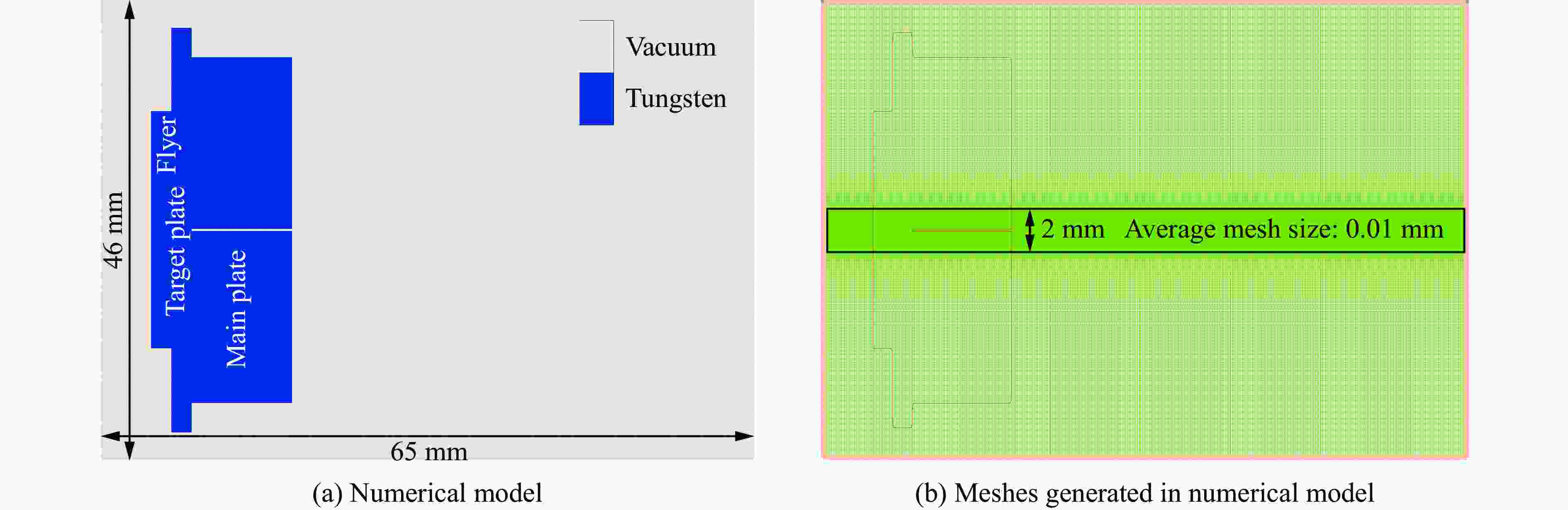
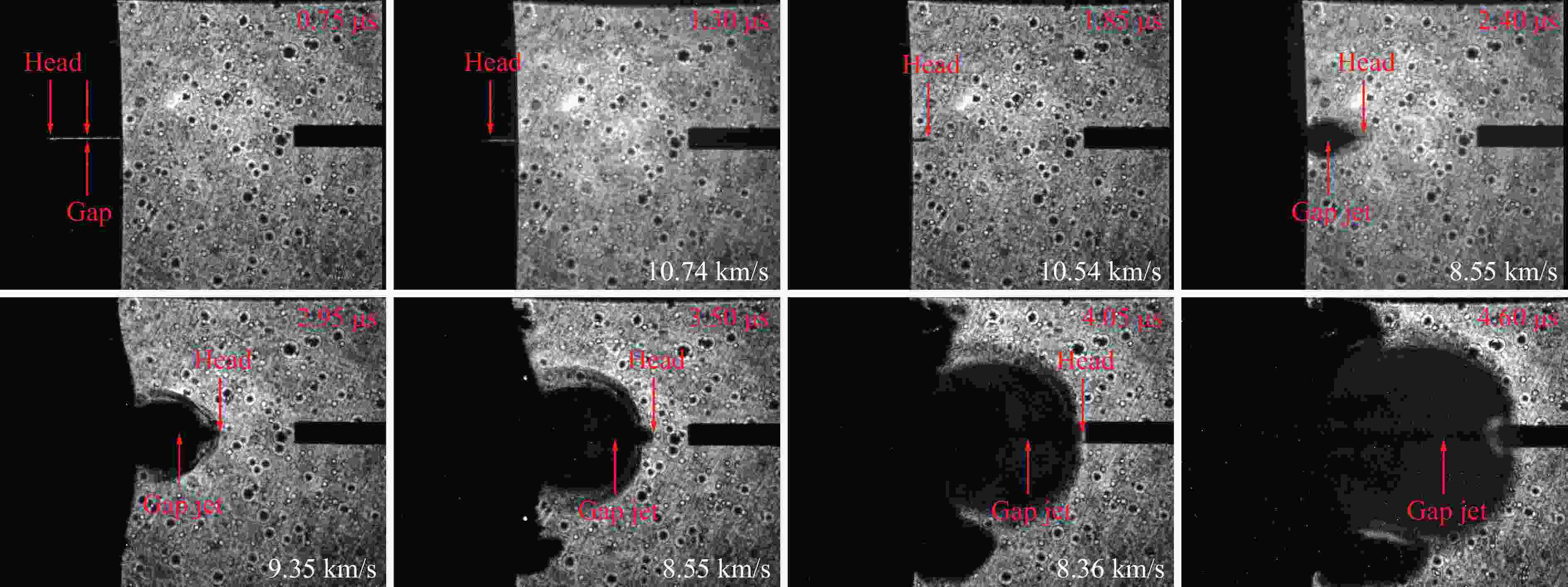
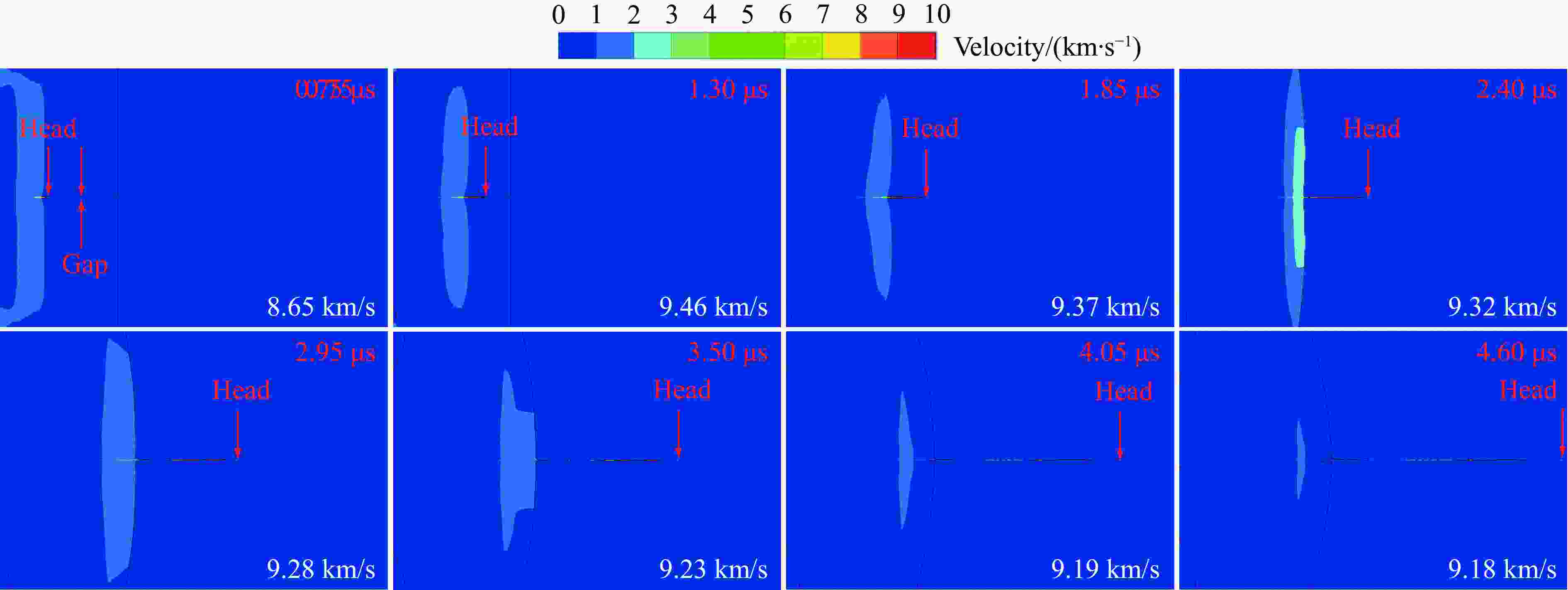
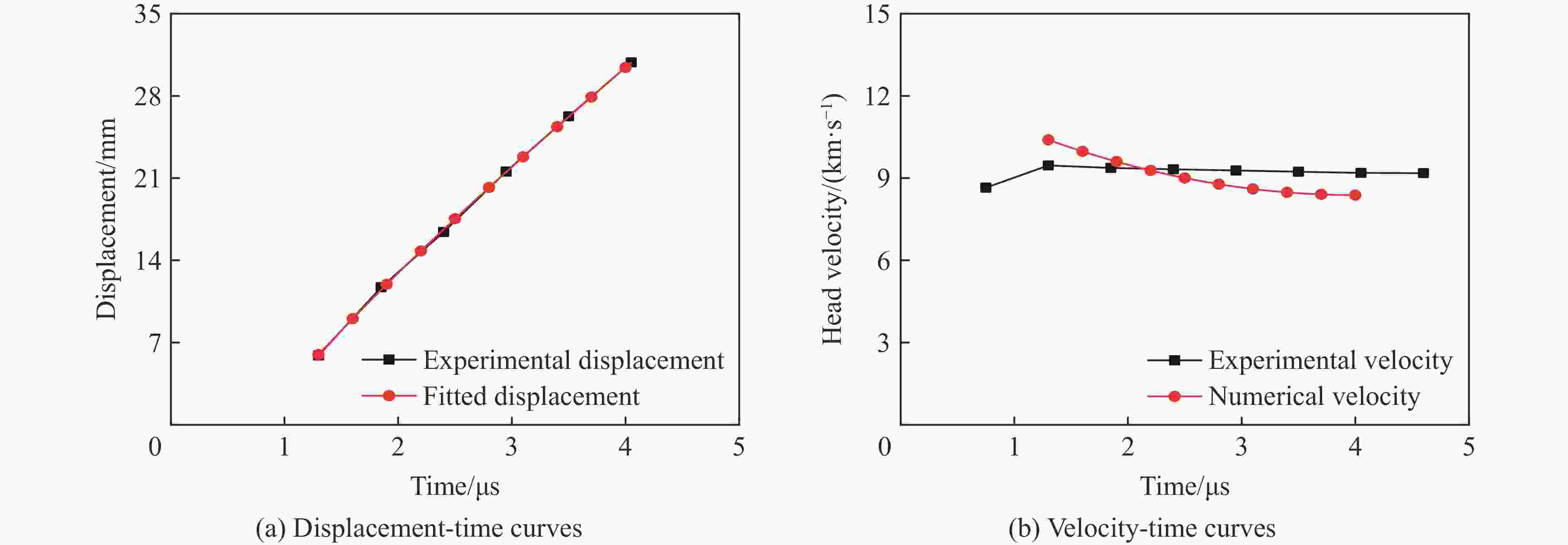
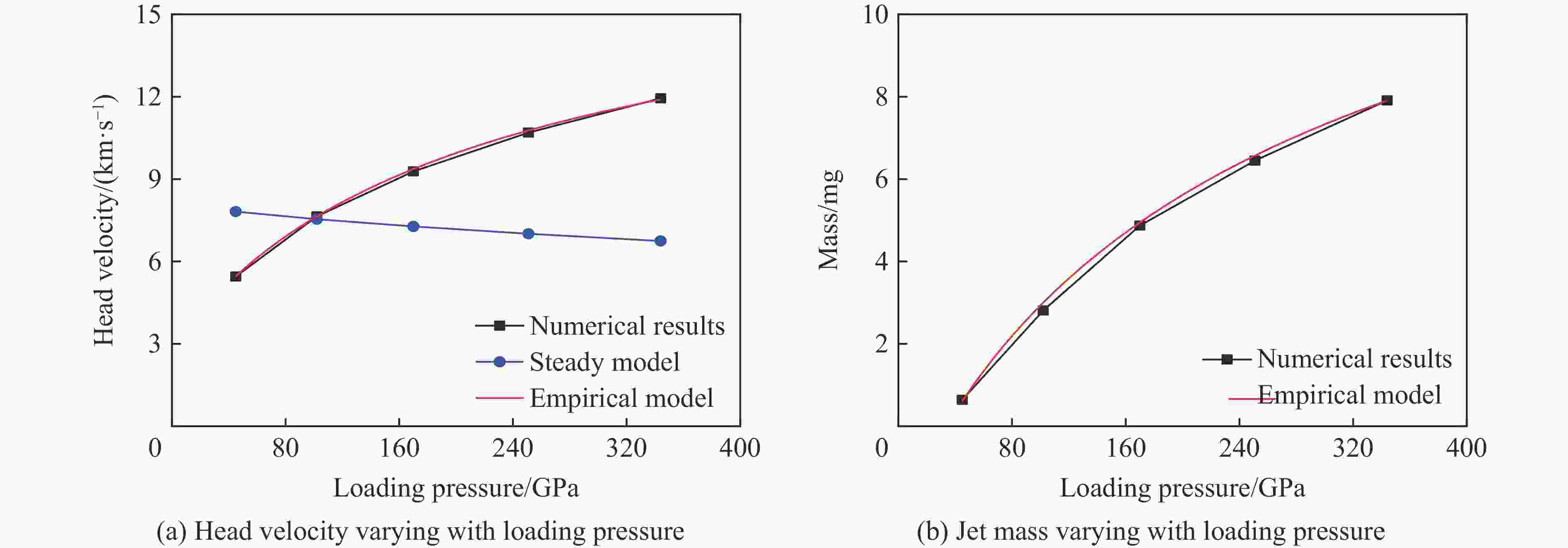
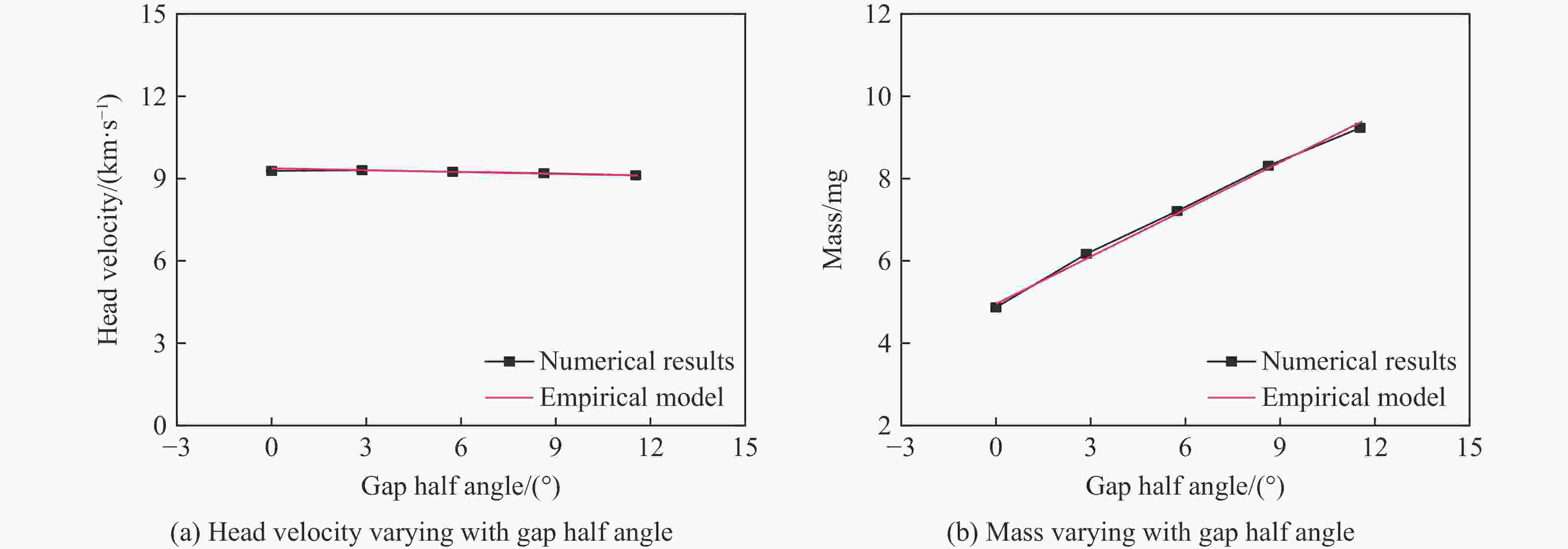
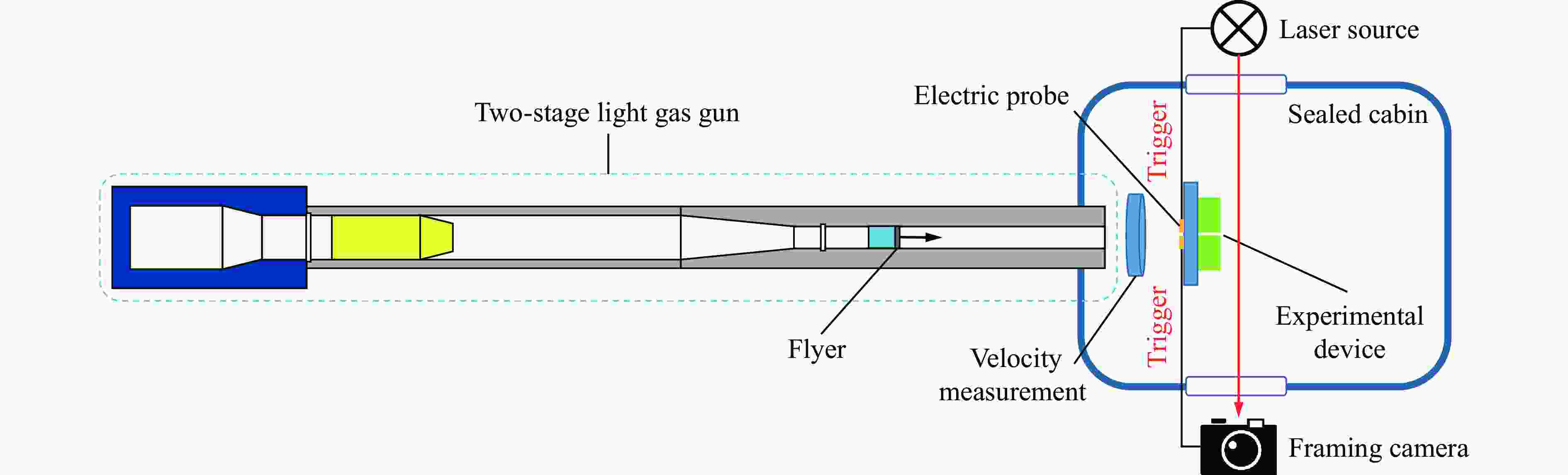
摘要: 加工与装配的公差导致间隙在工程结构中广泛存在，强动载下间隙内可能产生威胁结构可靠性与安全性的间隙射流，为了深入了解间隙射流的形成过程和形成机制，基于二级轻气炮对带有间隙的金属钨样品开展了超高速冲击加载实验，通过高速分幅照相系统记录了间隙射流的形成及演化过程。采用ANSYS Autodyn软件建立了预测间隙射流形成的数值模型，并基于代表性实验获取的射流形态及头部速度历史验证了该数值模型的适用性。通过调整数值模型中的飞片速度、间隙宽度和间隙半角，分别研究了这三者对间隙射流形成的影响，分析了定常射流模型的局限性。在此基础上，结合数值模拟结果，提出了预测间隙射流头部速度和质量的经验模型。研究表明，基于欧拉方法建立的数值模型能够较准确地预测强动载下间隙射流的形成。加载压力是控制射流头部速度和质量的主要因素，随着加载压力的提高，射流头部速度和质量也相应增大。间隙宽度和间隙半角对射流头部速度的影响较小，但间隙射流质量随间隙宽度和间隙半角的增大呈线性增大。由于间隙闭合速度的估算存在较大误差，定常射流模型未能准确预测间隙射流的形成，而所提出的经验模型与数值模拟结果具有较高的吻合度。Abstract: Tolerances in machining and assembly often result in gaps within engineering structures. Under strong dynamic loading, gap jets may form within these gaps, thereby posing a threat to the reliability and safety of the structure. However, the formation mechanism of gap jets differs from that of traditional high-speed metal jets, and its formation process still requires systematic study. Hypervelocity impact loading experiments on tungsten samples with gaps were conducted using a two-stage light gas gun, and the formation and evolution of the gap jet were recorded using a high-speed framing camera. A numerical model for predicting the formation of gap jets was established using ANSYS Autodyn, and the applicability of the numerical simulation method was validated by comparing the numerical results with the jet morphology and head velocity history data obtained from a representative experiment. The effects of flyer velocity, gap width, and gap half-angle on the formation of the gap jet were investigated by adjusting these parameters in the numerical model, and the variations in the gap jet head velocity and mass with respect to these factors were obtained. The limitations of the steady-state jet model were analyzed, and an empirical model was developed to predict the jet head velocity and mass based on the findings from numerical simulations. The results show that the numerical model based on the Eulerian method can accurately predict the formation of the gap jet under strong dynamic loading. Loading pressure is found to be the main factor controlling the jet head velocity and mass; as the loading pressure increases, both the jet head velocity and mass increase accordingly. The gap width and half-angle have little effect on the jet head velocity, but the mass increases linearly with the gap width and half-angle. Due to significant errors in estimating the gap closing velocity, the steady jet model fails to accurately predict the formation of the gap jet. In contrast, the developed empirical model shows good agreement with the numerical results.
-
Key words:
- hypervelocity collision /
- high-speed jet /
- loading pressure /
- gap width /
- gap half-angle
-
图 1 实验加载系统及诊断布局示意图
Figure 1. Schematic diagram of experimental loading system and diagnostic layout
图 4 间隙射流形成的实验图像（飞片速度为3 km/s，间隙宽度为0.2 mm）
Figure 4. Experimental images of gap jet formation (flyer velocity is 3 km/s, gap width is 0.2 mm)
图 5 间隙射流形成的模拟结果（飞片速度为3 km/s，间隙宽度为0.2 mm）
Figure 5. Numerical results of gap jet formation (flyer velocity is 3 km/s, gap width is 0.2 mm)
图 6 实验中位移-时间历程的拟合与实验及仿真中间隙射流头部速度的对比（飞片速度为3 km/s，间隙宽度为0.2 mm）
Figure 6. Fitting of displacement-time history in experiments and comparison of gap jet head velocity between experiment and simulation (flyer velocity is 3 km/s, gap width is 0.2 mm)
图 7 2.95 µs时间隙射流沿间隙方向的速度梯度分布（飞片速度为3 km/s，间隙宽度为0.2 mm）
Figure 7. Velocity gradient distribution of gap jet along the gap direction at 2.95 µs (flyer velocity is 3 km/s, gap width is 0.2 mm)
图 8 不同加载压力下形成的间隙射流（间隙宽度为0.2 mm）
Figure 8. Gap jets formed under different loading pressures (gap width is 0.2 mm)
图 9 不同间隙宽度下形成的间隙射流（飞片速度为3 km/s）
Figure 9. Gap jets formed under different gap widths (flyer velocity is 3 km/s)
图 10 不同间隙半角下形成的间隙射流（飞片速度为3 km/s）
Figure 10. Gap jet formed under different gap half angles (flyer velocity is 3 km/s)
图 11 加载压力对间隙射流头部速度和质量的影响（间隙宽度is 0.2 mm）
Figure 11. Effects of loading pressure on head velocity and mass of gap jet (gap width is 0.2 mm)
图 12 不同加载压力下射流在间隙内的加速历程（间隙宽度is 0.2 mm）
Figure 12. Acceleration process of jet within the gap under different loading pressures (gap width is 0.2 mm)
图 13 间隙宽度对间隙射流头部速度和质量的影响（飞片速度为3 km/s）
Figure 13. Effects of gap width on head velocity and mass of gap jet (flyer velocity is 3 km/s)
图 14 间隙半角对间隙射流头部速度和质量的影响（飞片速度为3 km/s）
Figure 14. Effects of gap half angle on head velocity and mass of gap jet (flyer velocity is 3 km/s)
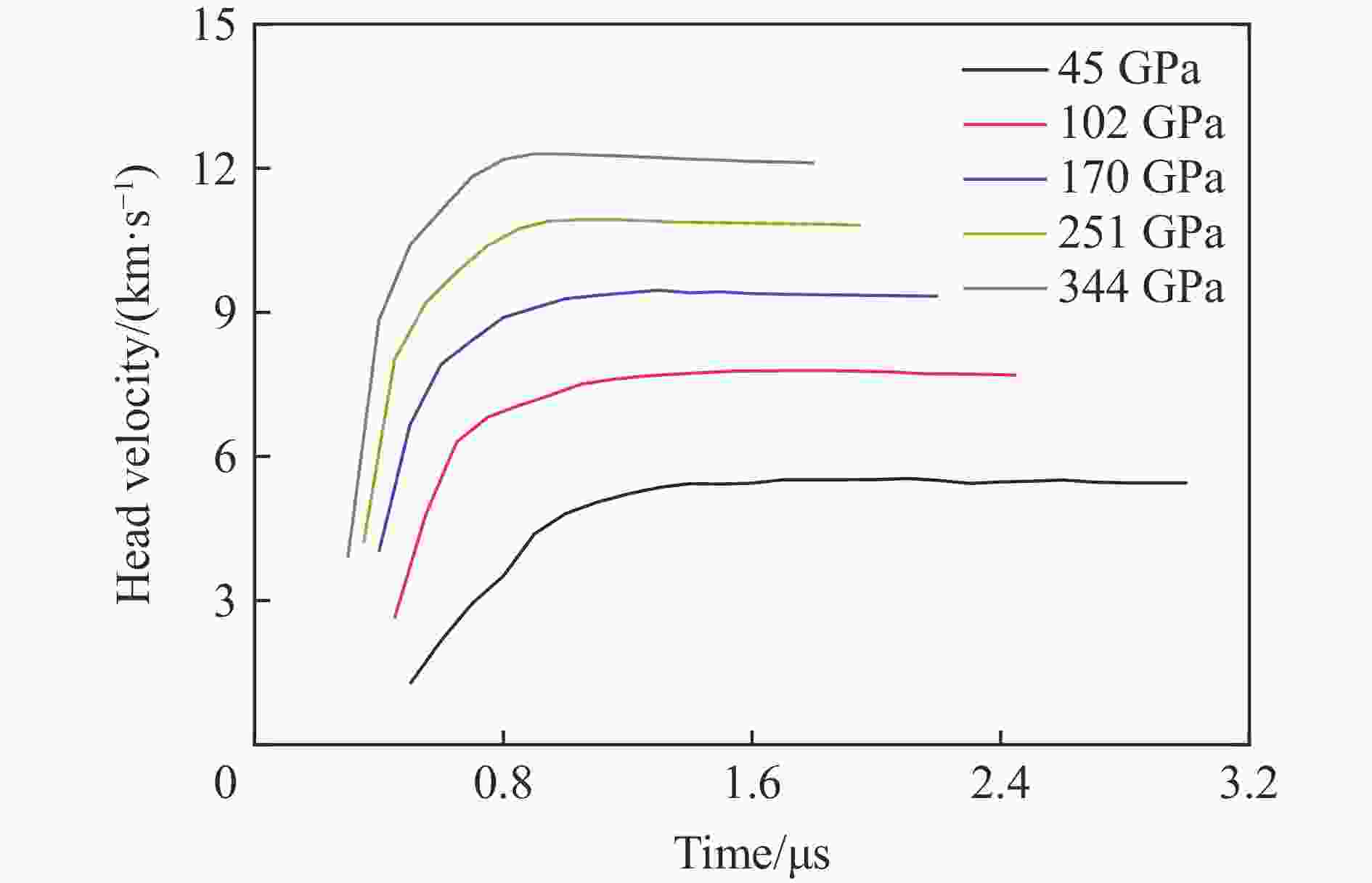
表 1 不同加载压力下间隙射流的相关参数
Table 1. Parameters of gap jets under different loading pressures
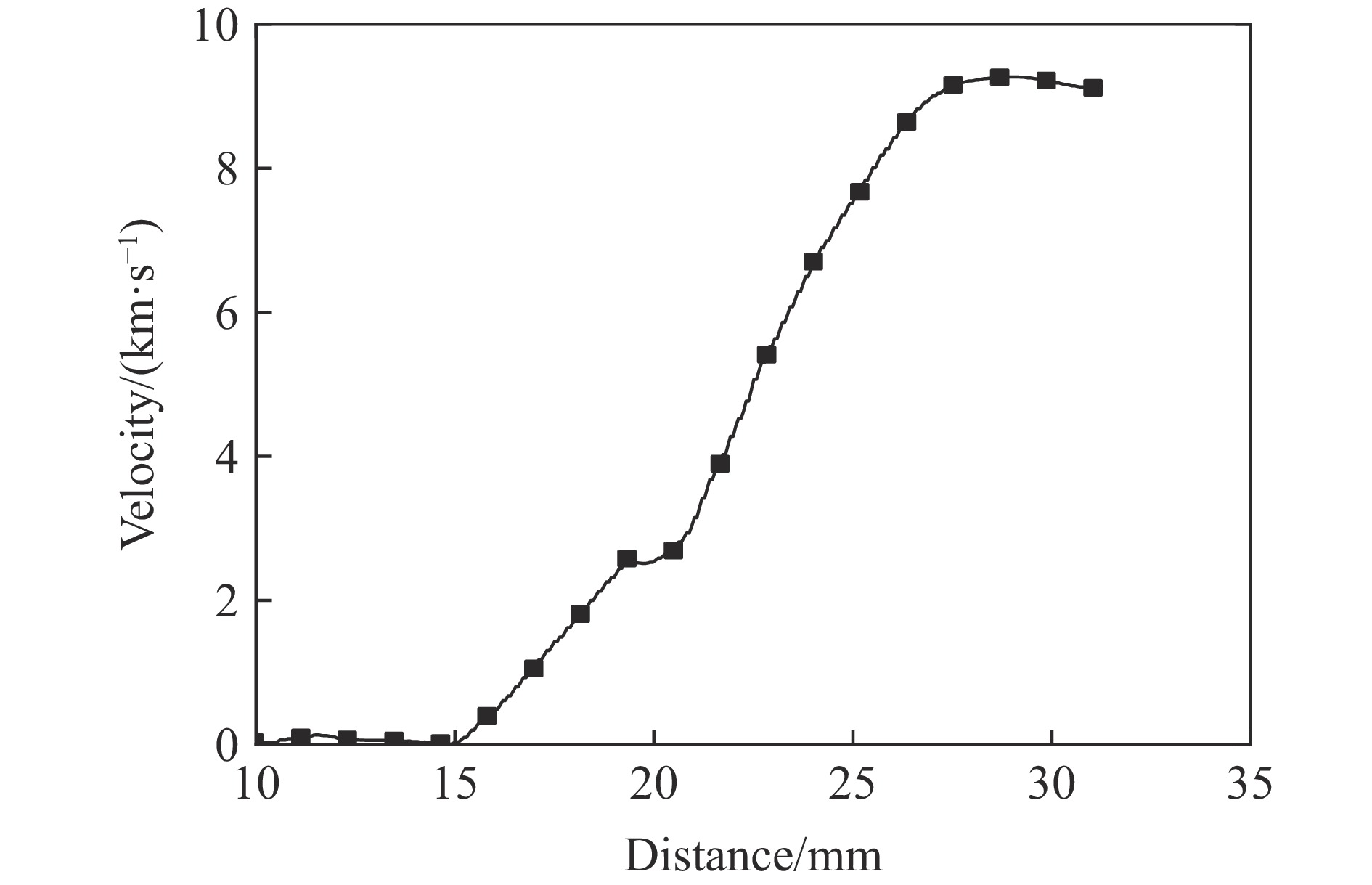
v0/(km·s−1) p/GPa u/(km·s−1) D/(km·s−1) θ/(°) 1.00 45.00 0.50 4.65 5.31 2.00 102.00 1.00 5.27 3.01 3.00 170.00 1.50 5.89 2.25 4.00 251.00 2.00 6.50 1.86 5.00 344.00 2.50 7.12 1.63 
下载: 导出CSV
表 2 不同间隙宽度下间隙射流的相关参数
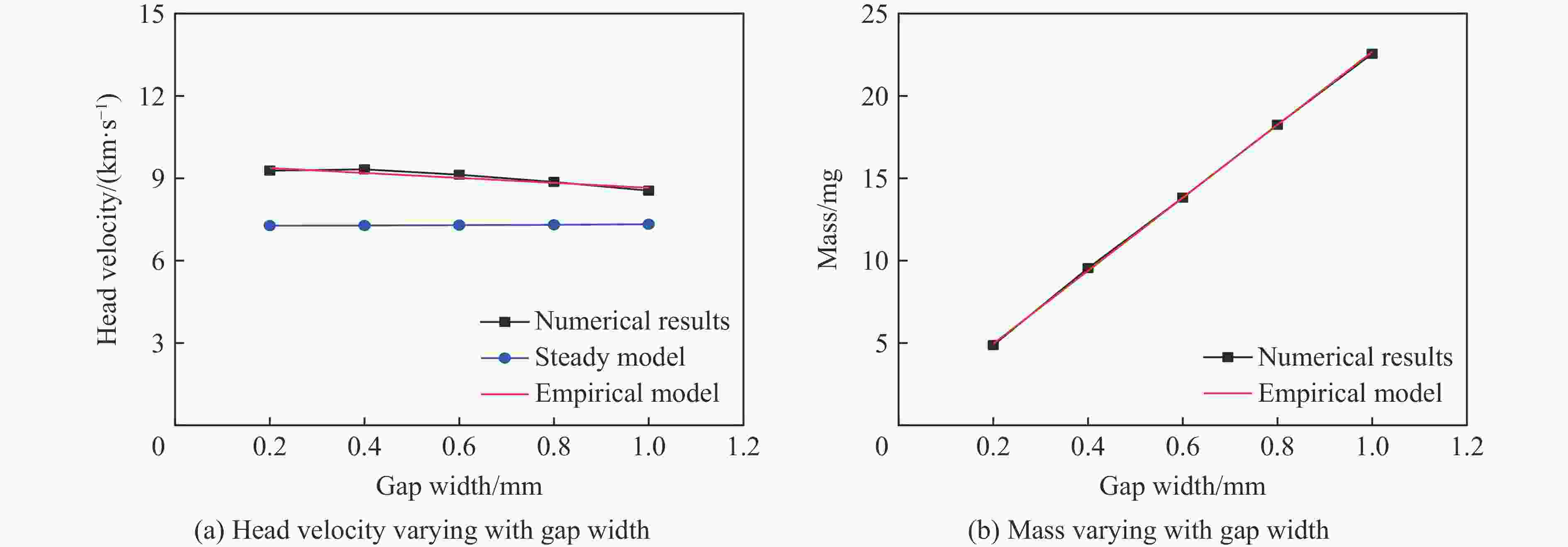
Table 2. Parameters of gap jets under different gap widths
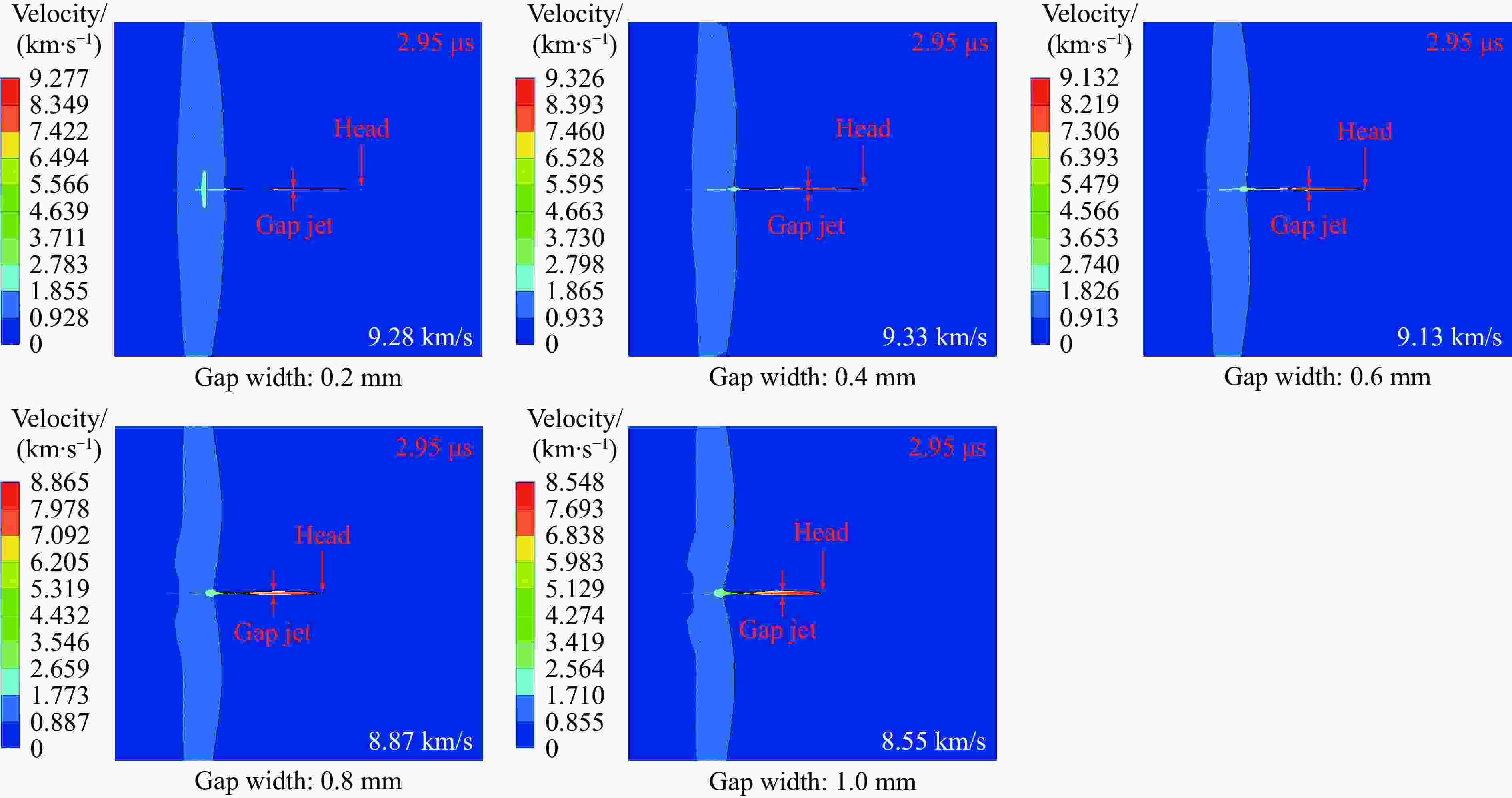
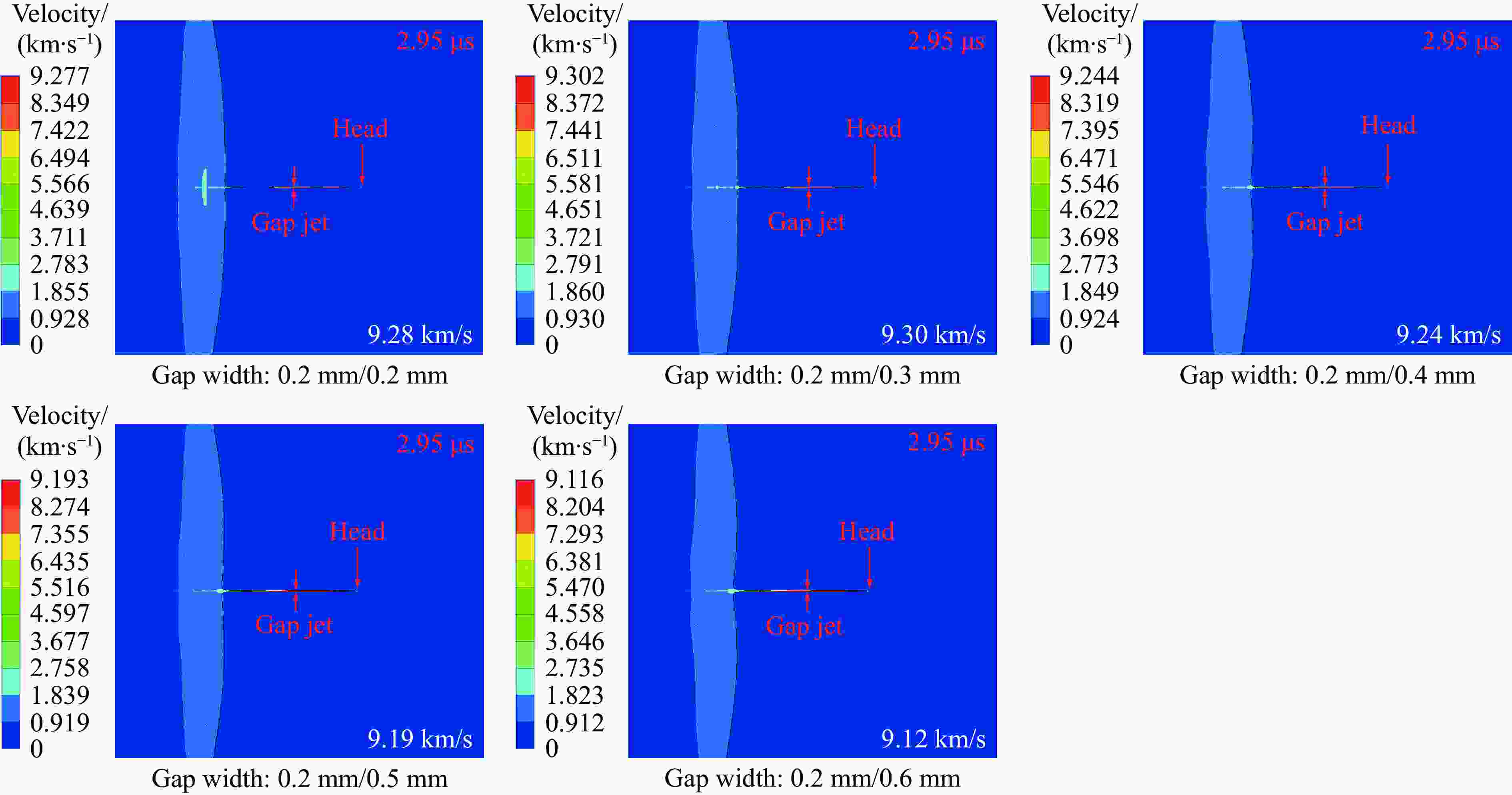
w/mm u/(km·s−1) D/(km·s−1) θ/(°) 0.2 1.50 5.89 2.25 0.4 1.50 5.89 4.49 0.6 1.50 5.89 6.71 0.8 1.50 5.89 8.92 1.0 1.50 5.89 11.10
下载: 导出CSV
-
[1] BUTTLER W T, ORÓ D M, PRESTON D L, et al. Unstable Richtmyer-Meshkov growth of solid and liquid metals in vacuum [J]. Journal of Fluid Mechanics, 2012, 703: 60–84. DOI: 10.1017/jfm.2012.190. [2] DIMONTE G, TERRONES G, CHERNE F J, et al. Ejecta source model based on the nonlinear Richtmyer-Meshkov instability [J]. Journal of Applied Physics, 2013, 113(2): 024905. DOI: 10.1063/1.4773575. [3] LIU Y, GRIEVES B. Ejecta production and transport from a shocked Sn coupon [J]. Journal of Fluids Engineering, 2014, 136(9): 091202. DOI: 10.1115/1.4026513. [4] REN G W, CHEN Y T, TANG T G, et al. Ejecta production from shocked Pb surface via molecular dynamics [J]. Journal of Applied Physics, 2014, 116(13): 133507. DOI: 10.1063/1.4896902. [5] GUO H G, SU C H, CAI Y Q, et al. Reactive jet density distribution effect on its penetration behavior [J]. Defence Technology, 2023, 24: 190–202. DOI: 10.1016/j.dt.2022.03.001. [6] SHI J, HUANG Z X, ZU X D, et al. Experimental and numerical investigation of jet performance based on Johnson-Cook model of liner material [J]. International Journal of Impact Engineering, 2022, 170: 104343. DOI: 10.1016/j.ijimpeng.2022.104343. [7] XU W L, WANG C, CHEN D P. The jet formation and penetration capability of hypervelocity shaped charges [J]. International Journal of Impact Engineering, 2019, 132: 103337. DOI: 10.1016/j.ijimpeng.2019.103337. [8] 方一舟, 张先锋, 熊玮, 等. 考虑形状分布特性的聚能射流侵彻作用规律研究 [J]. 北京理工大学学报, 2023, 43(10): 1047–1058. DOI: 10.15918/j.tbit1001-0645.2023.075.FANG Y Z, ZHANG X F, XIONG W, et al. Study on penetration law of shaped charge jet considering shape distribution characteristics [J]. Transactions of Beijing Institute of Technology, 2023, 43(10): 1047–1058. DOI: 10.15918/j.tbit1001-0645.2023.075. [9] 李干, 陈小伟. 聚能射流侵彻径向扩孔的可压缩模型 [J]. 爆炸与冲击, 2022, 42(7): 073301. DOI: 10.11883/bzycj-2021-0466.LI G, CHEN X W. A compressible model of radial crater growth by shaped-charge jet penetration [J]. Explosion and Shock Waves, 2022, 42(7): 073301. DOI: 10.11883/bzycj-2021-0466. [10] DE RESSÉGUIER T, PRUDHOMME G, ROLAND C, et al. Picosecond x-ray radiography of microjets expanding from laser shock-loaded grooves [J]. Journal of Applied Physics, 2018, 124(6): 065106. DOI: 10.1063/1.5040304. [11] TOKHEIM R E, CURRAN D R, SEAMAN L, et al. Hypervelocity shrapnel damage assessment in the NIF target chamber [J]. International Journal of Impact Engineering, 1999, 23(1): 933–944. DOI: 10.1016/S0734-743X(99)00136-0. [12] LIU J, SUN Z Y, FAN Z F, et al. Theoretical model of metal gap ejection under strong impact loading and its verification [J]. International Journal of Impact Engineering, 2022, 159: 104053. DOI: 10.1016/j.ijimpeng.2021.104053. [13] 童慧峰, 李庆忠, 谷岩, 等. 爆炸加载下金属缝隙射流定量诊断实验研究 [J]. 爆炸与冲击, 2016, 36(5): 590–595. DOI: 10.11883/1001-1455(2016)05-0590-06.TONG H F, LI Q Z, GU Y, et al. Experimental study of quantitative diagnosis of metal crack jet under explosive load [J]. Explosion and Shock Waves, 2016, 36(5): 590–595. DOI: 10.11883/1001-1455(2016)05-0590-06. [14] ASAY J R, MIX L P, PERRY F C. Ejection of material from shocked surfaces [J]. Applied Physics Letters, 1976, 29(5): 284–287. DOI: 10.1063/1.89066. [15] HE A M, LIU J, LIU C, et al. Numerical and theoretical investigation of jet formation in elastic-plastic solids [J]. Journal of Applied Physics, 2018, 124(18): 185902. DOI: 10.1063/1.5051527. [16] LIU W B, MA D J, HE A M, et al. Ejecta from periodic grooved Sn surface under unsupported shocks [J]. Chinese Physics B, 2018, 27(1): 016202. DOI: 10.1088/1674-1056/27/1/016202. [17] ROLAND C, DE RESSÉGUIER T, SOLLIER A, et al. Hydrodynamic simulations of microjetting from shock-loaded grooves [J]. AIP Conference Proceedings, 2017, 1793(1): 100027. DOI: 10.1063/1.4971652. [18] MESHKOV E E. Instability of the interface of two gases accelerated by a shock wave [J]. Fluid Dynamics, 1969, 4(5): 101–104. DOI: 10.1007/BF01015969. [19] RICHTMYER R D. Taylor instability in shock acceleration of compressible fluids [J]. Communications on Pure and Applied Mathematics, 1960, 13(2): 297–319. DOI: 10.1002/cpa.3160130207. [20] DE RESSÉGUIER T, LESCOUTE E, SOLLIER A, et al. Microjetting from grooved surfaces in metallic samples subjected to laser driven shocks [J]. Journal of Applied Physics, 2014, 115(4): 043525. DOI: 10.1063/1.4863719. [21] ZELLNER M B, GROVER M, HAMMERBERG J E, et al. Effects of shock-breakout pressure on ejection of micron-scale material from shocked tin surfaces [J]. Journal of Applied Physics, 2007, 102(1): 013522. DOI: 10.1063/1.2752130. [22] SHI J, HUANG Z X, ZU X D, et al. Cohesiveness and penetration performance of jet: theoretical, numerical, and experimental studies [J]. International Journal of Impact Engineering, 2023, 175: 104543. DOI: 10.1016/j.ijimpeng.2023.104543. [23] 张斐, 张春辉, 张磊, 等. 辅助药型罩材料对截顶药型罩形成高速射流的影响 [J]. 兵工学报, 2018, 39(S1): 52–56. DOI: 10.3969/j.issn.1000-1093.2018.S1.008.ZHANG F, ZHANG C H, ZHANG L, et al. Effect of materials of auxiliary liner on the formation of high-speed jet by truncated liner [J]. Acta Armamentarii, 2018, 39(S1): 52–56. DOI: 10.3969/j.issn.1000-1093.2018.S1.008. -
计量
- 文章访问数: 93
- HTML全文浏览量: 14
- PDF下载量: 28
- 被引次数: 0