有限长锥体诱导的斜爆轰波非定常结构的数值研究

刘江; 归明月; 张道平; 董刚

doi:10.11883/bzycj-2024-0356

有限长锥体诱导的斜爆轰波非定常结构的数值研究

doi: 10.11883/bzycj-2024-0356

南京理工大学瞬态物理全国重点实验室，江苏南京 210094

详细信息

作者简介:
刘　江（1999－　），男，硕士研究生，ljiang926@163.com

通讯作者:
归明月（1977－　），男，博士，副研究员，mygui@njust.edu.cn

中图分类号: O382
计量
- 文章访问数: 83
- HTML全文浏览量: 19
- PDF下载量: 23
- 被引次数: 0
出版历程
- 收稿日期: 2024-09-20
- 修回日期: 2025-04-02
- 网络出版日期: 2025-04-08

Numerical study on unsteady structure of oblique detonation wave induced by a finite cone

National Key Laboratory of Transient Physics, Nanjing University of Science and Technology, Nanjing 210094, Jiangsu, China

摘要

摘要: 基于开源代码OpenFOAM对有限长锥体诱导的斜爆轰波开展了数值模拟，并讨论了爆轰波后流场、波阵面结构和爆轰胞格结构。结果表明，在有限长锥体结构的影响下，爆轰波后流场先后受到Taylor-Maccoll流动和Prandtl-Meyer膨胀波的影响。爆轰波阵面不同位置流线上的压力和马赫数在这2种物理过程及波阵面三波结构的影响下，发生震荡变化，随后趋于稳定。在不同波后流场的影响下，爆轰波阵面结构呈现4种不同的结构：类Zel'dovich- Neumann-Döring (ZND)的光滑结构、类胞格的单三波点结构、胞格的双三波点结构和Prandtl-Meyer影响的双三波点结构。借助于激波极曲线理论对三波点附近的波系进行了分析，发现在双三波点结构中，面向来流的三波点具有比面向下游的三波点具有更强的爆轰强度，即更高的马赫数和压力。最后，结合上述分析，绘制了爆轰波阵面的三波点轨迹，得到4种不同的胞格结构：光滑平面结构、平行线结构、斜菱形结构和无规则的斜菱形结构。
- 有限长尖锥 /
- 斜爆轰波 /
- Taylor-Maccoll流动 /
- Prandtl-Meyer膨胀波 /
- 爆轰胞格
Abstract: Axisymmetric conical structures, as a common configuration, induce oblique detonation waves exhibiting significantly greater structural complexity compared to those generated by sharp wedges. Numerical simulations of oblique detonation waves induced by a finite cone were performed using the open-source code OpenFOAM, with analysis conducted on post-detonation flow fields, wavefront structure, and detonation cell structures. The numerical results show that under the effect of the finite cone the flow field behind the detonation wave is successively influenced by Taylor-Maccoll flow and Prandtl-Meyer expansion waves. The pressure and Mach number along the streamlines at different positions on the detonation wave front exhibit oscillatory changes with the influence of these two physical processes and triple points on oblique detonation surfaces, and then tend to stabilize. Depending on the different post-detonation flow field, the detonation wave front structure is divided into four sections: smooth ZND (Zel'dovich- Neumann-Döring)-like structure, single-headed triple points cell-like structure, dual-headed triple points cell structure and dual-headed triple point structure influenced by Prandtl-Meyer. The shock pole curve theory is used to analyze the wave structures. It is found that the upstream-facing triple points exhibits higher detonation intensity, i.e., higher Mach number and pressure, compared to the downstream-facing triple points in dual-headed triple points structure. Finally, based on the above analysis, triple point traces are recorded to obtain four different cell structures: smooth planar structure, parallel line structure, oblique rhombus structure, and irregular oblique rhombus structure.
- finite cone /
- oblique detonation wave /
- Taylor-Maccoll flow /
- Prandtl-Meyer expansion wave /
- detonation cell structure

HTML全文

图 1 计算区域示意图

Figure 1. Schematic of the computational domain

下载: 全尺寸图片幻灯片

图 2 自适应加密网格局部图

Figure 2. Localized view of the adaptive grids

下载: 全尺寸图片幻灯片

图 3 不同网格尺度下的密度云图

Figure 3. Density fields with different grid scales

下载: 全尺寸图片幻灯片

图 4 不同网格尺度下沿锥体表面的压力变化曲线

Figure 4. Pressure along the conical surface with different grid scales

下载: 全尺寸图片幻灯片

图 5 有限长锥体诱导的斜爆轰波密度云图

Figure 5. Density field of the oblique detonation structure induced by a finite cone

下载: 全尺寸图片幻灯片

图 6 沿锥体流线上的压力和马赫数变化曲线

Figure 6. Pressure and Mach number along the streamlines with cone

下载: 全尺寸图片幻灯片

图 7 AB段波阵面结构局部放大图与极曲线分析

Figure 7. Close-up view of wave front structure and polar curves in section AB

下载: 全尺寸图片幻灯片

图 8 BC段波阵面结构局部放大图与极曲线分析

Figure 8. Close-up view of wave front structure and polar curves in section BC

下载: 全尺寸图片幻灯片

图 9 CD段波阵面结构局部放大图与极曲线分析

Figure 9. Close-up view of wave front structure and polar curves in section CD

下载: 全尺寸图片幻灯片

图 10 Prandtl-Meyer流对斜爆轰波的影响区域

Figure 10. Region of influence of Prandtl-Meyer flow on oblique detonation waves

下载: 全尺寸图片幻灯片

图 11 DE段局部放大的密度云图

Figure 11. Close-up view of density field in section DE

下载: 全尺寸图片幻灯片

图 12 斜爆轰波的烟膜数值记录

Figure 12. Numerical smoke-foil record of oblique detonation waves

下载: 全尺寸图片幻灯片

表 1 初始条件和模型参数^[19]

Table 1. Initial conditions and model parameters^[19]

v₀/(m·s⁻¹) γ p₀/Pa T₀/K Q/(kJ·g⁻¹) E_a/(kJ·g⁻¹) m/(g·mol⁻¹) R_u/(J·K⁻¹·mol⁻¹)

7.5 1.3 101325 300 1.72 2.58 29 8.314

下载: 导出CSV

表 2 无量纲化初始条件与模型参数^[19]

Table 2. Non-dimensional initial conditions and model parameters^[19]

Ma₀ γ `p₀ `T₀ Q/(RT₀) E_a/(RT₀)

7.5 1.3 1 1 20 30

下载: 导出CSV

参考文献(29)

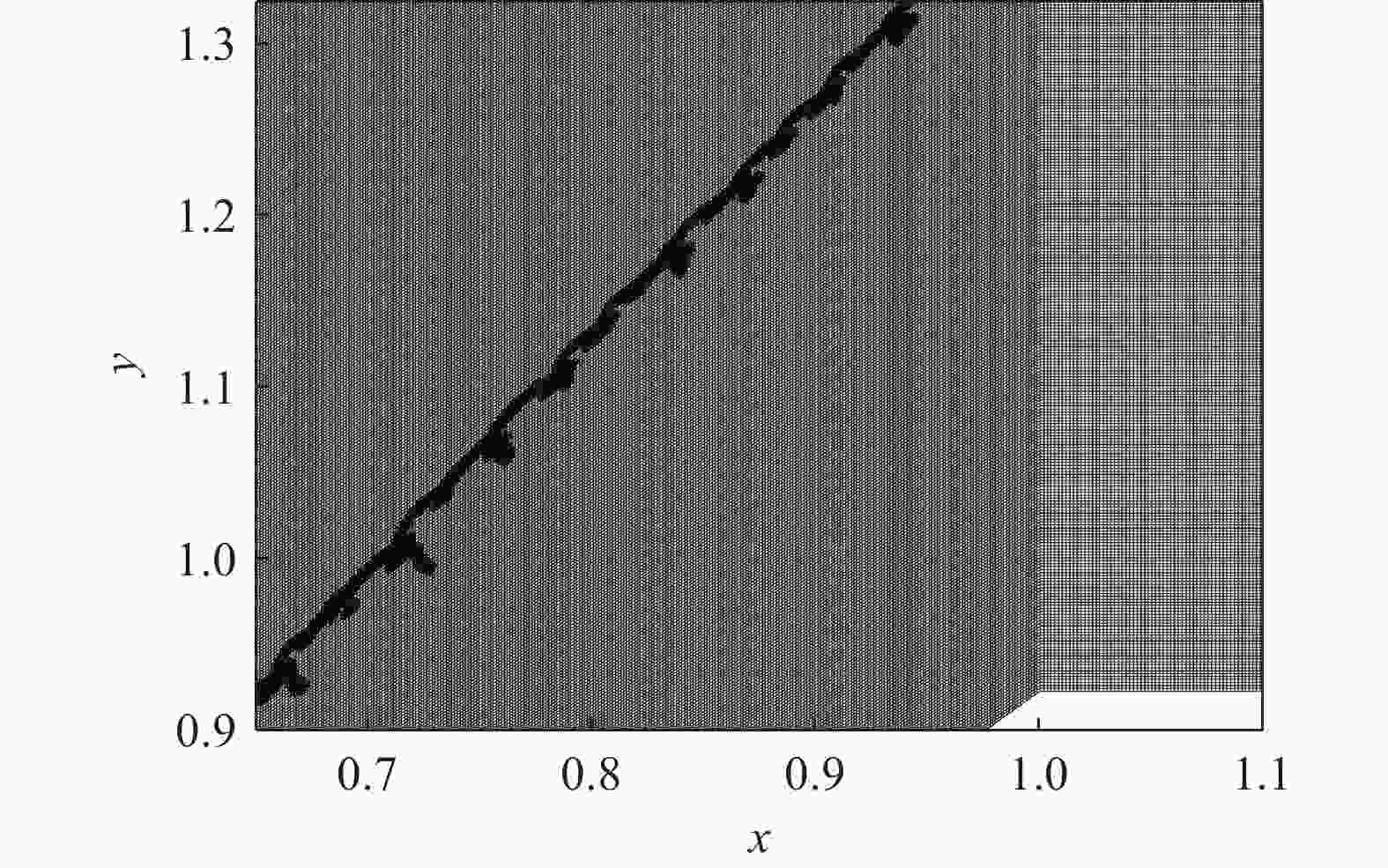
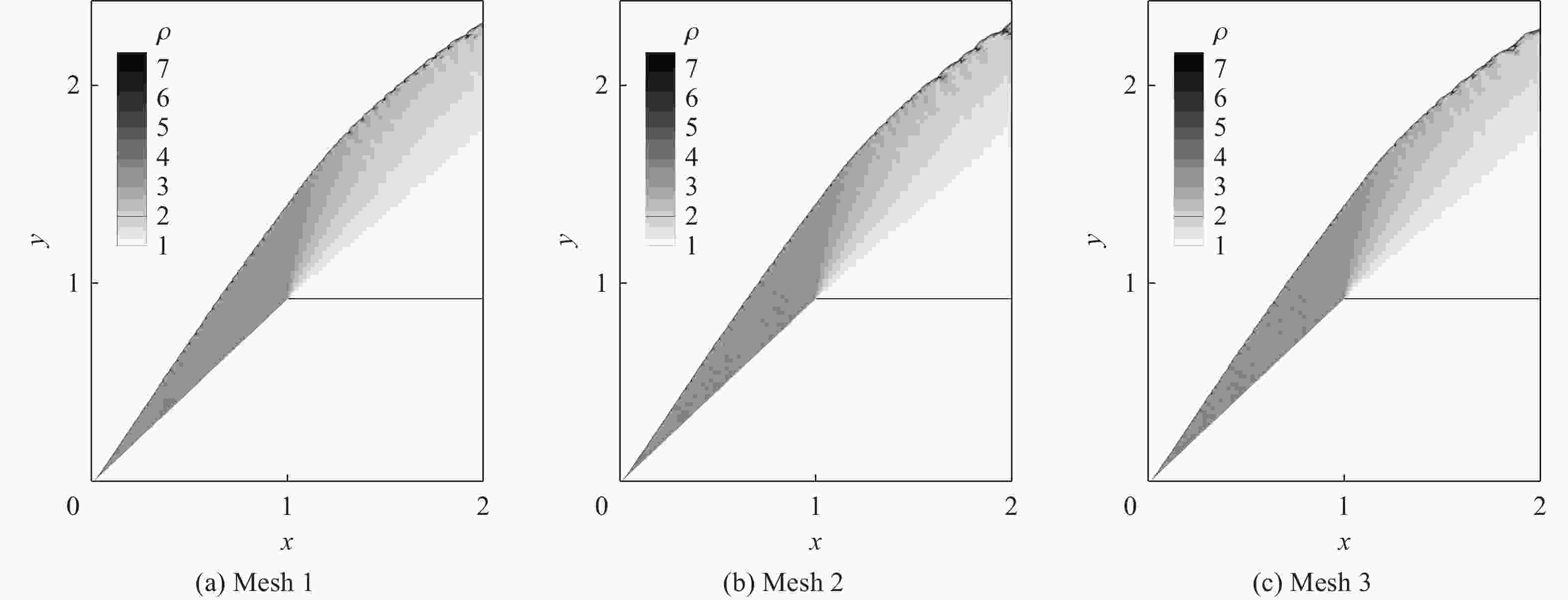
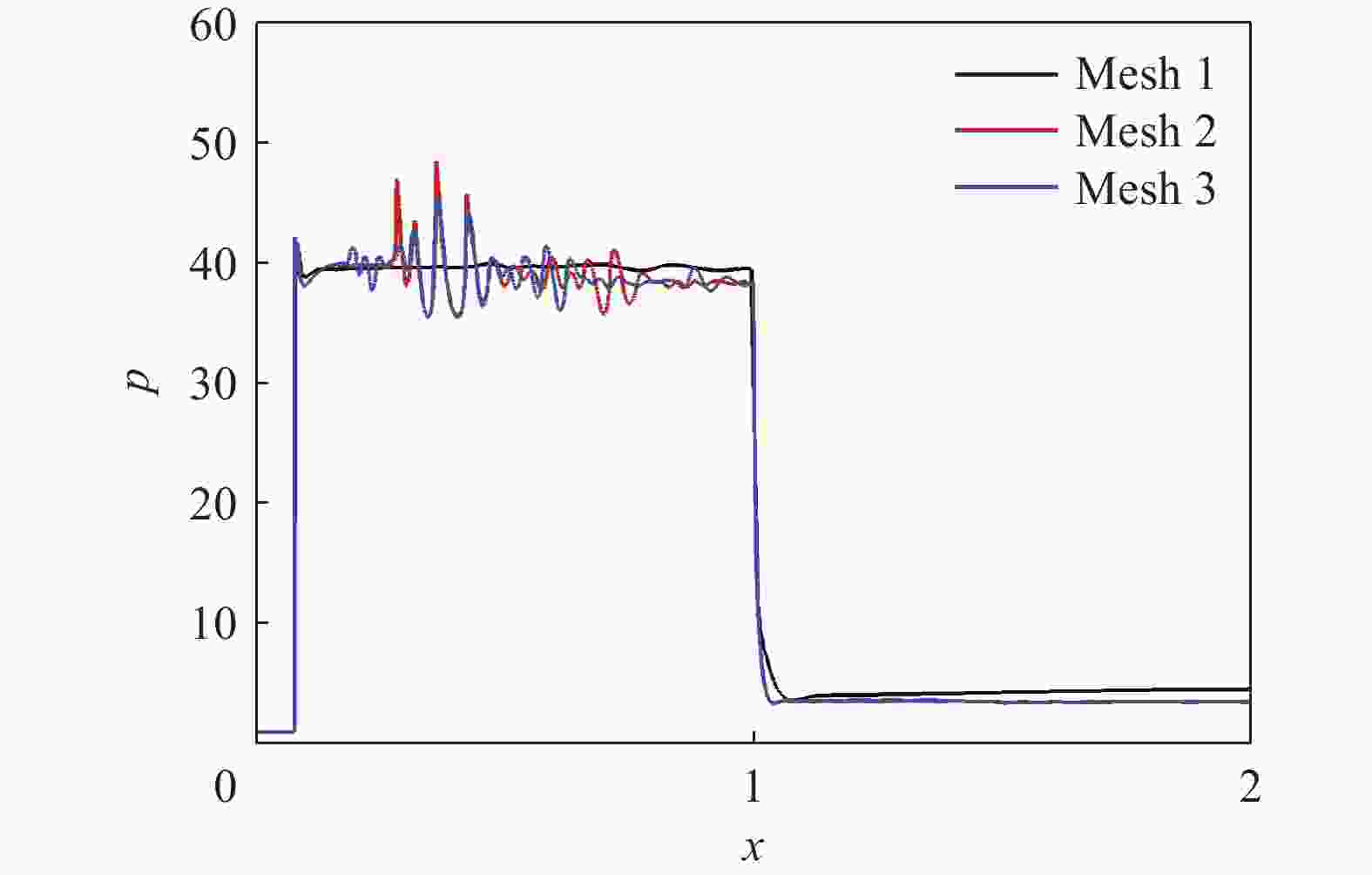
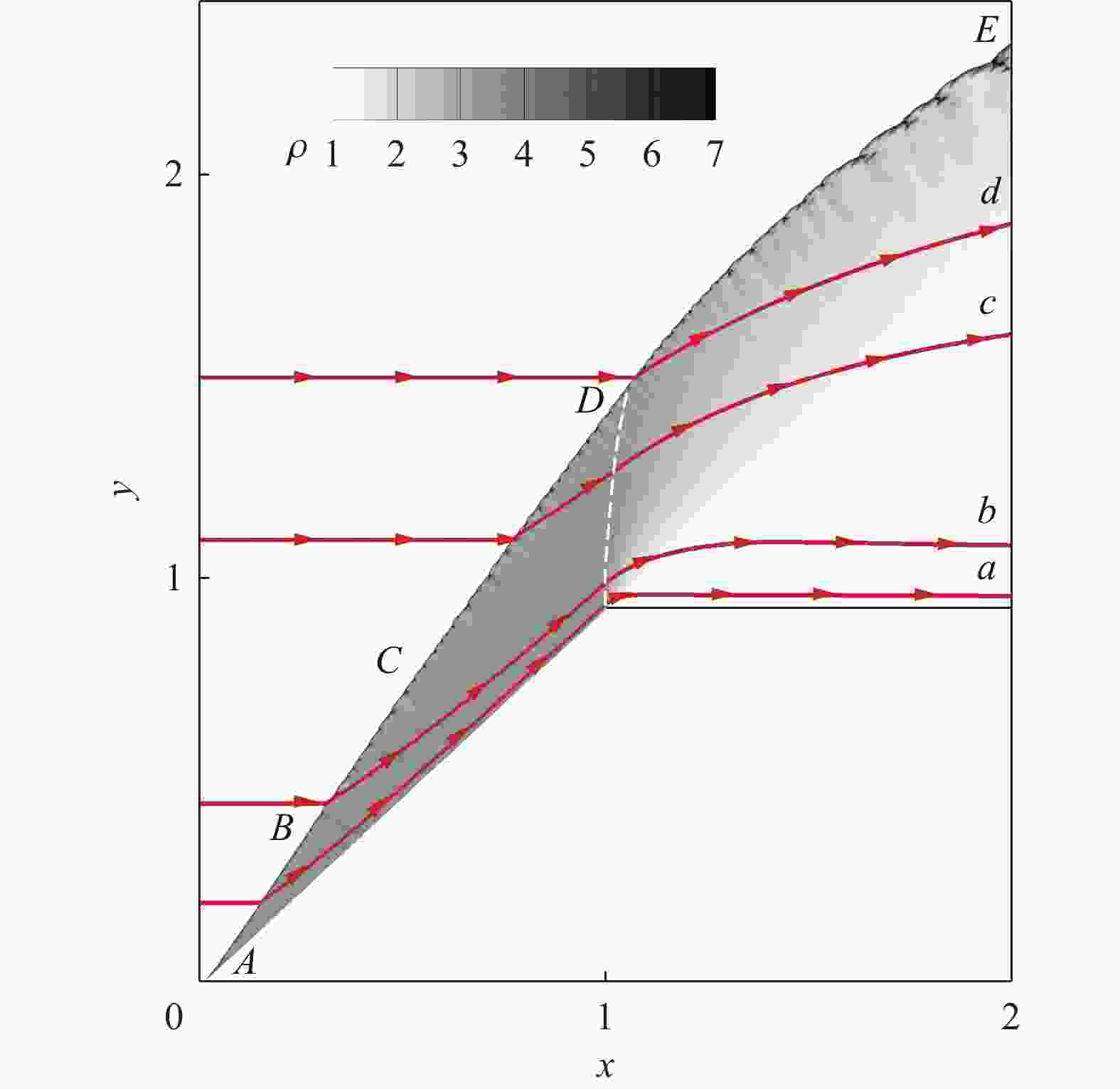
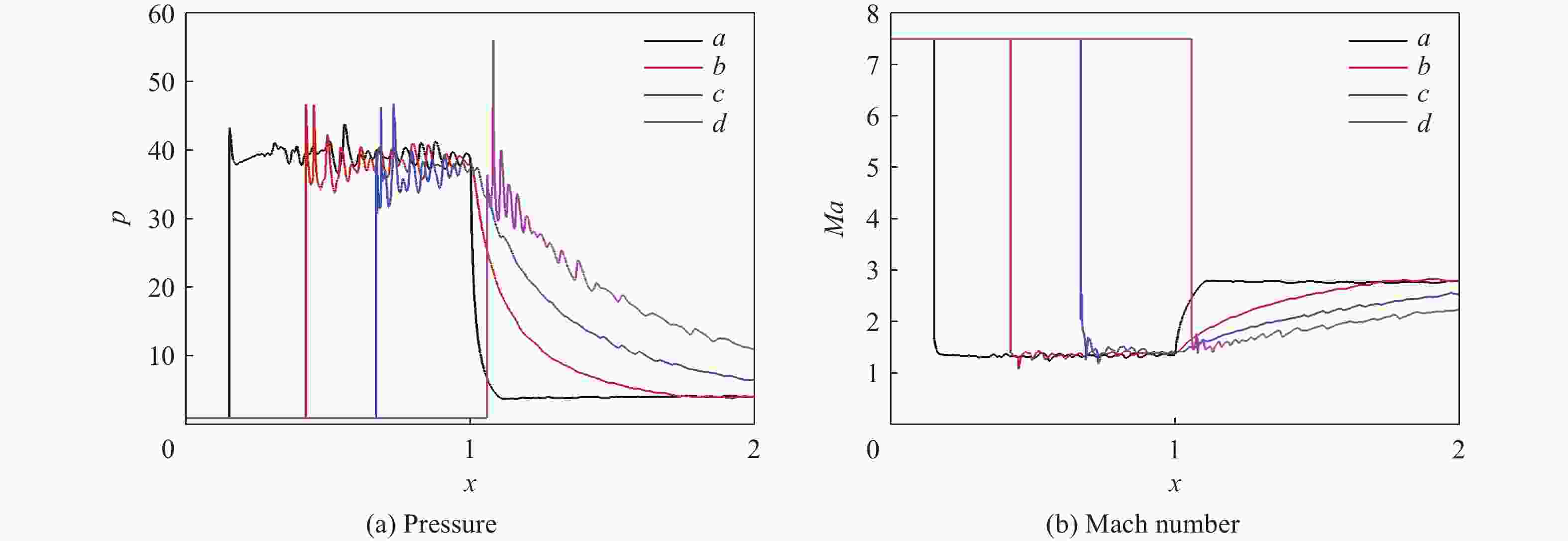
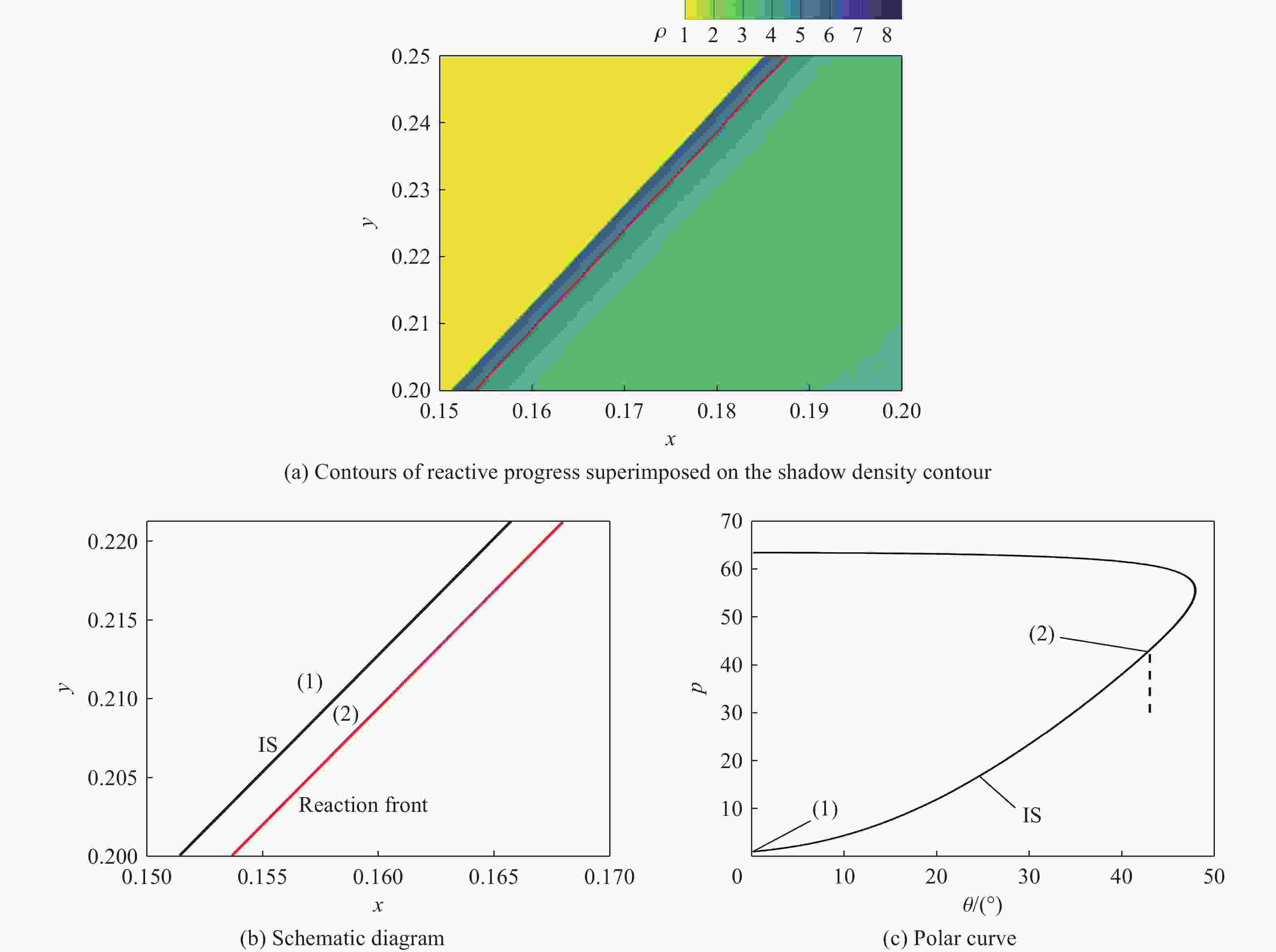
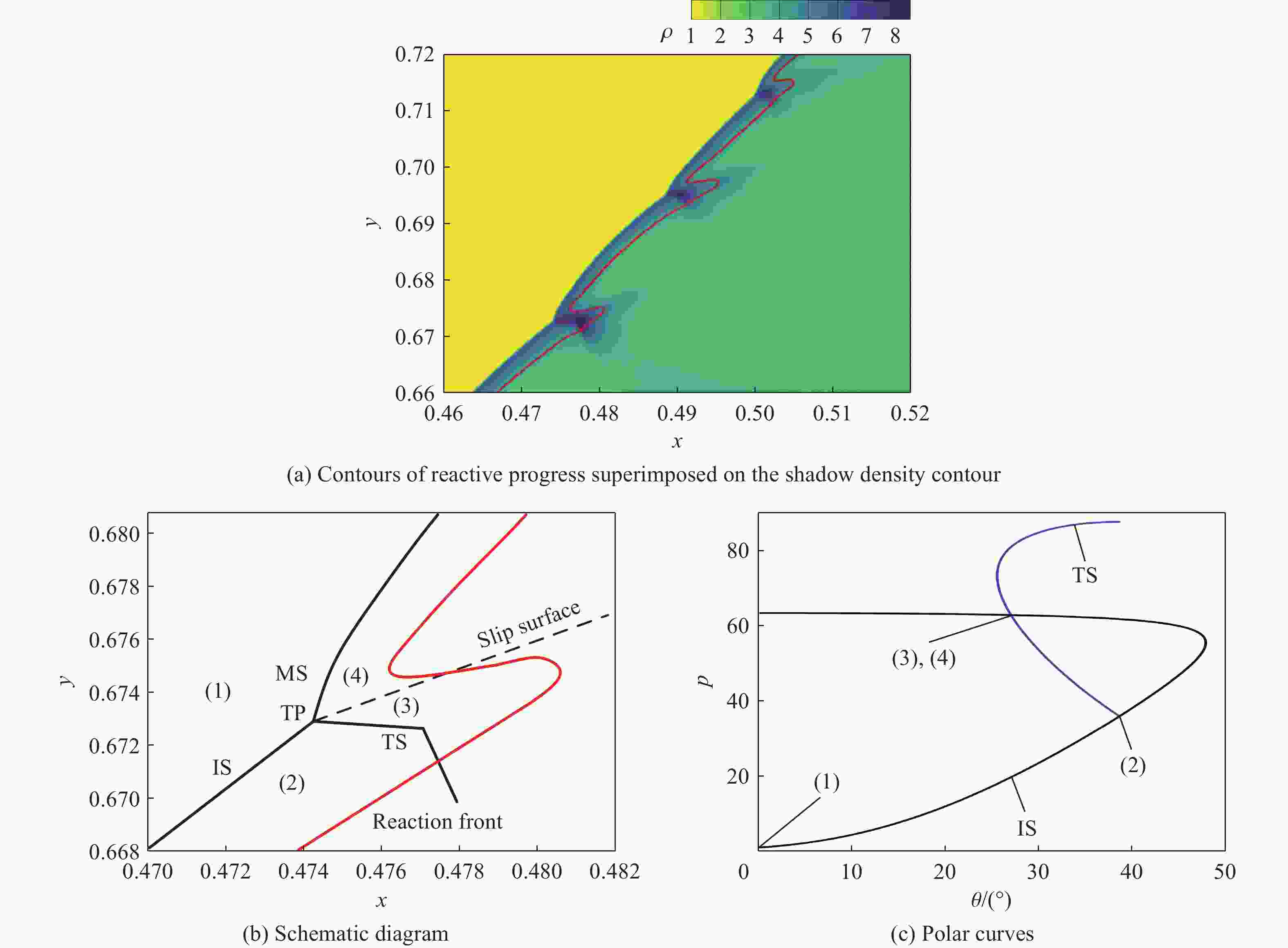
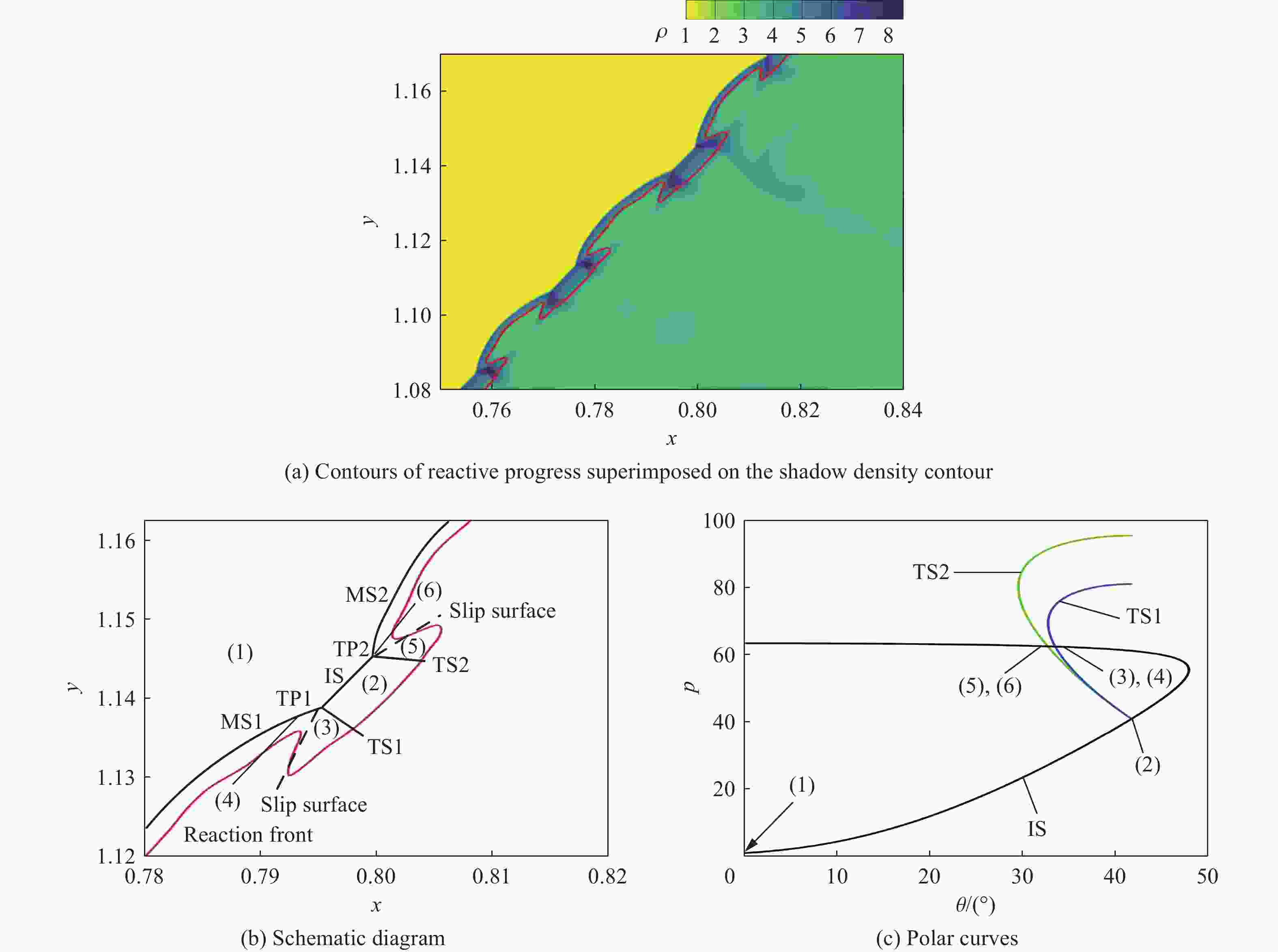
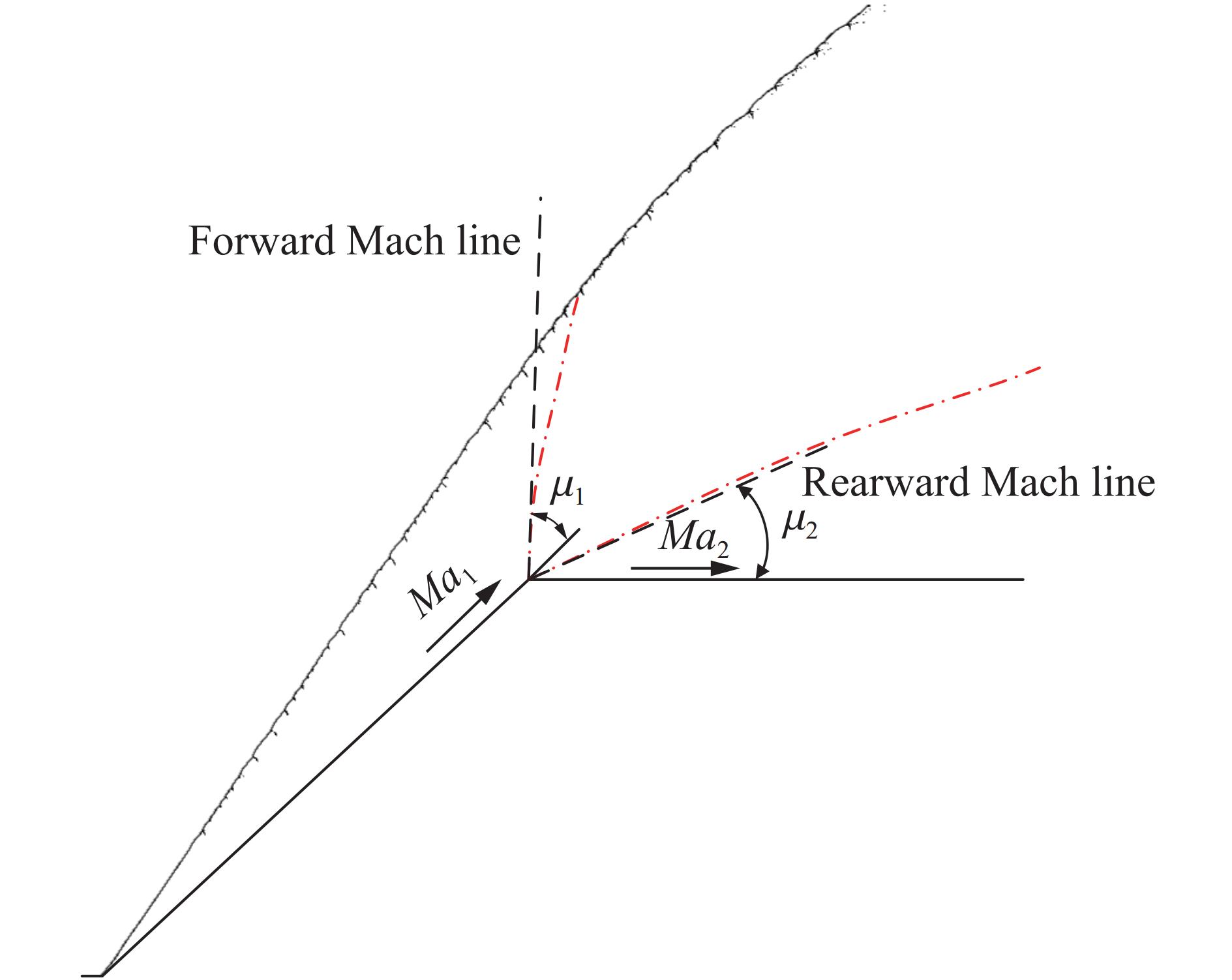
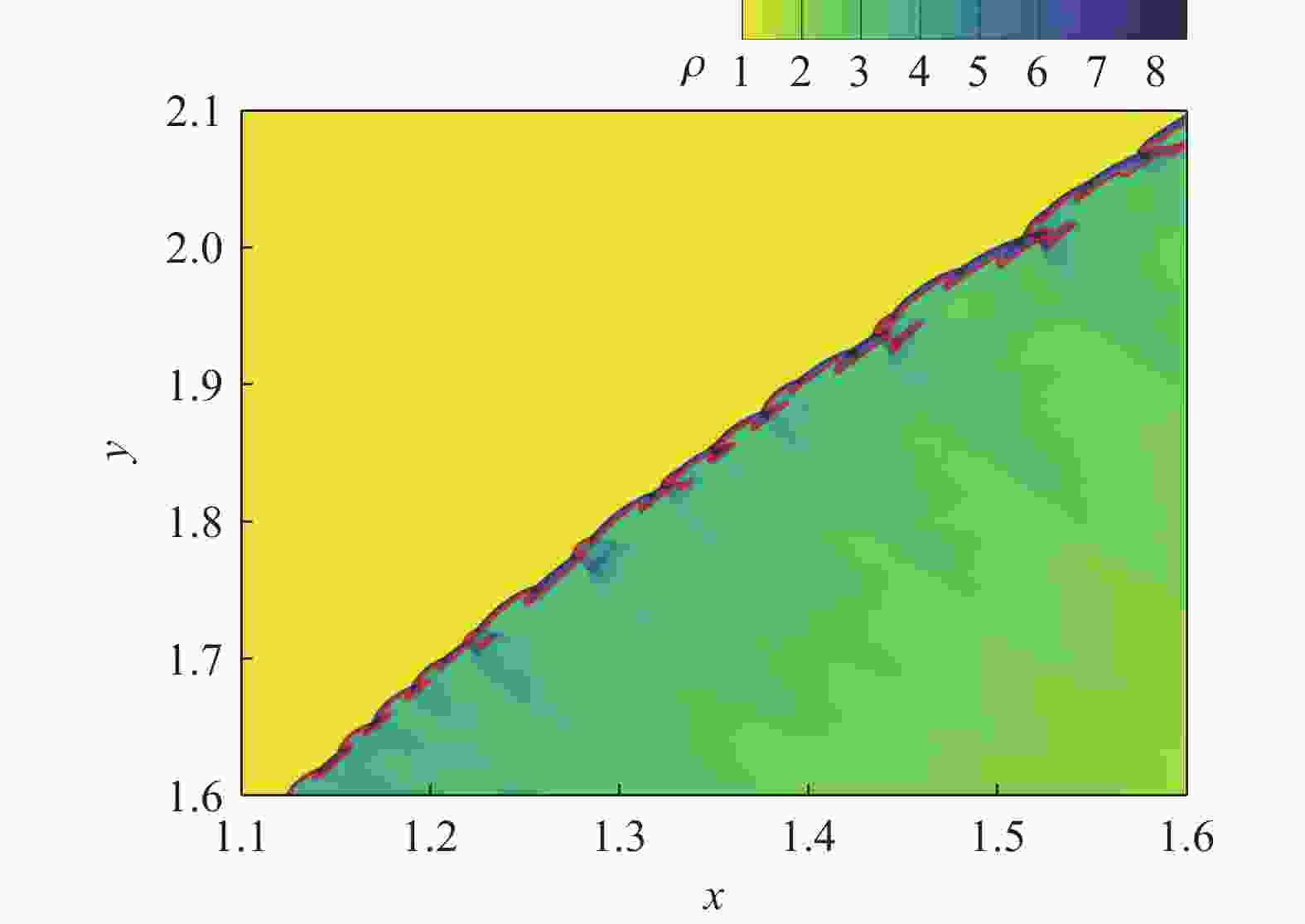
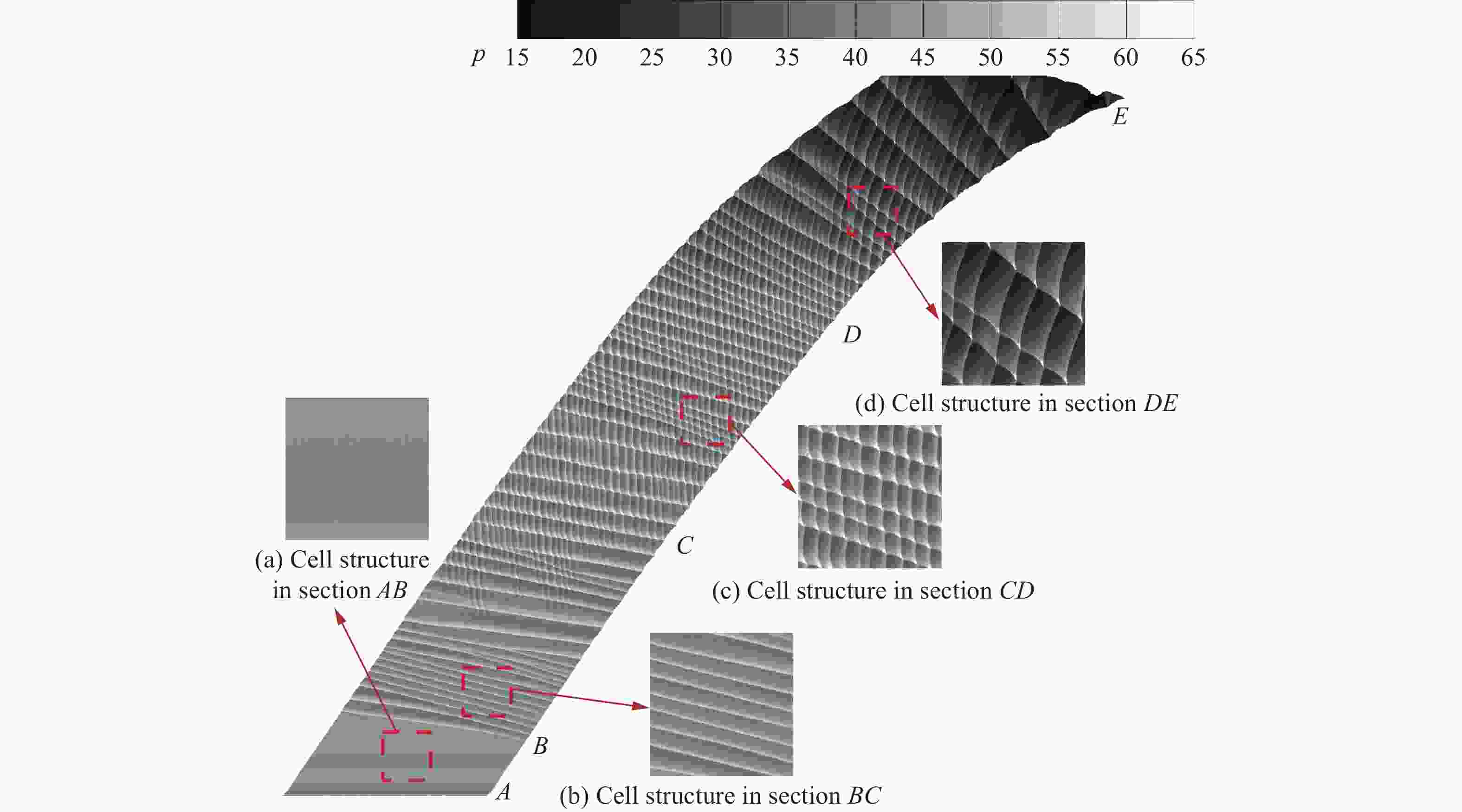
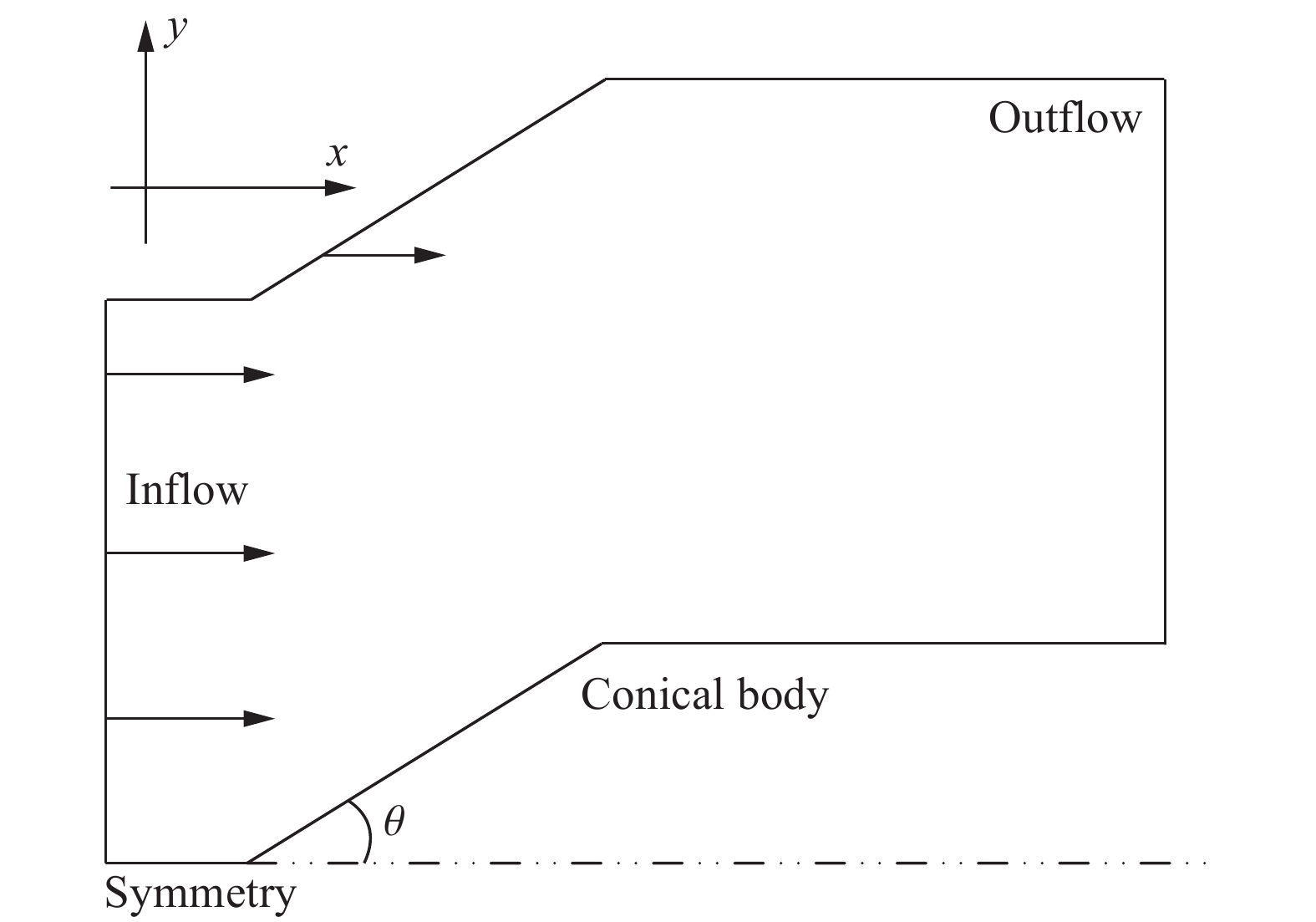
[1]	范宝春, 张旭东, 潘振华, 等. 用于推进的三种爆轰波的结构特征 [J]. 力学进展, 2012, 42(2): 162–169. DOI: 10.6052/1000-0992-2012-2-20120204. FAN B C, ZHANG X D, PAN Z H, et al. Fundamental characteristics of three types of detonation waves utilized in propulsion [J]. Advances in Mechanics, 2012, 42(2): 162–169. DOI: 10.6052/1000-0992-2012-2-20120204.
[2]	LI C, KAILASANATH K, ORAN E S. Detonation structures behind oblique shocks [J]. Physics of Fluids, 1994, 6(4): 1600–1611. DOI: 10.1063/1.868273.
[3]	TENG H H, ZHAO W, JIANG Z L. A novel oblique detonation structure and its stability [J]. Chinese Physics Letters, 2007, 24(7): 1985–1988. DOI: 10.1088/0256-307X/24/7/055.
[4]	归明月, 范宝春. 尖劈诱导的斜爆轰波的精细结构及其影响因素 [J]. 推进技术, 2012, 33(3): 490–494. DOI: 10.13675/j.cnki.tjjs.2012.03.002. GUI M Y, FAN B C. Fine structure and its influence factor of wedge-induced oblique detonation waves [J]. Journal of Propulsion Technology, 2012, 33(3): 490–494. DOI: 10.13675/j.cnki.tjjs.2012.03.002.
[5]	TENG H H, JIANG Z L, NG H D. Numerical study on unstable surfaces of oblique detonations [J]. Journal of Fluid Mechanics, 2014, 744(2): 111–128. DOI: 10.1017/jfm.2014.78.
[6]	LIU Y, LIU Y S, WU D, et al. Structure of an oblique detonation wave induced by a wedge [J]. Shock Waves, 2016, 26(2): 161–168. DOI: 10.1007/s00193-015-0600-5.
[7]	LIU Y, HAN X, YAO S, et al. A numerical investigation of the prompt oblique detonation wave sustained by a finite-length wedge [J]. Shock Waves, 2016, 26(6): 729–739. DOI: 10.1007/s00193-016-0626-3.
[8]	YANG L, YUE L, ZHANG Q, et al. Numerical study on the shock/combustion interaction of oblique detonation waves [J]. Aerospace Science and Technology, 2020, 104: 105938. DOI: 10.1016/j.ast.2020.105938.
[9]	王爱峰, 赵伟, 姜宗林. 斜爆轰的胞格结构及横波传播 [J]. 爆炸与冲击, 2010, 30(4): 349–354. DOI: 10.11883/1001-1455(2010)04-0349-06. WANG A F, ZHAO W, JIANG Z L. Cellular structure of oblique detonation and propagation of transverse wave [J]. Explosion and Shock Waves, 2010, 30(4): 349–354. DOI: 10.11883/1001-1455(2010)04-0349-06.
[10]	YANG P, LI H, CHEN Z, et al. Numerical investigation on movement of triple points on oblique detonation surfaces [J]. Physics of Fluids, 2022, 34(6): 066113. DOI: 10.1063/5.0091078.
[11]	YAO K, WANG C, JIANG Z. A numerical study of oblique detonation re-stabilization by expansion waves [J]. Aerospace Science and Technology, 2022, 122: 107409. DOI: 10.1016/j.ast.2022.107409.
[12]	刘岩, 武丹, 王健平. 低马赫数下斜爆轰波的结构 [J]. 爆炸与冲击, 2015, 35(2): 203–207. DOI: 10.11883/1001-1455(2015)02-0203-05. LIU Y, WU D, WANG J P. Structure of oblique detonation wave at low inflow mach number [J]. Explosion and Shock Waves, 2015, 35(2): 203–207. DOI: 10.11883/1001-1455(2015)02-0203-05.
[13]	ZHANG Z, WEN C, ZHANG W, et al. Formation of stabilized oblique detonation waves in a combustor [J]. Combustion and Flame, 2021, 223: 423–436. DOI: 10.1016/j.combustflame.2020.09.034.
[14]	ZHANG G Q, GAO S F, XIANG G X. Study on initiation mode of oblique detonation induced by a finite wedge [J]. Physics of Fluids, 2021, 33(1): 016102. DOI: 10.1063/5.0035960.
[15]	VERREAULT J, HIGGINS A J. Initiation of detonation by conical projectiles [J]. Proceedings of the Combustion Institute, 2011, 33(2): 2311–2318. DOI: 10.1016/j.proci.2010.07.086.
[16]	KASAHARA J, FUJIWARA T, ENDO T, et al. Chapman-Jouguet oblique detonation structure around hypersonic projectiles [J]. AIAA journal, 2001, 39(8): 1553–1561. DOI: 10.2514/2.1480.
[17]	董刚, 范宝春, 李鸿志. 圆锥激波诱导的爆燃和爆轰不稳定性研究 [J]. 兵工学报, 2010, 31(4): 401–408. DONG G, FAN B C, LI H Z. An investigation on instability of deflagration and detonation induced by conical shock wave [J]. Acta Armamentarii, 2010, 31(4): 401–408.
[18]	YANG P, NG H D, TENG H, et al. Initiation structure of oblique detonation waves behind conical shocks [J]. Physics of Fluids, 2017, 29(8): 086104. DOI: 10.1063/1.4999482.
[19]	HAN W, WANG C, LAW C K. Three-dimensional simulation of oblique detonation waves attached to cone [J]. Physical Review Fluids, 2019, 4(5): 053201. DOI: 10.1103/PhysRevFluids.4.053201.
[20]	ABISLEIMAN S, SHARMA V, BIELAWSKI R, et al. Structure of three-dimensional conical oblique detonation waves [J]. Combustion and Flame, 2025, 274: 113971. DOI: 10.1016/j.combustflame.2025.113971.
[21]	STURTZER M O, TOGAMI K, YAMASHITA S, et al. Detonation wave generated by a hypervelocity projectile [J]. Heat Transfer Research, 2007, 38(4): 291–297. DOI: 10.1615/HeatTransRes.v38.i4.10.
[22]	李俊红, 沈清, 程晓丽. 曲面激波诱导斜爆轰的数值模拟 [J]. 推进技术, 2019, 40(11): 2521–2527. DOI: 10.13675/j.cnki.tjjs.190041. LI J H, SHEN Q, CHENG X L. Numerical simulation on shock-induced detonation [J]. Journal of Propulsion Technology, 2019, 40(11): 2521–2527. DOI: 10.13675/j.cnki.tjjs.190041.
[23]	MAEDA S, KASAHARA J, MATSUO A. Oblique detonation wave stability around a spherical projectile by a high time resolution optical observation [J]. Combustion and flame, 2012, 159(2): 887–896. DOI: 10.1016/j.combustflame.2011.09.001.
[24]	周平, 范宝春, 归明月. 可燃介质中飞行圆球诱导斜爆轰的流场结构 [J]. 爆炸与冲击, 2012, 32(3): 278–282. DOI: 10.11883/1001-1455(2012)03-0278-05. ZHOU P, FAN B C, GUI M Y. Flow pattern of oblique detonation induced by a hyperve locity ball in combustible gas [J]. Explosion and Shock Waves, 2012, 32(3): 278–282. DOI: 10.11883/1001-1455(2012)03-0278-05.
[25]	ANDERSON J D. Modern compressible flow: with historical perspective [M]. New York: McGraw-Hill, 1990: 167-371.
[26]	BOURLIOUX A, MAJDA A J. Theoretical and numerical structure for unstable two-dimensional detonations [J]. Combustion and Flame, 1992, 90(3): 211–229. DOI: 10.1016/0010-2180(92)90084-3.
[27]	KURGANOV A, NOELLE S, PETROVA G. Semidiscrete central-upwind schemes for hyperbolic conservation laws and Hamilton-Jacobi equations [J]. SIAM Journal on Scientific Computing, 2001, 23(3): 707–740. DOI: 10.1137/S1064827500373413.
[28]	BADER G, DEUFLHARD P. A semi-implicit mid-point rule for stiff systems of ordinary differential equations [J]. Numerische Mathematik, 1983, 41(3): 373–398. DOI: 10.1007/BF01418331.
[29]	GUI M Y, FAN B C, DONG G. Periodic oscillation and fine structure of wedge-induced oblique detonation waves [J]. Acta Mechanica Sinica, 2011, 27(6): 922–928. DOI: 10.1007/s10409-011-0508-y.